NCERT Exercise Q & A

Exercise Problems

Q9.1: A small candle, 2.5 cm in size is placed at 27 cm in front of a concave mirror of radius of curvature 36 cm. At what distance from the mirror should a screen be placed in order to obtain a sharp image? Describe the nature and size of the image. If the candle is moved closer to the mirror, how would the screen have to be moved?

Answer: The object is kept between \(f\) and \(C\). So the image should be real, inverted and beyond \(C\). To locate the sharp image, screen should be placed at the position of image.

The focal length \(f=\frac{-R}{2}=-18 \mathrm{~cm}\)
Object distance \(u=-27 \mathrm{~cm}\)
Using mirror formula, \(\frac{1}{v}+\frac{1}{u}=\frac{1}{f}\)
or \(\frac{1}{v}+\frac{1}{-27}=\frac{1}{-18} \quad\) or \(\quad \frac{1}{v}=-\frac{1}{18}+\frac{1}{27}\)
\(
\begin{aligned}
& \frac{1}{v}=\frac{-3+2}{54} \Rightarrow \frac{1}{v}=-\frac{1}{54} \\
& v=-54 \mathrm{~cm}
\end{aligned}
\)
now, let the height of image be \(h^{\prime}\)
magnification of the image is given by
\(
m=\frac{h^{\prime}}{h}=-\frac{v}{u}
\)
from here
\(
h^{\prime}=-\frac{-54}{-27} \times 2.5=-5 \mathrm{~cm}
\)
Hence the size of the image will be -5 cm . negative sign implies that the image is inverted and real. So, the image is inverted and magnified. Thus in order to locate the sharp image, the screen should be kept 54 cm in front of concave mirror and image on the screen will be observed real, inverted and magnified. If the candle is moved closer to the mirror, we have to move the screen away from the mirror in order to obtain the image on the screen. if the image distance is less than the focal length image cannot be obtained on the screen and image will be virtual.

Q9.2: A 4.5 cm needle is placed 12 cm away from a convex mirror of focal length 15 cm . Give the location of the image and the magnification. Describe what happens as the needle is moved farther from the mirror.

Answer: A convex mirror always form virtual image, which is erect and small in size of an object kept in front of it. Focal length of convex mirror \(f=+15 \mathrm{~cm}\) Object distance \(u=-12 \mathrm{~cm}\) Using mirror formula

\(
\begin{aligned}
& \frac{1}{v}+\frac{1}{u}=\frac{1}{f} \\
& \frac{1}{v}+\frac{1}{-12}=\frac{1}{15} \quad \text { or } \quad \frac{1}{v}=\frac{1}{15}+\frac{1}{12} \\
& \text { or } \frac{1}{v}=\frac{4+5}{60} \\
& v=+\frac{60}{9} \mathrm{~cm}=+6.66 \mathrm{~cm}
\end{aligned}
\)
Therefore, image is virtual, formed at 6.67 cm at the back of the mirror.
\(
\begin{aligned}
& \text { Magnification } m=-\frac{v}{u} \\
& m=-\frac{\frac{60}{9}}{-12} \Rightarrow m=\frac{5}{9}=+0.55
\end{aligned}
\)
Size of image \(h_2=m \times h_1\)
\(
\begin{aligned}
& =\frac{5}{9} \times h_1=\frac{5}{9} \times 4.5 \\
h_2 & =2.5 \mathrm{~cm}
\end{aligned}
\)
It shows the image is erect, small in size and virtual. When the needle is moved farther from mirror the image also move towards focus and decreasing in size. As \(u\) approaches \(v\) approaches focus but never beyond \(f\).

Q9.3: A tank is filled with water to a height of 12.5 cm . The apparent depth of a needle lying at the bottom of the tank is measured by a microscope to be 9.4 cm . What is the refractive index of water? If water is replaced by a liquid of refractive index 1.63 up to the same height, by what distance would the microscope have to be moved to focus on the needle again?

Answer: We know the relation
\(
\text { Apparent depth }=\frac{\text { Real depth }}{{ }^a \mu_w}
\)
\(
9.4 \mathrm{~cm}=\frac{12.5 \mathrm{~cm}}{{ }^a \mu_w} \quad \text { or } \quad{ }^a \mu_w=\frac{12.5}{9.4}=1.33
\)
Now if the water is replaced by other liquid, the apparent depth will change and microscope will have to be further moved to focus the image. With new liquid
\(
\begin{aligned}
& \text { Apparent depth }=\frac{\text { Real depth }}{{ }^a \mu_l} \\
& \text { Apparent depth }=\frac{12.5}{1.63}=7.67 \mathrm{~cm}
\end{aligned}
\)
Now the microscope will have to shift from its initial position to focus on the needle again which is at 7.67 cm depth. Shift distance \(=9.4-7.67=1.73 \mathrm{~cm}\).

Q9.4: Figures 9.27 (a) and (b) show refraction of a ray in air incident at \(60^{\circ}\) with the normal to a glass-air and water-air interface, respectively. Predict the angle of refraction in glass when the angle of incidence in water is \(45^{\circ}\) with the normal to a water-glass interface [Fig. 9.27(c)].

Answer: As we know,by snell’s law \(\mu_1 \sin \theta_1=\mu_2 \sin \theta_2\) where, \(\mu_1=\) refrective index of medium 1
\(\theta_1=\) incident angle in medium 1
\(\mu_2=\) refrective index of medium 2
\(\theta_2=\) refraction angle in medium 2
Now, applying it for fig (a)
\(
\begin{aligned}
& 1 \sin 60=\mu_{\text {glass }} \sin 35 \\
& \mu_{\text {glass }}=\frac{\sin 60}{\sin 35}=\frac{0.866025}{0.573576}=1.509
\end{aligned}
\)
Now applying for fig (b)
\(
\begin{aligned}
& 1 \sin 60=\mu_{\text {water }} \sin 47 \\
& \mu_{\text {water }}=\frac{\sin 60}{\sin 47}=\frac{.8660}{.7313}=1.184
\end{aligned}
\)
Now in fig (c) Let refraction angle be \(\theta\) so,
\(
\begin{aligned}
& \mu_{\text {water }} \sin 45=\mu_{\text {giass }} \sin \theta \\
& \sin \theta=\frac{\mu_{\text {water }} * \sin 45}{\mu_{\text {glass }}} \\
& \sin \theta=\frac{1.184 * 0.707}{1.509}=0.5546 \\
& \theta=\sin ^{-1}(0.5546)=38.68
\end{aligned}
\)
Therefore the angle of refraction when ray goes from water to glass in fig(c) is 38.68 .

Q9.5: A small bulb is placed at the bottom of a tank containing water to a depth of 80 cm . What is the area of the surface of water through which light from the bulb can emerge out? Refractive index of water is 1.33 . (Consider the bulb to be a point source.)

Answer: As shown in the figure all those light rays which are incident on the surface at angle of incidence more than critical angle, does total internal reflection and are reflected back in water only. All those light rays which are incident below critical angle emerges out of surface bending away from normal. All those light beams which are incident at critical angle grazes the surface of water.

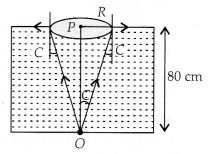
We know
\(
\begin{aligned}
& \sin C=\frac{1}{{ }^a \mu_w} \\
& C=\sin ^{-1}\left(\frac{1}{{ }^a \mu_w}\right)
\end{aligned}
\)
\(
C=\sin ^{-1}\left(\frac{1}{1.33}\right) \Rightarrow \sin C=\frac{1}{1.33}=\frac{3}{4}
\)
\(
\begin{aligned}
& \tan C=\frac{R}{O P} \text { (radius) } \quad\left[\because(0.80)^2=0.6400\right] \\
& R=\tan C \times O P=\tan C(0.80) \\
& \text { Area }=\pi R^2=\pi \times \tan ^2 C(0.64) \\
& A=\pi(0.64)+\tan ^2 C
\end{aligned}
\)
\(
\begin{aligned}
& =\pi(0.64) \times \frac{\operatorname{Sin}^2 C}{\operatorname{Cos}^2 C}=\pi(0.64) \times \frac{\operatorname{Sin}^2 C}{1-\operatorname{Sin}^2 C} \\
& =\pi(0.64) \times \frac{9}{16}=\pi(0.64) \times \frac{9}{16} \times \frac{16}{7} \\
& =\frac{22}{7} \times 0.64 \times \frac{9}{7}=2.6 \mathrm{~m}^2
\end{aligned}
\)

Q9.6: A prism is made of glass of unknown refractive index. A parallel beam of light is incident on a face of the prism. The angle of minimum deviation is measured to be \(40^{\circ}\). What is the refractive index of the material of the prism? The refracting angle of the prism is \(60^{\circ}\). If the prism is placed in water (refractive index 1.33), predict the new angle of minimum deviation of a parallel beam of light.

Answer: Angle of minimum deviation, \(\delta_{\mathrm{m}}=40^{\circ}\)
Angle of the prism, \(\mathrm{A}=60^{\circ}\)
Refractive index of water, \(\mu=1.33\)
Refractive index of the material of the prism \(=\mu^{\prime}\)
The angle of deviation is related to refractive index \(\left(\mu^{\prime}\right)\) as:
\(
\begin{aligned}
& \mu^{\prime}=\frac{\sin \frac{\left(\mathrm{A}+\delta_{\mathrm{m}}\right)}{2}}{\sin \frac{\mathrm{~A}}{2}} \\
& =\frac{\sin \frac{\left(60^{\circ}+40^{\circ}\right)}{2}}{\sin \frac{60^{\circ}}{2}} \\
& =\frac{\sin 50^{\circ}}{\sin 30^{\circ}} \\
& =1.532
\end{aligned}
\)
Hence, the refractive index of the material of the prism is 1.532 .
Since the prism is placed in water, let \(\delta_{\mathrm{m}}^{\prime}\) be the new angle of minimum deviation for the same prism.
The refractive index of glass with respect to water is given by the relation:
\(
\mu_{\mathrm{g}}^{\mathrm{w}}=\frac{\mu^{\prime}}{\mu}=\frac{\sin \frac{\left(\mathrm{A}+\delta_{\mathrm{m}}^{\prime}\right)}{2}}{\sin \frac{\mathrm{~A}}{2}}
\)
\(
\begin{aligned}
&\begin{aligned}
& \sin \frac{\left(A+\delta_m^{\prime}\right)}{2}=\frac{\mu^{\prime}}{\mu} \sin \frac{A}{2} \\
& \sin \frac{\left(A+\delta_m^{\prime}\right)}{2}=\frac{1.532}{1.33} \times \sin \frac{60^{\circ}}{2}=0.5759 \\
& \frac{\left(A+\delta_m^{\prime}\right)}{2}=\sin ^{-1} 0.5759=35.16^{\circ} \\
& 60^{\circ}+\delta_m^{\prime}=70.32^{\circ} \\
& \therefore \delta_m^{\prime}=70.32^{\circ}-60^{\circ}=10.32^{\circ}
\end{aligned}\\
&\text { Hence, the new minimum angle of deviation is } 10.32^{\circ} \text {. }
\end{aligned}
\)

Q9.7: Double-convex lenses are to be manufactured from a glass of refractive index 1.55 , with both faces of the same radius of curvature. What is the radius of curvature required if the focal length is to be 20 cm ?

Answer: Both faces should be of same radius of curvature
\(
\left|R_1\right|=\left|R_2\right|=R
\)
So, lens makers formula
\(
\begin{aligned}
& \frac{1}{f}=\left({ }^a \mu_g-1\right)\left[\frac{1}{R_1}-\frac{1}{R_2}\right] \\
& \Rightarrow \frac{1}{f}=(1.55-1)\left[\frac{1}{R}-\frac{1}{-R}\right] \\
& \frac{1}{f}=0.55\left[\frac{2}{R}\right] \\
& \Rightarrow \frac{1}{20}=\frac{1.10}{R} \Rightarrow R=20 \times 1.1=22 \mathrm{~cm}
\end{aligned}
\)
So, the radius of curvature should be 22 cm for each face of lens.

Q9.8: A beam of light converges at a point \(P\). Now a lens is placed in the path of the convergent beam 12 cm from \(P\). At what point does the beam converge if the lens is (a) a convex lens of focal length 20 cm , and (b) a concave lens of focal length 16 cm ?

Answer: (a) The convex lens is placed in the path of convergent beam.

\(
\begin{aligned}
&\text { Using lens formula, } \frac{1}{v}-\frac{1}{u}=\frac{1}{f}\\
&\begin{aligned}
& \frac{1}{v}-\frac{1}{+12}=\frac{1}{+20} \quad \text { or } \quad \frac{1}{v}=\frac{1}{20}+\frac{1}{12}=\frac{3+5}{60} \\
& v=\frac{60}{8}=+7.5 \mathrm{~cm}
\end{aligned}
\end{aligned}
\)
The image I is formed by further converging beams at a distance of 7.5 cm from lens.

(b) A concave lens is placed in the path of convergent’ beam, the concave lens further diverge the light.

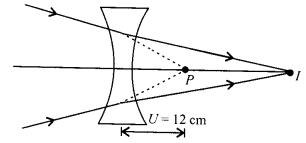
Using lens formula, \(\frac{1}{v}-\frac{1}{u}=\frac{1}{f}\)
\(
\begin{aligned}
& \frac{1}{v}-\frac{1}{+12}=\frac{1}{-16} \\
& \text { or } \frac{1}{v}=-\frac{1}{16}+\frac{1}{12}=\frac{-3+4}{48}=\frac{1}{48} \\
& v=+48 \mathrm{~cm}
\end{aligned}
\)
The image \(I\) is formed by diverged rays at 48 cm away from concave lens.

Q9.9: An object of size 3.0 cm is placed 14 cm in front of a concave lens of focal length 21 cm . Describe the image produced by the lens. What happens if the object is moved further away from the lens?

Answer: Object of size 3 cm is placed 14 cm in front of concave lens.

Lens formula, \(\frac{1}{v}-\frac{1}{u}=\frac{1}{f}\)
\(
\begin{aligned}
& \frac{1}{v}-\frac{1}{-14}=\frac{1}{-21} \\
& \frac{1}{v}=-\frac{1}{21}-\frac{1}{14}=\frac{-2-3}{42} \\
& v=-\frac{42}{5}=-8.4 \mathrm{~cm}
\end{aligned}
\)
Formula for magnification
\(
\begin{aligned}
& m=\frac{I}{O}=+\frac{v}{u} \quad \text { or } \quad \frac{I}{O}=\frac{v}{u} \quad \text { or } \quad \frac{I}{+3}=\frac{-8.4}{-14} \\
& \Rightarrow I=+1.8 \mathrm{~cm}
\end{aligned}
\)
So, the image is virtual, erect, of the size 1.8 cm and is located 8.4 cm from the lens on the same side as object. As the object is moved away from the lens, the virtual image moves towards the focus of the lens but never beyond it. The image also reduces in size as shift towards focus.

Q9.10: What is the focal length of a convex lens of focal length 30 cm in contact with a concave lens of focal length 20 cm ? Is the system a converging or a diverging lens? Ignore thickness of the lenses.

Answer: Equivalent focal length of the combination

\(
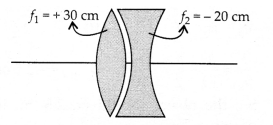
\begin{aligned}
&\begin{aligned}
& \frac{1}{f_{\mathrm{eq}}}=\frac{1}{f_1}+\frac{1}{f_2} \quad \text { or } \quad \frac{1}{f_{\mathrm{eq}}}=\frac{1}{30}+\frac{1}{-20}=\frac{2-3}{60} \\
& \text { or } f_{\mathrm{eq}}=-60 \mathrm{~cm}
\end{aligned}\\
&\text { Hence, system will behave as a diverging lens of focal length } 60 \mathrm{~cm} \text {. }
\end{aligned}
\)

Q9.11: A compound microscope consists of an objective lens of focal length 2.0 cm and an eyepiece of focal length 6.25 cm separated by a distance of 15 cm . How far from the objective should an object be placed in order to obtain the final image at (a) the least distance of distinct vision ( 25 cm ), and (b) at infinity? What is the magnifying power of the microscope in each case?

Answer: (a) We want the final image at least distance of distinct vision. Let the object in front of objective is at distance \(v_0\).

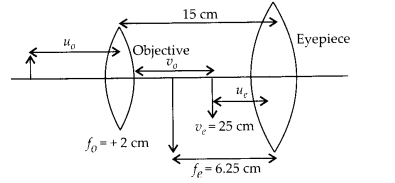
Let us first find the \(u_c\), the object distance for еуеріесе.
Here \(v_e=-25, f_e=6.25, u_e=\) ?
\(
\begin{aligned}
& \frac{1}{v_e}-\frac{1}{u_e}=\frac{1}{f_e} \\
& \frac{1}{-25}-\frac{1}{u_e}=\frac{1}{6.25}
\end{aligned}
\)
\(
\begin{aligned}
\text { or } & -\frac{1}{u_e}=\frac{1}{6.25}+\frac{1}{25}=\frac{4+1}{25} \\
u_e & =-5 \mathrm{~cm}
\end{aligned}
\)
So, image distance of objective lens
\(
\begin{aligned}
& v_o=15-u_e \\
& v_o=15-5=10 \mathrm{~cm}
\end{aligned}
\)
Now we can get required position of object in point of objective.
Now we can get required position of object in point of objective.
\(
\begin{aligned}
& \frac{1}{v_o}-\frac{1}{u_o}=\frac{1}{f_o} \quad \text { or } \quad \frac{1}{+10}-\frac{1}{u_o}=\frac{1}{2} \\
& \frac{1}{u_o}=\frac{1}{10}-\frac{1}{2}=\frac{1-5}{10} \Rightarrow u_o=-2.5 \mathrm{~cm}
\end{aligned}
\)
So, the object should be 2.5 cm in front of objective lens.
Magnifying power (most strained eye)
\(
\begin{aligned}
& m=-\frac{v_o}{u_o}\left[1+\frac{D}{f_e}\right] \quad \text { or } \quad m=-\frac{10}{-2.5}\left[1+\frac{25}{6.25}\right] \\
& =4[5]=20
\end{aligned}
\)
(b) We want the final image at infinity. Let us again assume the object in front of objective at distance \(v_0\).
The object distance \(v_e\) for the eyepiece should be equal to \(f_e=6.25 \mathrm{~cm}\) to obtain final image at \(\infty\). So, image distance of objective lens
\(
v_s=15-f_s=15-6.25=8.75 \mathrm{~cm}
\)
\(
v_o=15-f_e=15-6.25=8.75 \mathrm{~cm}
\)
Now, lens formula, \(\frac{1}{v_o}-\frac{1}{u_o}=\frac{1}{f_o}\)
\(
\begin{aligned}
& \frac{1}{8.75}-\frac{1}{u_o}=\frac{1}{2} \quad \text { or } \quad \frac{1}{u_o}=\frac{1}{8.75}-\frac{1}{2}=\frac{2-8.75}{17.5} \\
& u_o=-\frac{17.5}{6.75} \mathrm{~cm}=-2.59 \mathrm{~cm}
\end{aligned}
\)
Magnifying power (most relaxed eye)
\(
m=-\frac{v_o}{u_o}\left[\frac{D}{f_e}\right] \Rightarrow m=-\frac{8.75}{-2.59}\left[\frac{25}{6.25}\right]=13.5
\)

Q9.12: A person with a normal near point \((25 \mathrm{~cm})\) using a compound microscope with objective of focal length 8.0 mm and an eyepiece of focal length 2.5 cm can bring an object placed at 9.0 mm from the objective in sharp focus. What is the separation between the two lenses? Calculate the magnifying power of the microscope,

Answer: The image is formed at least distance of distinct vision for sharp focus. The separation between two lenses will be \(v_0+\left|v_e\right|\)

\(
\begin{aligned}
&\text { Let us find first } {v}_0 \text { the image distance for objective lens. }\\
&\frac{1}{v_0}-\frac{1}{u_0}=\frac{1}{f_o} \quad \text { or } \quad \frac{1}{v_0}-\frac{1}{-9}=\frac{1}{8}
\end{aligned}
\)
\(
\begin{aligned}
&\text { Also we can find object distance for eyepiece } v_e \text { as we know }\\
&v_e=D=25 \mathrm{~cm}=250 \mathrm{~mm}
\end{aligned}
\)
\(
\begin{aligned}
&\begin{aligned}
& \frac{1}{v_e}-\frac{1}{u_e}=\frac{1}{f_e} \\
& \frac{1}{-250}-\frac{1}{u_e}=\frac{1}{25}-\frac{1}{u_e}=\frac{1}{25}+\frac{1}{250}=\frac{10+1}{250} \\
& u_e=-\frac{250}{11} \mathrm{~mm}=-22.7 \mathrm{~mm}=-2.27 \mathrm{~cm}
\end{aligned}\\
&\text { Separation between lenses }\\
&\begin{aligned}
& L=v_o+\left|u_c\right|=72+22.7 \text { or } L=94.7 \mathrm{~mm} \\
&=9.47 \mathrm{~cm} \\
& \text { Magnifying power } m=-\frac{v_o}{u_o}\left[1+\frac{D}{f_e}\right] \\
& m=-\frac{72}{-9}\left[1+\frac{250}{25}\right]=8[11]=88
\end{aligned}
\end{aligned}
\)

Q9.13: A small telescope has an objective lens of focal length 144 cm and an eyepiece of focal length 6.0 cm . What is the magnifying power of the telescope? What is the separation between the objective and the eyepiece?

Answer: Given, \(f_0=144 \mathrm{~cm}\),
\(
\begin{aligned}
& f_c=6 \mathrm{~cm} \\
& m=?, L=?
\end{aligned}
\)
Magnifying power \(=\frac{f_0}{f_e}=\frac{144}{6}=24\)
Separation between objective and eyepiece
\(
(\mathrm{L})=f_0+f_c=144+6=150 \mathrm{~cm}
\)

Q9.14: (a) A giant refracting telescope at an observatory has an objective lens of focal length 15 m . If an eyepiece of focal length 1.0 cm is used, what is the angular magnification of the telescope?
(b) If this telescope is used to view the moon, what is the diameter of the image of the moon formed by the objective lens? The diameter of the moon is \(3.48 \times 10^6 \mathrm{~m}\), and the radius of lunar orbit is \(3.8 \times 10^8 \mathrm{~m}\).

Answer: (a) \({f}_0=15 \mathrm{~m}\) and \({f}_{\mathrm{e}}=1.0 \mathrm{~cm}\) angular magnification by the telescope normal adjustment

\(
m=-\frac{f_o}{f_e}=\frac{1500 \mathrm{~cm}}{1.0 \mathrm{~cm}}=-1500
\)
(b) The image of the moon by the objective at lens is formed on its focus only as the moon is nearly infinite distance as compared to focal length.
Height of object
i.e., Radius of moon \(R_m=\frac{3.48}{2} \times 10^6 \mathrm{~m}\)
\(
=1.74 \times 10^6 \mathrm{~m}
\)
Distance of object
i.e., Radius of lunar orbit, \(R_o=3.8 \times 10^8 \mathrm{~cm}\)
Distance of object i.e., Radius of lunar orbit, \(\mathrm{R}_0=3.8 \times 10^8 \mathrm{~cm}\) Distance of image for objective lens i.e., focal length of objective lens \(f_0=15 \mathrm{~m}\) Radius of image of moon by objective lens can be calculated.
\(
\begin{aligned}
& \tan \theta=-\frac{R_m}{R_o}=\frac{h}{f_o} \\
& h=\frac{R_m \times f_o}{R_o}=\frac{1.74 \times 10^6 \times 15}{3.8 \times 10^8}=6.87 \times 10^{-2} \mathrm{~m}
\end{aligned}
\)
Diameter of the image of moon
\(
D_l=2 h=13.74 \times 10^{-2} \mathrm{~m}=13.74 \mathrm{~cm}
\)

Q9.15: Use the mirror equation to deduce that:
(a) an object placed between \(f\) and \(2 f\) of a concave mirror produces a real image beyond \(2 f\).
(b) a convex mirror always produces a virtual image independent of the location of the object.
(c) the virtual image produced by a convex mirror is always diminished in size and is located between the focus and the pole.
(d) an object placed between the pole and focus of a concave mirror produces a virtual and enlarged image.
[Note: This exercise helps you deduce algebraically properties of images that one obtains from explicit ray diagrams.]

Answer: (a) We know for a concave mirror \({f}<0\) [negative] and \({u}<0\) [negative]
\(2 f<u<f\)
\(
\therefore \quad \frac{1}{2 f}>\frac{1}{u}>\frac{1}{f} \quad \text { or } \quad \frac{-1}{2 f}<\frac{-1}{u}<\frac{-1}{f}
\)
or \(\frac{1}{f}-\frac{1}{2 f}<\frac{1}{f}-\frac{1}{u}<\frac{1}{f}-\frac{1}{f}\)
or \(\frac{1}{2 f}<\frac{1}{v}<0 \quad\left\{\because \frac{1}{f}-\frac{1}{u}=\frac{1}{v}\right\}\)
Which implies that \(v<0\) to form image on the left.
Also \(2 f>v \quad\{\because 2 f\) and \(v\) are -ve \(\}\)
\(|2 f|<|v|\)
So, the real image is formed beyond \(2 f\).
(b) For a convex mirror, \({f}>0\), always positive and object distance \({u}<0\), always negative.

Now mirror formula, \(\frac{1}{f}=\frac{1}{v}+\frac{1}{u}\)
or \(\frac{1}{v}=\frac{1}{f}-\frac{1}{u}\)
This implies that \(\frac{1}{v}>0\), or \(v>0\).
So, whatever be the value of \(u\), a convex mirror always forms a virtual image.
(c) In convex mirror focal length is positive hence \(f>0\) and for an object distance from mirror with negative \(\operatorname{sign}(u<0)\)
So, \(\frac{1}{v}+\frac{1}{u}=\frac{1}{f}\) or \(\frac{1}{v}=\frac{1}{f}-\frac{1}{u}\)
The results \(\frac{1}{v}>\frac{1}{f}\) or \(v<f\) (both positive)
hence the image is located between pole and focus of the mirror
Also magnification \(m=-\frac{v}{u}=-\frac{+v}{-u}\)
\(m<[1]\) (positive)
So, the image is virtual and diminished.

(d) In concave mirror, \({f}<0\) for object placed between focus and pole of concave mirror \(f<u<0\) (both negative)
\(
\frac{1}{f}>\frac{1}{u}
\)
Now mirror formula, \(\frac{1}{v}=\frac{1}{f}-\frac{1}{u}\) \(\frac{1}{v}>0\) or \(v>0\) (positive)
hence the image is virtual.
Also magnification \(m=\frac{-v}{u}\)
here \(\frac{1}{v}<\frac{1}{|u|}\) or \(v>|u|\)
So, \(m>1\) hence the image is enlarged.

Q9.16: A small pin fixed on a table top is viewed from above from a distance of 50 cm . By what distance would the pin appear to be raised if it is viewed from the same point through a 15 cm thick glass slab held parallel to the table? Refractive index of glass \(=1.5\). Does the answer depend on the location of the slab?

Answer: The shift in the image by the thick glass slab can be calculated. Here, shift only depend upon thickness of glass slab and refractive index of glass.
Shift = Real thickness – Apparent of thickness
\(
\begin{aligned}
\text { Shift } & =t_g\left[1-\frac{1}{{ }^a \mu_g}\right]=15\left[1-\frac{1}{1.5}\right] \\
& =15 \times \frac{0.5}{1.5}=5 \mathrm{~cm}
\end{aligned}
\)
The answer does not depend on the location of the slab.

Q9.17: (a) Figure 9.28 shows a cross-section of a ‘light pipe’ made of a glass fibre of refractive index 1.68. The outer covering of the pipe is made of a material of refractive index 1.44. What is the range of the angles of the incident rays with the axis of the pipe for which total reflections inside the pipe take place, as shown in the figure.

(b) What is the answer if there is no outer covering of the pipe?

Answer: 

(a) Let us first derive the condition for total internal reflection. Critical angle for the interface of medium 1 and medium 2.
\(
\sin C=\frac{1}{{ }^1 \mu_2}=\frac{\mu_2}{\mu_1}=\frac{1.44}{1.68}=0.8571
\)
So, critical angle \(C=59^{\circ}\)
Condition for total internal reflection from core to cladding
\(
i_2>59^{\circ} \text { or } r \leq \frac{\pi}{2}-59^{\circ} \text { or } r \leq 31^{\circ}
\)
Now, for refraction at first surface air to core.
Snell’s law, \(\frac{\sin i_1}{\sin r}={ }^a \mu_1\)
\(
\sin i_1={ }^a \mu_1 \sin r=1.68 \sin 31^{\circ} \text { or } i_1 \approx 60^{\circ}
\)
Thus all incident rays which makes angle of incidence between \(0^{\circ}\) and \(60^{\circ}\) will suffer total internal reflection in the optical fiber.

(b) When there is no outer covering critical angle from core to surface.
\(
\begin{aligned}
& \sin C=\frac{1}{{ }^1 \mu_a}=\frac{\mu_a}{\mu_1} \\
& \sin C=\frac{1}{1.68} \Rightarrow C=\sin ^{-1}\left(\frac{1}{1.68}\right)=36.5^{\circ}
\end{aligned}
\)
So, condition for total internal reflection from core to surface
\(
i_2>36.5^{\circ} \text { or } r<\frac{\pi}{2}-36.5^{\circ} \text { or } r<53.5^{\circ}
\)
Let us find range of incident angle at first surface air to core.
\(
\begin{aligned}
& { }^a \mu_1=\frac{\sin i_1}{\sin r} \\
& \sin i_1=1.68 \times \sin 53.5^{\circ}=1.68 \times 0.8039 \\
& \sin i_1=1.35 \text { or } i \approx 90^{\circ}
\end{aligned}
\)
Thus all incident rays at first surface \(0^{\circ}\) to \(90^{\circ}\) will suffer total internal reflection inside core.

Q9.18: The image of a small electric bulb fixed on the wall of a room is to be obtained on the opposite wall 3 m away by means of a large convex lens. What is the maximum possible focal length of the lens required for the purpose?

Answer: Let the object be placed \(x \mathrm{m}\) in front of lens the distance of image from the lens is \((3-{x}) \mathrm{m}\).
Applying lens formula, \(\frac{1}{v}-\frac{1}{u}=\frac{1}{f}[latex]
[latex]
\frac{1}{(3-x)}-\frac{1}{-x}=\frac{1}{f} \quad \text { or } \quad \frac{1}{(3-x)}+\frac{1}{x}=\frac{1}{f}
\)
\(\frac{x+3-x}{x(3-x)}=\frac{1}{f} \quad\) or \(\quad 3 f=3 x-x^2\)
so, \(x^2-3 x+3 f=0\)
Now \(x=\frac{+3 \pm \sqrt{9-4 \times(3 f)}}{2}\)
or \(x=\frac{+3 \pm \sqrt{9-12 f}}{2}\)
Condition for image to be obtained on the screen, i.e.m real image. \(9-12 f \geq 0\) or \(9 \geq 12 f\) or \(f \leq 0.75 \mathrm{~m}\). so, maximum focal length is 0.75 m.

Q9.19: A screen is placed 90 cm from an object. The image of the object on the screen is formed by a convex lens at two different locations separated by 20 cm . Determine the focal length of the lens.

Answer: The image of the object can be located on the screen for two positions of convex lens such that \(u\) and \(v\) are exchanged. The separation between two positions of the lens is \(x=20 \mathrm{~cm}\). It can be observed from figure.

\(
\begin{aligned}
& u_1+v_1=90 \dots(i)\\
& v_1-u_1=20 \dots(ii)
\end{aligned}
\)
Solving equation (i) and equation (ii)
\(
v_1=55 \mathrm{~cm}, u_1=35 \mathrm{~cm}
\)
Now lens formula, \(\frac{1}{v}-\frac{1}{u}=\frac{1}{f}\)
\(
\frac{1}{55}-\frac{1}{-35}=\frac{1}{f} \quad \text { or } \quad \frac{1}{55}+\frac{1}{35}=\frac{1}{f}
\)
\(
\frac{35+55}{55 \times 35}=\frac{1}{f} \quad \text { or } \quad f=\frac{55 \times 35}{90}=+21.38 \mathrm{~cm}
\)

Q9.20: (a) Determine the ‘effective focal length’ of the combination of the two lenses in Exercise 9.10 , if they are placed 8.0 cm apart with their principal axes coincident. Does the answer depend on which side of the combination a beam of parallel light is incident? Is the notion of effective focal length of this system useful at all?
(b) An object 1.5 cm in size is placed on the side of the convex lens in the arrangement (a) above. The distance between the object and the convex lens is 40 cm . Determine the magnification produced by the two-lens system, and the size of the image.

Answer: (a)
(i) Let a parallel beam of light incident first on convex lens, refraction at convex lens
\(
\begin{aligned}
& \frac{1}{v_1}-\frac{1}{u_1}=\frac{1}{f_1} \Rightarrow \frac{1}{v_1}-\frac{1}{\infty}=\frac{1}{30} \\
& v_1=30 \mathrm{~cm}
\end{aligned}
\)
So, a virtual object for the concave lens at
\(
+22 \mathrm{~cm}
\)
Now refraction at concave lens
\(
\frac{1}{v_2}-\frac{1}{u_2}=\frac{1}{f_2} \quad \text { or } \quad \frac{1}{v_2}-\frac{1}{+22}=\frac{1}{-20}
\)
\(
\frac{1}{v_2}=-\frac{1}{20}+\frac{1}{22}=-\frac{1}{220} \quad \text { or } \quad v_2=-220 \mathrm{~cm}
\)

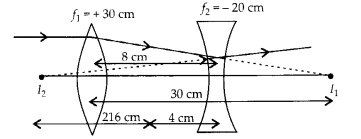
The parallel beam of light appears to diverge from a point 216 cm from the center of the two lens system.
(ii) Now let a parallel beam of light incident first on concave lens.

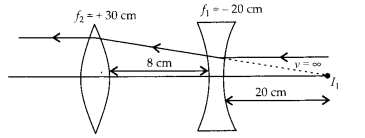
Refraction at concave lens
\(
\frac{1}{v_1}-\frac{1}{u_1}=\frac{1}{f_1} \text { or } \frac{1}{v_1}-\frac{1}{\infty}=\frac{1}{-20} \text { or } v_1=-20 \mathrm{~cm}
\)
The image 11 will act as real object for convex lens at 28 cm .
\(
\begin{aligned}
& \frac{1}{v_2}-\frac{1}{u_2}=\frac{1}{f_2} \quad \text { or } \quad \frac{1}{v_2}-\frac{1}{-28}=\frac{1}{30} \\
& \frac{1}{v_2}=\frac{1}{30}-\frac{1}{28}=-\frac{1}{420} \quad \text { or } \quad v_2=-420 \mathrm{~cm}
\end{aligned}
\)
Thus the parallel incident beam appears to diverge from a point \(420-4=416 \mathrm{~cm}\) on the left of the center of the two lens system. Hence the answer depend upon which side of the lens system the parallel beam is made incident. Therefore the effective focal length is different in two situations.

(b) Now an object of 1.5 cm size is kept 40 cm in front of convex lens in the same system of lenses.

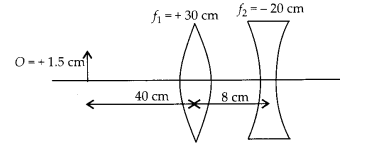
Refraction by the first lens
\(
\begin{aligned}
& \frac{1}{v_1}-\frac{1}{u_1}=\frac{1}{f_1} \quad \text { or } \quad \frac{1}{v_1}-\frac{1}{-40}=\frac{1}{30} \\
& \frac{1}{v_1}=\frac{1}{30}-\frac{1}{40}=\frac{1}{120} \quad \text { or } \quad v_1=120 \mathrm{~cm}
\end{aligned}
\)
Magnification by first lens
\(
m_1=\frac{I}{O}=\frac{v}{u} \quad \text { or } \quad m_1=\frac{I_1}{1.5}=\frac{120}{-40}
\)
\(m_1=-3\) and \(I_1=-4.5 \mathrm{~cm}\)
Refraction by the second lens
\(
\begin{aligned}
& \frac{1}{v_2}-\frac{1}{u_2}=\frac{1}{f_2} \quad \text { or } \quad \frac{1}{v_2}-\frac{1}{+112}=\frac{1}{-20} \\
& \frac{1}{v_2}=-\frac{1}{20}+\frac{1}{112} \quad \text { or } \quad v_2=-\frac{112 \times 20}{92} \mathrm{~cm}
\end{aligned}
\)
Magnification by concave lens
\(
m_2=\frac{v_2}{u_2}=\frac{-112 \times 20}{+112(92)}=-\frac{20}{92}
\)
Total magnification by two lenses
\(
m=m_1 \times m_2 \quad \text { or } \quad m=-3 \times\left(-\frac{20}{92}\right)=0.652
\)
Size of image finally obtained
\(
\begin{aligned}
& m=\frac{I_2}{O_1} \quad \text { or } \quad 0.652=\frac{I_2}{1.5} \\
& I_2=1.5 \times 0.652=0.98 \mathrm{~cm} .
\end{aligned}
\)

Q9.21: At what angle should a ray of light be incident on the face of a prism of refracting angle \(60^{\circ}\) so that it just suffers total internal reflection at the other face? The refractive index of the material of the prism is 1.524.

Answer: The beam should be incident at critical angle or more than critical angle, for total internal reflection at second surface of the prism.

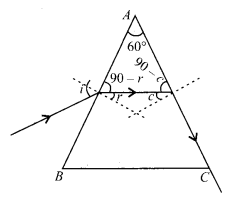
Let us first find critical angle for air glass interface.
We know
\(
\begin{aligned}
& \sin C=\frac{1}{{ }^a \mu_g} \\
& C=\sin ^{-1}\left(\frac{1}{{ }^a \mu_g}\right)=\sin ^{-1}\left(\frac{1}{1.524}\right)
\end{aligned}
\)
Critical angle \(C=41^{\circ}\)
Now we can calculate ‘ \(r\) ‘, as
\(
\begin{aligned}
& 60^{\circ}+\left(90^{\circ}-r\right)+\left(90^{\circ}-C\right)=180^{\circ} \\
& \text { or, } r=19^{\circ}
\end{aligned}
\)
Using Snell’s law, required angle of incidence \(i\) at first surface can be calculated.
\(
\begin{aligned}
{ }^” \mu_g= & \frac{\sin i}{\sin r} \quad \text { or } \quad 1.524=\frac{\sin i}{\sin 19^{\circ}} \\
& \sin i=1.524\left(\sin 19^{\circ}\right) \\
\text { or } i= & \sin i=1.524 \times 0.3256 \Rightarrow i \cong 29.75^{\circ}
\end{aligned}
\)

Q9.22: A card sheet divided into squares each of size \(1 \mathrm{~mm}^2\) is being viewed at a distance of 9 cm through a magnifying glass (a converging lens of focal length 9 cm ) held close to the eye.
(a) What is the magnification produced by the lens? How much is the area of each square in the virtual image?
(b) What is the angular magnification (magnifying power) of the lens?
(c) Is the magnification in (a) equal to the magnifying power in (b)? Explain.

Answer: (a) For magnification by the magnifying lens.

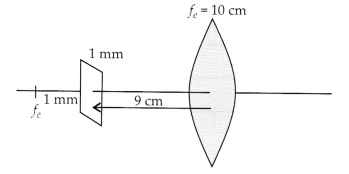
Let us use lens formula
\(
u=-9 \mathrm{~cm}, f=+10 \mathrm{~cm}
\)
\(\frac{1}{v}-\frac{1}{u}=\frac{1}{f} \quad\) or \(\quad \frac{1}{v}-\frac{1}{-9}=\frac{1}{10}\)
\(
\frac{1}{v}=\frac{1}{10}-\frac{1}{9}=-\frac{1}{90}
\)
Image position \(v=-90 \mathrm{~cm}\)
Magnification \(m=\frac{I}{O}=\frac{v}{u} \quad\) or \(\quad m=\frac{-90}{-9}=10\)
For area \(1 \mathrm{~mm}^2\), consider the height of object 1 mm , so height of image.
\(
\begin{aligned}
& \frac{I}{O}=\frac{v}{u}, \frac{I}{1 \mathrm{~mm}}=10 \\
& I=10 \mathrm{~mm}
\end{aligned}
\)
Area of image \(A=10 \times 10 \mathrm{~mm}^2=100 \mathrm{~mm}^2\) \(=1 \mathrm{~cm}^2\)

(b) Angular magnification,
\(
m=\frac{D}{u}=\frac{25}{9}=2.78
\)
(c) No, the linear magnification by a lens and magnifying power (angular magnification) of magnifying glass have different values. The linear magnification is calculated using \(m=\frac{v}{u}\), whereas angular magnification is \(m=\frac{D}{u}=\frac{\beta}{\alpha}\), the ratio of angle subtended by
image of object at eye lens ‘ \(p\) ‘ to the angle subtended by object assumed to be at least distance at eye lens ‘a’.
The linear magnification and angular magnification in microscope have similar magnitude when image is at least distance of distinct vision i.e., 25 cm.

Q9.23: (a) At what distance should the lens be held from the card sheet in Exercise 9.22 in order to view the squares distinctly with the maximum possible magnifying power?
(b) What is the magnification in this case?
(c) Is the magnification equal to the magnifying power in this case? Explain.

Answer: (a) For maximum magnifying power the image should be at least distance of distinct 1 vision i.e., 25 cm .
\(
\begin{aligned}
& \frac{1}{v}-\frac{1}{u}=\frac{1}{f} \text { or } \frac{1}{-25}-\frac{1}{u}=\frac{1}{10} \\
& \text { or }-\frac{1}{u}=\frac{1}{10}+\frac{1}{25}=\frac{7}{50} \\
& u=-\frac{50}{7} \mathrm{~cm}=-7.14 \mathrm{~cm}
\end{aligned}
\)

(b) Linear magnification in the situation of maximum magnifying power.
\(
m=\frac{I}{O}=\frac{v}{u}, m=\frac{v}{u}=\frac{-25}{\frac{-50}{7}}=3.5
\)

(c) Maximum magnifying power in the same situation
\(
\begin{aligned}
& m=\frac{D}{u_{\min }} \text { or }\left(1+\frac{D}{f_e}\right) \\
& m_{\max }=\frac{25}{\frac{50}{7}} \text { or }\left[1+\frac{25}{10}\right] \\
& m_{\max }=3.5
\end{aligned}
\)
So, it can be observed that in the situation when image is least distance of distinct vision the angular magnification and linear magnification have similar values.

Q9.24: What should be the distance between the object in Exercise 9.23 and the magnifying glass if the virtual image of each square in the figure is to have an area of \(6.25 \mathrm{~mm}^2\). Would you be able to see the squares distinctly with your eyes very close to the magnifier?
[Note: Exercises 9.22 to 9.24 will help you clearly understand the difference between magnification in absolute size and the angular magnification (or magnifying power) of an instrument.]

Answer: Now we want the area of square shaped virtual image as \(6.25 \mathrm{~mm}^2\). So, each side of image is \({I}=\sqrt{\mathbf{6 . 2 5}}=\) 2.5 mm (Linear magnification) For the given magnifying lens of focal length 10 cm we can calculate the required position of object.
\(
\begin{aligned}
& \text { Magnification } m=\frac{I}{O}=\frac{v}{u} \\
& \frac{I}{O}=\frac{v}{u} \Rightarrow \frac{2.5 \mathrm{~mm}}{1 \mathrm{~mm}}=\frac{v}{u} \\
& \text { So, } v=2.5 u
\end{aligned}
\)
Lens formula \(\frac{1}{v}=\frac{1}{u}=\frac{1}{f}\) or \(\frac{1}{2.5 u}-\frac{1}{u}=\frac{1}{+10}\)
\(
u=-6 \mathrm{~cm} \text { and } v=-2.5 \times u=-15 \mathrm{~cm}
\)
Thus the required virtual image is closer than normal near point. Thus the eye cannot observe the image distinctly.

Q9.25: Answer the following questions:
(a) The angle subtended at the eye by an object is equal to the angle subtended at the eye by the virtual image produced by a magnifying glass. In what sense then does a magnifying glass provide angular magnification?
(b) In viewing through a magnifying glass, one usually positions one’s eyes very close to the lens. Does angular magnification change if the eye is moved back?
(c) Magnifying power of a simple microscope is inversely proportional to the focal length of the lens. What then stops us from using a convex lens of smaller and smaller focal length and achieving greater and greater magnifying power?
(d) Why must both the objective and the eyepiece of a compound microscope have short focal lengths?
(e) When viewing through a compound microscope, our eyes should be positioned not on the eyepiece but a short distance away from it for best viewing. Why? How much should be that short distance between the eye and eyepiece?

Answer: (a) In magnifying glass the object is placed closer than 25 cm , which produces image at 25 cm . This closer object has larger angular size than the same object at 25 cm . In this way although the angle subtended by virtual image and object is same at eye but angular magnification is achieved.
(b) On moving the eye backward away from lens the angular magnification decreases slightly, as both the angle subtended by the image at eye ‘ \(a\) ‘ and by the object at eye ‘ \(\alpha\) ‘ decreases. Although the decrease in angle subtended by object \(a\) is relatively smaller.
(c) If we decrease focal length, the lens has to be thick with smaller radius of curvature. In a thick lens both the spherical aberrations and chromatic aberrations become pronounced. Further, grinding for small focal length is not easy. Practically we can not get magnifying power more than 3 with a simple convex lens.
(d) Magnifying power of a compound microscope is given by
\(
\begin{aligned}
& m=-\frac{v_o}{u_o}\left[1+\frac{D}{f_e}\right] \\
& \text { With approximations } m=-\frac{L}{f_o}\left[1+\frac{D}{f_e}\right] \text { where }
\end{aligned}
\)
(e) If we place our eye too close to the eyepiece, we shall not collect much of the light and also reduce our field of view. When we position our eye slightly away and the area of the pupil of our eye is greater, our eye will collect all the light refracted by the objective, and a clear image is observed by the eye.

Q9.26: An angular magnification (magnifying power) of 30 X is desired using an objective of focal length 1.25 cm and an eyepiece of focal length 5 cm . How will you set up the compound microscope?

Answer: Here we want the distance between given objective and eye lens for the required magnification of 30 . Let the final image is formed at least distance of distinct vision for eye piece.
\(
\begin{aligned}
& m_e=\left(1+\frac{D}{f_e}\right)=\frac{D}{u_e} \quad \text { or } \quad m_e=1+\frac{25}{5}=\frac{25}{u_e} \\
& m_e=6 \text { and } u_e=\frac{25}{6} \mathrm{~cm}
\end{aligned}
\)
Now magnification by objective lens
\(
\begin{aligned}
& m=m_o \times m_e \\
& m_v=\frac{m}{m_e}=\frac{30}{6}=5
\end{aligned}
\)
Also \(m_o=\frac{v_0}{u_o} \Rightarrow m_0=\frac{v_o}{u_o}=-5\) ( \(\because\) real image is formed by objective.)
So, the relation \(v_o=-5 u_o\)
Now lens formula for objective lens
\(\frac{1}{v_o}-\frac{1}{u_o}=\frac{1}{f_o} \quad\) or \(\quad \frac{1}{-5 u_o}-\frac{1}{u_o}=\frac{1}{1.25}\)
\(\frac{1+5}{5 u_0}=\frac{1}{1.25}\) or \(5 u_0=7.5\) or \(u_0=-1.5 \mathrm{~cm}\)
Also \(v_o=-5 u_o=7.5 \mathrm{~cm}\)
So, required distance between objective and еуеріесе
\(
L=v_o+\left|u_e\right|=7.5+\frac{25}{6}=11.67 \mathrm{~cm}
\)

Q9.27: A small telescope has an objective lens of focal length 140 cm and an eyepiece of focal length 5.0 cm . What is the magnifying power of the telescope for viewing distant objects when
(a) the telescope is in normal adjustment (i.e., when the final image is at infinity)?
(b) the final image is formed at the least distance of distinct vision \((25 \mathrm{~cm})\) ?

Answer: (a) In normal adjustment magnifying power
\(
m=-\frac{f_o}{f_e}=\frac{140}{5}=28
\)

(b) For the image at least distance of distinct vision
Magnifying power \(m=\frac{f_o}{f_e}\left[1+\frac{f_e}{D}\right]\)
\(
m=28\left[\frac{30}{25}\right]=33.6
\)

Q9.28: (a) For the telescope described in Exercise 9.27 (a), what is the separation between the objective lens and the eyepiece?
(b) If this telescope is used to view a 100 m tall tower 3 km away, what is the height of the image of the tower formed by the objective lens?
(c) What is the height of the final image of the tower if it is formed at 25 cm ?

Answer: (a) The separation between objective lens and the eyepiece can be calculated in both the conditions of most relaxed eye and most strained eye. Most relaxed eye \(L=f_0+f_e=140+5=145 \mathrm{~cm}\)
Most strained eye object distance ‘ \(u_e\) ‘ for eye lens
\(
\begin{aligned}
& \frac{1}{v_e}-\frac{1}{u_e}=\frac{1}{f_e} \quad \text { or } \quad \frac{1}{-25}-\frac{1}{u_e}=\frac{1}{5} \\
& -\frac{1}{u_e}=\frac{1}{5}+\frac{1}{25}=\frac{6}{25} \\
& \text { or } \quad u_e=-\frac{25}{6} \mathrm{~cm}=-4.16 \mathrm{~cm}
\end{aligned}
\)
Separation between lenses
\(
L=f_o+\left|u_e\right|=145+4.16=149.16 \mathrm{~cm}
\)

(b)

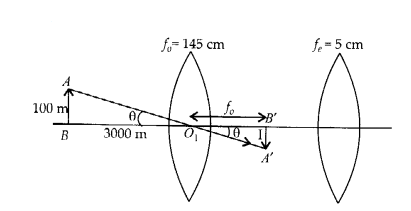
We can calculate height of image by objective lens
\(
\tan \theta=\frac{A^{\prime} B^{\prime}}{B^{\prime} O_1}=\frac{A B}{B O_1}
\)
height of image \(A^{\prime} B^{\prime}=B^{\prime} O_1 \times \frac{A B}{B O_1}\)
\(
A^{\prime} B^{\prime}=140 \times \frac{100}{3000}=4.7 \mathrm{~cm}
\)

(c) Now we want to find the height of final image \(A^{\prime \prime} B^{\prime \prime}\) assuming it to be formed at 25 cm.

Magnification by the eyepiece is
\(
m_e=\left(1+\frac{D}{f_e}\right)=\left(1+\frac{25}{5}\right)=6
\)
Now height of final image, \(m_e=\frac{A^{\prime \prime} B^{\prime \prime}}{A^{\prime} B^{\prime}}\)
\(
A^{\prime \prime} B^{\prime \prime}=m_e \times A^{\prime} B^{\prime}=6 \times 4.7=28.2 \mathrm{~cm}
\)

Q9.29: A Cassegrain telescope uses two mirrors as shown in Figure below. Such a telescope is built with the mirrors 20 mm apart. If the radius of curvature of the large mirror is 220 mm and the small mirror is 140 mm , where will the final image of an object at infinity be?

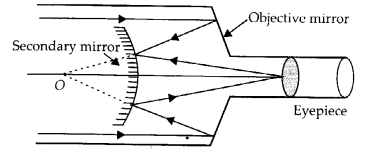
Answer: Image formed by concave mirror acts as a virtual object for convex mirror. Here parallel rays coming from infinity will focus at 110 mm an axis away from concave mirror. Distance of virtual object for convex mirror \(=110-20=90 \mathrm{~mm}\)
For convex mirror
\(
\begin{aligned}
& u=-90 \mathrm{~mm}, f=-70 \mathrm{~mm} \Rightarrow \frac{1}{v}+\frac{1}{u}=\frac{1}{f} \\
& \Rightarrow \quad v=-315 \mathrm{~mm}
\end{aligned}
\)
Hence image is formed at 315 mm from convex mirror.

Q9.30: Light incident normally on a plane mirror attached to a galvanometer coil retraces backwards as shown in Fig. 9.29. A current in the coil produces a deflection of \(3.5^{\circ}\) of the mirror. What is the displacement of the reflected spot of light on a screen placed 1.5 m away?

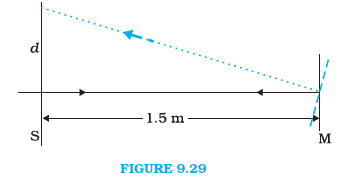
Answer: If the mirror deflect by \(3.5^{\circ}\), the reflected light deflect by \(7^{\circ}\), deflection of the spot d can be calculated.

\(
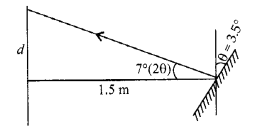
\begin{aligned}
& \tan 7^{\circ}=\frac{d}{1.5} \\
& d=1.5 \tan 7^{\circ}=1.5[0.1228] \mathrm{m} \\
& \text { or } d=0.1842 \mathrm{~m}=18.42 \mathrm{~cm}
\end{aligned}
\)

Q9.31: Figure 9.30 shows an equiconvex lens (of refractive index 1.50 ) in contact with a liquid layer on top of a plane mirror. A small needle with its tip on the principal axis is moved along the axis until its inverted image is found at the position of the needle. The distance of the needle from the lens is measured to be 45.0 cm . The liquid is removed and the experiment is repeated. The new distance is measured to be 30.0 cm . What is the refractive index of the liquid?

Answer: Let us first consider the situation when there is no liquid between lens and plane mirror and the image is formed at 30 cm i.e., at the position of object. As the image is formed on the object position itself, the object must be placed at focus of Biconvex lens.

\(f_0=30 \mathrm{~cm}\) Radius of curvature of convex lens can be calculated
\(
\begin{aligned}
& \frac{1}{f}=\left({ }^a \mu_g-1\right)\left(\frac{1}{R_1}-\frac{1}{R_2}\right) \\
& \text { or } \frac{1}{30}=\left(\frac{3}{2}-1\right)\left(\frac{1}{R}-\frac{1}{-R}\right) \\
& \text { or } \frac{1}{30}=\frac{1}{2}\left(\frac{2}{R}\right) \Rightarrow R=30 \mathrm{~cm}
\end{aligned}
\)
Now a liquid is filled between lens and plane mirror and the image is formed at position of object at 45 cm . The image is formed on the position of object itself, the object must be placed at focus of equivalent lens of Biconvex of glass and Plano convex lens of liquid.

\(
\frac{1}{f_{\mathrm{eq}}}=\frac{1}{f_1}+\frac{1}{f_2} \dots(i)
\)
Equivalent total length \(f_{\text {eq }}=45 \mathrm{~cm}\)
Focal length of Biconvex lens \(f_1=30 \mathrm{~cm}\)
Focal length of plano convex lens
\(
\begin{aligned}
& \frac{1}{f_2}=[\mu-1]\left[\frac{1}{-R}-\frac{1}{\infty}\right] \quad \text { or } \frac{1}{f_2}=[\mu-1]\left[\frac{-1}{30}\right] \\
& f_2=\frac{-30}{\mu-1}
\end{aligned}
\)
Now equation (i),
\(
\begin{aligned}
& \frac{1}{f_{\mathrm{eq}}}=\frac{1}{f_1}+\frac{1}{f_2} \Rightarrow \frac{1}{45}=\frac{1}{30}-\left(\frac{\mu-1}{30}\right) \\
& \frac{\mu-1}{30}=\frac{1}{90} \quad \text { or } \quad \mu-1=\frac{1}{3} \\
& \mu=\frac{1}{3}+1=\frac{4}{3}
\end{aligned}
\)

Exemplar Section

VSA

Q9.17: Will the focal length of a lens for red light be more, same or less than that for blue light?

Answer: By lens maker’s formula,
\(
\frac{1}{f}=(\mu-1)\left(\frac{1}{R_1}-\frac{1}{R_2}\right)
\)
The refractive index for red light is less than that for blue light, \(\mu_{\text {red }}<\mu_{\text {blue }}\)
Hence \(\frac{1}{f_{\text {red }}}<\frac{1}{f_{\text {blue }}} \Rightarrow f_{\text {red }}>f_{\text {blue }}\)
Thus, the focal length for red light will be greater than that for blue light.

Q9.18: The near vision of an average person is 25 cm . To view an object with an angular magnification of 10 , what should be the power of the microscope?

Answer: It is given, the least distance of distinct vision of an average person (i.e., \(D\) ) is 25 cm , in order to view an object with magnification 10 ,
Here, \(v=D=25 \mathrm{~cm}\) and \(u_i=f\)
But the magnifiçation \(m=\frac{v}{u}=\frac{D}{f}\)
\(
\Rightarrow \quad f=\frac{D}{m}=\frac{25}{10}=2.5 \mathrm{~cm}=0.025 \mathrm{~m}
\)
But power \(P=\frac{1}{f(\text { in } \mathrm{m})}=\frac{1}{0.025}=40 \mathrm{D}\)
This is the required power of lens.

Q9.19: An unsymmetrical double convex thin lens forms the image of a point object on its axis. Will the position of the image change if the lens is reversed?

Answer: Key concept: Thin iens formula: \(\frac{1}{v}-\frac{1}{u}=\frac{1}{f}\)
For a given object position if focal length of the lens does not change, the image position remains unchanged.

By lens maker’s formula,
\(
\frac{1}{f}=(\mu-1)\left(\frac{1}{R_1}-\frac{1}{R_2}\right)
\)
For this position \(R_1\) is positive
and \(R_2\) is negative. Hence focal length at this position
\(
\frac{1}{f_1}=(\mu-1)\left(\frac{1}{\left(+R_1\right)}-\frac{1}{\left(-R_2\right)}\right)=(\mu-1)\left(\frac{1}{R_1}+\frac{1}{R_2}\right)
\)
Now the lens is reversed,

At this position, \(R_2\) is positive and \(R_1\) is negative. Hence focal length at this position is
\(
\frac{1}{f_2}=(\mu-1)\left(\frac{1}{\left(+R_2\right)}-\frac{1}{\left(-R_1\right)}\right)=(\mu-1)\left(\frac{1}{R_1}+\frac{1}{R_2}\right)
\)
We can observe the focal length of the lens does not change in both positions, hence the image position remains unchanged.

Q9.20: For a glass prism \((\mu=\sqrt{3})\) the angle of minimum deviation is equal to the angle of the prism. Find the angle of the prism.

Answer: The refractive index of prism angle \(A\) and angle of minimum deviation \(\delta_m\) is given by
\(
\mu=\frac{\sin \left[\frac{\left(A+\delta_m\right)}{2}\right]}{\sin \left(\frac{A}{2}\right)}
\)
Here we are given, \(\delta_m=A\)
Substituting the value, we have \(\mu=\frac{\sin A}{\sin \frac{A}{2}}\)
\(
\begin{aligned}
& \Rightarrow \mu=\frac{2 \sin \frac{A}{2} \cos \frac{A}{2}}{\sin \frac{A}{2}}=2 \cos \frac{A}{2} \\
& \Rightarrow \mu=\frac{\sin A}{\sin \frac{A}{2}}=\frac{2 \sin \frac{A}{2} \cos \frac{A}{2}}{\sin \frac{A}{2}}=2 \cos \frac{A}{2}
\end{aligned}
\)
For the given value of refractive index, we have, \(\cos \frac{A}{2}=\frac{\sqrt{3}}{2} \Rightarrow \frac{A}{2}=30^{\circ}\) or \(A=60^{\circ}\)
This is the required value of prism angle.

SA

Q9.21: A short object of length \(L\) is placed along the principal axis of a concave mirror away from focus. The object distance is \(u\). If the mirror has a focal length \(f\), what will be the length of the image? You may take \(L \ll|v-f|\).

Answer: Since, the object distance is \(u\). Let us consider the two ends of the object be at distance \(u_1=u-L / 2\) and \(u_2=u+L / 2\), respectively so that \(\left|u_1-u_2\right|=L\) Let the image of the two ends be formed at \(v_1\) and \(v_2\), respectively so that the image length would be
\(
L^{\prime}=\left|v_1-v_2\right|
\)
Applying mirror formula, we have
\(
\frac{1}{u}+\frac{1}{v}=\frac{1}{f} \text { or } v=\frac{f u}{u-f}
\)
On solving, the positions of two images are given by
\(
v_1=\frac{f(u-L / 2)}{u-f-L / 2}, v_2=\frac{f(u+L / 2)}{u-f+L / 2}
\)
For length, substituting the value in (i), we have
\(
L^{\prime}=\left|v_1-v_2\right|=\frac{f^2 L}{(u-f)^2 \times L^2 / 4}
\)
Since, the object is short and kept away from focus, we have
\(
\begin{gathered}
L^2 / 4 \ll(u-f)^2 \\
L^{\prime}=\frac{f^2}{(u-f)^2} L
\end{gathered}
\)
This is the required expression of length of image.

Q9.22: A circular disc of radius ‘ \(R\) ‘ is placed co-axially and horizontally inside an opaque hemispherical bowl of radius ‘ \(\alpha\) ‘ (Fig. 9.5). The far edge of the disc is just visible when viewed from the edge of the bowl. The bowl is filled with transparent liquid of refractive index \(\mu\) and the near edge of the disc becomes just visible. How far below the top of the bowl is the disc placed?

Answer: Refering to the figure, \(A M\) is the direction of incidence ray before liquid is filled. After liquid is filled in, \(B M\) is the direction of the incident ray. Refracted ray in both cases is same as that along \(A M\).

Let the disc is separated by O at a distance \(d\) as shown in figure. Also, considering angle
\(
N=90^{\circ}, O M=a, C B=N B=a-R, A N=a+R
\)
Here, in figure
\(
\begin{aligned}
\sin t & =\frac{a-R}{\sqrt{d^2+(a-R)^2}} \\
\text { and } \sin \alpha & =\cos (90-\alpha)=\frac{a+R}{\sqrt{d^2+(a+R)^2}}
\end{aligned}
\)
But on applying Snell’s law,
\(
\frac{1}{\mu}=\frac{\sin t}{\sin r}=\frac{\sin t}{\sin \alpha}
\)
On substituting the values, we have the separation
\(
d=\frac{\mu\left(a^2-b^2\right)}{\sqrt{(a+r)^2-\mu(a-r)^2}}
\)
This is the required expression.

Q9.23: A thin convex lens of focal length 25 cm is cut into two pieces 0.5 cm above the principal axis. The top part is placed at \((0,0)\) and an object placed at \((-50 \mathrm{~cm}, 0)\). Find the coordinates of the image.

Answer: Key concept: If a symmetric lens is cut parallel to principal axis in two parts. Focal length remains the same for each part. Intensity of image formed by each part will be less compared as that of complete lens.
If there was no cut, then the object would have been at a height of 0.5 cm from the principal axis \(O O^{\prime}\).

The top part is placed at \((0,0)\) and an object placed at \((-50 \mathrm{~cm}, 0)\). There is no effect on the focal length of the lens.

\(
\begin{aligned}
\begin{aligned}
{u}=-50 \mathrm{~cm} {f}=+25 \mathrm{~cm}, {v}=? \\
\frac{1}{v}-\frac{1}{u}=\frac{1}{f} \\
\frac{1}{v}-\frac{1}{-50}=\frac{1}{25} \\
\frac{1}{v}=\frac{1}{25}-\frac{1}{50}
\end{aligned}\\
=\frac{2-1}{50}=\frac{1}{50}\\
v=50 \mathrm{~cm}\\
\text { Mangnification is } m=\frac{+v}{u}=\frac{+(50)}{-50}=-1
\end{aligned}
\)

Thus, the image would have been formed at 50 cm from the pole and 0.5 cm below the principal axis. Hence, with respect to the X-axis passing through the edge of the cut lens, the coordinates of the image are ( \(50 \mathrm{~cm},-1 \mathrm{~cm}\) ).

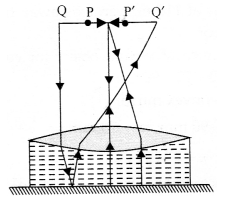
Q9.24: In many experimental set-ups the source and screen are fixed at a distance say \(D\) and the lens is movable. Show that there are two positions for the lens for which an image is formed on the screen. Find the distance between these points and the ratio of the image sizes for these two points.

Answer: Consider a convex lens \(L\) placed between an object \(O\) and a screen \(S\). The distance between the object and the screen is \(D\) and the positions of the object and the screen are held fixed. The lens can be moved along the axis of the system and at a position I a sharp image will be formed on the screen. Interestingly, there is another position on the same axis where a sharp image will once again be obtained on the screen. The position is marked as II. in Figure.

In the figure, let the distance of position I from the object be \(x_1\). Then the distance of the screen from the lens is \(D-x_1\).
Therefore, \(u=-x_I\) and \(v=+\left(D-x_1\right)\).
Plaçing it in the lens formula,
\(
\frac{1}{D-x_1}-\frac{1}{\left(-x_1\right)}=\frac{1}{f} \dots(i)
\)
At position II, let the distance of the lens from the screen be \(x_2\). Then the distance of the screen from the lens is \(D-x_2\).
Therefore, \(u=-x_2\) and \(v=+\left(D-x_2\right)\).
Placing it in the lens formula
\(
\frac{1}{D-x_2}-\frac{1}{\left(-x_2\right)}=\frac{1}{f} \dots(ii)
\)
Comparing Eqs. (i) and (ii), we realize that there are only two solutions:
1. \(x_1=x_2\); or
2. \(D-x_1=x_2\) and \(D-x_2=x_1\)

The first solution is trivial. Therefore, if the first position of the lens, for a sharp image, is \(x_1\) from the object, the second position is at \(D-x_1\) from the object.
Let the distance between the two positions I and II be \(d\). From the diagram, it is clear that
\(
D=x_1+x_2 \text { and } d=x_2-x_1 \dots(iii)
\)
On solving, two equations in (iii) we have
\(
x_1=\frac{D-d}{2} \text { and } D-x_1=\frac{D+d}{2} \dots(iv)
\)
Substituting Eq. (iv) in Eq. (i), we get
\(
\begin{aligned}
& \quad \frac{1}{\left(\frac{D+d}{2}\right)}-\frac{1}{\left(-\frac{D-d}{2}\right)}=\frac{1}{f} \Rightarrow f=\frac{D^2-d^2}{4 D} \dots(v) \\
& \text { or } \quad d=\sqrt{D^2-4 D f} \dots(vi)
\end{aligned}
\)
If \(u=\frac{D}{2}+\frac{d}{2}\), then the image is at \(\mathrm{k}=\frac{D}{2}-\frac{d}{2}\).
\(\therefore\) The magnification \(m_1=\frac{D-d}{D+d}\)
If \(u=\frac{D-d}{2}\), then \(v=\frac{D+d}{2}\)
\(\therefore\) The magnification \(m_2=\frac{D+d}{D-d}\)
Thus, \(\quad \frac{m_2}{m_1}=\left(\frac{D+d}{D-d}\right)^2\).
This is the required expression of magnification.

Important points:

• We notice from Eq. (vi) that a solution for \(d\) is possible only when
• \(D \geq 4 f\)
• When \(D<4 f\), there is no position for which a sharp image can be formed.
• When \(D=4 f\), there is only one position where a sharp image is formed.
• When \(D>4 f\), there are two positions where a sharp image is formed.
• The method is suitable for convex lenses only.

Q9.25: A jar of height \(h\) is filled with a transparent liquid of refractive index \(\mu\) (Fig. 9.6). At the centre of the jar on the bottom surface is a dot. Find the minimum diameter of a disc, such that when placed on the top surface symmetrically about the centre, the dot is invisible.

Answer: In figure, \(O\) is a small dot at the bottom of the jar. The ray from the dot emerges out of a circular patch of water surface of diameter \(A B\) till the angle of incidence for the rays \(O A\) and \(O B\) exceeds the critical angle ( \(i_c\) ).

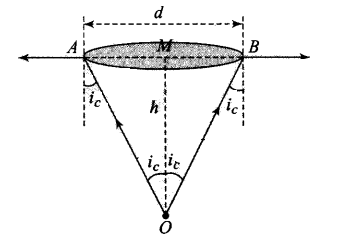
Rays of light incident at an angle greater than \(i_{{c}}\), are totally reflected within water and consequently cannot emerge out of the water surface.
As \(\sin i_c=\frac{1}{\mu} \Rightarrow \tan i_c=\frac{1}{\sqrt{\mu^2-1}}\),
Now, \(\quad \frac{d / 2}{h}=\tan i_c\)
\(
\begin{aligned}
& \Rightarrow \quad \frac{d}{2}=h \tan i_c=h \frac{1}{\sqrt{\mu^2-1}} \\
& \Rightarrow \quad d=\frac{2 h}{\sqrt{\mu^2-1}}
\end{aligned}
\)
This is the required expression of \(d\).

LA

Q9.26: Show that for a material with refractive index \(\mu \geq \sqrt{2}\), light incident at any angle shall be guided along a length perpendicular to the incident face.

Answer: Any ray entering at an angle \(i\) shall be guided along \(A C\) if the angle ray makes with the face \(A C(\phi)\) is greater than the critical angle as per the principle of total internal reflection \(\phi+r=90^{\circ}\), therefore \(\sin \phi=\cos r\)

\(
\begin{aligned}
& \sin \phi \geq \frac{1}{\mu} \\
& \cos r \geq \frac{1}{\mu} \\
& 1-\cos ^2 r \leq 1-\frac{1}{\mu^2} \\
& \sin ^2 r \leq \frac{1}{\mu^2} \\
& \sin ^2 r \leq 1-\frac{1}{\mu^2}
\end{aligned}
\)
since, \(\sin i=\mu \sin r\)
\(
\frac{1}{\mu_2} \sin ^2 i \leq 1-\frac{1}{\mu^2} \text { or } \sin ^2 i \leq \mu^2-1
\)
when \(i=\frac{\pi}{2}\)
Then, we have smallest angle \(\phi\).
If that is greater than the critical angle, then all other angles of incidence shall be more than the critical angle.
Thus, \(1 \leq \mu^2-1\)
\(
\begin{aligned}
\mu^2 & \geq 2 \\
\mu & \geq \sqrt{2}
\end{aligned}
\)

Q9.27: The mixture a pure liquid and a solution in a long vertical column (i.e, horizontal dimensions << vertical dimensions) produces diffusion of solute particles and hence a refractive index gradient along the vertical dimension. A ray of light entering the column at right angles to the vertical is deviated from its original path. Find the deviation in travelling a horizontal distance \(d \ll h\), the height of the column.

Answer: Let us consider a portion of a ray between \(x\) and \(x+d x\) inside the liquid solution. Let the angle of incidence of ray at \(x\) be \(\theta\) and let the ray enters the thin column at height \(y\). Because of the refraction it deviates from the original path and emerges at \(x+d x\) with an angle \(\theta+d \theta\) and at a height \(y+d y\).

From Snell’s law,
\(
\mu(y) \sin \theta=\mu(y+d y) \sin (\theta+d \theta)) \dots(i)
\)
Let refractive index of the liquid at position \(y\) be \(\mu(y)=\mu\), then \(\mu(y+d y)=\mu+\left(\frac{d \mu}{d y}\right) d y=\mu+k d y\)
where \(k=\left(\frac{d \mu}{d y}\right)=\) refractive index gradient along the vertical dimension.
Hence from (i), \(\mu \sin \theta=(\mu+k d y) \cdot \sin (\theta+d \theta)\)
\(
\begin{aligned}
& \mu \sin \theta=(\mu+k d y) \cdot(\sin \theta \cdot \cos d \theta+\cos \theta \cdot \sin d \theta) \\
& \mu \sin \theta=(\mu+k d y) \cdot(\sin \theta \cdot 1+\cos \theta \cdot d \theta) \dots(ii)
\end{aligned}
\)
For small angle \(\sin d \theta \approx d \theta\) and \(\cos d \theta \approx 1\)
\(
\mu \sin \theta=\mu \sin \theta+k d y \sin \theta+\mu \cos \theta \cdot d \theta+k \cos \theta d y \cdot d \theta
\)
\(
k d y \sin \theta+\mu \cos \theta \cdot d \theta=0 \Rightarrow d \theta=-\frac{k}{\mu} \tan \theta d y
\)
But \(\tan \theta=\frac{d x}{d y}\) and \(k=\left(\frac{d \mu}{d y}\right)\)
\(
d \theta=-\frac{k}{\mu}\left(\frac{d x}{d y}\right) d y \Rightarrow d \theta=-\frac{k}{\mu} d x
\)
Integrating both sides, \(\int_0^\delta d \theta=-\frac{k}{\mu} \int_0^d d x\)
\(
\Rightarrow \quad \delta=-\frac{k d}{\mu}=-\frac{d}{\mu}\left(\frac{d \mu}{d y}\right)
\)

Q9.28: If light passes near a massive object, the gravitational interaction causes a bending of the ray. This can be thought of as happening due to a change in the effective refrative index of the medium given by
\(
n(r)=1+2 G M / r c^2
\)
where \(r\) is the distance of the point of consideration from the centre of the mass of the massive body, \(G\) is the universal gravitational constant, \(M\) the mass of the body and c the speed of light in vacuum. Considering a spherical object find the deviation of the ray from the original path as it grazes the object.

Answer: Let us consider two spherical surfaces of radius \(r\) and \(r+d r\). Let the light be incident at an angle \(\theta\) at the surface at \(r\) and leave \(r+d r\) at an angle \(\theta+d \theta\). Then from Snell’s law,
\(
\begin{aligned}
n(r) \sin \theta= & n(r+d r) \sin (\theta+d \theta) \\
& =\left(n(r)+\left(\frac{d n}{d r}\right) d r\right)(\sin \theta \cdot \cos d \theta+\cos \theta \cdot \sin d \theta)
\end{aligned}
\)
\(
\Rightarrow \quad n(r) \sin \theta=\left(n(r)+\left(\frac{d n}{d r}\right) d r\right)(\sin \theta+\cos \theta \cdot d \theta)
\)
For small angle, \(\sin d \theta \approx d \theta\) and \(\cos d \theta \approx 1\)
Ignoring the product of differentials
\(
\Rightarrow \quad n(r) \sin \theta=n(r) \cdot \sin \theta+\left(\frac{d n}{d r}\right) d r \cdot \sin \theta+n(r) \cdot \cos \theta \cdot d \theta
\)
or we have, \(-\frac{d n}{d r} \tan \theta=n(r) \frac{d \theta}{d r}\)
\(
\begin{aligned}
& \frac{2 G M}{r^2 c^2} \tan \theta=\left(1+\frac{2 G M}{r c^2}\right) \frac{d \theta}{d r} \approx \frac{d \theta}{d r} \\
& \int_0^{\theta_0} d \theta=\frac{2 G M}{c^2} \int_{-\infty}^{\infty} \frac{\tan \theta^* d r}{r^2}
\end{aligned}
\)
Now, \(r^2=x^2+R^2\) and \(\tan \theta=\frac{R}{x}\)
\(
2 r d r=2 x d x
\)
Now substitution for integrals, we have
\(
\int_0^{\theta_0} d \theta=\frac{2 G M}{c^2} \int_{-\infty}^{\infty} \frac{R}{x} \frac{x d x}{\left(x^2+R^2\right)^{\frac{3}{2}}}
\)
Put \(x=R \tan \phi\)
\(
d x=R \sec ^2 \phi d \phi
\)
\(
\begin{aligned}
\therefore \quad \theta_0 & =\frac{2 G M R}{c^2} \int_{-\pi / 2}^{\pi / 2} \frac{R \sec ^2 \phi d \phi}{R^3 \sec ^3 \phi} \\
\theta_0 & =\frac{2 G M}{R c^2} \int_{-\pi / 2}^{\pi / 2} \cos \phi d \phi=\frac{4 G M}{R c^2}
\end{aligned}
\)
\(\Rightarrow \theta_0=\frac{4 G M}{R c^2}\). This is the required proof.

Q9.29: An infinitely long cylinder of radius \(R\) is made of an unusual exotic material with refractive index -1 (Figure below). The cylinder is placed between two planes whose normals are along the \(y\) direction. The center of the cylinder \(O\) lies along the \(y\)-axis. A narrow laser beam is directed along the \(y\) direction from the lower plate. The laser source is at a horizontal distance \(x\) from the diameter in the \(y\) direction. Find the range of \(x\) such that light emitted from the lower plane does not reach the upper plane.

Answer: Here the material has refractive index \(-1, \theta_r\) is negative and \(\theta_r^{\prime}\) positive.
Now, \(\quad\left|\theta_i\right|=\left|\theta_r\right|=\left|\theta_r^{\prime}\right|\)
The total deviation of the outcoming ray from the incoming ray is \(4 \theta_t\). Rays shall not reach the receiving plate if \(\frac{\pi}{2} \leq 4 \theta_i \leq \frac{3 \pi}{2}\) [angles measured clockwise from the \(y\)-axis]

On solving,
\(
\frac{\pi}{8} \leq \theta_i \leq \frac{3 \pi}{8}
\)
Now, \(\quad \sin \theta_i=\frac{x}{R}\)
\(
\begin{aligned}
& \frac{\pi}{8} \leq \sin ^{-1} \frac{x}{R} \leq \frac{3 \pi}{8} \\
& \frac{\pi}{8} \leq \frac{x}{R} \leq \frac{3 \pi}{8}
\end{aligned}
\)
Thus, for light emitted from the source shall not reach the receiving plate. If \(\frac{R \pi}{8} \leq x \leq \frac{R 3 \pi}{8}\).