NCERT Exercise Q & A

EXERCISE PROBLEMS

Q7.1: A \(100 \Omega\) resistor is connected to a \(220 \mathrm{~V}, 50 \mathrm{~Hz}\) ac supply.
(a) What is the rms value of current in the circuit?
(b) What is the net power consumed over a full cycle?

Answer: Resistance of the resistor, \(R=100 \Omega\)
Supply voltage, \(V=220 \mathrm{~V}\)
Frequency, \(v=50 \mathrm{~Hz}\)
(a) The rms value of current in the circuit is given as:
\(
\begin{aligned}
I & =\frac{V}{R} \\
& =\frac{220}{100}=2.20 \mathrm{~A}
\end{aligned}
\)
(b) The net power consumed over a full cycle is given as:
\(
\begin{aligned}
& P=V I \\
& =220 \times 2.2=484 \mathrm{~W}
\end{aligned}
\)

Q7.2: (a) The peak voltage of an ac supply is 300 V . What is the rms voltage?
(b) The rms value of current in an ac circuit is 10 A . What is the peak current?

Answer: (a) Peak voltage of the ac supply, \(V_0=300 \mathrm{~V}\)
Rms voltage is given as:
\(
\begin{aligned}
V & =\frac{V_0}{\sqrt{2}} \\
& =\frac{300}{\sqrt{2}}=212.1 \mathrm{~V}
\end{aligned}
\)

(b) Therms value of current is given as:
\(
I=10 \mathrm{~A}
\)
Now, peak current is given as:
\(
\begin{aligned}
I_0 & =\sqrt{2} I \\
& =10 \sqrt{2}=14.1 \mathrm{~A}
\end{aligned}
\)

Q7.3: A 44 mH inductor is connected to \(220 \mathrm{~V}, 50 \mathrm{~Hz}\) ac supply. Determine the rms value of the current in the circuit.

Answer: Inductance of inductor, \(L=44 \mathrm{mH}=44 \times 10^{-3} \mathrm{H}\)
Supply voltage, \(V=220 \mathrm{~V}\)
Frequency, \(v=50 \mathrm{~Hz}\)
Angular frequency, \(\omega=2 \pi v\)
Inductive reactance, \(X_L=\omega L=2 \pi v L=2 \pi \times 50 \times 44 \times 10^{-3} \Omega\)
Rms value of current is given as:
\(
\begin{aligned}
I & =\frac{v}{X_{\mathrm{L}}} \\
& =\frac{220}{2 \pi \times 50 \times 44 \times 10^{-3}}=15.92 \mathrm{~A}
\end{aligned}
\)
Hence, the rms value of current in the circuit is 15.92 A .

Q7.4: A \(60 \mu \mathrm{~F}\) capacitor is connected to a \(110 \mathrm{~V}, 60 \mathrm{~Hz}\) ac supply. Determine the rms value of the current in the circuit.

Answer: Capacitance of capacitor, \(C=60 \mu \mathrm{~F}=60 \times 10^{-6} \mathrm{~F}\)
Supply voltage, \(V=110 \mathrm{~V}\)
Frequency, \(v=60 \mathrm{~Hz}\)
Angular frequency, \(\omega=2 \pi v\)
Capacitive reactance
\(
X_{\mathrm{c}}=\frac{1}{\omega C}
\)
\(
\begin{aligned}
& =\frac{1}{2 \pi v C} \\
& =\frac{1}{2 \times 3.14 \times 60 \times 60 \times 10^{-6}} \Omega^{-1}
\end{aligned}
\)
Rms value of current is given as:
\(
\begin{aligned}
I & =\frac{v}{X_{\mathrm{c}}} \\
& =110 \times 2 \times 3.14 \times 60 \times 10^{-6} \times 60=2.49 \mathrm{~A}
\end{aligned}
\)
Hence, the rms value of current is 2.49 A .

Q7.5: In Exercises 7.3 and 7.4 , what is the net power absorbed by each circuit over a complete cycle. Explain your answer.

Answer: In the inductive circuit,
Rms value of current, \(I=15.92 \mathrm{~A}\)
Rms value of voltage, \(V=220 \mathrm{~V}\)
Hence, the net power absorbed can be obtained by the relation,
\(
P=V I \cos \phi
\)
Where,
\(\phi=\) Phase difference between \(V\) and \(I\)
For a pure inductive circuit, the phase difference between alternating voltage and current is \(90^{\circ}\) i.e., \(\phi=90^{\circ}\).
Hence, \(P=0\) i.e., the net power is zero.
In the capacitive circuit,
Rms value of current, \(I=2.49 \mathrm{~A}\)
Rms value of voltage, \(V=110 \mathrm{~V}\)
Hence, the net power absorbed can ve obtained as:
\(
P=V I \operatorname{Cos} \phi
\)
For a pure capacitive circuit, the phase difference between alternating voltage and current is \(90^{\circ}\) i.e., \(\phi=90^{\circ}\).
Hence, \(P=0\) i.e., the net power is zero.

Q7.6: A charged \(30 \mu \mathrm{~F}\) capacitor is connected to a 27 mH inductor. What is the angular frequency of free oscillations of the circuit?

Answer: Capacitance, \(C=30 \mu \mathrm{~F}=30 \times 10^{-6} \mathrm{~F}\)
Inductance, \(L=27 \mathrm{mH}=27 \times 10^{-3} \mathrm{H}\)
Angular frequency is given as:
\(
\begin{aligned}
\omega_r & =\frac{1}{\sqrt{L C}} \\
& =\frac{1}{\sqrt{27 \times 10^{-3} \times 30 \times 10^{-6}}}=\frac{1}{9 \times 10^{-4}}=1.11 \times 10^3 \mathrm{rad} / \mathrm{s}
\end{aligned}
\)
Hence, the angular frequency of free oscillations of the circuit is \(1.11 \times 10^3 \mathrm{rad} / \mathrm{s}\).

Q7.7: A series \(L C R\) circuit with \(R=20 \Omega, L=1.5 \mathrm{H}\) and \(C=35 \mu \mathrm{~F}\) is connected to a variable-frequency 200 V ac supply. When the frequency of the supply equals the natural frequency of the circuit, what is the average power transferred to the circuit in one complete cycle?

Answer: At resonance, the frequency of the supply power equals the natural frequency of the given \(L C R\) circuit.
Resistance, \(R=20 \Omega\)
Inductance, \(L=1.5 \mathrm{H}\)
Capacitance, \(C=35 \mu \mathrm{~F}=30 \times 10^{-6} \mathrm{~F}\)
AC supply voltage to the \(L C R\) circuit, \(V=200 \mathrm{~V}\)
Impedance of the circuit is given by the relation,
\(
Z=\sqrt{R^2+\left(\omega L-\frac{1}{\omega C}\right)^2}
\)
At resonance,
\(
\omega L=\frac{1}{\omega C}
\)
\(
\therefore Z=R=20 \Omega
\)
Current in the circuit can be calculated as:
\(
\begin{aligned}
I & =\frac{V}{Z} \\
& =\frac{200}{20}=10 \mathrm{~A}
\end{aligned}
\)
Hence, the average power transferred to the circuit in one complete cycle= \(V I\)
\(
=200 \times 10=2000 \mathrm{~W}
\)

Q7.8: Figure 7.17 shows a series \(L C R\) circuit connected to a variable frequency 230 V source. \(L=5.0 \mathrm{H}, C=80 \mu \mathrm{~F}, R=40 \Omega\).

(a) Determine the source frequency which drives the circuit in resonance.
(b) Obtain the impedance of the circuit and the amplitude of current at the resonating frequency.
(c) Determine the rms potential drops across the three elements of the circuit. Show that the potential drop across the \(L C\) combination is zero at the resonating frequency.

Answer: Inductance of the inductor, \(L=5.0 \mathrm{H}\)
Capacitance of the capacitor, \(C=80 \mu \mathrm{H}=80 \times 10^{-6} \mathrm{~F}\)
Resistance of the resistor, \(R=40 \Omega\)
Potential of the variable voltage source, \(V=230 \mathrm{~V}\)
(a) Resonance angular frequency is given as:
\(
\begin{aligned}
\omega_R & =\frac{1}{\sqrt{L C}} \\
& =\frac{1}{\sqrt{5 \times 80 \times 10^{-6}}}=\frac{10^3}{20}=50 \mathrm{rad} / \mathrm{s}
\end{aligned}
\)
Hence, the circuit will come in resonance for a source frequency of \(50 \mathrm{rad} / \mathrm{s}\).
(b) Impedance of the circuit is given by the relation,
\(
Z=\sqrt{R^2+\left(\omega L-\frac{1}{\omega C}\right)^2}
\)
At resonance,
\(
\begin{aligned}
& \omega L=\frac{1}{\omega C} \\
& \therefore Z=R=40 \Omega
\end{aligned}
\)
Amplitude of the current at the resonating frequency is given as:
\(
I_0=\frac{V_0}{Z}
\)
Where,
\(
\begin{aligned}
V_0 & =\text { Peak voltage } \\
& =\sqrt{2} V \\
\therefore I_0 & =\frac{\sqrt{2} V}{Z} \\
& =\frac{\sqrt{2} \times 230}{40}=8.13 \mathrm{~A}
\end{aligned}
\)
Hence, at resonance, the impedance of the circuit is \(40 \Omega\) and the amplitude of the current is 8.13 A .
(c) Rms potential drop across the inductor,
\(
\left(V_L\right)_{\mathrm{rms}}=I \times \omega_R L
\)
Where,
\(
I=\mathrm{rms} \text { current }
\)
\(
\begin{aligned}
& =\frac{I_0}{\sqrt{2}}=\frac{\sqrt{2} V}{\sqrt{2} Z}=\frac{230}{40} \mathrm{~A} \\
& \therefore\left(V_L\right)_{\text {ms }}=\frac{230}{40} \times 50 \times 5=1437.5 \mathrm{~V}
\end{aligned}
\)
Potential drop across the capacitor,
\(
\begin{aligned}
\left(V_c\right)_{\mathrm{rms}} & =I \times \frac{1}{\omega_R C} \\
& =\frac{230}{40} \times \frac{1}{50 \times 80 \times 10^{-6}}=1437.5 \mathrm{~V}
\end{aligned}
\)
Potential drop across the resistor,
\(
\begin{aligned}
& \left(V_R\right)_{\mathrm{rms}}=I R \\
& =\frac{230}{40} \times 40=230 \mathrm{~V}
\end{aligned}
\)
Potential drop across the \(L C\) combination,
\(
V_{L C}=I\left(\omega_R L-\frac{1}{\omega_R C}\right)
\)
At resonance,
\(
\omega_R L=\frac{1}{\omega_R C}
\)
\(
\therefore V_{L C}=0
\)
Hence, it is proved that the potential drop across the \(L C\) combination is zero at resonating frequency.

Exemplar Section

VSA (Very Short Answer Type)

Q7.15: Draw the effective equivalent circuit of the circuit shown in Fig 7.1, at very high frequencies and find the effective impedance.

Answer: Key concept: The element with infinite resistance will be considered as open circuit and the element with zero resistance will be considered as short circuited.
In similar way, \(X_C=\frac{1}{2 \pi \nu C}\)
At high frequency, \(\mathrm{X}_{\mathrm{C}}\) will be low.
So reactance of capacitance can be considered negligible and capacitor can be considered as short circuited.
Here the above figure is equivalent circuit to given circuit.
Total impedance, \(\mathrm{Z}=\mathrm{R}_1+\mathrm{R}_3\)

Q7.16: Study the circuits (a) and (b) shown in Fig 7.2 and answer the following questions.

(a) Under which conditions would the rms currents in the two circuits be the same?
(b) Can the rms current in circuit (b) be larger than that in (a)?

Answer: 

\(
\begin{aligned}
& \left(\mathrm{I}_{\mathrm{rms}}\right) \text { in }(\mathrm{a})=\frac{V_{r m s}}{R} \\
& \left(\mathrm{I}_{\mathrm{rms}}\right) \text { in } \mathrm{b}=\frac{V_{r m s}}{Z}=\frac{V_{r m s}}{\sqrt{R^2+\left(X_L-X_C\right)}}
\end{aligned}
\)

(a) \(\left(\mathrm{I}_{\mathrm{rms}}\right) \mathrm{a}=\left(\mathrm{I}_{\mathrm{rms}}\right) \mathrm{b}\)
\(
\therefore \mathrm{R}=\sqrt{R^2+\left(X_L-X_C\right)^2}
\)
Squaring both sides
\(
\mathrm{R}^2=\mathrm{R}^2+\left(\mathrm{X}_{\mathrm{L}}-\mathrm{X}_{\mathrm{C}}\right)^2
\)
Or \(\left(\mathrm{X}_{\mathrm{L}}-\mathrm{X}_{\mathrm{C}}\right)^2=0\)
\(
\mathrm{X}_{\mathrm{L}}=\mathrm{X}_{\mathrm{C}}
\)
\(
\begin{aligned}
& \text { (b) For }\left(\mathrm{I}_{\mathrm{rms}}\right) \mathrm{b}>\left(\mathrm{I}_{\mathrm{rms}}\right) \mathrm{a} \\
& \frac{V_{r m s}}{\sqrt{R^2+\left(X_L-X_C\right)^2}}>\frac{V_{r m s}}{R} \\
& \text { As } \mathrm{V}_{\mathrm{rms}}=\mathrm{V} \text { so, } \\
& \sqrt{R^2+\left(X_L-X_C\right)^2}<R
\end{aligned}
\)
Squaring both sides
\(
\begin{aligned}
& \mathrm{R}^2+\left(\mathrm{X}_{\mathrm{L}}-\mathrm{X}_{\mathrm{C}}\right)^2<\mathrm{R}^2 \\
& \left(\mathrm{X}_{\mathrm{L}}-\mathrm{X}_{\mathrm{C}}\right)^2<0
\end{aligned}
\)
Square of any number can never be negative. Reactance of \(\mathrm{X}_{\mathrm{L}}\) and \(\mathrm{X}_{\mathrm{C}}\) cannot be negative.
So the rms current in circuit (b) cannot be larger than that in (a).

Q7.17: Can the instantaneous power output of an ac source ever be negative? Can the average power output be negative?

Answer: Let the applied e.m.f \(\mathrm{E}=\mathrm{E}_0 \sin (\omega \mathrm{t})\)
\(
\mathrm{I}=\mathrm{I}_0 \sin (\omega \mathrm{t}-\phi)
\)
Instantaneous power output of ac source
\(
\begin{aligned}
& \mathrm{P}=\mathrm{EI} \\
& =\mathrm{E}_0 \sin \omega \mathrm{I}_0 \sin (\omega \mathrm{t}-\phi) \\
& =\mathrm{E}_0 \mathrm{I}_0 \sin \omega \mathrm{t}[\sin \omega \mathrm{t} \cos \phi-\cos \omega \mathrm{t} \sin \phi] \\
& =\mathrm{E}_0 \mathrm{I}_0\left[\sin ^2 \omega \mathrm{t} \cos \phi-\sin \omega \mathrm{t} \cos \omega \mathrm{t} \sin \phi\right] \\
& =\mathrm{E}_0 \mathrm{I}_0\left[\frac{(1-\cos 2 \omega t)}{2} \cos \phi-\frac{1}{2} \sin 2 \omega t \sin \phi\right] \\
& =\frac{E_0 I_0}{2}[\cos \phi-\cos 2 \omega t \cos \phi-\sin 2 \omega t \sin \phi] \\
& =\frac{E_0 I_0}{2}[\cos \phi-(\cos 2 \omega t \cos \phi+\sin 2 \omega t \sin \phi] \\
& \mathrm{P}=\frac{E_0 I_0}{2}[\cos \phi-\cos (2 \omega t-\phi)]
\end{aligned}
\)
Taken phase angle \(\phi, \pm\) ve.
Instantaneous Power \(\mathrm{P}=\frac{E_0 I_0}{2}[\cos \phi \pm \cos (2 \omega t-\phi)]\) as \(\cos \phi=\frac{R}{Z}, \mathrm{R}\) and \(Z\) can never be negative and value of \(\cos \phi(\phi=2 \omega t \pm \phi)\) can vary from (1 to 0 to -1) in any case \(P\) can never be negative.
We know that average power of LCR series ac circuit is \(\mathrm{P}_{\mathrm{av}}=\frac{E_0 I_0}{2} \cos \phi\)
Again as \(\cos \phi=\frac{R}{Z}\) is always positive, because \(R\) and \(Z\) , the reactances are always positive. So \(\mathrm{P}_{\mathrm{av}}\) can never be negative.

Q7.18: In series LCR circuit, the plot of \(I_{\max }\) vs \(\omega\) is shown in Fig 7.3. Find the bandwidth and mark in the figure.

Answer: Consider the diagram .

\(
\text { Bandwidth }=\omega_2-\omega_1
\)
where \(\omega_1\) and \(\omega_2\) corresponds to frequencies at which magnitude of current is \(\frac{1}{\sqrt{2}}\) times of maximum value.
\(
I_{\mathrm{rms}}=\frac{I_{\mathrm{max}}}{\sqrt{2}}=\frac{1}{\sqrt{2}} \approx 0.7 \mathrm{~A}
\)
Clearly from the diagram, the corresponding frequencies are \(0.8 \mathrm{rad} / \mathrm{s}\) and \(1.2 \mathrm{rad} / \mathrm{s}\).
\(
\Delta \omega=\text { Bandwidth }=1.2-0.8=0.4 \mathrm{rad} / \mathrm{s}
\)

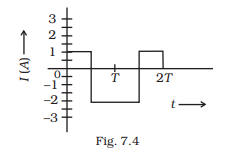
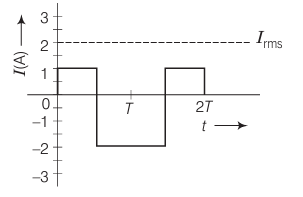
Q7.19: The alternating current in a circuit is described by the graph shown in Fig 7.4. Show rms current in this graph.

Answer: 

\(
\begin{aligned}
I_{\mathrm{rms}} & =\text { rms current } \\
& =\sqrt{\frac{1^2+2^2}{2}}=\sqrt{\frac{5}{2}}=1.58 \mathrm{~A} \approx 1.6 \mathrm{~A}
\end{aligned}
\)
The rms value of the current \(\left(I_{\mathrm{rms}}\right)=1.6 \mathrm{~A}\) is indicated in the graph.

Explanation:

\(
\begin{aligned}
& i_{r m s}=\sqrt{\frac{i_1^2 \times T_1+i_2^2 \times T_2+i_3^2 \times T_3}{T_{\text {total }}}} \\
& \Rightarrow i_{r m s}=\sqrt{\frac{1^2\left(\frac{T}{2}\right)+(-2)^2(T)+1^2\left(\frac{T}{2}\right)}{2 T}} \\
& \Rightarrow i_{r m s}=\sqrt{\frac{\frac{T}{2}+4 T+\frac{T}{2}}{2 T}} \\
& \Rightarrow i_{r m s}=\sqrt{\frac{5 T}{2 T}} \\
& \therefore i_{r m s}=1.58 \mathrm{~A} \approx 1.6 \mathrm{~A}
\end{aligned}
\)

Q7.20: How does the sign of the phase angle \(\phi\), by which the supply voltage leads the current in an \(L C R\) series circuit, change as the supply frequency is gradually increased from very low to very high values.

Answer: The phase angle \(\phi\) by which voltage leads the current in LCR series circuit where \(\mathrm{X}_{\mathrm{L}}>\mathrm{X}_{\mathrm{C}}\).
\(
\tan \phi=\frac{X_L-X_C}{R}=\frac{2 \pi \nu L-\frac{1}{2 \pi \nu C}}{R}
\)
If \(\nu\) is small \(\mathrm{X}_{\mathrm{C}}>\mathrm{X}_{\mathrm{L}}\) so \(\left[2 \pi \nu L-\frac{1}{2 \pi \nu C}\right]\) is negative, so \(\tan \phi<0\).
For \(\nu\) is large, \(\mathrm{X}_{\mathrm{L}}>\mathrm{X}_{\mathrm{C}}\)
So \(\mathrm{X}_{\mathrm{L}}=\mathrm{X}_{\mathrm{C}}\) is positive to \(\tan \phi>0\)
For \(\mathrm{X}_{\mathrm{L}}=\mathrm{X}_{\mathrm{C}}\) i.e. at resonant frequency
\(
\mathrm{X}_{\mathrm{L}}-\mathrm{X}_{\mathrm{C}}=0 \text { so } \tan \phi=0
\)
So phase angle in series LCR ac circuit will change from a negative to zero and then zero to positive value.

SA (Short Answer Type)

Q7.21: A device ‘ X ‘ is connected to an a.c source. The variation of voltage, current and power in one complete cycle is shown in Fig 7.5.
(a) Which curve shows power consumption over a full cycle?
(b) What is the average power consumption over a cycle?
(c) Identify the device ‘ X ‘.

Answer: (a) Power is the product of voltage and current (Power \(=\mathrm{P}=\mathrm{VI})\).
So, the curve of power will be having maximum amplitude, equals to the product of amplitudes of voltage \((\mathrm{V})\) and current \((\mathrm{I})\) curve. Frequencies, of B and C are-equal, therefore they represent \((\mathrm{V})\) and \((\mathrm{I})\) curves. So, the curve A represents power.
(b) The full cycle of the graph (as shown by shaded area in the diagram) consists of one positive and one negative symmetrical area.

Hence, average power consumption over a cycle is zero.
(c) Here phase difference between \((\mathrm{V})\) and \((\mathrm{I})\) is \(\pi / 2\) therefore, the device ‘ X ‘ may be an inductor ( L ) or capacitor (C) or the series combination of L and C.

Q7.22: Both alternating current and direct current are measured in amperes. But how is the ampere defined for an alternating current?

Answer: For a Direct Current (DC),
1 ampere \(=1\) coulomb \(/ \mathrm{sec}\)
Direction of AC changes with the frequency of source with the source frequency and the attractive force would average to zero. Thus, the AC ampere must be defined in terms of some property that is independent of the direction of current. Joule’s heating effect is such property and hence it is used to define rms value of AC.
So, r.m.s. value of AC is equal to that value of DC, which when passed through a resistance for a given time will produce the same amount of heat as produced by the alternating current when passed through the same resistance for same time.

Q7.23: A coil of 0.01 henry inductance and 1 ohm resistance is connected to 200 volt, 50 Hz ac supply. Find the impedance of the circuit and time lag between max. alternating voltage and current.

Answer:

\(
\begin{aligned}
X_L & =\omega L=2 \pi f L \\
& =3.14 \Omega \\
Z & =\sqrt{R^2+L^2} \\
& =\sqrt{(3.14)^2+(1)^2}=\sqrt{10.86} \\
& \simeq 3.3 \Omega
\end{aligned}
\)
\(
\begin{aligned}
\tan \phi & =\frac{\omega L}{R}=3.14 \\
\phi & =\tan ^{-1}(3.14) \\
& \simeq 72^{\circ} \\
& \simeq \frac{72 \times \pi}{180} \mathrm{rad}
\end{aligned}
\)
\(
\text { Time lag } \Delta t=\frac{\phi}{\omega}=\frac{72 \times \pi}{180 \times 2 \pi \times 50}=\frac{1}{250} \mathrm{~s}
\)

Q7.24: A 60 W load is connected to the secondary of a transformer whose primary draws line voltage. If a current of 0.54 A flows in the load, what is the current in the primary coil? Comment on the type of transformer being used.

Answer: \(\mathrm{P}_{\mathrm{S}}=60 \mathrm{~W}, \mathrm{I}_{\mathrm{S}}=0.54 \mathrm{~A}, \mathrm{I}_{\mathrm{P}}=\) ?
\(
\begin{aligned}
& \mathrm{P}_{\mathrm{S}}=\mathrm{V}_{\mathrm{S}} \mathrm{I}_{\mathrm{S}} \\
& 60=\mathrm{V}_{\mathrm{S}} \times 0.54 \\
& \frac{60}{0.54}=V_{\mathrm{S}}
\end{aligned}
\)
\(\mathrm{V}_{\mathrm{S}}=111.10 \mathrm{~Volt}\)
In multiple of 11
\(
\mathrm{V}_{\mathrm{S}} \cong 110 \mathrm{~Volt}
\)
Ratio factor of transformer ‘ r ‘ = output voltage/input voltage
\(
r=\frac{V_s}{V_P} \Rightarrow r=\frac{110 \mathrm{~Volt}}{220 \mathrm{~Volt}}=\frac{1}{2}
\)
Or \(\mathrm{r}<1\), so transformer is step down transformer.
In transformer, output power=input power
\(
\begin{aligned}
&\mathrm{I}_{\mathrm{S}} \mathrm{~V}_{\mathrm{S}}=\mathrm{I}_{\mathrm{P}} \mathrm{~V}_{\mathrm{P}}\\
&I_P=\frac{I_s V_S}{V_P}=\frac{0.54 \times 110}{220}=0.27 \mathrm{~A} .
\end{aligned}
\)

Q7.25: Explain why the reactance provided by a capacitor to an alternating current decreases with increasing frequency.

Answer: A capacitor does not allow flow of direct current through it as the resistance across the gap is infinite. When an alternating voltage is applled across the capacitor plates, the plates are alternately charged and discharged. The current through the capacitor is a result of this changing voltage (or charge). Thus, a capacitor will pass more current through it if the voltage is changing at a faster rate, i.e. If the frequency of supply is higher. This implies that the reactance offered by a capacitor is less with increasing frequency; it is given by \(X_C=1 / \omega C\).

Q7.26: Explain why the reactance offered by an inductor increases with increasing frequency of an alternating voltage.

Answer: An inductor opposes flow of current through it by developing a back emf according to Lenz’s law. The induced voltage has a polarity so as to maintain the current at its present value. If the current is decreasing, the polarity of the induced emf will be so as to increase the current and vice versa. Since the induced emf is proportional to the rate of change of current, it will provide greater reactance to the flow of current if the rate of change is faster, i.e. If the frequency is higher. The reactance of an inductor, therefore, is proportional to the frequency, being given by \(X_L=\omega L\).

LA (Long Answer Type)

Q7.27: An electrical device draws 2 kW power from AC mains (voltage \(223 \mathrm{~V}(\mathrm{rms})=\sqrt{50,000} \mathrm{~V}\) ). The current differs (lags) in phase by \(\phi\left(\tan \phi=\frac{-3}{4}\right)\) as compared to voltage. Find (i) \(R\), (ii) \(X_C-X_L\), and (iii) \(I_M\). Another device has twice the values for \(R, X_C\) and \(X_L\). How are the answers affected?

Answer:

\(
\begin{aligned}
&\text { Power } P=\frac{V^2}{Z} \Rightarrow \frac{50,000}{2000}=25=Z\\
&\begin{aligned}
& Z^2=R^2+\left(X_C-X_L\right)^2=625 \\
& \tan \phi=\frac{X_{\mathrm{C}}-X_{\mathrm{L}}}{R}=-\frac{3}{4}
\end{aligned}\\
&625=R^2+\left(-\frac{3}{4} R\right)^2=\frac{25}{16}\\
&R^2=400 \Rightarrow \mathrm{R}=20 \Omega\\
&\begin{aligned}
& X_C-X_L=-15 \Omega \\
& I=\frac{V}{Z}=\frac{223}{25} \simeq 9 \mathrm{~A} \\
& I_M=\sqrt{2} \times 9=12.6 \mathrm{~A}
\end{aligned}
\end{aligned}
\)
If \(R, X_C, X_L\) are all doubled, \(\tan \phi\) does not change. \(Z\) is doubled, current is halfed. Power drawn is halfed.

Q7.28: 1 MW power is to be delivered from a power station to a town 10 km away. One uses a pair of Cu wires of radius 0.5 cm for this purpose. Calculate the fraction of ohmic losses to power transmitted if
(i) power is transmitted at 220 V . Comment on the feasibility of doing this.
(ii) a step-up transformer is used to boost the voltage to 11000 V , power transmitted, then a step-down transfomer is used to bring voltage to 220 V .
\(
\left(\rho_{C u}=1.7 \times 10^{-8} \text { SI unit }\right)
\)

Answer: (i) Resistance of Cu wires, R
\(
=\rho \frac{l}{A}=\frac{1.7 \times 10^{-8} \times 20000}{\pi \times\left(\frac{1}{2}\right)^2 \times 10^{-4}}=4 \Omega
\)
\(I\) at \(220 \mathrm{~V}: V I=10^6 \mathrm{~W} ; I=\frac{10^6}{220}=0.45 \times 10^4 \mathrm{~A}\)
\(R I^2=\) Power loss
\(
\begin{aligned}
& =4 \times(0.45)^2 \times 10^8 \mathrm{~W} \\
& >10^6 \mathrm{~W}
\end{aligned}
\)
This method cannot be used for transmission

(ii) When power \(P=10^6 \mathrm{~W}\) is transmitted at 11000 V .
\(V^{\prime} I^{\prime}=10^6 \mathrm{~W}=11000 I^{\prime}\)
\(
\begin{aligned}
& I^{\prime}=\frac{1}{1.1} \times 10^2 \\
& R I^{\prime 2}=\frac{1}{1.21} \times 4 \times 10^4=3.3 \times 10^4 \mathrm{~W}
\end{aligned}
\)
Fraction of power loss \(=\frac{3.3 \times 10^4}{10^6}=3.3 \%\)

Q7.29: Consider the \(L C R\) circuit shown in Fig 7.6. Find the net current \(i\) and the phase of \(i\). Show that \(i=\frac{v}{Z}\). Find the impedence \(Z\) for this circuit.

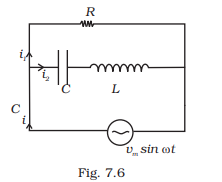
Answer: 

\(
\begin{aligned}
&\begin{aligned}
& R t_1=v_m \sin \omega t t_1=\frac{v_m \sin \omega t}{R} \\
& \frac{q_2}{C}+L \frac{d q_2^2}{d t^2}=v_m \sin \omega t
\end{aligned}\\
&\text { Let } q_2=q_m \sin (\omega \mathrm{t}+\phi)\\
&q_m\left(\frac{q_m}{C}-L \omega^2\right) \sin (\omega t+\phi)=v_m \sin \omega t\\
&q_m=\frac{v_m}{\frac{1}{C}-L \omega^2}, \phi=0 ; \frac{1}{C}-\omega^2 L>0
\end{aligned}
\)
\(
\begin{aligned}
& v_{\mathrm{R}}=\frac{v_m}{L w^2-\frac{1}{C}}, \phi=\pi L \omega^2-\frac{1}{C}>0 \\
& i_2=\frac{d q_2}{d t}=\omega q_m \cos (\omega t+\phi)
\end{aligned}
\)
\(t_1\) and \(t_2\) are out of phase. Let us assume \(\frac{1}{C}-\omega^2 L>0\)
\(
t_1+t_2=\frac{v_m \sin \omega t}{R}+\frac{v_m}{L \omega-\frac{1}{c \omega}} \cos \omega t
\)
Now \(\mathrm{A} \sin \omega t+\mathrm{B} \cos \omega t=\mathrm{C} \sin (\omega t+\phi)\)
\(
\mathrm{C} \cos \phi=\mathrm{A}, \mathrm{C} \sin \phi=\mathrm{B} ; \mathrm{C}=\sqrt{A^2+B^2}
\)
\(
\begin{aligned}
&\text { Therefore, } t_1+t_2=\left[\frac{v_m^2}{R^2}+\frac{v_m^2}{[\omega l-1 / \omega C]^2}\right]^{\frac{1}{2}} \sin (\omega t+\phi)\\
&\phi=\tan ^{-1} \frac{R}{X_L-X_C}\\
&\frac{1}{Z}=\left\{\frac{1}{R^2}+\frac{1}{(L \omega-1 / \omega C)^2}\right\}^{1 / 2}
\end{aligned}
\)