NCERT Exercise Q & A

EXERCISE PROBLEMS

Q5.1: A short bar magnet placed with its axis at \(30^{\circ}\) with a uniform external magnetic field of 0.25 T experiences a torque of magnitude equal to \(4.5^{\circ} \times 10^{-2} \mathrm{~J}\). What is the magnitude of magnetic moment of the magnet?

Solution: Magnetic field strength, \(B=0.25 \mathrm{~T}\)
Torque on the bar magnet, \(T=4.5 \times 10^{-2} \mathrm{~J}\)
Angle between the bar magnet and the external magnetic field, \(\theta=30^{\circ}\)
Torque is related to magnetic moment ( \(M\) ) as:
\(
T=M B \sin \theta
\)
\(
\begin{aligned}
\therefore M & =\frac{T}{B \sin \theta} \\
& =\frac{4.5 \times 10^{-2}}{0.25 \times \sin 30^{\circ}}=0.36 \mathrm{~J} \mathrm{~T}^{-1}=0.36 \mathrm{~A} \mathrm{~m}^{2}
\end{aligned}
\)
Hence, the magnetic moment of the magnet is \(0.36 \mathrm{~J} \mathrm{~T}^{-1}=0.36 \mathrm{~A} \mathrm{~m}^{2}\).

Q5.2: A short bar magnet of magnetic moment \(\mathrm{m}=0.32 \mathrm{JT}^{-1}\) is placed in a uniform magnetic field of 0.15 T . If the bar is free to rotate in the plane of the field, which orientation would correspond to its (a) stable, and (b) unstable equilibrium? What is the potential energy of the magnet in each case?

Answer: (a)The bar magnet is aligned along the magnetic field. This system is considered as being in stable equilibrium. Hence, the angle \(\theta\), between the bar magnet and the magnetic field is \(0^{\circ}\).
Potential energy of the system \(=-M B \cos \theta\)
\(
\begin{aligned}
& =-0.32 \times 0.15 \cos 0^{\circ} \\
& =-4.8 \times 10^{-2} \mathrm{~J}
\end{aligned}
\)
(b)The bar magnet is oriented \(180^{\circ}\) to the magnetic field. Hence, it is in unstable equilibrium.
\(
\theta=180^{\circ}
\)
Potential energy \(=-M B \cos \theta\)
\(
\begin{aligned}
& =-0.32 \times 0.15 \cos 180^{\circ} \\
& =4.8 \times 10^{-2} \mathrm{~J}
\end{aligned}
\)

Q5.3: A closely wound solenoid of 800 turns and area of cross section \(2.5 \times 10^{-4} \mathrm{~m}^2\) carries a current of 3.0 A. Explain the sense in which the solenoid acts like a bar magnet. What is its associated magnetic moment?

Answer: Number of turns in the solenoid, \(n=800\)
Area of cross-section, \(A=2.5 \times 10^{-4} \mathrm{~m}^2\)
Current in the solenoid, \(I=3.0 \mathrm{~A}\)
A current-carrying solenoid behaves as a bar magnet because a magnetic field develops along its axis, i.e., along its length.
The magnetic moment associated with the given current-carrying solenoid is calculated as:
\(
\begin{aligned}
& M=n I A \\
& =800 \times 3 \times 2.5 \times 10^{-4} \\
& =0.6 \mathrm{~J} \mathrm{~T}^{-1}==0.6 \mathrm{~A} \mathrm{~m}^{2}
\end{aligned}
\)

Q5.4: If the solenoid in Exercise 5.5 is free to turn about the vertical direction and a uniform horizontal magnetic field of 0.25 T is applied, what is the magnitude of torque on the solenoid when its axis makes an angle of \(30^{\circ}\) with the direction of applied field?

Answer: Magnetic field strength, \(B=0.25 \mathrm{~T}\)
Magnetic moment, \(M=0.6 \mathrm{~T}^{-1}\)
The angle \(\theta\), between the axis of the solenoid and the direction of the applied field is \(30^{\circ}\).
Therefore, the torque acting on the solenoid is given as:
\(
\begin{aligned}
\tau & =M B \sin \theta \\
& =0.6 \times 0.25 \sin 30^{\circ} \\
& =7.5 \times 10^{-2} \mathrm{~J}
\end{aligned}
\)

Q5.5: A bar magnet of magnetic moment \(1.5 \mathrm{~J} \mathrm{~T}^{-1}\) lies aligned with the direction of a uniform magnetic field of 0.22 T .
(a) What is the amount of work required by an external torque to turn the magnet so as to align its magnetic moment: (i) normal to the field direction, (ii) opposite to the field direction?
(b) What is the torque on the magnet in cases (i) and (ii)?

Answer: (a) Magnetic moment, \(M=1.5 \mathrm{~J} \mathrm{~T}^{-1}\)
Magnetic field strength, \(B=0.22 \mathrm{~T}\)
(i)Initial angle between the axis and the magnetic field, \(\theta_1=0^{\circ}\)
Final angle between the axis and the magnetic field, \(\theta_2=90^{\circ}\)
The work required to make the magnetic moment normal to the direction of magnetic
field is given as:
\(
\begin{aligned}
W & =-M B\left(\cos \theta_2-\cos \theta_1\right) \\
& =-1.5 \times 0.22\left(\cos 90^{\circ}-\cos 0^{\circ}\right) \\
& =-0.33(0-1) \\
& =0.33 \mathrm{~J}
\end{aligned}
\)
(ii) Initial angle between the axis and the magnetic field, \(\theta_1=0^{\circ}\)
Final angle between the axis and the magnetic field, \(\theta_2=180^{\circ}\)
The work required to make the magnetic moment opposite to the direction of magnetic
field is given as:
\(
\begin{aligned}
W & =-M B\left(\cos \theta_2-\cos \theta_1\right) \\
& =-1.5 \times 0.22\left(\cos 180-\cos 0^{\circ}\right) \\
& =-0.33(-1-1) \\
& =0.66 \mathrm{~J}
\end{aligned}
\)
(b) For case (i): \(\theta=\theta_2=90^{\circ}\)
\(\therefore\) Torque, \(\tau=M B \sin \theta\)
\(
\begin{aligned}
& =1.5 \times 0.22 \sin 90^{\circ} \\
& =0.33 \mathrm{~J}
\end{aligned}
\)
For case (ii): \(\theta=\theta_2=180^{\circ}\)
\(\therefore\) Torque, \(\tau=M B \sin \theta\)
\(
=M B \sin 180^{\circ}=0 \mathrm{~J}
\)

Q5.6: A closely wound solenoid of 2000 turns and area of cross-section \(1.6 \times 10^{-4} \mathrm{~m}^2\), carrying a current of 4.0 A , is suspended through its centre allowing it to turn in a horizontal plane.
(a) What is the magnetic moment associated with the solenoid?
(b) What is the force and torque on the solenoid if a uniform horizontal magnetic field of \(7.5 \times 10^{-2} \mathrm{~T}\) is set up at an angle of \(30^{\circ}\) with the axis of the solenoid?

Answer: Number of turns on the solenoid, \(n=2000\)
Area of cross-section of the solenoid, \(A=1.6 \times 10^{-4} \mathrm{~m}^2\)
Current in the solenoid, \(I=4 \mathrm{~A}\)
(a)The magnetic moment along the axis of the solenoid is calculated as:
\(
\begin{aligned}
& M=n A I \\
& =2000 \times 1.6 \times 10^{-4} \times 4 \\
& =1.28 \mathrm{Am}^2
\end{aligned}
\)
(b) Magnetic field, \(B=7.5 \times 10^{-2} \mathrm{~T}\)
Angle between the magnetic field and the axis of the solenoid, \(\theta=30^{\circ}\)
Torque, \(\tau=M B \sin \theta\)
\(
\begin{aligned}
& =1.28 \times 7.5 \times 10^{-2} \sin 30^{\circ} \\
& =4.8 \times 10^{-2} \mathrm{Nm}
\end{aligned}
\)
Since the magnetic field is uniform, the force on the solenoid is zero. The torque on the solenoid is \(4.8 \times 10^{-2} \mathrm{Nm}\).

Q5.7: A short bar magnet has a magnetic moment of \(0.48 \mathrm{~J} \mathrm{~T}^{-1}\). Give the direction and magnitude of the magnetic field produced by the magnet at a distance of 10 cm from the centre of the magnet on (a) the axis, (b) the equatorial lines (normal bisector) of the magnet.

Answer: Magnetic moment of the bar magnet, \(M=0.48 \mathrm{~J} \mathrm{~T}^{-1}\)
(a) Distance, \(d=10 \mathrm{~cm}=0.1 \mathrm{~m}\)
The magnetic field at distance \(d\), from the centre of the magnet on the axis is given by the relation:
\(
B=\frac{\mu_0}{4 \pi} \frac{2 M}{d^3}
\)
Where,
\(
\begin{aligned}
& \mu_0=\text { Permeability of free space }=4 \pi \times 10^{-7} \mathrm{Tm} \mathrm{~A}^{-1} \\
& \begin{aligned}
\therefore B & =\frac{4 \pi \times 10^{-7} \times 2 \times 0.48}{4 \pi \times(0.1)^3} \\
& =0.96 \times 10^{-4} \mathrm{~T}=0.96 \mathrm{G}
\end{aligned}
\end{aligned}
\)
The magnetic field is along the \(\mathrm{S}-\mathrm{N}\) direction.
(b) The magnetic field at a distance of 10 cm (i.e., \(d=0.1 \mathrm{~m}\) ) on the equatorial line of the magnet is given as:
\(
\begin{aligned}
&\begin{aligned}
B & =\frac{\mu_0 \times M}{4 \pi \times d^3} \\
& =\frac{4 \pi \times 10^{-7} \times 0.48}{4 \pi(0.1)^3} \\
& =0.48 \mathrm{G}
\end{aligned}\\
&\text { The magnetic field is along the } \mathrm{N}-\mathrm{S} \text { direction. }
\end{aligned}
\)

Q5.8: A short bar magnet placed in a horizontal plane has its axis aligned along the magnetic north-south direction. Null points are found on the axis of the magnet at 14 cm from the centre of the magnet. The earth’s magnetic field at the place is 0.36 G and the angle of dip is zero. What is the total magnetic field on the normal bisector of the magnet at the same distance as the null-point (i.e., 14 cm ) from the centre of the magnet? (At null points, field due to a magnet is equal and opposite to the horizontal component of earth’s magnetic field.)

Answer: Earth’s magnetic field at the given place, \(H=0.36 \mathrm{G}\)
The magnetic field at a distance \(d\), on the axis of the magnet is given as:
\(
B_1=\frac{\mu_0}{4 \pi} \frac{2 M}{d^3}=H \dots(i)
\)
Where,
\(\mu_0=\) Permeability of free space
\(M=\) Magnetic moment
The magnetic field at the same distance \(d\), on the equatorial line of the magnet is given as:
\(
B_2=\frac{\mu_0 M}{4 \pi d^3}=\frac{H}{2} \quad \quad[\text { Using equation }(i)]
\)
Total magnetic field, \(B=B_1+B_2\)
\(
\begin{aligned}
& =H+\frac{H}{2} \\
& =0.36+0.18=0.54 \mathrm{G}
\end{aligned}
\)
Hence, the magnetic field is 0.54 G in the direction of earth’s magnetic field.

Q5.9: If the bar magnet in exercise 5.8 is turned around by \(180^{\circ}\), where will the new null points be located?

Answer: The magnetic field on the axis of the magnet at a distance \(d_1=14 \mathrm{~cm}\), can be written as:
\(
B_1=\frac{\mu_0 2 M}{4 \pi\left(d_1\right)^3}=H \dots(1)
\)
Where,
\(M=\) Magnetic moment
\(\mu_0=\) Permeability of free space
\(H=\) Horizontal component of the magnetic field at \(d_1\)
If the bar magnet is turned through \(180^{\circ}\), then the neutral point will lie on the equatorial line.
Hence, the magnetic field at a distance \(d_2\), on the equatorial line of the magnet can be written as:
\(
B_2=\frac{\mu_0 M}{4 \pi\left(d_2\right)^3}=H \dots(2)
\)
Equating equations (1) and (2), we get:
\(
\begin{aligned}
& \frac{2}{\left(d_1\right)^3}=\frac{1}{\left(d_2\right)^3} \\
& \left(\frac{d_2}{d_1}\right)^3=\frac{1}{2} \\
& \therefore d_2=d_1 \times\left(\frac{1}{2}\right)^{\frac{1}{3}}
\end{aligned}
\)
\(
\begin{aligned}
&=14 \times 0.794=11.1 \mathrm{~cm}\\
&\text { The new null points will be located } 11.1 \mathrm{~cm} \text { on the normal bisector. }
\end{aligned}
\)

EXEMPLAR PROBLEMS

VSA

Q5.11: A proton has spin and magnetic moment just like an electron. Why then its effect is neglected in magnetism of materials?

Answer: Spinning of a proton is negligible as compared to that of electron spin because its mass is very larger than the mass of an electron.
The comparison between the spinning of a proton and an electron can be done by comparing their magnetic dipole moment which can be given by
As we know that magnetic moment of a charged particle of charge e and mass m is \({M_e}=\frac{e h}{4 \pi m e}\) and \({M_p}=\) \(\frac{e h}{4 \pi m p}\)
As charge on proton and electron are equal in magnitude so
\(
M \infty \frac{1}{m} \text { or } \frac{M_e}{M_p}=\frac{m_p}{m_e}
\)
As the mass of proton is 1836 times as of electron, so magnetic moment of proton
\(
M_p=\frac{M_e}{m_p} m_e=\frac{M_e \cdot m_e}{1836 m_e}, M_p=\frac{1}{1836} \text { of } M_e
\)
So magnetic moment of proton is \(\frac{1}{1836}\) times of electron, so can be neglected.

Q5.12: Explain quantitatively the order of magnitude difference between the diamagnetic susceptibility of \(\mathrm{N}_2\left(\sim 5 \times 10^{-9}\right)\) (at STP) and \(\mathrm{Cu}\left(\sim 10^{-5}\right)\).

Answer: Magnetic susceptibility: It is the property of the substance which shows how easily a substance can be magnetised. It can also be defined as the ratio of intensity of magnetisation (\(I\)) in a substance to the magnetic intensity \((H)\) applied to the substance, i.e., \(X_M=I / H\).
According to the problem, we have
Density of nitrogen \(\rho_{N_2}=\frac{28 \mathrm{~g}}{22.4 \mathrm{~L}}=\frac{28 \mathrm{~g}}{22400 \mathrm{cc}}\)
Also. density of copper \(\rho_{C_u}=\frac{8 \mathrm{~g}}{22.4 \mathrm{~L}}=\frac{8 \mathrm{~g}}{22400 \mathrm{cc}}\)
So, ratio of both densities
\(
\frac{\rho_{N_2}}{\rho_{C u}}=\frac{28}{22400} \times \frac{1}{8}=1.6 \times 10^{-4}
\)
\(
x \alpha \text { density } \rho
\)
Also given \(\frac{\chi_{N_2}}{\chi_{C u}}=\frac{5 \times 10^{-9}}{10^{-5}}=5 \times 10^{-4}\), Hence major difference is accounted for by density.
\(
\left(\chi_m\right)_{\text {Fero }} \approx 10^3
\)
\(
\text { and }\left(\chi_m\right)_{\text {Para }} \approx 10^{-5}
\)
\(
\Rightarrow \quad \frac{\left(\chi_m\right)_{\text {Fero }}}{\left(\chi_m\right)_{\text {Para }}}=\frac{10^3}{10^{-5}}=10^8
\)

Q5.13: From molecular view point, discuss the temperature dependence of susceptibility for diamagnetism, paramagnetism and ferromagnetism.

Answer: Diamagnetism is due to orbital motion of electrons developing magnetic moments opposite to applied fleld and hence is not much affected by temperature.
Paramagnetism and ferromagnetism is due to alignments of atomic magnetic moments in the direction of the applied field. As temperature increases, this aligment is disturbed and hence susceptibilitles of both decrease as temperature increases.

Q5.14: A ball of superconducting material is dipped in liquid nitrogen and placed near a bar magnet. (i) In which direction will it move?
(ii) What will be the direction of it’s magnetic moment?

Answer: Key concept: A superconducting material and nitrogen both are diamagnetic in nature.
When a diamagnetic material is dipped in liquid nitrogen, it again behaves as a diamagnetic material. Thus, superconducting material will again behave as a diamagnetic material. When this diamagnetic material is placed near a bar magnet, it will be feebly magnetised opposite to the direction of magnetising field.

(i) So it will move away from the magnet.
(ii) Magnetic moment is from left to right and it is opposite to the direction of magnetic field.

SA (Short Answer Type)

Q5.16: Verify the Gauss’s law for magnetic field of a point dipole of dipole moment \(m\) at the origin for the surface which is a sphere of radius \(R\).

Answer:

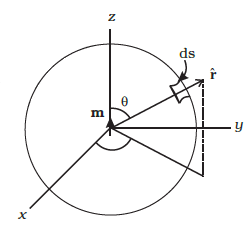
We know by Gauss’s law in magnetism \(\oint_s \vec{B} \cdot \vec{s}=0\)
Magnetic moment ( m ) of dipole at origin O is \(\vec{m}=m \hat{k}\).
Let P be a point at a distance \(r\) from O and OP makes an angle \(\theta\) with z axis. Component of \(\vec{m}\) along \(O P\) is equal to \(\vec{m} \cos \theta\).
Now the magnetic field of induction at P due to dipole of moment \(\vec{m} \cos \theta\) is
\(
B=\frac{\mu_0}{4 \pi} \frac{2 \vec{m} \cos \theta}{r^3} \hat{r}
\)
From the diagram, \(r\) is the radius of a sphere with a centre at 0 lying in the \(y z\)-plane.
An elementary area \(dS\) is taken at \(P\)
\(
d S=r(r \sin \theta d \theta) \hat{r}=\left(r^2 \sin \theta d \theta\right) \hat{r}
\)
\(
\oint B . d s=\oint \frac{\mu_0}{4 \pi} 2 \vec{m} \frac{\cos \theta}{r^3} \widehat{r}\left(r^2 \sin \theta d \theta\right) \widehat{r}
\)
\(
=\frac{\frac{\mu_0}{4 \pi} \vec{m}}{r} \int_0^{2 \pi} 2 \sin \theta \cos \theta d \theta
\)
\(
\begin{aligned}
& =\frac{\mu_0}{4 \pi} \frac{\vec{m}}{r} \int_0^{2 \pi} \sin 2 \theta d \theta \\
& =-\frac{\mu_0}{4 \pi} \frac{\vec{m}}{2 r} \int_0^{2 \pi}\left(-\frac{\cos 2 \theta}{2}\right) \\
& =-\frac{\mu_0}{4 \pi} \frac{\vec{m}}{2 r}[\cos 4 \pi-\cos 0]=-\frac{\mu_0}{4 \pi} \frac{\vec{m}}{2 r}[1-1]=0
\end{aligned}
\)

Q5.17: Three identical bar magnets are rivetted together at centre in the same plane as shown in Figure below. This system is placed at rest in a slowly varying magnetic field. It is found that the system of magnets does not show any motion. The north-south poles of one magnet is shown in the Figure below. Determine the poles of the remaining two.

Answer: If the net force on the system is zero and the net torque on the system is also zero, then the system will be in equilibrium. This is possible only when the poles of the remaining two magnets are as shown below.

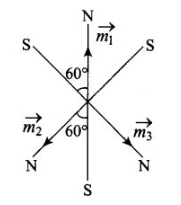
The north pole of the magnet (1) is equally attracted by the south pole of (2) and (3) magnets placed at equal distances. Similarly, one pole of any one magnet is attracted by the opposite poles of the other two magnets. The resultant force or moment of each magnet is zero (Net \(m=0\))

Q5.18: Suppose we want to verify the analogy between electrostatic and magnetostatic by an explicit experiment. Consider the motion of (i) electric dipole \(\mathbf{p}\) in an electrostatic field \(\mathbf{E}\) and (ii) magnetic dipole \(\mathbf{m}\) in a magnetic field \(\mathbf{B}\). Write down a set of conditions on \(\mathbf{E}, \mathbf{B}, \mathbf{p}\), \(\mathbf{m}\) so that the two motions are verified to be identical. (Assume identical initial conditions.)

Answer: Let \(\theta\) is the angle between \(\vec{m}\) and \(\vec{B}\)
\(\therefore\) Torque on magnetic dipole in a magnetic field \(\vec{B}\) is
\(
\tau=\vec{m} \vec{B} \sin \theta \dots(I)
\)
Similarly of \(\theta\) is the angle between electric dipole moment \(\vec{p}\) and electric field \(\vec{E}\) then torque on electric dipole in \(\vec{E}\) is
\(
\tau=\vec{p} \vec{E} \sin \theta \dots(II)
\)
For if motion in I and II of electric and magnetic dipole are identical then \(\tau^{\prime}=\tau\)
\(
\vec{p} \vec{E} \sin \theta=\vec{m} \vec{B} \sin \theta
\)
Or \(\vec{p} \vec{E}=\vec{m} \vec{B} \dots(III)\)
We know that \(\vec{E}=\vec{c} \vec{B} \dots(IV)\) (relation between \(\vec{E}\) and \(\vec{B}\) )
c is velocity of light
Put the value of \(\vec{E}\) from IV in III
\(
\vec{p} c B=\vec{m} \vec{B}
\)
\(
\vec{p}=\frac{\vec{m}}{c}
\)
It is the required relation.

Q5.19: A bar magnet of magnetic moment \(m\) and moment of inertia I (about centre, perpendicular to length) is cut into two equal pieces, perpendicular to length. Let \(T\) be the period of oscillations of the original magnet about an axis through the mid point, perpendicular to length, in a magnetic field \(\mathbf{B}\). What would be the similar period \(T^{\prime}\) for each piece?

Answer: So original time period \(\mathrm{T}=\sqrt[2 \pi]{\frac{I}{m B}}\)
where \(I\) is the moment of inertia, \(m\) is the magnetic moment, and \(B\) is the magnetic field.
When the bar magnet is cut into two equal pieces, each piece will have:
Magnetic moment \(m^{\prime}=\frac{m}{2}\) (since the magnetic moment is proportional to the length of the magnet)
Let the new moment of inertia is \(I^{\prime}\)
The moment of inertia \(I\) of a bar magnet about its center (perpendicular to its length) is given by:
\(
I=\frac{m l^2}{12}
\)
When the magnet is cut into two equal pieces, the mass of each piece is \(\frac{m}{2}\) and the length is \(=l^{\prime}=\frac{l}{2}\). The new moment of inertia \(I^{\prime}\) for each piece is:
\(
I^{\prime}=\frac{1}{12}\left(\frac{m}{2}\right)\left(\frac{l}{2}\right)^2=\frac{1}{12} \cdot \frac{m}{2} \cdot \frac{l^2}{4}=\frac{m l^2}{96}
\)
The new period of oscillation \(T^{\prime}\) for each piece can be expressed as:
\(
T^{\prime}=2 \pi \sqrt{\frac{I^{\prime}}{m^{\prime} B}}
\)
\(
I^{\prime}=\frac{1}{2} \times \frac{1}{4} I
\)
\(
\text { and } m^{\prime}=\frac{m}{2}
\)
\(
T^{\prime}=\frac{1}{2} T
\)

Q5.20: Use (i) the Ampere’s law for \(\mathbf{H}\) and (ii) continuity of lines of \(\mathbf{B}\), to conclude that inside a bar magnet, (a) lines of \(\mathbf{H}\) run from the \(N\) pole to \(S\) pole, while (b) lines of \(\mathbf{B}\) must run from the \(S\) pole to \(N\) pole.

Answer:

Consider a line of \(\mathbf{B}\) through the bar magnet. It must be closed. Let C be the amperian loop.
\(
\int_Q^p \mathbf{H} \cdot d \mathbf{l}=\int_Q^p \frac{\mathbf{B}}{\mu_0} \cdot d \mathbf{l}
\)
\(\mathbf{B}\) and \(d \mathbf{l}\) are at an acute angle to each other inside the magnet.
\(
\int_Q^p \mathbf{H} \cdot d \mathbf{l}=\int_Q^p \frac{\mathbf{B}}{\mu_0} \cdot d \boldsymbol{l}>0, \text { i.e. positive }
\)
Hence, the lines of \(\mathbf{B}\) must run from south pole \(S\) to north pole \(N\) inside the bar magnet.
According to ampere’s law
\(
\int_{PQP} \mathbf{H} \cdot d \mathbf{l}=0
\)
\(
\int_{PQP} \mathbf{H} \cdot d \mathbf{l}=\int_P^Q \mathbf{H} \cdot d \mathbf{l}+ \int_Q^P \mathbf{H} \cdot d \mathbf{l}
\)
As \(\int_Q^p \mathbf{H} \cdot d \mathbf{l} >0\), So, \(\int_P^Q \mathbf{H} \cdot d \mathbf{l}<0\)
\(P \rightarrow Q\) is inside the bar.
Hence \(\mathbf{H}\) is making an obtuse angle with \(d l\) (meaning that the lines run from N pole to S pole inside the bar magnet).

LA (Long Answer Type)

Q5.21. Verify the Ampere’s law for magnetic field of a point dipole of dipole moment \(\mathbf{m}=m \hat{\mathbf{k}}\). Take C as the closed curve running clockwise along (i) the \(z\)-axis from \(z=a>0\) to \(z=R\); (ii) along the quarter circle of radius \(R\) and centre at the origin, in the first quadrant of \(x-z\) plane; (iii) along the \(x\)-axis from \(x=R\) to \(x=a\), and (iv) along the quarter circle of radius \(a\) and centre at the origin in the first quadrant of \(x-z\) plane.

Answer: Assume the \(x-z\) plane (shown below). All points from \(P\) to \(Q\) lie on the axial line NS placed at the origin.

The magnetic field at a distance \(r\) is
\(
B=\frac{\mu_0 2|M|}{4 \pi r^3}=\frac{\mu_0 M}{2 \pi r^3}
\)
Along the \(z\)-axis from \(P\) to \(Q\)
\(
\int_P^Q B \cdot d l=\int_P^Q B \cdot d l \cos 0^{\circ}=\int_a^R B d z
\)
\(
=\int_a^R \frac{\mu_0 M}{2 \pi r^3} d z=\frac{\mu_0 M}{2 \pi}\left(-\frac{1}{-2}\right)\left(\frac{1}{R^2}-\frac{1}{a^2}\right)=\frac{\mu_0 M}{4 \pi}\left(\frac{1}{a^2}-\frac{1}{R^2}\right)
\)

(ii) Along the quarter circle QS (radius R)

Consider point A to lie on the equatorial line of the magnetic dipole of moment \(\mathrm{M} \sin \theta\).
The magnetic field at A is
\(
\begin{aligned}
& B_t= \frac{\frac{\mu_0}{4 \pi} M \sin \theta}{R^3} ; d l=R d \theta \\
& B_r= \frac{\frac{\mu_0}{2 \pi} m \cos \theta}{R^3} \\
& \int_0^{\frac{\pi}{2}} B \cdot d l=\int_0^{\frac{\pi}{2}} B_t d l \cos 0^{\circ}+\int_0^{\frac{\pi}{2}} B_r d l \cos 90^{\circ}=\int_0^{\frac{\pi}{2}} \frac{\mu_0 m}{4 \pi R^3} \sin \theta(R d \theta)= \\
& \frac{\mu_0 m}{4 \pi R^2} \int_0^{\frac{\pi}{2}} \sin \theta d \theta=\frac{\mu_0 m}{4 \pi R^2}
\end{aligned}
\)

(iii) Along the \(x\)-axis over the path ST, consider the figure given below.

From the figure, every point lies on the equatorial line of the magnetic dipole.
Magnetic field induction at a point distance \(x\) from the dipole is
\(
B=\frac{\frac{\mu_0}{4 \pi} M}{x^3}
\)
\(\int_S^T B \cdot d l=\int_R^a-\frac{\mu_0 M}{4 \pi x^3}=0\), the angle between M and dl is \(90^{\circ}\)

(iv) Along the quarter circle TP of radius a.
Let’s consider the figure given below.

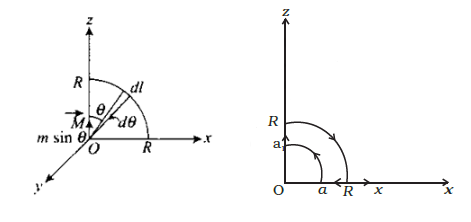
From case ii we get the line integral of \(B\) along the quarter circle \(T P\) of radius \(a\) is circular arc TP?
\(
\begin{aligned}
& \int B \cdot d l=\int_{\frac{\pi}{2}}^0 \frac{\mu_0}{4 \pi} M \frac{\sin \theta}{a^3} a d \theta=\frac{\mu_0 M}{4 \pi a^2} \int_{\frac{\pi}{2}}^0 \sin \theta d \theta=\frac{\frac{\mu_0}{4 \pi} M}{a^2} \int_{\frac{\pi}{2}}^0[-\cos \theta] \\
& =-\frac{\frac{\mu_0}{4 \pi} M}{a^2}
\end{aligned}
\)
\(
\int B . d l=\int_P^Q B . d l+\int_Q^S B . d l+\int_S^T B . d l+\int_T^P B . d l
\)
\(
=\frac{\mu_0 M}{4}\left[\frac{1}{a^2}-\frac{1}{R^2}\right]+\frac{\mu_0 M}{4 \pi R^2}+0+\left(-\frac{\mu_0 M}{4 \pi R^2}\right)=0
\)

Q5.22. What are the dimensions of \(\chi\), the magnetic susceptibility? Consider an H-atom. Guess an expression for \(\chi\), upto a constant by constructing a quantity of dimensions of \(\chi\), out of parameters of the atom: \(e, m, v, R\) and \(\mu_0\). Here, \(m\) is the electronic mass, \(v\) is the electronic velocity, \(R\) is Bohr radius. Estimate the number so obtained and compare with the value of \(|\chi| \sim 10^{-5}\) for many solid materials.

Answer: \(\text { Magnetic susceptibility, } \chi_m=\frac{I}{H} \frac{\text { (intensity of magnetisation) }}{\text { (magnetising force) }}\)
As land H have same units and dimensions, hence \(\chi\) has no dimensions.
In this question, \(\chi\) is to be related with e, \(\mathrm{m}, \mathrm{v}, \mathrm{R}\) and \(\mu_0\). We know that dimensions of \(\mu_0=\left[M L Q^{-2}\right]\)
[From Biot Savart’s law, \(d B=\frac{\mu_0}{4 \pi} \frac{I d l \sin \theta}{r^2}\)
or \(\mu_0=\frac{4 \pi r^2 d B}{I d l \sin \theta}=\frac{4 \pi r^2}{I d l \sin \theta} \times \frac{F}{q v \sin \theta^{\prime}}\left[\because d B=\frac{F}{q v \sin \theta^{\prime}}\right]\)
\(\therefore\) Dimensions of
\(
\begin{aligned}
\mu_0 & =\frac{L^2 \times\left(M L T^{-2}\right)}{\left(Q T^{-1}\right)(L) \times 1 \times(Q)\left(L T^{-1}\right) \times(1)} \\
& =\left[M L Q^{-2}\right]
\end{aligned}
\)
where \(Q\) is for charge.
\(\chi\) is dimensionless.
\(\chi\) depends on magnetic moment induced when \(H\) is turned on. \(H\) couples to atomic electrons through its charge \(e\). The effect on \(m\) is via current \(I\) which involves another factor of ‘ \(e\) ‘. The combination ” \(\mu_0 e^{2 }\)” does not depend on the “charge” Q dimension.
Let \(\chi=\mu_0 e^2 m^a v^b R^c \ldots\) (i)
where \(a, b, c\) are the powers of \(m, v\) and \(R\) such that relation (i) is satisfied. Dimensional equation of (i) is
\(
\begin{aligned}
& M^0 L^0 T^0 Q^0=\left(M^1 L^1 Q^{-2}\right) \times\left(Q^2\right)\left(M^a\right) \\
& \times\left(L T^{-1}\right)^b \times(L)^c \\
& =M^{1+a} L^{1+b+c} T^{-b} Q^0
\end{aligned}
\)
\(
\begin{aligned}
&\text { Equating the powers of } \mathrm{M}, \mathrm{~L} \text { and } \mathrm{T} \text {, we get }\\
&\begin{aligned}
& 0=1+a \text { or } a=-1,0=1+b+c \ldots \text { (ii) } \\
& 0=-b \text { 아 } b=0, \text { From (ii), } 0=1+0+c \text { or } c=-1
\end{aligned}
\end{aligned}
\)
\(
\begin{aligned}
&\text { Putting values in (i), we get }\\
&\chi=\mu_0 e^2 m^{-1} v^0 R^{-1}=\frac{\mu_0 e^2}{m R} \ldots(iii)
\end{aligned}
\)
\(
\text { Here, } \mu_0=4 \pi \times 10^{-7} T m A^{-1}, e=1.6 \times 10^{-19} C \text {, }
\)
\(
\begin{aligned}
& m=9 \cdot 1 \times 10^{-31} \mathrm{~kg}, R=10^{-10} \mathrm{~m} \\
& \therefore \chi=\frac{\left(4 \pi \times 10^{-7}\right) \times\left(1 \cdot 6 \times 10^{-19}\right)^2}{\left(9 \cdot 1 \times 10^{-31}\right) \times 10^{-10}} \approx 10^{-4} \\
& \therefore \frac{\chi}{\chi_{(\text {givensol id })}}=\frac{10^{-4}}{10^{-5}}=10
\end{aligned}
\)

Q5.23: There are two current carrying planar coils made each from identical wires of length \(L . \mathrm{C}_1\) is circular (radius \(R\) ) and \(\mathrm{C}_2\) is square (side \(a\) ). They are so constructed that they have same frequency of oscillation when they are placed in the same uniform \(\mathbf{B}\) and carry the same current. Find \(a\) in terms of \(R\).

Answer: 

\(
\begin{aligned}
&\text { For circular coil } C_1, n_1=\frac{L}{2 \pi R} \text {, Magnetic moment, }\\
&M_1=n_1 i A_1=\frac{L}{2 \pi R} \times i \times \pi R^2=\frac{L i R}{2}
\end{aligned}
\)
\(
\begin{aligned}
&\text { For square coil } C_2, n_2=\frac{L}{4 a} \text {, Magnetic moment, }\\
&M_2=n_2 i A_2=\frac{L}{4 a} \times i \times a^2=\frac{L i a}{4}
\end{aligned}
\)
Moment of inertia of circular coil about the diameter as axis,
\(
I_1=\frac{\text { mass } \times(\text { radius })^2}{2}=\frac{m R^2}{2}
\)
Moment of inertia of square coil about an axis passing through its centre parallel to breadth
\(
I_2=\frac{m a^2}{12}
\)
Time period of oscillation of the magnet in magnetic field is given by
\(
\begin{aligned}
& T=2 \pi \frac{\sqrt{I}}{M B} \text { and } \omega=\frac{2 \pi}{T}=\sqrt{\frac{M B}{I}} \\
& \therefore \omega_1^2=\frac{M_1 B}{I_1} \text { and } \omega_2^2=\frac{M_2 B}{I_2}
\end{aligned}
\)
Given, \(\omega_1^2=\omega_2^2\), so \(\frac{M_1}{I_1}=\frac{M_2}{I_2}\) or \(\frac{L I R / 2}{m R^2 / 2}=\frac{L I a / 4}{m a^2 / 12}\)
On solving, \(a=3 R\)