NCERT Exercise Q & A

Exercise Problems

Q3.1: The storage battery of a car has an emf of 12 V . If the internal resistance of the battery is \(0.4 \Omega\), what is the maximum current that can be drawn from the battery?

Answer: Emf of the battery, \(E=12 \mathrm{~V}\)
Internal resistance of the battery, \(r=0.4 \Omega\)
Maximum current drawn from the battery \(=I\)
According the Ohm’s law,
\(
\begin{aligned}
& E=I r \\
& I=\frac{E}{r} \\
& =\frac{12}{0.4}=30 \mathrm{~A}
\end{aligned}
\)
The maximum current drawn from the given battery is 30 A.

Q3.2: A battery of emf 10 V and internal resistance \(3 \Omega\) is connected to a resistor. If the current in the circuit is 0.5 A , what is the resistance of the resistor? What is the terminal voltage of the battery when the circuit is closed?

Answer: Emf of the battery, \(E=10 \mathrm{~V}\)
Internal resistance of the battery, \(r=3 \Omega\)
Current in the circuit, \(I=0.5 \mathrm{~A}\)
Resistance of the resistor \(=R\)
The relation for current using Ohm’s law is,
\(
\begin{aligned}
& I=\frac{E}{R+r} \\
& R+r=\frac{R}{I} \\
& =\frac{10}{0.5}=20 \Omega \\
& \therefore R=20-3=17 \Omega
\end{aligned}
\)
Terminal voltage of the resistor \(=\mathrm{V}\)
According to Ohm’s law,
\(
\begin{aligned}
& V=I R \\
& =0.5 \times 17 \\
& =8.5 \mathrm{~V}
\end{aligned}
\)
Therefore, the resistance of r the resistor is \(17 \Omega\) and the terminal voltage is 8.5 V.

Q3.3: At room temperature \(\left(27.0^{\circ} \mathrm{C}\right)\) the resistance of a heating element is \(100 \Omega\). What is the temperature of the element if the resistance is found to be \(117 \Omega\), given that the temperature coefficient of the material of the resistor is \(1.70 \times 10^{-4}{ }^{\circ} \mathrm{C}^{-1}\).

Answer: To solve the problem, we will use the formula for the resistance of a conductor as a function of temperature:
\(
R_t^{\prime}=R_t \times(1+\alpha \Delta T)
\)
Where:
\(R_t^{\prime}\) is the resistance at the new temperature ( \(117 \Omega\) )
\(R_t\) is the resistance at the reference temperature ( \(100 \Omega\) )
\(\alpha\) is the temperature coefficient of resistance \(=1.70 \times 10^{-4}{ }^{\circ} \mathrm{C}^{-1}\)
\(\Delta T\) is the change in temperature, which can be expressed as \(T^{\prime}-T\) (where \(T^{\prime}\) is the new temperature and \(T\) is the reference temperature, \(27^{\circ} \mathrm{C}\) )
\(
\begin{aligned}
& R_t=100 \Omega \\
& R_t^{\prime}=117 \Omega \\
& T=27^{\circ} \mathrm{C} \\
& \alpha=1.70 \times 10^{-4}{ }^{\circ} \mathrm{C}^{-1}
\end{aligned}
\)
\(
\begin{aligned}
&R_t^{\prime}=R_t \times(1+\alpha \Delta T)\\
&\text { Substituting the known values: }\\
&117=100 \times\left(1+1.70 \times 10^{-4} \times\left(T^{\prime}-27\right)\right)
\end{aligned}
\)
Solving the above equation we get,
\(
T^{\prime}-27=\frac{0.17}{1.70 \times 10^{-4}}
\)
\(
T^{\prime}=1000+27=1027^{\circ} \mathrm{C}
\)
The temperature of the heating element when the resistance is found to be \(117 \Omega\) is \(1027^{\circ} \mathrm{C}\).

Q3.4: A negligibly small current is passed through a wire of length 15 m and uniform cross-section \(6.0 \times 10^{-7} \mathrm{~m}^2\), and its resistance is measured to be \(5.0 \Omega\). What is the resistivity of the material at the temperature of the experiment?

Answer: Length of the wire, \(l=15 \mathrm{~m}\)
Area of cross-section of the wire, \(a=6.0 \times 10^{-7} \mathrm{~m}^2\)
Resistance of the material of the wire, \(R=5.0 \Omega\)
Resistivity of the material of the wire \(=\rho\)
Resistance is related with the resistivity as
\(
\begin{aligned}
R & =\rho \frac{l}{A} \\
\rho & =\frac{R A}{l} \\
& =\frac{5 \times 6 \times 10^{-7}}{15}=2 \times 10^{-7} \Omega \mathrm{~m}
\end{aligned}
\)
Therefore, the resistivity of the material is \(2 \times 10^{-7} \Omega \mathrm{~m}\).

Q3.5: A silver wire has a resistance of \(2.1 \Omega\) at \(27.5^{\circ} \mathrm{C}\), and a resistance of \(2.7 \Omega\) at \(100^{\circ} \mathrm{C}\). Determine the temperature coefficient of resistivity of silver.

Answer: Temperature, \(T_1=27.5^{\circ} \mathrm{C}\)
Resistance of the silver wire at \(T_1, R_1=2.1 \Omega\)
Temperature, \(T_2=100^{\circ} \mathrm{C}\)
Resistance of the silver wire at \(T_2, R_2=2.7 \Omega\)
Temperature coefficient of silver \(=a\)
It is related with temperature and resistance as
\(
\begin{aligned}
\alpha & =\frac{R_2-R_1}{R_1\left(T_2-T_1\right)} \\
& =\frac{2.7-2.1}{2.1(100-27.5)}=0.0039^{\circ} \mathrm{C}^{-1}
\end{aligned}
\)
Therefore, the temperature coefficient of silver is \(0.0039^{\circ} \mathrm{C}^{-1}\).

Q3.6: A heating element using nichrome connected to a 230 V supply draws an initial current of 3.2 A which settles after a few seconds to a steady value of 2.8 A . What is the steady temperature of the heating element if the room temperature is \(27.0^{\circ} \mathrm{C}\) ? Temperature coefficient of resistance of nichrome averaged over the temperature range involved is \(1.70 \times 10^{-4}{ }^{\circ} \mathrm{C}^{-1}\).

Answer: Supply voltage, \(V=230 \mathrm{~V}\)
Initial current drawn, \(I_1=3.2 \mathrm{~A}\)
Initial resistance \(=R_1\), which is given by the relation,
\(
\begin{aligned}
R_1 & =\frac{V}{I} \\
& =\frac{230}{3.2}=71.87 \Omega
\end{aligned}
\)
Steady state value of the current, \(I_2=2.8 \mathrm{~A}\)
Resistance at the steady state \(=R_2\), which is given as
\(
R_2=\frac{230}{2.8}=82.14 \Omega
\)
Temperature co-efficient of nichrome, \(a=1.70 \times 10^{-4}{ }^{\circ} \mathrm{C}^{-1}\)
Initial temperature of nichrome, \(T_1=27.0^{\circ} \mathrm{C}\)
Study state temperature reached by nichrome \(=T_2\)
\(T_2\) can be obtained by the relation for \(a\),
\(
\begin{aligned}
& \alpha=\frac{R_2-R_1}{R_1\left(T_2-T_1\right)} \\
& T_2-27^{\circ} \mathrm{C}=\frac{82.14-71.87}{71.87 \times 1.7 \times 10^{-4}}=840.5 \\
& T_2=840.5+27=867.5^{\circ} \mathrm{C}
\end{aligned}
\)
Therefore, the steady temperature of the heating element is \(867.5^{\circ} \mathrm{C}\)

Q3.7: Determine the current in each branch of the network shown in Fig. 3.20:

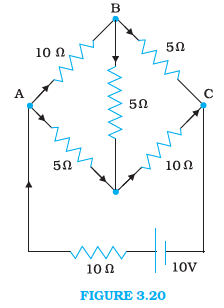
Answer: 

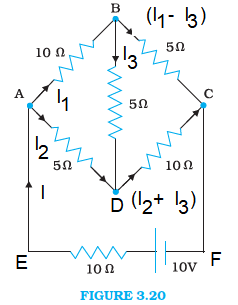
where \(I_1, I_2\) and \(I_3\) are the different currents through shown branches.
Now, Applying KVL in the Loop,
\(
10-I 10-I_2 5-\left(I_2+I_3\right) 10=0
\)
Also, we have \(I=I_1+I_2\)
so putting it in the KVL equation, we get
\(
\begin{aligned}
& 10-\left(I_1+I_2\right) 10-I_2 5-\left(I_2+I_3\right) 10=0 \\
& 10-10 I_1-10 I_2-5 I_2-10 I_2-10 I_3=0 \\
& 10-10 I_1-25 I_2-10 I_3=0 \dots(1)
\end{aligned}
\)
Now let’s apply KVL in the loop involving \(I_1, I_2\) and \(I_3\),
\(
5 I_2-10 I_1-5 I_3=0 \dots(2)
\)
now, the third equation of KVL,
\(
\begin{aligned}
& 5 I_3-5\left(I_1-I_3\right)+10\left(I_2+I_3\right)=0 \\
& -5 I_1+10 I_2+20 I_3=0 \dots(3)
\end{aligned}
\)
Now we have 3 equations and 3 variables, on solving we get,
\(
\begin{aligned}
& I_1=\frac{4}{17} A \\
& I_2=\frac{6}{17} A \\
& I_3=\frac{-2}{17} A
\end{aligned}
\)
And, the total Current in the circuit is,
\(
I=I_1+I_2=\frac{4}{17}+\frac{6}{17}=\frac{10}{17} \mathrm{Amp}
\)

Q3.8: A storage battery of emf 8.0 V and internal resistance \(0.5 \Omega\) is being charged by a 120 V dc supply using a series resistor of \(15.5 \Omega\). What is the terminal voltage of the battery during charging? What is the purpose of having a series resistor in the charging circuit?

Answer: Given,
EMF of the storage battery, \(\mathrm{E}=8 \mathrm{~V}\)
Internal resistance, \(\mathrm{r}=0.5 \Omega\)
D.C supply voltage, \(\mathrm{V}=120 \mathrm{~V}\)
Resistance of the resistor i.e, external resistance, \(R=15.5 \Omega\)
Using the formula, \(I=\frac{V-E}{R+r}\)
Therefore,
Charging current, \(I=\frac{120-8}{15.5+0.5}\)
\(
=\frac{112}{16}=7 \mathrm{~A}
\)
Terminal voltage, \(\mathrm{V}=\mathrm{E}+1 \mathrm{r}\)
\(
\begin{aligned}
& =8+7 \times 0.5 \\
& =11.5 \mathrm{~V}
\end{aligned}
\)
The series resistor limits the current from the external source. The flow of current may be drastically high in the absence of series resistor which is dangerous.

Q3.9: The number density of free electrons in a copper conductor estimated in Example 3.1 is \(8.5 \times 10^{28} \mathrm{~m}^{-3}\). How long does an electron take to drift from one end of a wire 3.0 m long to its other end? The area of cross-section of the wire is \(2.0 \times 10^{-6} \mathrm{~m}^2\) and it is carrying a current of 3.0 A .

Answer: Number density of free electrons, \(n=8.5 \times 10^{28} \mathrm{~m}^{-3}\) Length of the wire, \(L=3.0 \mathrm{~m}\)
Area of cross-section of the wire, \(A=2.0 \times 10^{-6} \mathrm{~m}^2\)
Current flowing through the wire, \(I=3.0 \mathrm{~A}\)
The current \(I\) in a conductor can be expressed as:
\(
I=n \cdot e \cdot A \cdot v_d
\)
where:
\(e\) is the charge of an electron, approximately \(1.6 \times 10^{-19} \mathrm{C}\)
\(v_d\) is the drift velocity of the electrons.
\(
v_d=\frac{I}{n \cdot e \cdot A}
\)
\(
=\frac{3.0 \mathrm{~A}}{\left(8.5 \times 10^{28} \mathrm{~m}^{-3}\right) \cdot\left(1.6 \times 10^{-19} \mathrm{C}\right) \cdot\left(2.0 \times 10^{-6} \mathrm{~m}^2\right)}
\)
\(
v_d=\frac{3.0}{2.72 \times 10^4} \approx 1.10 \times 10^{-4} \mathrm{~m} / \mathrm{s}
\)
Now, we can calculate the time \(t\) taken for an electron to drift the length of the wire:
\(
t=\frac{L}{v_d}=\frac{3.0 \mathrm{~m}}{1.10 \times 10^{-4} \mathrm{~m} / \mathrm{s}} \approx 27272.73 \mathrm{~s}=2.7 \times 10^4 \mathrm{~s}(7.5 \mathrm{~h})
\)

Q3.10: (a) In a meter bridge [Fig. below], the balance point is found to be at 39.5 cm from the end \(A\), when the resistor S is of \(12.5 \Omega\). Determine the resistance of \(R\). Why are the connections between resistors in a Wheatstone or meter bridge made of thick copper strips?
(b) Determine the balance point of the bridge above if \(R\) and \(S\) are interchanged.
(c) What happens if the galvanometer and cell are interchanged at the balance point of the bridge? Would the galvanometer show any current?

Answer: (a) Balance point from end \(\mathrm{A}, l_1=39.5 \mathrm{~cm}\)
Resistance of the resistor \(Y=12.5 \Omega\)
Condition for the balance is given as,
\(
\begin{aligned}
& \frac{X}{Y}=\frac{100-l_1}{l_1} \\
& X=\frac{100-39.5}{39.5} \times 12.5=8.2 \Omega
\end{aligned}
\)
Therefore, the resistance of resistor \(X\) is \(8.2 \Omega\).
The connection between resistors in a Wheatstone or metre bridge is made of thick copper strips to minimize the resistance, which is not taken into consideration in the bridge formula.
(b) If \(X\) and \(Y\) are interchanged, then \(l_1\) and \(100-l_1\) get interchanged.
The balance point of the bridge will be \(100-l_1\) from A.
\(
100-l_1=100-39.5=60.5 \mathrm{~cm}
\)
Therefore, the balance point is 60.5 cm from A.
(c) When the galvanometer and cell are interchanged at the balance point of the bridge, the galvanometer will show no deflection. Hence, no current would flow through the galvanometer.

Q3.11: The earth’s surface has a negative surface charge density of \(10^{-9} \mathrm{C}\) \(\mathrm{m}^{-2}\). The potential difference of 400 kV between the top of the atmosphere and the surface results (due to the low conductivity of the lower atmosphere) in a current of only 1800 A over the entire globe. If there were no mechanism of sustaining atmospheric electric fleld, how much time (roughly) would be required to neutrallse the earth’s surface? (This never happens in practice because there is a mechanism to replenish electric charges, namely the continual thunderstorms and lightning in different parts of the globe). (Radius of earth \(=6.37 \times 10^6 \mathrm{~m}\).)

Answer: Surface charge density of the earth, \(\sigma=10^{-9} \mathrm{C} \mathrm{m}^{-2}\)
Current over the entire globe, \(I=1800 \mathrm{~A}\)
Radius of the earth, \(r=6.37 \times 10^6 \mathrm{~m}\)
Surface area of the earth,
\(
\begin{aligned}
& A=4 \pi r^2 \\
& =4 \pi \times\left(6.37 \times 10^6\right)^2 \\
& =5.09 \times 10^{14} \mathrm{~m}^2
\end{aligned}
\)
Charge on the earth surface,
\(
\begin{aligned}
& q=\sigma \times A \\
& =10^{-9} \times 5.09 \times 10^{14} \\
& =5.09 \times 10^5 \mathrm{C}
\end{aligned}
\)
Time taken to neutralize the earth’s surface \(=t\)
Current, \(I=\frac{q}{t}\)
\(
\begin{aligned}
t & =\frac{q}{I} \\
& =\frac{5.09 \times 10^5}{1800}=282.77 \mathrm{~s}
\end{aligned}
\)
Therefore, the time taken to neutralize the earth’s surface is 282.77 s.

Q3.12: (a) Stx lead-acid type of secondary cells each of emf 2.0 V and internal resistance \(0.015 \Omega\) are joined in serles to provide a supply to a resistance of \(8.5 \Omega\). What are the current drawn from the supply and its terminal voltage?
(b) A secondary cell after long use has an emf of 1.9 V and a large internal resistance of \(380 \Omega\). What maximum current can be drawn from the cell? Could the cell drive the starting motor of a car?

Answer: (a) Number of secondary cells, \(n=6\)
Emf of each secondary cell, \(E=2.0 \mathrm{~V}\)
Internal resistance of each cell, \(r=0.015 \Omega\)
series resistor is connected to the combination of cells.
Resistance of the resistor, \(R=8.5 \Omega\)
Current drawn from the supply \(=I\), which is given by the relation,
\(
\begin{aligned}
I & =\frac{n E}{R+n r} \\
& =\frac{6 \times 2}{8.5+6 \times 0.015} \\
& =\frac{12}{8.59}=1.39 \mathrm{~A}
\end{aligned}
\)
Terminal voltage, \(V=I R=1.39 \times 8.5=11.87 \mathrm{~A}\)
Therefore, the current drawn from the supply is 1.39 A and terminal voltage is 11.87 A.
(b) After a long use, emf of the secondary cell, \(E=1.9 \mathrm{~V}\)
Internal resistance of the cell, \(r=380 \Omega\)
Hence, maximum current
\(
=\frac{E}{r}=\frac{1.9}{380}=0.005 \mathrm{~A}
\)
Therefore, the maximum current drawn from the cell is 0.005 A. Since a large current is required to start the motor of a car, the cell cannot be used to start a motor.

Q3.13: Two wires of equal length, one of aluminium and the other of copper have the same resistance. Which of the two wires is lighter? Hence explain why alumintum wires are preferred for overhead power cables. \(\left(\rho_{\mathrm{Al}}=2.63 \times 10^{-8} \Omega \mathrm{~m}, \quad \rho_{\mathrm{Cu}}=1.72 \times 10^{-8} \Omega \mathrm{~m}\right.\), Relative density of \(\mathrm{Al}=2.7\), of \(\mathrm{Cu}=8.9\).)

Answer: Resistivity of aluminium, \(\rho_{\mathrm{Al}}=2.63 \times 10^{-8} \Omega \mathrm{~m}\)
Relative density of aluminium, \(d_1=2.7\)
Let \(l_1\) be the length of aluminium wire and \(m_1\) be its mass.
Resistance of the aluminium wire \(=R_1\)
Area of cross-section of the aluminium wire \(=A_1\)
Resistivity of copper, \(\rho_{\mathrm{Cu}}=1.72 \times 10^{-8} \Omega \mathrm{~m}\)
Relative density of copper, \(d_2=8.9\)
Let \(l_2\) be the length of copper wire and \(m_2\) be its mass.
Resistance of the copper wire \(=R_2\)
Area of cross-section of the copper wire \(=A_2\)
The two relations can be written as
\(
\begin{aligned}
& R_1=\rho_1 \frac{l_1}{A_1} \dots(1)\\
& R_2=\rho_2 \frac{l_2}{A_2} \dots(2)
\end{aligned}
\)
And,
\(
\begin{aligned}
& l_1=l_2 \\
& \therefore \frac{\rho_1}{A_1}=\frac{\rho_2}{A_2} \\
& \frac{A_1}{A_2}=\frac{\rho_1}{\rho_2} \\
& \quad=\frac{2.63 \times 10^{-8}}{1.72 \times 10^{-8}}=\frac{2.63}{1.72}
\end{aligned}
\)
Mass of the aluminium wire,
\(m_1=\) Volume \(\times\) Density
\(
=A_1 l_1 \times d_1=A_1 l_1 d_1 \ldots(3)
\)
Mass of the copper wire,
\(
\begin{aligned}
& m_2=\text { volume } \times \text { Density } \\
& =A_2 l_2 \times d_2=A_2 l_2 d_2 \ldots(4)
\end{aligned}
\)
Dividing equation (3) by equation (4), we obtain
\(
\begin{aligned}
& \frac{m_1}{m_2}=\frac{A_1 l_1 d_1}{A_2 l_2 d_2} \\
& \text { For } l_1=l_2 \\
& \frac{m_1}{m_2}=\frac{A_1 d_1}{A_2 d_2}
\end{aligned}
\)
\(
\text { For } \frac{A_1}{A_2}=\frac{2.63}{1.72}
\)
\(
\frac{m_1}{m_2}=\frac{2.63}{1.72} \times \frac{2.7}{8.9}=0.46
\)
It can be inferred from this ratio that \(m_1\) is less than \(m_2\). Hence, aluminium is lighter than copper.
Since aluminium is lighter, it is preferred for overhead power cables over copper.

Q3.14: Answer the following questions:
(a) A steady current flows in a metallic conductor of non-untform cross-section. Which of these quantities is constant along the conductor: current, current density, electric fleld, drift speed?
(b) Is Ohm’s law untversally applicable for all conducting elements? If not, give examples of elements which do not obey Ohm’s law.
(c) A low voltage supply from which one needs high currents must have very low internal resistance. Why?
(d) A high tension (HT) supply of, say, 6 kV must have a very large internal resistance. Why?

Answer: (a) When a steady current flows in a metallic conductor of non-uniform cross-section, the current flowing through the conductor is constant. Current density, electric field, and drift speed are inversely proportional to the area of cross-section. Therefore, they are not constant.
(b) No, Ohm’s law is not universally applicable for all conducting elements. Vacuum diode semi-conductor is a non-ohmic conductor. Ohm’s law is not valid for it.
(c) According to Ohm’s law, the relation for the potential is \(V=I R\)
Voltage \((V)\) is directly proportional to current ( \(I\) ).
\(R\) is the internal resistance of the source.
\(
I=\frac{V}{R}
\)
If \(V\) is low, then \(R\) must be very low, so that high current can be drawn from the source.
(d) In order to prohibit the current from exceeding the safety limit, a high tension supply must have a very large internal resistance. If the internal resistance is not large, then the current drawn can exceed the safety limits in case of a short circuit.

Q3.15: (a) Given \(n\) resistors each of resistance \(R\), how will you combine them to get the (i) maximum (ii) minimum effective resistance? What is the ratio of the maximum to minimum resistance?
(b) Given the resistances of \(1 \Omega, 2 \Omega, 3 \Omega\), how will be combine them to get an equivalent resistance of (i) \((11 / 3) \Omega\) (ii) \((11 / 5) \Omega\), (iii) 6 \(\Omega\), (iv) (6/11) \(\Omega\) ?
(c) Determine the equivalent resistance of networks shown in Fig. 3.31.

Answer: (a) Total number of resistors \(=n\)
Resistance of each resistor \(=R\)
(i) When \(n\) resistors are connected in series, effective resistance \(R_1\) is the maximum, given by the product \(n R\).
Hence, maximum resistance of the combination, \(R_1=n R\)
(ii) When \(n\) resistors are connected in parallel, the effective resistance \(\left(R_2\right)\) is the minimum, given by the ratio \(\frac{R}{n}\).
Hence, minimum resistance of the combination, \(R_2=\frac{R}{n}\)
(iii) The ratio of the maximum to the minimum resistance is,
\(
\frac{R_1}{R_2}=\frac{n R}{\frac{R}{n}}=n^2
\)
(b) The resistance of the given resistors is,
\(
R_1=1 \Omega, R_2=2 \Omega, R_3=3 \Omega 2
\)
(i) Equivalent resistance
\(
R=\frac{2 \times 1}{2+1}+3=\frac{2}{3}+3=\frac{11}{3} \Omega
\)
(ii) Equivalent resistance of the circuit is given by,
\(
R=\frac{2 \times 3}{2+3}+1=\frac{6}{5}+1=\frac{11}{5} \Omega
\)
(iii) Equivalent resistance of the circuit is given by the sum,
\(
R^{\prime}=1+2+3=6 \Omega
\)
(iv) Equivalent resistance of the circuit is given by,
\(
R=\frac{1 \times 2 \times 3}{1 \times+2 \times 3+3 \times 1}=\frac{6}{11} \Omega
\)
(c)
(a) equivalent resistance of the given circuit is \(\frac{4}{3} \times 4=\frac{16}{3} \Omega\)
(b) equivalent resistance of the circuit \(=R+R+R+R+R=5R\)

Q3.16: Determine the current drawn from a 12 V supply with internal resistance \(0.5 \Omega\) by the infinite network shown in Fig. below. Each resistor has \(1 \Omega\) resistance.

Answer: The resistance of each resistor connected in the given circuit, \(R=1 \Omega\)
Equivalent resistance of the given circuit \(=R^{\prime}\)
The network is infinite. Hence, equivalent resistance is qiven by the relation,
\(
\begin{aligned}
& \therefore R^{\prime}=2+\frac{R^{\prime}}{\left(R^{\prime}+1\right)} \\
& \left(R^{\prime}\right)^2-2 R^{\prime}-2=0 \\
& R^{\prime}=\frac{2 \pm \sqrt{4+8}}{2} \\
& \quad=\frac{2 \pm \sqrt{12}}{2}=1 \pm \sqrt{3}
\end{aligned}
\)
Negative value of \(R^{\prime}\) cannot be accepted. Hence, equivalent resistance,
\(
R^{\prime}=(1+\sqrt{3})=1+1.73=2.73 \Omega
\)
Internal resistance of the circuit, \(r=0.5 \Omega\)
Hence, total resistance of the given circuit \(=2.73+0.5=3.23 \Omega\)
Supply voltage, \(V=12 \mathrm{~V}\)
According to Ohm’s Law, current drawn from the source is given by the ratio, \(\frac{12}{3.23}=3.72 \mathrm{~A}\)

Exemplar Problems and Solutions

(VSA)

Q3.12: Is the momentum conserved when charge crosses a junction in an electric circuit? Why or why not?

Answer: In the circuit when an electron approaches a junction, in addition to the uniform E that faces it normally (which keep the drift velocity fixed), as drift velocity \(\left(\mathrm{v}_{\mathrm{d}}\right)\) is directly proportional to Electric field (E). That’s why there are accumulation of charges on the surface of wires at the junction. These produce additional electric fields. These fields alter the direction of momentum. Thus, the motion of a charge across junction is not momentum conserving.

Q3.13: The relaxation time \(\tau\) is nearly independent of applied \(E\) field whereas it changes significantly with temperature \(T\). First fact is (in part) responsible for Ohm’s law whereas the second fact leads to variation of \(\rho\) with temperature. Elaborate why?

Answer: Key concept: Time interval between two successive collisions of electron with positive ions in the metallic lattice is defined as relaxation time \(\tau=\frac{\text { mean free path }}{\text { r.m.s. velocity of electrons }}=\frac{\lambda}{v_{\mathrm{rms}}}\) with rise in temperature \(\mathrm{v}_{\mathrm{rms}}\) increase consequently as \(\tau\) decreases.
The drift velocity of the electrons is small because of the frequent collisions suffered by electrons.
Relaxation time is inversely proportional to the velocities of electrons and ions. The applied electric field produces the insignificant change in velocities of electrons at the order of \(1 \mathrm{~mm} / \mathrm{s}\), whereas the change in temperature \((T)\) affects velocities at the order of \(10^2 \mathrm{~m} / \mathrm{s}\).
This decreases the relaxation time considerably in metals and consequently resistivity of metal or conductor increases as
\(
\rho=\frac{1}{\sigma}=\frac{m}{n e^2 \tau}
\)

Q3.14: What are the advantages of the null-point method in a Wheatstone bridge? What additional measurements would be required to calculate \(R_{\text{unknown}}\) by any other method?

Answer: In a Wheatstone bridge the main advantage of null point method is that the resistance of galvanometer does not affect the balance point, there is no need to determine current in resistances and the internal resistance of a galvanometer. It is convenient and easy method for observer.
The \(R_{\text{unknown}}\) can calculated applying Kirchhoff’s rules to the circuit. We would need additional accurate measurement of all the currents in resistances and galvanometer and internal resistance of the galvanometer.
Important point: The necessary and sufficient condition for balanced Wheatstone bridge is \(P / Q=R / S\)
where \(P\) and \(Q\) are ratio arms and \(R\) is known resistance and \(S\) is unknown resistance.

Q3.15: For wiring in the home, one uses Cu wires or Al wires. What considerations are involved in this?

Answer: For the selection of metal for wiring in home the main criterion are: the availability, conductivity and the cost of the metal.
The Cu wires or Al wires are used for wiring in the home. The main considerations are involved in this process are cost of metal and good conductivity of metal.

Q3.16: Why are alloys used for making standard resistance coils?

Answer: Alloys are used for making standard resistance coil because they have low temperature coefficient of resistance with less temperature sensitivity.This keeps the resistance of the wire almost constant even in small temperature change. The alloys also have high resistivity and hence high resistance, because for given length and cross-section area of conductor ( L and A are constant)[Note: \(R=\frac{\rho L}{A} \)]

Q3.17: Power \(P\) is to be delivered to a device via transmission cables having resistance \(R_c\). If \(V\) is the voltage across \(R\) and \(I\) the current through it, find the power wasted and how can it be reduced.

Answer: The power consumed in transmisssion lines is given by \(P=i^2 R_c\), where \(R_c\) is the resistance of connecting cables. The power is given by
\(
P=V I
\)
There are two ways to transmit the given power (i) at low voltage and high current or (ii) high voltage and low current. In power transmission at low voltage and high current more power is consumed as \(P \propto i^2\) whereas power transmission at high voltage and low current facilitates the power transmission with minimal power consumption. The power wastage can be reduced by transmitting power at high voltage.

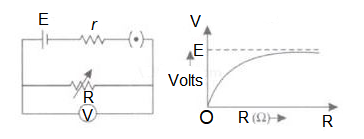
Q3.18: A cell of emf \(E\) and internal resistance \(r\) is connected across an external resistance \(R\). Plot a graph showing the variation of P.D. across R , verses \(R\).

Answer:

\(
\begin{aligned}
&\text { Ans. We know that } I=\frac{E}{R+r} \text { and } \mathrm{V}=\mathrm{IR} \text {. }\\
&\begin{aligned}
& \text { So } V=\frac{E R}{R+r} \dots(I)\\
& V=\frac{E}{1+\frac{r}{R}} \dots(II)
\end{aligned}
\end{aligned}
\)
Here \(E\), \(r\) are constants. So
\(
V \propto \frac{1}{1+\frac{r}{R}} (\text { from II })
\)
and \(V \propto R (\text { from I })\)
With increase in R, P.D. across R is increased upto maximum value E.

Short Questions Answer (SA)

Q.3.22: First a set of \(n\) equal resistors of \(R\) each are connected in series to a battery of emf \(E\) and internal resistance \(R\). A current \(I\) is observed to flow. Then the \(n\) resistors are connected in parallel to the same battery. It is observed that the current is increased 10 times. What is ” \(n\) “?

Answer: In series combination of resistors, current \(I\) is given by \(I=\frac{E}{R+n R}\) whereas in parallel combination current \(10 I\) is given by
\(
\frac{E^B}{R+\frac{R}{n}}=10 I
\)
Now, according to problem,
\(
\begin{aligned}
& \frac{1+n}{1+\frac{1}{n}} \Rightarrow 10=\left(\frac{1+n}{n+1}\right) n \\
\Rightarrow & n=10
\end{aligned}
\)

Q3.23: Let there be \(n\) resistors \(R_1 \ldots \ldots \ldots \ldots . R_{\mathrm{n}}\) with \(R_{\max }=\max \left(R_1 \ldots \ldots \ldots R_{\mathrm{n}}\right)\) and \(\mathrm{R}_{\min }=\min \left\{R_1 \ldots \ldots R_{\mathrm{n}}\right\}\). Show that when they are connected in parallel, the resultant resistance \(R_{\mathrm{p}}<R_{\min }\) and when they are connected in series, the resultant resistance \(R_{\mathrm{s}}>R_{\max }\). Interpret the result physically.

Answer: In parallel combination: When all resistances are connected in parallel, the equivalent resistance \(R_p\) is given by
\(
\frac{1}{R_p}=\frac{1}{R_1}+\ldots+\frac{1}{R_n}
\)
On multiplying both sides by \(R_{\min }\), we have
\(
\frac{R_{\min }}{R_p}=\frac{R_{\min }}{R_1}+\frac{R_{\min }}{R_2}+\ldots+\frac{R_{\min }}{R_n}
\)
Here, in RHS, there exist one term \(\frac{R_{\min }}{R_{\min }}=1\) and other terms are positive, so we have
\(
\frac{R_{\min }}{R_p}=\frac{R_{\min }}{R_1}+\frac{R_{\min }}{R_2}+\ldots+\frac{R_{\min }}{R_n}>1
\)
This shows that the resultant resistance \(R_p<R_{\text {min }}\).
Thus, in parallel combination, the equivalent resistance of resistors even less than the minimum resistance available in combination of resistors.
In series combination: When all resistances are connected in series, the equivalent resistance \(R_s\) is given by
\(
R_s=R_1+\ldots+R_n
\)
Here, in RHS, there exist one term having resistance \(R_{\max }\). So, we have
\(
\begin{aligned}
& R_s=R_1+\ldots+R_{\max } \ldots+\ldots+R_n \\
& R_s=R_1+\ldots+R_{\max } \ldots+R_n=R_{\max }+\ldots\left(R_1+\ldots+\right) R_n \\
& R_s \geq R_{\max } \\
& R_s=R_{\max }\left(R_1+6 E+R_n\right)
\end{aligned}
\)
Thus, in series combination, the equivalent resistance of resistors is greater than the maximum resistance available in combination of resistors.
Physical interpretation:

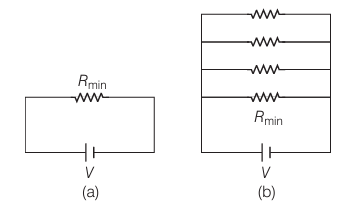
In Fig. (b), \(R_{\min }\) provides an equivalent route as in Fig. (a) for current. But in addition there are \((n-1)\) routes by the remaining \((n-1)\) resistors. Current in Fig. (b) is greater than current in Fig. (a). Effective resistance in Fig. (b) \(<R_{\min }\). Second circuit evidently affords a greater resistance.

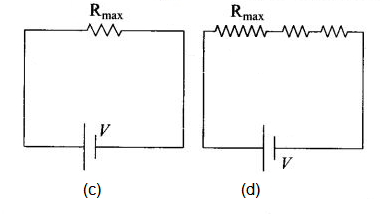
In Fig. (d), \(R_{\max }\) provides an equivalent route as in Fig. (c) for current. Current in Fig. (d) \(<\) current in Fig. (c). Effective resistance in Fig. (d) \(>R_{\max }\). Second circuit evidently affords a greater resistance.

Q3.24: The circuit in Fig below shows two cells connected in opposition to each other. Cell \(E_1\) is of emf 6 V and internal resistance \(2 \Omega\); the cell \(E_2\) is of emf 4 V and internal resistance \(8 \Omega\). Find the potential difference between the points A and B.

Answer: 

Applying Ohm’s law, Equivalent emf of two cells \(=6-4=2 \mathrm{~V}\) and equivalent resistance \(=2 \Omega+8 \Omega=10 \Omega\), so the electric current is given by
\(
I=\frac{6-4}{2+8}=0.2 \mathrm{~A}
\)
Taking loop in anti-clockwise direction, since \(E_1>E_2\)
The direction of flow of current is always from high potential to low potential.
Therefore \(V_B>V_A\).
\(
\begin{aligned}
& \Rightarrow \quad V_B-4 V-(0.2) \times=V_A \\
& \text { Therefore, } \quad V_B-V_A=3.6 \mathrm{~V}
\end{aligned}
\)
Important point: Sign convention for the application of Kirchoff’s law: For the application of Kirchoff’s laws following sign convention are to be considered.
(i) The change in potential in traversing a resistance in the direction of current is \(-i R\) while in the opposite direction \(+i R\).

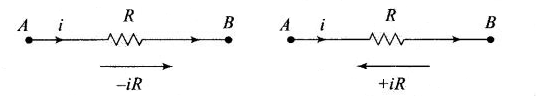
(ii) The change in potential in traversing an emf source from negative to positive terminal is \(+E\) while in the opposite direction – \(E\) irrespective of the direction of current in the circuit.

Q3.25: Two cells of same emf \(E\) but internal resistance \(r_1\) and \(r_2\) are connected in series to an external resistor \(R\) (Fig below). What should be the value of \(R\) so that the potential difference across the terminals of the first cell becomes zero.

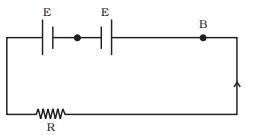
Answer: In this problem first we apply Ohm’s law to find current in the circuit.
Effective emf of two cells \(=E+E=2 E\)
Effective resistance \(=R+r_1+r_2\)
So the electric current is given by
\(
I=\frac{E+E}{R+r_1+r_2}
\)
\(
\mathrm{V}_1=\mathrm{E}-\mathrm{Ir}_1=E-\frac{2 E \cdot r_1}{R+r_1+r_2}
\)
The net potential difference across \(1^{\text {st }}\) cell \(\mathrm{V}_1=0\) (Given)
\(
E-\frac{2 E r_1}{R+r_1+r_2}=0
\)
\(
1-\frac{2 r_1}{R+r_1+r_2}=0
\)
\(
\begin{aligned}
& \frac{2 r_1}{r_1+r_2+R}=\frac{1}{1} \\
& \text { Or } 2 \mathrm{r}_1=\mathrm{r}_1+\mathrm{r}_2+\mathrm{R} \\
& r_1-r_2=R
\end{aligned}
\)
It is the required condition for the potential difference across Ist cell to be zero.

Q3.26: Two conductors are made of the same material and have the same length. Conductor A is a solid wire of diameter 1 mm . Conductor B is a hollow tube of outer diameter 2 mm and inner diameter 1 mm . Find the ratio of resistance \(R_{\mathrm{A}}\) to \(R_{\mathrm{B}}\).

Answer: Key concept: We know that the resistance of wire is \(R=\rho \frac{l}{A}\) where \(A\) is cross-sectional area of conductor, \(\rho\) is the specific resistance or resistivity and \(L\) is the length of conductor.
The resistance of first conductor
\(
R_A=\frac{\rho l}{\pi\left(10^{-3} \times 0.5\right)^2}
\)
The resistance of second conductor,
\(
R_B=\frac{\rho l}{\pi\left[\left(10^{-3}\right)^2-\left(0.5 \times 10^{-3}\right)^2\right]}
\)
Now, the ratio of two resistors is given by
\(
\frac{R_A}{R_B}=\frac{\left(10^{-3}\right)^2-\left(0.5 \times 10^{-3}\right)^2}{\left(0.5 \times 10^{-3}\right)^2}=3: 1
\)

Q3.27: Suppose there is a circuit consisting of only resistances and batteries and we have to double (or increase it to \(n\)-times) all voltages and all resistances. Show that currents are unaltered. Do this for circuit of Example 3.7 in the NCERT Text Book for Class XII.

Answer: Let us first assume the equivalent internal resistance of the battery is \(R_{\text {eff }}\), the equivalent external resistance \(R\) and the equivalent voltage of the battery is \(V_{\text {eff }}\)

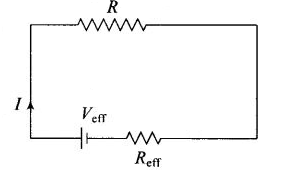
Now by applying Ohm’s law,
Then current through \(R\) is given by
\(
I=\frac{V_{\mathrm{eff}}}{R_{\mathrm{eff}}+R}
\)
Now according to the question if all the resistances and the effective voltage are increased \(n\)-times, then we have
\(
V_{\mathrm{eff}}^{\text {new }}=n V_{\mathrm{eff}}, R_{\mathrm{eff}}^{\text {new }}=n R_{\mathrm{eff}}
\)
\(
\text { and } R^{\text {new }}=n R
\)
Then, the new current is given by
\(
I^{\prime}=\frac{n V_{\mathrm{eff}}}{n R_{\mathrm{eff}}+n R}=\frac{n\left(V_{\mathrm{eff}}\right)}{n\left(R_{\mathrm{eff}}+R\right)}=\frac{\left(V_{\mathrm{eff}}\right)}{\left(\mathrm{R}_{\mathrm{eff}}+R\right)}=I
\)
The last result of two equations is same, so we can say that current remains the same.

Long Answer Questions (LA)

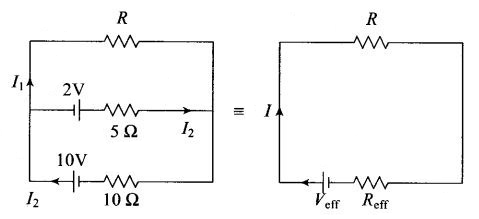
Q3.28: Two cells of voltage 10 V and 2 V and internal resistances \(10 \Omega\) and \(5 \Omega\) respectively, are connected in parallel with the positive end of 10 V battery connected to negative pole of 2 V battery (Fig below). Find the effective voltage and effective resistance of the combination.

Answer: Applying Kirchhoff’s junction rule, \(\quad I_1=I+I_2\)
Applying Kirchhoff’s II law / loop rule applied in outer loop containing 10 V cell and resistance \(R\), we have
\(
10=I R+10 I_1 \dots(i)
\)
Applying Kirchhoff II law / loop rule applied in outer loop containing 2 V cell and resistance \(R\), we have
\(
\begin{aligned}
2 & =5 I_2-R I=5\left(I_1-I\right)-R I \\
4 & =10 I_1-10 I-2 R I \dots(ii)
\end{aligned}
\)
Solving Eqs. (i) and (ii), gives
\(
\begin{aligned}
& 6=3 R I+10 I \\
& 2=I\left(R+\frac{10}{3}\right)
\end{aligned}
\)
Also, the external resistance is \(R\). The Ohm’s law states that
\(
V=I\left(R+R_{\text {eff }}\right)
\)
On comparing, we have \(V=2 V\) and effective internal resistance
\(
\left(R_{\text {eff }}\right)=\left(\frac{10}{3}\right) \Omega
\)
Since, the effective internal resistance \(\left(R_{\text {eff }}\right)\) of two cells is \(\left(\frac{10}{3}\right) \Omega\), being the parallel combination of \(5 \Omega\) and \(10 \Omega\). The equivalent circuit is given below

Q3.29: A room has \(A C\) run for 5 hours a day at a voltage of 220 V . The wiring of the room consists of Cu of 1 mm radius and a length of 10 m . Power consumption per day is 10 commercial units. What fraction of it goes in the joule heating in wires? What would happen if the wiring is made of aluminium of the same dimensions?
\(
\left[\rho_{\mathrm{cu}}=1.7 \times 10^{-8} \Omega \mathrm{~m}, \rho_{\mathrm{Al}}=2.7 \times 10^{-8} \Omega \mathrm{~m}\right]
\)

Answer: Key concept: The energy dissipated per unit time is the power dissipated \(P=\frac{\Delta W}{\Delta t}\) and,
The power across a resistor is \(P=I^2 R\)

Power consumption in a day, i.e., in \(5=10\) units Or power consumption per hour \(=2\) units Or power consumption \(=2\) units \(=2 \mathrm{~kW}=2000 \mathrm{~J} / \mathrm{s}\) Also, we know that power consumption in resistor,
\(
\begin{aligned}
& P=V \times I \\
\Rightarrow \quad & 2000 \mathrm{~W}=220 \mathrm{~V} \times I \text { or } I \approx 9 \mathrm{~A}
\end{aligned}
\)
Now, the resistance of wire with cross-sectional area \(A\) is given by \(R=\rho \frac{l}{A}\) Power consumption in first current carrying wire is given by
\(
\begin{aligned}
& P=I^2 R \\
& \rho \frac{l}{A} I^2=1.7 \times 10^{-8} \times \frac{10}{\pi \times 10^{-6}} \times 81 \mathrm{~J} / \mathrm{s} \approx 4 \mathrm{~J} / \mathrm{s}
\end{aligned}
\)
The fractional loss due to the joule heating in first wire \(=\frac{4}{2000} \times 100=0.2 \%\)
Power loss in Al wire \(= 4 \frac{\rho_{\mathrm{Al}}}{\rho_{\mathrm{Cu}}}=1.6 \times 4=6.4 \mathrm{~J} / \mathrm{s}\)
\(\begin{aligned} \text { The fractional loss due to the joule heating in second wire } & =\frac{6.4}{2000} \times 100 \\ & =0.32 \%\end{aligned}\)

Q3.30: (a) Consider circuit in Fig below. How much energy is absorbed by electrons from the initial state of no current (ignore thermal motion) to the state of drift velocity?
(b) Electrons give up energy at the rate of \(R I^2\) per second to the thermal energy. What time scale would one associate with energy in problem (a)? \(n=\) no of electron/volume \(=10^{29} / \mathrm{m}^3\), length of circuit \(=10 \mathrm{~cm}\), cross-section \(=\mathrm{A}=(1 \mathrm{~mm})^2\)

Answer:(a) Key concept: Relation between current and drift velocity is given by
\(
I=n e A v_d
\)
where \(v_d\) is the drift speed of electrons and \(n\) is the number density of electrons.
According to the Ohm’s law current in the circuit
\(
\begin{aligned}
& I=\frac{V}{R} \\
& I=6 \mathrm{~V} / 6 \Omega=1 \mathrm{~A}
\end{aligned}
\)
But, \(I=n e A v_d\)
or \(\quad v_d=\frac{I}{n e A}\)
On substituting the values,
For, \(n=\) number of electrons \(/\) volume \(=10^{29} / \mathrm{m}^3\) length of circuit \(=10 \mathrm{~cm}\), cross-section \(=A=(1 \mathrm{~mm})^2\)
\(
\begin{aligned}
v_d & =\frac{1}{10^{29} \times 16 \times 10^{-19} \times 10^{-6}} \\
& =\frac{1}{1.6} \times 10^{-4} \mathrm{~m} / \mathrm{s}
\end{aligned}
\)
Therefore, the energy absorbed in the form of KE is given by Total KE \(=\) KE of 1 electron \(\times\) no. of electrons
\(
\mathrm{KE}=\frac{1}{2} m_e v_d^2 \times n A I
\)
\(
=\frac{1}{2} \times 9.1 \times 10^{31} \times \frac{1}{2.56} \times 10^{-8} \times 10^{29} \times 10^{-6} \times 10^{-1}=2 \times 10^{-17} \mathrm{~J}
\)
(b) Ohmic loss (Power loss) is \(P=I^2 R=6 \times 1^2=6 \mathrm{~W}=6 \mathrm{~J} / \mathrm{s}\) Since, the energy dissipated per unit time is the power dissipated.
So, \(P=\frac{E}{t}\)
Therefore, \(E=P \times t\)
or \(\quad t=\frac{E}{P}=\frac{2 \times 10^{-17}}{6} \approx 10^{-17} \mathrm{~s}\)

Important point: The energy dissipated per unit time is the power dissipated \(P=\frac{\Delta W}{\Delta t}\) and, The power across a resistor is \(P=I^2 R\)