NCERT Exercise Q & A

Exercise Problems

Q11.1: Find the
(a) maximum frequency, and
(b) minimum wavelength of X-rays produced by 30 kV electrons.

Answer: Potential of the electrons, \(V=30 \mathrm{kV}=3 \times 10^4 \mathrm{~V}\)
Hence, the energy of the electrons, \(E=3 \times 10^4 \mathrm{eV}\)
Where,
\(e=\) Charge on an electron \(=1.6 \times 10^{-19} \mathrm{C}\)
(a)Maximum frequency produced by the \(X\)-rays \(=\nu\)
The energy of the electrons is given by the relation:
\(
E=h \nu
\)
Where,
\(
\begin{aligned}
& h=\text { Planck’s constant }=6.626 \times 10^{-34} \mathrm{Js} \\
& \begin{aligned}
\therefore \nu & =\frac{E}{h} \\
& =\frac{1.6 \times 10^{-19} \times 3 \times 10^4}{6.626 \times 10^{-34}}=7.24 \times 10^{18} \mathrm{~Hz}
\end{aligned}
\end{aligned}
\)
(b)The minimum wavelength produced by the \(X\)-rays is given as:
\(
\begin{aligned}
& \lambda=\frac{c}{\nu} \\
& =\frac{3 \times 10^8}{7.24 \times 10^{18}}=4.14 \times 10^{-11} \mathrm{~m}=0.0414 \mathrm{~nm}
\end{aligned}
\)
Hence, the minimum wavelength of \(X\)-rays produced is 0.0414 nm.
Hence, the maximum frequency of X-rays produced is \(7.24 \times 10^{18} \mathrm{~Hz}\).

Q11.2: The work function of caesium metal is 2.14 eV. When light of frequency \(6 \times 10^{14} \mathrm{~Hz}\) is incident on the metal surface, photoemission of electrons occurs. What is the
(a) maximum kinetic energy of the emitted electrons,
(b) Stopping potential, and
(c) maximum speed of the emitted photoelectron?

Answer: Work function of caesium metal, \(\phi_0=2.14 \mathrm{eV}\)
Frequency of light, \(\nu=6.0 \times 10^{14} \mathrm{~Hz}\)
(a)The maximum kinetic energy is given by the photoelectric effect as:
\(
K=h \nu-\phi_0
\)
Where,
\(
\begin{aligned}
& h=\text { Planck’s constant }=6.626 \times 10^{-34} \mathrm{Js} \\
& \begin{aligned}
\therefore K & =\frac{6.626 \times 10^{34} \times 6 \times 10^{14}}{1.6 \times 10^{-19}}-2.14 \\
& =2.485-2.140=0.345 \mathrm{eV}
\end{aligned}
\end{aligned}
\)
Hence, the maximum kinetic energy of the emitted electrons is 0.345 eV.
(b)For stopping potential \(V_0\), we can write the equation for kinetic energy as:
\(
\begin{aligned}
K & =e V_0 \\
\therefore V_0 & =\frac{K}{e} \\
& =\frac{0.345 \times 1.6 \times 10^{-19}}{1.6 \times 10^{-19}}=0.345 \mathrm{~V}
\end{aligned}
\)
Hence, the stopping potential of the material is 0.345 V.
(c) Maximum speed of the emitted photoelectrons \(=v\)
Hence, the relation for kinetic energy can be written as:
\(
K=\frac{1}{2} m v^2
\)
Where,
\(
m=\text { Mass of an electron }=9.1 \times 10^{-31} \mathrm{~kg}
\)
\(
\begin{aligned}
v^2 & =\frac{2 K}{m} \\
& =\frac{2 \times 0.345 \times 1.6 \times 10^{-19}}{9.1 \times 10^{-31}}=0.1104 \times 10^{12} \\
\therefore v & =3.323 \times 10^5 \mathrm{~m} / \mathrm{s}=332.3 \mathrm{~km} / \mathrm{s}
\end{aligned}
\)
Hence, the maximum speed of the emitted photoelectrons is \(332.3 \mathrm{~km} / \mathrm{s}\).

Q11.3: The photoelectric cut-off voltage in a certain experiment is 1.5 V . What is the maximum kinetic energy of photoelectrons emitted?

Answer: Photoelectric cut-off voltage, \(V_0=1.5 \mathrm{~V}\)
The maximum kinetic energy of the emitted photoelectrons is given as:
\(
K_e=e V_0
\)
Where,
\(
\begin{aligned}
& e=\text { Charge on an electron }=1.6 \times 10^{-19} \mathrm{C} \\
& \begin{aligned}
\therefore K_e & =1.6 \times 10^{-19} \times 1.5 \\
& =2.4 \times 10^{-19} \mathrm{~J}
\end{aligned}
\end{aligned}
\)
Therefore, the maximum kinetic energy of the photoelectrons emitted in the given experiment is \(2.4 \times 10^{-19} \mathrm{~J}\).

Q11.4: Monochromatic light of wavelength 632.8 nm is produced by a helium-neon laser. The power emitted is 9.42 mW.
(a) Find the energy and momentum of each photon in the light beam,
(b) How many photons per second, on the average, arrive at a target irradiated by this beam? (Assume the beam to have uniform cross-section which is less than the target area), and
(c) How fast does a hydrogen atom have to travel in order to have the same momentum as that of the photon?

Answer: Wavelength of the monochromatic light, \(\lambda=632.8 \mathrm{~nm}=632.8 \times 10^{-9} \mathrm{~m}\)
Power emitted by the laser, \(P=9.42 \mathrm{~mW}=9.42 \times 10^{-3} \mathrm{~W}\)
Planck’s constant, \(h=6.626 \times 10^{-34} \mathrm{Js}\)
Speed of light, \(c=3 \times 10^8 \mathrm{~m} / \mathrm{s}\)
Mass of a hydrogen atom, \(m=1.66 \times 10^{-27} \mathrm{~kg}\)
(a)The energy of each photon is given as:
\(
\begin{aligned}
E & =\frac{h c}{\lambda} \\
& =\frac{6.626 \times 10^{-34} \times 3 \times 10^8}{632.8 \times 10^{-9}}=3.141 \times 10^{-19} \mathrm{~J}
\end{aligned}
\)
The momentum of each photon is given as:
\(
\begin{aligned}
P & =\frac{h}{\lambda} \\
& =\frac{6.626 \times 10^{-34}}{632.8}=1.047 \times 10^{-27} \mathrm{~kg} \mathrm{~ms}^{-1}
\end{aligned}
\)
(b)Number of photons arriving per second, at a target irradiated by the beam \(=n\) Assume that the beam has a uniform cross-section that is less than the target area. Hence, the equation for power can be written as:
\(
P=n E
\)
\(
\begin{aligned}
\therefore n & =\frac{P}{E} \\
& =\frac{9.42 \times 10^{-3}}{3.141 \times 10^{-19}} \approx 3 \times 10^{16} \text { photon } / \mathrm{s}
\end{aligned}
\)
(c) The momentum of the hydrogen atom is the same as the momentum of the photon,
\(
p=1.047 \times 10^{-27} \mathrm{~kg} \mathrm{~m} \mathrm{~s}^{-1}
\)
Momentum is given as:
\(
p=m v
\)
Where, \(v=\) Speed of the hydrogen atom
\(
\begin{aligned}
\therefore v & =\frac{p}{m} \\
& =\frac{1.047 \times 10^{-27}}{1.66 \times 10^{-27}}=0.621 \mathrm{~m} / \mathrm{s}
\end{aligned}
\)

Q11.5: In an experiment on photoelectric effect, the slope of the cut-off voltage versus frequency of incident light is found to be \(4.12 \times 10^{-15} \mathrm{~V} \mathrm{~s}\). Calculate the value of Planck’s constant.

Answer: The slope of the cut-off voltage \((V)\) versus frequency \((\nu)\) of an incident light is given as:
\(
\frac{V}{\nu}=4.12 \times 10^{-15} \mathrm{Vs}
\)
\(V\) is related to frequency by the equation:
\(
h \nu=e V
\)
Where,
\(e=\) Charge on an electron \(=1.6 \times 10^{-19} \mathrm{C}\)
\(h=\) Planck’s constant
\(
\begin{aligned}
\therefore h & =e \times \frac{V}{\nu} \\
& =1.6 \times 10^{-19} \times 4.12 \times 10^{-15}=6.592 \times 10^{-34} \mathrm{Js}
\end{aligned}
\)
Therefore, the value of Planck’s constant is \(6.592 \times 10^{-34} \mathrm{Js}\).

Q11.6: The threshold frequency for a certain metal is \(3.3 \times 10^{14} \mathrm{~Hz}\). If light of frequency \(8.2 \times 10^{14} \mathrm{~Hz}\) is incident on the metal, predict the cutoff voltage for the photoelectric emission.

Answer: Threshold frequency of the metal, \(v_0=3.3 \times 10^{14} \mathrm{~Hz}\)
Frequency of light incident on the metal, \(\nu=8.2 \times 10^{14} \mathrm{~Hz}\)
Charge on an electron, \(e=1.6 \times 10^{-19} \mathrm{C}\)
Planck’s constant, \(h=6.626 \times 10^{-34} \mathrm{Js}\)
Cut-off voltage for the photoelectric emission from the metal \(=V_0\)
The equation for the cut-off energy is given as:
\(
\begin{aligned}
e V_0 & =h\left(\nu-\nu_0\right) \\
V_0 & =\frac{h\left(\nu-\nu_0\right)}{e} \\
& =\frac{6.626 \times 10^{-34} \times\left(8.2 \times 10^{14}-3.3 \times 10^{14}\right)}{1.6 \times 10^{-19}}=2.0292 \mathrm{~V}
\end{aligned}
\)
Therefore, the cut-off voltage for the photoelectric emission is 2.0292 V.

Q11.7: The work function for a certain metal is 4.2 eV. Will this metal give photoelectric emission for incident radiation of wavelength 330 nm?

Answer: No
Work function of the metal, \(\phi_0=4.2 \mathrm{eV}\)
Charge on an electron, \(e=1.6 \times 10^{-19} \mathrm{C}\)
Planck’s constant, \(h=6.626 \times 10^{-34} \mathrm{Js}\)
Wavelength of the incident radiation, \(\lambda=330 \mathrm{~nm}=330 \times 10^{-9} \mathrm{~m}\)
Speed of light, \(c=3 \times 10^8 \mathrm{~m} / \mathrm{s}\)
The energy of the incident photon is given as:
\(
\begin{aligned}
E & =\frac{h c}{\lambda} \\
& =\frac{6.626 \times 10^{-34} \times 3 \times 10^8}{330 \times 10^{-9}}=6.0 \times 10^{-19} \mathrm{~J} \\
& =\frac{6.0 \times 10^{-19}}{1.6 \times 10^{-19}}=3.76 \mathrm{eV}
\end{aligned}
\)
It can be observed that the energy of the incident radiation is less than the work function of the metal. Hence, no photoelectric emission will take place.

Q11.8: Light of frequency \(7.21 \times 10^{14} \mathrm{~Hz}\) is incident on a metal surface. Electrons with a maximum speed of \(6.0 \times 10^5 \mathrm{~m} / \mathrm{s}\) are ejected from the surface. What is the threshold frequency for the photoemission of electrons?

Answer: Frequency of the incident photon, \(\nu=7.21 \times 10^{14} \mathrm{~Hz}\)
Maximum speed of the electrons, \(v=6.0 \times 10^5 \mathrm{~m} / \mathrm{s}\)
Planck’s constant, \(h=6.626 \times 10^{-34} \mathrm{Js}\)
Mass of an electron, \(m=9.1 \times 10^{-31} \mathrm{~kg}\)
For threshold frequency \(\nu_0\), the relation for kinetic energy is written as:
\(
\begin{aligned}
&\begin{aligned}
& \frac{1}{2} m v^2=h\left(\nu-\nu_0\right) \\
& \begin{aligned}
\nu_0 & =\nu-\frac{m v^2}{2 h} \\
& =7.21 \times 10^{14}-\frac{\left(9.1 \times 10^{-31}\right) \times\left(6 \times 10^5\right)^2}{2 \times\left(6.626 \times 10^{-34}\right)} \\
& =7.21 \times 10^{14}-2.472 \times 10^{14} \\
& =4.738 \times 10^{14} \mathrm{~Hz}
\end{aligned}
\end{aligned}\\
&\text { Therefore, the threshold frequency for the photoemission of electrons is } 4.738 \times 10^{14} \mathrm{~Hz} \text {. }
\end{aligned}
\)

Q11.9: Light of wavelength 488 nm is produced by an argon laser which is used in the photoelectric effect. When light from this spectral line is incident on the emitter, the stopping (cut-off) potential of photoelectrons is 0.38 V . Find the work function of the material from which the emitter is made.

Answer: Wavelength of light produced by the argon laser, \(\lambda=488 \mathrm{~nm}\)
\(
=488 \times 10^{-9} \mathrm{~m}
\)
Stopping potential of the photoelectrons, \(V_0=0.38 \mathrm{~V}\)
\(
1 \mathrm{eV}=1.6 \times 10^{-19} \mathrm{~J}
\)
\(
\therefore V_0=\frac{0.38}{1.6 \times 10^{-19}} \mathrm{eV}
\)
Planck’s constant, \(h=6.6 \times 10^{-34} \mathrm{Js}\)
Charge on an electron, \(e=1.6 \times 10^{-19} \mathrm{C}\)
Speed of light, \(c=3 \times 10 \mathrm{~m} / \mathrm{s}\)
From Einstein’s photoelectric effect, we have the relation involving the work function \(\Phi_0\) of the material of the emitter as:
\(
e V_0=\frac{h c}{\lambda}-\phi_0
\)
\(
\begin{aligned}
&\begin{aligned}
\phi_0 & =\frac{h c}{\lambda}-e V_0 \\
& =\frac{6.6 \times 10^{-34} \times 3 \times 10^8}{1.6 \times 10^{-19} \times 488 \times 10^{-9}}-\frac{1.6 \times 10^{-19} \times 0.38}{1.6 \times 10^{-19}} \\
& =2.54-0.38=2.16 \mathrm{eV}
\end{aligned}\\
&\text { Therefore, the material with which the emitter is made has the work function of } 2.16 \mathrm{eV} \text {. }
\end{aligned}
\)

Q11.10: What is the de Broglie wavelength of
(a) a bullet of mass 0.040 kg travelling at the speed of \(1.0 \mathrm{~km} / \mathrm{s}\),
(b) a ball of mass 0.060 kg moving at a speed of \(1.0 \mathrm{~m} / \mathrm{s}\), and
(c) a dust particle of mass \(1.0 \times 10^{-9} \mathrm{~kg}\) drifting with a speed of 2.2 \(\mathrm{m} / \mathrm{s}\)?

Answer: (a) Mass of the bullet, \(m=0.040 \mathrm{~kg}\)
Speed of the bullet, \(v=1.0 \mathrm{~km} / \mathrm{s}=1000 \mathrm{~m} / \mathrm{s}\)
Planck’s constant, \(h=6.6 \times 10^{-34} \mathrm{Js}\)
De Broglie wavelength of the bullet is given by the relation:
\(
\begin{aligned}
& \lambda=\frac{h}{m v} \\
& =\frac{6.6 \times 10^{-34}}{0.040 \times 1000}=1.65 \times 10^{-35} \mathrm{~m}
\end{aligned}
\)
(b) Mass of the ball, \(m=0.060 \mathrm{~kg}\)
Speed of the ball, \(v=1.0 \mathrm{~m} / \mathrm{s}\)
De Broglie wavelength of the ball is given by the relation:
\(
\begin{aligned}
& \lambda=\frac{h}{m v} \\
& =\frac{6.6 \times 10^{-34}}{0.060 \times 1}=1.1 \times 10^{-32} \mathrm{~m}
\end{aligned}
\)
(c) Mass of the dust particle, \(m=1 \times 10^{-9} \mathrm{~kg}\)
Speed of the dust particle, \(v=2.2 \mathrm{~m} / \mathrm{s}\)
De Broglie wavelength of the dust particle is given by the relation:
\(
\begin{aligned}
& \lambda=\frac{h}{m v} \\
& =\frac{6.6 \times 10^{-34}}{2.2 \times 1 \times 10^{-9}}=3.0 \times 10^{-25} \mathrm{~m}
\end{aligned}
\)

Q11.11: Show that the wavelength of electromagnetic radiation is equal to the de Broglie wavelength of its quantum (photon).

Answer: The momentum of a photon having energy \((h v)\) is given as:
\(
\begin{aligned}
& p=\frac{h v}{c}=\frac{h}{\lambda} \\
& \lambda=\frac{h}{p} \dots(i)
\end{aligned}
\)
Where,
\(\lambda=\) Wavelength of the electromagnetic radiation
\(c=\) Speed of light
\(h=\) Planck’s constant
De Broglie wavelength of the photon is given as:
\(
\lambda=\frac{h}{m v}
\)
But \(p=m v\)
\(
\therefore \lambda=\frac{h}{p} \dots(ii)
\)
Where,
\(m=\) Mass of the photon
\(v=\) Velocity of the photon
Hence, it can be inferred from equations (i) and (ii) that the wavelength of the electromagnetic radiation is equal to the de Broglie wavelength of the photon.

Exemplar Section

VSA

Q11.14: A proton and an \(\alpha\)-particle are accelerated, using the same potential difference. How are the deBroglie wavelengths \(\lambda_{\mathrm{p}}\) and \(\lambda_{\mathrm{a}}\) related to each other?

Answer: Key concept: Hence de-Broglie wavelength:
\(
\lambda=\frac{h}{p}=\frac{h}{\sqrt{2 m E}}=\frac{h}{\sqrt{2 m q V}}
\)
In this problem since both proton and \(\alpha\)-particle are accelerated through same potential difference,
We know that, \(\lambda=\frac{h}{\sqrt{2 m q v}}\)
\(
\begin{array}{ll}
\therefore & \lambda \propto \frac{1}{\sqrt{m q}} \\
& \frac{\lambda_p}{\lambda_\alpha}=\frac{\sqrt{m_\alpha q_\alpha}}{m_p q_p}=\frac{\sqrt{4 m_p \times 2 e}}{\sqrt{m_p \times e}}=\sqrt{8} \\
\therefore & \lambda_p=\sqrt{8} \lambda_\alpha
\end{array}
\)
i.e., wavelength of proton is \(\sqrt{8}\) times wavelength of \(\alpha\)-particle.

Note: \(\lambda_p / \lambda_d=p_x / p_p=\frac{\sqrt{2 m_\alpha E_\alpha}}{\sqrt{2 m_p E_p}}=\sqrt{8}: 1\)

Q11.15: (i) In the explanation of the photoelectric effect, we assume one photon of frequency \(\nu\) collides with an electron and transfers
its energy. This leads to the equation for the maximum energy \(E_{\max }\) of the emitted electron as
\(
E_{\max }=h \nu-\phi_o
\)
where \(\phi_0\) is the work function of the metal. If an electron absorbs 2 photons (each of frequency \(\nu\) ) what will be the maximum energy for the emitted electron?
(ii) Why is this fact (two-photon absorption) not taken into consideration in our discussion of the stopping potential?

Answer: (i) According to the question, an electron absorbs the energy of two photons each of frequency \(\nu\) then \(\nu^{\prime}=2 \nu\) where \(\nu^{\prime}\) is the frequency of emitted electron.
Here, \(\quad E_{\max }=h \nu-\phi_0\)
Thus, the maximum energy for emitted electrons is
\(
E_{\max }=h(2 \nu)-\phi_0=2 h \nu-\phi_0
\)
(ii) The probability of absorbing two photons by the same electron is very low. Hence, such emission will be negligible.

Q11.16: There are materials which absorb photons of shorter wavelength and emit photons of longer wavelength. Can there be stable substances which absorb photons of larger wavelength and emit light of shorter wavelength.

Answer: In the first case, when the materials which absorb photons of shorter wavelength has the energy of the incident photon on the material is high and the energy of the emitted photon is low when it has a longer wavelength or in short we can say that energy given out is less than the energy supplied. But in the second case, the energy of the incident photon is low for the substances which has to absorb photons of larger wavelength and the energy of the emitted photon is high to emit light Of shorter wavelength. This means in this statement material has to supply the energy for the emission of photons. But this is not possible for a stable substance.

Q11.17: Do all the electrons that absorb a photon come out as photoelectrons?

Answer: In the photoelectric effect, we can observe that most electrons get scattered into the metal by absorbing a photon. Therefore, all the electrons that absorb a photon doesn’t come out as photoelectron. Only a few come out of metal whose energy becomes greater than the work function of metal.

Q11.18: There are two sources of light, each emitting with a power of 100 W. One emits X-rays of wavelength 1 nm and the other visible light at 500 nm. Find the ratio of number of photons of X-rays to the photons of visible light of the given wavelength?

Answer: Here in this problem, total energy will be constant.
Let us assume the wavelength of X-rays is \(\lambda_1\) and the wavelength of visible light is \(\lambda_2\).
Given,
\(
\begin{array}{ll}
\text { Given, } & P=100 \mathrm{~W} \\
& \lambda_1=1 \mathrm{~nm} \\
\text { and } & \lambda_2=500 \mathrm{~nm}
\end{array}
\)
Total \(E\) is constant
Let \(n_1\) and \(n_2\) be the number of photons of X-rays and visible region
\(
n_1 E_1=n_2 E_2
\)
\(
\begin{aligned}
& n_1 \frac{h c}{\lambda_1}=n_2 \frac{h c}{\lambda_2} \\
& \frac{n_1}{n_2}=\frac{\lambda_1}{\lambda_2} . \\
& \frac{n_1}{n_2}=\frac{1}{500} .
\end{aligned}
\)

SA

Q11.19: Consider Fig. 11.1 for photoemission. How would you reconcile with momentum-conservation? Note light (photons) have momentum in a different direction than the emitted electrons.

Answer: The momentum of the incident photon is transferred to the metal,during photoelectric emission. At the microscopic level, atoms of a metal absorb the photon, and its momentum is transferred mainly to the nucleus and electrons. The excited electron is emitted. Therefore, the conservation of momentum is to be considered as the momentum of incident photon transferred to the nucleus and electron.

Q11.20: Consider a metal exposed to light of wavelength 600 nm. The maximum energy of the electron doubles when light of wavelength 400 nm is used. Find the work function in eV.

Answer: Key concept: Work function (or threshold energy) \(\left(W_0\right)\): The minimum energy of incident radiation required to eject the electrons from metallic surface is defined as the work function of that surface.
\(
\begin{aligned}
W_0 & =h \nu_0=\frac{h c}{\lambda_0} \text { Joules; } \nu_0=\text { Threshold frequency; } \\
\lambda_0 & =\text { Threshold wavelength }
\end{aligned}
\)
Work function in electron volt, \(W_0(\mathrm{eV})=\frac{h c}{e \lambda_0}=\frac{12375}{\lambda_0(Å)}\)
Einstein’s photoelectric equation is \(E=W_0+K_{\max }\)

\(
\text { Maximum energy }=h \nu-\phi
\)
According to the problem for the first condition wavelength of light \(\lambda=600 \mathrm{~nm}\) and for the second condition, the wavelength of light \(\lambda^{\prime}=400 \mathrm{~nm}\)
Also, the maximum kinetic energy for the second condition is equal to the twice of the kinetic energy in the first condition.
\(
\begin{array}{ll}
\text { i.e., } & K_{\max }^{\prime}=2 K_{\max } \\
\text { then } & K_{\max }^{\prime}=\frac{h c}{\lambda}-\phi \\
\Rightarrow & 2 K_{\max }=\frac{h c}{\lambda^{\prime}}-\phi_0 \\
\Rightarrow & 2\left(\frac{1230}{600}-\phi\right)=\left(\frac{1230}{400}-\phi\right) \quad [\because h c \approx 1240 \mathrm{eV} \mathrm{~nm}]\\
\Rightarrow & \phi=\frac{1230}{1230}=1.02 \mathrm{eV}
\end{array}
\)

Q11.21: Assuming an electron is confined to a 1 nm wide region, find the uncertainty in momentum using the Heisenberg Uncertainty principle(\((\Delta x \times \Delta p=h)\)). You can assume the uncertainty in position \(\Delta x\) as 1 nm. Assuming \(p \approx \Delta p\), find the energy of the electron in electron volts.

Answer: In this problem, \(\Delta x=1 \mathrm{~nm}=10^{-9} \mathrm{~m}\), we have to find \(\Delta p\). As we know \(\Delta x \cdot \Delta p\) \(=h\)
Therefore, \(\quad \Delta p=\frac{h}{\Delta x}=\frac{h}{2 \pi \Delta x}\)
\(
\begin{aligned}
\Rightarrow \quad & =\frac{6.62 \times 10^{-34} \mathrm{Js}}{2 \times(22 / 7)\left(10^{-9}\right) \mathrm{m}} \\
& =1.05 \times 10^{-25} \mathrm{~kg} \mathrm{~m} / \mathrm{s}
\end{aligned}
\)
Energy,
\(
\begin{aligned}
E & =\frac{p^2}{2 m}=\frac{(\Delta p)^2}{2 m} [\because p \approx \Delta p] \\
& =\frac{\left(1.05 \times 10^{-25}\right)^2}{2 \times 9.1 \times 10^{-31}} \mathrm{~J}=3.8 \times 10^{-2} \mathrm{eV}
\end{aligned}
\)

Q11.22: Two monochromatic beams A and B of equal intensity \(I\), hit a screen. The number of photons hitting the screen by beam \(A\) is twice that by beam \(B\). Then what inference can you make about their frequencies?

Answer: Let us assume \(n_A\) is the number of photons falling per second of beam \(A\) and \(n_B\) is the number of photons falling per second of beam \(B\).
And it is given that the number of photons hitting the screen by beam \(A\) is twice that by beam \(B . n_A=2 n_B\)
The energy of falling photon of beam \(A=h v_A\)
The energy of falling photon of beam \(B=h v_B\)
Now, according to the question, the intensity of \(A\) is equal to the intensity of \(B\).
\(
\begin{array}{ll}
\text { Therefore, } & I=n_A v_A=n_B v_B \\
\Rightarrow & \frac{v_A}{v_B}=\frac{n_B}{n_A}=\frac{n_B}{2 n_B}=\frac{1}{2} \\
\Rightarrow & v_B=2 v_A
\end{array}
\)

Q11.23: Two particles A and B of de Broglie wavelengths \(\lambda_1\) and \(\lambda_2\) combine to form a particle C. The process conserves momentum. Find the de Broglie wavelength of the particle C. (The motion is one dimensional).

Answer: By the law of conservation of momentum,
\(
\left|p_C\right|=\left|p_A\right|+\left|p_B\right|
\)
Let us first take the case I when both \(p_A\) and \(p_B\) are positive, then
\(
\lambda_C=\frac{\lambda_A \lambda_B}{\lambda_A+\lambda_B}
\)
In second case when both \(p_A\) and \(p_B\) are negative, then
\(
\lambda_C=\frac{\lambda_A \lambda_B}{\lambda_A+\lambda_B}
\)
In case III when \(p_A>0, p_B<0\) i.e., \(p_A\) is positive and \(p_B\) is negative,
\(
\begin{aligned}
\frac{h}{\lambda_C} & =\frac{h}{\lambda_A}-\frac{h}{\lambda_B}=\frac{\left(\lambda_B-\lambda_A\right) h}{\lambda_A \lambda_B} \\
\Rightarrow \quad \lambda_C & =\frac{\lambda_A \lambda_B}{\lambda_B-\lambda_A}
\end{aligned}
\)
\(
\begin{aligned}
&\text { And in case IV when } p_A<0, p_B>0 \text {, i.e., } p_A \text { is negative and } p_B \text { is positive. }\\
&\begin{array}{ll}
\therefore & \frac{h}{\lambda_C}=\frac{-h}{\lambda_A}+\frac{h}{\lambda_B} \\
\Rightarrow & =\frac{\left(\lambda_A-\lambda_B\right) h}{\lambda_A \lambda_B} \Rightarrow \lambda_C=\frac{\lambda_A \lambda_B}{\lambda_A-\lambda_B}
\end{array}
\end{aligned}
\)

Q11.24: A neutron beam of energy \(E\) scatters from atoms on a surface with a spacing \(d=0.1 \mathrm{~nm}\). The first maximum of intensity in the reflected beam occurs at \(\theta=30^{\circ}\). What is the kinetic energy \(E\) of the beam in eV?

Answer: Here, \(d=0.1 \mathrm{~nm}, \theta=30^{\circ}, n=1\).
According to Bragg’s law
\(
\begin{aligned}
& 2 d \sin \theta=n \lambda \text { or } 2 \times 0.1 \times \sin 30^{\circ}=1 \times \lambda \\
& \text { or } \lambda =0.1 n m=10^{-10} m
\end{aligned}
\)
\(
p=\frac{h}{\lambda}=\frac{6.62 \times 10^{-34}}{10^{-10}}=6.6 \times 10^{-24} \mathrm{kgms}^{-1}
\)
\(
K E=\frac{1}{2} \frac{p^2}{m}=\frac{1}{2} \times \frac{\left(6.6 \times 10^{-24}\right)^2}{1.67 \times 10^{-27}} J=0.21 \mathrm{eV}
\)

LA

Q11.25: Consider a thin target ( \(10^{-2} \mathrm{~m}\) square, \(10^{-3} \mathrm{~m}\) thickness) of sodium, which produces a photocurrent of \(100 \mu \mathrm{~A}\) when a light of intensity \(100 \mathrm{~W} / \mathrm{m}^2(\lambda=660 \mathrm{~nm})\) falls on it. Find the probability that a photoelectron is produced when a photon strikes a sodium atom. [Take density of \(\mathrm{Na}=0.97 \mathrm{~kg} / \mathrm{m}^3\) ].

Answer: According to the problem, area of the target \(A=10^{-2} \mathrm{~cm}^2=10^{-4} \mathrm{~m}^2\)
And thickness, \(d=10^{-3} \mathrm{~m}\)
Photocurrent, \(i=100 \times 10^{-6} \mathrm{~A}=10^{-4} \mathrm{~A}\)
Intensity, \(\quad I=100 \mathrm{~W} / \mathrm{m}^2\)
\(
\begin{aligned}
\Rightarrow \quad & =660 \mathrm{~nm}=660 \times 10^{-9} \mathrm{~m} \\
\rho_{N a} & =0.97 \mathrm{~kg} / \mathrm{m}^3
\end{aligned}
\)
Avogadro’s number \(=6 \times 10^{26} \mathrm{~kg}\) atom
\(
\begin{aligned}
\text { Volume of sodium target } & =A \times d \\
& =10^{-4} \times 10^{-3} \\
\Rightarrow \quad & =10^{-7} \mathrm{~m}^3
\end{aligned}
\)
We know that \(6 \times 10^{26}\) atoms of sodium weighs \(=23 \mathrm{~kg}\)
Density of sodium \(=0.97 \mathrm{~kg} / \mathrm{m}^3\)
Hence the, volume of \(6 \times 10^6\) sodium atoms \(=\frac{23}{0.97} \mathrm{~m}^3\)
Volume occupied by one sodium atom. \(=\frac{23}{.0 .97 \times\left(6 \times 10^{26}\right)}=3.95 \times 10^{-26} \mathrm{~m}^3\)
Number of sodium atoms in target \(\left(\mathrm{N}_{\text {sodium }}\right)=\frac{10^{-7}}{3.95 \times 10^{-26}}=2.53 \times 10^{18}\)
Let \(n\) be the number of photons falling per second on the target.
Energy of each photon \(=h c / \lambda\)
Total energy falling per second on target \(=\frac{n h c}{\lambda}=I A\)
\(
\therefore \quad n=\frac{I A \lambda}{h c}
\)
\(
=\frac{100 \times 10^{-4} \times\left(660 \times 10^{-9}\right)}{\left(6.62 \times 10^{-34}\right) \times\left(3 \times 10^8\right)}=3.3 \times 10^{16}
\)
Let \(P\) be the probability of emission per atom per photon. The number of photoelectrons emitted per second
\(
\begin{aligned}
N & =P \times n \times\left(\mathrm{N}_{\text {sodium }}\right) \\
& =P \times\left(33 \times 10^{16}\right) \times\left(2.53 \times 10^{18}\right)
\end{aligned}
\)
\(
\begin{aligned}
&\text { Now, according to question, }\\
&\begin{aligned}
& i=100 \mu \mathrm{~A}=100 \times 10^{-6}=10^{-4} \mathrm{~A} \\
& \text { Current, } \quad i=N e \\
& \therefore \quad 10^{-4}=P \times\left(3.3 \times 10^{16}\right) \times\left(2.53 \times 10^{18}\right) \times\left(1.6 \times 10^{-19}\right)
\end{aligned}
\end{aligned}
\)
\(
\begin{aligned}
P & =\frac{10^{-4}}{\left(3.3 \times 10^{16}\right) \times\left(2.53 \times 10^{18}\right) \times\left(1.6 \times 10^{-19}\right)} \\
& =7.48 \times 10^{-21}
\end{aligned}
\)
Then, the probability of photoemission by a single photon on a single atom is very much less than 1. Because the absorption of two photons by an atom is negligible.

Q11.26: Consider an electron in front of the metallic surface at a distance \(d\) (treated as an infinite plane surface). Assume the force of attraction by the plate is given as \(\frac{1}{4} \frac{q^2}{4 \pi \varepsilon_0 d^2}\). Calculate work in taking the charge to an infinite distance from the plate. Taking \(d=0.1 \mathrm{~nm}\), find the work done in electron volts. [Such a force law is not valid for \(d<0.1 \mathrm{~nm}\) ].

Answer: Force of attraction: \(F=\frac{1}{4} \frac{q^2}{4 \pi \varepsilon_0 d^2}\)
Distance \(d=0.1 \mathrm{~nm}=0.1 \times 10^{-9} \mathrm{~m}\)
Elementary charge \(q=1.602 \times 10^{-19} \mathrm{C}\)
Permittivity of free space \(\varepsilon_0=8.854 \times 10^{-12} \frac{C^2}{N m^2}\)
Work done is the integral of force over distance:
\(W=\int F d x\)
\(
1 \mathrm{eV}=1.602 \times 10^{-19} \mathrm{~J}
\)
\(
\begin{aligned}
&\text { The work done in moving the charge from } d \text { to infinity is given by: }\\
&\begin{aligned}
& W=\int_d^{\infty} F d x \\
& W=\int_d^{\infty} \frac{1}{4} \frac{q^2}{4 \pi \varepsilon_0 x^2} d x \\
& W=\frac{q^2}{16 \pi \varepsilon_0} \int_d^{\infty} \frac{1}{x^2} d x \\
& W=\frac{q^2}{16 \pi \varepsilon_0}\left[-\frac{1}{x}\right]_d^{\infty} \\
& W=\frac{q^2}{16 \pi \varepsilon_0}\left(0-\left(-\frac{1}{d}\right)\right) \\
& W=\frac{q^2}{16 \pi \varepsilon_0 d}
\end{aligned}
\end{aligned}
\)
\(
\begin{aligned}
& W=\frac{\left(1.602 \times 10^{-19} \mathrm{C}\right)^2}{16 \pi\left(8.854 \times 10^{-12} \frac{c^2}{N m^2}\right)\left(0.1 \times 10^{-9} \mathrm{~m}\right)} \\
& W=\frac{2.566 \times 10^{-38}}{4.454 \times 10^{-20}} \mathrm{~J} \\
& W=5.761 \times 10^{-19} \mathrm{~J}
\end{aligned}
\)
\(
\begin{aligned}
&\text { Convert to electron volts }\\
&\begin{aligned}
W_{e V} & =\frac{W}{1.602 \times 10^{-19} \mathrm{~J} / \mathrm{eV}} \\
W_{e V} & =\frac{5.761 \times 10^{-19} \mathrm{~J}}{1.602 \times 10^{-19} \mathrm{~J} / \mathrm{eV}} \\
W_{e V} & =3.6 \mathrm{eV}
\end{aligned}
\end{aligned}
\)
The work done in taking the charge to infinity is 3.6 eV.

Q11.27: A student performs an experiment on the photoelectric effect, using two materials A and B. A plot of \(V_{\text {stop }} v s\) \(v\) is given in Fig. 11.2.
(i) Which material A or B has a higher work function?
(ii) Given the electric charge of an electron \(=1.6 \times 10^{-19} \mathrm{C}\), find the value of \(h\) obtained from the experiment for both A and B.
Comment on whether it is consistent with Einstein’s theory:

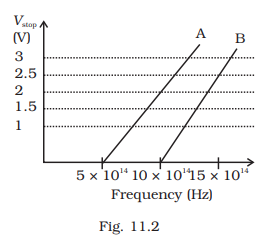
Answer: Key concept: Threshold frequency \(\left(n_0\right)\): The minimum frequency of incident radiations, required to eject the electron from the metal surface is defined as the threshold frequency.
If incident frequency \(n<n_0 \Rightarrow\) No photoelectron emission
For most metals the threshold frequency is in the ultraviolet (corresponding to wavelengths between 200 and 300 nm ), but for potassium and cesium oxides it is in the visible spectrum ( \(\lambda\) between 400 and 700 nm ).

(i)Here we are given threshold frequency of \(A\)
For \(B\),
\(
\begin{aligned}
& \nu_{O A}=5 \times 10^{14} \mathrm{~Hz} \text { and } \\
& \nu_{O B}=10 \times 10^{14} \mathrm{~Hz}
\end{aligned}
\)
\(
\begin{aligned}
&\text { We know that }\\
&\begin{array}{ll}
\text { Work function, } & \phi=h \nu_0 \text { or } \phi_0 \propto \nu_0 \\
\Rightarrow & \phi_0 \propto \nu_0
\end{array}
\end{aligned}
\)
\(
\begin{array}{ll}
\text { So, } & \frac{\phi_{O A}}{\phi_{O B}}=\frac{5 \times 10^{14}}{10 \times 10^{14}}<1 \\
\Rightarrow & \phi_{O A}<\phi_{O B}
\end{array}
\)
Thus, the work function of \(B\) is higher than \(A\).
(ii) For metal \(A\), slope \(=\frac{h}{e}=\frac{2}{(10-15) 10^{14}}\)
\(
\begin{aligned}
h & =\frac{2 e}{5 \times 10^{14}}=\frac{2 \times 1.6 \times 10^{-19}}{5 \times 10^{14}} \\
& =6.4 \times 10^{-34} \mathrm{Js}
\end{aligned}
\)
For metal \(B\), slope \(=\frac{h}{e}=\frac{2.5}{(15-10) 10^{14}}\)
\(
h=\frac{2.5 \times e}{5 \times 10^{14}}=\frac{2.5 \times 1.6 \times 10^{-19}}{5 \times 10^{14}}=8 \times 10^{-34} \mathrm{Js}
\)
Since the value of \(h\) from the experiment for metals \(A\) and \(B\) is different. Hence, the experiment is not consistent with the theory.

Q11.28: A particle A with a mass \(m_A\) is moving with a velocity \(v\) and hits a particle B (mass \(m_B\) ) at rest (one-dimensional motion). Find the change in the de Broglie wavelength of the particle A. Treat the collision as elastic.

Answer: As collision is elastic so the law of conservation of momentum and Kinetic energy is obeyed.
\(
m_A v+m_B(0)=m_A v_1+m_B v_2
\)
\(
m_A\left(v-v_1\right)=m_B v_2 \dots(1)
\)
and \(\frac{1}{2} m_A v^2+\frac{1}{2} m_B(0)^2=\frac{1}{2} m_A v_1^2+\frac{1}{2} m_B v_2^2\)
\(
m_A\left(v^2-v_1^2\right)=m_B v_2^2 \dots(ii)
\)
Dividing (ii) by (i) we get,
\(
\therefore v+v_1=v_2 \dots(iii)
\)
or \(v=v_2-v_1\)
Putting Eq (iii) in Eq (i)
\(
{m}_A v-{m}_A v_1=m_B\left(v+v_1\right)
\)
\(
v_1=\frac{\left(m_A-m_B\right)}{\left(m_A+m_B\right)} v
\)
and \(v_2=\left(\frac{2 m_A}{m_A+m_B}\right) v\)
\(
\therefore \lambda_{\text {Initial}}=\frac{h}{m_A v}
\)
\(
\lambda_{\text {final }}=\frac{h}{m_A v}=\left|\frac{h\left(m_A+m_B\right)}{m_A\left(m_A-m_B\right) v}\right|
\)
\(
\Delta \lambda=\lambda_{\text {final }}-\lambda_{\text {initial }}=\frac{h}{m_A v}\left[\left|\frac{\left.m_A+m_B\right)}{m_A-m_B}\right|-1\right]
\)

Q11.29: Consider a 20 W bulb emitting light of wavelength \(5000 Å\) and shining on a metal surface kept at a distance 2 m. Assume that the metal surface has work function of 2 eV and that each atom on the metal surface can be treated as a circular disk of radius 1.5 A.
(i) Estimate no. of photons emitted by the bulb per second. [Assume no other losses]
(ii) Will there be photoelectric emission?
(iii) How much time would be required by the atomc disk to receive energy equal to work function ( 2 eV )?
(iv) How many photons would atomic disk receive within the time duration calculated in (iii) above?
(v) Can you explain how the photoelectric effect was observed instantaneously?
[Hint: Time calculated in part (iii) is from classical consideration and you may further take the target of the surface area say \(1 \mathrm{~cm}^2\) and estimate what would happen?]

Answer: According to the problem, \(P=20 \mathrm{~W}, \lambda=5000 Å=5000 \times 10^{-10} \mathrm{~m}\), distance \((d)=2 \mathrm{~m}\), work function \(\phi_0=2 \mathrm{eV}\), radius \(r=1.5 \mathrm{~A}=1.5 \times 10^{-10} \mathrm{~m}\) Now, Number of photon emitted by bulb per second, \(n^{\prime}=\frac{d N}{d t}\)
(i) Number of photon emitted by bulb per second is \(n^{\prime}=\frac{p}{h c / \lambda}=\frac{p \lambda}{h c}\)
\(
\begin{aligned}
&=\frac{20 \times\left(5000 \times 10^{-10}\right)}{\left(6.62 \times 10^{-34}\right) \times\left(3 \times 10^8\right)} \\
& \Rightarrow \quad n^{\prime}=5 \times 10^{19} / \mathrm{sec}
\end{aligned}
\)
(ii) Energy of the incident photon
\(
=\frac{h c}{\lambda}=\frac{\left(6.62 \times 10^{-34}\right)\left(3 \times 10^8\right)}{5000 \times 10^{-10} \times 1.6 \times 10^{-19}}=2.48 \mathrm{eV}
\)
As this energy is greater than 2 eV (i.e., the work function of the metal surface), hence photoelectric emission takes place.

(iii) Let \(\Delta t\) be the time spent in getting the energy \(\phi=\) (work function of metal).
Consider the figure, if \(P\) is the power of the source then energy received by atomic disc
\(
\frac{P}{4 \pi d^2} \times \pi r^2 \Delta t=\phi_0
\)
\(
\Rightarrow \quad \Delta t=\frac{4 \phi_0 d^2}{P r^2}
\)
\(
=\frac{4 \times\left(2 \times 1.6 \times 10^{-19}\right) \times 2^2}{20 \times\left(1.5 \times 10^{-10}\right)^2} \approx 28.4 \mathrm{~s}
\)
(iv) Number of photons received by atomic disc in time \(\Delta t\) is
\(
\begin{aligned}
N & =\frac{n^{\prime} \times \pi r^2}{4 \pi d^2} \times \Delta t \\
& =\frac{n^{\prime} r^2 \Delta t}{4 d^2} \\
& =\frac{\left(5 \times 10^{19}\right) \times\left(1.5 \times 10^{-10}\right)^2 \times 28.4}{4 \times(2)^2} \approx 2
\end{aligned}
\)
Now let us discuss the last part in detail. As the time of emission of electrons is 11.04 s.
Hence, in this problem, the photoelectric emission is not instantaneous.
(v) In photoelectric emission, there is a collision between the incident photon and free electron of the metal surface, which lasts for a very very short interval of time ( \(\approx 10^{-9} \mathrm{~s}\) ), hence we say photoelectric emission is instantaneous.