7.6 AC Voltage Applied to a Series LCR Circuit

Figure 7.10 shows a series LCR circuit connected to an ac source \(\varepsilon\). As usual, we take the voltage of the source to be \(v=v_m \sin \omega t\).
If \(q\) is the charge on the capacitor and \(i\) the current, at time \(t\), we have, from Kirchhoff’s loop rule:
\(
L \frac{\mathrm{~d} i}{\mathrm{~d} t}+i R+\frac{q}{C}=v \dots(7.20)
\)
We want to determine the instantaneous current \(i\) and its phase relationship to the applied alternating voltage \(v\). We shall solve this problem by two methods. First, we use the technique of phasors and in the second method, we solve Eq. (7.20) analytically to obtain the timedependence of \(i\).

7.6.1 Phasor-diagram solution

From the circuit shown in Fig. 7.10, we see that the resistor, inductor and capacitor are in series. Therefore, the ac current in each element is the same at any time, having the same amplitude and phase. Let it be
\(
i=i_m \sin (\omega t+\phi) \dots(7.21)
\)
where \(\phi\) is the phase difference between the voltage across the source and the current in the circuit. On the basis of what we have learnt in the previous sections, we shall construct a phasor diagram for the present case.

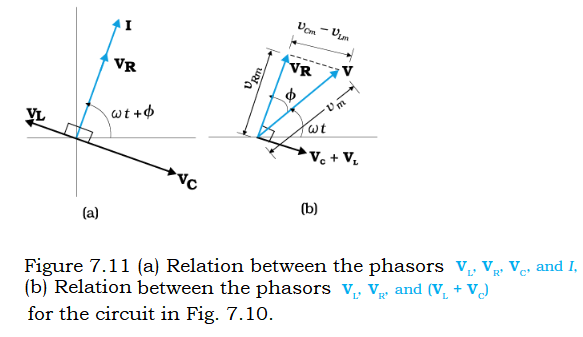
Let \(\mathbf{I}\) be the phasor representing the current in the circuit as given by Eq. (7.21). Further, let \(\mathbf{V}_{\mathbf{L}}, \mathbf{V}_{\mathbf{R}}, \mathbf{V}_{\mathbf{C}}\), and \(\mathbf{V}\) represent the voltage across the inductor, resistor, capacitor and the source, respectively. From previous section, we know that \(\mathbf{V}_{\mathbf{R}}\) is parallel to \(\mathbf{I}, \mathbf{V}_{\mathbf{C}}\) is \(\pi / 2\) behind \(\mathbf{I}\) and \(\mathbf{V}_{\mathbf{L}}\) is \(\pi / 2\) ahead of \(\mathbf{I} . \mathbf{V}_{\mathbf{L}}, \mathbf{V}_{\mathbf{R}}, \mathbf{V}_{\mathbf{C}}\) and \(\mathbf{I}\) are shown in Fig. 7.11(a) with apppropriate phase relations.
The length of these phasors or the amplitude of \(\mathbf{V}_{\mathbf{R}}, \mathbf{V}_{\mathbf{C}}\) and \(\mathbf{V}_{\mathbf{L}}\) are:
\(
v_{R m}=i_m R, v_{C m}=i_m X_C, v_{L m}=i_m X_L \dots(7.22)
\)
The voltage Equation (7.20) for the circuit can be written as
\(
v_{\mathrm{L}}+v_{\mathrm{R}}+v_{\mathrm{C}}=v \dots(7.23)
\)
The phasor relation whose vertical component gives the above equation is
\(
\mathbf{v}_{\mathbf{L}}+\mathbf{v}_{\mathbf{R}}+\mathbf{v}_{\mathbf{C}}=\mathbf{v} \dots(7.24)
\)
This relation is represented in Fig. 7.11(b). Since \(\mathbf{V}_{\mathbf{C}}\) and \(\mathbf{V}_{\mathbf{L}}\) are always along the same line and in opposite directions, they can be combined into a single phasor \(\left(\mathbf{V}_{\mathbf{c}}+\mathbf{V}_{\mathbf{L}}\right)\) which has a magnitude \(\left|v_{C_m}-v_{L m}\right|\). Since \(\mathbf{V}\) is represented as the hypotenuse of a right-triangle whose sides are \(\mathbf{V}_{\mathbf{R}}\) and \(\left(\mathbf{V}_{\mathbf{C}}+\mathbf{V}_{\mathbf{L}}\right)\), the pythagorean theorem gives:
\(
v_m^2=v_{R m}^2+\left(v_{C m}-v_{L m}\right)^2
\)
Substituting the values of \(v_{R m}, v_{C m}\), and \(v_{L m}\) from Eq. (7.22) into the above equation, we have
\(
\begin{aligned}
v_m^2 & =\left(i_m R\right)^2+\left(i_m X_C-i_m X_L\right)^2 \\
& =i_m^2\left[R^2+\left(X_C-X_L\right)^2\right]
\end{aligned}
\)
or, \(i_m=\frac{v_m}{\sqrt{R^2+\left(X_C-X_L\right)^2}} \dots[7.25(a)]\)
By analogy to the resistance in a circuit, we introduce the impedance \(Z\) in an ac circuit:
\(
i_m=\frac{v_m}{Z} \dots[7.25(b)]
\)
where \(Z=\sqrt{R^2+\left(X_C-X_L\right)^2} \dots(7.26)\)

Since phasor \(\mathbf{I}\) is always parallel to phasor \(\mathbf{V}_{\mathbf{R}}\), the phase angle \(\phi\) is the angle between \(\mathbf{V}_{\mathbf{R}}\) and \(\mathbf{V}\) and can be determined from Fig. 7.12:
\(
\tan \phi=\frac{v_{C m}-v_{L m}}{v_{R m}}
\)
Using Eq. (7.22), we have
\(
\tan \phi=\frac{X_C-X_L}{R} \dots(7.27)
\)
Equations (7.26) and (7.27) are graphically shown in Fig. (7.12). This is called Impedance diagram which is a right-triangle with \(Z\) as its hypotenuse.
Equation 7.25 (a) gives the amplitude of the current and Eq. (7.27) gives the phase angle. With these, Eq. (7.21) is completely specified.

If \(X_C>X_L, \phi\) is positive and the circuit is predominantly capacitive. Consequently, the current in the circuit leads the source voltage. If \(X_C<X_L, \phi\) is negative and the circuit is predominantly inductive. Consequently, the current in the circuit lags the source voltage.

Figure 7.13 shows the phasor diagram and variation of \(v\) and \(i\) with \(\omega t\) for the case \(X_C>X_L\).

Thus, we have obtained the amplitude and phase of current for an \(LCR\) series circuit using the technique of phasors. But this method of analysing ac circuits suffers from certain disadvantages. First, the phasor diagram say nothing about the initial condition. One can take any arbitrary value of \(t\) (say, \(t_1\), as done throughout this chapter) and draw different phasors which show the relative angle between different phasors. The solution so obtained is called the steady-state solution. This is not a general solution. Additionally, we do have a transient solution which exists even for \(v=0\). The general solution is the sum of the transient solution and the steady-state solution. After a sufficiently long time, the effects of the transient solution die out and the behaviour of the circuit is described by the steady-state solution.

7.6.2 Resonance

An interesting characteristic of the series \(R L C\) circuit is the phenomenon of resonance. The phenomenon of resonance is common among systems that have a tendency to oscillate at a particular frequency. This frequency is called the system’s natural frequency. If such a system is driven by an energy source at a frequency that is near the natural frequency, the amplitude of oscillation is found to be large. A familiar example of this phenomenon is a child on a swing. The swing has a natural frequency for swinging back and forth like a pendulum. If the child pulls on the rope at regular intervals and the frequency of the pulls is almost the same as the frequency of swinging, the amplitude of the swinging will be large (Chapter 13, Class XI).

For an \(R L C\) circuit driven with voltage of amplitude \(v_m\) and frequency \(\omega\), we found that the current amplitude is given by
\(
i_m=\frac{v_m}{Z}=\frac{v_m}{\sqrt{R^2+\left(X_C-X_L\right)^2}}
\)
with \(X_c=1 / \omega C\) and \(X_L=\omega L\). So if \(\omega\) is varied, then at a particular frequency \(\omega_0, X_c=X_L\), and the impedance is minimum \(\left(Z=\sqrt{R^2+0^2}=R\right)\). This frequency is called the resonant frequency:
\(
X_c=X_L \text { or } \frac{1}{\omega_0 C}=\omega_0 L
\)
\(
\text { or } \quad \omega_0=\frac{1}{\sqrt{L C}} \dots(7.28)
\)
At resonant frequency, the current amplitude is maximum; \(i_m=v_m / R\).

Figure 7.14 shows the variation of \(i_m\) with \(\omega\) in a \(R L C\) series circuit with \(L=1.00 \mathrm{mH}, C=\) 1.00 nF for two values of \(R\) : (i) \(R=100 \Omega\) and (ii) \(R=200 \Omega\). For the source applied \(v_m=100 \mathrm{~V} . \omega_0\) for this case is \(\frac{1}{\sqrt{L C}}=1.00 \times 10^6\) \(\mathrm{rad} / \mathrm{s}\).

We see that the current amplitude is maximum at the resonant frequency. Since \(i_m=\) \(v_m / R\) at resonance, the current amplitude for case (i) is twice to that for case (ii).

Resonant circuits have a variety of applications, for example, in the tuning mechanism of a radio or a TV set. The antenna of a radio accepts signals from many broadcasting stations. The signals picked up in the antenna acts as a source in the tuning circuit of the radio, so the circuit can be driven at many frequencies. But to hear one particular radio station, we tune the radio. In tuning, we vary the capacitance of a capacitor in the tuning circuit such that the resonant frequency of the circuit becomes nearly equal to the frequency of the radio signal received. When this happens, the amplitude of the current with the frequency of the signal of the particular radio station in the circuit is maximum.

It is important to note that resonance phenomenon is exhibited by a circuit only if both \(L\) and \(C\) are present in the circuit. Only then do the voltages across \(L\) and \(C\) cancel each other (both being out of phase) and the current amplitude is \(v_m / R\), the total source voltage appearing across \(R\). This means that we cannot have resonance in a \(R L\) or \(R C\) circuit.

Example 7.6: A resistor of \(200 \Omega\) and a capacitor of \(15.0 \mu \mathrm{~F}\) are connected in series to a \(220 \mathrm{~V}, 50 \mathrm{~Hz}\) ac source. (a) Calculate the current in the circuit; (b) Calculate the voltage (rms) across the resistor and the capacitor. Is the algebraic sum of these voltages more than the source voltage? If yes, resolve the paradox.

Solution: 

\(
\begin{aligned}
&\text { Given }\\
&\begin{aligned}
& R=200 \Omega, C=15.0 \mu \mathrm{~F}=15.0 \times 10^{-6} \mathrm{~F} \\
& V=220 \mathrm{~V}, \nu=50 \mathrm{~Hz}
\end{aligned}
\end{aligned}
\)
(a) In order to calculate the current, we need the impedance of the circuit. It is
\(
\begin{aligned}
Z & =\sqrt{R^2+X_C^2}=\sqrt{R^2+(2 \pi \nu C)^{-2}} \\
& =\sqrt{(200 \Omega)^2+\left(2 \times 3.14 \times 50 \times 15.0 \times 10^{-6} \mathrm{~F}\right)^{-2}} \\
& =\sqrt{(200 \Omega)^2+(212.3 \Omega)^2} \\
& =291.67 \Omega
\end{aligned}
\)
Therefore, the current in the circuit is
\(
I=\frac{V}{Z}=\frac{220 \mathrm{~V}}{291.5 \Omega}=0.755 \mathrm{~A}
\)
(b) Since the current is the same throughout the circuit, we have
\(
\begin{aligned}
& V_R=I R=(0.755 \mathrm{~A})(200 \Omega)=151 \mathrm{~V} \\
& V_C=I X_C=(0.755 \mathrm{~A})(212.3 \Omega)=160.3 \mathrm{~V}
\end{aligned}
\)
The algebraic sum of the two voltages, \(V_R\) and \(V_C\) is 311.3 V which is more than the source voltage of 220 V . How to resolve this paradox? As you have learnt in the text, the two voltages are not in the same phase. Therefore, they cannot be added like ordinary numbers. The two voltages are out of phase by ninety degrees. Therefore, the total of these voltages must be obtained using the Pythagorean theorem:
\(
\begin{aligned}
V_{R+C} & =\sqrt{V_R^2+V_C^2} \\
& =220 \mathrm{~V}
\end{aligned}
\)
Thus, if the phase difference between two voltages is properly taken into account, the total voltage across the resistor and the capacitor is equal to the voltage of the source.