Stress

When a body is subjected to a deforming force, a restoring force is developed in the body. This restoring force is equal in magnitude but opposite in direction to the applied force. The restoring force per unit area is known as stress.

If \(F\) is the force applied normal to the cross-section and \(A\) is the area of cross-section of the body,

Magnitude of the stress \(=F / A \dots(9.1)\)

The SI unit of stress is \(\mathrm{N} \mathrm{m}^{-2}\) or pascal (Pa) and its dimensional formula is \(\left[\mathrm{ML}^{-1} \mathrm{~T}^{-2}\right]\).

Types of Stress: Stress is of three types.

• Linear Stress (longitudinal or tensile stress or compressive stress): It is the stress developed when the applied force produces a change in the length of the body. The restoring force per unit area in this case is called tensile stress.
• Volume stress (or Bulk stress): It is the stress developed in the body when the applied force produces a change in the volume of the body.
• Shearing Stress (or tangential stress): It is the stress developed in the body, when the applied force produces, a change in shape of the body.

Example 1: A load of \(4.0 \mathrm{~kg}\) is suspended from a ceiling through a steel wire of radius \(2.0 \mathrm{~mm}\). Find the tensile stress developed in the wire when equilibrium is achieved. Take \(g=3.1 \pi \mathrm{m} \mathrm{s}^{-2}\)

Solution:

Tension in the wire is
\(
F=4 \cdot 0 \times 3.1 \pi \mathrm{N} .
\)
The area of cross-section is
\(
\begin{aligned}
A & =\pi r^2=\pi \times\left(2.0 \times 10^{-3} \mathrm{~m}\right)^2 \\
& =4.0 \pi \times 10^{-6} \mathrm{~m}^2 .
\end{aligned}
\)
Thus, the tensile stress developed
\(
\begin{aligned}
& =\frac{F}{A}=\frac{4.0 \times 3.1 \pi}{4 \cdot 0 \pi \times 10^{-6}} \mathrm{~N} \mathrm{~m}^{-2} \\
& =3 \cdot 1 \times 10^6 \mathrm{~N} \mathrm{~m}^{-2}
\end{aligned}
\)

Strain

The deforming force applied on a body produces generally a change in its dimensions and the body is said to be strained.

Strain is defined as the ratio of change in dimension to the original dimension.

Strain \(=\frac{\text { Change in dimension }}{\text { Original dimension }}\)

Strain has no unit and dimension.

Types of Strain:

• Longitudinal Strain: If the deforming force produces a change in length, the strain produced in the body is called longitudinal strain or tensile strain or linear strain.
  \(
  \begin{aligned}
  \text { Longitudinal Strain } & =\frac{\text { Change in length }}{\text { Original length }} \\
  & =\frac{\Delta L}{L} \dots(9.2)
  \end{aligned}
  \)

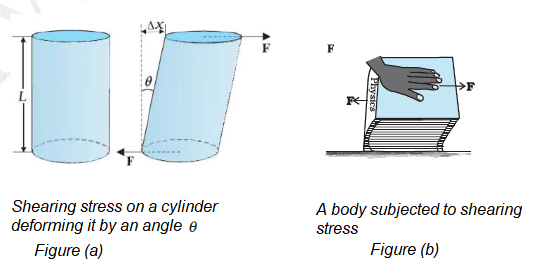
• Shearing Strain: If the deforming force produces a change in the shape of the body without changing volume, the strain produced is called shearing strain.

  Shearing Strain \(=\frac{\Delta x}{L}=\tan \theta \dots(9.3)\)

where \(\theta\) is the angular displacement of the cylinder from the vertical (original position of the cylinder). Usually \(\theta\) is very small, \(\tan \theta\) is nearly equal to angle \(\theta\), (if \(\theta=10^{\circ}\), for example, there is only \(1 \%\) difference between \(\theta\) and \(\tan \theta\) ).

It can also be visualised, when a book is pressed with the hand and pushed horizontally, as shown in Figure (b).

Thus, shearing strain \(=\tan \theta \approx \theta \dots(9.4)\)

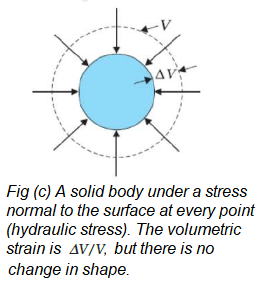
• Volume Strain: The strain produced by a hydraulic pressure is called volume strain and is defined as the ratio of change in volume \((\Delta V)\) to the original volume \((V)\).

  Volume strain \(=\frac{\Delta V}{V} \dots(9.5)\)

  Since the strain is a ratio of change in dimension to the original dimension, it has no units or dimensional formula.