8.3 Electromagnetic Waves

8.3.1 Sources of electromagnetic waves

How are electromagnetic waves produced? Neither stationary charges nor charges in uniform motion (steady currents) can be sources of electromagnetic waves. The former produces only electrostatic fields, while the latter produces magnetic fields that, however, do not vary with time. It is an important result of Maxwell’s theory that accelerated charges radiate electromagnetic waves. The proof of this basic result is beyond the scope of this book, but we can accept it on the basis of rough, qualitative reasoning. Consider a charge oscillating with some frequency. (An oscillating charge is an example of accelerating charge.) This produces an oscillating electric field in space, which produces an oscillating magnetic field, which in turn, is a source of oscillating electric field, and so on. The oscillating electric and magnetic fields thus regenerate each other, so to speak, as the wave propagates through the space. The frequency of the electromagnetic wave naturally equals the frequency of oscillation of the charge. The energy associated with the propagating wave comes at the expense of the energy of the source – the accelerated charge.

From the preceding discussion, it might appear easy to test the prediction that light is an electromagnetic wave. We might think that all we needed to do was to set up an ac circuit in which the current oscillate at the frequency of visible light, say, yellow light. But, alas, that is not possible. The frequency of yellow light is about \(6 \times 10^{14} \mathrm{~Hz}\), while the frequency that we get even with modern electronic circuits is hardly about \(10^{11} \mathrm{~Hz}\). This is why the experimental demonstration of electromagnetic wave had to come in the low frequency region (the radio wave region), as in the Hertz’s experiment (1887).

Hertz’s successful experimental test of Maxwell’s theory created a sensation and sparked off other important works in this field. Two important achievements in this connection deserve mention. Seven years after Hertz, Jagdish Chandra Bose, working at Calcutta (now Kolkata), succeeded in producing and observing electromagnetic waves of much shorter wavelength ( 25 mm to 5 mm ). His experiment, like that of Hertz’s, was confined to the laboratory.

At around the same time, Guglielmo Marconi in Italy followed Hertz’s work and succeeded in transmitting electromagnetic waves over distances of many kilometres. Marconi’s experiment marks the beginning of the field of communication using electromagnetic waves.

8.3.2 Nature of electromagnetic waves

It can be shown from Maxwell’s equations that electric and magnetic fields in an electromagnetic wave are perpendicular to each other, and to the direction of propagation. It appears reasonable, say from our discussion of the displacement current. Consider Fig. 8.2. The electric field inside the plates of the capacitor is directed perpendicular to the plates. The magnetic field this gives rise to via the displacement current is along the perimeter of a circle parallel to the capacitor plates. So \(\mathbf{B}\) and \(\mathbf{E}\) are perpendicular in this case. This is a general feature.

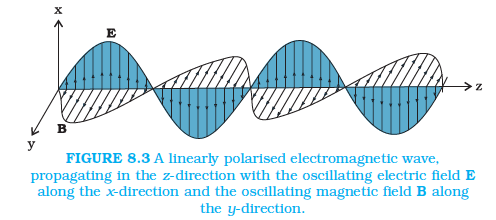
In Fig. 8.3, we show a typical example of a plane electromagnetic wave propagating along the \(z\) direction (the fields are shown as a function of the \(z\) coordinate, at a given time \(t\) ). The electric field \(E_x\) is along the \(x\)-axis, and varies sinusoidally with \(z\), at a given time. The magnetic field \(B_y\) is along the \(y\)-axis, and again varies sinusoidally with \(z\). The electric and magnetic fields \(E_x\) and \(B_y\) are perpendicular to each other, and to the direction \(z\) of propagation. We can write \(E_x\) and \(B_y\) as follows:
\(
\begin{aligned}
& E_x=E_0 \sin (k z-\omega t) \dots[8.7(a)]\\
& B_y=B_0 \sin (k z-\omega t) \dots[8.7(b)]
\end{aligned}
\)
Here \(k\) is related to the wave length \(\lambda\) of the wave by the usual equation
\(
k=\frac{2 \pi}{\lambda} \dots(8.8)
\)
and \(\omega\) is the angular frequency. \(k\) is the magnitude of the wave vector (or propagation vector) \(\mathbf{k}\) and its direction describes the direction of propagation of the wave. The speed of propagation of the wave is \((\omega / k)\). Using Eqs. [8.7(a) and (b)] for \(E_x\) and \(B_y\) and Maxwell’s equations, one finds that
\(
\omega=c k, \text { where, } \mathrm{c}=1 / \sqrt{\mu_0 \varepsilon_0} \dots[8.9(a)]
\)
The relation \(\omega=c k\) is the standard one for waves (see for example, Section 15.4 of class XI Physics textbook). This relation is often written in terms of frequency, \(v(=\omega / 2 \pi)\) and wavelength, \(\lambda(=2 \pi / k)\) as
\(
\begin{aligned}
& 2 \pi v=c\left(\frac{2 \pi}{\lambda}\right) \text { or } \\
& v \lambda=c \dots[8.9(b)]
\end{aligned}
\)
It is also seen from Maxwell’s equations that the magnitude of the electric and the magnetic fields in an electromagnetic wave are related as
\(
B_0=\left(E_0 / c\right) \dots(8.10)
\)
We here make remarks on some features of electromagnetic waves. They are self-sustaining oscillations of electric and magnetic fields in free space, or vacuum. They differ from all the other waves we have studied so far, in respect that no material medium is involved in the vibrations of the electric and magnetic fields.

But what if a material medium is actually there? We know that light, an electromagnetic wave, does propagate through glass, for example. We have seen earlier that the total electric and magnetic fields inside a medium are described in terms of a permittivity \(\varepsilon\) and a magnetic permeability \(\mu\) (these describe the factors by which the total fields differ from the external fields). These replace \(\varepsilon_0\) and \(\mu_0\) in the description to electric and magnetic fields in Maxwell’s equations with the result that in a material medium of permittivity \(\varepsilon\) and magnetic permeability \(\mu\), the velocity of light becomes,
\(
v=\frac{1}{\sqrt{\mu \varepsilon}} \dots(8.11)
\)
Thus, the velocity of light depends on electric and magnetic properties of the medium. We shall see in the next chapter that the refractive index of one medium with respect to the other is equal to the ratio of velocities of light in the two media.

The velocity of electromagnetic waves in free space or vacuum is an important fundamental constant. It has been shown by experiments on electromagnetic waves of different wavelengths that this velocity is the same (independent of wavelength) to within a few metres per second, out of a value of \(3 \times 10^8 \mathrm{~m} / \mathrm{s}\). The constancy of the velocity of em waves in vacuum is so strongly supported by experiments and the actual value is so well known now that this is used to define a standard of length.

The great technological importance of electromagnetic waves stems from their capability to carry energy from one place to another. The radio and TV signals from broadcasting stations carry energy. Light carries energy from the sun to the earth, thus making life possible on the earth.

Example 8.1: A plane electromagnetic wave of frequency 25 MHz travels in free space along the \(x\)-direction. At a particular point in space and time, \(\mathbf{E}=6.3 \hat{\mathbf{j}} \mathrm{~V} / \mathrm{m}\). What is \(\mathbf{B}\) at this point?

Solution: Using Eq. (8.10), the magnitude of \(\mathbf{B}\) is
\(
\begin{aligned}
B & =\frac{E}{c} \\
& =\frac{6.3 \mathrm{~V} / \mathrm{m}}{3 \times 10^8 \mathrm{~m} / \mathrm{s}}=2.1 \times 10^{-8} \mathrm{~T}
\end{aligned}
\)
To find the direction, we note that \(\mathbf{E}\) is along \(y\)-direction and the wave propagates along \(x\)-axis. Therefore, \(\mathbf{B}\) should be in a direction perpendicular to both \(x\) – and \(y\)-axes. Using vector algebra, \(\mathbf{E} \times \mathbf{B}\) should be along \(x\)-direction. Since, \((+\hat{\mathbf{j}}) \times(+\hat{\mathbf{k}})=\mathbf{i}, \mathbf{B}\) is along the \(z\)-direction. Thus,
\(
\mathbf{B}=2.1 \times 10^{-8} \mathbf{k} \mathrm{~T}
\)

Example 8.2: The magnetic field in a plane electromagnetic wave is given by \(B_y=\left(2 \times 10^{-7}\right) \mathrm{T} \sin \left(0.5 \times 10^3 x+1.5 \times 10^{11} t\right)\).
(a) What is the wavelength and frequency of the wave?
(b) Write an expression for the electric field.

Solution: (a) Comparing the given equation with
\(
B_y=B_0 \sin \left[2 \pi\left(\frac{x}{\lambda}+\frac{t}{T}\right)\right]
\)
We get, \(\lambda=\frac{2 \pi}{0.5 \times 10^3} \mathrm{~m}=1.26 \mathrm{~cm}\), and \(\quad \frac{1}{T}=v=\left(1.5 \times 10^{11}\right) / 2 \pi=23.9 \mathrm{GHz}\)
(b) \(E_0=B_0 c=2 \times 10^{-7} \mathrm{~T} \times 3 \times 10^8 \mathrm{~m} / \mathrm{s}=6 \times 10^1 \mathrm{~V} / \mathrm{m}\)
The electric field component is perpendicular to the direction of propagation and the direction of magnetic field. Therefore, the electric field component along the \(z\)-axis is obtained as
\(
E_{\mathrm{z}}=60 \sin \left(0.5 \times 10^3 x+1.5 \times 10^{11} t\right) \mathrm{V} / \mathrm{m}
\)