Equilibrium of a particle in mechanics refers to the situation when the net external force on the particle is zero. According to the first law, this means that the particle is either at rest or in uniform motion.

If two forces \(\mathbf{F}_{1}\) and \(\mathbf{F}_{2}\), act on a particle, equilibrium requires
\(
\mathbf{F}_{1}=-\mathbf{F}_{2}
\)
i.e. the two forces on the particle must be equal and opposite. Equilibrium under three concurrent forces \(\mathbf{F}_{1}, \mathbf{F}_{2}\) and \(\mathbf{F}_{3}\) requires that the vector sum of the three forces is zero.
\(
\mathbf{F}_{1}+\mathbf{F}_{2}+\mathbf{F}_{3}=0 \dots (5.3)
\)

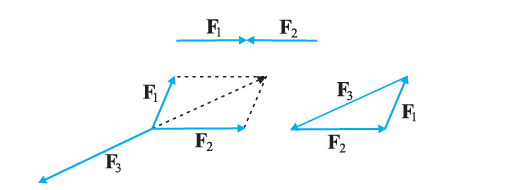Figure 5.3

In other words, the resultant of any two forces say \(\mathbf{F}_{1}\) and \(\mathbf{F}_{2}\), obtained by the parallelogram law of forces must be equal and opposite to the third force, \(\mathbf{F}_{3}\). As seen in Figure 5.3, the three forces in equilibrium can be represented by the sides of a triangle with the vector arrows taken in the same sense. The result can be generalized to any number of forces. A particle is in equilibrium under the action of forces \(\mathbf{F}_{1}\), \(\mathbf{F}_{2}, \ldots \mathbf{F}_{\mathrm{n}}\) if they can be represented by the sides of a closed n-sided polygon with arrows directed in the same sense. Equation (5.3) implies that
\(
\begin{aligned}
&F_{1 x}+F_{2 x}+F_{3 x}=0 \\
&F_{1 y}+F_{2 y}+F_{3 y}=0 \\
&F_{1 z}+F_{2 z}+F_{3 z}=0
\end{aligned}
\)
where \(F_{1 x}, F_{1 y}\) and \(F_{1 z}\) are the components of \(F_{1}\) along \(x, y\) and \(z\) directions respectively.

Example 5.11: See Fig. 5.8. A mass of \(6 \mathrm{~kg}\) is suspended by a rope of length \(2 \mathrm{~m}\) from the ceiling. A force of \(50 \mathrm{~N}\) in the horizontal direction is applied at the midpoint P of the rope, as shown. What is the angle the rope makes with the vertical in equilibrium? (Take \(g=10 \mathrm{~m} \mathrm{~s}^{-2}\) ). Neglect the mass of the rope.

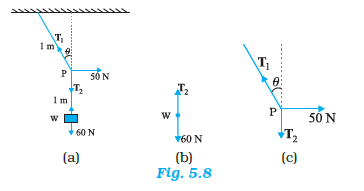
Answer: Figures 5.8(b) and 5.8(c) are known as free-body diagrams. Figure 5.8(b) is the free-body diagram of \(\mathrm{W}\) and Fig. 5.8(c) is the free-body diagram of point \(P\).
Consider the equilibrium of the weight W. Clearly, \(T_2=6 \times 10=60 \mathrm{~N}\).
Consider the equilibrium of the point \(\mathrm{P}\) under the action of three forces – the tensions \(T_1\) and \(T_2\), and the horizontal force \(50 \mathrm{~N}\). The horizontal and vertical components of the resultant force must vanish separately :
\(
\begin{aligned}
& T_1 \cos \theta=T_2=60 \mathrm{~N} \\
& T_1 \sin \theta=50 \mathrm{~N}
\end{aligned}
\)
which gives that
\(
\tan \theta=\frac{5}{6} \text { or } \theta=\tan ^{-1}\left(\frac{5}{6}\right)=40^{\circ}
\)
Note the answer does not depend on the length of the rope (assumed massless) nor on the point at which the horizontal force is applied.

Previous Topic

Back to Lesson

Next Topic