3.4 Ohm’s law

A basic law regarding flow of currents was discovered by G.S. Ohm in 1828, long before the physical mechanism responsible for flow of currents was discovered. Imagine a conductor through which a current \(I\) is flowing and let \(V\) be the potential difference between the ends of the conductor.

Then Ohm’s law states that: If the physical conditions of the conductor (length, temperature, mechanical strain etc.), remains same, then the current flowing through the conductor is directly proportional to the potential difference across it’s two ends i.e.,
\(
\begin{gathered}
V \propto I \\
\text { or, } V=R I \dots(3.3)
\end{gathered}
\)
where the constant of proportionality \(R\) is called the resistance of the conductor. The SI units of resistance is ohm, and is denoted by the symbol \(\Omega\). The resistance \(R\) not only depends on the material of the conductor but also on the dimensions of the conductor. The dependence of \(R\) on the dimensions of the conductor can easily be determined as follows.

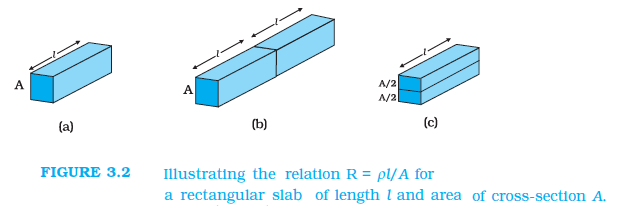
Consider a conductor satisfying Eq. (3.3) to be in the form of a slab of length \(l\) and cross-sectional area \(A\) [Fig. 3.2(a)]. Imagine placing two such identical slabs side by side [Fig. 3.2(b)], so that the length of the combination is \(2 l\). The current flowing through the combination is the same as that flowing through either of the slabs. If \(V\) is the potential difference across the ends of the first slab, then \(V\) is also the potential difference across the ends of the second slab since the second slab is identical to the first and the same current \(I\) flows through both. The potential difference across the ends of the combination is clearly the sum of the potential difference across the two individual slabs and hence equals \(2 V\). The current through the combination is \(I\) and the resistance of the combination \(R_{ C }\) is [from Eq. (3.3)],
\(
R_C=\frac{2 V}{I}=2 R \dots(3.4)
\)
since \(V / I=R\), the resistance of either of the slabs.

Thus, doubling the length of a conductor doubles the resistance. In general, then resistance is proportional to length,
\(
R \propto l \dots(3.5)
\)
Next, imagine dividing the slab into two by cutting it lengthwise so that the slab can be considered as a combination of two identical slabs of length \(l\), but each having a cross-sectional area of \(A / 2\) [Fig. 3.2(c)].

For a given voltage \(V\) across the slab, if \(I\) is the current through the entire slab, then clearly the current flowing through each of the two half-slabs is \(I / 2\). Since the potential difference across the ends of the half-slabs is \(V\), i.e., the same as across the full slab, the resistance of each of the half-slabs \(R_1\) is
\(
R_1=\frac{V}{(I / 2)}=2 \frac{V}{I}=2 R \dots(3.6)
\)
Thus, halving the area of the cross-section of a conductor doubles the resistance. In general, then the resistance \(R\) is inversely proportional to the cross-sectional area,
\(
R \propto \frac{1}{A} \dots(3.7)
\)
Combining Eqs. (3.5) and (3.7), we have
\(
R \propto \frac{l}{A} \dots(3.8)
\)
and hence for a given conductor
\(
R=\rho \frac{l}{A} \dots(3.9)
\)
where the constant of proportionality \(\rho\) depends on the material of the conductor but not on its dimensions. \(\rho\) is called resistivity.
Using the last equation, Ohm’s law reads
\(
V=I \times R=\frac{I \rho l}{A} \dots(3.10)
\)
Current per unit area (taken normal to the current), \(I / A\), is called current density and is denoted by \(J\). The SI units of the current density are \(A / m ^2\).

If \(E\) is the magnitude of the uniform electric field in the conductor whose length is \(l\), then the potential difference \(V\) across its ends is \(E l\). Using these, the eqn(3.10)
\(
V=I \times R=\frac{I \rho l}{A}=J {\rho l} \text { as } J=I / A
\)
\(
\begin{aligned}
E l & =J \rho l \\
\text { or, } \quad E & =J \rho \dots(3.11)
\end{aligned}
\)
The above relation for magnitudes \(E\) and \(J\) can indeed be cast in a vector form. The current density, (which we have defined as the current through unit area normal to the current) is also directed along \(\vec E\), and is also a vector \(\vec J\). Thus, the last equation can be written as,
\(
\begin{aligned}
\vec E = \vec J \rho \dots(3.12) \\
\text { or, } \vec J =\sigma \vec E \dots(3.13)
\end{aligned}
\)
where \(\sigma \equiv 1 / \rho\) is called the conductivity and depends on the material of the conductor and its temperature. Ohm’s law is often stated in an equivalent form, Eq. (3.13) in addition to Eq.(3.3). In the next section, we will try to understand the origin of the Ohm’s law as arising from the characteristics of the drift of electrons.

Current Density (J)

Current is a macroscopic quantity and deals with the overall rate of flow of charge through a section. To specify the current with direction in the microscopic level at a point, the term current density is introduced. Current density at any point inside a conductor is defined as a vector having magnitude equal to current per unit area surrounding that point. Remember area is normal to the direction of charge flow (or current passes) through that point.

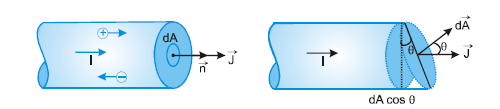
Current density at point \(P\) is given by \(\vec{J}=\frac{d I}{d A} \vec{n}\) as current flows normal to the surface of the conductor.
If the cross-sectional area is not normal to the current, but makes an angle \(\theta\) with the direction of the current then
\(
J =\frac{ dI }{ dA \cos \theta} \Rightarrow dI = JdA \cos \theta=\overrightarrow{ J } \cdot d \overrightarrow{ A } \Rightarrow I =\int \overrightarrow{ J } \cdot \overrightarrow{ dA }
\)
Current density \(\vec{J}\) is a vector quantity. Its direction is the same as that of \(\vec{E}\). Its S.I. unit is ampere \(/ m ^2\) and dimension \(\left[ L ^{-2} A\right]\).

Example 4: The current density at a point is \(\overrightarrow{ J }=\left(2 \times 10^4 \tilde{ j }\right) Jm ^{-2}\).
Find the rate of charge flow through a cross-sectional area \(\overrightarrow{ S }=(2 \tilde{ i }+3 \tilde{ j }) cm ^2\)

Solution: The rate of flow of charge = current = \(I=\int \vec{J} \cdot d \vec{S} \Rightarrow I=\vec{J} \cdot \vec{S}=\left(2 \times 10^4\right)[\tilde{j} \cdot(2 \tilde{i}+3 \tilde{j})] \times 10^{-4} A=6 A\)

Example 5: A potential difference applied to the ends of a wire made up of an alloy drives a current through it. The current density varies as \(J =3+2 r\), where r is the distance of the point from the axis. If R be the radius of the wire, then the total current through any cross-section of the wire.

Solution: Consider a circular strip of radius \(r\) and thickness \(dr\)

\(
d I=\vec{J} \cdot d \vec{S}=(3+2 r)(2 \pi r d r) \cos 0^{\circ}=2 \pi\left(3 r+2 r^2\right) d r
\)
\(
I =\int_0^{ R } 2 \pi\left(3 r +2 r ^2\right) dr =2 \pi\left(\frac{3 r^2}{2}+\frac{2}{3} r^3\right)_0^R=2 \pi\left(\frac{3 R ^2}{2}+\frac{2 R ^3}{3}\right) \text { units }
\)

Relation between Current density, Conductivity and Electric field

Let the number of free electrons per unit volume in a conductor \(= n\)

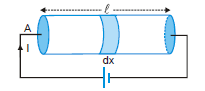
Total number of electrons in dx distance \(= n ( Adx )\)
Total charge \(d Q=n(A d x) e\)
Current \(I =\frac{ dQ }{ dt }= nA e \frac{ dx }{ dt }= neAv _{ d } \quad\)

Current density \(J =\frac{ I }{ A }= nev _{ d }, \quad \text { where } {v}_d \text { is the drift velocity of the free electrons }\)
 \(
=n e\left(\frac{e E}{m}\right) \tau \quad \because v_d=\left(\frac{e E}{m}\right) \tau \Rightarrow J=\left(\frac{n e^2 \tau}{m}\right) E \Rightarrow J=\sigma E \text {, where conductivity } \sigma=\frac{n e^2 \tau}{m}
\)
\(\sigma\) depends only on the material of the conductor and its temperature.
In vector form \(\overrightarrow{ J }=\sigma \overrightarrow{ E }\) Ohm’s law (at microscopic level)

Ohm’s Law V-I Characteristics

If the physical conditions of the conductor (length, temperature, mechanical strain, etc). remains the same, then the current flowing through the conductor is directly proportional to the potential difference across its two ends i.e., \(I \propto V \Rightarrow V = IR\) where R is a proportionality constant, known as electric resistance.

• Ohm’s law is not a universal law, the substances, which obey Ohm’s law are known as ohmic substance,
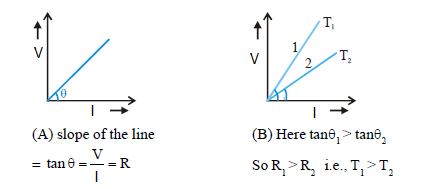
• The graph between V and I for a metallic conductor is a straight line (linear) as shown below. At different temperatures V-I curves are different.

       

Resistance

• Definition: The property of substance by virtue of which it opposes the flow of current through it, is known as the resistance. In other words, the resistance of a conductor is the opposition to the flow of charge which the conductor offers. When a potential difference is applied across a conductor, free electrons get accelerated and collide with positive ions, and their motion is thus opposed. This opposition offered by the ions is called the resistance of the conductor.
• Unit and Dimension: It’s S.I. unit is Volt/amp. or Ohm \((\Omega)\). Also ohm \(=\frac{1 volt }{1 Amp }=\frac{10^8 emu \text { of potential }}{10^{-1} emu \text { of current }}=10^9 emu\) of resistance. It’s dimension is \(\left[ ML ^2 T^{-3} A^{-2}\right]\)
• Formula of resistance: For a conductor if \(l=\) length of a conductor, \(A =\) Area of cross-section of conductor, \(n =\) No. of free electrons per unit volume in conductor, \(\tau=\) relaxation time then resistance of conductor \(R =\rho \frac{l}{A}=\frac{ m }{ ne ^2 \tau} \cdot \frac{l}{A}\) where \(\rho=\) resistivity of the material of conductor.

Dependence of resistance

Resistance of a conductor depends upon the following factors.

• Length of the conductor: Resistance of a conductor is directly proportional to its length i.e \(R \propto l\)
• Inversely proportional to its area of cross-section i.e., \(R \propto \frac{1}{A}\)
• Nature of material of the conductor \(R=\frac{\rho \ell}{A}\)
• Resistance corresponding to temperature difference ( \(\Delta T )\) is given as \(R _t= R _0(1+\alpha \Delta T )\)
  Where \(\quad R _{ t }=\) Resistance at \(t^{\circ} C , R _0=\) Resistance at \(0^{\circ} C\)
  \(\Delta T =\) Change in temperature, \(\alpha=\) Temperature coefficient of resistance
  [For metals : \(\alpha\) is positive but for semiconductors and insulators : \(\alpha\) is negative]
• Unit of \(\alpha\) is \(\frac{1}{{ }^{\circ} C }\)
• Resistance of the conductor decreases linearly with decrease in temperature and becomes zero at a specific temperature. This temperature is called critical temperature. Below this temperature, a conductor becomes a superconductor.

Resistivity ( \(\rho\) ), Conductivity ( \(\sigma\) ) and Conductance ( \(C\) or \(G\))

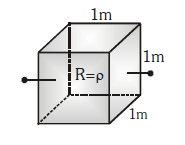
Resistivity 

\(\rho=R A / \ell\), if \(\ell=1\) units, \(A=1 m^2\) units then \(\rho=R\) units
The specific resistance of a material is equal to the resistance of a wire of that material with unit cross-sectional area and unit length.

• Unit and dimension: It’s S.I. unit ohm \(\times m\) and dimension is \(\left[ ML ^3 T^{-3} A^{-2}\right]\)
• It’s formula : \(\rho=\frac{m}{n e^2 \tau}\)
• Resistivity is the intrinsic property of the substance. It is independent of the shape and size of the body (i.e., \(l\) and \(A\) ).
• Resistivity depends on the temperature. For metal \(\rho_t=\rho_0(1+\alpha \Delta t)\) i.e., resistivity increases with temperature.
• Resistivity increases with impurity and mechanical stress.
• Magnetic field increases the resistivity of all metal except iron, cobalt, and nickel.
• Resistivity of certain substance like selenium, cadmium, sulphide is inversely proportional to the intensity of light falling upon them.
• Resistivity depends on (i) Nature of the material (ii) Temperature of the material
• Resistivity does not depend on the size and shape of the material because it is a characteristic property of the conducting material.

Conductivity

Conductivity is the reciprocal of resistivity \( \quad \sigma=\frac{1}{\rho}\) with unit \(mho / m\) and dimensions \(\left[ M ^{-1} L^3 T^{-3} A^2\right]
\)

Conductance 

Reciprocal of resistance is known as conductance. \(C=\frac{1}{R}\). It’s unit is \(\frac{1}{\Omega}\) or \(\Omega^{-1}\) or “siemen”.

Stretching of wire

If a conducting wire stretches, its length increases, area of cross-section decreases so resistance increases but volume remains constant.
Suppose for a conducting wire before stretching its length \(=\ell_1\), area of cross-section \(=A_1\) radius \(=r_1\)
diameter \(= d _1\), and resistance \(R _1=\rho \frac{\ell_1}{A_1}\)

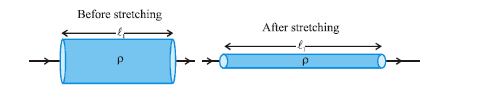
Volume remains constant i.e., \(A _1 \ell_1=\)
After stretching length \(\ell_2\) area of cross-section \(= A _2\) radius diameter \(= d _2\) and resistance \(= R _2=\rho \frac{\ell_2}{A_2}\)
The ratio of resistance before and after stretching
\(
\frac{ R _1}{ R _2}=\frac{\ell_1}{\ell_2} \times \frac{ A _2}{A_1}=\left(\frac{\ell_1}{\ell_2}\right)^2=\left(\frac{ r _2}{ r _1}\right)^4=\left(\frac{ d _2}{d_1}\right)^4
\)

• If length is given by \(R \propto \ell^2 \Rightarrow \frac{ R _1}{ R _2}=\left(\frac{\ell_1}{\ell_2}\right)^2\). That means, if a wire is stretched to n times of it’s original dength, its new resistance will be \(n ^2\) times.
• If radius is given then \(R \propto \frac{1}{r_4} \Rightarrow \frac{R_1}{R_2}=\left(\frac{r_2}{r_1}\right)^4\). That means if a wire is stretched such that its radius is reduced to \(\frac{1}{n}\) th of its original value, then its new resistance will increase to \(n ^4\) times; similarly resistance will decrease to \(n ^4\) times if radius is increased to \(n\) times by contraction.

Golden Points

• 1 ampere of current means the flow of \(6.25 \times 10^{18}\) electrons per second through any cross-section of the conductor.
• Current is a scalar quantity but current density is a vector quantity.
• Order of free electron density in conductors \(=10^{28}\) electrons \(/ m ^3\)
• \(
  \begin{array}{|c|c|c|l|c|}
  \hline \text { Terms } & \begin{array}{c}
  \text { Thermal speed } \\
  v_T
  \end{array} & \text { Mean free path } & \text { Relaxation time } & \begin{array}{c}
  \text { Drift speed } \\
  v_{ d }
  \end{array} \\
  \hline \text { Order } & 10^5 m / s & 10 Å & 10^{-14} m / s & 10^{-4} m / s \\
  \hline
  \end{array}
  \)
• If a steady current flows in a metallic conductor of non uniform cross section.
  (a) Along the wire \(I\) is the same.
  (b) Current density and drift velocity depends on area
  \(
  I _1= I _2, A_1< A _2 \Rightarrow J _1> J _2, E _1> E _2, v _{ d _1}> V _{ d _2}
  \)

         

• If the temperature of the conductor increases, the amplitude of the vibrations of the positive ions in the conductor also increase. Due to this, the free electrons collide more frequently with the vibrating ions and as a result, the average relaxation time decreases.
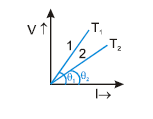
• At different temperatures V-I curves are different.
  Here \(\tan \theta_1>\tan \theta_2 \quad\) , So \(R_1>R_2 \quad\) i.e. \(T_1>T_2\)

       

• In general :
  • \(\rho_{\text {alloy }}>\rho_{\text {semiconductor }}>\rho_{\text {conductor }}\)
  • Temperature coefficients of alloys are lower than pure metals.
  • Resistance of most of non metals decrease with increase in temperature. (e.g. carbon)
  • The resistivity of an insulator (e.g. amber) is greater than a metal by a factor of \(10^{22}\).
• The temperature coefficient ( \(\alpha\) ) of semiconductor including carbon (graphite), insulator, and electrolytes is negative.

Example 6: What will be the number of electrons passing through a heater wire in one minute, if it carries a current of 8 A?

Solution:
\(
I =\frac{ N e}{ t } \Rightarrow N =\frac{ It }{ e }=\frac{8 \times 60}{1.6 \times 10^{-19}}=3 \times 10^{21} \text { electrons }
\)

Example 7: An electron moves in a circle of radius 10 cm with a constant speed of \(4 \times 10^6 m / s\). Find the electric current at a point on the circle.

Solution: Consider a point A on the circle. The electron crosses this point once in every revolution. The number of revolutions made by electron in one second is
\(
n =\frac{ v }{2 \pi r }=\frac{4 \times 10^6}{2 \pi \times 10 \times 10^{-2}}=\frac{2}{\pi} \times 10^7 rotation / s
\)
\(
\therefore \text { Current } \quad I=\frac{n e}{t}=\frac{2}{\pi} \times 10^7 \times 1.6 \times 10^{-19} \quad(\because t =1 s .)=\frac{3.2}{\pi} \times 10^{-12} \cong 1 \times 10^{-12} A
\)

Example 8: A current of 1.34 A exists in a copper wire of cross-section \(1.0 ~mm^2\). Assuming each copper atom contributes one free electron. Calculate the drift speed of the free electrons in the wire. The density of copper is \(8990 ~kg / m ^3\) and atomic mass \(=63.50\).

Solution: Mass of \(1 ~m^3\) volume of the copper is \(=8990 kg=8990 \times 10^3 g\)
Number of moles in \(1 m^3=\frac{8990 \times 10^3}{63.5}=1.4 \times 10^5\)
Since each mole contains \(6 \times 10^{23}\) atoms therefore number of atoms in \(1 ~m^3\)
\(
n =\left(1.4 \times 10^5\right) \times\left(6 \times 10^{23}\right)=8.4 \times 10^{28}
\)
\(
\because I = neAv _{ d } \quad \therefore v _{ d }=\frac{ I }{ neA }=\frac{1.34}{8.4 \times 10^{28} \times 1.6 \times 10^{-19} \times 10^{-6}}=10^{-4} ~m / s =0.1 ~mm / s \left(\because 1 ~mm^2=10^{-6} ~m^2\right)
\)

Example 9: The current through a wire depends on time as \(i=(2+3 t)\) A. Calculate the charge crossed through a cross-section of the wire in 10 s.

Solution:
\(
\begin{aligned}
& I =\frac{ dq }{ dt } \Rightarrow dq =(2+3 t ) dt \Rightarrow \int_0^{10} dq =\int_0^{10}(2+3 t ) dt \Rightarrow q =\left(2 t +\frac{3 t ^2}{2}\right)_0^{10} \\
& q =2 t +\frac{3}{2} \times 100=20+150=170 C
\end{aligned}
\)

Example 10: Figure shows a conductor of length \(\ell\) carrying current I and having a circular cross-section. The radius of cross-section varies linearly from \(a\) to \(b\). Assuming that \((b-a) \ll \ell\). Calculate current density at distance \(x\) from the left end.

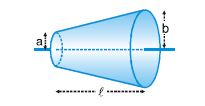
Solution: Since the radius at left end is a and that of right end is \(b\), therefore increase in radius over length \(\ell\) is \((b-a)\). Hence rate of increase of radius per unit length \(=\left(\frac{b-a}{\ell}\right)\) Increase in radius over length \(x=\left(\frac{b-a}{\ell}\right) x\)
Since radius at left end is a so radius at distance \(x , r = a +\left(\frac{ b – a }{\ell}\right) x\)
Area at this particular section \(A=\pi r^2=\pi\left[a+\left(\frac{b-a}{\ell}\right) x\right]^2\)
Hence current density \(J=\frac{I}{A}=\frac{I}{\pi r^2}=\frac{I}{\pi\left[a+\frac{x(b-a)}{\ell}\right]^2}\)

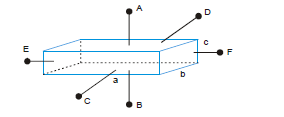
Example 11: The dimensions of a conductor of specific resistance \(\rho\) are shown below. Find the resistance of the conductor across \(AB, CD\) and EF.

Solution: 

\(
R =\frac{\rho \ell}{ A }=\frac{\text { Resistivity } \times \text { length }}{\text { Area of cross section }}
\)
\(
R_{A B}=\frac{\rho c}{a b}, R_{C D}=\frac{\rho b}{a c}, R_{E F}=\frac{\rho a}{b c}
\)

Example 12: If a wire is stretched to double its length, find the new resistance if original resistance of the wire was \(R\).

Solution: As we know that \(R=\frac{\rho \ell}{A}\)
in case \(R^{\prime}=\frac{\rho \ell^{\prime}}{A^{\prime}} \quad \Rightarrow \quad \ell^{\prime}=2 \ell\)
\(A ^{\prime} \ell^{\prime}= A \ell \quad\) (volume of the wire remains constant)
\(
A^{\prime}=\frac{A}{2} \Rightarrow \quad R^{\prime}=\frac{\rho \times 2 \ell}{A / 2}=4 \frac{\rho \ell}{A}=4 R
\)

Example 13: The wire is stretched to increase the length by \(1 \%\) find the percentage change in the Resistance.

Solution: As we known that \(R =\frac{\rho \ell}{ A }\)
\(
\frac{\Delta R }{ R }=\frac{\Delta \rho}{\rho}+\frac{\Delta \ell}{\ell}-\frac{\Delta A }{ A } \text { and } \frac{\Delta \ell}{\ell}=-\frac{\Delta A }{ A }
\)
\(
\frac{\Delta R }{ R }=0+1+1=2
\)
Hence percentage increase in the Resistance \(=2 \%\)

Example 14:The resistance of a thin silver wire is \(1.0 \Omega\) at \(20^{\circ} C\). The wire is placed in a liquid bath and its resistance rises to \(1.2 \Omega\). What is the temperature of the bath? \(\alpha\) for silver is \(3.8 \times 10^{-3} /{ }^{\circ} C\).

Solution: \(R(T)=R_0\left[1+\alpha\left(T-T_0\right)\right]\)
Here, \(R(T)=1.2 \Omega, \quad R_0=1.0 \Omega, \quad \alpha=3.8 \times 10^{-3} /{ }^{\circ} C\) and \(T_0=20^{\circ} C\)
Substituting the values, we have \(1.2=1.0\left[1+3.8 \times 10^{-3}(T-20)\right]\)
\(
\begin{aligned}
3.8 \times 10^{-3}(T-20) & =0.2 \\
T & =72.6^{\circ} C
\end{aligned}
\)

Example 15: A conductive wire has resistance of 10 ohm at \(0^{\circ} C\), and \(\alpha\) is \(\frac{1}{273} /{ }^{\circ} C\), then determine its resistance at \(273^{\circ} C\).

Solution: In such a problem, term \(\alpha \Delta T\) will have a larger value so could not be used directly in \(R=R_0(1+\alpha \Delta T )\). We need to go for basics as
As we know that \(\alpha=\frac{ dR }{ RdT }\)
\(
\begin{array}{llll}
\Rightarrow & \int \frac{d R}{R}=\int \alpha d T & \Rightarrow & \ell n \frac{R_2}{R_1}=\alpha\left(T_2-T_1\right) \\
\Rightarrow & R_2=R_1 e^{\alpha\left(T_2-T_1\right)} & \Rightarrow & R_2=10 e ^1 \\
\Rightarrow & R_2=10 e \Omega
\end{array}
\)

Example 16: An electric current flows along an insulated strip PQ of a metallic conductor. The current density in the strip varies as shown in the graph. Which one of the following statements could explain this variation?
(A) The strip is narrower at P than at Q
(B) The strip is narrower at Q than at P
(C) The potential gradient along the strip is uniform
(D) The resistance per unit length of the strip is constant.

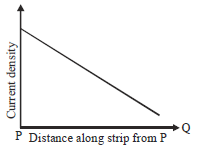
Solution: (Ans-A) The current density at \(P\) is higher than at \(Q\). For the same current flowing through the metallic conductor \(P Q\), the cross-sectional area at \(P\) is narrower than at \(Q\). The resistance per unit length \(r\) is given by \(r=\frac{\rho}{A}\) where \(\rho\) is the resistivity and \(A\) is the cross-sectional area of the conductor PQ. Thus, \(r\) is inversely proportional to the cross-sectional area A of the conductor.