3.3 Electric Currents in Conductors
An electric charge will experience a force if an electric field is applied. If it is free to move, it will thus move contributing to a current. In atoms and molecules, the negatively charged electrons and the positively charged nuclei are bound to each other and are thus not free to move. Bulk matter is made up of many molecules, a gram of water, for example, contains approximately \(10^{22}\) molecules. These molecules are so closely packed that the electrons are no longer attached to individual nuclei. In some materials, the electrons will still be bound, i.e., they will not accelerate even if an electric field is applied. In other materials, notably metals, some of the electrons are practically free to move within the bulk material. These materials, generally called conductors, develop electric currents in them when an electric field is applied. We will focus only on solid conductors so that the current is carried by the negatively charged electrons in the background of fixed positive ions.
Consider first the case when no electric field is present. The electrons will be moving due to thermal motion during which they collide with the fixed ions. An electron colliding with an ion emerges with the same speed as before the collision. However, the direction of its velocity after the collision is completely random. At a given time, there is no preferential direction for the velocities of the electrons. Thus on the average, the number of electrons travelling in any direction will be equal to the number of electrons travelling in the opposite direction. So, there will be no net electric current.
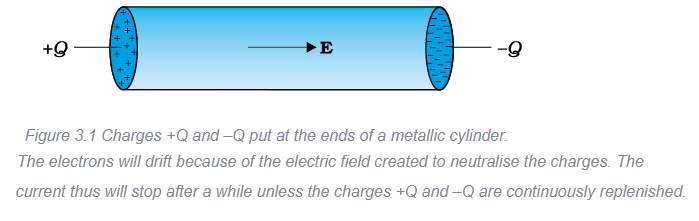
Let us now see what happens to such a piece of conductor if an electric field is applied. To focus our thoughts, imagine the conductor in the shape of a cylinder of radius \(R\) (Fig. 3.1). Suppose we now take two thin circular discs of a dielectric of the same radius and put positive charge \(+Q\) distributed over one disc and similarly \(-Q\) at the other disc. We attach the two discs on the two flat surfaces of the cylinder. An electric field will be created and is directed from the positive towards the negative charge. The electrons will be accelerated due to this field towards \(+Q\). They will thus move to neutralise the charges. The electrons, as long as they are moving, will constitute an electric current. Hence in the situation considered, there will be a current for a very short while and no current thereafter.
We can also imagine a mechanism where the ends of the cylinder are supplied with fresh charges to make up for any charges neutralised by electrons moving inside the conductor. In that case, there will be a steady electric field in the body of the conductor. This will result in a continuous current rather than a current for a short period of time. We shall study the steady current that results from a steady electric field in conductors in the next section.
Golden Points
- Current is a fundamental quantity with dimension \(\left[ M ^0 L^0 T^0 A^1\right]\)
- Current is a scalar quantity with its SI unit ampere.
Ampere: The current through a conductor is said to be one ampere if one coulomb of charge is flowing per second through a cross-section of wire. - The conventional direction of current is the direction of flow of positive charge or applied field. It is opposite to the direction of flow of negatively charged electrons.

- The conductor remains uncharged when current flows through it because the charge entering at one end per second is equal to the charge leaving the other end per second.
- For a given conductor current does not change with change in its cross-section because current is simply rate of flow of charge.
- If \(n\) particles each having a charge \(q\) pass per second per unit area then current associated with cross-sectional area \(A\) is \(I=\frac{\Delta q}{\Delta t}=n q A\).
- If there are \(n\) particles per unit volume each having a charge \(q\) and moving with velocity \(v\) then current through the cross-sectional area \(A\) is \(I=\frac{\Delta q}{\Delta t}=n q v A\)
- If a charge \(q\) is moving in a circle of radius \(r\) with speed \(v\) then its time period is \(T=2 \pi r / v\). The equivalent current \(I =\frac{ q }{ T }=\frac{ qv }{2 \pi r }\).
Behavior of conductor in the absence of applied potential difference
The free electrons present in a conductor gain energy due to the temperature of surroundings and move randomly in the conductor. The average transport and average velocity is zero for a large number of free electrons. There is no flow of current due to thermal motion of free electrons in a conductor. In the absence of applied potential difference, electrons have random motion.
The speed gained by virtue of temperature is called root mean square speed of an electron \(\frac{1}{2} mv _{ rms }^2=\frac{3}{2} kT\), So root mean square speed \(v _{ rms }=\sqrt{\frac{3 kT }{ m }}\) where m is the mass of an electron, \(k =\) Boltzmann’s constant, At room temperature \(T=300 K, v _{ rms }=10^5 m / s\)
Mean free path (\(\lambda)\): The average distance travelled by an electron between two successive collisions is the mean free path.
Mean free path (\(\lambda)\): \(\lambda=\frac{\text { total distance travelled }}{\text { number of collisions }}, (\lambda= 10 Å) \text { in metals }\)
Relaxation time \((\tau)\) : The average time taken by an electron between two successive collisions is the relaxation
time : \(\tau=\frac{\text { total time between two collisions for all the free electrons }}{\text { number of free electrons }},\left(\tau \approx 10^{-14} s\right.\) in metals \()\)
Behavior of conductor in the presence of applied potential difference
When two ends of a conductor are joined to a battery then one end is at higher potential and another at lower potential. This produces an electric field inside the conductor from the point of higher to lower potential \(E =\frac{ V }{ L }\) where \(V = emf\) of the battery, \(L =\) length of the conductor.
The field exerts an electric force on free electrons causing acceleration of each electron.
Acceleration of electron \(\vec{a}=\frac{\vec{F}}{m}=\frac{-e \vec{E}}{m}\)
Thermal speed
Conductors contain a large number of free electrons, which are in continuous random motion. Due to random motion, the free electrons collide with positive metal ions with high frequency and undergo change in direction at each collision. So, the thermal velocities are randomly distributed in all possible directions.
Let \(\vec{u}_1, \vec{u}_2, \ldots \vec{u}_N\) be the individual thermal velocities of the free electrons at any given time.
If the total number of free electrons in the conductor \(= N\)
then average velocity \(\overrightarrow{ u }_{ave}=\frac{\overrightarrow{ u }_1+\overrightarrow{ u }_2+\ldots+\overrightarrow{ u }_{ N }}{ N }=0\)
Note: The average thermal velocity is zero but the average thermal speed is non-zero.
Example 1: What will be the number of electrons passing through a heater wire in one minute, if it carries a current of 8 A ?
Solution:
\(
I =\frac{ ne }{ t } \quad \Rightarrow \quad n =\frac{ It }{ e }=\frac{8 \times 60}{1.6 \times 10^{-19}}=3 \times 10^{21} \text { electrons. }
\)
Example 2: The current through a wire depends on time as \(i=(2+3 t) A\). Calculate the charge crossed through a cross-section of the wire in the first 10 seconds.
Solution:
\(i =\frac{ dq }{ dt } \quad \Rightarrow \quad dq =(2+3 t ) dt\)
\(
\int_0^{ q } dq =\int_0^{10}(2+3 t ) dt \Rightarrow q =\left(2 t +\frac{3 t ^2}{2}\right)_0^{10}=2 \times 10+\frac{3}{2} \times 100=20+150=170 C .
\)
Example 3: Current through a wire decreases linearly from 4 A to zero in 10 s. Calculate the charge flown through the wire during this interval of time.
Solution:
\(\begin{aligned}
\text { Charge flown } & =\text { average current } \times \text { time } \\
& =\left[\frac{4+0}{2}\right] \times 10=20 C
\end{aligned}
\)