12.7 Thermodynamic state variables and equation of state

Every equilibrium state of a thermodynamic system is completely described by specific values of some macroscopic variables, also called state variables. For example, an equilibrium state of a gas is completely specified by the values of pressure, volume, temperature, and mass (and composition if there is a mixture of gases). A thermodynamic system is not always in equilibrium. For example, a gas allowed to expand freely against vacuum is not an equilibrium state [Fig. 12.6(a)]. During the rapid expansion, pressure of the gas may not be uniform throughout.

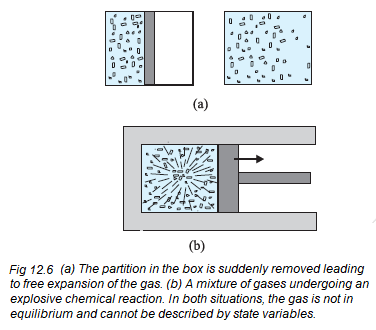
Similarly, a mixture of gases undergoing an explosive chemical reaction (e.g. a mixture of petrol vapour and air when ignited by a spark) is not an equilibrium state; again its temperature and pressure are not uniform [Fig. 12.6(b)]. Eventually, the gas attains a uniform temperature and pressure and comes to thermal and mechanical equilibrium with its surroundings. 

Thermodynamic state variables describe the equilibrium states of systems. The connection between the state variables is called the equation of state. For example, for an ideal gas, the equation of state is the ideal gas relation

For a fixed amount of the gas i.e. given \(\mu\), there are thus, only two independent variables, say \(P\) and \(V\) or \(T\) and \(V\). The pressure-volume curve for a fixed temperature is called an isotherm.

The thermodynamic state variables are of two kinds:

• Extensive: Extensive variables indicate the ‘size’ of the system.
• Intensive: Intensive variables such as pressure and temperature do not. 

To decide which variable is extensive and which intensive, think of a relevant system in equilibrium, and imagine that it is divided into two equal parts. The variables that remain unchanged for each part are intensive. The variables whose values get halved in each part are extensive.

It is easily seen, for example, that internal energy \(U\), volume \(V\), total mass \(M\) are extensive variables. Pressure \(P\), temperature \(T\), and density \(\rho\) are intensive variables. It is a good practice to check the consistency of thermodynamic equations using this classification of variables. For example, in the equation
\(
\Delta Q=\Delta U+P \Delta V
\)
quantities on both sides are extensive (As emphasised earlier, \(Q\) is not a state variable. However, \(\Delta Q\) is clearly proportional to the total mass of system and hence is extensive.). The product of an intensive variable like \(P\) and an extensive quantity \(\Delta V\) is extensive.