Past NEET Entrance Papers

Hint

(b) The electromagnetic radiation with the smallest wavelength among the given options is Gamma rays.

Let’s go through each option to understand more clearly:
Option A: X-rays
X-rays have very short wavelengths, typically in the range of 0.01 to 10 nanometers.
They are used for medical imaging and other applications due to their ability to penetrate materials.

Option B: Gamma rays
Gamma rays have the smallest wavelengths of all electromagnetic radiation, often less than 0.01 nanometers (or 0.1 Å). They are highly energetic and are produced by nuclear reactions, radioactive decay, and other high-energy processes.

Option C: Ultraviolet rays
Ultraviolet (UV) rays have wavelengths ranging from about 10 to 400 nanometers. They are responsible for causing sunburns and are used in various scientific and industrial applications.

Option D: Microwaves
Microwaves have much longer wavelengths, typically ranging from 1 millimeter to 1 meter. They are commonly used in communication technologies and for heating food in microwave ovens.

Based on these explanations, the correct answer is: Gamma rays

Hint

(c)

\(
\begin{aligned}
& \frac{\mu_r}{E_r}=\frac{1}{4} \\
& \frac{E}{H}=\frac{E \mu}{B}=v \mu \\
&=\frac{1}{\sqrt{\mu \varepsilon}} \mu=\sqrt{\frac{\mu}{\varepsilon}}=\sqrt{\frac{\mu_0 \mu_r}{\varepsilon_0 \varepsilon_r}} \\
&=\sqrt{\frac{\mu_0}{\varepsilon_0}} \sqrt{\frac{\mu_r}{\varepsilon_r}} \\
&=120 \pi\left(\frac{1}{2}\right) \\
&=\frac{60 \pi}{1}
\end{aligned}
\)

Hint

(a) Displacement current:
This is a current that appears to flow through a dielectric material (like the gap between the capacitor plates) when the electric field within that material changes.

Charging process:
As the capacitor charges, the electric field between the plates increases, causing a displacement current to flow even though no actual charge carriers are moving through the gap.

Magnitude of displacement current:
This displacement current is equal to the conduction current flowing through the circuit
(i) because the changing electric field within the capacitor is directly related to the rate of charge accumulation on the plates.

Key points to remember:
The displacement current is not a flow of actual charges, but rather a representation of the changing electric field.
The direction of the displacement current is the same as the direction of the conduction current in the circuit.

Hint

(d) Electromagnetic waves have several defining characteristics when they propagate through free space. Let’s evaluate each of the options provided:

Option a: They are transverse in nature.

This is true. Electromagnetic waves are transverse waves, meaning the directions of the electric field and magnetic field oscillations are perpendicular to the direction of wave propagation. The electric field (\(E\)) and magnetic field (\(B\)) vectors are also perpendicular to each other and to the direction of propagation.

Option b: The energy density in the electric field is equal to the energy density in the magnetic field.

This is also true. In electromagnetic waves, the energy density stored in the electric field is equal to the energy density stored in the magnetic field. This is because the magnitudes of the electric and magnetic fields are related by \(c=\frac{E}{B}\) where \(c\) is the speed of light in vacuum. The energy density for each is given by \(\frac{1}{2} \epsilon_0 E^2\) for the electric field and \(\frac{1}{2} \frac{B^2}{\mu_0}\) for the magnetic field. Given the relationship between \(E\) and \(B\) in a wave, these two energy densities are equal.
Option c: They travel with a speed equal to \(\frac{1}{\sqrt{\mu_0 \varepsilon_0}}\).
This statement is true. The speed of electromagnetic waves in vacuum is given by \(c=\frac{1}{\sqrt{\mu_0 \varepsilon_0}}\), where \(\mu_0\) is the magnetic permeability of free space and \(\varepsilon_0\) is the electric permittivity of free space. This relationship derives from Maxwell’s equations in a vacuum.

Option d: They originate from charges moving with uniform speed.

This statement is false. Electromagnetic waves are not generally produced by charges moving with a uniform speed; rather, they are produced by charges that are accelerating. Uniform motion (where velocity is constant and acceleration is zero) does not result in radiation of electromagnetic waves. If a charge is accelerating – changing either the speed or direction of its motion – it emits electromagnetic radiation.

Therefore, the correct answer is Option d, as it is the property that is not true for electromagnetic waves traveling in free space.

Hint

(c)

Direction of propagation of electromagnetic waves is perpendicular to both electric field vector \(\vec{E}\) and magnetic field vector \(\vec{B}\). From right hand screw rule it is in direction of \(\vec{E} \times \vec{B}\) which is shown in figure.

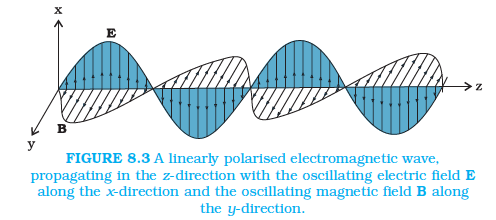
Here electromagnetic wave is in z-direction. Which is given by cross product of \(\vec{E}\) and \(\vec{B}\).

Hint

(d) Gauss law in electrostatic \(\oint \overrightarrow{\mathrm{E}} \cdot \overrightarrow{\mathrm{ds}}=\frac{\mathrm{q}}{\varepsilon_0}\)
Faradays law \(\oint \overrightarrow{\mathrm{E}} \cdot \overrightarrow{\mathrm{dl}}=\frac{\mathrm{d} \phi_B}{\mathrm{dt}}\)
Gauss law in magnetism \(\oint \overrightarrow{\mathrm{B}} \cdot \overrightarrow{\mathrm{dA}}=0\)
Ampere Maxwell law \(\oint \overrightarrow{\mathrm{B}} \cdot \overrightarrow{\mathrm{dl}}=\mu_0 \mathrm{i}_{\mathrm{c}}+\mu_0 \varepsilon_0 \frac{\mathrm{~d} \phi_{\mathrm{E}}}{\mathrm{dt}}\)

Hint

(d) Use the relationship between the amplitudes of the electric and magnetic fields to find the amplitude of the magnetic field.

Step 1: Calculate the amplitude of the magnetic field
Use the formula \(E_0=c B_0\) to find \(B_0\).
Rearrange the formula to solve for \(\boldsymbol{B}_0\) :
\(\boldsymbol{B}_0=\frac{E_0}{c}\)

Substitute the given values:
\(B_0=\frac{48 \mathrm{Vm}^{-1}}{3 \times 10^8 \mathrm{~ms}^{-1}}\)
\(B_0=1.6 \times 10^{-7} \mathrm{~T}\)

Solution: The amplitude of the oscillating magnetic field is \(1.6 \times 10^{-7} \mathrm{~T}\).

Hint

(b) Calculate the refractive index using the given permittivity and permeability of the medium and the relationship between refractive index, permittivity, and permeability.

Step 1: Calculate the speed of light in the medium
The speed of light in the medium is given by:
\(v=\frac{1}{\sqrt{\varepsilon \mu}}\)
\(v=\frac{1}{\sqrt{2 \varepsilon_0 \cdot 1.5 \mu_0}}\)
\(v=\frac{1}{\sqrt{3 \varepsilon_0 \mu_0}}\)

Step 2: Calculate the refractive index of the medium
The refractive index is given by:
\(n=\frac{c}{v}\)
\(n=\frac{\frac{1}{\sqrt{-0 \mu_0}}}{\frac{1}{\sqrt{3 \varepsilon_0 \mu_0}}}\)
\(n=\sqrt{3}\)

Solution: The refractive index of the medium is \(\sqrt{3}\).

Hint

(b) Solve the displacement current formula for \(\frac{d V}{d t}\).

Step 1: Calculate the rate of change of potential difference
Rearrange the formula \(I_d=C \frac{d V}{d t}\) to solve for \(\frac{d V}{d t}\) :
\(\frac{d V}{d t}=\frac{I_d}{C}\)

Substitute the given values:
\(\frac{d V}{d t}=\frac{2 \times 10^{-3} \mathrm{~A}}{4 \times 10^{-6} \mathrm{~F}}\)

Calculate the result: \(\frac{d V}{d t}=500 \mathrm{~V} / \mathrm{s}\)

Solution: The rate of change of potential difference is \(500 \mathrm{~V} / \mathrm{s}\).

Hint

(d) (a) – (ii), (b) – (iii), (c) – (iv), (d) – (i)

Hint

(a) Substitute the expressions for absolute permittivity and permeability into the formula for the speed of light in a medium.

Step 1: Express the velocity of light in a medium
The velocity of light \(v\) in a medium is given by:
\(v=\frac{1}{\sqrt{\varepsilon \mu}}\)

Step 2: Substitute absolute permittivity and permeability
Substitute \(\varepsilon=\varepsilon_r \varepsilon_0\) and \(\mu=\mu_r \mu_0\) into the equation:
\(v=\frac{1}{\sqrt{\varepsilon_r \varepsilon_0 \mu_r \mu_0}}\)
\(v=\frac{1}{\sqrt{\varepsilon_r \mu_r} \sqrt{\varepsilon_0 \mu_0}}\)

Step 3: Simplify using the speed of light in vacuum
Since \(c=\frac{1}{\sqrt{\varepsilon_0 \mu_0}}\), substitute \(c\) into the equation:
\(v=\frac{c}{\sqrt{\varepsilon_r \mu_r}}\)

Solution: The velocity of light in the material medium is \(\frac{c}{\sqrt{\varepsilon_r \mu_r}}\).

Hint

(a) We know that energy in terms of wavelength \(\lambda\), Planck’s constant \(h\) and speed of light \(c\) is given by \(E=\frac{h c}{\lambda}\) From above, \(\Rightarrow E \propto \frac{1}{\lambda}\)

\(\lambda_\gamma \lt \lambda_X \lt \lambda_I \lt \lambda_M\)

Hint

(b) In an electromagnetic wave. the electric field \((E)\) and magnetic field \((B)\) are perpendicular to each other and to the direction of wave propagation. The relationship between the electric field and magnetic field in a vacuum is given by the equation:
\(
E=c B
\)
where \(c\) is the speed of light. Given that the electric field vector is \(3 \hat{\jmath} \mathrm{~V} / \mathrm{m}\) and the wave is moving in the negative \(z\) direction, the magnetic field will be in the negative \(x\) direction (according to the right-hand rule). To find the magnetic field. we can rearrange the equation to find B :
\(
B=\frac{E}{c}
\)
Substituting the values, we have:
\(
B=\frac{3 \hat{\jmath}}{3 \times 10^8}=\frac{1}{10^8} \hat{j}
\)
Since the magnetic field is in the negative \(x\) direction, we have:
\(
B=-\frac{1}{10^8} \hat{i}
\)
Converting this to Tesla, we get:
\(
B=-10 \hat{i} \mathrm{~T}
\)

Alternate:

Use the right-hand rule and the relationship between the magnitudes of the electric and magnetic fields to find the magnetic field vector.

Step 1: Determine the direction of the magnetic field
The wave propagates in the \(-\hat{k}\) direction.
The electric field is in the \(\hat{j}\) direction.
Using the right-hand rule, the magnetic field must be in the \(-\hat{i}\) direction to satisfy \(\overrightarrow{\boldsymbol{E}} \times \overrightarrow{\boldsymbol{B}} \propto-\hat{\boldsymbol{k}}\).

Step 2: Calculate the magnitude of the magnetic field
Use the formula \(\boldsymbol{B}=\frac{\boldsymbol{E}}{\boldsymbol{c}}\).
\(
B=\frac{3 \frac{V}{m}}{3 \times 10^8 \frac{\mathrm{~m}}{\mathrm{~s}}}=10 T
\)

Step 3: Express the magnetic field vector
Combine the direction and magnitude.
\(
\vec{B}=-10 \hat{i} T
\)

Solution: The magnetic field at that point and instant is \(-10 \hat{i} T\).

Hint

(a) For electromagnetic wave,
\(
|\vec{B}|=\frac{|\vec{E}|}{c}
\)
Here \(\vec{B}\) is magnetic field associated with EM wave
\(\vec{E}\) is electric field associated with EM wave
c is the speed of EM wave
\(
\begin{aligned}
& \Rightarrow|\vec{E}|=c|\vec{B}| \\
& =3 \times 10^8 \times 3 \times 10^{-8} \cos \left(1.6 \times 10^3 x+48 \times 10^{10} t\right) \mathrm{V} / \mathrm{m}
\end{aligned}
\)
Direction can be determined from
\(
\text { Poynting vector }=\frac{\vec{E} \times \vec{B}}{\mu_0}
\)
\(
\vec{E}=9 \cos \left(1.6 \times 10^3 x+48 \times 10^{10} t\right) \hat{k} \mathrm{~V} / \mathrm{m}
\)

Hint

(b) We know,
\(
\begin{aligned}
& \frac{E_0}{B_0}(\text { in vacuum })=\mathrm{c} \\
& \therefore \frac{B_0}{E_0}=\frac{1}{c}
\end{aligned}
\)
or, \(\frac{B_0}{E_0}=\sqrt{\mu_0 \varepsilon_0}\)

Hint

(d)

\(
\begin{aligned}
&\text { ( } \vec{E} \times \vec{B} \text { ) gives the direction of propagation }\\
&\begin{aligned}
& \vec{E}=-\hat{j}+\hat{k}, \vec{B}=-\hat{j}-\hat{k} \\
& \vec{E} \times \vec{B}=\left|\begin{array}{ccc}
\hat{i} & \hat{j} & \hat{k} \\
0 & -1 & 1 \\
0 & -1 & -1
\end{array}\right|=2 \hat{i}
\end{aligned}
\end{aligned}
\)

Hint

(c)

\(
I_d=V_o \omega C \cos \omega t
\)
Given \(V=V_0 \sin \omega t\)
Now, displacement current \(i_d\) is given by,
\(
\begin{aligned}
& I_d=C \frac{d V}{d t}=C \frac{d}{d t}\left(V_0 \sin \omega t\right) \\
& =C\left(V_0 \omega\right) \cos \omega t \\
& =V_0 \omega C \cos \omega t
\end{aligned}
\)

Hint

(a) Intensity of electromagnetic wave, \(I=U_{a v} c\)
In terms of electric field, \(U_{a v}=\frac{1}{2} \varepsilon_0 E_0^2\)
In terms of magnetic field, \(U_{a v}=\frac{1}{2} \frac{B_0^2}{\mu_0}\)
Now \(U_{a v}\) (due to the electric field) \(=\frac{1}{2} \varepsilon_0 E_0^2\)
\(
=\frac{1}{2} \varepsilon_0\left(c B_0\right)^2=\frac{1}{2} \varepsilon_0 \times \frac{1}{\mu_0 \varepsilon_0} B_0^2\left(\because \frac{E_0}{B_0}=c\right)
\)
\(
=\frac{1}{2} \frac{B_0^2}{\mu_0}=U_{a v}(\text { due to magnetic field })
\)
Therefore, the ratio of contributions by the electric field and magnetic field components to the intensity of electromagnetic wave is \(1: 1\).

Hint

(b)

\(
\begin{aligned}
&\text { Energy received }=\text { Intensity } \times \text { Area } \times \text { Time }\\
&\begin{aligned}
& I=\frac{E}{A} \\
& E=I A t \\
& =\frac{20}{10^{-4}} \times 20 \times 10^{-4} \times 60 \\
& =24 \times 10^3 J
\end{aligned}
\end{aligned}
\)

Hint

(c) The EM wave with the shortest wavelength among the given options is c . Gamma-rays.

Explanation: Gamma rays have the shortest wavelengths and highest energy on the electromagnetic spectrum, compared to ultraviolet rays, X-rays, and microwaves.

Why other options are incorrect:
Ultraviolet rays: While ultraviolet rays are considered high energy on the electromagnetic spectrum, they have longer wavelengths than X -rays and gamma rays.

X-rays: \(X\)-rays have shorter wavelengths than ultraviolet rays but longer wavelengths than gamma rays.

Microwaves: Microwaves have the longest wavelengths among the given options, situated on the opposite end of the spectrum from gamma rays.

Hint

(b) To solve the problem of finding the wavelength of the electromagnetic wave given by the magnetic field \(B_y=2 \times 10^{-7} \sin \left(\pi \times 10^3 x+3 \pi \times 10^{11} t\right) T\), we can follow these steps:
Step 1: Identify the wave equation format
The magnetic field is given in the form:
\(
B_y=B_0 \sin (k x+\omega t)
\)
where \(B_0\) is the maximum magnetic field, \(k\) is the wave number, and \(\omega\) is the angular frequency.
Step 2: Extract the wave number \(k\)
From the given equation, we can identify the coefficient of \(x\) in the sine function:
\(
k=\pi \times 10^3 \mathrm{~m}^{-1}
\)
Step 3: Relate wave number \(k\) to wavelength \(\lambda\)
The wave number \(k\) is related to the wavelength \(\lambda\) by the formula:
\(
k=\frac{2 \pi}{\lambda}
\)
We can rearrange this to find \(\lambda\) :
\(
\lambda=\frac{2 \pi}{k}
\)
Step 4: Substitute the value of \(k\) into the wavelength formula
Substituting the value of \(k\) :
\(
\lambda=\frac{2 \pi}{\pi \times 10^3}
\)
Step 5: Simplify the expression
The \(\pi\) in the numerator and denominator cancels out:
\(
\lambda=\frac{2}{10^3}=2 \times 10^{-3} \mathrm{~m}
\)
Step 6: Final answer
Thus, the wavelength \(\lambda\) of the electromagnetic wave is:
\(
\lambda=2 \times 10^{-3} \mathrm{mor} 2 \mathrm{~mm}
\)

Hint

(b) Since, Red colour has least frequency. So, red has the longest wavelength among the given colour.
As, \(\nu=c \lambda\)

Hint

(c)
\(
\begin{aligned}
& Q=C V \\
& \frac{d Q}{d t}=i=C \frac{d v}{d t} \\
& =20 \mu F \times \frac{3 V}{s} \\
& =60 \mu \mathrm{~A}
\end{aligned}
\)
For circuit to be completed displacement current should be equal to conduction current.

Hint

(a) Calculate the refractive index of the medium and then calculate the velocity of light in the medium.

Step 1
Calculate the refractive index of the medium
Use the formula \(n=\sqrt{\mu_r \varepsilon_r}\).
Substitute the given values:
\(n=\sqrt{1.0 \times 1.44}\)
\(n=\sqrt{1.44}\)
\(n=1.2\)

Step 2
Calculate the velocity of light in the medium
Use the formula \(\boldsymbol{v}=\frac{\boldsymbol{c}}{\boldsymbol{n}}\).
Substitute the values:
\(v=\frac{3 \times 10^8 \frac{\mathrm{~m}}{\mathrm{~s}}}{1.2}\)
\(v=2.5 \times 10^8 \frac{\mathrm{~m}}{\mathrm{~s}}\)

Solution: The velocity of light in the medium is \(2.5 \times 10^8 \frac{\mathrm{~m}}{\mathrm{~s}}\).

Hint

(b) Velocity of em wave in a medium is given by \(\vec{v}=\vec{E} \times \vec{B}\)
\(
\therefore \quad v \hat{i}=(E \hat{j}) \times(\vec{B}) \quad[\because \vec{E}=E \hat{j} \text { (Given) }]
\)
As \(\hat{i}=\hat{j} \times \hat{k}\), so \(\vec{B}=B \hat{k}\)
Direction of oscillating magnetic field of the em wave will be along \(+z\) direction.

Hint

\(
\begin{aligned}
&\text { (b) Given, } \mathrm{E}_{\mathrm{rms}}=6 \mathrm{~V} / \mathrm{m}\\
&\begin{aligned}
& \frac{E_{\mathrm{rms}}}{B_{\mathrm{rms}}}=c \\
& \Rightarrow B_{\mathrm{rms}}=\frac{E_{\mathrm{rms}}}{c} \dots(i)
\end{aligned}
\end{aligned}
\)
\(
\mathrm{B}_{\mathrm{rms}}=\frac{\mathrm{B}_0}{\sqrt{2}} \Rightarrow \mathrm{~B}_0=\sqrt{2} \mathrm{~B}_{\mathrm{rms}}
\)
\(
B_0=\sqrt{2} \times \frac{E_{\text {rms }}}{c} \text { From equation (i) }
\)
\(
=\frac{\sqrt{2} \times 6}{3 \times 10^8}=2.83 \times 10^{-8} \mathrm{~T}
\)

Hint

(b) An accelerating charge is used to produce oscillating electric and magnetic fields, hence the electromagnetic wave. In other words, to generate electromagnetic waves we need accelerating charge particle.

Hint

(a) Here, \(R=100 \Omega, X_c=100 \Omega\)

Net impedance, \(Z=\sqrt{R^2+X_C^2}=100 \sqrt{2} \Omega\)
Peak value of displacement current \(=\) Maximum conduction current in the circuit
\(
=\frac{\varepsilon_0}{Z}=\frac{220 \sqrt{2}}{100 \sqrt{2}}=2.2 \mathrm{~A}
\)

Hint

(a) The correct answer is \(\mathrm{X}\)-rays.
Explanation:
keV (kiloelectronvolts) is a unit of energy often used to describe the energy of high-energy radiation like X-rays
and gamma rays. X-rays typically have energies ranging from around 100 eV to 100 keV . The given energy of 15 keV falls squarely within this range.

Why other options are incorrect:
Infrared rays:
Infrared radiation has much lower energies than X-rays, typically on the order of 0.001 to 10 eV .

Ultraviolet rays:
While ultraviolet radiation has higher energy than visible light, it is still significantly lower than X-rays. Ultraviolet rays fall in the range of 4 to 100 eV .
\(\gamma\)-rays:
Gamma rays have the highest energies on the electromagnetic spectrum, with energies generally exceeding 100 keV . While 15 keV is a high energy, it is not considered high enough to be classified as a gamma ray.

Hint

(d)

\(
\begin{aligned}
&\text { Momentum transferred to the surface }\\
&\begin{aligned}
& =\text { change in momentum } \\
& =\mathrm{P}_{\mathrm{f}}-\mathrm{P}_{\mathrm{i}} \\
& =+\frac{E}{C}-\left(-\frac{E}{C}\right) \\
& =\frac{2 E}{C}
\end{aligned}
\end{aligned}
\)

Hint

(b) Here, Energy flux, \(I=25 \times 10^4 \mathrm{~W} \mathrm{~m}^{-2}\)
Area, \(A=15 \mathrm{~cm}^2=15 \times 10^{-4} \mathrm{~m}^2\)
Speed of light, \(c=3 \times 10^8 \mathrm{~m} \mathrm{~s}^{-1}\)
For a perfectly reflecting surface, the average force exerted on the surface is
\(\)
\begin{aligned}
F=\frac{2 I A}{c} & =\frac{2 \times 25 \times 10^4 \mathrm{Wm}^{-2} \times 15 \times 10^{-4} \mathrm{~m}^2}{3 \times 10^8 \mathrm{~m} \mathrm{~s}^{-1}} \\
& =250 \times 10^{-8} \mathrm{~N}=2.50 \times 10^{-6} \mathrm{~N}
\end{aligned}
[/latex

Hint

(b) Frequency of electromagnetic wave does not change with change in medium but wavelength and velocity of wave changes with change in medium.
Velocity of electromagnetic wave in vacuum
\(
c=\frac{1}{\sqrt{\mu_0 \epsilon_0}}=\nu \lambda_{\text {vacuum }} \dots(i)
\)
Velocity of electromagnetic wave in the medium
\(
v_{\text {medium }}=\frac{1}{\sqrt{\mu_0 \mu_r \in_0 \epsilon_r}}=\frac{c}{\sqrt{\mu_r \epsilon_r}}
\)
where \(\mu_r[latex] and [latex]\epsilon_r\) be relative permeability and relative permittivity of the medium.
For dielectric medium, \(\mu_r=1\)
\(
\therefore \quad v_{\text {medium }}=\frac{c}{\sqrt{\epsilon_r}} \dots(ii)
\)
Here, \(\epsilon_r=4.0\)
\(
\therefore \quad v_{\text {medium }}=\frac{c}{\sqrt{4}}=\frac{c}{2}
\)
Wavelength of the wave in medium
\(
\lambda_{\text {medium }}=\frac{v_{\text {medium }}}{\nu}=\frac{c}{2 v}=\frac{\lambda_{\text {vacuum }}}{2} \quad \text { (Using (i) and (ii)) }
\)

Hint

(c) In microwave oven, the frequency of the microwaves must match the resonant frequency of water molecules so that energy from the waves is transferred efficiently to the kinetic energy of the molecules.

Note:

A microwave oven heats up a food item containing water molecules most efficiently when the frequency of the microwaves matches the resonant frequency of the water molecules. 

Explanation: When the microwave frequency aligns with the natural vibration frequency of water molecules, it causes them to vibrate with maximum amplitude, generating the most heat energy.

Hint

(b) Step 1: Write the order of penetrating power for alpha, beta, and gamma particles.

\(
\alpha<\beta<\gamma
\)

Step 2: State that for the same energy, the lighter particle has a higher penetrating power. For same energy, the lighter particle has higher penetrating power.

Hint

(a) Compare the given equation with
\(
E=E_0 \cos (k z-\omega t)
\)
we get, \(\omega=6 \times 10^8 \mathrm{~s}^{-1}\)
Wave vector, \(k=\frac{\omega}{c}=\frac{6 \times 10^8 \mathrm{~s}^{-1}}{3 \times 10^8 \mathrm{~m} \mathrm{~s}^{-1}}=2 \mathrm{~m}^{-1}\)

Hint

(b) The amplitude of magnetic field and electric field for an electromagnetic wave propagating in vacuum are related as
\(
E_0=B_0 c
\)
where \(c\) is the speed of light in vacuum.
\(
\therefore \quad \frac{B_0}{E_0}=\frac{1}{c}
\)

Hint

(a) The electromagnetic wave is propagating along the \(+z\) axis.
Since the electric and magnetic fields are perpendicular to each other and also perpendicular to the direction of propagation of wave.
Also, \(\vec{E} \times \vec{B}\) gives the direction of wave propagation.
\(
\therefore \quad \vec{E}=E_0 \hat{i}, B=B_0 \hat{j} \quad(\because \hat{i} \times \hat{j}=\hat{k})
\)

Hint

(a)

The correct answer is A: microwave, infrared, ultraviolet, gamma rays.
Explanation:
In the electromagnetic spectrum, wavelengths decrease from longest to shortest, with gamma rays having the shortest wavelength and radio waves having the longest.

Here’s the order for the given options:
Microwave: Longest wavelength among the listed options
Infrared: Longer than microwaves
Ultraviolet: Shorter than infrared
Gamma rays: Shortest wavelength among the listed options

Hint

\(
\begin{aligned}
&\text { (d) As given }\\
&E=10 \cos \left(10^7 t+k x\right) \dots(i)
\end{aligned}
\)
Comparing it with standard equation of e.m. wave,
\(
E=E_0 \cos (\omega t+k x) \dots(ii)
\)
Amplitude \(E_0=10 \mathrm{~V} / \mathrm{m}\) and \(\omega=10^7 \mathrm{rad} / \mathrm{s}\)
\(
\begin{aligned}
& \because \quad c=v \lambda=\frac{\omega \lambda}{2 \pi} \\
& \text { or } \lambda=\frac{2 \pi c}{\omega}=\frac{2 \pi \times 3 \times 10^8}{10^7}=188.4 \mathrm{~m}
\end{aligned}
\)
Also, \(c=\frac{\omega}{k}\) or \(k=\frac{\omega}{c}=\frac{10^7}{3 \times 10^8}=0.033 \mathrm{~m}^{-1}\)
The wave is propagating along \(-x\) direction.

Hint

(c) In an electromagnetic wave it is observed that electric and magnetic vectors are perpendicular to each other and are perpendicular to the direction of propagation of wave.

Hint

\(
\text { (b) } E_y=2.5 \frac{\mathrm{~N}}{\mathrm{C}}\left[\left(2 \pi \times 10^6 \frac{\mathrm{rad}}{\mathrm{~m}}\right) t-\left(\pi \times 10^{-2} \frac{\mathrm{rad}}{\mathrm{~s}}\right) x\right]
\)
\(
E_z=0 \text { and } E_x=0
\)
The wave is moving in the positive direction of \(x\).
This is the form \(E_y=E_0(\omega t-k x)\)
\(
\begin{aligned}
& \omega=2 \pi \times 10^6 \text { or } 2 \pi \nu=2 \pi \times 10^6 \Rightarrow \nu=10^6 \mathrm{~Hz} \\
& \frac{2 \pi}{\lambda}=k \Rightarrow \frac{2 \pi}{\lambda}=\pi \times 10^{-2} \Rightarrow \lambda=2 \times 10^2=200 \mathrm{~m}
\end{aligned}
\)

Hint

(a) The velocity of electromagnetic radiation in vacuum is \(\frac{1}{\sqrt{\mu_0 \varepsilon_0}}\), where \(\mu_0\) and \(\varepsilon_0\) are the permeability and permittivity of vacuum.

Hint

(c) In electromagnetic wave, electric and magnetic field are in phase and perpendicular to each other and also perpendicular to the direction of the propagation of the wave.

Explanation:

Variation in magnetic field causes electric field and vice-versa.
In electromagnetic waves, \(\vec{E} \perp \vec{B}\). Both \(\vec{E}\) and \(\vec{B}\) are in the same phase.
In electromagnetic waves
\(
\begin{aligned}
& E=E_0 \sin (\omega t-k x) \\
& B=B_0 \sin (\omega t-k x)
\end{aligned}
\)
The electromagnetic waves travel in the direction of \((\vec{E} \times \vec{B})\).

Hint

\(
\begin{aligned}
&\text { (b) We know } E=\frac{h c}{\lambda} \Rightarrow E \propto \frac{1}{\lambda}\\
&\begin{aligned}
& \Rightarrow E_m<E_v<E_x \\
& \therefore \lambda_m>\lambda_v>\lambda_x
\end{aligned}
\end{aligned}
\)

Note: Energy of electromagnetic wave, \(E=\frac{h c}{\lambda}\) For em wave of lower energy, \((v)\) is small and \(\lambda\) is larger. For em wave of higher energy ( \(v\) ) is large and \(\lambda\) is small.

Hint

(a)  The radiation emitted is in the infrared region.
Explanation:
The radiation emitted by the human body is primarily infrared radiation, which is invisible to the human eye. This radiation is a form of thermal radiation emitted by all objects at a non-zero temperature.

Why other options are incorrect:
(b) The radiation is emitted only during the day: The human body emits infrared radiation continuously, day and night.
(c) The radiation is emitted during the summers and absorbed during the winters: While the amount of radiation emitted might vary slightly depending on body temperature, the body always emits infrared radiation, regardless of the season.
(d) The radiation emitted lies in the ultraviolet region and hence is not visible: Infrared radiation is not visible to the human eye, but it is not in the ultraviolet region either. Ultraviolet radiation has a shorter wavelength than infrared radiation and is associated with sunburn and other harmful effects.

Hint

(c) \(\beta\)-rays.

Explanation: \(\beta\)-rays are charged particles, not electromagnetic waves, while \(X\)-rays, \(\gamma\)-rays, and heat rays are all considered forms of electromagnetic radiation.

Key points:
Electromagnetic waves: These are waves that consist of oscillating electric and magnetic fields, traveling through space at the speed of light.

\(\beta \text {-rays. }\): These are high-energy particles, typically electrons or positrons, emitted from radioactive decay.

Hint

(b) According to Maxwell, the electromagnetic waves are those waves in which there are sinusoidal variation of electric and magnetic field vectors at right angles to each other as well as at right angles to the direction of wave propagation.

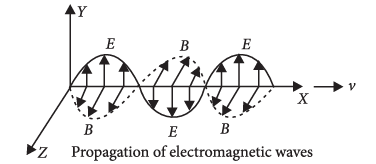
If the electric field \((\vec{E})\) and magnetic field \((\vec{B})\) are vibrating along \(Y\) and \(Z\) direction, propagation of electromagnetic wave will be along the \(X\)-axis. Therefore, the velocity of electromagnetic wave is parallel to \(\vec{E} \times \vec{B}\).

Hint

(b) The greenhouse effect is caused by infrared rays. As the electromagnetic radiations from Sun pass through the atmosphere, some of them are absorbed by it while other reach the surface of earth. The range of wavelength which reaches earth lies in infrared region. This part of the radiation from the sun has shorter wavelength and can penetrate through the layer of gases like \(\mathrm{CO}_2\) and reach earth surface. But the radiation from the earth being of longer wavelength can escape through this layer. As a result the earth surface gets warm. This is known as green house effect.

Explanation: Greenhouse gases in the atmosphere absorb infrared radiation emitted by the Earth’s surface, trapping heat and causing the planet to warm.

Why other options are incorrect:
UItraviolet rays: While the Earth receives ultraviolet radiation from the Sun, this type of radiation is not primarily responsible for the greenhouse effect.

X-rays: X-rays are a type of high-energy radiation that penetrates matter easily, not causing the greenhouse effect.

Radio waves: Radio waves have a very long wavelength and are not involved in the energy transfer that drives the greenhouse effect.

Hint

(c) \(\gamma\)-rays.

Explanation:
Gamma rays have the shortest wavelength of all the listed options.
Why other options are incorrect:
X-rays: While X-rays have a relatively short wavelength, they are longer than gamma rays.
Ultraviolet rays: Ultraviolet rays have even longer wavelengths than X-rays.
Cosmic rays: Although sometimes considered to have the shortest wavelengths due to their high energy, the term “cosmic rays” encompasses a broad range of particles, including high-energy protons and other particles, not just electromagnetic radiation with the shortest wavelength.

Hint

(a) The ozone layer absorbs the harmful ultraviolet rays coming from sun.

Hint

(a) \(\alpha\) rays contain Helium nuclei which contains 2 unit of positive charge.

Hint

(b) \(\gamma\) rays has lowest wavelength and highest frequency among them while ultraviolet ray has highest wavelength and lowest frequency.
Order of frequency: \(b>a>c\)

Hint

(a) Use the formula \(\lambda=\frac{c}{f}\) to calculate the wavelength.
Step 1: Calculate the wavelength
Substitute the given values into the formula:
\(\lambda=\frac{3 \times 10^8 \mathrm{~m} / \mathrm{s}}{10^7 \mathrm{~Hz}}\)
\(\lambda=3 \times 10^{8-7} \mathrm{~m}\)
\(\lambda=3 \times 10^1 \mathrm{~m}\)
\(\lambda=30 \mathrm{~m}\)

Hint

(b)

\(
\lambda=\frac{3 \times 10^8}{100 \mathrm{~Hz}}=3 \times 10^6 \mathrm{~m}
\)

Hint

(d) The range is from 380 nm to even 200 nm to 120 nm .

Hint

(c) A stationary charge produces electric field only; an uniformly moving charge produces localised electromagnetic field; an accelerated charge produces electromagnetic radiations.

Hint

\(
\begin{aligned}
& \text { (b) } c=\frac{1}{\sqrt{\mu_0 \varepsilon_0}} \text { (free space) } \\
& v=\frac{1}{\sqrt{\mu \varepsilon}} \text { (medium) } \therefore \quad \mu=\frac{c}{v}=\sqrt{\frac{\mu \varepsilon}{\mu_0 \varepsilon_0}}
\end{aligned}
\)

Hint

(c) The direction of oscillations of E and B fields are perpendicular to each other as well as to the direction of propagation. So, electromagnetic waves are transverse in nature. The electric and magnetic fields oscillate in same phase.

Hint

(c) Rays Wavelength [Range in m ]
\(X[latex]-rays [latex]1 \times 10^{-11}\) to \(3 \times 10^{-8}\)
\(\gamma\)-rays \(6 \times 10^{-14}\) to \(1 \times 10^{-11}\)
Microwaves \(\quad 10^{-3}\) to 0.3
Radiowaves 10 to \(10^4\)
Wavelength of U.V. Rays ranges from \(6 \times 10^{-8}\) to \(4 \times 10^{-7}\).

Explanation: 

Gamma rays have the smallest wavelength among all electromagnetic radiations. The electromagnetic spectrum arranges different types of radiation based on their wavelength and frequency. Shorter wavelengths correspond to higher frequencies and greater energy. Gamma rays are at the very short end of this spectrum, meaning they have the shortest wavelength and the highest energy of all electromagnetic waves.

Hint

(d) A signal emitted by an antenna can be received as both a “sky wave” and a “ground wave” depending on the frequency and conditions, so the answer is (d) both (a) and (b).

Explanation:
Sky wave: This refers to radio waves that bounce off the ionosphere in the upper atmosphere, allowing long-distance communication beyond the horizon.

Ground wave: This refers to radio waves that travel along the surface of the Earth, typically used for low-frequency signals.

Key point: The type of wave received depends on the frequency of the signal and the propagation conditions.

Hint

(b) The structure of solids is investigated using X-rays.

Explanation:

X-rays have a wavelength that is comparable to the spacing between atoms in a solid, making them ideal for studying the crystal structure of materials.

Hint

(b) Estimate the wavelength of the electromagnetic wave to be of the order of the particle’s radius and then calculate the frequency using the relationship between wavelength, frequency, and the speed of light.

Step 1: Estimate the wavelength
The wavelength should be of the order of the particle’s radius:
\(\lambda \approx r=3 \times 10^{-4} \mathrm{~cm}\)

Step 2: Calculate the frequency
Use the formula \(c=\lambda f\) to find the frequency:
\(f=\frac{c}{\lambda}\)
\(f=\frac{3 \times 10^{10} \mathrm{~cm} / \mathrm{s}}{3 \times 10^{-4} \mathrm{~cm}}\)
\(f=10^{14} \mathrm{~Hz}\)

Solution: The frequency of the electromagnetic wave is of the order of \(10^{14} \mathrm{~Hz}\).

Hint

(d)

How to solve: Compare the wavelengths of the given types of radiation based on their position in the electromagnetic spectrum.

Step 1: Identify the order of wavelengths
List the given radiations from longest to shortest wavelength:
Red light
Blue light
X-rays
Gamma rays

Thus, \(\lambda_{\text {red }}>\lambda_{\text {blue }}>\lambda_{X-\text { rays }}>\lambda_{\gamma}\).
Solution
The longest wavelength is that of red light.

Hint

(d)

\(
\begin{array}{ll}
\text { Radiations } & \text { Wavelength [Range in m] } \\
X \text {-rays } & 1 \times 10^{-11} \text { to } 3 \times 10^{-8} \\
\gamma \text {-rays } & 6 \times 10^{-14} \text { to } 1 \times 10^{-11} \\
\text { Microwaves } & 10^{-3} \text { to } 0.3 \\
\text { Radiowaves } & 10 \text { to } 10^4
\end{array}
\)