Past NEET Entrance Papers
Quiz Summary
0 of 81 Questions completed
Questions:
Information
You have already completed the quiz before. Hence you can not start it again.
Quiz is loading…
You must sign in or sign up to start the quiz.
You must first complete the following:
Results
Results
0 of 81 Questions answered correctly
Your time:
Time has elapsed
You have reached 0 of 0 point(s), (0)
Earned Point(s): 0 of 0, (0)
0 Essay(s) Pending (Possible Point(s): 0)
Categories
- Not categorized 0%
- 1
- 2
- 3
- 4
- 5
- 6
- 7
- 8
- 9
- 10
- 11
- 12
- 13
- 14
- 15
- 16
- 17
- 18
- 19
- 20
- 21
- 22
- 23
- 24
- 25
- 26
- 27
- 28
- 29
- 30
- 31
- 32
- 33
- 34
- 35
- 36
- 37
- 38
- 39
- 40
- 41
- 42
- 43
- 44
- 45
- 46
- 47
- 48
- 49
- 50
- 51
- 52
- 53
- 54
- 55
- 56
- 57
- 58
- 59
- 60
- 61
- 62
- 63
- 64
- 65
- 66
- 67
- 68
- 69
- 70
- 71
- 72
- 73
- 74
- 75
- 76
- 77
- 78
- 79
- 80
- 81
- Current
- Review
- Answered
- Correct
- Incorrect
-
Question 1 of 81
1. Question
In an ideal transformer, the turns ratio is \(\frac{N_p}{N_s}=\frac{1}{2}\). The ratio \(V_s: V_p\) is equal to (the symbols carry their usual meaning): [NEET 2024]
CorrectIncorrectHint
(a) In an ideal transformer, the voltage ratio is related to the turns ratio by the formula:
\(
\frac{V_s}{V_p}=\frac{N_s}{N_p}
\)
Where:
\(V_s\) is the secondary voltage,
\(V_p\) is the primary voltage,
\(N_s\) is the number of turns in the secondary winding,
\(N_p\) is the number of turns in the primary winding.
Given that the turns ratio is \(\frac{N_p}{N_s}=\frac{1}{2}\), this implies:
\(
\frac{N_s}{N_p}=2
\)
Now, using the relationship for the voltage ratio:
\(
\frac{V_s}{V_p}=\frac{N_s}{N_p}=2
\)
Therefore, the voltage ratio \(V_s: V_p\) is \(2: 1\). -
Question 2 of 81
2. Question
A \(10 \mu \mathrm{~F}\) capacitor is connected to a \(210 \mathrm{~V}, 50 \mathrm{~Hz}\) source as shown in the figure. The peak current in the circuit is nearly \((\pi=3.14)\) : [NEET 2024]
 CorrectIncorrect
CorrectIncorrectHint
(a) The capacitive reactance \(X_C\) for a capacitor is given by the formula:
\(
X_C=\frac{1}{2 \pi f C}
\)
Where:
\(f\) is the frequency of the AC source \((50 \mathrm{~Hz})\),
\(C\) is the capacitance \(\left(10 \mu \mathrm{~F}=10 \times 10^{-6} \mathrm{~F}\right)\),
\(\pi \approx 3.14\).
Substituting the values:
\(
X_C=\frac{1}{2 \pi \times 50 \times 10 \times 10^{-6}}=\frac{1000}{3.14}
\)
\(
\begin{aligned}
& V_{\mathrm{rms}}=210 \mathrm{~V} \\
& i_{\mathrm{rms}}=\frac{V_{\mathrm{rms}}}{X_C}=\frac{210}{X_C}
\end{aligned}
\)
\(
\begin{aligned}
& \text { Peak current }=\sqrt{2} i_{\mathrm{rms}}=\sqrt{2} \times \frac{210}{1000} \times 3.14=0.932 \\
& \quad=0.93 \mathrm{~A}
\end{aligned}
\) -
Question 3 of 81
3. Question
\(L, C\) and \(R\) represent the value of inductance, capacitance, and resistance, respectively. The factor which has the same dimensions as that of the inverse of the resonance frequency is: [NEET 2024]
CorrectIncorrectHint
(a) To find the factor with the same dimensions as the inverse of the resonance frequency, let’s first recall the formula for the resonance frequency \(f_0\) of an LC circuit:
\(
f_0=\frac{1}{2 \pi \sqrt{L C}}
\)
The inverse of the resonance frequency \(\frac{1}{f_0}\) would be:
\(
\frac{1}{f_0}=2 \pi \sqrt{L C}
\)
Now, let’s analyze the dimensional consistency:
The resonance frequency \(f_0\) has the dimension of time (specifically, \(T^{-1}\) ).
Therefore, the inverse resonance frequency \(\frac{1}{f_0}\) has the dimension of time (i.e., \(T\) ).
We need to find which of the given factors has the dimension of time \((T)\).
The dimensions of \(L\) (inductance) are \([L]=\mathrm{H}=\mathrm{kg} \cdot \mathrm{m}^2 \cdot \mathrm{~s}^{-2} \cdot \mathrm{~A}^{-2}\).
The dimensions of \(C\) (capacitance) are \([C]=\mathrm{F}=\mathrm{A}^2 \cdot \mathrm{~s}^4 \cdot \mathrm{~kg}^{-1} \cdot \mathrm{~m}^{-2}\).
The dimensions of \(L C\) will be \([L] \cdot[C]=\mathrm{s}^2\), so \(\sqrt{L C}\) has the dimension of time \(T\).
Thus, \(\sqrt{L C}\), has the same dimensions as the inverse of the resonance frequency. -
Question 4 of 81
4. Question
In the circuit shown below, the inductance \(L\) is connected to a source. The current flowing in the circuit is \(I=I_0 \sin \omega t\). The voltage drop ( \(V_L\) ) across \(L\) is: [NEET 2024]
 CorrectIncorrect
CorrectIncorrectHint
(d)
\(
\begin{aligned}
& V_L \text { leads current } I \text { by } \frac{\pi}{2} \\
& \therefore V_L=V_0 \sin \left(\omega t+\frac{\pi}{2}\right) \quad\left(\because I=I_0 \sin \omega t\right) \\
& V_0=I_0 X_L \\
& \Rightarrow V_L=I_0 X_L \cos (\omega t)=I_0 \omega L \cos (\omega t)
\end{aligned}
\) -
Question 5 of 81
5. Question
A step-up transformer is connected to an AC mains supply of 220 V to operate at \(11000 \mathrm{~V}, 88 \mathrm{~W}\). The current in the secondary circuit, ignoring the power loss in the transformer, is: [NEET 2024]
CorrectIncorrectHint
(a) To calculate the current in the secondary circuit of the step-up transformer, we can use the principle of power conservation (assuming no power loss). The power input to the transformer is equal to the power output. Therefore, we can write:
\(
P_p=P_s
\)
Where:
\(P_p\) is the power input (primary side),
\(P_s\) is the power output (secondary side).
The power is given by:
\(
P=V \cdot I
\)
Thus, the input power \(P_p=V_p \cdot I_p\) and the output power \(P_s=V_s \cdot I_s\), where:
\(V_p=220 \mathrm{~V}\) is the primary voltage,
\(V_s=11000 \mathrm{~V}\) is the secondary voltage,
\(I_p\) is the primary current,
\(I_s\) is the secondary current.
Given that the transformer operates at 88 W on the secondary side:
\(
P_s=88 \mathrm{~W}
\)
Thus:
\(
88=11000 \cdot I_s
\)
Solving for \(I_s\) :
\(
I_s=\frac{88}{11000}
\)
Let’s calculate this:
It seems like I can’t do more advanced data analysis right now. Please try again later. However, I can guide you through the calculation.
You can calculate the secondary current \(I_s\) using the formula:
\(
I_s=\frac{P_s}{V_s}=\frac{88 \mathrm{~W}}{11000 \mathrm{~V}}=0.008 \mathrm{~A}=8 \mathrm{~mA}
\) -
Question 6 of 81
6. Question
The amplitude of the charge oscillating in a circuit decreases exponentially as \(Q=Q_0 e^{-R t / 2 L}\), where \(Q_0\) is the charge at \(t=0 \mathrm{~s}\). The time at which charge amplitude decreases to \(0.50 Q_0\) is nearly: [NEET 2024]
[Given that \(R=1.5 \Omega, L=12 \mathrm{mH}, \ln (2)=0.693\) ]CorrectIncorrectHint
(b) The given equation for the charge in the circuit is:
\(
Q(t)=Q_0 e^{-\frac{Rt}{2 L}}
\)
Where:
\(Q_0\) is the initial charge at \(t=0\),
\(R\) is the resistance ( \(1.5 \Omega\) ),
\(L\) is the inductance \(\left(12 \mathrm{mH}=12 \times 10^{-3} \mathrm{H}\right)\),
\(t\) is the time,
\(Q(t)\) is the charge at time \(t\).
We are asked to find the time when the charge amplitude decreases to \(0.50 Q_0\), which means:
\(
Q(t)=0.50 Q_0
\)
Substituting this into the equation for \(Q(t)\) :
\(
0.50 Q_0=Q_0 e^{-\frac{Rt}{2 L}}
\)
Now, take the natural logarithm of both sides:
\(
\ln (0.50)=-\frac{R t}{2 L}
\)
Since \(\ln (0.50)=-\ln (2)\) and \(\ln (2) \approx 0.693\), we get:
\(
-\ln (2)=-\frac{R t}{2 L}
\)
Simplifying:
\(
\ln (2)=\frac{R t}{2 L}
\)
Substitute the given values for \(R\) and \(L\) :
\(
0.693=\frac{1.5 t}{2 \times 12 \times 10^{-3}}
\)
\(
t=0.011088 \text { seconds }=11.09 \mathrm{~ms}
\) -
Question 7 of 81
7. Question
An AC source is connected to a capacitor \(C\). Due to decrease in its operating frequency: [NEET 2023]
CorrectIncorrectHint
When an AC source is connected to a capacitor \(C\), the behavior of the capacitor in the circuit depends on the frequency of the AC signal. The capacitive reactance \(X_C\), which opposes the current flow in the circuit, is given by:
\(
X_C=\frac{1}{2 \pi f C}
\)
Where:
\(X_C\) is the capacitive reactance,
\(f\) is the frequency of the AC source,
\(C\) is the capacitance of the capacitor.Effect of Decrease in Frequency:
When the operating frequency \(f\) decreases, the capacitive reactance \(X_C\) increases because \(X_C\) is inversely proportional to the frequency. As a result, the opposition to current flow in the circuit becomes greater.Current Decreases: Since the current in an AC circuit with a capacitor is related to the voltage and the capacitive reactance by Ohm’s law \(I=\frac{V}{X_C}\), a higher \(X_C\) (due to lower frequency) will cause the current to decrease.
In summary, if the frequency decreases, the capacitive reactance increases, leading to a decrease in current.
-
Question 8 of 81
8. Question
A \(12 \mathrm{~V}, 60 \mathrm{~W}\) lamp is connected to the secondary of a step-down transformer, whose primary is connected to AC mains of 220 V . Assuming the transformer to be ideal, what is the current in the primary winding? [NEET 2023]
CorrectIncorrectHint
(b) To calculate the current in the primary winding of the transformer, we can use the principle of power conservation, assuming the transformer is ideal (no losses).
Given:
The lamp operates at 12 V and 60 W , so the power output \(P_s\) on the secondary side is 60 W .
The primary voltage \(V_p=220 \mathrm{~V}\).
The secondary voltage \(V_s=12 \mathrm{~V}\).
Since the transformer is ideal, the power input \(P_p\) equals the power output \(P_s\) :
\(
P_p=P_s
\)
We can express the power in terms of voltage and current:
\(
P=V \times I
\)
So, the current in the secondary winding \(I_s\) is:
\(
I_s=\frac{P_s}{V_s}=\frac{60}{12}=5 \mathrm{~A}
\)
Now, we use the transformer equation for an ideal transformer, which relates the primary and secondary voltages and currents:
\(
\frac{V_p}{V_s}=\frac{I_s}{I_p}
\)
Rearranging to solve for the primary current \(I_p\) :
\(
I_p=\frac{V_s \cdot I_s}{V_p}
\)
Substituting the known values:
\(
I_p=\frac{12 \times 5}{220}=\frac{60}{220} \approx 0.27 \mathrm{~A}
\)
Thus, the current in the primary winding is approximately: 0.27 A -
Question 9 of 81
9. Question
In a series \(L C R\) circuit, the inductance \(L\) is 10 mH , capacitance \(C\) is \(1 \mu \mathrm{~F}\) and resistance \(R\) is \(100 \Omega\). The frequency at which resonance occurs is: [NEET 2023]
CorrectIncorrectHint
(a) The goal is to find the frequency at which resonance occurs in a series LCR circuit. To achieve resonance, the inductive reactance \(\left(X_L\right)\) should equal the capacitive reactance \(\left(X_C\right)\).
Given are:
Inductance, \(L=10 \mathrm{mH}=10 \times 10^{-3} \mathrm{H}\)
Capacitance, \(C=1 \mu \mathrm{~F}=1 \times 10^{-6} \mathrm{~F}\)
Resistance, \(R=100 \Omega\) (which does not directly impact the resonance frequency)
Resonance occurs when the angular frequency \((\omega)\) meets the condition \(\omega L=\frac{1}{\omega C}\), leading to the formula for calculating the resonance frequency \((f)\) as
\(
f=\frac{\omega}{2 \pi}=\frac{1}{2 \pi \sqrt{L C}}
\)
Substituting the given values:
\(
f=\frac{1}{2 \pi \sqrt{10 \times 10^{-3} \cdot 1 \times 10^{-6}}}=1.59 \mathrm{kHz}
\)
Thus, the frequency at which resonance occurs in this LCR circuit is 1.59 kHz. -
Question 10 of 81
10. Question
The net impedance of the circuit (as shown in the figure) will be: [NEET 2023]
 CorrectIncorrect
CorrectIncorrectHint
(d)
\(
\begin{aligned}
& X_L=2 \pi \mathrm{fL} \\
& =2 \pi \times 50 \times \frac{50}{\pi} \times 10^{-3} \\
& X_L=5 \Omega
\end{aligned}
\)
\(
\begin{aligned}
& \mathrm{X}_{\mathrm{C}}=\frac{1}{2 \pi \mathrm{fC}}=\frac{10^6}{2 \pi \times 50 \times \frac{10^3}{\pi}} \\
& \mathrm{X}_{\mathrm{C}}=10 \Omega \\
& \mathrm{Z}=\sqrt{(10)^2+(10-5)^2} \\
& \mathrm{Z}=\sqrt{125}=5 \sqrt{5} \Omega
\end{aligned}
\) -
Question 11 of 81
11. Question
An AC source is connected to the given circuit. The value of \(\phi\) will be: [NEET 2023]
 CorrectIncorrect
CorrectIncorrectHint
(d) In a \(LR\) circuit, phase difference between current and voltage, is given by tan \(L\)
\(
\begin{aligned}
& \tan \phi=\frac{\mathrm{X}_{\mathrm{L}}}{\mathrm{R}}=\frac{L \omega}{R} \\
& \tan \phi=\frac{1}{\pi} \frac{100 \pi}{100}=1
\end{aligned}
\)
\(\phi=45^{\circ}\) voltage is leading the current. -
Question 12 of 81
12. Question
If \(Z_1\) and \(Z_2\) are the impedances of the given circuits (a) and (b) as shown in the figures, then choose the correct option: [NEET 2023]
 CorrectIncorrect
CorrectIncorrectHint
(a)
\(
\begin{aligned}
& Z_1=\sqrt{X_L^2+R^2} \quad X_L=0 \text { (D.C. circuit) } \\
& Z_1=10 \Omega \\
& Z_2=\sqrt{X_C^2+R^2} \\
& X_C=\frac{1}{2 \pi \times 50 \times \frac{10^3}{\pi} \times 10^{-6}}=10 \Omega \\
& Z_2=\sqrt{(10)^2+(10)^2} \\
& =10 \sqrt{2} \\
& Z_1<Z_2
\end{aligned}
\) -
Question 13 of 81
13. Question
The maximum power is dissipated for an AC in a/an: [NEET 2023]
CorrectIncorrectHint
(a) The maximum power is dissipated for an AC in a purely resistive circuit.
Explanation: In a purely resistive circuit, the voltage and current are in phase, meaning the power factor is 1 , which leads to the maximum power dissipation.Power consumed in an AC circut is given by the formula
\(
\mathrm{P}=\mathrm{E}_{\mathrm{rms}} \cdot \mathrm{I}_{\mathrm{rms}} \cos \phi
\)
For a purely resistive circuit \(\cos \phi=1\) So, power consumption will be maximum. -
Question 14 of 81
14. Question
For very high frequencies, the effective impedance of the circuit (shown in the figure) will be:
 CorrectIncorrect
CorrectIncorrectHint
(c) For very high frequency, \(\omega L\) will be very high, so branch will not conduct current (behaves as open circuit) which has inductance; whereas, at high frequency, \(\frac{1}{\omega \mathrm{C}}\) will be very small, so effective reactance of the capacitor will be zero (behaves as short). So, from the given circuit, total impedance will be \(1+2=3 \Omega\)
-
Question 15 of 81
15. Question
The magnetic energy stored in an inductor of inductance \(4 \mu \mathrm{H}\) carrying a current of 2 A is : [NEET 2023]
CorrectIncorrectHint
(c) The formula for calculating the magnetic energy stored in an inductor is given by:
\(
E=\frac{1}{2} L I^2
\)
where:
\(E\) is the energy stored (in joules, J ),
\(L\) is the inductance of the inductor (in henrys, H ),
\(I\) is the current flowing through the inductor (in amperes, A).
Plugging the given values into the formula:
\(
\begin{aligned}
& E=\frac{1}{2} \times 4 \mu H \times(2 A)^2 \\
& E=\frac{1}{2} \times 4 \times 10^{-6} H \times 4 A^2 \\
& E=2 \times 10^{-6} H \cdot 4 \\
& E=8 \times 10^{-6} J
\end{aligned}
\)
Hence, the energy stored in the inductor is \(8 \mu J\). -
Question 16 of 81
16. Question
The peak voltage of the AC source is equal to: [NEET 2022]
CorrectIncorrectHint
(c) We know,
RMS value of A.C. \(E_{r m s}=\frac{E_0}{\sqrt{2}}\)
\(
E_0=\sqrt{2} E_{r m s}
\) -
Question 17 of 81
17. Question
A series \(L C R\) circuit with inductance 10 H , capacitance \(10 \mu \mathrm{~F}\), resistance \(50 \Omega\) is connected to an AC source of voltage, \(V=200 \sin (100 t)\) volt . If the resonant frequency of the \(L C R\) circuit is \(\nu_0\) and the frequency of the AC source is \(\nu\), then: [NEET 2022]
CorrectIncorrectHint
(c) At resonance,
\(
\begin{aligned}
& \omega L=\frac{1}{\omega C} \\
& \omega=\frac{1}{\sqrt{L C}}=\frac{1}{\sqrt{10 \times 10 \times 10^{-6}}} \\
& \omega=100 \\
& \omega=2 \pi f \Rightarrow f=\frac{\omega}{2 \pi} \\
& \nu_0=f_0=\frac{100}{2 \pi}=\frac{50}{\pi} \mathrm{~Hz}, \omega=100 \\
& \nu=f=\frac{100}{2 \pi}=\frac{50}{\pi}
\end{aligned}
\) -
Question 18 of 81
18. Question
An AC source given by \(V=V_m \sin (\omega t)\) is connected to a pure inductor \(L\) in a circuit and \(I_m\) is the peak value of the AC current. The instantaneous power supplied to the inductor is: [NEET 2022]
CorrectIncorrectHint
(a) To find the instantaneous power supplied to a pure inductor, we need to use the voltage and current expressions. For ar inductor, the voltage and current are out of phase by 90 degrees. Given the voltage \(V=V_m \sin (\omega t)\), the current throug the inductor is \(I=I_m \sin \left(\omega t-\frac{\pi}{2}\right)=I_m \cos (\omega t)\). The instantaneous power \(P(t)\) is given by \(P(t)=V(t) \cdot I(t)\). Substituting the expressions for \(V(t)\) and \(I(t)\). we get \(P(t)=V_m \sin (\omega t) \cdot I_m \cos (\omega t)\). Using the trigonometric identity \(\sin (2 \theta)=\) \(2 \sin (\theta) \cos (\theta)\). we can \(\operatorname{simplify}\) this to \(P(t)=\frac{V_m I_m}{2} \sin (2 \omega t)\).
Step by Step Solution:
Step 1: Write the expressions for voltage and current in the inductor: \(V=V_m \sin (\omega t)\) and \(I=I_m \cos (\omega t)\).Step 2: Express the instantaneous power \(P(t)\) as the product of voltage and current: \(P(t)=V(t) \cdot I(t)\).
Step 3: Substitute the expressions for \(V(t)\) and \(I(t): P(t)=V_m \sin (\omega t) \cdot I_m \cos (\omega t)\).
Step 4: Use the trigonometric identity \(\sin (2 \theta)=2 \sin (\theta) \cos (\theta)\) to simplify: \(P(t)=\frac{V_m I_m}{2} \sin (2 \omega t)\).
-
Question 19 of 81
19. Question
Given below are two statements
Statement I: In an a.c circuit, the current through a capacitor leads the voltage across it.
Statement II: In a.c circuit containing pure capacitance only, the phase difference between the current and voltage is \(\pi\).
In the light of the above statements, choose the most appropriate answer from the options given below [NEET 2022]CorrectIncorrectHint
(d)
\(
\begin{aligned}
&\begin{aligned}
& V=V_0 \sin \omega t \\
& V=\frac{q}{C} \\
& q=C V \sin \omega t \\
& \frac{d q}{d t}=C V \frac{d}{d t} \sin \omega t \\
& i=C V \omega \cos \omega t \\
& i=C V \omega \sin \left(\omega t+\frac{\pi}{2}\right)
\end{aligned}\\
&\text { In a.c circuit current lead by voltage by a phase } \frac{\pi}{2}
\end{aligned}
\)
Thus statement I is correct while II is incorrect. -
Question 20 of 81
20. Question
An inductor of inductance 2 mH is connected to a \(220 \mathrm{~V}, 50 \mathrm{~Hz}\) ac source. Let the inductive reactance in the circuit is \(X_1\). If a 220 V DC source replaces the AC source in the circuit, then the inductive reactance in the circuit is \(X_2 . X_1\) and \(X_2\), respectively, are: [NEET 2022]
CorrectIncorrectHint
(c) We know, for A.C. source
\(
\begin{aligned}
& X_L=\omega L \\
& =2 \pi f(L) \\
& =100 \pi\left(2 \times 10^{-3}\right) \\
& =0.2 \pi \Omega=0.628 \Omega
\end{aligned}
\)
For D.C. source, the inductor behaves as a closed circuit offering no resistance at all (at steady state) as \(\omega=0\) (For D.C.)
\(
\therefore X_L=0 \Omega
\) -
Question 21 of 81
21. Question
A standard filament lamp consumes 100 W when connected to 200 V AC mains supply. The peak current through the bulb will be: [NEET 2022]
CorrectIncorrectHint
(a)
\(
\begin{aligned}
&P_{a v g}=V_{r m s} \times I_{r m s} \times \cos \phi\\
&\text { Here, } \phi=0^{\circ} \text { as current and voltage are in same phase in a resistor. }\\
&\Rightarrow 100=200 \times I_{r m s} \Rightarrow I_{r m s}=\frac{1}{2} \Rightarrow \frac{I_0}{\sqrt{2}}=\frac{1}{2} \Rightarrow I_0=\frac{1}{\sqrt{2}}=0.707 \mathrm{~A}
\end{aligned}
\) -
Question 22 of 81
22. Question
An inductor of inductance \(L\), a capacitor of capacitance \(C\) and a resistor of resistance \(R\) are connected in series to an AC source of potential difference \(V\) volts as shown in Figure. The potential difference across \(L, C\) and \(R\) is \(40 \mathrm{~V}, 10 \mathrm{~V}\) and 40 V , respectively. The amplitude of the current flowing through the \(L C R\) series circuit is \(10 \sqrt{2} \mathrm{~A}\). The impedance of the circuit will be: [NEET 2021]
 CorrectIncorrect
CorrectIncorrectHint
(b)
\(
\begin{aligned}
&{I}_0=10 \sqrt{2} \mathrm{~A}\\
&I_{\mathrm{RMS}}=\frac{I_0}{\sqrt{2}}=10 \mathrm{~A}
\end{aligned}
\)
\(
\begin{aligned}
& V_{R M S}=\sqrt{V_R^2+\left(V_L-V_C\right)^2} \\
& =\sqrt{(40)^2+(40-10)^2} \\
& =50 \mathrm{~V} \\
& \text { Impedance, } Z=\frac{V_{R M S}}{I_{R M}}=\frac{50 V}{10 \mathrm{~V}}=5 \Omega
\end{aligned}
\) -
Question 23 of 81
23. Question
A step-down transformer connected to an AC mains supply of 220 V is made to operate at \(11 \mathrm{~V}, 44 \mathrm{~W}\) lamp. Ignoring power losses in the transformer, what is the current in the primary circuit? [NEET 2021]
CorrectIncorrectHint
(c) For an ideal transformer (no power losses), the input power equals the output power: \(\boldsymbol{P}_{\text {in }}=\boldsymbol{P}_{\text {out }}\).
Power can be calculated using the formula: \(P=V I\), where \(V\) is voltage and \(I\) is current.
Calculate the current in the primary circuit using the power equation and the principle of power conservation in an ideal transformer.Step 1: Calculate the current in the secondary circuit
Use the formula \(P=V_s I_s\) to find \(I_s\).
Rearrange the formula to solve for \(I_s: I_s=\frac{P}{V_s}\).
Substitute the given values: \(I_s=\frac{44 \mathrm{~W}}{11 \mathrm{~V}}\).
Calculate: \(\boldsymbol{I}_s=4 \mathrm{~A}\).Step 2: Calculate the current in the primary circuit
Since power is conserved in an ideal transformer, \(\boldsymbol{P}_{\text {in }}=\boldsymbol{P}_{\text {out }}\).
Therefore, \(V_p I_p=V_s I_s\).
Rearrange the formula to solve for \(I_p: I_p=\frac{V_s I_s}{V_p}\).
Substitute the known values: \(I_p=\frac{11 \mathrm{~V} \cdot 4 \mathrm{~A}}{220 \mathrm{~V}}\).
Calculate: \(I_p=\frac{44}{220} \mathrm{~A}=0.2 \mathrm{~A}\). -
Question 24 of 81
24. Question
A series \(L C R\) circuit containing 5.0 H inductor, \(80 \mu \mathrm{~F}\) capacitor and \(40 \Omega\) resistor is connected to 230 V variable frequency AC source. The angular frequencies of the source at which power transferred to the circuit is half the power at the resonant angular frequency are likely to be: [NEET 2021]
CorrectIncorrectHint
(a)
\(
\begin{aligned}
& Q=\frac{\omega}{\Delta \omega}=\frac{\omega L}{R} \Rightarrow \Delta \omega=R / L=\frac{50}{4}=8 \mathrm{rad} / \mathrm{sec} \\
& \omega_0=\frac{1}{\sqrt{L C}}=\frac{1}{\sqrt{5 \times 80 \times 10^{-6}}}=50 \mathrm{rad} / \mathrm{sec} \\
& \omega_{\min }=\omega_0-\frac{\Delta \omega}{2}=46 \mathrm{rad} / \mathrm{sec} \\
& \omega_{\max }=\omega_0-\frac{\Delta \omega}{2}=54 \mathrm{rad} / \mathrm{sec}
\end{aligned}
\) -
Question 25 of 81
25. Question
A capacitor of capacitance ‘ \(C\) ‘, is connected across an ac source of voltage \(V\) , given by
\(
V=V_0 \sin \omega t
\)
The displacement current between the plates of the capacitor, would then be given by : [NEET 2021]CorrectIncorrectHint
(b) Given, \(\mathrm{V}=\mathrm{V}_0 \sin \omega \mathrm{t}\)
We know, \(q=C V\)
Now displacement current \(I_d\) is given by,
\(
\begin{aligned}
& \mathrm{I}_{\mathrm{d}}=\frac{d q}{d t}=\frac{C d V}{d t} \\
& I_d=C\left(V_0 \omega \cos \omega t\right) \\
& =V_0 \omega C \cos \omega t
\end{aligned}
\) -
Question 26 of 81
26. Question
A \(40 \mu \mathrm{~F}\) capacitor is connected to a \(200 \mathrm{~V}, 50 \mathrm{~Hz}\) AC supply. The RMS value of the current in the circuit is, nearly: [NEET 2020]
CorrectIncorrectHint
(b)
\(
\begin{aligned}
&\mathrm{i}_{\mathrm{rms}}=\mathrm{C} \omega \varepsilon_{\mathrm{rms}}\\
&\mathrm{C}=40 \times 10^{-6} \mathrm{~F}\\
&\omega=2 \pi f=100 \pi\\
&\varepsilon_{\mathrm{rms}}=200 \mathrm{~V}\\
&\begin{aligned}
& \mathrm{i}_{\mathrm{rms}}=200 \times 40 \times 10^{-6} \times 2 \pi \times 50 \\
& =2.5 \mathrm{~A}
\end{aligned}
\end{aligned}
\) -
Question 27 of 81
27. Question
An AC voltage source is connected to a series \(L C R\) circuit. When \(L\) is removed from the circuit, the phase difference between current and voltage is \(\frac{\pi}{3}\). If \(C\) is instead removed from the circuit, the phase difference is again \(\frac{\pi}{3}\) between current and voltage. The power factor of the circuit is: [NEET 2020]
CorrectIncorrectHint
(b) Find the relationship between \(\boldsymbol{X}_{\boldsymbol{L}}, \boldsymbol{X}_{\boldsymbol{C}}\) and \(\boldsymbol{R}\), then calculate the power factor.
Step 1: Find the relationship between \(X_C\) and \(R\)
When \(L\) is removed, \(\tan \phi=\frac{X_C}{R}\).
Given \(\phi=\frac{\pi}{3}\), so \(\tan \frac{\pi}{3}=\frac{X_C}{R}\).
\(
\begin{aligned}
& \tan \frac{\pi}{3}=\sqrt{3}, \text { so } \sqrt{3}=\frac{X_C}{R} . \\
& X_C=R \sqrt{3} .
\end{aligned}
\)
Step 2: Find the relationship between \(X_L\) and \(R\)
When \(C\) is removed, \(\tan \phi=\frac{X_L}{R}\).
Given \(\phi=\frac{\pi}{3}\), so \(\tan \frac{\pi}{3}=\frac{X_L}{R}\).
\(
\begin{aligned}
& \tan \frac{\pi}{3}=\sqrt{3}, \text { so } \sqrt{3}=\frac{X_L}{R} . \\
& X_L=R \sqrt{3} .
\end{aligned}
\)
Step 3: Find the phase difference in the LCR circuit
In the LCR circuit, \(\tan \phi=\frac{X_L-X_C}{R}\).
Substitute \(X_L\) and \(X_C: \tan \phi=\frac{R \sqrt{3}-R \sqrt{3}}{R}\).
\(
\begin{aligned}
& \tan \phi=0 . \\
& \phi=0 .
\end{aligned}
\)
Step 4: Calculate the power factor
Power factor is \(\cos \phi\).
\(
\cos 0=1 .
\)
The power factor of the circuit is 1. -
Question 28 of 81
28. Question
A light bulb and an inductor coil are connected to an AC source through a key as shown in the figure below. The key is closed and after some time an iron rod is inserted into the interior of the inductor. The glow of the light bulb: [NEET 2020]
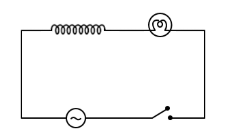 CorrectIncorrect
CorrectIncorrectHint
(a)
\(
L=\frac{\mu_0 N^2\left(\pi r^2\right)}{l}
\)
After inserting the iron rod
\(
\begin{array}{ll}
L_{\text {final }}=\frac{\mu N^2\left(\pi r^2\right)}{l} & \mu>\mu_0 \\
e=-L\left(\frac{d i}{d t}\right) &
\end{array}
\)
As \(L\) increases, emf induced across the inductor will increase leaving less voltage across the bulb. Therefore, the glow of the light bulb decreases. -
Question 29 of 81
29. Question
The variation of EMF with time for four types of generators is shown in the figures. Which amongst them can be called AC voltage? [NEET 2019]
 CorrectIncorrect
CorrectIncorrectHint
(b) For Direct current magnitude and direction remain the same with time. For Alternating current both magnitude and direction change with time.
\(\therefore\) All 4 cases satisfy the condition of AC current. -
Question 30 of 81
30. Question
A circuit when connected to an AC source of 12 V gives a current of 0.2 A . The same circuit when connected to a DC source of 12 V , gives a current of 0.4 A . The circuit is: [NEET 2019]
CorrectIncorrectHint
(a) In a circuit with an AC source, RMS current is given as:
\(I_{\mathrm{rms}}=\mathrm{V}_{\mathrm{rms}} / \mathrm{Z} \dots(1)\)
where, \(\mathrm{V}_{\text {rms }}=\) potential of AC source, \(\mathrm{Z}=\) impedance
Also, \(\mathrm{Z}=\sqrt{R^2+\left(X_L-X_C\right)^2} \dots(2)\)
In a circuit with a DC source, the current is defined as:
\(
\mathrm{I}=\mathrm{V} / \mathrm{R} \dots(3)
\)
Given:
\(
\mathrm{V}_{\mathrm{ms}}=12 \mathrm{~V}, \mathrm{I}_{\mathrm{rms}}=0.2 \mathrm{~A}, \mathrm{~V}=12 \mathrm{~V}, \mathrm{I}=0.4 \mathrm{~A}
\)
From equation (1) we get,
\(
Z=12 / 0.2=60 \Omega
\)
And from equation (3) we get,
\(
R=12 / 0.4=30 \Omega
\)
\(\because\) There is current in the circuit at a steady state (DC source) hence capacitor cannot be in series with Resistance.
Also, since \(Z>R\) and from equation (3) we can conclude there must be another component in the series which is nothing but Inductor.
\(\therefore\) It is a series LR circuit. -
Question 31 of 81
31. Question
An inductor of 20 mH , a capacitor of \(100 \mu \mathrm{~F}\), and a resistor of \(50 \Omega\) are connected in series across a source of emf, \(V=10 \sin (314 t)\). What is the power loss in this circuit? [NEET 2018]
CorrectIncorrectHint
(a) Impedance \(Z\) in an ac circuit is
\(Z=\sqrt{R^2+\left(X_C-X_L\right)^2}\); where \(X_C=\) capacitive reactance and \(X_L=\) inductive reactance.
Also \(X_C=\frac{1}{\omega C}\) and \(X_L=\omega L\)
\(
\therefore Z=\sqrt{(50)^2+\left(\frac{1}{314 \times 100 \times 10^{-6}}-314 \times 20 \times 10^{-3}\right)^2}
\)
or \(Z=56 \Omega\)
The power loss in the circuit is \(P_{a v}=\left(\frac{V_{r m s}}{Z}\right)^2 R\)
\(
\therefore \quad P_{a v}=\left(\frac{10}{(\sqrt{2}) 56}\right)^2 \times 50=0.79 \mathrm{~W}
\) -
Question 32 of 81
32. Question
Figure shows a circuit that contains three identical resistors with resistance \(R=9.0 \Omega\) each, two identical inductors with inductance \(L=2.0 \mathrm{mH}\) each, and an ideal battery with \(\operatorname{emf} \varepsilon=18 \mathrm{~V}\). The current \(i\) through the battery just after the switch closed is [NEET 2017]
 CorrectIncorrect
CorrectIncorrectHint
(b)
At time, \(t=0\) i.e., when switch is closed, inductor in the circuit provides very high resistance (open circuit) while capacitor starts charging with maximum current (low resistance).
Equivalent circuit of the given circuit.
Current drawn from battery,
\(
i=\frac{\varepsilon}{(R / 2)}=\frac{2 \varepsilon}{R}=\frac{2 \times 18}{9}=4 \mathrm{~A}
\) -
Question 33 of 81
33. Question
The potential differences across the resistance, capacitance and inductance are \(80 \mathrm{~V}, 40 \mathrm{~V}\) and 100 V respectively in an \(L C R\) circuit. What is the power factor of this circuit? [NEET 2016]
CorrectIncorrectHint
(c) Here, \(V_R=80 \mathrm{~V}, V_C=40 \mathrm{~V}, V_L=100 \mathrm{~V}\)
Power factor, \(\cos \phi=\frac{R}{Z}=\frac{V_R}{V}=\frac{V_R}{\sqrt{V_R^2+\left(V_L-V_C\right)^2}}\)
\(=\frac{80}{\sqrt{(80)^2+(100-40)^2}}=\frac{80}{100}=0.8\) -
Question 34 of 81
34. Question
A \(100 \Omega\) resistance and a capacitor of \(100 \Omega\) reactance are connected in series across a 220 V source. When the capacitor is \(50 \%\) charged, the peak value of the displacement current is [NEET 2016]
CorrectIncorrectHint
(a) Peak voltage: \(V_0=V_{r m s} \sqrt{2}\)
Impedance in a series RC circuit: \(\boldsymbol{Z}=\sqrt{R^2+X_C^2}\)
Peak current: \(I_0=\frac{V_0}{Z}\)
The displacement current is equal to the conduction current in a series RC circuit.
\(
\begin{aligned}
& Z=\sqrt{R^2+X_C^2} \\
& Z=\sqrt{(100 \Omega)^2+(100 \Omega)^2} \\
& Z=\sqrt{10000 \Omega^2+10000 \Omega^2} \\
& Z=\sqrt{20000 \Omega^2} \\
& Z=100 \sqrt{2} \Omega
\end{aligned}
\)
Use the formula for peak voltage
\(V_0=V_{r m s} \sqrt{2}\)
\(V_0=220 \mathrm{~V} \cdot \sqrt{2}\)
\(V_0=220 \sqrt{2} V\)
Use the formula for peak current:
\(I_0=\frac{V_0}{Z}\)
\(I_0=\frac{220 \sqrt{2} V}{100 \sqrt{2} \Omega}\)
\(I_0=\frac{220}{100} A\)
\(I_0=2.2 \mathrm{~A}\)
The peak displacement current is equal to the peak current:
\(I_{d 0}=I_0\)
\(I_{d 0}=2.2 \mathrm{~A}\)
The peak value of the displacement current is 2.2 A. -
Question 35 of 81
35. Question
A small-signal voltage \(\mathrm{V}(\mathrm{t})=\mathrm{V}_0\) sin \(\omega t\) is applied across an ideal capacitor \(C\) [NEET 2016]
CorrectIncorrectHint
(a) When an ideal capacitor is connected with an ac voltage source, current leads voltage by \(90^{\circ}\). Since, energy stored in capacitor during charging is spent in maintaining charge on the capacitor during discharging. Hence over a full cycle the capacitor does not consume any energy from the voltage source.
Power, \(\mathrm{P}=\mathrm{V}_{\mathrm{rms}} \mathrm{I}_{\mathrm{rms}} \cos \phi\)
\(\Rightarrow \cos \phi=0\). (as \(\phi=90^{\circ}\) between \(\mathrm{I}_{\mathrm{rms}}\) and \(\mathrm{V}_{\mathrm{rms}}\) across a capacitor)Therefore, power consumed \(=0\) in one complete cycle across a capacitor.
-
Question 36 of 81
36. Question
Which of the following combinations should be selected for better tuning of an \(L C R\) circuit used for communication? [NEET 2016]
CorrectIncorrectHint
(c) Quality factor of an \(L-C-R\) circuit is given by,
\(
\begin{aligned}
& Q=\frac{1}{R} \sqrt{\frac{L}{C}} \\
& Q_1=\frac{1}{20} \sqrt{\frac{1.5}{35 \times 10^{-6}}}=50 \times \sqrt{\frac{3}{70}}=10.35 \\
& Q_2=\frac{1}{25} \times \sqrt{\frac{2.5}{45 \times 10^{-6}}}=40 \times \sqrt{\frac{5}{90}}=9.43 \\
& Q_3=\frac{1}{15} \sqrt{\frac{3.5}{30 \times 10^{-6}}}=\frac{100}{15} \sqrt{\frac{35}{3}}=22.77 \\
& Q_4=\frac{1}{25} \times \sqrt{\frac{1.5}{45 \times 10^{-6}}}=\frac{40}{\sqrt{30}}=7.30
\end{aligned}
\)
Clearly \(Q_3\) is maximum of \(Q_1, Q_2, Q_3\), and \(Q_4\). Hence, option (c) should be selected for better tuning of an \(L-C-R\) circuit. -
Question 37 of 81
37. Question
An inductor 20 mH , a capacitor \(50 \mu \mathrm{~F}\), and a resistor \(40 \Omega\) are connected in series across a source of emf \(V=10 \sin 340 t\). The power loss in the AC circuit is: [NEET 2016]
CorrectIncorrectHint
\(
\begin{aligned}
&\begin{aligned}
& \text { (d) Given: } \mathrm{L}=20 \mathrm{mH} ; \mathrm{C}=50 \mu \mathrm{~F} ; \mathrm{R}=40 \Omega \\
& \mathrm{~V}=10 \sin 340 \mathrm{t} \\
& \therefore \quad \mathrm{~V}_{\text {runs }}=\frac{10}{\sqrt{2}} \\
& \mathrm{X}_{\mathrm{C}}=\frac{1}{\omega \mathrm{C}}=\frac{1}{340 \times 50 \times 10^{-6}}=58.8 \Omega \\
& \mathrm{X}_{\mathrm{L}}=\omega \mathrm{L}=340 \times 20 \times 10^{-3}=6.8 \Omega \\
& \text { Impedance, } \mathrm{Z}=\sqrt{\mathrm{R}^2+\left(\mathrm{X}_{\mathrm{C}}-\mathrm{X}_{\mathrm{L}}\right)^2} \\
& =\sqrt{40^2+(58.8-6.8)^2}=\sqrt{4304} \Omega
\end{aligned}\\
&\text { Power loss in A.C. circuit, }\\
&\begin{aligned}
& \mathrm{P}=\mathrm{i}_{\mathrm{ms}}^2 \mathrm{R}=\left(\frac{\mathrm{V}_{\mathrm{rms}}}{\mathrm{Z}}\right)^2 \mathrm{R} \\
& =\left(\frac{10 / \sqrt{2}}{\sqrt{4304}}\right)^2 \times 40=\frac{50 \times 40}{4304} \simeq 0.51 \mathrm{~W}
\end{aligned}
\end{aligned}
\) -
Question 38 of 81
38. Question
A series \(R C\) circuit is connected to an alternating voltage source. Consider two situations:
(1) When the capacitor is air-filled.
(2) When the capacitor is mica filled.
The current through the resistor is \(i\) and the voltage across the capacitor is \(V\) then: [NEET 2015]CorrectIncorrectHint
(b)
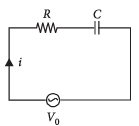
Current through resistor, \(i=\) Current in the circuit
\(
=\frac{V_0}{\sqrt{R^2+X_C^2}}=\frac{V_0}{\sqrt{R^2+(1 / \omega C)^2}}
\)
Voltage across capacitor, \(V=i X_C\)
\(
=\frac{V_0}{\sqrt{R^2+(1 / \omega C)^2}} \times \frac{1}{\omega C}=\frac{V_0}{\sqrt{R^2 \omega^2 C^2+1}}
\)
As \(C_a<C_b\) \(\therefore \quad i_a<i_b\) and \(V_a>V_b\) -
Question 39 of 81
39. Question
A resistance \(\boldsymbol{R}\) draws power \(\boldsymbol{P}\) when connected to an AC source. If an inductance is now placed in series with the resistance, such that the impedance of the circuit becomes \(Z\), the power drawn will be: [NEET 2015]
CorrectIncorrectHint
(a) For pure resistor circuit, power
\(
\begin{aligned}
& \mathrm{P}=\frac{V_{r m s}^2}{R} \\
& \Rightarrow V_{r m s}^2=\mathrm{PR}
\end{aligned}
\)
For L-R series circuit, power
\(
\begin{aligned}
& \mathrm{P}^{\prime}={V}_{\mathrm{rms}} {I}_{\mathrm{rms}} \cos \phi \\
& =V_{r m s} \times \frac{V_{r m s}}{Z} \times \frac{R}{Z} \\
& =\frac{V_{r m s}^2 R}{Z^2}=\frac{P R \times R}{Z^2} \\
& \therefore \mathrm{P}^{\prime}=P\left(\frac{R}{Z}\right)^2
\end{aligned}
\) -
Question 40 of 81
40. Question
A transformer has an efficiency of \(90 \%\) working on 200 V and 3 kW power supply. If the current in the secondary coil is 6 A , the voltage across the secondary coil and the current in the primary coil, respectively, are: [NEET 2014]
CorrectIncorrectHint
(b) Here, Efficiency of the transformer, \(\eta=90 \%\)
Input power, \(P_{\text {in }}=3 \mathrm{~kW}=3 \times 10^3 \mathrm{~W}=3000 \mathrm{~W}\)
Voltage across the primary coil, \(V_p=200 \mathrm{~V}\)
Current in the secondary coil, \(I_s=6 \mathrm{~A}\)
As \(P_{\text {in }}=I_p V_p\)
\(\therefore \quad\) Current in the primary coil,
\(
I_p=\frac{P_{\mathrm{in}}}{V_p}=\frac{3000 \mathrm{~W}}{200 \mathrm{~V}}=15 \mathrm{~A}
\)
Efficiency of the transformer,
\(
\begin{aligned}
& \eta=\frac{P_{\text {out }}}{P_{\text {in }}}=\frac{V_s I_s}{V_p I_p} \\
\therefore \quad & \frac{90}{100}=\frac{6 V_s}{3000} \text { or } V_s=\frac{90 \times 3000}{100 \times 6}=450 \mathrm{~V}
\end{aligned}
\) -
Question 41 of 81
41. Question
The primary coil of a transformer, when connected to a 10 V -DC battery, draws a current of 1 mA . The number of turns in the primary and secondary coils are 50 and 100 , respectively. The voltage across the secondary coil and the current drawn by the circuit in the secondary are respectively: [NEET 2013]
CorrectIncorrectHint
\(
\begin{aligned}
&\text { (c): Transformer cannot work on dc. }\\
&\therefore \quad V_s=0 \text { and } I_s=0
\end{aligned}
\) -
Question 42 of 81
42. Question
A coil of self-inductance \(L\) is connected in series with a bulb \(B\) and an \(A C\) source. Brightness of the bulb decreases when [NEET 2013]
CorrectIncorrectHint
(b) By inserting iron rod in the coil,
\(\mathrm{L} \uparrow \mathrm{Z} \uparrow \mathrm{I} \downarrow\) so brightness \(\downarrow\)Note: Inductive reactance, \(X_L=\omega L\). On inserting iron rod in the coil, \(L\) increases. As a result Impedance \(\mathrm{Z}=\sqrt{X_L^2+R^2}=\sqrt{(\omega L)^2+R^2}\) also increases.
So, Current, \(I=\frac{V}{Z}\) decreases. -
Question 43 of 81
43. Question
In an electrical circuit \(R, L, C\) and an AC voltage source are all connected in series. When \(L\) is removed from the circuit, the phase difference between the voltage and the current in the circuit is \(\tan ^{-1} \sqrt{3}\). If instead, \(C\) is removed from the circuit, the phase difference is again \(\tan ^{-1} \sqrt{3}\). The power factor of the circuit is: [NEET 2012]
CorrectIncorrectHint
(d) When \(L\) is removed, the phase difference between the voltage and current is
\(
\begin{aligned}
& \tan \phi_1=\frac{X_C}{R} \\
& \tan \frac{\pi}{3}=\frac{X_C}{R} \text { or } X_C=R \tan 60^{\circ} \text { or } X_C=\sqrt{3} R
\end{aligned}
\)
When \(C\) is removed, the phase difference between the voltage and current is \(\tan \phi_2=\frac{X_L}{R}\) or \(\tan \frac{\pi}{3}=\frac{X_L}{R}\) or \(X_L=R \tan 60^{\circ}=\sqrt{3} R\)
As \(X_L=X_C\), the series \(L C R\) circuit is in resonance. Impedance of the circuit,
\(
Z=\sqrt{R^2+\left(X_L-X_C\right)^2}=R
\)
Power factor, \(\cos \phi=\frac{R}{Z}=\frac{R}{R}=1\) -
Question 44 of 81
44. Question
The instantaneous values of alternating current and voltages in a circuit are given as,
\(
\begin{aligned}
& i=\frac{1}{\sqrt{2}} \sin (100 \pi t) \text { Ampere } \\
& e=\frac{1}{\sqrt{2}} \sin (100 \pi t+\pi / 3) \mathrm{Volt}
\end{aligned}
\)
What is the average power consumed by the circuit in watts? [NEET 2012]CorrectIncorrectHint
(d) Given : \(i=\frac{1}{\sqrt{2}} \sin (100 \pi t)\) ampere Compare it with \(i=i_0 \sin (\omega t)\), we get
\(
i_0=\frac{1}{\sqrt{2}} \mathrm{~A}
\)
Given : \(e=\frac{1}{\sqrt{2}} \sin \left(100 \pi t+\frac{\pi}{3}\right)\) volt
Compare it with \(e=e_0 \sin (\omega t+\phi)\), we get
\(
\begin{aligned}
& e_0=\frac{1}{\sqrt{2}} \mathrm{~V}, \phi=\frac{\pi}{3} \\
\therefore \quad & i_{\mathrm{rms}}=\frac{i_0}{\sqrt{2}}=\frac{1}{2} \mathrm{~A} \text { and } e_{\mathrm{rms}}=\frac{e_0}{\sqrt{2}}=\frac{1}{2} \mathrm{~V}
\end{aligned}
\)
Average power consumed in the circuit,
\(
\begin{aligned}
& P=i_{\mathrm{rms}} e_{\mathrm{rms}} \cos \phi \\
& =\left(\frac{1}{2}\right)\left(\frac{1}{2}\right) \cos \frac{\pi}{3}=\left(\frac{1}{2}\right)\left(\frac{1}{2}\right)\left(\frac{1}{2}\right)=\frac{1}{8} \mathrm{~W}
\end{aligned}
\) -
Question 45 of 81
45. Question
The current \((I)\) in the inductance is varying with time according to the plot shown in figure. [NEET 2012]
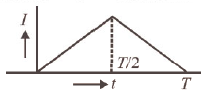
Which one of the following is the correct variation of voltage with time in the coil?
 CorrectIncorrect
CorrectIncorrectHint
(d) \(V=-L \frac{d i}{d t}\)
Here \(\frac{d i}{d t}\) is + ve for \(\frac{T}{2}\) time and \(\frac{d i}{d t}\) is – ve for next \(\frac{T}{2}\) time.
-
Question 46 of 81
46. Question
In an AC circuit, an alternating voltage \(\varepsilon=200 \sqrt{2} \sin (100 t) \mathrm{V}\) is connected to a capacitor of capacity \(1 \mu \mathrm{~F}\). The RMS value of the current in the circuit is: [NEET 2011]
CorrectIncorrectHint
(c) The given equation of alternating voltage is
\(
e=200 \sqrt{2} \sin 100 t \dots(i)
\)
The standard equation of alternating voltage is
\(
e=e_0 \sin \omega t \dots(ii)
\)
Comparing (i) and (ii), we get
\(
e_0=200 \sqrt{2} \mathrm{~V}, \omega=100 \mathrm{rad} \mathrm{~s}^{-1}
\)
The capacitive reactance is
\(
X_C=\frac{1}{\omega C}=\frac{1}{100 \times 1 \times 10^{-6}} \Omega
\)
The r.m.s. value of the current in the circuit is
\(
\begin{aligned}
& i_{\text {r.m.s. }}=\frac{v_{\text {r.m.s. }}}{X_C}=\frac{e_0 / \sqrt{2}}{1 / \omega C}=\frac{(200 \sqrt{2} / \sqrt{2})}{\left(1 / 100 \times 10^{-6}\right)} \\
& =200 \times 100 \times 10^{-6} \mathrm{~A}=2 \times 10^{-2} \mathrm{~A}=20 \mathrm{~mA}
\end{aligned}
\) -
Question 47 of 81
47. Question
An AC voltage is applied to a resistance R and an inductor L in series. If R and the inductive reactance are both equal to \(3 \Omega\), the phase difference between the applied voltage and the current in the circuit is: [AIPMT 2011]
CorrectIncorrectHint
(a) Here, \(R=3 \Omega, X_L=3 \Omega\)
The phase difference between the applied voltage and the current in the circuit is
\(
\tan \phi=\frac{X_L}{R}=\frac{3 \Omega}{3 \Omega}=1 \quad \text { or } \quad \phi=\tan ^{-1}(1)=\frac{\pi}{4}
\) -
Question 48 of 81
48. Question
The rms value of the potential difference \(V\) shown in the figure is: [AIPMT 2011]
 CorrectIncorrect
CorrectIncorrectHint
(b)
\(
\begin{aligned}
& V=V_0 \text { for } 0 \leq t \leq \frac{T}{2} \\
& V=0 \text { for } \frac{T}{2} \leq t \leq T
\end{aligned}
\)
\(
V_{\mathrm{rms}}=\left[\frac{\int_0^T V^2 d t}{\int_0^T d t}\right]^{1 / 2}=\left[\frac{\int_0^{T / 2} V_0^2 d t+\int_{T / 2}^T(0) d t}{\int_0^T d t}\right]^{1 / 2}
\)
\(
\begin{aligned}
& =\left[\frac{V_0^2}{T}[t]_0^{T / 2}\right]^{1 / 2}=\left[\frac{V_0^2}{T}\left(\frac{T}{2}\right)\right]^{1 / 2}=\left[\frac{V_0^2}{2}\right]^{1 / 2} \\
& \therefore V_{\mathrm{rms}}=\frac{V_0}{\sqrt{2}}
\end{aligned}
\)Alternate:
\(
\mathrm{V}_{\mathrm{rms}}=\sqrt{\frac{(\mathrm{T} / 2) \mathrm{V}_0^2+0}{\mathrm{~T}}}=\frac{\mathrm{V}_0}{\sqrt{2}} .
\) -
Question 49 of 81
49. Question
A coil has a resistance of 30 ohm and inductive reactance of 20 ohm at a 50 Hz frequency. If an \(A C\) source of \(200 \mathrm{~volts}, 100 \mathrm{~Hz}\) is connected across the coil, the current in the coil will be: [AIPMT 2011]
CorrectIncorrectHint
(b)
\(
\begin{aligned}
&\text { Current flowing in the coil is }\\
&\mathrm{I}=\frac{200}{Z}=\frac{200}{\sqrt{R^2+(\omega L)^2}}=\frac{200}{\sqrt{(30)^2+(40)^2}}=4 \mathrm{~A}
\end{aligned}
\) -
Question 50 of 81
50. Question
In the given circuit, the reading of voltmeter \(V_1\) and \(V_2\) are 300 V each. The reading of the voltmeter \(V_3\) and ammeter \(A\) are respectively: [AIPMT 2010]
 CorrectIncorrect
CorrectIncorrectHint
(b) As \(V_L=V_C=300 \mathrm{~V}\), therefore the given series \(L C R\) circuit is in resonance.
\(
\therefore \quad V_R=V=220 \mathrm{~V}, Z=R=100 \Omega
\)
Current, \(I=\frac{V}{Z}=\frac{220 \mathrm{~V}}{100 \Omega}=2.2 \mathrm{~A}\)
Hence, the reading of the voltmeter \(V_3\) is 220 V and the reading of ammeter \(A\) is 2.2 A . -
Question 51 of 81
51. Question
A 220 V input is supplied to a transformer. The output circuit draws a current of 2.0 A at 440 V . If the efficiency of the transformer is \(80 \%\), the current drawn by the primary windings of the transformer is: [AIPMT 2010]
CorrectIncorrectHint
(d) Here, Input voltage, \(V_p=220 \mathrm{~V}\)
Output voltage, \(V_s=440 \mathrm{~V}\)
Input current, \(I_p=\) ?
Output current, \(I_s=2 \mathrm{~A}\)
Efficiency of the transformer, \(\eta=80 \%\)
Efficiency of the transformer, \(\eta=\frac{\text { Output power }}{\text { Input power }}\)
\(
\begin{aligned}
\eta=\frac{V_s I_s}{V_p I_p} \text { or } I_p & =\frac{V_s I_s}{\eta V_p}=\frac{(440 \mathrm{~V})(2 \mathrm{~A})}{\left(\frac{80}{100}\right)(220 \mathrm{~V})} \\
& =\frac{(440 \mathrm{~V})(2 \mathrm{~A})(100)}{(80)(220 \mathrm{~V})}=5 \mathrm{~A}
\end{aligned}
\) -
Question 52 of 81
52. Question
A condenser of capacity \(C\) is charged to a potential difference of \(V_1\). The plates of the condenser are then connected to an ideal inductor of inductance \(L\). The current through the inductor when the potential difference across the condenser reduces to \(V_2\) is [AIPMT 2010]
CorrectIncorrectHint
(d) In case of oscillatory discharge of a capacitor through an inductor, charge at instant \(t\) is given by
\(
\begin{gathered}
\quad q=q_0 \cos \omega t ; \text { where, } \omega=\frac{1}{\sqrt{L C}} \\
\therefore \cos \omega t=\frac{q}{q_0}=\frac{C V_2}{C V_1}=\frac{V_2}{V_1} \quad(\because q=C V)
\end{gathered}
\)
\(
\begin{aligned}
&\text { Current through the inductor }\\
&\begin{aligned}
& I=\frac{d q}{d t}=\frac{d}{d t}\left(q_0 \cos \omega t\right)=-q_0 \omega \sin \omega t \\
& |I|=C V_1 \frac{1}{\sqrt{L C}}\left[1-\cos ^2 \omega t\right]^{1 / 2} \\
& \\
& =V_1 \sqrt{\frac{C}{L}}\left[1-\left(\frac{V_2}{V_1}\right)^2\right]^{1 / 2}=\left[\frac{C\left(V_1^2-V_2^2\right)}{L}\right]^{1 / 2}
\end{aligned}
\end{aligned}
\) -
Question 53 of 81
53. Question
Power dissipated in an \(L C R\) series circuit connected to an A.C. source of emf \(\varepsilon\) is [AIPMT 2009]
CorrectIncorrectHint
\(
\begin{aligned}
&\text { (d) : Average power, } P=E_{\text {r.m. } \mathrm{s}} I_{\mathrm{r} . \mathrm{m} . \mathrm{s}} \cos \phi\\
&\begin{aligned}
& Z=\sqrt{R^2+\left(X_L-X_C\right)^2}, \cos \phi=\frac{R}{Z} \\
& \text { But } I_{\text {r.m.s }}=\frac{E_{\text {r.m.s }}}{Z} \quad \therefore \quad P=E_{\text {r.m.s }}^2 \cdot \frac{R}{Z^2} \\
& \therefore P=E_{\text {r.m.s }}^2 \frac{R}{\left\{R^2+\left(X_L-X_C\right)^2\right\}}=\frac{\varepsilon^2 R}{\left[R^2+\left(L \omega-\frac{1}{C \omega}\right)^2\right]}
\end{aligned}
\end{aligned}
\) -
Question 54 of 81
54. Question
In an a.c. circuit the e.m.f. \((\varepsilon)\) and the current \((i)\) at any instant are given respectively by
\(
e=E_0 \sin \omega t, \quad i=I_0 \sin (\omega t-\phi)
\)
The average power in the circuit over one cycle of a.c. is [AIPMT 2008]CorrectIncorrectHint
(a) \(\text { Average power }=\frac{E_0 I_0}{2} \cos \phi\)
-
Question 55 of 81
55. Question
What is the value of inductance \(L\) for which the current is maximum in a series LCR circuit with \(\mathrm{C}=10 \mu \mathrm{~F}\) and \(\omega=1000 \mathrm{~s}^{-1}\)? [AIPMT 2007]
CorrectIncorrectHint
(d) In series \(L C R\), current is maximum at resonance.
\(\therefore\) Resonant frequency, \(\omega=\frac{1}{\sqrt{L C}}\)
\(\therefore \quad \omega^2=\frac{1}{L C} \quad\) or, \(L=\frac{1}{\omega^2 C}\)
Given \(\omega=1000 \mathrm{~s}^{-1}\) and \(C=10 \mu \mathrm{~F}\)
\(
\therefore L=\frac{1}{1000 \times 1000 \times 10 \times 10^{-6}}=0.1 \mathrm{H}=100 \mathrm{mH}
\) -
Question 56 of 81
56. Question
A transformer is used to light a 100 W and 110 V lamp from a 220 V main. If the main current is 0.5 A , the efficiency of the transformer is approximately: [AIPMT 2007]
CorrectIncorrectHint
(c) Given : Output power \(P=100 \mathrm{~W}\)
Voltage across primary \(V_p=220 \mathrm{~V}\)
Current in the primary \(I_p=0.5 \mathrm{~A}\)
Efficiency of a transformer
\(
\begin{aligned}
\eta & =\frac{\text { output power }}{\text { input power }} \times 100 \\
& =\frac{P}{V_p I_p} \times 100=\frac{100}{220 \times 0.5} \times 100=90 \%
\end{aligned}
\) -
Question 57 of 81
57. Question
The primary and secondary coils of a transformer have 50 and 1500 turns respectively. If the magnetic flux \(\phi\) linked with the primary coil is given by \(\phi=\phi_0+4 t\), where \(\phi\) is in webers, \(t\) is time in seconds and \(\phi_0\) is a constant, the output voltage across the secondary coil is [AIPMT 2007]
CorrectIncorrectHint
(a) No. of turns across primary \(N_p=50\)
Number of turns across secondary \(N_s=1500\)
Magnetic flux linked with primary, \(\phi=\phi_0+4 t\)
\(\therefore \quad\) Voltage across the primary,
\(
\begin{aligned}
& V_p=\frac{d \phi}{d t}=\frac{d}{d t}\left(\phi_0+4 t\right)=4 \mathrm{volt} \\
& \frac{V_s}{V_p}=\frac{N_s}{N_p} \text { or } V_s=\left(\frac{1500}{50}\right) \times 4=120 \mathrm{~V}
\end{aligned}
\) -
Question 58 of 81
58. Question
The core of a transformer is laminated so that: [AIPMT 2006]
CorrectIncorrectHint
(a) The core of a transformer is laminated to minimise the energy losses due to eddy currents.
-
Question 59 of 81
59. Question
A coil of inductive reactance \(31 \Omega\) has a resistance of \(8 \Omega\). It is placed in series with a condenser of capacitive reactance \(25 \Omega\). The combination is connected to an a.c. source of 110 V . The power factor of the circuit is: [AIPMT 2006]
CorrectIncorrectHint
(c) Impedance of series LCR is
\(
\begin{aligned}
& Z=\sqrt{R^2+\left(X_L-X_C\right)^2} \\
& =\sqrt{8^2+(31-25)^2} \\
& =\sqrt{64+36}=10 \Omega
\end{aligned}
\)
Power factor, \(\cos \phi=\frac{R}{Z}=\frac{8}{10}=0.8\) -
Question 60 of 81
60. Question
A transistor-oscillator using a resonant circuit with an inductor \(L\) (of negligible resistance) and a capacitor \(C\) in series produces oscillations of frequency \(f\). If \(L\) is doubled and \(C\) is changed to \(4 C\), the frequency will be [AIPMT 2006]
CorrectIncorrectHint
\(
\begin{aligned}
&\text { (d) : Frequency of } L C \text { oscillation }=\frac{1}{2 \pi \sqrt{L C}}\\
&\begin{aligned}
& \text { or, } \frac{f_1}{f_2}=\left(\frac{L_2 C_2}{L_1 C_1}\right)^{1 / 2}=\left(\frac{2 L \times 4 C}{L \times C}\right)^{1 / 2}=(8)^{1 / 2} \\
& \therefore \frac{f_1}{f_2}=2 \sqrt{2} \Rightarrow f_2=\frac{f_1}{2 \sqrt{2}} \text { or, } f_2=\frac{f}{2 \sqrt{2}} \quad\left(\because f_1=f\right)
\end{aligned}
\end{aligned}
\) -
Question 61 of 81
61. Question
In a circuit \(L, C\) and \(R\) are connected in series with an alternating voltage source of frequency \(f\). The current leads the voltage by \(45^{\circ}\). The value of \(C\) is [AIPMT 2005]
CorrectIncorrectHint
(d) \(\tan \phi=\frac{X_C-X_L}{R}\)
or \(\tan \left(\frac{\pi}{4}\right)=\frac{\frac{1}{\omega C}-\omega L}{R}\)
\(R=\frac{1}{\omega C}-\omega L\)
or \((R+2 \pi f L)=\frac{1}{2 \pi f C}\)
or \(C=\frac{1}{2 \pi f(R+2 \pi f L)}\) -
Question 62 of 81
62. Question
A coil of 40 henry inductance is connected in series with a resistance of 8 ohm and the combination is joined to the terminals of a 2 volt battery. The time constant of the circuit is [AIPMT 2004]
CorrectIncorrectHint
\(
\begin{aligned}
&\text { (a) Time constant of } L R \text { circuit is } \tau=L / R \text {. }\\
&\tau=\frac{40}{8}=5 \mathrm{~s}
\end{aligned}
\) -
Question 63 of 81
63. Question
For a series \(L C R\) circuit, the power loss at resonance is [AIPMT 2002]
CorrectIncorrectHint
(c) The impedance \(Z\) of a series \(L C R\) circuit is given by, \(Z=\sqrt{R^2+\left(X_L-X_C\right)^2}\)
where \(X_L=\omega L\) and \(X_C=\frac{1}{\omega C}, \omega\) is angular frequency.
At resonance, \(X_L=X_C\), hence \(Z=R\).
\(\therefore \quad V_R=V\) (supply voltage)
\(\therefore\) r.m.s. current, \(I=\frac{V_R}{R}=\frac{V}{R}\)
\(\therefore \quad\) Power loss \(=I^2 R=V^2 / R\) -
Question 64 of 81
64. Question
A capacitor of capacity \(C\) has reactance \(X\). If capacitance and frequency are doubled, then the reactance will be: [AIPMT 2001]
CorrectIncorrectHint
\(
\begin{aligned}
&\text { (c) Capacitive reactance, } X=\frac{1}{\omega C}=\frac{1}{2 \pi f C}\\
&\begin{aligned}
& \Rightarrow X \propto \frac{1}{f C} \\
& \therefore \frac{X^{\prime}}{X}=\frac{f}{f^{\prime}} \times \frac{C}{C^{\prime}}=\frac{f}{2 f} \times \frac{C}{2 C}=\frac{1}{4} \\
& \Rightarrow X^{\prime}=\frac{X}{4}
\end{aligned}
\end{aligned}
\) -
Question 65 of 81
65. Question
An inductance \(L\) having a resistance \(R\) is connected to an alternating source of angular frequency \(\omega\). The quality factor \(Q\) of the inductance is [AIPMT 2000]
CorrectIncorrectHint
\(
\begin{aligned}
&\text { (d) Quality factor }\\
&\begin{aligned}
& =\frac{\text { Potential drop across capacitor or inductor }}{\text { Potential drop across } \mathrm{R}} \\
& =\frac{I \omega L}{I R}=\frac{\omega L}{R}
\end{aligned}
\end{aligned}
\) -
Question 66 of 81
66. Question
In the circuit given in figure, 1 and 2 are ammeters. Just after key \(K\) is pressed to complete the circuit, the reading will be [AIPMT 1999]
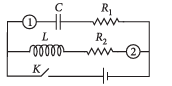 CorrectIncorrect
CorrectIncorrectHint
(d) At \(t=0\)
(i) capacitor offers negligible resistance (acts as short).
(ii) inductor offers large resistance (acts as open) to current flow. -
Question 67 of 81
67. Question
A step-up transformer operates on a 230 V line and supplies a load of 2 ampere. The ratio of the primary and secondary windings is \(1: 25\). The current in the primary is [AIPMT 1998]
CorrectIncorrectHint
(b) \(\frac{E_P}{E_S}=\frac{I_S}{I_P}=\frac{N_P}{N_S}=\frac{1}{25}\);
Here, \(I_S=2 \mathrm{~A}\)
\(
I_P=25 I_S=50 \mathrm{~A}
\) -
Question 68 of 81
68. Question
The primary winding of a transformer has 500 turns whereas its secondary has 5000 turns. The primary is connected to an A.C. supply of \(20 \mathrm{~V}, 50 \mathrm{~Hz}\). The secondary will have an output of [AIPMT 1997]
CorrectIncorrectHint
(c) Turns on primary winding \(=500\); Turns on secondary winding \(=5000\); Primary winding voltage \(\left(E_p\right)\) \(=20 \mathrm{~V}\) and frequency \(=50 \mathrm{~Hz}\).
\(
\begin{aligned}
& \quad \frac{N_s}{N_p}=\frac{E_s}{E_p} \text { or } \frac{5000}{500}=\frac{E_s}{20} \\
& \text { or } E_s=\frac{5000 \times 20}{500}=200 \mathrm{~V}
\end{aligned}
\)
and frequency remains the same. Therefore secondary winding will have an output of \(200 \mathrm{~V}, 50 \mathrm{~Hz}\). -
Question 69 of 81
69. Question
In an A.C. circuit with voltage \(V\) and current \(I\) the power dissipated is [AIPMT 1997]
CorrectIncorrectHint
(a) Power dissipated \(=V_{\mathrm{rms}} \cdot I_{\mathrm{rms}}\)
\(
=\left(V_{\mathrm{rms}}\right)\left(I_{\mathrm{rms}}\right) \cos \theta
\)
Hence, power dissipated depends upon phase difference. -
Question 70 of 81
70. Question
In an experiment, \(200 \mathrm{VA.C}\). is applied at the ends of an LCR circuit. The circuit consists of an inductive reactance \(\left(X_L\right)=50 \Omega\), capacitive reactance \(\left(X_C\right)=50 \Omega\) and ohmic resistance \((\mathrm{R})\) \(=10 \Omega\). The impedance of the circuit is [AIPMT 1996]
CorrectIncorrectHint
(a) Given : Supply voltage \(\left(V_{a c}\right)=200 \mathrm{~V}\)
Inductive reactance \(\left(X_L\right)=50 \Omega\)
Capacitive reactance \(\left(X_C\right)=50 \Omega\)
Ohmic resistance \((R)=10 \Omega\).
We know that impedance of the LCR circuit \((Z)\)
\(
\begin{aligned}
& =\sqrt{\left\{\left(X_L-X_C\right)^2+R^2\right\}} \\
& =\sqrt{\left\{(50-50)^2+(10)^2\right\}}=10 \Omega
\end{aligned}
\) -
Question 71 of 81
71. Question
When the key \(K\) is pressed at time \(t=0\), then which of the following statement about the current \(I\) in the resistor \(A B\) of the given circuit is true? [AIPMT 1995]
CorrectIncorrectHint
(b) Initially, the current will pass through the capacitor (and not through the resistance which is parallel to the capacitor). So effective resistance in the circuit is \(R_{A B}\). Therefore the current in the resistor is 2 mA . After some time, the capacitor will become fully charged and will be in its steady state. Now no current will pass through the capacitor and the effective resistance of the circuit that is
\(
(1000+1000)=2000 \Omega
\)
Therefore final current in the resistor
\(
=\frac{V}{R}=\frac{2}{2000}=1 \times 10^{-3} \mathrm{~A}=1 \mathrm{~mA}
\) -
Question 72 of 81
72. Question
In an A.C. circuit, the current flowing is \(I=5 \sin (100 t-\pi / 2)\) ampere and the potential difference is \(V=200 \sin (100 t)\) volts. The power consumption is equal to [AIPMT 1995]
CorrectIncorrectHint
(b) Current \((I)=5 \sin (100 t-\pi / 2)\) and voltage \((V)\) \(=200 \sin (100 t)\). Comparing the given equation, with the standard equation, we find that phase between current and voltage is \(\phi=\frac{\pi}{2}=90^{\circ}\)
Power consumption \(P=I_{\mathrm{rms}} V_{\mathrm{rms}} \cos \phi=I_{\mathrm{rms}} V_{\mathrm{rms}} \cos 90^{\circ}=0\) -
Question 73 of 81
73. Question
An series \(L-C-R\) circuit is connected to a source of A.C. current. At resonance, the phase difference between the applied voltage and the current in the circuit, is [AIPMT 1994]
CorrectIncorrectHint
(b) For resonance condition, the impedance will be minimum and the current will be maximum. This is only possible when \(X_L=X_C\).
Therefore \(\tan \phi=\frac{X_L+X_C}{R}=0\) or \(\phi=0\). -
Question 74 of 81
74. Question
In an a.c. circuit, the r.m.s. value of current, \(i_{\text {rms }}\) is related to the peak current, \(\mathrm{i}_0\) by the relation [AIPMT 1994]
CorrectIncorrectHint
\(
\text { (d) } \quad I_{\mathrm{rms}}=\frac{I_0}{\sqrt{2}}
\) -
Question 75 of 81
75. Question
The current \(I\) in an A.C. circuit with inductance coil varies with time according to the graph given below. [AIPMT 1994]
Which one of the following graphs gives the variation of voltage with time?
 CorrectIncorrect
CorrectIncorrectHint
(a) In an A.C. circuit with inductance coil, the voltage \(V\) leads the current \(I\) by a phase difference of \(90^{\circ}\) or the current \(I\) lags behind the voltage \(V\) by a phase difference of \(90^{\circ}\). Thus the voltage goes on decreasing with the increase in time as shown in the graph (a).
-
Question 76 of 81
76. Question
Two cables of copper are of equal lengths. One of them has a single wire of area of cross-section \(A\), while other has 10 wires of cross-sectional area \(A / 10\) each. Give their suitability for transporting A.C. and D.C. [AIPMT 1994]
CorrectIncorrectHint
(a) The major portion of the A.C. flows on the surface of the wire. So where a thick wire is required, a number of thin wires are joined together to give an equivalent effect of a thick wire. Therefore multiple strands are suitable for transporting A.C. Similarly multiple strands can also be used for D.C.
Explanation:
step 1: Compare the resistance of the two cables.
The total cross-sectional area of the second cable is \(10 \times \frac{A}{10}=A\).
Since both cables have the same length and total cross-sectional area, their resistances are equal for DC.
\(
\begin{aligned}
& R_1=\rho \frac{l}{A} \\
& R_2=\rho \frac{l}{10 \cdot \frac{A}{10}}=\rho \frac{l}{A}
\end{aligned}
\)
Therefore, \(\boldsymbol{R}_1=\boldsymbol{R}_2\).Step 2: Analyze the skin effect for AC.
For AC, the current tends to flow on the surface of the conductor (skin effect).
The multi-wire cable has a larger surface area compared to the single-wire cable.
Therefore, the multi-wire cable is more suitable for AC due to reduced skin effect losses.Step 3: Conclude the suitability for AC and DC .
Multi-wire cable is better for AC due to the skin effect.
Both cables are equally suitable for DC as they have the same resistance.Therefore, Multi-wire cable is more suitable for AC, while both cables are equally suitable for DC.
-
Question 77 of 81
77. Question
The time constant of \(C\) – \(R\) circuit is [AIPMT 1992]
CorrectIncorrectHint
(c) The time constant for \(R-C\). circuit, \(\tau=C R\)
Growth of charge in a circuit containing capacitance and resistance is given by the formula,
\(
q=q_0\left(1-e^{-t / C R}\right)
\)
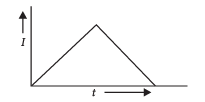
\(C R\) is known as time constant in this formula. -
Question 78 of 81
78. Question
In a region of uniform magnetic induction \(\mathrm{B}=10^{-2}\) tesla, a circular coil of radius 30 cm and resistance \(\pi^2\) ohm is rotated about an axis which is perpendicular to the direction of \(B\) and which forms a diameter of the coil. If the coil rotates at 200 rpm the amplitude of the alternating current induced in the coil is [AIPMT 1988]
CorrectIncorrectHint
(c) \(I_0=\frac{E_0}{R}=\frac{n B A \omega}{R}\)
Given, \(n=1, B=10^{-2} \mathrm{~T}\),
\(
\begin{aligned}
& A=\pi(0.3)^2 \mathrm{~m}^2, R=\pi^2 \\
& f=(200 / 60) \text { and } \omega=2 \pi(200 / 60)
\end{aligned}
\)
Substituting these values and solving, we get
\(
I_0=6 \times 10^{-3} \mathrm{~A}=6 \mathrm{~mA}
\) -
Question 79 of 81
79. Question
Eddy currents are produced when [AIPMT 1988]
CorrectIncorrectHint
(a) Eddy currents are produced when a metal is kept in a varying magnetic field.
-
Question 80 of 81
80. Question
\(A B\) is a part of an electrical circuit (see figure). The potential difference ” \(V_A-V_B\) “, at the instant when current \(i=2 \mathrm{~A}\) and is increasing at a rate of \(1 \mathrm{amp} /\) second is: [NEET 2025]
 CorrectIncorrect
CorrectIncorrectHint
(a)
\(
\begin{aligned}
&\text { Given, } I=2 \mathrm{~A} \text { and } \frac{d i}{d t}=+1 \mathrm{~A} / \mathrm{s}\\
&\begin{aligned}
& V_A-L \frac{d i}{d t}-5-i \times 2=V_B \\
& \Rightarrow V_A-1 \times 1-5-2 \times 2=V_B \\
& \Rightarrow V_A-V_B=10 \text { volt }
\end{aligned}
\end{aligned}
\) -
Question 81 of 81
81. Question
To an ac power supply of 220 V at 50 Hz , a resistor of \(20 \Omega\), a capacitor of reactance \(25 \Omega\) and an inductor of reactance \(45 \Omega\) are connected in series. The corresponding current in the circuit and the phase angle between the current and the voltage is, respectively [NEET 2025]
CorrectIncorrectHint
(c)
\(
\begin{aligned}
& X_L=45 \mathrm{~W}, X_C=25 \mathrm{~W}, R=20 \mathrm{~W} \\
& I=\frac{220}{\sqrt{\left(X_L-X_C\right)^2+R^2}}=\frac{220}{\sqrt{(45-25)^2+20^2}} \\
& =\frac{220}{2 \sqrt{2}}=\frac{11}{\sqrt{2}}=7.779 \mathrm{~A} \\
& \tan \phi=\frac{X_L-X_C}{R}=\frac{45-25}{20}=1 \\
& \phi=45^{\circ}
\end{aligned}
\)