Past NEET Entrance Papers
Quiz Summary
0 of 176 Questions completed
Questions:
Information
You have already completed the quiz before. Hence you can not start it again.
Quiz is loading…
You must sign in or sign up to start the quiz.
You must first complete the following:
Results
Results
0 of 176 Questions answered correctly
Your time:
Time has elapsed
You have reached 0 of 0 point(s), (0)
Earned Point(s): 0 of 0, (0)
0 Essay(s) Pending (Possible Point(s): 0)
Categories
- Not categorized 0%
- 1
- 2
- 3
- 4
- 5
- 6
- 7
- 8
- 9
- 10
- 11
- 12
- 13
- 14
- 15
- 16
- 17
- 18
- 19
- 20
- 21
- 22
- 23
- 24
- 25
- 26
- 27
- 28
- 29
- 30
- 31
- 32
- 33
- 34
- 35
- 36
- 37
- 38
- 39
- 40
- 41
- 42
- 43
- 44
- 45
- 46
- 47
- 48
- 49
- 50
- 51
- 52
- 53
- 54
- 55
- 56
- 57
- 58
- 59
- 60
- 61
- 62
- 63
- 64
- 65
- 66
- 67
- 68
- 69
- 70
- 71
- 72
- 73
- 74
- 75
- 76
- 77
- 78
- 79
- 80
- 81
- 82
- 83
- 84
- 85
- 86
- 87
- 88
- 89
- 90
- 91
- 92
- 93
- 94
- 95
- 96
- 97
- 98
- 99
- 100
- 101
- 102
- 103
- 104
- 105
- 106
- 107
- 108
- 109
- 110
- 111
- 112
- 113
- 114
- 115
- 116
- 117
- 118
- 119
- 120
- 121
- 122
- 123
- 124
- 125
- 126
- 127
- 128
- 129
- 130
- 131
- 132
- 133
- 134
- 135
- 136
- 137
- 138
- 139
- 140
- 141
- 142
- 143
- 144
- 145
- 146
- 147
- 148
- 149
- 150
- 151
- 152
- 153
- 154
- 155
- 156
- 157
- 158
- 159
- 160
- 161
- 162
- 163
- 164
- 165
- 166
- 167
- 168
- 169
- 170
- 171
- 172
- 173
- 174
- 175
- 176
- Current
- Review
- Answered
- Correct
- Incorrect
-
Question 1 of 176
1. Question
A uniform wire of diameter \(d\) carries a current of 100 mA when the mean drift velocity of electrons in the wire is \(v\). For a wire of diameter \(\frac{d}{2}\) of the same material to carry a current of 200 mA , the mean drift velocity of electrons in the wire is [NEET 2024 (Re-Examination)]
CorrectIncorrectHint
(a)
\(
I=n \cdot A \cdot e \cdot v_d
\)
The diameter of the first wire is \(d\). The cross-sectional area \(A_1\) is given by:
\(
A_1=\frac{\pi d^2}{4}
\)
\(
I=n \cdot\left(\frac{\pi d^2}{4}\right) \cdot e \cdot v
\)
The diameter of the second wire is \(\frac{d}{2}\). The cross-sectional area \(A_2\) is given by:
\(
A_2=\frac{\pi\left(\frac{d}{2}\right)^2}{4}=\frac{\pi d^2}{16}
\)
For the second wire, the current is given by:
\(
I=n \cdot A_2 \cdot e \cdot v_d^{\prime}
\)
Substituting \(A_2\) :
\(
I=n \cdot\left(\frac{\pi d^2}{16}\right) \cdot e \cdot v_d^{\prime}
\)
Now, we can solve for \(v_d^{\prime}\) :
\(
\frac{d^2}{4} \cdot v=\frac{d^2}{16} \cdot v_d^{\prime}
\)
Multiplying both sides by 16 :
\(
4 d^2 \cdot v=d^2 \cdot v_d^{\prime}
\)
Dividing both sides by \(d^2\) :
\(
4 v=v_d^{\prime}
\)
Thus, the mean drift velocity \(v_d^{\prime}\) in the second wire (with diameter \(\frac{d}{2}\) ) is:
\(
v_d^{\prime}=4 v
\) -
Question 2 of 176
2. Question
A uniform metal wire of length \(l\) has \(10 \Omega\) resistance. Now this wire is stretched to a length \(2 l\) and then bent to form a perfect circle. The equivalent resistance across any arbitrary diameter of that circle is [NEET 2024 (Re-Examination)]
CorrectIncorrectHint
(a)

When the wire is stretched to twice its length, its cross-sectional area decreases by half (since the volume remains constant).
\(
\begin{aligned}
R & =\rho \frac{l}{A} \\
R^{\prime} & =\rho \frac{2 l}{A / 2} \\
R^{\prime} & =4 \rho \frac{l}{A} \\
R^{\prime} & =4 R \\
R^{\prime} & =4 \cdot 10 \Omega \\
R^{\prime} & =40 \Omega
\end{aligned}
\)
When the wire is bent into a circle, the resistance across any diameter is equivalent to two \(20 \Omega\) resistors in parallel (since the circle is divided into two equal halves).
\(
\begin{aligned}
& \frac{1}{R_{e q}}=\frac{1}{20 \Omega}+\frac{1}{20 \Omega} \\
& \frac{1}{R_{e q}}=\frac{2}{20 \Omega} \\
& R_{e q}=10 \Omega
\end{aligned}
\) -
Question 3 of 176
3. Question
The given circuit shows a uniform straight wire \(A B\) of 40 cm length fixed at both ends. In order to get zero reading in the galvanometer \(G\), the free end of \(J\) is to be placed from the end \(B\) at: [NEET 2024 (re-examination)]
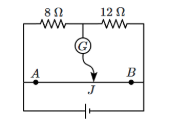 CorrectIncorrect
CorrectIncorrectHint
(d)

\(
\begin{aligned}
&\begin{aligned}
& \frac{8}{x}=\frac{12}{40-x} \\
& \frac{2}{x}=\frac{3}{40-x} \\
& 80-2 x=3 x \\
& 16=x
\end{aligned}\\
&\text { from } B\\
&=40-16=24 \mathrm{~cm}
\end{aligned}
\) -
Question 4 of 176
4. Question
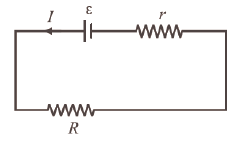
The terminal voltage of the battery, whose emf is 10 V and internal resistance \(1 \Omega\), when connected through an external resistance of \(4 \Omega\) as shown in the figure is: [NEET 2024]
 CorrectIncorrect
CorrectIncorrectHint
(c)
\(
\begin{aligned}
\text { Current in circuit } i & =\frac{10}{4+1}=2 \mathrm{~A} \\
\text { Terminal voltage } & =E-i R \\
& =10-2 \times 1=8 \mathrm{~V}
\end{aligned}
\) -
Question 5 of 176
5. Question
A wire of length ‘ \(l\) ‘ and resistance \(100 \Omega\) is divided into 10 equal parts. The first 5 parts are connected in series while the next 5 parts are connected in parallel. The two combinations are again connected in series. The resistance of this final combination is: [NEET 2024]
CorrectIncorrectHint
(b) Given: Original length of the wire, \(l\).
Total resistance of the wire \(R=100 \Omega\).
The wire is divided into 10 equal parts.
Resistance of each part:
Since the wire is divided into 10 equal parts, the length of each part is \(\frac{l}{10}\). Resistance is proportional to length (as long as the cross-sectional area and material of the wire remain constant). Therefore, the resistance of each part, denoted as \(r\), is \(\frac{1}{10}\) th of the total resistance:
\(
r=\frac{R}{10}=\frac{100 \Omega}{10}=10 \Omega
\)
First 5 parts in series:
When resistors are connected in series, the total resistance is the sum of the individual resistances:
\(
R_{\text {series }}=5 \times 10 \Omega=50 \Omega
\)
Next 5 parts in parallel:
When resistors are connected in parallel, the total resistance \(R_{\text {parallel }}\) can be calculated using the reciprocal formula:
\(
\frac{1}{R_{\text {parallel }}}=\frac{1}{10 \Omega}+\frac{1}{10 \Omega}+\frac{1}{10 \Omega}+\frac{1}{10 \Omega}+\frac{1}{10 \Omega}=5 \times \frac{1}{10 \Omega}=\frac{5}{10 \Omega}=\frac{1}{2 \Omega}
\)
Thus,
\(
R_{\text {parallel }}=2 \Omega
\)
Final combination in series:
The total resistance of the combination, where the series and parallel groups are again connected in series, will be:
\(
R_{\text {total }}=R_{\text {series }}+R_{\text {parallel }}=50 \Omega+2 \Omega=52 \Omega
\) -
Question 6 of 176
6. Question
Two heaters \(A\) and \(B\) have power rating of 1 kW and 2 kW , respectively. Those two are first connected in series and then in parallel to a fixed power source. The ratio of power outputs for these two cases is: [NEET 2024]
CorrectIncorrectHint
(b) Find the resistances of the heaters.
\(
\begin{aligned}
& P=\frac{V^2}{R} \\
& R=\frac{V^2}{P} \\
& R_A=\frac{V^2}{1000} \\
& R_B=\frac{V^2}{2000}
\end{aligned}
\)
Calculate the total resistance in series.
\(
\begin{aligned}
& R_s=R_A+R_B \\
& R_s=\frac{V^2}{1000}+\frac{V^2}{2000} \\
& R_s=\frac{3 V^2}{2000}
\end{aligned}
\)
Calculate the power output in series.
\(
\begin{aligned}
P_s & =\frac{V^2}{R_s} \\
P_s & =\frac{V^2}{\frac{3 V^2}{2000}} \\
P_s & =\frac{2000}{3} \mathrm{~W}
\end{aligned}
\)
Calculate the total resistance in parallel.
\(
\begin{aligned}
& \frac{1}{R_p}=\frac{1}{R_A}+\frac{1}{R_B} \\
& \frac{1}{R_p}=\frac{1000}{V^2}+\frac{2000}{V^2} \\
& \frac{1}{R_p}=\frac{3000}{V^2} \\
& R_p=\frac{V^2}{3000}
\end{aligned}
\)
Calculate the power output in parallel.
\(
\begin{aligned}
& P_p=\frac{V^2}{R_p} \\
& P_p=\frac{V^2}{\frac{V^2}{3000}} \\
& P_p=3000 \mathrm{~W}
\end{aligned}
\)
Find the ratio of power outputs.
\(
\frac{P_s}{P_p}=\frac{\frac{2000}{3}}{3000}=\frac{2}{9}
\) -
Question 7 of 176
7. Question
Choose the correct circuit which can achieve the bridge balance.
 CorrectIncorrect
CorrectIncorrectHint
(b) In option (2),
\(
\frac{10}{15}=\frac{10}{5+R_D}
\)
The diode can conduct and have resistance \(R_D=10 \Omega\) because diode have dynamic resistance. In that case bridge will be balanced. -
Question 8 of 176
8. Question
The equivalent resistance \(R_{A B}\) between points \(A\) and \(B\) in the given network is: [NEET 2024]
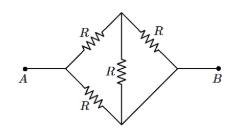 CorrectIncorrect
CorrectIncorrectHint
(b) \(R+(R/2)=3R/2\)
\(R_{AB}=[(3R/2) \times R]/[(3R/2)+R]=3R/5\) -
Question 9 of 176
9. Question
The value of \(R\) in the given circuit when there is no current in the \(5 \Omega\) resistor is: [NEET 2024]
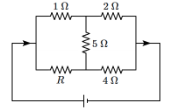 CorrectIncorrect
CorrectIncorrectHint
(d) \(2 \times R = 4 \times 1\)
\(R = 2 \Omega\) -
Question 10 of 176
10. Question
There are two heaters \(A\) and \(B\). The heater \(A\) takes time \(t_1\) to boil a given quantity of water, while \(B\) takes time \(t_2\) to boil the same quantity of water across the same supply voltage. If the two heaters are connected in series, the time taken by this combination to boil the same quantity of water will be: [NEET 2024]
CorrectIncorrectHint
(b) When A and B are connected in series:
1. Total Resistance in Series:
The total resistance \(R_s\) when connected in series is:
\(
R_s=R_1+R_2
\)
2. Substituting the Resistance Values:
Using the earlier expressions for \(R_1\) and \(R_2\) :
\(
R_s=\frac{V^2}{H} \cdot t_1+\frac{V^2}{H} \cdot t_2
\)
Simplifying gives:
\(
R_s=\frac{V^2}{H}\left(t_1+t_2\right)
\)
3. Heat Generation for Series Connection:
The heat generated in time \(t_s\) is:
\(
H=\frac{V^2}{R_s} \cdot t_s
\)
4. Final Equation for Time \(t_s\) :
Setting the heat generated equal gives:
\(
\frac{V^2}{R_s} \cdot t_s=H
\)
Substituting \(R_s\) :
\(
t_s=t_1+t_2
\) -
Question 11 of 176
11. Question
There are two heaters \(A\) and \(B\). The heater \(A\) takes time \(t_1\) to boil a given quantity of water, while \(B\) takes time \(t_2\) to boil the same quantity of water across the same supply voltage. If the two heaters are connected in parallel, the time taken by this combination to boil the same quantity of water will be: [NEET 2024]
CorrectIncorrectHint
(a) When \(A\) and \(B\) are connected in parallel:
1. Heat Generation Formula: The heat generated by a coil can be expressed using the formula:
\(
H=\frac{V^2}{R} \cdot t
\)
where \(H\) is the heat generated, \(V\) is the voltage, \(R\) is the resistance, and \(t\) is the time.
2. Resistance Calculation:
For coil A:
\(
R_1=\frac{V^2}{H} \cdot t_1
\)
For coil B:
\(
R_2=\frac{V^2}{H} \cdot t_2
\)
3. Equivalent Resistance in Parallel:
The equivalent resistance \(R_p\) for coils in parallel is given by:
\(
\frac{1}{R_p}=\frac{1}{R_1}+\frac{1}{R_2}
\)
4. Substituting the Resistance Values:
Substitute \(R_1\) and \(R_2\) into the equation:
\(
\frac{1}{R_p}=\frac{H}{V^2 t_1}+\frac{H}{V^2 t_2}
\)
Simplifying gives:
\(
\frac{1}{R_p}=\frac{H}{V^2}\left(\frac{1}{t_1}+\frac{1}{t_2}\right)
\)
5. Heat Generation for Parallel Connection:
The heat generated in time \(t_p\) is:
\(
H=\frac{V^2}{R_p} \cdot t_p
\)
Setting the heat generated equal for both cases:
\(
H=H
\)
Thus:
\(
\frac{V^2}{R_p} \cdot t_p=H
\)
6. Final Equation for Time \(t_p\) :
By substituting \(R_p\) back into the equation, we find:
\(
t_p=\frac{t_1 t_2}{t_1+t_2}
\) -
Question 12 of 176
12. Question
\(
\begin{aligned}
&\text { Arrange the following in the order of their resistance. }\\
&\begin{array}{|l|l|}
\hline \text { A. } & (0 \text { to } 1 \mathrm{~A}) \text { ranged ammeter. } \\
\hline \text { B. } & (0 \text { to } 100 \mathrm{~mA}) \text { ranged milli-ammeter. } \\
\hline \text { C. } & (0 \text { to } 500 \mu \mathrm{~A}) \text { ranged micro-ammeter. } \\
\hline \text { D. } & (0 \text { to } 100 \mathrm{~V}) \text { ranged voltmeter. } \\
\hline
\end{array}
\end{aligned}
\)
Choose the correct answer from the options given below: [NEET 2024]CorrectIncorrectHint
(c) In general, resistance of voltmeter is large. For ideal voltmeter, resistance should be infinite.
So, voltmeter of range 100 V , resistance will be less than the ideal voltmeter.
In general, resistance of ammeter is small. For smaller range, shunt resistance should be larger to facilitate larger current through the galvanometer. For larger range, shunt resistance should be smaller to facilitate smaller current through the galvanometer. So, the resistance of ammeter with larger range will be smaller than the resistance of ammeter with smaller range.
\(
\mathrm{D}>\mathrm{A}>\mathrm{B}>\mathrm{C}
\) -
Question 13 of 176
13. Question
In an electrical circuit, the voltage is measured as \(V=(200 \pm 4)\) volts and the current is measured as \(I=(20 \pm 0.2) \mathrm{A}\). The value of the resistance is: [NEET 2024]
CorrectIncorrectHint
(b) Use Ohm’s Law to calculate the resistance.
\(
\begin{aligned}
R & =\frac{V}{I} \\
R & =\frac{200 \mathrm{~V}}{20 \mathrm{~A}} \\
R & =10 \Omega
\end{aligned}
\)
Calculate the uncertainty in the voltage.
\(
\frac{\Delta V}{V}=\frac{4}{200}=0.02
\)
Calculate the uncertainty in the current.
\(
\frac{\Delta I}{I}=\frac{0.2}{20}=0.01
\)
Calculate the uncertainty in the resistance.
\(
\begin{aligned}
& \frac{\Delta R}{R}=\frac{\Delta V}{V}+\frac{\Delta I}{I} \\
& \frac{\Delta R}{R}=0.02+0.01=0.03
\end{aligned}
\)
Calculate the absolute uncertainty in the resistance.
\(
\begin{aligned}
& \Delta R=0.03 \cdot R \\
& \Delta R=0.03 \cdot 10 \Omega \\
& \Delta R=0.3 \Omega
\end{aligned}
\)
The value of the resistance is \((10 \pm 0.3) \Omega\). -
Question 14 of 176
14. Question
The amplitude of the charge oscillating in a circuit decreases exponentially as \(\mathrm{Q}=\mathrm{Q}_0 \mathrm{e}^{-R t / 2 L}\) where \(Q_0\) is the charge at \(t=0 \mathrm{~s}\). The time at which charge amplitude decreases to \(0.50 Q_0\) is nearly: [NEET 2024]
(Given that \(R=1.5 \Omega, L=12 \mathrm{mH}, \ln (2)=0.693\) )CorrectIncorrectHint
\(
\begin{aligned}
&\text { Substitute the given values into the equation for the charge amplitude. }\\
&\begin{aligned}
& Q=Q_0 e^{-R t / 2 L} \\
& 0.50 Q_0=Q_0 e^{-(1.5 \Omega) t / 2(12 \mathrm{mH})}
\end{aligned}
\end{aligned}
\)
\(
0.50=e^{-62.5 t}
\)
Take the natural logarithm of both sides of the equation.
\(
\begin{aligned}
& \ln (0.50)=\ln \left(e^{-62.5 t}\right) \\
& \ln (0.50)=-62.5 t
\end{aligned}
\)
\(
\begin{aligned}
&\text { Solve for } \boldsymbol{t} \text {. }\\
&\begin{aligned}
& t=\frac{\ln (0.50)}{-62.5} \\
& t=\frac{-\ln (2)}{-62.5} \\
& t=\frac{0.693}{62.5} \\
& t=0.01109 \mathrm{~s} \\
& t \approx 11.09 \mathrm{~ms}
\end{aligned}
\end{aligned}
\) -
Question 15 of 176
15. Question
The steady-state current in the circuit shown below is: [NEET 2024]
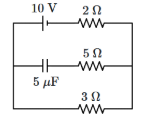 CorrectIncorrect
CorrectIncorrectHint
(c) In the steady state Capacitor will be fully charged and behave like open ciruit.
\(
I=\frac{V}{R}=\frac{10}{5}=2 A
\) -
Question 16 of 176
16. Question
A certain wire A has resistance \(81 \Omega\). The resistance of another wire B of same material and equal length but of diameter thrice the diameter of A will be : [NEET 2023 Manipur]
CorrectIncorrectHint
(b)
\(
\mathrm{R}=\frac{\rho \mathrm{L}}{\mathrm{~A}}
\)
If diameter becomes thrice then cross section area will become 9 times so
\(
\mathrm{R} \propto \frac{1}{\mathrm{~A}}
\)
Resistance will become \(\frac{1}{9}\) times
\(
\mathrm{R}^{\prime}=\frac{81 \Omega}{9}=9 \Omega
\) -
Question 17 of 176
17. Question
A copper wire of radius 1 mm contains \(10^{22}\) free electrons per cubic metre. The drift velocity for free electrons when 10 A current flows through the wire will be (Given, charge on electron \(\left.=1.6 \times 10^{-19} \mathrm{C}\right)\) : [NEET 2023 Manipur]
CorrectIncorrectHint
(b)
\(
\begin{aligned}
& \mathrm{I}=\mathrm{neAV}_{\mathrm{d}} \\
& \mathrm{~V}_{\mathrm{d}}=\frac{\mathrm{I}}{\mathrm{neA}}=\frac{10}{10^{22} \times 1.6 \times 10^{-19} \times \pi \times 10^{-6}} \\
& \mathrm{~V}_{\mathrm{d}}=\frac{6.25}{\pi} \times 10^3 \mathrm{~m} / \mathrm{sec}
\end{aligned}
\) -
Question 18 of 176
18. Question
The emf of a cell having internal resistance \(1 \Omega\) is balanced against a length of 330 cm on a potentiometer wire. When an external resistance of \(2 \Omega\) is connected across the cell, the balancing length will be : [NEET 2023 Manipur]
CorrectIncorrectHint
(a)
\(
\begin{aligned}
& \mathrm{r}=\left(\frac{\ell_{\mathrm{o}}-\ell_{\mathrm{c}}}{\ell_{\mathrm{c}}}\right) \mathrm{R} \\
& 1=\left(\frac{330-\ell_{\mathrm{c}}}{\ell_{\mathrm{c}}}\right) \times 2 \\
& 3 \ell_{\mathrm{c}}=660 \\
& \ell_{\mathrm{c}}=220 \mathrm{~cm}
\end{aligned}
\) -
Question 19 of 176
19. Question
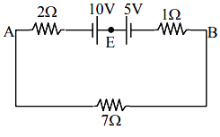
The magnitude and direction of the current in the following circuit is:
 CorrectIncorrect
CorrectIncorrectHint
(a)
\(
\mathrm{i}=\frac{10-5}{10}=\frac{5}{10} \mathrm{~A}
\)
\(
=0.5 \mathrm{~A}
\)
from \(A\) to \(B\) through \(E\). -
Question 20 of 176
20. Question
If the galvanometer \(G\) does not show any deflection in the circuit shown, the value of \(R\) is given by; [NEET 2023]
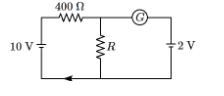 CorrectIncorrect
CorrectIncorrectHint
(d) For no reading galvanometer. Potential across it is same.
\(
\begin{aligned}
& \mathrm{i}_{400 \Omega} \Rightarrow \frac{10-2}{400}=\frac{8}{400}=\frac{1}{50}=\mathrm{i}_{\mathrm{R}} \\
& \mathrm{i}_{\mathrm{R}} \Rightarrow \frac{\mathrm{~V}_{\mathrm{R}}}{\mathrm{R}} \Rightarrow \frac{2}{\mathrm{R}}=\frac{1}{50} \Rightarrow \mathrm{R}=100 \Omega
\end{aligned}
\) -
Question 21 of 176
21. Question
10 resistors, each of resistance R are connected in series to a battery of emf \(E\) and negligible internal resistance. Then those are connected in parallel to the same battery, the current is increased \(n\) times. The value of \(n\) is : [NEET 2023]
CorrectIncorrectHint
(a)
\(
\begin{aligned}
& \mathrm{I}_{\mathrm{S}}=\frac{E}{10 R} \ldots . .(1) \\
& \mathrm{I}_{\mathrm{P}}=\frac{\mathrm{E}}{\mathrm{R} / 10}=\frac{10 \mathrm{E}}{\mathrm{R}} \ldots .(2) \\
& \mathrm{n}=\frac{\mathrm{I}_{\mathrm{P}}}{\mathrm{I}_{\mathrm{S}}}=100 \Rightarrow \mathrm{n}=100
\end{aligned}
\) -
Question 22 of 176
22. Question
The resistance of platinum wire at \(0^{\circ} \mathrm{C}\) is \(2 \Omega\) and \(6.8 \Omega\) at \(80^{\circ} \mathrm{C}\). The temperature coefficient of resistance of the wire is : [NEET 2023]
CorrectIncorrectHint
(b)
\(
\begin{aligned}
& \mathrm{R}_{\mathrm{T}}=\mathrm{R}_0\left[1+\alpha\left(\mathrm{T}-\mathrm{T}_0\right)\right] \\
& 6.8=2[1+\alpha(80-\alpha)] \\
& \alpha=\frac{2.4}{80}=0.03 /{ }^{\circ} \mathrm{C}=3 \times 10^{-2} /{ }^{\circ} \mathrm{C}
\end{aligned}
\) -
Question 23 of 176
23. Question
On the basis of electrical conductivity, which one of the following material has the smallest resistivity? [NEET 2023]
CorrectIncorrectHint
(b) Silver has the smallest resistivity.
Explanation: Resistivity is a measure of how much a material resists the flow of electric current, and a lower resistivity indicates better conductivity. Silver is considered the best electrical conductor among common metals, meaning it has the lowest resistivity.Why other options are incorrect:
Germanium: While a semiconductor, it has a much higher resistivity than silver.
Glass: Glass is an insulator, meaning it has extremely high resistivity.
Silicon: Like germanium, silicon is a semiconductor with higher resistivity than silver. -
Question 24 of 176
24. Question
A certain wire \(A\) has resistance \(81 \Omega\). The resistance of another wire \(B\) of the same material and equal length but of diameter thrice the diameter of \(A\) will be: [NEET 2023]
CorrectIncorrectHint
(b)
\(
\begin{aligned}
&\text { Write the resistance formula for wire } \boldsymbol{A} \text {. }\\
&R_A=\frac{\rho L_A}{A_A}
\end{aligned}
\)
\(
\begin{aligned}
&\text { Write the resistance formula for wire } B \text {. }\\
&R_B=\frac{\rho L_B}{A_B}
\end{aligned}
\)
Since the wires are made of the same material and have the same length, we can simplify the equations.
\(
\begin{aligned}
R_A & =\frac{\rho L}{A_A} \\
R_B & =\frac{\rho L}{A_B}
\end{aligned}
\)
\(
\begin{aligned}
&\text { Write the cross-sectional area of wire } A \text { in terms of its radius } \boldsymbol{r}_{\boldsymbol{A}} \text {. }\\
&A_A=\pi r_A^2
\end{aligned}
\)
\(
\begin{aligned}
&\text { Write the cross-sectional area of wire } B \text { in terms of its radius } r_{\boldsymbol{B}} \text {. }\\
&A_B=\pi r_B^2
\end{aligned}
\)
Since the diameter of wire \(B\) is thrice the diameter of wire \(A\), the radius of wire \(B\) is also thrice the radius of wire \(\boldsymbol{A}\).
\(
r_B=3 r_A
\)
\(
\begin{aligned}
&\text { Substitute } r_{\boldsymbol{B}}=3 r_A \text { into the equation for } \boldsymbol{A}_{\boldsymbol{B}} \text {. }\\
&\begin{aligned}
& A_B=\pi\left(3 r_A\right)^2 \\
& A_B=9 \pi r_A^2
\end{aligned}
\end{aligned}
\)
\(
\begin{aligned}
&\text { Substitute } A_B=9 \pi r_A^2 \text { into the equation for } R_B \text {. }\\
&R_B=\frac{\rho L}{9 \pi r_A^2}
\end{aligned}
\)
\(
\begin{aligned}
&\text { Substitute } A_A=\pi r_A^2 \text { into the equation for } \boldsymbol{R}_{\boldsymbol{A}} \text {. }\\
&R_A=\frac{\rho L}{\pi r_A^2}
\end{aligned}
\)
\(
\begin{aligned}
&\text { Compare the equations for } R_A \text { and } R_B \text {. }\\
&R_B=\frac{R_A}{9}
\end{aligned}
\)
\(
\begin{aligned}
&\text { Substitute } \boldsymbol{R}_{\boldsymbol{A}}=81 \boldsymbol{\Omega} \text { into the equation for } \boldsymbol{R}_{\boldsymbol{B}} \text { : }\\
&\begin{aligned}
& R_B=\frac{81 \Omega}{9} \\
& R_B=9 \Omega
\end{aligned}
\end{aligned}
\) -
Question 25 of 176
25. Question
The reciprocal of resistance is : [NEET 2022 Phase 2]
CorrectIncorrectHint
(a)
\(
\begin{aligned}
&R=\frac{1}{G}\\
&\text { Thus reciprocal of resistance (R) is conductance (G) }
\end{aligned}
\) -
Question 26 of 176
26. Question
A cell of emf 4 V and internal resistance \(0.5 \Omega\) is connected to a \(7.5 \Omega\) external resistance. The terminal potential difference of the cell is [NEET 2022 Phase 2]
CorrectIncorrectHint
(b)

From Kirchhoff’s loop law :
\(
\begin{aligned}
& V-I r-I R=0 \\
& \Rightarrow I=\left(\frac{V}{r+R}\right)
\end{aligned}
\)
Terminal potential difference across cell,
\(
\begin{aligned}
& V_{A B}=I R \\
& V_{A B}=\frac{R V}{r+R} \\
& =\frac{7.5 \times 4}{0.5+7.5}=3.75 \mathrm{~V}
\end{aligned}
\) -
Question 27 of 176
27. Question
Two resistors of resistance, \(100 \Omega\) and \(200 \Omega\) are connected in parallel in an electrical circuit. The ratio of the thermal energy developed in \(100 \Omega\) to that in \(200 \Omega\) in a given time is [NEET 2022]
CorrectIncorrectHint
(b)

\(
\begin{aligned}
&\text { As both resistors are in parallel combination so potential drop }(V) \text { across both are same. }\\
&\begin{aligned}
& P=\frac{V^2}{R} \Rightarrow P \propto \frac{1}{R} \\
& \frac{P_1}{P_2}=\frac{R_2}{R_1}=\frac{200}{100}=\frac{2}{1} \\
& =2: 1
\end{aligned}
\end{aligned}
\) -
Question 28 of 176
28. Question
A copper wire of length 10 m and radius \(\left(10^{-2} / \sqrt{\pi}\right) \mathrm{m}\) has an electrical resistance of \(10 \Omega\). The current density in the wire for an electric field strength of \(\mathbf{1 0 ( V / m )}\) is: [NEEt 2022]
CorrectIncorrectHint
(a) The radius \(r\) of the wire is given as:
\(
r=\frac{10^{-2}}{\sqrt{\pi}} \mathrm{~m}
\)
The cross-sectional area \(A\) of the wire can be calculated using the formula for the area of a circle:
\(
A=\pi r^2
\)
Substituting the value of \(r\) :
\(
A=\pi\left(\frac{10^{-2}}{\sqrt{\pi}}\right)^2=\pi\left(\frac{10^{-4}}{\pi}\right)=10^{-4} \mathrm{~m}^2
\)
The electric field \(E\) is given as \(10 \mathrm{~V} / \mathrm{m}\) and the length \(L\) of the wire is 10 m . The potential difference \(V\) across the wire can be calculated using the formula:
\(
V=E \cdot L
\)
Substituting the values:
\(
V=10 \mathrm{~V} / \mathrm{m} \times 10 \mathrm{~m}=100 \mathrm{~V}
\)
\(
\begin{aligned}
&I=\frac{V}{R}\\
&\text { Substituting the values: }\\
&I=\frac{100 \mathrm{~V}}{10 \Omega}=10 \mathrm{~A}
\end{aligned}
\)
Current density \(J\) is defined as the current per unit area:
\(
J=\frac{I}{A}
\)
Substituting the values of current \(I\) and area \(A\) :
\(
J=\frac{10 \mathrm{~A}}{10^{-4} \mathrm{~m}^2}=10 \times 10^4 \mathrm{~A} / \mathrm{m}^2=10^5 \mathrm{~A} / \mathrm{m}^2
\) -
Question 29 of 176
29. Question
A Wheatstone bridge is used to determine the value of unknown resistance \(X\) by adjusting the variable resistance \(Y\) as shown in the figure. For the most precise measurement of \(X\), the resistances \(P\) and \(Q\) : [NEET 2022]
 CorrectIncorrect
CorrectIncorrectHint
(b)
\(
\begin{aligned}
&\text { The resistances in wheat stone bridge follows: }\\
&\mathrm{P} / \mathrm{X}=\mathrm{Q} / \mathrm{Y}
\end{aligned}
\)
As \(P / X=Q / Y\) so we can conclude that Resistance of \(P\) and \(Q\) should be roughly identical since it reduces experiment error. -
Question 30 of 176
30. Question
The plot of current \(I(\mathrm{~A})\) flowing through a metallic conductor versus the applied voltage \(V\) (volt) across the ends of a conductor is: [NEET 2022]
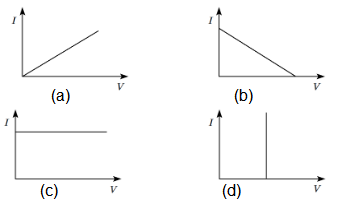 CorrectIncorrect
CorrectIncorrectHint
(a) The plot of current (I) versus the applied voltage (V) across a metallic conductor is generally a straight line, obeying Ohm’s law. Ohm’s law states that the current flowing through a conductor is directly proportional to the voltage applied across its ends, at a constant temperature:
\(
\mathrm{I}=\mathrm{V} / \mathrm{R}
\)
Where,
\(I\) is the current in amperes (A),
\(V\) is the voltage in volts \((\mathrm{V})\),
R is the resistance of the conductor in ohms \((\Omega)\). -
Question 31 of 176
31. Question
A network of resistors is connected across a 10 V battery with an internal resistance of \(1 \Omega\) as shown in the circuit diagram. The equivalent resistance of the circuit is: [NEET 2022]
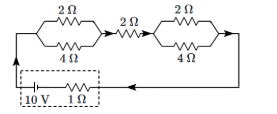 CorrectIncorrect
CorrectIncorrectHint
(a) \(\frac{4}{3}+\frac{4}{3}+2+1=\frac{17}{3}\)
-
Question 32 of 176
32. Question
In a meter bridge experiment, the null point is at a distance of 30 cm from \(A\). If a resistance of \(16 \Omega\) is connected in parallel with resistance \(Y\), the null point occurs at 50 cm from \(A\). The value of the resistance \(Y\) is: [NEET 2022]
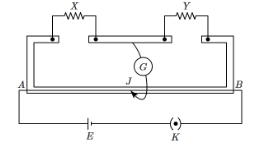 CorrectIncorrect
CorrectIncorrectHint
(c)
\(
\text { The formula for the meter bridge is } \frac{R}{S}=\frac{l}{100-l}
\)
Let \(R\) be the resistance in the left gap and \(Y\) be the resistance in the right gap.
Use the meter bridge formula to find the value of \(\frac{R}{Y}\).
\(
\begin{aligned}
\frac{R}{Y} & =\frac{l}{100-l} \\
\frac{R}{Y} & =\frac{30}{100-30} \\
\frac{R}{Y} & =\frac{30}{70} \\
\frac{R}{Y} & =\frac{3}{7}
\end{aligned}
\)
When a resistance of \(16 \Omega\) is connected in parallel with \(Y\), the equivalent resistance becomes \(\frac{16 Y}{16+Y}\).
Use the meter bridge formula again to find the value of \(\frac{R(16+Y)}{16 Y}\).
Substitute \(\frac{R}{Y}=\frac{3}{7}\) into the equation.
The value of the resistance \(Y\) is \(\frac{64}{3} \Omega\). -
Question 33 of 176
33. Question
The equivalent resistance of the infinite network given below is: [NEET 2022]
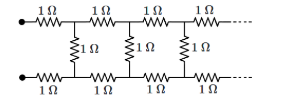 CorrectIncorrect
CorrectIncorrectHint
(c) Let net resistance of the given infinite network be ‘ \(R\) ‘
The above infinite network can be considered as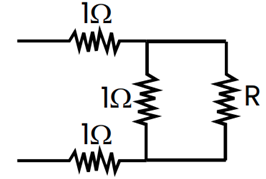
Now the total resistnace is: \(R=1+1+\frac{R}{R+1}\)
\(
\begin{aligned}
& \therefore R=2+\frac{R}{R+1} \\
& R^2+R=2 R+2+R \\
& R^2-2 R-2=0 \\
& R=\left(\frac{2 \pm \sqrt{4+8}}{2}\right) \\
& R=(1+\sqrt{3}) \Omega
\end{aligned}
\) -
Question 34 of 176
34. Question
The effective resistance of a parallel connection that consists of four wires of equal length, equal area of cross-section and same material is \(0.25 \Omega\). What will be the effective resistance if they are connected in series? [NEET 2021]
CorrectIncorrectHint
(a)
\(
\begin{aligned}
&\begin{aligned}
& \frac{R}{4}=.25 \\
& \mathrm{R}=1
\end{aligned}\\
&\text { Now these four resistances are arranged in series }\\
&\begin{aligned}
& \mathrm{R}_{\mathrm{S}}=\mathrm{R}+\mathrm{R}+\mathrm{R}+\mathrm{R}=4 \mathrm{R} \\
& =4 \times 1=4 \Omega
\end{aligned}
\end{aligned}
\) -
Question 35 of 176
35. Question
Match Column I and Column II with appropriate relations. [NEET 2021]
\(
\begin{array}{|l|l|l|l|}
\hline & \text { Column I } & & \text { Column II } \\
\hline \text { (A) } & \text { Drift Velocity } & \text { (P) } & \frac{\mathrm{m}}{\mathrm{ne}^2 \rho} \\
\hline \text { (B) } & \text { Electrical Resistivity } & \text { (Q) } & n e v_d \\
\hline \text { (C) } & \text { Relaxation Period } & \text { (R) } & \frac{\mathrm{eE}}{\mathrm{~m}} \tau \\
\hline \text { (D) } & \text { Current Density } & \text { (S) } & \frac{E}{J} \\
\hline
\end{array}
\)CorrectIncorrectHint
(a) Current density, \(\mathrm{J}=\frac{I}{A}=\) \(nev_{d}\)
Drift velocity, \({V}_{d}=\frac{e E}{m} \tau\);
Electrical resistivity, \(\rho=\frac{m}{n e^2 \tau}\) or \(\rho=\frac{E}{J}\)
Relaxation period, \(\tau=\frac{m}{n e^2 \rho}\)
\(A \rightarrow R\)
\(B \rightarrow S\)
\(D \rightarrow Q\)
\(C \rightarrow P\) -
Question 36 of 176
36. Question
Three resistors having resistances \(r_1, r_2\) and \(r_3\) are connected as shown in the given circuit. The ratio \(\frac{i_3}{i_1}\) of currents in terms of resistances used in the circuit is: [NEET 2021]
 CorrectIncorrect
CorrectIncorrectHint
(c)
\(
\begin{aligned}
&I_3=\frac{I_1 r_2}{r_1+r_3}\\
&\frac{I_3}{I_1}=\frac{I_1 r_2}{\left(r_2+r_3\right) I_1}=\frac{r_2}{r_2+r_3}
\end{aligned}
\) -
Question 37 of 176
37. Question
Which of the following graph represents the variation of resistivity \((\rho)\) with temperature \((T)\) for copper? [NEET 2020]
 CorrectIncorrect
CorrectIncorrectHint
(b) For some metals like copper, resistivity is nearly proportional to temperature although a non linear region always exists at very low temperature (\(\text { lower than } 0^{\circ} \mathrm{C}\))
-
Question 38 of 176
38. Question
A charged particle having drift velocity of \(7.5 \times 10^{-4} \mathrm{~ms}^{-1}\) in an electric field of \(3 \times 10^{-10} \mathrm{Vm}^{-1}\), has mobility of: [NEET 2020]
CorrectIncorrectHint
(a) The relation between the mobility and drift velocity is,
Mobility, \(\mu=\frac{v_d}{E}\)
\(
\begin{aligned}
& =\frac{7.5 \times 10^{-4}}{3 \times 10^{-10}} \\
& =2.5 \times 10^6 \mathrm{~m}^2 V^{-1} \mathrm{~s}^{-1}
\end{aligned}
\) -
Question 39 of 176
39. Question
A resistance wire connected in the left gap of a meter bridge balances a \(10 \Omega\) resistance in the right gap at a point which divides the bridge wire in the ratio \(3: 2\). If the length of the resistance wire is 1.5 m , then the length of \(1 \Omega\) of the resistance wire will be: [NEET 2020]
CorrectIncorrectHint
(a) Initially, \(\frac{P}{10}=\frac{l_1}{l_2}=\frac{3}{2}\)
\(
\Rightarrow P=\frac{30}{2}=15 \Omega
\)
Now Resistance, \(R=\frac{\rho l}{A}\)
\(
\begin{aligned}
& \frac{R_1}{R_2}=\frac{l_1}{l_2} \\
& \Rightarrow \frac{15}{1}=\frac{1.5}{l_2} \\
& l_2=0.1 m \\
& =1.0 \times 10^{-1} \mathrm{~m}
\end{aligned}
\) -
Question 40 of 176
40. Question
For the circuit shown in the figure, the current \(I\) will be: [NEET 2020]
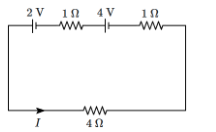 CorrectIncorrect
CorrectIncorrectHint
(b)
\(
I=\frac{2+4}{4+1+1}=\frac{6}{6}=1 A m p
\) -
Question 41 of 176
41. Question
Two solid conductors are made up of the same material and have the same length and the same resistance. One of them has a circular cross-section of area \(A_1\) and the other one has a square cross-section of area \(A_2\). The ratio of \(A_1 / A_2\) is: [NEET 2020]
CorrectIncorrectHint
(b)
\(
R=\frac{\rho L}{A}
\)
Since both conductors are made of the same material, have the same length, and the same resistance, we can write:
\(
R_1=R_2
\)
This implies:
\(
\frac{\rho L}{A_1}=\frac{\rho L}{A_2}
\)
Since \(\rho\) (resistivity) and \(L\) (length) are the same for both conductors, we can cancel them out from both sides of the equation:
\(
\frac{1}{A_1}=\frac{1}{A_2}
\)
Thus, the ratio of the areas is:
\(
\frac{A_1}{A_2}=1
\) -
Question 42 of 176
42. Question
The solids which have the negative temperature coefficient of resistance are [NEET 2020]
CorrectIncorrectHint
(c) The temperature coefficient of resistance is negative for semiconductor and insulators, and it is positive for metals.
-
Question 43 of 176
43. Question
For the circuit given below, Kirchhoff’s loop rule for the loop BCDEB is given by the equation:
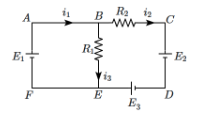 CorrectIncorrect
CorrectIncorrectHint
(b)
By \(K V L\)
\(
-I_2 R_2-E_2+E_3+I_3 R_1=0
\)
or
\(
I_2 R_2+E_2-E_3-I_3 R_1=0
\) -
Question 44 of 176
44. Question
The equivalent resistance between \(A\) and \(B\) for the mesh shown in the figure is: [NEET 2020]
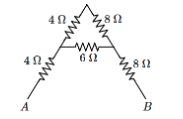 CorrectIncorrect
CorrectIncorrectHint
(b)
\(
\begin{aligned}
& R_{e q}=\left(4+8+\frac{12 \times 6}{12+6}\right) \Omega \\
& =(12+4) \Omega=16 \Omega
\end{aligned}
\) -
Question 45 of 176
45. Question
In the circuits shown below, the readings of the voltmeters and the ammeters will be: [NEET 2019]
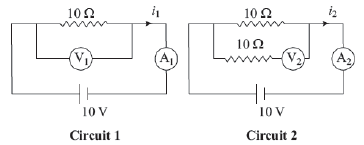 CorrectIncorrect
CorrectIncorrectHint
(c) Resistance for ideal voltmeter \(=\infty\)
Resistance for ideal ammeter \(=0\)
For \(1^{\text {st }}\) circuit,
\(
\mathrm{V}_1=\mathrm{i}_1 \times 10=\frac{10}{10} \times 10=10 \mathrm{volt}
\)
For \(2^{\text {nd }}\) circuit,
\(10 \Omega\) is in series with ideal voltmeter. Therefore it will not affect the circuit.
So, \(\mathrm{V}_2=\mathrm{i}_2 \times 10=\frac{10}{10} \times 10=10 \mathrm{volt}\)
\(
\therefore \mathrm{v}_1=\mathrm{V}_2
\)
Hence, \(\mathrm{i}_1=\mathrm{i}_2=\frac{10 \mathrm{~V}}{10 \Omega}=1 \mathrm{~A}\) -
Question 46 of 176
46. Question
Which of the following acts as a circuit protection device?
CorrectIncorrectHint
(a) Fuse is used as circuit protector
-
Question 47 of 176
47. Question
Six similar bulbs are connected as shown in the figure with a DC source of emf \(E\) and zero internal resistance. The ratio of power consumption by the bulbs when (i) all are glowing and (ii) in the situation when two from section \(\mathbf{A}\) and one from section \(\mathbf{B}\) are glowing, will be: [NEET 2019]
 CorrectIncorrect
CorrectIncorrectHint
(b) When all bulbs are glowing
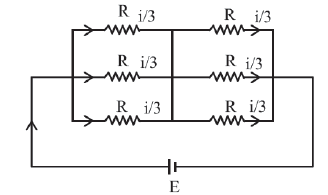
\(
\mathrm{R}_{\mathrm{eq}}=\frac{\mathrm{R}}{3}+\frac{\mathrm{R}}{3}=\frac{2 \mathrm{R}}{3}
\)
\(\operatorname{Power}\left(P_i\right)=\frac{E^2}{R_{e q}}=\frac{3 E^2}{2 R} \dots(i)\)
When two from section \(A\) and one from section \(B\) are glowing, then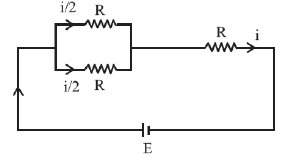
\(
\mathrm{R}_{\mathrm{eq}}=\frac{\mathrm{R}}{2}+\mathrm{R}=\frac{3 \mathrm{R}}{2}
\)
\(
\operatorname{Power}\left(\mathrm{P}_{\mathrm{f}}\right)=\frac{2 \mathrm{E}^2}{3 R} \dots(ii)
\)
Dividing equation (i) by (ii) we get
\(
\frac{P_i}{P_f}=\frac{3 E^2 3 R}{2 R 2 E^2}=9: 4
\) -
Question 48 of 176
48. Question
The reading of an ideal voltmeter in the circuit shown is: [NEET 2019]
 CorrectIncorrect
CorrectIncorrectHint
(a) Current in first branch \(=\frac{2}{50} \mathrm{~A}\)
Current in second branch \(=\frac{2}{50} \mathrm{~A}\) \(\Delta \mathrm{V}\) from A to P
\(
\Delta \mathrm{V}_1=2-\frac{2}{50} \times 20
\)
\(
\begin{aligned}
& \Delta \mathrm{V} \text { from } \mathrm{A} \text { to } \mathrm{Q} \\
& \Delta \mathrm{~V}_2=2-\frac{2}{50} \times 30 \\
\Rightarrow & \text { Voltage difference }=0.4 \mathrm{~V}
\end{aligned}
\) -
Question 49 of 176
49. Question
The metre bridge shown is in a balanced position with \(\frac{P}{Q}=\frac{l_1}{l_2}\). If we now interchange the position of the galvanometer and the cell, will the bridge work? If yes, what will be the balanced condition? [NEET 2019]
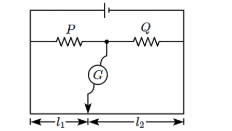 CorrectIncorrect
CorrectIncorrectHint
(d) Yes, the bridge will work. For a balanced condition, the current drawn from the battery will be zero
Also, \(p \propto l_1\) and \(Q \propto l_2\) Therefore, the condition \(\frac{P}{Q}=\frac{l_1}{l_2}\) will remain same after interchanging the cell and galvanometer. -
Question 50 of 176
50. Question
A set of ‘ \(n\) ‘ equal resistors, of value ‘ \(R\) ‘ each, are connected in series to a battery of emf ‘ \(E\) ‘ and internal resistance ‘ \(R\) ‘. The current drawn is \(I\). Now, if ‘ \(n\) ‘ resistors are connected in parallel to the same battery, then the current drawn becomes \(10 I\). The value of ‘ \(n\) ‘ is: [NEET 2018]
CorrectIncorrectHint
(a) In series grouping equivalent resistance
\(
\mathrm{R}_{\text {series }}=\mathrm{nR}
\)
In parallel grouping equivalent resistance
\(
\begin{aligned}
& \mathrm{R}_{\text {parallel }}=\frac{R}{n} \\
& I=\frac{E}{n R+R} \dots(i) \\
& 10 \mathrm{I}=\frac{E}{\frac{R}{n}+R} \dots(ii)
\end{aligned}
\)
Dividing eq. (ii) by (i),
\(
10=\frac{(n+1) R}{\left(\frac{1}{n}+1\right) R}
\)
Solving we get, \(\mathrm{n}=10\) -
Question 51 of 176
51. Question
A battery consists of a variable number \(n\) of identical cells (having internal resistance \(r\) each) which are connected in series. The terminals of the battery are short-circuited and the current \(I\) is measured. Which of the graphs shows the correct relationship between \(I\) and \(n\) ? [NEET 2018]
 CorrectIncorrect
CorrectIncorrectHint
(a) \(I=\frac{n E}{n r}=\frac{E}{r}\)
From the above equation, it is clear that the total current in the circuit is independent of \({n}\), the number of cells.
-
Question 52 of 176
52. Question
The resistance of a wire is \(R\) ohm. If it is melted and stretched to \(n\) times its original length, its new resistance will be: [NEET 2017]
CorrectIncorrectHint
(b) We know that, \(\mathrm{R}=\frac{\rho^{\ell}}{\mathrm{A}}\)
or \(\mathrm{R}=\frac{\rho \ell^2}{\text { Volume }} \Rightarrow \mathrm{R} \propto \ell^2\)
\(
\begin{aligned}
&\text { According to question } \ell_2=\mathrm{n} \ell_1\\
&\begin{aligned}
& \frac{R_2}{R_1}=\frac{\mathrm{n}^2 l_1^2}{l_1^2} \\
& \text { or, } \frac{\mathrm{R}_2}{\mathrm{R}_1}=\mathrm{n}^2 \\
& \Rightarrow \quad \mathrm{R}_2=\mathrm{n}^2 \mathrm{R}_1
\end{aligned}
\end{aligned}
\) -
Question 53 of 176
53. Question
A potentiometer is an accurate and versatile device to make electrical measurements of EMF because the method involves [NEET 2017]
CorrectIncorrectHint
(b) A potentiometer is an accurate and versatile device to make electrical measurements of E.M.F. because the method involves zero deflection without any current in galvanometer.
Note: Reading of potentiometer is accurate because during taking reading it does not draw any current from the circuit.
-
Question 54 of 176
54. Question
The figure shows a circuit that contains three identical resistors with resistance \(R=9.0 \Omega\) each, two identical inductors with inductance \(L=2.0 \mathrm{mH}\) each, and an ideal battery with emf \(\varepsilon=18 \mathrm{~V}\). The current ‘ \(i\) ‘ through the battery just after the switch is closed will be: [NEET 2017]
 CorrectIncorrect
CorrectIncorrectHint
(c) At time, \(\mathrm{t}=0\) i.e., when switch is closed, inductor in the circuit provides very high resistance (open circuit) while capacitor starts charging with maximum current (low resistance). Equivalent circuit of the given circuit Current drawn from battery,

\(
i=\frac{\varepsilon}{(R / 2)}=\frac{2 \varepsilon}{R}=\frac{2 \times 18}{9}=4 \mathrm{~A}
\) -
Question 55 of 176
55. Question
The sliding contact \(C\) is at one fourth of the length of the potentiometer wire \((A B)\) from \(A\) as shown in the circuit diagram. If the resistance of the wire \(A B\) is \(R_0\), then the potential drop \((\boldsymbol{V})\) across the resistor \(R\) is: [NEET 2022]
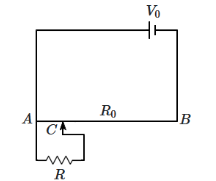 CorrectIncorrect
CorrectIncorrectHint
(a)

Equivalent resistance across point AC
\(
R_{A C}=\frac{\frac{R_0}{4} \times R}{\frac{R_0}{4}+R}=\frac{R R_0}{R_0+4 R}
\)
From voltage divider rule
\(
\begin{aligned}
& V_{A C}=\frac{R_{A C}}{R_{A C}+R_{C B}} V_0=\frac{\frac{R R_0}{R_0+4 R} V_0}{\frac{R R_0}{R_0+4 R}+\frac{3 R_0}{4}} \\
& =\frac{4 R R_0 V_0}{4 R R_0+3 R_0^2+12 R R_0}=\frac{4 R V_0}{3 R_0+16 R}
\end{aligned}
\) -
Question 56 of 176
56. Question
In a potentiometer circuit, a cell of emf 1.5 V gives a balance point at 36 cm length of wire. If another cell of emf 2.5 V replaces the first cell, then at what length of the wire, the balance point occur? [NEET 2021]
CorrectIncorrectHint
(c) Use the Proportionality Relation:
The relationship between the EMFs and the lengths is given by:
\(
\frac{V 1}{V 2}=\frac{L 1}{L 2}
\)
Rearrange the Equation to Find L2
Rearranging the equation gives:
\(
L 2=\frac{L 1 \times V 2}{V 1}
\)
Substitute the Known Values:
Substitute the known values into the equation:
\(
L 2=\frac{36 \mathrm{~cm} \times 2.5 \mathrm{~V}}{1.5 \mathrm{~V}}
\)
Calculate L2:
\(
\begin{aligned}
& L 2=\frac{36 \times 2.5}{1.5} \\
& L 2=\frac{90}{1.5} \\
& L 2=60 \mathrm{~cm}
\end{aligned}
\) -
Question 57 of 176
57. Question
The potential difference \(\left(V_A-V_B\right)\) between the points \(A\) and \(B\) in the given figure is [ NEET 2016]
 CorrectIncorrect
CorrectIncorrectHint
(d) \(V_A-V_B=(2 A \times 2 \Omega)+3 V+(2 A \times 1 \Omega)=4 V+3 V+2 V=9 V\)
-
Question 58 of 176
58. Question
A filament bulb \((500 \mathrm{~W}, 100 \mathrm{~V})\) is to be used in a 230 V main supply. When a resistance \(R\) is connected in series, the bulb works perfectly and consumes 500 W . The value of \(R\) is: [NEET 2016]
CorrectIncorrectHint
(c) Resistance of bulb, \(R_B=\frac{V^2}{P}=\frac{(100)^2}{500}=20 \Omega\)
Power of the bulb in the circuit?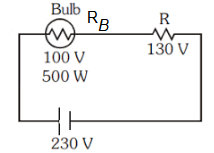
\(
\begin{aligned}
P & =V I \\
I & =\frac{P}{V_B} \\
& =\frac{500}{100}=5 \mathrm{~A}
\end{aligned}
\)
\(
\begin{aligned}
& V_R=I R \Leftrightarrow(230-100)=5 \times R \\
\therefore \quad & R=26 \Omega
\end{aligned}
\) -
Question 59 of 176
59. Question
A potentiometer wire is 100 cm long and a constant potential difference is maintained across it. Two cells are connected in series first to support one another and then in opposite directions. The balance points are obtained at 50 cm and 10 cm from the positive end of the wire in the two cases. The ratio of the emf of the two cells is: [NEET 2016]
CorrectIncorrectHint
(c) Suppose two cells have emfs \(\varepsilon_1\) and \(\varepsilon_2\) (also \(\varepsilon_1>\varepsilon_2\) ).
Potential difference per unit length of the potentiometer wire \(=k\) (say)
When \(\varepsilon_1\) and \(\varepsilon_2\) are in series and support each other then
\(
\varepsilon_1+\varepsilon_2=50 \times k \dots(i)
\)
When \(\varepsilon_1\) and \(\varepsilon_2\) are in opposite direction
\(
\varepsilon_1-\varepsilon_2=10 \times k \dots(ii)
\)
On adding eqn. (i) and eqn. (ii)
\(
\begin{aligned}
2 \varepsilon_1 & =60 k \Rightarrow \varepsilon_1=30 k \text { and } \varepsilon_2=50 k-30 k=20 k \\
\therefore \quad \frac{\varepsilon_1}{\varepsilon_2} & =\frac{30 k}{20 k}=\frac{3}{2}
\end{aligned}
\) -
Question 60 of 176
60. Question
The charge flowing through a resistance R varies with time \(t\) as \(Q=a t-b t^2\), where \(a\) and \(b\) are positive constants. The total heat produced in R is: [NEET 2016]
CorrectIncorrectHint
(a) Given, \(Q=a t-b t^2\)
\(
\therefore \quad I=\frac{d Q}{d t}=a-2 b t
\)
At \(t=0, Q=0 \Rightarrow I=0\)
Also, \(I=0\) at \(t=a / 2 b\)
\(\therefore \quad\) Total heat produced in resistance \(R\),
\(
H=\int_0^{a / 2 b} I^2 R d t=R \int_0^{a / 2 b}(a-2 b t)^2 d t
\)
\(
=\frac{a^3 R}{b}\left[\frac{1}{2}+\frac{1}{6}-\frac{1}{2}\right]=\frac{a^3 R}{6 b}
\) -
Question 61 of 176
61. Question
Two metal wires of identical dimensions are connected in series. If \(\sigma_1\) and \(\sigma_2\) are the conductivity of the combination is [NEET 2015]
CorrectIncorrectHint
(c)

As both metal wires are of identical dimensions, so their length and area of cross-section will be same. Let them be \(l\) and \(A\) respectively. Then The resistance of the first wire is
\(
R_1=\frac{l}{\sigma_1 A} \dots(i)
\)
and that of the second wire is
\(
R_2=\frac{l}{\sigma_2 A} \dots(ii)
\)
As they are connected in series, so their effective resistance is
\(
\begin{aligned}
R_s & =R_1+R_2 \\
& =\frac{l}{\sigma_1 A}+\frac{l}{\sigma_2 A} \quad \text { (using (i) and (ii)) } \\
& =\frac{l}{A}\left(\frac{1}{\sigma_1}+\frac{1}{\sigma_2}\right) \dots(iii)
\end{aligned}
\)
If \(\sigma_{\text {eff }}\) is the effective conductivity of the combination, then
\(
R_s=\frac{2 l}{\sigma_{\mathrm{eff}} A} \dots(iv)
\)
\(
\begin{aligned}
&\text { Equating eqns. (iii) and (iv), we get }\\
&\begin{aligned}
& \frac{2 l}{\sigma_{\mathrm{eff}} A}=\frac{l}{A}\left(\frac{1}{\sigma_1}+\frac{1}{\sigma_2}\right) \\
& \frac{2}{\sigma_{\mathrm{eff}}}=\frac{\sigma_2+\sigma_1}{\sigma_1 \sigma_2} \text { or } \quad \sigma_{\mathrm{eff}}=\frac{2 \sigma_1 \sigma_2}{\sigma_1+\sigma_2}
\end{aligned}
\end{aligned}
\) -
Question 62 of 176
62. Question
A potentiometer wire of length \(L\) and a resistance \(r\) are connected in series with a battery of e.m.f. \(E_0\) and a resistance \(r_1\). An unknown e.m.f. \(E\) is balanced at a length \(l\) of the potentiometer wire. The e.m.f. \(E\) will be given by [NEET 2015]
CorrectIncorrectHint
(d)
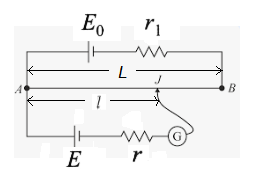
The current through the potentiometer wire is
\(
I=\frac{E_0}{\left(r+r_1\right)}
\)
and the potential difference across the wire is
\(
V=I r=\frac{E_0 r}{\left(r+r_1\right)}
\)
The potential gradient along the potentiometer wire is
\(
k=\frac{V}{L}=\frac{E_0 r}{\left(r+r_1\right) L}
\)
As the unknown e.m.f. \(E\) is balanced against length \(l\) of the potentiometer wire,
\(
\therefore \quad E=k l=\frac{E_0 r}{\left(r+r_1\right)} \frac{l}{L}
\) -
Question 63 of 176
63. Question
A circuit contains an ammeter, a battery of 30 V and a resistance 40.8 ohm all connected in series. If the ammeter has a coil of resistance 480 ohm and a shunt of 20 ohm , the reading in the ammeter will be [NEET 2015]
CorrectIncorrectHint
(c)

Resistance of the ammeter is
\(
R_A=\frac{(480 \Omega)(20 \Omega)}{(480 \Omega+20 \Omega)}=19.2 \Omega
\)
(As \(480 \Omega\) and \(20 \Omega\) are in parallel)
As ammeter is in series with \(40.8 \Omega\),
\(\therefore\) Total resistance of the circuit is
\(
R=40.8 \Omega+R_A=40.8 \Omega+19.2 \Omega=60 \Omega
\)
By Ohm’s law,
Current in the circuit is
\(
I=\frac{V}{R}=\frac{30 \mathrm{~V}}{60 \Omega}=\frac{1}{2} \mathrm{~A}=0.5 \mathrm{~A}
\) -
Question 64 of 176
64. Question
A potentiometer wire has length 4 m and resistance \(8 \Omega\). The resistance that must be connected in series with the wire and an accumulator of e.m.f. 2 V , so as to get a potential gradient 1 mV per cm on the wire is [NEET 2015]
CorrectIncorrectHint
(c)

\(
\begin{aligned}
& \text { Required potential gradient }=1 \mathrm{mV} \mathrm{~cm} \\
& =\frac{1}{10} \mathrm{Vm}^{-1}
\end{aligned}
\)
Length of potentiometer wire, \(l=4 \mathrm{~m}\)
So potential difference across potentiometer wire
\(
=\frac{1}{10} \times 4=0.4 \mathrm{~V} \dots(i)
\)
In the circuit, potential difference across \(8 \Omega\)
\(
=I \times 8=\frac{2}{8+R} \times 8 \dots(ii)
\)
\(
\begin{aligned}
&\text { Using equation (i) and (ii), we get, } 0.4=\frac{2}{8+R} \times 8\\
&\begin{aligned}
& \frac{4}{10}=\frac{16}{8+R}, 8+R=40 \\
\therefore \quad & R=32 \Omega
\end{aligned}
\end{aligned}
\) -
Question 65 of 176
65. Question
\(A, B\) and \(C\) are voltmeters of resistance \(R, 1.5 R\) and \(3 R\) respectively as shown in the figure above. When some potential difference is applied between \(X\) and \(Y\), the voltmeter readings are \(V_A, V_B\) and \(V_C\) respectively. Then: [NEET 2015]
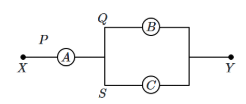 CorrectIncorrect
CorrectIncorrectHint
(c)
Effective resistance of B and C
\(
=\frac{\mathrm{R}_{\mathrm{B}} \cdot \mathrm{R}_{\mathrm{C}}}{\mathrm{R}_{\mathrm{B}}+\mathrm{R}_{\mathrm{C}}}=\frac{1.5 \mathrm{R} \times 3 \mathrm{R}}{1.5 \mathrm{R}+3 \mathrm{R}}=\frac{4.5 \mathrm{R}^2}{4.5 \mathrm{R}}=\mathrm{R}
\)
i.e., equal to resistance of voltmeter A .
In parallel potential difference is same so, \(\mathrm{V}_{\mathrm{B}}=\mathrm{V}_{\mathrm{C}}\) and in series current is same So, \(\mathrm{V}_{\mathrm{A}}=\mathrm{V}_{\mathrm{B}}=\mathrm{V}_{\mathrm{C}}\) -
Question 66 of 176
66. Question
Across a metallic conductor of non-uniform cross section a constant potential difference is applied. The quantity which remains constant along the conductor is [NEET 2015]
CorrectIncorrectHint
(d) The area of cross section of conductor is non uniform so current density will be different but the flow of electrons will be uniform so current will be constant.
-
Question 67 of 176
67. Question
Two cities are 150 km apart. The electric power is sent from one city to another city through copper wires. The fall of potential per km is 8 volts and the average resistance per km is 0.5 ohm . The power loss in the wire is: [NEET 2014]
CorrectIncorrectHint
(b) Distance between twe cities \(=150 \mathrm{~km}\)
Resistance of the wire, \(R=\left(0.5 \Omega \mathrm{~km}^{-1}\right)(150 \mathrm{~km})=75 \Omega\)
Voltage drop across the wire,
\(
V=\left(8 \mathrm{~V} \mathrm{km}^{-1}\right)(150 \mathrm{~km})=1200 \mathrm{~V}
\)
Power loss in the wire is
\(
P=\frac{V^2}{R}=\frac{(1200 \mathrm{~V})^2}{75 \Omega}=19200 \mathrm{~W}=19.2 \mathrm{~kW}
\) -
Question 68 of 176
68. Question
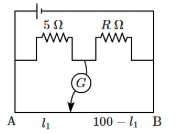
The figure given below shows a circuit when resistances in the two arms of the meter bridge are \(5 \Omega\) and \(R\), respectively. When the resistance \(R\) is shunted with equal resistance, the new balance point is at \(1.6 l_1\). The resistance \(R\) is: [NEET 2014]
 CorrectIncorrect
CorrectIncorrectHint
(b) In the first case: This is a balanced wheatstone bridge condition,
\(
\frac{5}{R}=\frac{\ell_1}{100-\ell_1} \dots(i)
\)
In the second case:
At balance point
\(
\frac{5}{(R / 2)}=\frac{1.6 l_1}{100-1.6 l_1} \dots(ii)
\)
Divide eqn. (i) by eqn. (ii), we get
\(
\begin{aligned}
& \frac{1}{2}=\frac{100-1.6 l_1}{1.6\left(100-l_1\right)} \\
& 160-1.6 l_1=200-3.2 l_1 \\
& 1.6 l_1=40 \text { or } l_1=\frac{40}{1.6}=25 \mathrm{~cm}
\end{aligned}
\)
Substituting this value in eqn. (i), we get
\(
\begin{aligned}
& \frac{5}{R}=\frac{25}{75} \\
& R=\frac{375}{25} \Omega=15 \Omega
\end{aligned}
\) -
Question 69 of 176
69. Question
A potentiometer circuit has been set up for finding the internal resistance of a given cell. The main battery, used across the potentiometer wire, has an emf of 2.0 V and negligible internal resistance. The potentiometer wire itself is 4 m long. When the resistance, \(R\), connected across the given cell, has values of (i) infinity (ii) 9.5 , the ‘balancing lengths, on the potentiometer wire, are found to be 3 m and 2.85 m , respectively. The value of the internal resistance of the cell is (in ohm): [NEET 2014]
CorrectIncorrectHint
(c) The internal resistance of the cell is
\(
r=\left(\frac{l_1}{l_2}-1\right) R
\)
Here, \(l_1=3 \mathrm{~m}, l_2=2.85 \mathrm{~m}, R=9.5 \Omega\)
\(
\therefore \quad r=\left(\frac{3}{2.85}-1\right)(9.5 \Omega)=\frac{0.15}{2.85} \times 9.5 \Omega=0.5 \Omega
\) -
Question 70 of 176
70. Question
A wire of resistance \(4 \Omega\) is stretched to twice its original length. The resistance of a stretched wire would be: [NEET 2013]
CorrectIncorrectHint
(d) Resistance of a wire, \(R=\rho \frac{l}{A}=4 \Omega\)
When wire is stretched twice, its new length be \(l^{\prime}\). Then
\(
l^{\prime}=2 l
\)
On stretching volume of the wire remains constant.
\(\therefore l A=l^{\prime} A^{\prime}\) where \(A^{\prime}\) is the new cross-sectional area or \(\quad A^{\prime}=\frac{l}{l^{\prime}} A=\frac{l}{2 l} A=\frac{A}{2}\)
\(\therefore \quad\) Resistance of the stretched wire is
\(
\begin{aligned}
R^{\prime} & =\rho \frac{l^{\prime}}{A^{\prime}}=\rho \frac{2 l}{(A / 2)}=4 \rho \frac{l}{A} \\
& =4(4 \Omega)=16 \Omega \text { (Using (i)) }
\end{aligned}
\) -
Question 71 of 176
71. Question
The internal resistance of a 2.1 V cell which gives a current of 0.2 A through a resistance of \(10 \Omega\) is: [NEET 2013]
CorrectIncorrectHint
(a)
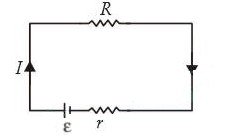
\(
\begin{aligned}
&\begin{aligned}
& I=\frac{\varepsilon}{R+r} \\
& \Rightarrow I R+I r=\varepsilon
\end{aligned}\\
&\text { Here, }\\
&\begin{aligned}
& R=10 \Omega, r=?, \varepsilon=2.1 V, I=0.2 A \\
& \therefore 0.2 \times 10+0.2 \times r=2.1 \\
& 2+0.2 r=2.1 \\
& 0.2 r=0.1 \Rightarrow r=\frac{1}{2}=0.5 \Omega
\end{aligned}
\end{aligned}
\) -
Question 72 of 176
72. Question
The resistances of the four arms \(P, Q, R\) and \(S\) in a Wheatstone’s bridge are \(10 \Omega, 30 \Omega, 30 \Omega\) and \(90 \Omega\) respectively. The emf and internal resistance of the cell are 7 V and \(5 \Omega\) respectively. If the galvanometer resistance is \(50 \Omega\) the current drawn from the cell will be: [NEET 2013]
CorrectIncorrectHint
(a) For a balanced Wheatstone’s bridge
\(
\begin{aligned}
& \frac{P}{Q}=\frac{R}{S} \\
\therefore & \frac{10 \Omega}{30 \Omega}=\frac{30 \Omega}{90 \Omega} \text { or } \frac{1}{3}=\frac{1}{3}
\end{aligned}
\)
It is a balanced Wheatstone’s bridge. Hence no current flows in the galvanometerarm. Hence, resistance 50 \(\Omega\) becomes ineffective.
\(\therefore\) The equisalent resistance of the circuit is
\(
\begin{aligned}
\mathrm{R}_{\mathrm{qg}} & =5 \Omega+\frac{(10 \Omega+30 \Omega)(30 \Omega+90 \Omega)}{(10 \Omega+30 \Omega)+(30 \Omega+90 \Omega)} \\
& =5 \Omega+\frac{(40 \Omega)(120 \Omega)}{40 \Omega+120 \Omega}=5 \Omega+30 \Omega=35 \Omega
\end{aligned}
\)
Current drawn from the cell is
\(
I=\frac{7 \mathrm{~V}}{35 \Omega}=\frac{1}{5} \mathrm{~A}=0.2 \mathrm{~A}
\) -
Question 73 of 176
73. Question
Ten identical cells connected in series are needed to heat a wire of length one meter and radius ‘\(r\)’ by \(10^{\circ} \mathrm{C}\) in time \(t\) . How many cells will be required to heat the wire of length two meter of the same radius by the same temperature in time ‘\(t\)’? [NEET 2013]
CorrectIncorrectHint
(a) Let \(\rho\) be the resistivity of the material of the wire and r be the radius of the wire.
Therefore, the resistance of 1 m wire is
\(
\begin{aligned}
& R=\frac{\rho(1)}{\pi r^2}=\frac{\rho}{\pi r^2} \\
& \left(\because R=\frac{\rho l}{A}\right)
\end{aligned}
\)
Let \(\varepsilon\) be emf of each cell.
In the first case, 10 cells each of emf \(\varepsilon\) are connected in series to heat the wire of length 1 m by \(\Delta\) \(T\left(=10^{\circ} \mathrm{C}\right)\) in time t.
\(
\therefore \frac{(10 \varepsilon)}{R} r=m s \Delta T \quad \ldots \text { (i) }
\)
In second case, Resistance of same wire of length 2 m is
\(
R^{\prime}=\frac{\rho(2)}{\pi r^2}=\frac{2 \rho}{\pi r^2}=2 R
\)
Let n cells each of emf \(\varepsilon\) are connected in series to heat the same wire of length 2 m , by the same temperature \(\Delta \mathrm{T}\left(=10^{\circ} \mathrm{C}\right)\) in the same time t.
\(
\therefore \frac{(n \varepsilon)^2 t}{2 R}=(2 m) s \Delta T
\)
Divide (ii) by (i), we get
\(
\begin{aligned}
& \frac{n^2}{200}=2 \Rightarrow n^2=400 \\
& \therefore n=20
\end{aligned}
\) -
Question 74 of 176
74. Question
Two rods are joined end to end, as shown. Both have a cross-sectional area of \(0.01 \mathrm{~cm}^2\). Each is 1 m long. One rod is of copper with a resistivity of \(1.7 \times 10^{-6} \Omega\)-cm, the other is of iron with a resistivity of \(10^{-5} \Omega-\mathrm{cm}\). How much voltage is required to produce a current of 1 A in the rods? [NEET 2013]
 CorrectIncorrect
CorrectIncorrectHint
(d) Here,
Length of each rod, \(l=1 \mathrm{~m}\)
Area of cross-section of each rod,
\(
A=0.01 \mathrm{~cm}^2=0.01 \times 10^{-4} \mathrm{~m}^2
\)
Resistivity of copper rod,
\(
\begin{aligned}
\rho_{\mathrm{cu}} & =1.7 \times 10^{-6} \Omega \mathrm{~cm} \\
& =1.7 \times 10^{-6} \times 10^{-2} \Omega \mathrm{~m}=1.7 \times 10^{-8} \Omega \mathrm{~m}
\end{aligned}
\)
Resistivity of iron rod,
\(
\begin{aligned}
\rho_{\mathrm{Fe}} & =10^{-5} \Omega \mathrm{~cm} \\
& =10^{-5} \times 10^{-2} \Omega \mathrm{~m}=10^{-7} \Omega \mathrm{~m}
\end{aligned}
\)
\(\therefore\) Resistance of copper rod,
\(
R_{\mathrm{Cu}}=\rho_{\mathrm{Cu}} \frac{l}{A}
\)
and resistance of iron rod, \(R_{\mathrm{Fe}}=\rho_{\mathrm{Fe}} \frac{l}{A}\)
As copper and iron rods are connected in series, therefore equivalent resistance is
\(
R=R_{\mathrm{Cu}}+R_{\mathrm{Fe}}=\rho_{\mathrm{Cu}} \frac{l}{A}+\rho_{\mathrm{Fe}} \frac{l}{A}=\left(\rho_{\mathrm{Cu}}+\rho_{\mathrm{Fe}}\right) \frac{l}{A}
\)
\(
\begin{aligned}
&\text { Voltage required to produce } 1 \text { A current in the tods is }\\
&\begin{aligned}
V & =I R=(1)\left(R_{\mathrm{Cu}}+R_{\mathrm{Fe}}\right) \\
& =\left(\rho_{\mathrm{Cu}}+\rho_{\mathrm{Fe}}\right)\left(\frac{l}{A}\right) \\
& =\left(1.7 \times 10^{-8}+10^{-7}\right)\left(\frac{1}{0.01 \times 10^{-4}}\right) \mathrm{V} \\
& =10^{-7}(0.17+1)\left(10^6\right) \mathrm{V}=17 \times 10^{-1} \mathrm{~V}=0.117 \mathrm{~V}
\end{aligned}
\end{aligned}
\) -
Question 75 of 176
75. Question
A 12 cm wire is given a shape of a right-angled triangle ABC having sides \(3 \mathrm{~cm}, 4 \mathrm{~cm}\) and 5 cm , as shown in the figure. The resistance between two ends \((\mathrm{AB}, \mathrm{BC}, \mathrm{CA})\) of the respective sides are measured one by one by a multi-meter. The resistances will be in the ratio: [NEET 2013]
 CorrectIncorrect
CorrectIncorrectHint
(c) Resistance is directly proportional to length,
\(
\begin{aligned}
& \frac{1}{R_{A B}}=\frac{1}{3}+\frac{1}{4+5}=\frac{(4+5)+3}{(3)(4+5)} \\
& R_{A B}=\frac{3 \times(4+5)}{3+(4+5)}=\frac{27}{12}
\end{aligned}
\)
Similarly,
\(
\begin{aligned}
& R_{B C}=\frac{4 \times(3+5)}{4+(3+5)}=\frac{32}{12} \\
& R_{A C}=\frac{5 \times(3+4)}{5+(3+4)}=\frac{35}{12} \\
& \therefore R_{A B}: R_{B C}: R_{A C}=27: 32: 35
\end{aligned}
\) -
Question 76 of 176
76. Question
In the circuit shown cells, \(A\) and \(B\) have negligible resistance. For \(V_A=12 \mathrm{~V}, R_1=500 \Omega\), and \(R=100 \Omega\), the galvanometer (G) shows no deflection. The value of \(V_B\) is:
 CorrectIncorrect
CorrectIncorrectHint
(b) Since the galvanometer shows no deflection so current will flow as shown in the figure.
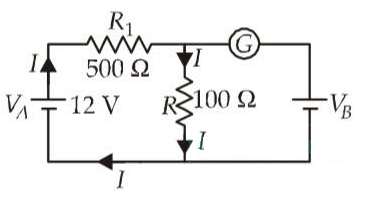
\(
\begin{aligned}
&\text { Current, }\\
&\begin{aligned}
& I=\frac{V_A}{R_1+R}=\frac{12 V}{(500+100) \Omega}=\frac{12}{600} A \\
& V_B=I R=\left(\frac{12}{600} A\right)(100 \Omega)=2 V
\end{aligned}
\end{aligned}
\) -
Question 77 of 176
77. Question
If the voltage across a bulb rated \((220 \mathrm{~V}-100 \mathrm{~W})\) drops by \(2.5 \%\) of its rated value, the percentage of the rated value by which the power would decrease is: [NEET 2012]
CorrectIncorrectHint
(c) Power, \(P=\frac{V^2}{R}\)
As the resistance of the bulb is constant
\(
\begin{aligned}
& \therefore \quad \frac{\Delta P}{P}=\frac{2 \Delta V}{V} \\
& \% \text { decrease in power }=\frac{\Delta P}{P} \times 100=\frac{2 \Delta V}{V} \times 100 \\
& =2 \times 2.5 \%=5 \%
\end{aligned}
\) -
Question 78 of 176
78. Question
A ring is made of a wire having a resistance of \(R_0=12 \Omega\). Find points A and B , as shown in the figure, at which a current-carrying conductor should be connected so that the resistance \(R\) of the subcircuit between these points equals \(\frac{8}{3} \Omega\) ? [NEET 2012]
 CorrectIncorrect
CorrectIncorrectHint
(d) Let \(x\) is the resistance per unit length then

\(
\text { equivalent resistance } R=\frac{R_1 R_2}{R_1+R_2} \frac{\left(x l_1\right)\left(x l_2\right)}{x l_1+x l_2}
\)
\(
\begin{aligned}
& \Rightarrow \quad \frac{8}{3}=x \frac{l_1 l_2}{l_1+l_2} \\
& \frac{8}{3}=x \frac{l_1}{\frac{l_1}{l_2}+1} \dots(i)
\end{aligned}
\)
\(
\text { also } \begin{aligned}
R_0 & =x l_1+x l_2 \\
12 & =x\left(l_1+l_2\right) \\
12 & =x l_2\left(\frac{l_1}{l_2}+1\right) \dots(ii)
\end{aligned}
\)
Divide (i) by (ii), we get
\(
\frac{\frac{8}{3}}{\frac{12}{1}}=\frac{\frac{x l_1}{\left(\frac{l_1}{l_2}+1\right)}}{x l_2\left(\frac{l_1}{l_2}+1\right)}=\frac{l_1}{l_2\left(\frac{l_1}{l_2}+1\right)^2}
\)
\(
\left(\frac{l_1}{l_2}+1\right)^2 \times \frac{8}{36}=\frac{l_1}{l_2}
\)
\(
\begin{aligned}
& \left(y^2+1+2 y\right) \times \frac{8}{36}=y\left(\text { where } y=\frac{l_1}{l_2}\right) \\
& \quad 8 y^2+8+16 y=36 y \\
& \Rightarrow 8 y^2-20 y+8=0 \\
& \Rightarrow 2 y^2-5 y+2=0 \\
& \Rightarrow 2 y^2-4 y-y+2=0 \\
& \Rightarrow 2 y(y-2)-1(y-2)=0 \\
& \Rightarrow \\
& (2 y-1)(y-2)=0 \\
& \Rightarrow \quad y=\frac{l_1}{l_2}=\frac{1}{2} \text { or } 2
\end{aligned}
\) -
Question 79 of 176
79. Question
The power dissipated in the circuit shown in the figure is 30 Watts. The value of \(R\) is: [NEET 2012]
 CorrectIncorrect
CorrectIncorrectHint
(b) The equivalent resistance of the given circuit is
\(
R_{\mathrm{eq}}=\frac{5 R}{5+R}
\)
Power dissipated in the given circuit is
\(
\begin{aligned}
& P=\frac{V^2}{R_{\mathrm{eq}}} \text { or } 30=\frac{(10)^2}{\left(\frac{5 R}{5+R}\right)} \\
& 150 R=100(5+R) \\
& 150 R=500+100 R \text { or } 50 R=500 \\
& R=\frac{500}{50}=10 \Omega
\end{aligned}
\) -
Question 80 of 176
80. Question
A cell having an emf \(\varepsilon\) and internal resistance \(r\) is connected across a variable external resistance \(R\). As the resistance \(R\) is increased, the plot of potential difference \(V\) across \(R\) is given by: [NEET 2012]
 CorrectIncorrect
CorrectIncorrectHint
(b)
Current in the circuit, \(I=\frac{\varepsilon}{R+r}\)
Potential difference across R,
\(
\begin{aligned}
& V=I R=\left(\frac{\varepsilon}{R+r}\right) R \\
& V=\frac{\varepsilon}{1+\frac{r}{R}}
\end{aligned}
\)
When \(\mathrm{R}=0, \mathrm{~V}=0\) and when \(\mathrm{R}=\infty, \mathrm{V}=\varepsilon\)
Thus \(V\) increases as \(R\) increases upto certain limit, but it does not increase fürther. -
Question 81 of 176
81. Question
If power dissipated in the \(9 \Omega\) resistor in the circuit shown is 36 W , the potential difference across the \(2 \Omega\) resistor will be: [NEET 2011]
 CorrectIncorrect
CorrectIncorrectHint
(b) Current flows through the \(9 \Omega\) resistor is
\(
\begin{aligned}
& I_1^2=\frac{36}{9}=4 \text { (As } P=I^2 R \text { ) }\\
& I_1=2 \mathrm{~A}
\end{aligned}
\)
As the resistors \(9 \Omega\) and \(6 \Omega\) are connected in parallel, therefore potential difference across them is same.
\(
\begin{aligned}
\therefore \quad 9 I_1= & 6 I_2 \\
& I_2=\frac{9 \times 2}{6}=3 \mathrm{~A}
\end{aligned}
\)
Current drawn from the battery is
\(
I=I_1+I_2=(2+3) \mathrm{A}=5 \mathrm{~A}
\)
The potential difference across the \(2 \Omega\) resistor is
\(
=(5 \mathrm{~A})(2 \Omega)=10 \mathrm{~V}
\) -
Question 82 of 176
82. Question
A current of 2 A flows through a \(2 \Omega\) resistor when connected across a battery. The same battery supplies a current of 0.5 A when connected across a \(9 \Omega\) resistor. The internal resistance of the battery is: [NEET 2011]
CorrectIncorrectHint
(b) Let \(\varepsilon\) be the emf and \(r\) be internal resistance of the battery.
In the first case,
\(
2=\frac{\varepsilon}{2+r}
\)
In the second case,
\(
\begin{aligned}
&0.5=\frac{\varepsilon}{9+r}\\
&\text { Divide (i) by (ii), we get }\\
&\begin{aligned}
& \frac{2}{0.5}=\frac{9+r}{2+r} \Rightarrow 4+2 r=4.5+0.5 r \\
& 1.5 r=0.5 \Rightarrow r=\frac{0.5}{1.5}=\frac{1}{3} \Omega
\end{aligned}
\end{aligned}
\) -
Question 83 of 176
83. Question
The rate of increase of thermo-emf with the temperature at the neutral temperature of a thermocouple: [NEET 2011]
CorrectIncorrectHint
(a) We have, \(\mathrm{e}=\mathrm{at}+\mathrm{bt}{ }^2\)
\(
\Rightarrow \frac{\mathrm{de}}{\mathrm{dt}}=\mathrm{a}+2 \mathrm{bt}
\)
At neutral temperature,
\(
\begin{aligned}
& \mathrm{t}=-\frac{\mathrm{a}}{2 \mathrm{~b}} \\
& \therefore \frac{\mathrm{de}}{\mathrm{dt}}=0
\end{aligned}
\) -
Question 84 of 176
84. Question
In the circuit shown in the figure below, if the potential at point \(A\) is taken to be zero, the potential at point \(B\) will be: [AIPMT 2011]
 CorrectIncorrect
CorrectIncorrectHint
\(
\begin{aligned}
&\text { (d) Current from } \mathrm{D} \text { to } \mathrm{C}=1 \mathrm{~A}\\
&\begin{aligned}
& \therefore V_D-V_C=2 \times 1=2 V \\
& \\
& V_A=0 \quad \therefore V_C=1 V, \therefore V_D-V_C=2 \\
& \therefore V_D-1=2 \quad \therefore V_D=3 V \\
&
\end{aligned}
\end{aligned}
\)
\(
\therefore V_D-V_B=2 \quad \therefore 3-V_B=2 \therefore V_B=1 V
\) -
Question 85 of 176
85. Question
A thermocouple of negligible resistance produces an e.m.f. of \(40 \mu \mathrm{~V} /{ }^{\circ} \mathrm{C}\) in the linear range of temperature. A galvanometer of resistance 10 ohm whose sensitivity is 1 \(\mu \mathrm{A} /\) division, is employed with the thermocouple. The smallest value of temperature difference that can be detected by the system will be: [AIPMT 2011]
CorrectIncorrectHint
(a)
\(
\text { For minimum deflection of } 1 \text { division required current }=1 \mu \mathrm{~A}
\)
\(
\begin{aligned}
& \Rightarrow \text { Voltage required }=\mathrm{IR}=(1 \mu \mathrm{~A})(10)=10 \mu \mathrm{~V} \\
& \therefore 40 \mu \mathrm{~V} \equiv 1^{\circ} \mathrm{C} \\
& \Rightarrow 10 \mu \mathrm{~V} \equiv \frac{1}{4}^{\circ} \mathrm{C}=0.25^{\circ} \mathrm{C}
\end{aligned}
\) -
Question 86 of 176
86. Question
A potentiometer circuit is set up as shown in the figure below. The potential gradient across the potentiometer wire is k volt/ cm . Ammeter present in the circuit reads 1.0 A when the two-way key is switched off. The balance points, when the key between the terminals (i) 1 and 2 (ii) 1 and 3 , is plugged in, are found to be at lengths \(l_1 c m\) and \(l_2 \mathrm{~cm}\) respectively. The magnitudes of the resistors R and X in ohm, are then, respectively, equal to: [AIPMT 2010]
 CorrectIncorrect
CorrectIncorrectHint
(b) (i) When key between the terminals 1 and 2 is plugged in,
P.D. across \(R=I R=k l_1\)
\(
\Rightarrow R=k l_1 \text { as } I=1 A
\)
(ii) When key between terminals 1 and 3 is plugged in.
P.D. \(\operatorname{across}(X+R)=I(X+R)=k l_2\)
\(
\begin{array}{ll}
\Rightarrow & \mathrm{X}+\mathrm{R}=k l_2 \\
\therefore & X=k\left(l_2-l_1\right) \\
\therefore & R=k l_1 \text { and } X=k\left(l_2-l_1\right)
\end{array}
\) -
Question 87 of 176
87. Question
Consider the following two statements.
(A) Kirchhoff’s junction law follows from the conservation of charge.
(B) Kirchhoff’s loop law follows from the conservation of energy.
Which of the following is correct? [AIPMT 2010]CorrectIncorrectHint
(d) Kirchhoff’s junction law or Kirchhoff’s first law is based on the conservation of charge. Kirchhoff’s loop law or Kirchhoff’s second law is based on the conservation of energy. Hence both statements (A) and (B) are correct.
-
Question 88 of 176
88. Question
The thermo e.m.f E in volts of a certain thermocouple is found to vary with temperature difference \(\theta\) in \({ }^{\circ} \mathrm{C}\) between the two junctions according to the relation \(E=30 \theta-\frac{\theta^2}{15}\) The neutral temperature for the thermo-couple will be: [AIPMT 2010]
CorrectIncorrectHint
(b) \(E=30 \theta-\frac{\theta^2}{15}\)
For neutral temperature, \(\frac{d E}{d \theta}=0\)
\(
\begin{aligned}
& 0=30-\frac{2}{15} \theta \\
& \therefore \theta=15 \times 15 \\
& =225^{\circ} \mathrm{C}
\end{aligned}
\)
Hence, neutral temperature is \(225^{\circ} \mathrm{C}\). -
Question 89 of 176
89. Question
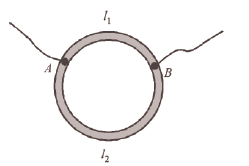
A wire of resistance \(12 \Omega \mathrm{~m}^{-1}\) is bent to form a complete circle of radius 10 cm . The resistance between its two diametrically opposite points, \(A\) and \(B\) as shown in the figure, is: [AIPMT 2009]
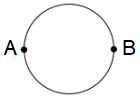 CorrectIncorrect
CorrectIncorrectHint
(d)
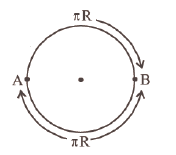
\(
\text { Length of wire }=2 \pi r=2 \pi(0.1)=0.2 \pi \mathrm{~m}
\)Total resistance of wire \(=12 \Omega \times 2 \pi \times 10^{-1}=2.4 \pi\)
Resistance of each half \(=\frac{2.4 \pi}{2}=1.2 \pi\)
and as about diameter both parts are in parallel
\(
R_{AB}=\frac{1.2 \pi}{2}=0.6 \pi \Omega
\) -
Question 90 of 176
90. Question
A student measures the terminal potential difference \(V\) of a cell (of emf \(E\) and internal resistance \(R\) ) as a function of the current \(I\) flowing through it. The slope and intercept of the graph between \(V\) and \(I\), respectively, is equal to: [AIPMT 2009]
CorrectIncorrectHint
(b) The terminal potential difference of a cell is given by \(\mathrm{V}+\mathrm{Ir}=\varepsilon\)
\(
\begin{aligned}
& \mathrm{V}=\mathrm{V}_{\mathrm{A}}-\mathrm{V}_{\mathrm{B}} \\
& \Rightarrow \mathrm{~V}=\varepsilon-\mathrm{Ir}
\end{aligned}
\)
\(\Rightarrow \frac{d V}{d I}=-r\), Also for, \(\mathrm{i}=0\) then \(\mathrm{V}=\varepsilon\)
\(\therefore\) slope \(=-r\), intercept \(=\varepsilon\) -
Question 91 of 176
91. Question
See the electrical circuit shown in this figure. Which of the following is a correct equation for it?

Which of the following equations is a correct equation for it? [AIPMT 2009]
CorrectIncorrectHint
(d)

Applying Kirchhoff’s rule in loop abefa \(\varepsilon_1-\left(\mathrm{i}_1+\mathrm{i}_2\right) \mathrm{R}-\mathrm{i}_1 \mathrm{r}_1=0\).
-
Question 92 of 176
92. Question
The mean free path of electrons in a metal is \(4 \times 10^{-8} \mathrm{~m}\). The electric field which can give on an average 2 eV energy to an electron in the metal will be in units \(\mathrm{V} / \mathrm{m}\) [AIPMT 2009]
CorrectIncorrectHint
\(
\begin{aligned}
&\text { (c) Energy }=2 \mathrm{eV}=e E \lambda\\
&\therefore E=\frac{2 e V}{e \lambda}=\frac{2}{4 \times 10^{-8}}=5 \times 10^7 \mathrm{~V} / \mathrm{m}
\end{aligned}
\) -
Question 93 of 176
93. Question
A current of 3 A flows through the \(2 \Omega\) resistor shown in the circuit. The power dissipated in the \(5 \Omega\) resistor is: [AIPMT 2008]
 CorrectIncorrect
CorrectIncorrectHint
(d)

Clearly, \(2 \Omega, 4 \Omega\) and \((1+5) \Omega\) resistors are in parallel. Hence, potential difference is same across each of them.
\(
\therefore I_1 \times 2=I_2 \times 4=I_3 \times 6
\)
Given \(\mathrm{I}_1=3 \mathrm{~A} \quad \therefore \mathrm{I}_1 \times 2=\mathrm{I}_3 \times 6\)
Given \(I_1=3 \mathrm{~A}\).
\(
\begin{aligned}
& \therefore I_1 \times 2=I_3 \times 6 \text { provides } \\
& I_3=\frac{I_1 \times 2}{6}=\frac{3 \times 2}{6}=1 \mathrm{~A}
\end{aligned}
\)
Total P.D. \(=5 \times 1+1 \times 1=6 \mathrm{~V}\)
Now, the potential across the \(5 \Omega\) resistor is 5V.
\(\therefore\) the power dissipated in the \(5 \Omega\) resistor
\(
\mathrm{P}=\frac{\mathrm{V}^2}{\mathrm{R}}=\frac{5^2}{5}=5 \mathrm{watt} .
\) -
Question 94 of 176
94. Question
A wire of a certain material is stretched slowly by ten percent, it’s new resistance and specific resistance become, respectively: [AIPMT 2008]
CorrectIncorrectHint
(b)
\(
\frac{\Delta l}{l}=0.1 \therefore l=1.1
\)
but the area also decreases by 0.1.
\(
\text { mass }=\rho l A=V \rho, \ln l+\ln A=\ln \text { mass. }
\)
\(
\therefore \frac{\Delta l}{l}+\frac{\Delta A}{A}=0 \Rightarrow \frac{\Delta l}{l}=\frac{-\Delta A}{A}
\)
Length increases by 0.1 , resistance increases, area decreases by 0.1 , then also resistance will increase. Total increase in resistance is approximately 1.2 times, due to increase in length and decrease in area. But specific resistance does not change. -
Question 95 of 176
95. Question
An electric kettle takes 4 A current at 220 V . How much time will it take to boil 1 kg of water at a temperature of \(20^{\circ} \mathrm{C}\) ? The temperature of boiling water is \(100^{\circ} \mathrm{C}\). [AIPMT 2008]
CorrectIncorrectHint
(c) Power \(=220 \mathrm{~V} \times 4 \mathrm{~A}=880\) watts. \(=880 \mathrm{~J} / \mathrm{s}\). Heat needed to raise the temperature of 1 kg water through \(80^{\circ} \mathrm{C}\)
\(
\begin{aligned}
& =m s . \Delta T \times 4.2 \mathrm{~J} / \mathrm{cal} \\
& =1000 \mathrm{~g} \times 1 \mathrm{cal} / \mathrm{g} \times 80 \times 4.2 \mathrm{~J} / \mathrm{cal} .
\end{aligned}
\)
\(
\begin{aligned}
& \therefore \text { Time taken }=\frac{1000 \times 1 \times 80 \times 4.2}{880}=\frac{336 \times 10^3}{880} \\
& =382 \mathrm{~s}=6.3 \mathrm{~min} .
\end{aligned}
\) -
Question 96 of 176
96. Question
A cell can be balanced against 100 cm and 110 cm of potentiometer wire, respectively with and without being short-circuited through a resistance of \(10 \Omega\). Its internal resistance is: [AIPMT 2008]
CorrectIncorrectHint
(a) [In the question, the length \(110 \mathrm{~cm} \& 100\) cm are interchanged ] as \(\varepsilon>\frac{\varepsilon R}{R+r}\) Without being short circuited through \(R\), only the battery \(\varepsilon\) is balanced.
\(
\varepsilon=\frac{V}{L} \times l_1=\frac{V}{L} \times 110 \mathrm{~cm} \dots(i)
\)
\(
\begin{aligned}
&\text { When } R \text { is connected across } \varepsilon \text {, }\\
&R i=R \cdot\left(\frac{\varepsilon}{R+r}\right)=\frac{V}{L} \times I_2 \Rightarrow \frac{R \varepsilon}{R+r}=\frac{V}{L} \times 100 \dots(ii)
\end{aligned}
\)
\(
\begin{aligned}
&\text { Dividing eqn. (i) and (ii), } \frac{(R+r)}{R}=\frac{110}{100}\\
&\begin{aligned}
& \Rightarrow 1+\frac{r}{R}=\frac{110}{100} \Rightarrow \frac{r}{R}=\frac{110}{100}-\frac{100}{100} \\
& \Rightarrow r=R \cdot \frac{10}{100}=\frac{R}{10} . \quad \text { As } R=10 \Omega ; r=1 \Omega
\end{aligned}
\end{aligned}
\) -
Question 97 of 176
97. Question
In the circuit shown, the current through the \(4 \Omega\) resistors is 1 A when the points P and M are connected to a DC voltage source. The potential difference between the points \(M\) and \(N\) is: {AIPMT 2008]
 CorrectIncorrect
CorrectIncorrectHint
(d) As the P.D. between \(4 \Omega\) and \(3 \Omega\) (in parallel), are the same,
\(
4 \times 1 \mathrm{amp}=3 \times i_1 \Rightarrow i_1=\frac{4}{3} \mathrm{~A}
\)
Total resistance of \(4 \Omega\) and \(3 \Omega=12 / 7 \Omega\).
Current in \(M Q P(\) upper one \()=1+\frac{4}{3}=\frac{7}{3} \mathrm{~A}\)
\(
\therefore \text { P.D. }=\frac{12}{7} \times \frac{7}{3}=4 \mathrm{~V}
\)
Current in \(M N P=\frac{4}{1.25}=\frac{4 \times 4}{5}=\frac{16}{5} \mathrm{~A}\)
\(\therefore\) P.D. across \(1 \Omega=\frac{16}{5} \mathrm{~A} \times 1 \Omega=\frac{16}{5}\) volt
\(\Rightarrow\) PD. across \(1 \Omega=3.2\) volt. -
Question 98 of 176
98. Question
The total power dissipated in watts in the circuit shown below is: [AIPMT 2007]
 CorrectIncorrect
CorrectIncorrectHint
\(
\begin{aligned}
&\text { (c) Power dissipiated }=P\\
&=\frac{V^2}{R}=\frac{(18)^2}{6}=54 \mathrm{~W}
\end{aligned}
\) -
Question 99 of 176
99. Question
Three resistances \(P, Q\), and \(R\), each of \(2 \Omega\) and an unknown resistance \(S\) form the four arms of a Wheatstone bridge circuit. When the resistance of \(6 \Omega\) is connected in parallel to \(S\), the bridge gets balanced. What is the value of \(S\) ? [AIPMT 2007]
CorrectIncorrectHint
(b) A balanced wheatstone bridge simply requires
\(
\frac{P}{Q}=\frac{R}{S} \Rightarrow \frac{2}{2}=\frac{2}{S}
\)
Therefore, S should be \(2 \Omega\).
A resistance of \(6 \Omega\) is connected in parallel.
In parallel combination,
\(
\begin{aligned}
& \frac{1}{R}=\frac{1}{R_1}+\frac{1}{R_2} \\
& \frac{1}{2}=\frac{1}{6}+\frac{1}{S} \\
& \Rightarrow S=3 \Omega
\end{aligned}
\) -
Question 100 of 176
100. Question
In the circuit shown below, if a conducting wire is connected between points \(A\) and \(B\), the current in this wire will: (All resistances are given in ohms) [AIPMT 2006]
 CorrectIncorrect
CorrectIncorrectHint
(c) Current will flow from \(B\) to \(A\)
Potential drop over the resistance \(C A\) will be more due to higher value of resistance. So potential at \(A\) will be less as compared with at \(B\). Hence, current will flow from \(B\) to \(A\).
-
Question 101 of 176
101. Question
Two cells having the same emf, are connected in series through an external resistance \(R\). Cells have internal resistance \(r_1\) and \(r_2\) respectively. When the circuit is closed, the potential difference across the first cell is zero. The value of \(R\) is: [AIPMT 2006]
CorrectIncorrectHint
(a)

Current in the circuit
\(
\begin{aligned}
& =\frac{E+E}{r_1+r_2+R}=\frac{2 E}{r_1+r_2+R} \\
& \text { P.D. across first cell }=E-i r_1 \\
& =E-\frac{2 E \times r_1}{\left(r_1+r_2\right)+R} \\
& \text { Now, } E=\frac{2 E r_1}{\left(r_1+r_2\right)+R}=0 \\
& \Rightarrow E=\frac{2 E r_1}{r_1+r_2+R} \Rightarrow 2 r_1=r_1+r_2+R \\
& R=r_1-r_2
\end{aligned}
\) -
Question 102 of 176
102. Question
The power dissipated across the \(8 \Omega\) resistor in the circuit shown here is 2 W . The power dissipated in watts across the \(3 \Omega\) resistor is: [AIPMT 2006]
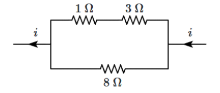 CorrectIncorrect
CorrectIncorrectHint
(d)
\(
\begin{aligned}
&\text { Power }=\mathrm{V} . I=I^2 \mathrm{R}\\
&\begin{aligned}
& i_2=\sqrt{\frac{\text { Power }}{R}}=\sqrt{\frac{2}{8}} \\
& =\sqrt{\frac{1}{4}}=\frac{1}{2} A
\end{aligned}
\end{aligned}
\)
Potential over \(8 \Omega=R i_2=8 \times \frac{1}{2}=4 V\)
This is the potential over parallel branch. So,
\(
i_1=\frac{4}{4}=1 A
\)
Power of \(3 \Omega=i_1{ }^2 R=1 \times 1 \times 3=3 W\) -
Question 103 of 176
103. Question
Kirchhoff’s first and second laws for electrical circuits are consequences of: [AIPMT 2006]
CorrectIncorrectHint
(a) Kirchhoff ‘ s first law deals with conservation of electrical charge and the second law deals with conservation of electrical energy.
-
Question 104 of 176
104. Question
For the network shown in the figure below, the value of the current \(i\) is: [AIPMT 2005]
 CorrectIncorrect
CorrectIncorrectHint
(d) It is a balanced Wheatstone bridge. Hence resistance \(4 \Omega\) can be eliminated.
\(
\begin{aligned}
& \therefore R_{\mathrm{eq}}=\frac{6 \times 9}{6+9}=\frac{18}{5} \\
& \therefore i=\frac{V}{R_{\mathrm{eq}}}=\frac{5 V}{18}
\end{aligned}
\) -
Question 105 of 176
105. Question
An 5 A fuse wire can withstand a maximum power of 1 W in a circuit. The resistance of the fuse wire is: [AIPMT 2005]
CorrectIncorrectHint
\(
\begin{aligned}
& \text { (b) } P=i^2 R \quad \text { or } \quad 1=25 \times R \\
& R=\frac{1}{25}=0.04 \Omega
\end{aligned}
\) -
Question 106 of 176
106. Question
When a wire of uniform cross-section a, length \(I\), and resistance \(R\) is bent into a complete circle, the resistance between any two diametrically opposite points will be: [AIPMT 2005]
CorrectIncorrectHint
(b)

\(
\mathrm{R}_{\mathrm{eq}}=\frac{R_1 R_2}{R_1+R_2}=\frac{\left(\frac{R}{2} \cdot \frac{R}{2}\right)}{\frac{R}{2}+\frac{R}{2}} \quad \therefore R_{\mathrm{eq}}=\frac{R}{4}
\) -
Question 107 of 176
107. Question
Two batteries, one of emf 18 volts and internal resistance \(2 \Omega\) and the other of emf 12 V and internal resistance \(1 \Omega\), are connected as shown. The voltmeter V will record a reading of: [AIPMT 2005]
 CorrectIncorrect
CorrectIncorrectHint
\(
\text { (c) } \mathrm{V}=\frac{\frac{E_1}{r_1}+\frac{E_2}{r_2}}{\frac{1}{r_1}+\frac{1}{r_2}}=\frac{\frac{18}{2}+\frac{12}{1}}{\frac{1}{2}+\frac{1}{1}}=14 \mathrm{~V}
\) (Since the cells are in parallel) -
Question 108 of 176
108. Question
The electric resistance of a certain wire of iron is \(R\). If its length and radius are both doubled, then [AIPMT 2004]
CorrectIncorrectHint
(c) \(R=\frac{\rho \ell_1}{A_1}\), now \(\ell_2=2 \ell_1\)
\(
\begin{aligned}
& A_2=\pi\left(r_2\right)^2=\pi\left(2 r_1\right)^2=4 \pi r_1^2=4 A_1 \\
& \therefore \quad R_2=\frac{\rho\left(2 \ell_1\right)}{4 A_1}=\frac{\rho \ell_1}{2 A_1}=\frac{R}{2}
\end{aligned}
\)
\(\therefore\) Resistance is halved, but specific resistance remains the same. -
Question 109 of 176
109. Question
A 6-volt battery is connected to the terminals of a three-metre-long wire of uniform thickness and resistance of 100 ohms. The difference of potential between two points on the wire separated by a distance of 50 cm will be: [AIPMT 2004]
CorrectIncorrectHint
(b) \(\mathrm{R} \propto \ell\)
For \(300 \mathrm{~cm}, \mathrm{R}=100 \Omega\)
For \(50 \mathrm{~cm}, R^{\prime}=\frac{100}{300} \times 50=\frac{50}{3} \Omega\)
\(
\begin{aligned}
& \therefore \mathrm{IR}=6 \\
& \Rightarrow I R^{\prime}=\frac{6}{R} \times R^{\prime}=\frac{6}{100} \times \frac{50}{3}=1 \mathrm{volt}
\end{aligned}
\) -
Question 110 of 176
110. Question
Five equal resistances each of resistance \(R\) are connected as shown in the figure below. A battery of \(V\) volts is connected between \(A\) and \(B\). The current flowing in \(A F C E B\) will be: [AIPMT 2004]
 CorrectIncorrect
CorrectIncorrectHint
(b) The given circuit can be redrawn as shown.

From circuit,
\(
\frac{F C}{C E}=\frac{F D}{D E}=1
\)
Thus, it is balanced Wheatstone’s bridge, so resistance in arm \(C D\) is indffective and so, current flows in arm.
Net resistance of the circuit is
\(
\begin{aligned}
& \frac{1}{R^{\prime}}=\frac{1}{(R+R)}+\frac{1}{(R+R)} \\
& =\frac{1}{2 R}+\frac{1}{2 R}=\frac{2}{2 R}=\frac{1}{R} \\
& R^{\prime}=R
\end{aligned}
\)
So, net current drawn from the battery
\(
i^{\prime}=\frac{V}{R^{\prime}}=\frac{V}{R}
\)
\(
\text { Current through } \mathrm{AFCEB}=\mathrm{V} / 2 \mathrm{R}
\) -
Question 111 of 176
111. Question
A battery is charged at a potential of 15 V for 8 hours when the current flowing is 10 A . The battery on discharge supplies a current of 5 A for 15 hours. The mean terminal voltage during discharges is 14 V . The “Watt hour” efficiency of the battery is: [AIPMT 2004]
CorrectIncorrectHint
\(
\begin{aligned}
&\text { (c) Efficiency is given by } \eta=\frac{\text { output }}{\text { input }}\\
&=\frac{5 \times 15 \times 14}{10 \times 8 \times 15}=0.875 \text { or } 87.5 \%
\end{aligned}
\) -
Question 112 of 176
112. Question
In India electricity is supplied for domestic use at 220 V . It is supplied at 110 V in the USA. If the resistance of a 60 W bulb for use in India is \(R\), the resistance of a 60 W bulb for use in the USA will be: [AIPMT 2004]
CorrectIncorrectHint
(c)
\(
\begin{aligned}
& P=\frac{V^2}{R} \\
& \Rightarrow R=\frac{V^2}{P}=\frac{(220)^2}{60}=\frac{4 \times(110)^2}{60} \\
& R^{\prime}=\frac{(110)^2}{60}=\frac{R}{4}
\end{aligned}
\) -
Question 113 of 176
113. Question
Resistances \(n\), each of \(r\) ohm, when connected in parallel give an equivalent resistance of \(R\) ohm. If these resistances were connected in series, the combination would have resistance in ohms, equal to: [AIPMT 2004]
CorrectIncorrectHint
(d) When \(n\) resistance of \(r\) ohm connected in parallel then their equivalent resistance is
\(
\begin{aligned}
& \Rightarrow \frac{1}{R}=\frac{1}{r}+\frac{1}{r}+\frac{1}{r}+\ldots \ldots . . . . . n \text { times } \\
& \therefore \quad \frac{1}{R}=\frac{n}{r} \Rightarrow R=\frac{r}{n} \Rightarrow r=n R
\end{aligned}
\)
When these resistance connected in series
\(
\begin{aligned}
R_s & =r+r+\ldots \ldots \ldots . . . . . n \text { times } \\
& =n r=n \times n R=n^2 R
\end{aligned}
\) -
Question 114 of 176
114. Question
When three identical bulbs of 60 watts and 200 -volt rating are connected in series to a 200 -volt supply, the power drawn by them will be: [AIPMT 2004]
CorrectIncorrectHint
(c) The resistance of each bulb
\(
=\frac{V^2}{P}=\frac{(200)^2}{60} \Omega
\)
When three bulbs are connected in series their resultant resistance
\(
=\frac{3 \times(200)^2}{60}
\)
Thus power drawn by bulb when connected across 200 V supply
\(
P=\frac{V^2}{R_{r e}}=\frac{(200)^2}{3 \times(200)^2 / 60}=20 \mathrm{~W}
\) -
Question 115 of 176
115. Question
A fuse wire is a wire of: [AIPMT 2003]
CorrectIncorrectHint
(b) Fuse wire should have high resistance and low melting point.
-
Question 116 of 176
116. Question
In a Wheatstone bridge, all four arms have equal resistance \(R\). If the resistance of the galvanometer arm is also \(R\), the equivalent resistance of the combination is: [AIPMT 2003]
CorrectIncorrectHint
(c) In balance Wheatstone bridge, the galvanometer arm can be neglected so equivalent resistance \(={R}\).
-
Question 117 of 176
117. Question
An electric kettle has two heating coils. When one of the coils is connected to an a.c. source, the water in the kettle boils in 10 minutes. When the other coil is used the water boils in 40 minutes. If both the coils are connected in parallel, the time taken by the same quantity of water to boil will be: [AIPMT 2003]
CorrectIncorrectHint
(a) Let \(R_1\) and \(R_2\) be the resistance of the two coils and \(V\) be the voltage supplied.
Effective resistance of two coils in parallel \(=\frac{R_1 R_2}{R_1+R_2}\)
Let \(H\) be the heat required to begin boiling in kettle.
Then \(H=\) Power \(\times\) time \(=\frac{V^2 t_1}{R_1}=\frac{V^2 t_2}{R_2}\)
For parallel combination, \(H=\frac{V^2\left(R_1+R_2\right) t_p}{R_1 R_2}\)
\(
\begin{aligned}
& \Rightarrow \frac{1}{t_p}=\left(\frac{t_2+t_1}{t_2 t_1}\right) \\
& \therefore t_p=\frac{t_1 t_2}{t_1+t_2}=\frac{10 \times 40}{10+40}=8 \text { minute. }
\end{aligned}
\) -
Question 118 of 176
118. Question
Two 220 -volts, 100 -watts bulbs are connected first in series and then in parallel. Each time the combination is connected to a 220 -volts AC supply line. The power drawn by the combination in each case respectively will be: [AIPMT 2003]
CorrectIncorrectHint
(d) \(
R=\frac{V^2}{P}=\frac{220 \times 220}{100}=484 \Omega
\)
In series, \(\mathrm{R}_{\text {eq }}=484+484=968 \Omega\)
\(
\therefore P_{e q}=\frac{V^2}{968}=\frac{220 \times 220}{968}=50 \mathrm{watt}
\)
In parallel, \(R_{e q}=242 \Omega\)
\(
\therefore P_{e q}=\frac{V^2}{242}=\frac{220 \times 220}{242}=200 \mathrm{watt}
\) -
Question 119 of 176
119. Question
For a cell terminal potential difference is 2.2 V when circuit is open and reduces to 1.8 V when cell is connected to a resistance of \(R=5 \Omega\). Determine internal resistance of cell \((r)\) [AIPMT 2002]
CorrectIncorrectHint

\(
\begin{aligned}
& \text { (a) } \quad E=V+i r \\
& 2.2=1.8+\frac{1.8}{5} \times r \\
& \Rightarrow \quad r=\frac{10}{9} \Omega
\end{aligned}
\) -
Question 120 of 176
120. Question
Specific resistance of a conductor increases with [AIPMT 2002]
CorrectIncorrectHint
(a) Resistance of a conductor is given by \({R}=\rho \frac{l}{A}\), where \(\rho\) is the specific resistance, \(l\) is the length and A is the cross-sectional area of the conductor. Now, when \(l=1\) and \(\mathrm{A}=1, {R}=\rho\).
So specific resistance or resistivity of a material may be defined as the resistance of a specimen of the material having unit length and unit cross-section. Hence, specific resistance is a property of a material and it will increase with the increase of temperature, but will not vary with the dimensions (length, crosssection) of the conductor.
-
Question 121 of 176
121. Question
If copper and silicon are cooled from 300 K to 60 K , then the specific resistance will: [AIPMT 2001]
CorrectIncorrectHint
(a) For metals specificresistance decrease with decrease in temperature whereas for semiconductors specific resistance increases with decrease in temperature.
-
Question 122 of 176
122. Question
The resistance of each arm of the wheat stone bridge is \(10 \Omega\). A resistance of \(10 \Omega\) is connected in series with a galvanometer. The equivalent resistance across the battery will be: [AIPMT 2001]
CorrectIncorrectHint
(a) Here, \(\mathrm{P}=\mathrm{Q}=\mathrm{S}=\mathrm{R}=10 \Omega\)
since \(\frac{P}{Q}=\frac{S}{R}=1\), so it is balanced Wheatstone bridge and no current pass through galvanometer.
\(\therefore\) Equivalent resistance is given by
\(
\begin{aligned}
& \mathrm{R}=\frac{(10+10)(10+10)}{(10+10)+(10+10)} \\
& =\frac{20 \times 20}{40}=10 \Omega
\end{aligned}
\) -
Question 123 of 176
123. Question
If the specific resistance of a potentiometer wire is \(10^{-7} \Omega \mathrm{~m}\) and the current flow through it is 0.1 A , the cross-sectional area of the wire is \(10^{-6} \mathrm{~m}^2\), then potential gradient will be: [AIPMT 2001]
CorrectIncorrectHint
(a)
\(
\begin{aligned}
\frac{V}{l} & =\frac{I R}{l}=\frac{I \rho l}{A l}=\frac{0.1 \times 10^{-7}}{10^{-6}} \\
& =0.01=10^{-2} \mathrm{~V} / \mathrm{m} .
\end{aligned}
\) -
Question 124 of 176
124. Question
A wire has a resistance of \(3.1 \Omega\) at \(30^{\circ} \mathrm{C}\) and a resistance \(4.5 \Omega\) at \(100^{\circ} \mathrm{C}\). The temperature coefficient of resistance of the wire [AIPMT 2001]
CorrectIncorrectHint
\(
\begin{aligned}
& \text { (a) } R_1=3.1 \Omega \text { at } t=30^{\circ} \mathrm{C} \\
& R_2=4.5 \Omega \text { at } t=100^{\circ} \mathrm{C} \\
& \text { We have, } R=R_0(1+\alpha t) \\
& \therefore R_1=R_0[1+\alpha(30)] \\
& R_2=R_0[1+\alpha(100)]
\end{aligned}
\)
\(
\begin{aligned}
& \Rightarrow \frac{R_1}{R_2}=\frac{1+30 \alpha}{1+100 \alpha} \\
& \Rightarrow \frac{3.1}{4.5}=\frac{1+30 \alpha}{1+100 \alpha} \Rightarrow \alpha=0.008^{\circ} \mathrm{C}^{-1}
\end{aligned}
\) -
Question 125 of 176
125. Question
If \(25 \mathrm{~W}, 220 \mathrm{~V}\) and \(100 \mathrm{~W}, 220 \mathrm{~V}\) bulbs are connected in series across a 440 V line, then [AIPMT 2001]
CorrectIncorrectHint
(a) As for an electric appliance \(R=\left(V_s^2 / W\right)\), so for same specified voltage \(V_s\)
\(
\frac{R_{25}}{R_{100}}-\frac{100}{25}-4
\)
i.e, \(R_{25}=4 R\) with \(R_{100}=R\)
Now in series potential divides in proportion to resistance.
So, \(V_1=\frac{R_1}{\left(R_1+R_2\right)} V\)
i.e., \(V_{25}=\frac{4}{5} \times 440=352 \mathrm{~V}\)
and \(V_2=\frac{R_2}{\left(R_1+R_2\right)} V\)
i.e., \(V_{100}=\frac{1}{5} \times 440=88 \mathrm{~V}\)
From this, it is clear that voltage across 100 W bulb ( \(=88 \mathrm{~V}\) ) is lesser than specified \((220 \mathrm{~V})\) while across 25 W bulb \((=352 \mathrm{~V})\) is greater than specified ( 220 V ), so, 25 W bulb will fuse. -
Question 126 of 176
126. Question
A battery of 10 V and internal resistance \(0.5 \Omega\) is connected across a variable resistance \(R\). The value of R for which the power delivered is maximum is equal to [AIPMT 2001, 1992]
CorrectIncorrectHint
\(
\text { (b) Power is maximum when } r=R, R=r=0.5 \Omega \text {. }
\)Note: If a cell of internal resistance \(r\) and emf \(E\) is connected to an external resistance of radius \(R\), then current given by cell, \(I=\frac{E}{R+r}\)
Power dissipated in external resistance (load)
\(
\begin{aligned}
& P=V I=(I R) I=I^2 R \\
& \Rightarrow P=\left(\frac{E}{R+r}\right)^2 R
\end{aligned}
\)
when \(R=r\)
Power, \(P=\frac{E^2}{4 r}\) (Maximum) -
Question 127 of 176
127. Question
The potentiometer is best for measuring voltage, as: [AIPMT 2000]
CorrectIncorrectHint
(a) When we measure the emf of a cell by the potentiometer then no current draws in the circuit in zero-deflection condition i.e., cell is in open circuit. Thus, in this condition the actual value of a cell is found. In this way potentiometer is equivalent to an ideal voltmeter of infinite resistance.
Note: The emf by the potentiometer is measured from null method in which zero deflection position is found on the wire.
-
Question 128 of 176
128. Question
In electrolysis, the amount of mass deposited or liberated at an electrode is directly proportional to [AIPMT 2000]
CorrectIncorrectHint
(b) By Faraday’s Ist Law
Amount deposited \((\mathrm{m})=\mathrm{Zit}=\mathrm{Zq}\)
\(
m \propto q
\)
Amount deposited is directly proportional to charge. -
Question 129 of 176
129. Question
If there are two bulbs of \((40 \mathrm{~W}, 200 \mathrm{~V})\), and \((100 \mathrm{~W}, 200 \mathrm{~V})\), then the correct relation for their resistance is: [AIPMT 2000]
CorrectIncorrectHint
\(
\begin{aligned}
& \text { (b) } P=\frac{V^2}{R} \text { or, } R \propto \frac{1}{P} \\
& R_{40}>R_{100}
\end{aligned}
\) -
Question 130 of 176
130. Question
A car battery of emf 12 V and internal resistance \(5 \times 10^{-2} \Omega\) receives a current of 60 A from an external source. The terminal voltage of the battery is: [AIPMT 2000]
CorrectIncorrectHint
(c) \(\frac{V-E}{r}=I \Rightarrow \frac{V-12}{5 \times 10^{-2}}=60 \Rightarrow V=15 \mathrm{~V}\)
-
Question 131 of 176
131. Question
The net resistance of the circuit between \(A\) and \(B\) is: [AIPMT 2000]
 CorrectIncorrect
CorrectIncorrectHint
(b) It is a balanced wheatstone bridge \(\left(\because \frac{3}{4}=\frac{6}{8}\right)\), so the \(7 \Omega\) resistance is ineffective. Equivalent resistance of \(3 \Omega\) and \(4 \Omega=3+4\) \(=7 \Omega\) (series)
Equivalent resistance of \(6 \Omega\) and \(8 \Omega=6+8\) \(=14 \Omega\) (series)
Equivalent resistance of \(7 \Omega\) and \(14 \Omega\) (parallel)
\(
=\frac{7 \times 14}{7+14}=\frac{14}{3} \Omega
\) -
Question 132 of 176
132. Question
The terminal potential difference of a cell is greater than its emf when: [AIPMT 1999]
CorrectIncorrectHint
(c) A battery of higher emf is connected in its parallel.
The emf \((E)\) and the terminal voltage ( \(V\) ) can be related by,
\(
v=E-I r
\)
Where,
\(E\) is the emf of the cell
\(V\) is the terminal voltage
\(I\) is current
\(r\) is internal resistance of the cell. -
Question 133 of 176
133. Question
The value of \(R\) for which power in it is maximum is: [AIPMT 1999]
 CorrectIncorrect
CorrectIncorrectHint
(b) Maximum power transfer happens when source resistance is equal to load resistance (R=r).
-
Question 134 of 176
134. Question
If the power dissipated in \(5 \Omega\) is 20 W then the power dissipated in \(4 \Omega\) is: [AIPMT 1999]
 CorrectIncorrect
CorrectIncorrectHint
(a) \( V^2/R = 20\)
\( V = 10 \text { Volt} \)
Current through 4 Ohm = 10/10 = 1 A.
Power dissiplation across 4 ohm = \(I^2 \times R=1 \times 4=4 W\) -
Question 135 of 176
135. Question
The current in \(8 \Omega\) resistance is (in the figure below): [AIPMT 1999]
 CorrectIncorrect
CorrectIncorrectHint
(a) By mesh analysis
For mesh 1 let current be \(\mathrm{I}_1\)
For mesh 2 let current be \(\mathrm{I}_2\)
\(
\begin{aligned}
& 12 \mathrm{I}_1+8 \mathrm{I}_2=8 \\
& 8 \mathrm{I}_1+14 \mathrm{I}_2=6
\end{aligned}
\)
Current in \(8 \Omega=\mathrm{I}_1+\mathrm{I}_2=0.615+0.076=0.69 \mathrm{~A}\) -
Question 136 of 176
136. Question
The current (I) in the given circuit is [AIPMT 1999]
 CorrectIncorrect
CorrectIncorrectHint
(b) In circuit, \(R_B\) and \(R_C\) are in series, so, \(R_s=\) \(6+6=12 \Omega\). This \(12 \Omega\) resistance is in parallel with \(R_A=3 \Omega\),
So, equivalent resistance of circuit
\(
R=\frac{3 \times 12}{3+12}=\frac{36}{15}=\frac{12}{5} \Omega
\)
\(\therefore\) Current in circuit, \(I=\frac{V}{R}\)
\(
=\frac{4.8}{\left(\frac{12}{5}\right)}=\frac{4.8 \times 5}{12}=2 \mathrm{~A}
\) -
Question 137 of 176
137. Question
The resistance of a discharge tube is [AIPMT 1999]
CorrectIncorrectHint
(c) In discharge tube the current is due to flow of positive ions and electrons. Moreover, secondary emission of electrons is also possible. So V-I curve is non-linear; hence resistance is non-ohmic.
-
Question 138 of 176
138. Question
The internal resistance of a cell of e.m.f. 2 V is \(0.1 \Omega\). It is connected to a resistance of 3.9 \(\Omega\). The voltage across the cell will be [AIPMT 1999]
CorrectIncorrectHint
\(
\begin{aligned}
& \text { (a) } i=\frac{2}{4}=0.5 \text { Ampere } \\
& V=\varepsilon-i r \\
& V=2-0.5 \times 0.1=1.95 \text { Volt }
\end{aligned}
\) -
Question 139 of 176
139. Question
In a meter bridge, the balancing length from the left end (standard resistance of one ohm is in the right gap) is found to be 20 cm . The value of the unknown resistance is [AIPMT 1999]
CorrectIncorrectHint
(d) Metre bridge works on the principle of Wheatstone bridge.
\(
\begin{aligned}
& \therefore \quad \frac{P}{Q}=\frac{l}{100-l} \\
& \text { or, } P=\frac{l}{100-l} \times Q=\frac{20}{80} \times 1=0.25 \Omega .
\end{aligned}
\) -
Question 140 of 176
140. Question
A potentiometer consists of a wire of length 4 m and resistance \(10 \Omega\). It is connected to a cell of e.m.f. 2 V . The potential difference per unit length of the wire will be [AIPMT 1999]
CorrectIncorrectHint
(c) \(i=\frac{2}{10}=0.2 \mathrm{~A}, \frac{R}{l}=10 / 4\)
Potential difference per unit length
\(
=0.2 \times(10 / 4)=0.5 \mathrm{~V} / \mathrm{m}
\) -
Question 141 of 176
141. Question
In a hot wire ammeter due to the flowing of the current, the temperature of the wire is increased by \(5^{\circ} \mathrm{C}\). If the value of the current is doubled, then the increase in temperature will be: [AIPMT 1998]
CorrectIncorrectHint
\(
\begin{aligned}
& \text { (b) } H=I^2 R t=m s \Delta T \\
& \frac{I_1^2}{I_2^2}=\frac{\Delta T_1}{\Delta T_2} \text { or, } \Delta T_2=\frac{\Delta T_1 I_2^2}{I_1^2} \\
& \Delta T_2=5 \times \frac{\left(2 I_1\right)^2}{I_1^2}=20 ; \quad \Delta T_2=20^{\circ} \mathrm{C}
\end{aligned}
\) -
Question 142 of 176
142. Question
When three identical bulbs are connected in series, the consumed power is 10 W . If they are now connected in parallel then the consumed power will be: [AIPMT 1998]
CorrectIncorrectHint
(b) For series, \(R_{\mathrm{eq}}=3 r\)
Power \(=\frac{V^2}{3 r}=10 \Rightarrow \frac{V^2}{r}=30\).
For parallel \(R_{\text {eq }}=r / 3\)
power \(=\frac{V^2}{r / 3}=\frac{3 V^2}{r}=3 \times 30=90 \mathrm{watt}\). -
Question 143 of 176
143. Question
If nearly \(10^5\) coulombs liberate 1 gm -equivalent of aluminium, then the amount of aluminium (equivalent weight 9), deposited through electrolysis in 20 minutes by a current of 50 amp will be [AIPMT 1998]
CorrectIncorrectHint
(c) \(m=Zit\), \(\quad 9=Z \times 10^5, Z=9 \times 10^{-5}\)
Again,
\(
m=Z i t=9 \times 10^{-5} \times 50 \times 20 \times 60=5.4 \mathrm{gm}
\) -
Question 144 of 176
144. Question
There are three copper wires of length and cross sectional area \((L, A),\left(2 L, \frac{1}{2} A\right),\left(\frac{1}{2} L, 2 A\right)\). In which case is the resistance minimum? [AIPMT 1997]
CorrectIncorrectHint
\(
\begin{aligned}
& \text { (b) } R=\rho \frac{l}{A} \\
& R_1=\rho \frac{L}{A} \\
& R_2=\rho \frac{2 L}{A} \times 2 \\
& R_3=\rho \frac{L}{2 \times 2 A}=\frac{\rho L}{4 A} \\
& \Rightarrow R_3<R_1<R_2
\end{aligned}
\) -
Question 145 of 176
145. Question
The current in the following circuit is [AIPMT 1997]
 CorrectIncorrect
CorrectIncorrectHint
(a) Resistance of \(A C B, R^{\prime}=3 \Omega+3 \Omega=6 \Omega\).
For net resistance between \(A\) and \(B ; R_{AB}=6 \Omega\) and \(3 \Omega\) are in parallel.
\(
R_{A B}=\frac{3 \times 6}{3+6}=\frac{18}{9}=2 \Omega
\)
Current in circuit \((I)=\frac{E}{R_{A B}}=\frac{2}{2}=1 A\) -
Question 146 of 176
146. Question
Kirchhoff’s first law, i.e. \(\Sigma i=0\) at a junction, deals with the conservation of [AIPMT 1997, 1992]
CorrectIncorrectHint
(c) We know from the Kirchhoff ‘s first law that the algebraic sum of the current meeting at any junction in the circuit is zero (i.e. \(\Sigma i=0\) ) or the total charge remains constant. Therefore, Kirchhoff’s first law at a junction deals with the conservation of charge.
-
Question 147 of 176
147. Question
From the graph between current \((I)\) and voltage \((I)\) is shown below. Identify the portion corres-ponding to negative resistance [AIPMT 1997]
 CorrectIncorrect
CorrectIncorrectHint
(a) For the negative resistance, when we increase the voltage, the current will decrease. Therefore from the graph, we find that the current in \(C D\) is decreased when voltage is increased.
-
Question 148 of 176
148. Question
A \((100 \mathrm{~W}, 200 \mathrm{~V})\) bulb is connected to a 160 volts supply. The power consumption would be [AIPMT 1997]
CorrectIncorrectHint
(c) Power \(=100 \mathrm{~W}\), Voltage of bulb \(=200 \mathrm{~V}\) and supply voltage \(\left(V_s\right)=160 \mathrm{~V}\). Therefore resistance of bulb \((R)\)
\(
=\frac{V^2}{P}=\frac{(200)^2}{100}=400 \Omega
\)
and power consumption \((P)\)
\(
=\frac{V_s^2}{R}=\frac{(160)^2}{400}=64 \mathrm{~W}
\) -
Question 149 of 176
149. Question
One kilowatt hour is equal to [AIPMT 1997]
CorrectIncorrectHint
\(
\begin{aligned}
& \text { (c) } 1 \mathrm{kWh}=1000 \mathrm{~Wh} \\
& =(1000 \mathrm{~W}) \times(3600 \mathrm{~s})=36 \times 10^5 \mathrm{~J}
\end{aligned}
\) -
Question 150 of 176
150. Question
If two bulbs, whose resistances are in the ratio of \(1: 2\) are connected in series, the power dissipated in them has the ratio of [AIPMT 1997]
CorrectIncorrectHint
(d) Ratio of resistance \(R_1: R_2=1: 2\) or \(\frac{{R_1}}{R_2}=\frac{1}{2}\). In series combination, power dissipated \((P)=I^2 R\)
\(\Rightarrow P \propto R\). Therefore \(\frac{P_1}{P_2}=\frac{R_1}{R_3}=\frac{1}{2}\) or \(P_1: P_2=1: 2\) -
Question 151 of 176
151. Question
What will be the equivalent resistance of circuit shown in figure between two points A and D [AIPMT 1996]
 CorrectIncorrect
CorrectIncorrectHint
(a) Lower resistance on extreme left and upper resistance oñ extreme right are ineffective.
Equivalent Circuit is shown below: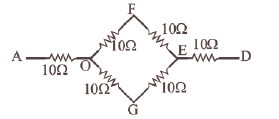
Equivalent Resistance of circuit,
\(
=10 \Omega+\frac{20 \times 20}{20+20}+10=10+10+10=30 \Omega
\) -
Question 152 of 176
152. Question
In the network shown in the figure, each of the resistance is equal to \(2 \Omega\). The resistance between the points \(A\) and \(B\) is [AIPMT 1995]
 CorrectIncorrect
CorrectIncorrectHint
(d) The equivalent circuits are as shown below Clearly, the circuit is a balanced Wheatstone bridge. So effective resistance between \(A\) and \(B\) is \(2 \Omega\).

-
Question 153 of 176
153. Question
Two wires of the same metal have same length, but their cross-sections are in the ratio \(3: 1\). They are joined in series. The resistance of thicker wire is \(10 \Omega\). The total resistance of the combination will be [AIPMT 1995]
CorrectIncorrectHint
(a) Ratio of cross-sectional areas of the wires \(=3: 1\) and resistance of thick wire \(\left(R_1\right)=10 \Omega\).
Resistance \((R)=\rho \frac{l}{A} \propto \frac{1}{A}\).
Therefore \(\frac{R_1}{R_2}=\frac{A_2}{A_1}=\frac{1}{3}\) or \(R_2=3 R_1=3 \times 10=30 \Omega\) and equivalent resistance of these two resistances in series combination:
\(
=R_1+R_2=30+10=40 \Omega
\) -
Question 154 of 176
154. Question
In good conductors of electricity, the type of bonding that exists is [AIPMT 1995]
CorrectIncorrectHint
(a) Presence of large number of free electrons in metallic bond solids, make then good conductor of electricity.
-
Question 155 of 176
155. Question
A heating coil is labelled \(100 \mathrm{~W}, 220 \mathrm{~V}\). The coil is cut in half and the two pieces are joined in paralle to the same source. The energy now liberated per second is [AIPMT 1995]
CorrectIncorrectHint
(b) Power of heating coil \(=100 \mathrm{~W}\) and voltage \((\mathrm{V})=220\) volts.
Let the resistance of heating coil is R.
\(
P=\frac{V^2}{R} \Rightarrow R=\frac{V^2}{P}=\frac{(220)^2}{100}
\)
Hence the resistance of each part is \(\mathrm{R} / 2\)
When the heating coil is cut into two equal parts and these parts are joined in parallel, the resistance of the coil is reduced to one-fourth of the previous value.
As they are join in parallel so equivalent resistance \(R_{e q}=\frac{\left(\frac{R}{2}\right)\left(\frac{R}{2}\right)}{\frac{R}{2}+\frac{R}{2}}=\frac{R}{4}\)
Energy liberated per second is the power dissipation.
\(
\text { Thus dissipated power }=\frac{\mathrm{V}^2}{\mathrm{R}_{\mathrm{eq}}}=\frac{4(220)^2}{\mathrm{R}}=\frac{4(220)^2 \times 100}{(220)^2}=400 J
\) -
Question 156 of 176
156. Question
A \(4\mu \mathrm{~F}\) capacitor is charged to 400 V . If its plates are joined through a resistance of \(2 \mathrm{k} \Omega\), then heat produced in the resistance is [AIPMT 1995]
CorrectIncorrectHint
(d) Capacitance \((C)=4 \mu \mathrm{~F}=4 \times 10^{-6} \mathrm{~F}\); Voltage \((V)=400\) volts and resistance \((R)=2 \mathrm{k} \Omega=2 \times 10^3 \Omega\). Heat produced \(=\) Electrical energy stored \(=\frac{1}{2} \mathrm{Cl}^2\) \(=\frac{1}{2} \times\left(4 \times 10^{-6}\right) \times(400)^2=0.32 \mathrm{~J}\).
-
Question 157 of 176
157. Question
A wire 50 cm long and \(1 \mathrm{~mm}^2\) in cross-section carries a current of 4 A when connected to a 2 V battery. The resistivity of the wire is [AIPMT 1994]
CorrectIncorrectHint
(b) Length \((l)=50 \mathrm{~cm}=0.5 \mathrm{~m}\);
Area \((A)=1 \mathrm{~mm}^2=1 \times 10^{-6} \mathrm{~m}^2\);
Current \((I)=4 \mathrm{~A}\) and voltage \((V)=2\) volts.
Resistance \((R)=\frac{V}{l}=\frac{2}{4}=0.5 \Omega\) and
\(
\begin{aligned}
\operatorname{Resistivity}(\rho) & =R \times \frac{A}{l}=0.5 \times \frac{1 \times 10^{-6}}{0.5} \\
& =1 \times 10^{-6} \Omega \mathrm{~m}
\end{aligned}
\) -
Question 158 of 176
158. Question
Six resistors of \(3 \Omega\) each are connected along the sides of a hexagon and three resistors of 6 \(\Omega\) each are connected along \(A C, A D\) and \(A E\) as shown in the figure. The equivalent resistance between \(A\) and \(B\) is equal to [AIPMT 1994]
 CorrectIncorrect
CorrectIncorrectHint
(a) Resistances \(R_{A F}\) and \(R_{F E}\) are in series combination. Therefore their equivalent resistance \(R^{\prime}=R_{A F}+R_{F E}=3+3=6 \Omega\).
Now the resistance \(R_{A E}\) and equivalent resistance \(R^{\prime}\) are in parallel combination.
Therefore relation for their equivalent resistance
\(
\begin{aligned}
& \frac{1}{R^{\prime \prime}}=\frac{1}{R^{\prime}}+\frac{1}{R_{A E}}=\frac{1}{6}+\frac{1}{6}=\frac{2}{6}=\frac{1}{3} \\
& \Rightarrow R^{\prime \prime}=3 \Omega
\end{aligned}
\)
We can calculate in the same manner for \(R_{E D}, R_{A C}, R_{D C}\) etc.
Therefore, the equivalent resistance between \(A\) and \(B\)
\(
=\frac{(3+3) \times 3}{(3+3)+3}=\frac{18}{9}=2 \Omega .
\) -
Question 159 of 176
159. Question
A flow of \(10^7\) electrons per second in a conducting wire constitutes a current of [AIPMT 1994]
CorrectIncorrectHint
\(
\begin{aligned}
&\text { (a) Flow of electrons, } \frac{n}{t}=10^7 / \mathrm{sec} \text {. }\\
&\begin{aligned}
& \text { Therefore, current }(I)=\frac{q}{t}=\frac{n e}{t}=\frac{n}{t} \times e \\
& =10^7 \times\left(1.6 \times 10^{-19}\right)=1.6 \times 10^{-12} \mathrm{~A}
\end{aligned}
\end{aligned}
\) -
Question 160 of 176
160. Question
Identify the set in which all the three materials are good conductors of electricity [AIPMT 1994]
CorrectIncorrectHint
Set (c) contains Cu (Copper), Ag (Silver), and Au (Gold). All of these materials are metals known for their excellent electrical conductivity, which makes this set the correct answer. Copper, silver, and gold are used in various electrical applications due to their ability to conduct electricity well.
-
Question 161 of 176
161. Question
An electric bulb is rated \(60 \mathrm{~W}, 220 \mathrm{~V}\). The resistance of its filament is [AIPMT 1994]
CorrectIncorrectHint
(d) Power \((P)=60 \mathrm{~W}\) and voltage \((V)=220\) volts. Resistance of the filament, \(R=\frac{V^2}{P}=\frac{(220)^2}{60}=807 \Omega\).
-
Question 162 of 176
162. Question
Three resistances each of \(4 \Omega\) are connected to form a triangle. The resistance between any two terminals is [AIPMT 1993]
CorrectIncorrectHint
(d) The two resistances are connected in series and the resultant is connected in parallel with the third resistance.
\(
\therefore R=4 \Omega+4 \Omega=8 \Omega
\)
and \(\frac{1}{R^{\prime \prime}}=\frac{1}{8}+\frac{1}{4}=\frac{3}{8}\)
or \(R^{\prime \prime}=\frac{8}{3} \Omega\) -
Question 163 of 176
163. Question
Current through \(3 \Omega\) resistor is 0.8 ampere, then potential drop through \(4 \Omega\) resistor is [AIPMT 1993]
 CorrectIncorrect
CorrectIncorrectHint
(c) Voltage across \(3 \Omega\) resistance \(=3 \times 0.8=\)
2.4 V This voltage is the same across \(6 \Omega\) resistance. Hence current through this resistance
\(
i=\frac{V}{R}=\frac{2.4}{6}=0.4 \mathrm{amp}
\)
Total current in the circuit
\(
=0.8+0.4=1.2 \mathrm{amp}
\)
Voltage across \(4 \Omega\) resistance
\(
=4 \times 1.2=4.8 \text { volts }
\) -
Question 164 of 176
164. Question
A battery of e.m.f 10 V and internal resistance \(0.5 \Omega\) is connected acrossia variable resistance \(R\). The value of \(R\) for which the power delivered in it is maximum is given by [AIPMT 1992]
CorrectIncorrectHint
(a) The output power of a cell iș given by
\(
P=\frac{V^2}{(r+R)^2} R
\)
Maximum power is delivered to the load only when the internal resistance ofthe source is equal to the load resistance \((R)\) : Then
\(
P_{\max }=\frac{V^2}{4 R}-\frac{V^2}{4 r} \quad(r=R)
\) -
Question 165 of 176
165. Question
The velocity of change carriers of current (about 1 ampere) tha metal under normal conditions is of the order of [AIPMT 1991]
CorrectIncorrectHint
(a) The velocity of charge carriers of current (about 1 ampere) in a metal under normal conditions is of the order of a fraction of \(\mathrm{mm} / \mathrm{sec}\).
-
Question 166 of 176
166. Question
In the network shown in figure each resistance is \(1 \Omega\). The effective resistance between \(A\) and \(B\) is [AIPMT 1990]
 CorrectIncorrect
CorrectIncorrectHint
(d) At \(A\) current is distributed and at \(B\) currents are collected. Between \(A\) and \(B\), the distribution is symmetrical. It has been shown in the figure. It appears that current in \(A O\) and \(O B\) remains same. At \(O\), current \(i_4\) returns back without any change. If we detach \(O\) from \(A B\) there will not be any change in distribution.
Now, \(C O \& O D\) will be in series hence its total resistance \(=2 \Omega\)
It is in parallel with \(C D\), so, equivalent resistance
\(
=\frac{2 \times 1}{2+1}=\frac{2}{3} \Omega
\)
This equivalent resistance is in series with \(A C\) \(\& D B\), so, total resistance
\(
=\frac{2}{3}+1+1=\frac{8}{3} \Omega
\)
Now \(\frac{8}{3} \Omega\) is parallel to \(A B\), that is, \(2 \Omega\), so total resistance
\(
=\frac{8 / 3 \times 2}{8 / 3+2}=\frac{16 / 3}{14 / 3}=\frac{16}{14}=\frac{8}{7} \Omega
\) -
Question 167 of 176
167. Question
You are given several identical resistances each of value \(R=10 \Omega\) and each eapoble of carrying a maximum currentefone ampere. It is required to make a suitablẽ combination of these resistances of \(5 \Omega\) which can carry a current of 4 ampere, The minimum number of resistances of the type \(R\) that will be required for this job is
CorrectIncorrectHint
(c) To carry a current of 4 ampere, we need four path, each earrying a current of one ampere. Let \(r\) be the resisfance of each path. These are connected in parallel. Hence their equivalent resistance will be \(r / 4\).
Aecording to the given problem \(\frac{r}{4}=5\) or \(r=20 \Omega\). For this purpose two resistances should be connected. There are four such combinations. Hence, the total number of resistance \(=4 \times 2=8\) -
Question 168 of 176
168. Question
Two identical batteries each of e.m.f 2 V and internal resistance \(1 \Omega\) are available to produce heat in an external resitance by passing a current through it. The maximum power that can be developed across \(R\) using these batteries is [AIPMT 1990]
CorrectIncorrectHint
(b) For maximum current, the two batteries should be connected in series, The current will be maximum when external resistance is equal to the total internal resistance of cells i.c. \(2 \Omega\). Hence power developed across the resistance \(R\) will be
\(
I^2 R=\left(\frac{2 E}{R+2 r}\right)^2 R=\left(\frac{2 \times 2}{2+2}\right)^2 \times 2=2 \mathrm{~W}
\) -
Question 169 of 176
169. Question
A current of 2 A , passing through a conductor produces 80 J of heat in 10 seconds. The resistance of the conductor in ohm is [AIPMT 1989]
CorrectIncorrectHint
(b) \(\mathrm{H}=I^2 R t \text { or } \quad R=\frac{H}{\left(I^2 t\right)}=\frac{80}{\left(2^2 \times 10\right)}=2 \Omega\)
-
Question 170 of 176
170. Question
40 electric bulbs are connected in series across a 220 V supply. After one bulb is fused the remaining 39 are connected again in series across the same supply. The illumination will be [AIPMT 1989]
CorrectIncorrectHint
(b) Since, the voltage is same for the two combinations, the resistance is less for 39 bulbs. Hence the combination of 39 bulbs will glow more as current is more.
Note: Since, the voltage is same for the two combinations, therefore \(H \propto \frac{1}{R}\). Hence, the combination of 39 bulbs will glow more.
-
Question 171 of 176
171. Question
\(n\) equal resistors are first connected in series and then connected in parallel. What is the ratio of the maximum to the minimum resistance ? [AIPMT 1989]
CorrectIncorrectHint
(c) In series \(R_s=n R\)
In parallel \(\frac{1}{R_p}=\frac{1}{R}+\frac{1}{R}+\ldots . n\) terms \(=\frac{n}{R}\)
\(
\Rightarrow \quad R_p=\frac{R}{n} \quad \therefore R_3 / R_p=n^2
\) -
Question 172 of 176
172. Question
Two batteries of emf 4 V and 8 V with internal resistance \(1 \Omega\) and \(2 \Omega\) are connected in a circuit with a resistance of \(9 \Omega\) as shown in figure. The current and potential difference between the points \(P\) and \(Q\) are [AIPMT 1988]
 CorrectIncorrect
CorrectIncorrectHint
\(
\begin{aligned}
& \text { (a) } \quad I=\frac{8-4}{1+2+9}=\frac{4}{12}=\frac{1}{3} \mathrm{~A} \\
& V_P-V_Q=4-\frac{1}{3} \times 3=3 \mathrm{volt}
\end{aligned}
\) -
Question 173 of 176
173. Question
The masses of the wires of copper is in the ratio of \(1: 3: 5\) and their lengths are in the ratio of \(5: 3: 1\). The ratio of their electrical resistance is [AIPMT 1988]
CorrectIncorrectHint
(d)
\(
m=l \times \text { area } \times \text { density }
\)
\(
\begin{aligned}
& \text { Area } \propto \frac{m}{l} \\
& \qquad R \propto \frac{l}{\text { Area }} \propto \frac{l^2}{m}
\end{aligned}
\)
\(
\begin{aligned}
& R_1: R_2: R_3=\frac{l_1^2}{m_1}: \frac{l_2^2}{m_2}: \frac{l_2^2}{m_3} \\
& R_1: R_2: R_3=\frac{25}{1}: \frac{9}{3}: \frac{1}{5}=125: 15: 1
\end{aligned}
\) -
Question 174 of 176
174. Question
The current passing through the battery in the given circuit, is: [NEET 2025]
 CorrectIncorrect
CorrectIncorrectHint
(c) ACDF forms a balanced wheastone bridge. So the current through 6 ohm is 0 and can be removed.
\(\therefore\) its equivalent \(R^{\prime}=\frac{4 \times 8}{12}=\frac{8}{3} \Omega\)
The circuit can be redrawn as
\(
\begin{aligned}
& \mathrm{R}_{\mathrm{eq}}=\frac{8}{3}+\frac{1}{3}+1.5+5.5 \\
& =10 \Omega \\
& i=\frac{V}{\mathrm{R}_{\mathrm{eq}}}=\frac{5}{10}=0.5 \mathrm{~A}
\end{aligned}
\) -
Question 175 of 176
175. Question
A constant voltage of 50 V is maintained between the points \(A\) and \(B\) of the circuit shown in the figure. The current through the branch \(C D\) of the circuit is: [NEET 2025]
 CorrectIncorrect
CorrectIncorrectHint
(c)

\(
\begin{aligned}
& R_{A B}=(1 \Omega / 3 \Omega) \text { in series with }(2 \Omega / 4 \Omega) \\
& =\frac{3 \times 1}{3+1}+\frac{2 \times 4}{2+4} \\
& =\frac{3}{4}+\frac{8}{6}=\frac{9+16}{12}=\frac{25}{12} \Omega
\end{aligned}
\)
Now total current through cell
\(
\begin{aligned}
& I=\frac{50}{25 / 12}=24 \mathrm{~A} \\
& I_{1 \Omega}=\frac{3}{4} \times 24=18 \mathrm{~A}, I_{3 \Omega}=\frac{1}{4} \times 24=6 \mathrm{~A} \\
& I_{2 \Omega}=\frac{4}{6} \times 24=16 \mathrm{~A}, I_{4 \Omega}=\frac{2}{6} \times 24=8 \mathrm{~A}
\end{aligned}
\)
Using junction rule at \(C, I_{C D}=18-16=2 \mathrm{~A}\) (From \(C\) to \(D\) ) -
Question 176 of 176
176. Question
A wire of resistance \(R\) is cut into 8 equal pieces. From these pieces, two equivalent resistances are made by adding four of these together in parallel. Then these two sets are added in series. The net effective resistance of the combination is: [NEET 2025]
CorrectIncorrectHint
(d) After being cut into 8 equal pieces, \(\Rightarrow\) Resistance of each piece \(=R^{\prime}=\frac{R}{8}\)
Each set has 4 pieces in parallel combination
\(\Rightarrow\) Resistance of each set \(=R^{\prime \prime}=\frac{R^{\prime}}{4}=\frac{R}{32}\)
Both sets are connected in series
\(
\therefore \quad R_{\text {eq }}=R^{\prime \prime}+R^{\prime \prime}=2 \times \frac{R}{32}=\frac{R}{16}
\)