NEET Practice Q & A
Quiz Summary
0 of 115 Questions completed
Questions:
Information
You have already completed the quiz before. Hence you can not start it again.
Quiz is loading…
You must sign in or sign up to start the quiz.
You must first complete the following:
Results
Results
0 of 115 Questions answered correctly
Your time:
Time has elapsed
You have reached 0 of 0 point(s), (0)
Earned Point(s): 0 of 0, (0)
0 Essay(s) Pending (Possible Point(s): 0)
Categories
- Not categorized 0%
- 1
- 2
- 3
- 4
- 5
- 6
- 7
- 8
- 9
- 10
- 11
- 12
- 13
- 14
- 15
- 16
- 17
- 18
- 19
- 20
- 21
- 22
- 23
- 24
- 25
- 26
- 27
- 28
- 29
- 30
- 31
- 32
- 33
- 34
- 35
- 36
- 37
- 38
- 39
- 40
- 41
- 42
- 43
- 44
- 45
- 46
- 47
- 48
- 49
- 50
- 51
- 52
- 53
- 54
- 55
- 56
- 57
- 58
- 59
- 60
- 61
- 62
- 63
- 64
- 65
- 66
- 67
- 68
- 69
- 70
- 71
- 72
- 73
- 74
- 75
- 76
- 77
- 78
- 79
- 80
- 81
- 82
- 83
- 84
- 85
- 86
- 87
- 88
- 89
- 90
- 91
- 92
- 93
- 94
- 95
- 96
- 97
- 98
- 99
- 100
- 101
- 102
- 103
- 104
- 105
- 106
- 107
- 108
- 109
- 110
- 111
- 112
- 113
- 114
- 115
- Current
- Review
- Answered
- Correct
- Incorrect
-
Question 1 of 115
1. Question
Mobility of electrons in a semiconductor is defined as the ratio of their drift velocity to the applied electric field. If, for an \(n\)-type semiconductor the density of electrons is \(10^{18} \mathrm{~m}^{-3}\) and their mobility is \(1.5 \mathrm{~m}^2(\mathrm{~V} . \mathrm{S})\) then the resistivity of the semiconductor (since it is an \(n\)-type semiconductor) is close to :
CorrectIncorrectHint
(d) Concept:
Relation between Current Density and Electric Field:
Current Density:
The amount of electric current travelling per unit cross-section area is called as current density and expressed in amperes per square meter.
Formula for Current Density is given as,
\(
\mathrm{J}=\mathrm{I} / \mathrm{A}
\)
Where,
I = current flowing through the conductor in Amperes
\(A=\) cross sectional area in \(\mathrm{m}^2\).
Consider a conductor of length I and area of cross-section A. Let its two ends be raised to potentials \(\mathrm{V}_1[latex] and [latex]\mathrm{V}_2\left(\mathrm{~V}_1>\mathrm{V}_2\right)\).
The electric current density is given by the formula:
\(
j=\sigma E=n e v_d
\)
Calculation:
Given:
Density of electrons, \(\mathrm{n}_{\mathrm{e}}=10^{19} \mathrm{~m}^{-3}\)
Charge of electron, \(\mathrm{e}=1.6 \times 10^{-19} \mathrm{C}\)
Mobility of electron, \(\mu_e=1.6 \mathrm{~m}^2 /(\mathrm{V} . \mathrm{s})\)
The electric current density is given by the formula:
\(
\mathrm{j}=\sigma \mathrm{E}=\mathrm{nev}_{\mathrm{d}}
\)
For intrinsic semiconductors (no impurities), the number of electrons will be equal to the number of holes.
\(
\sigma=\mathrm{ne} \frac{\mathrm{v}_{\mathrm{d}}}{\mathrm{E}}=\mathrm{ne} \mu
\)
For N -type semiconductor, electrons are majority carriers.
Conductivity, \(\sigma \approx[latex] ne [latex]\mu\)
Resistivity, \(\rho=\frac{1}{\sigma}=\frac{1}{\mathrm{n}_{\mathrm{e}} \mathrm{e} \mu_{\mathrm{e}}} \dots(1)\)
By substituting the given values in the equation (1),
\(
\begin{aligned}
& \rho=\frac{1}{10^{19} \times 1.6 \times 10^{-19} \times 1.6} \\
& \rho=0.4 \Omega \mathrm{~m}
\end{aligned}
\) -
Question 2 of 115
2. Question
The temperature coefficient of resistance of a semiconductor is :
CorrectIncorrectHint
(c) The temperature coefficient of resistance is equal to the change in resistance of the wire of resistance one ohm at \(0^{\circ} \mathrm{C}\) when the temperature changes by \(1^0 \mathrm{C}\).
That is denoted by \(\alpha\).
\(
\alpha=\frac{R_t-R_o}{R_o t}
\)
Where,
\(\alpha=\) temperature coefficient
Let’s consider a case,
If \(R_o=1\) and \(\mathrm{t}=1^0 \mathrm{C}\) then, \(\alpha=R_t-R_o\)
In general, for two different temperature and resistance, we can write the equation as,
\(
\alpha=\frac{R_2-R_1}{R_1 t-R_2 t}
\)
From this equation, we can say that as the temperature of a semiconductor increases, resistance decreases.
Then the value \(R_2-R_1\) becomes a negative value. Therefore the entire value becomes negative in the case of the semiconductor.
Thus, the temperature coefficient of resistance \((\alpha)\) of a semiconductor is always negative. -
Question 3 of 115
3. Question
Energy bands in solid are consequence of :
CorrectIncorrectHint
(b) Energy bands in solids are a consequence of (b) Pauli exclusion principle.
Explanation: The Pauli exclusion principle states that no two electrons in an atom can have the same set of quantum numbers, which leads to the formation of distinct energy levels when atoms come together to form a solid.
Why other options are incorrect:
(a) Ohm’s law:Ohm’s law relates current, voltage, and resistance in a conductor. It doesn’t explain the formation of energy bands.
(c) Bohr’s theory:Bohr’s theory describes the quantized energy levels of electrons in individual atoms, but it doesn’t explain how these levels merge into bands when atoms form a solid.
(d) Heisenberg’s uncertainty principle:Heisenberg’s uncertainty principle deals with the limitations in simultaneously knowing the position and momentum of a particle. While it’s a fundamental principle in quantum mechanics, it doesn’t directly explain the formation of energy bands.
-
Question 4 of 115
4. Question
A P-N junction diode is connected with an ammeter \(A_1\) in series and a resistance \(R\) is connected with an ammeter \(A_2\) in series. The combination in parallel is connected to a \(d c\) battery as shown in figure. For a certain battery voltage, the readings of \(A_1\) and \(A_2\) are same. If the battery voltage is now increased, then [temperature of resistance remains constant]
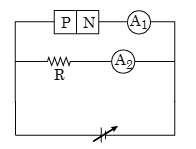 CorrectIncorrect
CorrectIncorrectHint
(b) Compare the change in current through the diode and the resistor when the voltage is increased.
Step 1: Initially, the readings of A1 and A2 are the same, meaning the current through the diode ( \(I_D\) ) and the current through the resistor ( \(I_R\) ) are equal:
\(I_D=I_R\)Step 2: The current through the diode increases exponentially with voltage:
\(I_D=I_0\left(e^{\frac{c V}{n k T}}-1\right)\)
Where \(I_0\) is the reverse saturation current, \(\boldsymbol{e}\) is the electron charge, \(V\) is the voltage, \(n\) is the ideality factor, \(k\) is Boltzmann’s constant, and \(T\) is the temperature.
When the voltage increases, the exponential term dominates, and the current through the diode increases significantly.Step3: The current through the resistor increases linearly with voltage according to Ohm’s law:
\(I_R=\frac{V}{R}\)
When the voltage increases, the current through the resistor also increases, but linearly.Step4: Therefore, the reading of A1 (diode current) will be greater than the reading of A2 (resistor current).
-
Question 5 of 115
5. Question
The depletion region of a P-N junction diode contains immobile ions. Which of the following figures correctly show the sign of those ions :
 CorrectIncorrect
CorrectIncorrectHint
(d) When an electron diffuses from \(n \rightarrow p\), it leaves behind an ionised donor on \(n\)-side. This ionised donor (positive charge) is immobile as it is bonded to the surrounding atoms. As the electrons continue to diffuse from \(n \rightarrow p\), a layer of positive charge (or positive space-charge region) on n -side of the junction is developed.
Similarly, when a hole diffuses from \(\mathrm{p} \rightarrow \mathrm{n}\) due to the concentration gradient, it leaves behind an ionised acceptor (negative charge) which is immobile. As the holes continue to diffuse, a layer of negative charge (or negative space-charge region) on the p-side of the junction is developed. This space-charge region on either side of the junction together is known as depletion region as the electrons and holes taking part in the initial movement across the junction depleted the region of its on diffusion free charges (Fig. D).
-
Question 6 of 115
6. Question
If a full wave rectifier circuit is operating at a source frequency of 50 Hz mains. The frequency in the ripple of waveform will be :
CorrectIncorrectHint
(c) The given frequency of the input \(=50 \mathrm{~Hz}\)
The fundamental frequency of the output ripples \(=2\) times the input frequency \(=2 \times 50=100 \mathrm{~Hz}\).
-
Question 7 of 115
7. Question
To obtain P-type silicon semiconductor we need to dope pure silicon with :
CorrectIncorrectHint
(a) To obtain a P-type silicon semiconductor, you need to dope pure silicon with a trivalent impurity like boron, aluminum, or gallium.
Explanation: When a trivalent impurity is added to silicon, it creates “holes” in the crystal lattice, allowing for positive charge carriers to move, which is characteristic of a P-type semiconductor.
-
Question 8 of 115
8. Question
For the circuit shown in figure below, the current through the zener diode is :
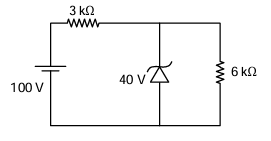 CorrectIncorrect
CorrectIncorrectHint
(c)
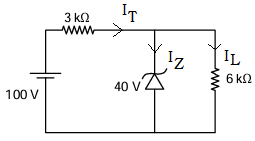
\(
\begin{aligned}
&\text { By Kirchhoff’s node law, }\\
&I_T=I_Z+I_L
\end{aligned}
\)
\(
I_T=\frac{(100-40)}{3K}=20 \mathrm{~mA}
\)
\(
I_L=\frac{40}{6K}=\frac{20}{3} \mathrm{~mA}
\)
\(
I_Z=20-\frac{20}{3}= \frac{40}{3} \mathrm{~mA}
\) -
Question 9 of 115
9. Question
The circuit shown below contain 4 ideal diodes, each with a forward resistance of \(50 \Omega\), if the battery voltage is 12 V , the current through the \(150 \Omega\) resistance is :
 CorrectIncorrect
CorrectIncorrectHint
(b) Diode D1 and D4 will be forward biased (D2 and D3 will be reverse biased) and replaced with 50 Ohm resistor each.
\(
\begin{aligned}
I & =\frac{V}{R_t} \\
I & =\frac{12 \mathrm{~V}}{300 \Omega} \\
I & =0.04 \mathrm{~A}
\end{aligned}
\) -
Question 10 of 115
10. Question
To get output ‘ 1 ‘ at \(R\), for the given logic gate circuit shown in figure below, choose the option which gives appropriate input values:
 CorrectIncorrect
CorrectIncorrectHint
(d) \(X=1, Y=0\)
-
Question 11 of 115
11. Question
The output of the given logic circuit shown in figure below is :
 CorrectIncorrect
CorrectIncorrectHint
(d)
\(
Y=A\bar{B}
\) -
Question 12 of 115
12. Question
The electrical conductivity of a semiconductor increases when electromagnetic radiation of wavelength shorter than 2480 nm is incident on it. The band gap in \((\mathrm{eV})\) for the semiconductor is \(n \times 10^{-2} \mathrm{eV}\) find \(n\).
CorrectIncorrectHint
(d)
Calculate the energy of the photon with the given wavelength and equate it to the band gap energy. Then, solve for \(n\).
Calculate the energy of the photon:
The energy of the photon is given by:
\(E=\frac{h c}{\lambda}\)Substituting the given values:
\(E=\frac{\left(6.626 \times 10^{-34} \mathrm{~J} \mathrm{~s}\right)\left(3 \times 10^8 \mathrm{~m} / \mathrm{s}\right)}{2480 \times 10^{-9} \mathrm{~m}}\)
\(E=\frac{19.878 \times 10^{-26}}{2480 \times 10^{-9}} \mathrm{~J}\)
\(E=8.015 \times 10^{-20} \mathrm{~J}\)Convert the energy to electron volts (eV)
Using the conversion factor \(1 \mathrm{eV}=1.602 \times 10^{-19} \mathrm{~J}\) :
\(E=\frac{8.015 \times 10^{-20} \mathrm{~J}}{1.602 \times 10^{-19} \mathrm{~J} / \mathrm{eV}}\)
\(E=0.500 \mathrm{eV}\)Equate the energy to the band gap and solve for \(\boldsymbol{n}\)
The band gap is given as \(n \times 10^{-2} \mathrm{eV}\) :
\(0.500 \mathrm{eV}=n \times 10^{-2} \mathrm{eV}\)
\(n=\frac{0.500}{10^{-2}}\)
\(n=50\) -
Question 13 of 115
13. Question
If the ratio of the concentration of electrons to that of holes in a semiconductor is \(7 / 5\), and the ratio of currents is \(7 / 4\), then. The ratio of their drift velocities is \(n / 4\) find \(n\) ?
CorrectIncorrectHint
(b) Step 1: Express the ratio of currents in terms of concentrations and drift velocities.
The electron current is \(I_e=n_e e A v_e\).
The hole current is \(I_h=n_h e A v_h\).
The ratio of currents is \(\frac{I_e}{I_h}=\frac{n_e e A v_e}{n_h e A v_h}\).
Simplify the ratio: \(\frac{I_e}{I_h}=\frac{n_e v_e}{n_h v_h}\).
Step 2: Solve for the ratio of drift velocities.
Rearrange the equation from Step 1: \(\frac{v_e}{v_h}=\frac{I_e}{I_h} \cdot \frac{n_h}{n_e}\).
Substitute the given ratios: \(\frac{v_e}{v_h}=\frac{7}{4} \cdot \frac{5}{7}\).
Simplify: \(\frac{v_e}{v_h}=\frac{5}{4}\).Step 3: Find \(n\).
We are given that \(\frac{v_e}{v_h}=\frac{n}{4}\).
From Step 2, we found that \(\frac{v_e}{v_h}=\frac{5}{4}\).
Equate the two expressions: \(\frac{n}{4}=\frac{5}{4}\).
Solve for \(n: n=5\).
Solution
The value of \(n\) is 5 . -
Question 14 of 115
14. Question
If voltage across a zener diode is 6 V, then find out the value of maximum resistance in this condition. [AIIMS 2019]
 CorrectIncorrect
CorrectIncorrectHint
(d) Given, voltage across Zener diode, \(V_Z=6 \mathrm{~V}\) Since, Zener diode provide stabilised supply of 6 V.

In this case, the value of \(R\) should be chosen such that negligible current flows through the Zener diode.
\(
\begin{array}{lrl}
\therefore & & I=I_Z+I_L=0+I_L \\
& & I=\frac{30}{R+10^3} \\
\text { But } & & I=6 \mathrm{~mA}=6 \times 10^{-3} \mathrm{~A} \\
\therefore & & 6 \times 10^{-3}
\end{array}=\frac{30}{R+10^3} \Rightarrow R=4 \times 10^3 \Omega=4 \mathrm{k} \Omega \text { llor }
\) -
Question 15 of 115
15. Question
Assertion: Photodiode and solar cell works on same mechanism.
Reason: Area is large for solar cell. [AIIMS 2019]CorrectIncorrectHint
(b) A p-n junction photodiode can be operated under photovoltaic conditions similar to that of a solar cell. The current-voltage characteristics of a photodiode and solar cell under illumination are also similar.
When light energy falls on the solar cell, then it converts solar energy into electrical energy.
The area of solar cell should be larger, so that it could absorbed more amount of sunlight and hence more amount of electrical energy is obtained.
Hence, Assertion and Reason both are correct but Reason is not the correct explanation of Assertion. -
Question 16 of 115
16. Question
Following circuit will act as [JIPMER 2019]
 CorrectIncorrect
CorrectIncorrectHint
\(
\begin{aligned}
&\text { (a) According to give logic diagram, }\\
&\begin{aligned}
& & Y^{\prime} & =\left(A^{\prime} B^{\prime}\right)^{\prime}=\left(A^{\prime}\right)^{\prime}+\left(B^{\prime}\right)^{\prime}=A+B \\
\therefore & & Y & =Y^{\prime}=(A+B)^{\prime}=\text { output of NOR gate }
\end{aligned}
\end{aligned}
\) -
Question 17 of 115
17. Question
The diode used at a constant potential drop of 0.5 V at all currents and maximum power rating of 100 mW. What resistance must be connected in series to diode, so that current in circuit is maximum? [AIIMS 2018]
 CorrectIncorrect
CorrectIncorrectHint
(c) Current passing in the circuit,
\(
I=\frac{P}{V}=\frac{100 \times 10^{-3}}{0.5}=0.2 \mathrm{~A}
\)
Value of connected series resistance,
\(
\begin{aligned}
R & =\frac{1.5-0.5}{0.2} \Rightarrow R=\frac{1}{0.2} \\
\therefore \quad R & =5 \Omega
\end{aligned}
\) -
Question 18 of 115
18. Question
A specimen of silicon is to be made \(p\)-type semiconductor, for this one atom of indium, on an average, is doped in \(5 \times 10^7\) silicon atoms. If the number density of silicon is \(5 \times 10^{28}\) atom \(/ \mathrm{m}^3\), then the number of acceptor atoms per \(\mathrm{cm}^3\) will be [AIIMS 2017]
CorrectIncorrectHint
(c) Given, one indium atom to be doped in \(5 \times 10^7\) silicon atoms.
Number density of silicon
\(
\begin{aligned}
& =5 \times 10^{28} \text { atom m } \mathrm{m}^{-3} \\
& \approx 5 \times 10^{22} \text { atom } \mathrm{cm}^{-3}
\end{aligned}
\)
Number of acceptor atom \(\mathrm{cm}^{-3}\)
\(
=\frac{5 \times 10^{22}}{5 \times 10^7}=1 \times 10^{15} \mathrm{~cm}^{-3}
\)
Hence, number of acceptor atom \(/ \mathrm{cm}^3\) is \(1 \times 10^{15} \mathrm{~cm}^{-3}\). -
Question 19 of 115
19. Question
A proper combination of 3 NOT and 1 NAND gates is shown in the figure. If \(A=0, B=1, C=1\), then the output of this combination is [AIIMS 2017]
 CorrectIncorrect
CorrectIncorrectHint
(a) So, output \(Y=\overline{\bar{A} \cdot \bar{B} \cdot \bar{C}}=\stackrel{=}{A}+\stackrel{=}{B}+\stackrel{=}{C}=A+B+C\)
If \(A=0, B=1, C=1\)
\(
\therefore \quad Y=0+1+1=0+1=1
\) -
Question 20 of 115
20. Question
The boolean expression \(P+\bar{P} Q\), where \(P\) and \(Q\) are the inputs of the logic circuit, represents [AIIMS 2015]
CorrectIncorrectHint
(d) If \(Y\) represents the given Boolean expression \(P+\bar{P} Q\), then
\(
\begin{array}{rlr}
Y & =P+\bar{P} Q=P(1)+\bar{P} Q & \\
& =P(1+Q)+\bar{P} Q & (\because 1=1+X) \\
& =P+P Q+\bar{P} Q & \\
& =P+Q(P+\bar{P}) & \\
& =P+Q & (\because \bar{X}+X=1) \\
& =\text { output of OR gate } &
\end{array}
\)
Hence, given expression represents OR gate. -
Question 21 of 115
21. Question
A semiconductor is having electron and hole mobilities \(\mu_n\) and \(\mu_p\), respectively.
If its intrinsic carrier density is \(n_i\), then what will be the value of hole concentration \(P\) for which the conductivity will be minimum at a given temperature? [AIIMS 2015]CorrectIncorrectHint
(a) The overall conductivity of a semiconductor,
\(
\begin{gathered}
\sigma=n_e e \mu_e+n_p e \mu_p \\
\sigma=e\left[\mu_e n_e+n_p \mu_p\right]
\end{gathered}
\)
Also, \(n_e n_p=n_i^2\) [for an intrinsic semiconductor]
\(
\Rightarrow \quad n_e=\frac{n_i^2}{n_p}
\)
Now,
\(
\sigma=e\left[\frac{n_i^2}{n_p} \mu_n+\mu_p n_p\right]
\)
On differentiating, \(\frac{d \sigma}{d n_p}=e\left[-\frac{n_i^2}{n_p^2} \mu_n+\mu_p\right]=0\)
\(
\Rightarrow \quad \mu_p=\frac{n_i^2}{n_p^2} \mu_n \Rightarrow n_p=n_i \sqrt{\frac{\mu_n}{\mu_p}}
\) -
Question 22 of 115
22. Question
Zener diode is used for [UK PMT 2015]
CorrectIncorrectHint
(d) The common application of Zener diode is stabilisation or voltage regulation.
-
Question 23 of 115
23. Question
The arrangement shown in figure performs the logic function of [UK PMT 2015]
 CorrectIncorrect
CorrectIncorrectHint
(b) Gate I \(\rightarrow\) NAND gate
Gate II \(\rightarrow\) AND gate
Above arrangement represents NAND gate. -
Question 24 of 115
24. Question
Which gate can be obtained by shorting both the input terminals of a NOR gate? [Guj. CET 2015]
CorrectIncorrectHint
(a) The output of NOR gate
\(
\overline{A+B}=Y
\)
When, \(A=B\), then \(\overline{A+A}=\bar{A}=\) NOT gate -
Question 25 of 115
25. Question
An LED is constructed from a \(p-n\) junction based on a certain semi-conducting material whose energy gap is 1.9 eV. Then, the wavelength of the emitted light is [KCET 2015]
CorrectIncorrectHint
(a) Given, energy gap, \(E_g=1.9 \mathrm{eV}=1.9 \times 1.6 \times 10^{-19} \mathrm{~V}\)
We know that, \(E_g=\frac{h c}{\lambda} \Rightarrow \lambda=\frac{h c}{E_g}\)
Wavelength of the emitted light,
\(
\begin{aligned}
& \lambda=\frac{6.63 \times 10^{-34} \times 3 \times 10^8}{1.9 \times 1.6 \times 10^{-19}} \\
& \lambda=6.5 \times 10^{-7} \mathrm{~m}
\end{aligned}
\) -
Question 26 of 115
26. Question
The equivalent circuit is

The circuit represents [Manipal 2015]
CorrectIncorrectHint
(d) Output of NOR gate, \(Y^{\prime}=\overline{A+B}\)
Output of NAND gate,
\(
\begin{aligned}
Y^{\prime \prime} & =\overline{(A+B) \cdot(A+B)}=\overline{\overline{(A+B)}} \cdot \overline{\overline{(A+B)}} \\
& =(A+B)(A+B)=A+B
\end{aligned}
\)
Output of NOT gate,
\(
Y^{\prime \prime \prime}=\overline{A+B}
\)
\(\therefore\) The boolean expression is for NOR gate. -
Question 27 of 115
27. Question
The inputs to the digital circuit are as shown below. The output \(Y\) is [WB JEE 2015]
 CorrectIncorrect
CorrectIncorrectHint
(c) The output of digital circuit can be given as \(Y=\overline{A B}+\bar{C}\).
It will be same as \(Y=\bar{A}+\bar{B}+\bar{C}\). -
Question 28 of 115
28. Question
The following logic gate circuit in equivalent to an [UP CPMT 2015]
 CorrectIncorrect
CorrectIncorrectHint
(c) The output of \(G_1=A+B\)
Output of \(G_2=\overline{A \cdot B}\)
and output of \(G_3=(A+B)(\overline{A \cdot B})=A \bar{B}+B \bar{A}\)
This output is same as the output of an XOR gate. -
Question 29 of 115
29. Question
Assume that, each diode as shown in the figure has a forward bias resistance of \(50 \Omega\) and an infinite reverse bias resistance. The current through the resistance \(150 \Omega\) is [WB JEE 2015]
 CorrectIncorrect
CorrectIncorrectHint
(b) As the lower diode attached to \(100 \Omega\) resistance is in reverse biased, so it is non-conducting (we can remove that).
\(
\therefore \text { Current through the circuit, } I=\frac{V}{R}=\frac{10}{200}=0.05 \mathrm{~A}
\) -
Question 30 of 115
30. Question
In a semiconducting material, the mobilities of electrons and holes are \(\mu_e[latex] and [latex]\mu_h\), respectively. Which of the following is true? [Manipal 2015]
CorrectIncorrectHint
(d) The mobility of electron is greater than holes because electron require less energy to move.
Explanation: Electrons are lighter and experience less resistance when moving through the crystal lattice compared to holes, which are essentially the absence of an electron and are more influenced by the positively charged nucleus.
-
Question 31 of 115
31. Question
If in a \(p-n\) junction, a square input signal of 10 V is applied as shown,

then the output across \(R_L\) will be [CG PMT 2015]
 CorrectIncorrect
CorrectIncorrectHint
(d) As the diode is forward biased, so it takes only positive value. Hence, option (d) is correct.
-
Question 32 of 115
32. Question
The circuit has two oppositely connected ideal diodes in parallel. What is the current flowing in the circuit? [KCET 2015]
 CorrectIncorrect
CorrectIncorrectHint
(b) In the given circuit, \(D_1\) is forward biased and \(D_2\) is reverse biased, so \(D_1\) works like a closed switch and \(D_2\) works like an open circuit, so circuit becomes
\(
\begin{aligned}
&\text { Now, current in the circuit, }\\
&\begin{array}{ll}
& I=\frac{E}{R_S}=\frac{12}{4+3} \\
\Rightarrow & I=\frac{12}{7} \\
\Rightarrow & I=1.71 \mathrm{~A}
\end{array}
\end{aligned}
\) -
Question 33 of 115
33. Question
The conductivity in the intrinsic semiconductor does not depend on [CG PMT 2015]
CorrectIncorrectHint
(c) The conductivity of the intrinsic semicondutor is independent of fermi energy. It depends upon band gap, temperature as well as effective mass of charge carriers.
Fermi Energy:
In an intrinsic semiconductor, the Fermi level (Fermi energy) lies roughly in the middle of the band gap. The Fermi level itself does not directly determine conductivity; rather, it indicates the energy level at which the probability of finding an electron is \(50 \%\). The conductivity is determined by the number of electrons that have enough energy to jump into the conduction band and the number of holes left behind. -
Question 34 of 115
34. Question
The combination of gates shown below yields is [UK PMT 2014]
 CorrectIncorrect
CorrectIncorrectHint
(d) \(Y_1=\) Output due to NAND gate \(1=\overline{A \cdot A}=\bar{A}\)
\(Y_2=\) Output due to NAND gate \(2=\overline{B \cdot B}=\bar{B}\)
Output due to NAND gate 3
\(
Y=\overline{Y_1 \cdot Y_2}=\overline{Y_1}+\overline{Y_2}=\overline{\bar{A}}+\overline{\bar{B}}=A+B
\)
This is OR gate. Hence, the combination is equivalent to an OR gate. -
Question 35 of 115
35. Question
In insulators ( CB is Conduction Band and VB is Valence Band) [MHT CET 2014]
CorrectIncorrectHint
(c) In an insulator, the conduction band (CB) is empty and the valence band (VB) is filled with electrons. This means there are no free electrons in the conduction band to carry an electrical current, while the valence band, the highest energy band containing electrons, is completely occupied.
-
Question 36 of 115
36. Question
Identify the wrong statement. [Kerala CEE 2014]
CorrectIncorrectHint
(c) The resistivity of semiconductors is greater than metals.
Explanation: While semiconductors have lower resistivity than insulators, their resistivity is still higher than that of metals.
-
Question 37 of 115
37. Question
In \(n\)-type semiconductor, electrons are majority charge carriers but it does not show any negative charge. The reason is [KCET 2014]
CorrectIncorrectHint
(d) The \(n\)-type semiconductor region has (negative) electrons as majority charge carriers and an equal number of fixed positively charged donor ions. Again, the material as a whole is neutral. That is a reason, that atom is electrically neutral.
-
Question 38 of 115
38. Question
Identify the wrong statement with reference to solar cell. [Kerala CEE 2014]
CorrectIncorrectHint
(c) The wrong statement with reference to a solar cell is that, it uses materials with band gap of 5 eV. Because, for solar cell, band gap is less than 3 eV.
-
Question 39 of 115
39. Question
In the circuit shown below, assume the diode to be ideal. When \(V_i\) increases from 2 V to 6 V, then the change in the current is (in mA ) [WB JEE 2014]
 CorrectIncorrect
CorrectIncorrectHint
(b) Initial current, \(I_{\mathrm{in}}=0\)
Final current, \(I_f=\frac{(6-3)}{150}=0.02 \mathrm{~A}\)
So, change in current, \(\Delta I=I_f-I_{\mathrm{in}}=0.02-0\) \(=0.02 \mathrm{~A}=20 \mathrm{~mA}\) -
Question 40 of 115
40. Question
The minimum number of NAND gates used to construct an OR gate is [Kerala CEE 2014]
CorrectIncorrectHint
(b) If the two inputs of a NAND gate are joined together, it works as a NOT gate. Now, if the inputs \(A\) and \(B\) are inverted by using two NOT gates (obtained from two NAND gates) and the resulting output \(\bar{A}\) and \(\bar{B}\) are fed to a third NAND gates.
So, three NAND gates are required to make an OR gate. -
Question 41 of 115
41. Question
The output \(Y\) of the logic circuit given below is [J & K CET 2014]
 CorrectIncorrect
CorrectIncorrectHint
\(
\text { (b) By absorption law, output } Y=\bar{A}+\bar{A} \cdot B
\)
\(
Y=\bar{A} \cdot B+\bar{A}=\bar{A} \cdot(B+1)=\bar{A} \cdot 1=\bar{A}
\) -
Question 42 of 115
42. Question
For the given digital circuit, identify the logic gate.
 CorrectIncorrect
CorrectIncorrectHint
(d) According to the logical relationship, Output, \(Y=\overline{\bar{A}+\bar{B}}=\bar{A} \cdot \bar{B}=A \cdot B\)
So, it is AND gate. -
Question 43 of 115
43. Question
Of the diodes shown in the following diagrams, which one is reverse-biased? [UK PMT 2014]
 CorrectIncorrect
CorrectIncorrectHint
(c) In this case, diode P side is 0 V and the N side is connected to 5 V. So the diode is reverse biased.
-
Question 44 of 115
44. Question
The output of an AND gate is connected to both the inputs of a NOR gate, then this circuit will act as a [Kerala CET 2013]
CorrectIncorrectHint
(d) \(Y_1=A B \quad Y_2=\bar{Y}_1 \cdot Y_1=\bar{Y}_1\)
-
Question 45 of 115
45. Question
In \(p\)-type semiconductor, the acceptor level lies [Kerala CET 2013]
CorrectIncorrectHint
(c) In a p-type semiconductor, the acceptor energy level lies near the valence band.
Explanation:
In p-type semiconductors, acceptor impurities (like Boron in Silicon) introduce energy levels near the valence band. These acceptor levels are slightly above the valence band and facilitate the movement of electrons from the valence band to these acceptor levels, creating holes (positive charge carriers) in the valence band. -
Question 46 of 115
46. Question
When a \(p-n\) junction is reverse biased, then the current through the junction is mainly due to [MP PMT 2013]
CorrectIncorrectHint
(d) When a p-n junction is reverse biased, the current through the junction is mainly due to only drift of charges.
Explanation:
Reverse biasing:
In reverse bias, the applied voltage creates a strong electric field across the p-n junction, which pushes the minority carriers (electrons from the p-side and holes from the \(n\)-side) to drift across the junction. -
Question 47 of 115
47. Question
What is the value of \(\bar{A}+A\) in the Boolean algebra? [UP CPMT 2013]
CorrectIncorrectHint
(c) \(\bar{A}+A\) should always be equal to 1. As, addition of inputs like 1 to 0 or 0 to 1 always gives 1.
-
Question 48 of 115
48. Question
The depletion layer in the \(p-n\) junction region is caused by [UP CPMT 2013]
CorrectIncorrectHint
(c) The depletion layer in the \(p-n\) junction region is caused due to the diffusion of carriers on either side of the junction.
Explanation:
Diffusion of carriers:
In a p-n junction, the majority carriers (holes in the p-type region and electrons in the n-type region) naturally diffuse across the junction due to their concentration gradients. This diffusion causes electrons to move from the n-side to the p-side and holes to move from the p-side to the n-side. -
Question 49 of 115
49. Question
Out of the following curves, which one represents a digital signal? [UP CPMT 2013]
 CorrectIncorrect
CorrectIncorrectHint
(c) A digital signal has only two values of voltage variation with time. Its value is either zero or maximum (1).
-
Question 50 of 115
50. Question
The circuit has two oppositely connected ideal diodes in parallel as shown in the figure. What is the current flowing in the circuit? [UP CPMT 2013]
 CorrectIncorrect
CorrectIncorrectHint
(c) The diode \(D_1\) is in reversed biased and \(D_2\) is in forward biased. The resistance of \(D_1\) in in becomes infinite and of \(D_2\) is zero. Therefore, current in the circuit,
\(
I=\frac{\mathrm{emf}}{\text { total resistance }}=\frac{12}{4+2}=2 \mathrm{~A}
\) -
Question 51 of 115
51. Question
A diode is connected to 220 V (rms) AC in series with a capacitor as shown in the figure. The voltage across the capacitor is [JCECE 2012]
 CorrectIncorrect
CorrectIncorrectHint
\(
\begin{aligned}
& \text { (c) As, } V_{\mathrm{rms}}=\frac{V_0}{\sqrt{2}} \Rightarrow 220=\frac{V_0}{\sqrt{2}} \\
& \Rightarrow \quad V_0=311.1 \mathrm{~V}
\end{aligned}
\) -
Question 52 of 115
52. Question
Which one of the following statements is not correct in case of a semiconductor? [BCECE (Mains) 2012]
CorrectIncorrectHint
(c) The incorrect statement about semiconductors is At absolute zero temperature, it behaves like a conductor.
Explanation: At absolute zero temperature, a semiconductor behaves like an insulator because all the electrons are bound to their atoms and there are no free electrons available to conduct electricity.
-
Question 53 of 115
53. Question
In intrinsic semiconductor, at room temperature number of electrons and holes are [Manipal 2012]
CorrectIncorrectHint
(a) At room temperature, due to thermal vibrations, the few bonds of intrinsic semiconductor are broken and producing equal number of electrons and holes in the semiconductor.
-
Question 54 of 115
54. Question
Which of the following is not a rectifier circuit? [AMU 2012]
 CorrectIncorrect
CorrectIncorrectHint
(b) is not a rectifier circuit because it does not follow the typical configuration of diodes used in rectifier circuits.
-
Question 55 of 115
55. Question
Number of electrons in the valence shell of a semiconductor is
CorrectIncorrectHint
(c) A semiconductor typically has four electrons in its valence shell. This characteristic allows semiconductors to form covalent bonds and exhibit properties between those of conductors and insulators.
-
Question 56 of 115
56. Question
A piece of copper and the other of germanium are cooled from the room temperature to 80 K, then which of the following would be a correct statement?
CorrectIncorrectHint
(d) Copper is a metal.
Germanium is a semiconductor.
Both are cooled from room temperature to 80 K.
Metals have a positive temperature coefficient of resistance.
Semiconductors have a negative temperature coefficient of resistance.
Copper is a metal.
For metals, resistance decreases as temperature decreases.
Therefore, the resistance of copper decreases when cooled to 80 K .
Germanium is a semiconductor.
For semiconductors, resistance increases as temperature decreases. Therefore, the resistance of germanium increases when cooled to 80 K.
The resistance of copper decreases while that of germanium increases. -
Question 57 of 115
57. Question
In a semiconductor, the separation between conduction band and valence band is of the order of
CorrectIncorrectHint
(c) The separation between the conduction band and valence band in a semiconductor is of the order of 1 eV .
Explanation:
Semiconductors have a band gap (energy difference between the valence and conduction bands) that is relatively small, allowing some electrons to jump from the valence band to the conduction band with moderate energy input. This allows for some electrical conductivity, which is why they are called semiconductors. A band gap of 1 eV is characteristic of many common semiconductors like silicon and germanium. -
Question 58 of 115
58. Question
In a good conductor, the energy gap between the conduction band and the valence band is
CorrectIncorrectHint
(d) zero.
Explanation:
In a good conductor, the valence band and conduction band essentially overlap, meaning there is no significant energy difference between them. This allows electrons to move freely between the bands, facilitating electrical conduction. -
Question 59 of 115
59. Question
In semiconductors, at room temperature the
CorrectIncorrectHint
(a) At room temperature in semiconductors, the valence band is partially empty and the conduction band is partially filled. This is because some electrons in the valence band gain enough thermal energy to jump to the conduction band, leaving behind “holes” (vacancies) in the valence band. These holes and the electrons in the conduction band can then contribute to electrical conductivity.
-
Question 60 of 115
60. Question
A piece of semiconductor is connected in series in an electric circuit. On increasing the temperature, the current in the circuit will
CorrectIncorrectHint
(c) On increasing the temperature, the current in the circuit will increase because with rise in temperature, resistance of semiconductor decreases, hence overall resistance of the circuit decreases, which in turn increases the current in the circuit.
-
Question 61 of 115
61. Question
The forbidden energy gap in the energy bands of germanium at room temperature is about
CorrectIncorrectHint
(c)\(\Delta E_{g(\text { Germanium })}=0.67 \mathrm{eV}\)
-
Question 62 of 115
62. Question
The energy band gap of Si is
CorrectIncorrectHint
(b) The energy band gap of Silicon \((\mathrm{Si})\) at room temperature is a well-known physical constant. It is approximately 1.1 eV.
-
Question 63 of 115
63. Question
What enables Ge to behave as semiconductor even though all electrons in the valence band form covalent bonds? It is due to the small width of
CorrectIncorrectHint
(c) the small width of the forbidden energy gap.
Explanation:
Semiconductor behavior: In a semiconductor like germanium (Ge), not all electrons are completely free to move, but they can be excited to a higher energy level with a relatively small amount of energy. This allows them to conduct electricity to a certain degree. -
Question 64 of 115
64. Question
\(E_g\) for silicon is 1.12 eV and that for germanium is 0.72 eV. Therefore, it can be concluded that
CorrectIncorrectHint
(b) Germanium has a smaller bandgap energy than silicon.
\(
E_{g, G e}<E_{g, S i}
\)
A smaller bandgap means less energy is needed to create electron-hole pairs.
Therefore, more electron-hole pairs are generated in materials with smaller bandgaps at a given temperature.
Since germanium has a smaller bandgap, more electron-hole pairs will be generated in germanium than in silicon at room temperature.
This implies less electron-hole pairs will be generated in silicon than in germanium.
Less number of electron-hole pairs will be generated in silicon than in germanium at room temperature. -
Question 65 of 115
65. Question
When the electrical conductivity of a semiconductor is due to the breaking of its covalent bonds, then the semiconductor is said to be
CorrectIncorrectHint
(c) When the electrical conductivity of a semiconductor is solely due to the breaking of its covalent bonds, the semiconductor is said to be intrinsic.
Explanation: Intrinsic semiconductors are pure semiconductors (without any doping) where the electrical conductivity arises from thermally generated charge carriers (electrons and holes) formed when covalent bonds break.
-
Question 66 of 115
66. Question
A \(p-n\) junction has a thickness of the order of
CorrectIncorrectHint
(c) \(\text { The thickness of a } p-n \text { junction is of the order of } 10^{-6} \mathrm{~m} \text {. }\)
-
Question 67 of 115
67. Question
At room temperature, a \(p\)-type semiconductor has
CorrectIncorrectHint
(a) At room temperature, a \(p\)-type semiconductor has large number of holes and few electrons, as holes are majority charge carriers.
Explanation:
p-type semiconductor:
In a p-type semiconductor, impurities are added that create “holes” – essentially vacancies where electrons should be. These holes act as positive charge carriers.Few electrons:
While there are still a few free electrons present in the semiconductor, their number is significantly smaller compared to the number of holes. -
Question 68 of 115
68. Question
Which of the following statements is not true?
CorrectIncorrectHint
(c) “The majority carriers in \(n\)-type semiconductors are holes”.
Explanation: In \(n\)-type semiconductors, the majority carriers are electrons, not holes.
-
Question 69 of 115
69. Question
\(n\)-type semiconductor is formed
CorrectIncorrectHint
(d) An \(n\)-type semiconductor is formed when a germanium crystal is doped with an impurity containing five valence electrons.
-
Question 70 of 115
70. Question
An \(n\)-type semiconductor is
CorrectIncorrectHint
(c) An \(n\)-type semiconductor is neutral.
Explanation:
” n ” stands for negative: In an \(n\)-type semiconductor, the majority charge carriers are electrons, which are negatively charged. However, this doesn’t mean the semiconductor is overall negatively charged.
Electrical neutrality: Despite the presence of more free electrons, there are also an equal number of positive charges (protons) within the semiconductor, resulting in a net neutral charge. -
Question 71 of 115
71. Question
Electrical conductivity of intrinsic and \(p\)-type semiconductor increases with increase in
CorrectIncorrectHint
(d) The electrical conductivity of both intrinsic and \(p\)-type semiconductors increases with an increase in temperature.
Explanation:
Intrinsic semiconductors:
In these materials, the conductivity arises from thermally generated electron-hole pairs. When temperature increases, more electrons gain enough energy to jump from the valence band to the conduction band, creating more charge carriers (electrons and holes) and thus increasing conductivity.P-type semiconductors:
Similar to intrinsic semiconductors, increasing temperature in p-type semiconductors also enhances conductivity. The added dopant atoms in p-type materials create an abundance of holes, but the conductivity is still significantly influenced by the number of free charge carriers. As temperature increases, more electrons are excited into the conduction band, and while some holes may be filled, the overall effect is an increase in conductivity. -
Question 72 of 115
72. Question
The valency of the impurity atom that is to be added to germanium crystal, so as to make it a \(n\)-type semiconductor, is
CorrectIncorrectHint
(b) Pentavalent impurity atom is to be added to Ge crystal, so as to make a \(n\)-type semiconductor.
-
Question 73 of 115
73. Question
The potential barrier for silicon diode is approximately
CorrectIncorrectHint
(b) 0.6 V .
Explanation:
A silicon diode’s barrier potential is typically around 0.6 to 0.7 volts. This is the voltage that needs to be applied across the diode to overcome the internal barrier and allow current to flow through it. -
Question 74 of 115
74. Question
In comparison to half-wave rectifier, full wave rectifier gives lower
CorrectIncorrectHint
(d) A full-wave rectifier does not have lower efficiency, average DC, or average output voltage compared to a half-wave rectifier. In fact, it has higher efficiency, and higher average DC and output voltage. Therefore, the correct answer is none of these.
-
Question 75 of 115
75. Question
Zener diode is used as
CorrectIncorrectHint
(d) A Zener diode is primarily used as a AC voltage stabilizer. While it can be used in conjunction with other components to create rectifiers, its main function is to maintain a constant voltage output even when the input voltage or load conditions change.
-
Question 76 of 115
76. Question
Serious drawback of the semiconductor device is that they
CorrectIncorrectHint
(a) The serious drawback of a semiconductor device is that they cannot be used with high voltage.
Explanation: Semiconductors have a limited tolerance for high voltages, which can damage them.
-
Question 77 of 115
77. Question
Intrinsic semiconductor is electrically neutral. Extrinsic semiconductor having large number of current carriers would be
CorrectIncorrectHint
(d) Extrinsic semiconductors are also electrically neutral.
Explanation:
Intrinsic semiconductors:
These are pure semiconductors like silicon or germanium where the number of electrons is equal to the number of holes, resulting in no net charge, making them electrically neutral.Extrinsic semiconductors:
These are created by adding impurities to an intrinsic semiconductor, which introduces additional charge carriers (electrons or holes). However, even with a large number of current carriers, the overall charge remains neutral because the added dopants are electrically neutral. -
Question 78 of 115
78. Question
Potential barrier developed in a junction diode opposes
CorrectIncorrectHint
(b) majority carrier only. A potential barrier in a junction diode opposes the flow of majority carriers across the junction.
Explanation:
Majority carriers:
In a p-n junction diode, the majority carriers in the p-type material are holes, and the majority carriers in the n-type material are electrons.
Potential barrier:
When a \(p-n\) junction is formed, a depletion region (also called a space charge region) forms at the junction, creating a potential barrier.Opposition to majority carriers:
This potential barrier opposes the flow of majority carriers (holes from the p-region and electrons from the \(n\)-region) from crossing the junction.Minority carriers:
While the potential barrier opposes majority carriers, it actually aids the flow of minority carriers (electrons in the p-region and holes in the n-region). -
Question 79 of 115
79. Question
If \(n_e\) and \(v_d\) be the number of electrons and drift velocity in a semiconductor. When the temperature is increased,
CorrectIncorrectHint
(a) As, \(\quad i=n_e A v_d\)
So, \(\quad v_d=\frac{i}{n_e A}\)
So, when temperature is increased, \(n_e\) increases and \(v_d\) decreases. -
Question 80 of 115
80. Question
The dominant mechanisms for motion of charge carriers in forward and reverse biased silicon \(p-n\) junctions are
CorrectIncorrectHint
(b) In forward biasing, the diffusion current increases and drift current remains constant, so net current is due to diffusion. In reverse biasing diffusion is not possible, so net current (very small) is due to the drift.
-
Question 81 of 115
81. Question
In \(p-n\) junction, avalanche current flows in circuit when biasing is
CorrectIncorrectHint
(b) At a specific reverse voltage (breakdown voltage) in \(p-n\) junction, a huge current flows in reverse direction known as avalanche current.
-
Question 82 of 115
82. Question
In an unbiased \(p-n\) junction, holes diffuse from the \(p\)-region to \(n\)-region because
CorrectIncorrectHint
(c) In an unbiased p-n junction, holes diffuse from the p-region to the n-region primarily due to the concentration gradient, meaning the higher concentration of holes in the p -region compared to the n -region.
Explanation:
Concentration Gradient:
The fundamental driving force for diffusion is the tendency of particles (in this case, holes) to move from an area of high concentration to an area of low concentration. Since the p-region has a higher concentration of holes than the \(n\)-region, holes will naturally move across the junction from the \(p\)-side to the \(n\)-side. -
Question 83 of 115
83. Question
Select the correct statement.
CorrectIncorrectHint
(a) In a full wave rectifier, two diodes work alternately.
Explanation: A full wave rectifier uses two diodes to convert the AC input into DC, but they alternate which diode conducts during each half cycle, effectively utilizing both halves of the waveform.
-
Question 84 of 115
84. Question
Temperature coefficient of resistance of semiconductor is
CorrectIncorrectHint
(d) Resistance of semiconductor decreases with increase in temperature. Hence, its temperature coefficient is negative.
Explanation:
We know that the temperature coefficient of resistance is equal to the change in resistance of the wire of resistance one ohm at \(0^{\circ} \mathrm{C}\) when the temperature changes by \(1^{\circ} \mathrm{C}\).
It is denoted by \(\alpha\) and given as,
\(
\alpha=\frac{R_t-R_0}{R_0 t}
\)
Where, \(R_t\) is the resistance of the material at temperature \((\mathrm{t})\).
\(R_0\) is the resistance of the material at absolute zero temperature.
\(t\) is the temperature.
Let’s consider a case,
If \(R_0=1\) and \(t=1^{\circ} C\). Then, \(\alpha=R_t-1\).
In general, for two different temperature and resistance, we can write the equation as,
\(
\alpha=\frac{R_2-R_1}{R_1 t-R_2 t}
\)
From this equation, we can say that as the temperature of a semiconductor increases, resistance decreases. Then the value of \(R_2-R_1\) becomes a negative value. Therefore, the entire value becomes negative in the case of the semiconductor. Thus, the temperature coefficient of resistance ( \(\alpha\) ) of a semiconductor is always negative. -
Question 85 of 115
85. Question
A semiconducting device is connected in a series circuit with a battery and a resistance. A current is found to pass through the circuit. If the polarity of the battery is reversed, then the current drops to almost zero, the device may be
CorrectIncorrectHint
(c) p-n junction. Reversing the polarity of the battery in a p-n junction causes it to be reverse-biased, significantly reducing the current flow.
-
Question 86 of 115
86. Question
GaAs (with a band gap \(=1.5 \mathrm{eV}\) ) as an LED can emit
CorrectIncorrectHint
(c) The band gap \(=1.5 \mathrm{e} \mathrm{V}\)
\(
\begin{aligned}
& =1.5 \times 1.6 \times 10^{-19} J \\
& =2.40 \times 1^{-19} J
\end{aligned}
\)
Wavelength of radiation emitted \((\lambda)=\frac{h c}{E}\)
\(
\begin{aligned}
& =\frac{6.6 \times 10^{-34} \times 3 \times 10^8}{2.4 \times 10^{-19}} \\
& =8.25 \times 10^{-7} \mathrm{~m} \\
& =8250 Å
\end{aligned}
\)
This wavelength belongs to infrared rays. -
Question 87 of 115
87. Question
If no external voltage is applied across \(p-n\) junction, then there would be
CorrectIncorrectHint
(b) When no external voltage is applied across a p-n junction, there would be an electric field pointing from the \(n\)-type to the \(p\)-type side across the junction.
Explanation:
Diffusion of charge carriers: In a p-n junction, electrons from the n-type region naturally diffuse into the p-type region, leaving behind positively charged ions in the \(n-\) type region. Similarly, holes from the p-type region diffuse into the n-type region, creating negatively charged ions in the p-type region.Depletion region formation: This diffusion of charge carriers creates a region near the junction depleted of mobile charge carriers, called the depletion region.
Electric field development: Due to the separation of positive and negative charges, an electric field is established across the depletion region, pointing from the n-type side to the p-type side.
-
Question 88 of 115
88. Question
Carbon, silicon and germanium have four valence electrons each. These are characterised by valence and conduction bands separated by energy band gap respectively equal to \(\left(E_g\right)_{\mathrm{C}},\left(E_g\right)_{\mathrm{Si}}\) and \(\left(E_g\right)_{\mathrm{Ge}}\). Which of the following statement(s) is/are true?
CorrectIncorrectHint
\(
\begin{aligned}
& \text { (d) }\left(E_g\right)_{\mathrm{Ge}}<\left(E_g\right)_{\mathrm{Si}}<\left(E_g\right)_{\mathrm{C}} \\
& {\left[\because\left(E_g\right)_{\mathrm{Ge}}=0.7 \mathrm{eV},\left(E_g\right)_{\mathrm{Si}}=1.1 \mathrm{eV},\left(E_g\right)_{\mathrm{C}}=5.5 \mathrm{eV}\right]}
\end{aligned}
\) -
Question 89 of 115
89. Question
When a diode is heavily doped, then
CorrectIncorrectHint
(a) When a diode is heavily doped, the Zener voltage will be low. This is correct.
Explanation: Heavy doping reduces the width of the depletion region, which leads to a lower Zener breakdown voltage.
(b) the avalanche voltage will be high: Avalanche breakdown is related to the Zener voltage. A lower Zener voltage due to heavy doping also means a lower avalanche voltage. This is incorrect.
(c) the depletion region will be thin: This is correct. Heavy doping results in a thin depletion region.
(d) the leakage current will be low: While the specific relationship between leakage current and doping level is complex, heavy doping doesn’t necessarily guarantee a low leakage current. Leakage current is a small reverse current that always flows through a diode even when it’s reverse biased. This is incorrect. -
Question 90 of 115
90. Question
The electrical circuit used to get smooth DC output from a rectifier circuit is called
CorrectIncorrectHint
(b) From rectifier we get pulsating DC, which consists of AC ripples, to remove these unwanted ripples, we use filter circuits.
-
Question 91 of 115
91. Question
The maximum efficiency of full wave rectifier is
CorrectIncorrectHint
\(
\begin{aligned}
& \text { (d) For full wave rectifier, } \eta=\frac{81.2}{1+\frac{r_f}{R_L}} \\
& \Rightarrow \quad n_{\max }=81.2 \% \quad\left(\because r_f \ll R_L\right)
\end{aligned}
\) -
Question 92 of 115
92. Question
Given below are symbols for some logic gates.

The XOR gate and NOR gates respectively are
CorrectIncorrectHint
(b) An XOR gate (Exclusive OR) is represented by symbol II, and a NOR gate (NOT OR ) is represented by symbol III.
-
Question 93 of 115
93. Question
If a full wave rectifier, circuit is operating in 50 Hz mains, then the fundamental frequency in the ripple will be
CorrectIncorrectHint
(b)
\(
\begin{aligned}
&\text { For a full-wave rectifier, the ripple frequency is twice the input frequency. }\\
&f_{\text {ripple }}=2 \times f_{\text {input }}
\end{aligned}
\)
\(
\begin{aligned}
&\text { The input frequency is } 50 \mathrm{~Hz} \text {. }\\
&f_{\text {ripple }}=2 \times 50 \mathrm{~Hz}= 100 \mathrm{~Hz}
\end{aligned}
\) -
Question 94 of 115
94. Question
With an AC input from 50 Hz power line, the ripple frequency is
CorrectIncorrectHint
(c) Ripple frequency (half-wave) = Input AC frequency .
Ripple frequency (half-wave) = 50 Hz.
In a full-wave rectifier, the ripple frequency is twice the input \(A C\) frequency.
Ripple frequency (full-wave) \(=2 \times\) Input AC frequency.
Ripple frequency (full-wave) \(=2 \times 50 \mathrm{~Hz}=100 \mathrm{~Hz}\). -
Question 95 of 115
95. Question
The temperature \((T)\) dependence on resistivity \((\rho)\) of a semiconductor is represented by
 CorrectIncorrect
CorrectIncorrectHint
(c) As the temperature (T) increases, the conductivity ( \(\sigma\) ) increases.
Therefore, the resistivity ( \(\rho\) ) must decrease.
This can be expressed mathematically as:
\(\sigma \propto T\) (as T increases, \(\sigma\) increases)
Consequently, we can say:
\(\rho \propto \frac{1}{T} \quad\) (as T increases, \(\rho\) decreases)
Resistivity ( \(\rho\) ) on the \(y\)-axis and temperature (T) on the \(x\)-axis, the graph will be a rectangular hyperbola. -
Question 96 of 115
96. Question
Under which of the following conditions, does an avalanche breakdown in a semiconductor diode occur?
CorrectIncorrectHint
(b) Avalanche breakdown in a semiconductor diode occurs when reverse bias exceeds a certain value.
Explanation: Avalanche breakdown happens when a high reverse bias voltage is applied to the diode, causing a strong electric field that accelerates minority charge carriers to high speeds, resulting in the creation of more electron-hole pairs through impact ionization. This rapid increase in current is known as avalanche breakdown.
-
Question 97 of 115
97. Question
The width of depletion region in a \(p-n\) junction diode is 500 nm and an intense electric field of \(6 \times 10^5 \mathrm{Vm}^{-1}\) is also found to exist. The height of potential barrier is
CorrectIncorrectHint
(a) Given, \(E=6 \times 10^5 \mathrm{Vm}^{-1}\)
\(
\begin{aligned}
d & =500 \mathrm{~nm}=500 \times 10^{-9} \mathrm{~m} \\
V & =?
\end{aligned}
\)
\(\therefore \quad\) Potential barrier, \(V=E d=\left(6 \times 10^5\right)\left(500 \times 10^{-9}\right)=0.30 \mathrm{~V}\) -
Question 98 of 115
98. Question
Mobilities of electrons and holes in a sample of intrinsic germanium at room temperature are \(0.36 \mathrm{~m}^2 / \mathrm{Vs}\) and \(0.17 \mathrm{~m}^2 / \mathrm{Vs}\). The electron and hole densitils are each equal to \(2.5 \times 10^{19} \mathrm{~m}^{-3}\). The electrical conductivity of germanium is
CorrectIncorrectHint
\(
\begin{aligned}
&\text { (c) The electrical conductivity of germanium, }\\
&\begin{aligned}
\sigma & =\frac{1}{\rho}=e\left(\mu_e n_e+\mu_h n_n\right) \\
& =\left(1.6 \times 10^{-19}\right)[0.36+0.17]\left(2.5 \times 10^{19}\right) \\
& \quad\left(\because n_e=n_h=2.5 \times 10^{19} \mathrm{~m}^{-3}\right) \\
& =2.12 \mathrm{Sm}^{-1}
\end{aligned}
\end{aligned}
\) -
Question 99 of 115
99. Question
A Si specimen is made into \(p\)-type semiconductor by doping on an average one indium atom per \(6 \times 10^7\) silicon atoms. If the number density of atoms in Si be \(6 \times 10^{28} \mathrm{~m}^{-3}\), then what is the indium atom per \(\mathrm{cm}^3\)?
CorrectIncorrectHint
(b) In \(1 \mathrm{~cm}^3\) of Si , there will be total \(\frac{6 \times 10^{28}}{10^6}=6 \times 10^{22}\) atoms Per \(6 \times 10^7\) silicon atoms, there is one indium atom. Therefore, total number of indium atoms will be \(\frac{6 \times 10^{22}}{6 \times 10^7}=10^{15}\)
-
Question 100 of 115
100. Question
In the diagram, the input is across the terminals \(A\) and \(C\) and the output is across \(B\) and \(D\). Then, the output is
 CorrectIncorrect
CorrectIncorrectHint
(a) During the first half cycle when \(V_A>V_C[//latex], all the four diodes are forward biased. Hence, no current will flow through [latex]R_L\). During second half cycle when \(V_C>V_A\), all the four diodes are reverse biased. Again, no current will flow through \(R_L\).
-
Question 101 of 115
101. Question
When forward bias is applied to a \(p-n\) junction, then what happens to the potential barrier \(V_B\) and the width of charge depleted region \(x\)?
CorrectIncorrectHint
(d) In forward biasing, both potential barrier \(V_B\) and the width of charge depleted region \(x\) decreases.
-
Question 102 of 115
102. Question
What is the current in the circuit shown below?
 CorrectIncorrect
CorrectIncorrectHint
(a) Here, diode is in reverse biased condition. In reverse biasing, it acts as open circuit, hence no current flows.
-
Question 103 of 115
103. Question
The diode shown in the circuit is a silicon diode. The potential difference between the points \(A\) and \(B\) will be
 CorrectIncorrect
CorrectIncorrectHint
(a) In the given circuit, diode is in reverse biasing, so it acts as open circuit. Hence, potential difference between \(A\) and \(B\) is 6 V.
-
Question 104 of 115
104. Question
The value of DC voltage in half-wave rectifier in converting AC voltage \(V=100 \sin (314 t)\) into DC is
CorrectIncorrectHint
(c) The average DC voltage \(\left(V_{D C}\right)\) for a half-wave rectifier is given by the formula:
\(
V_{D C}=\frac{V_0}{\pi}=\frac{100}{3.14} \simeq 31 \mathrm{~V}
\) -
Question 105 of 115
105. Question
The forbidden energy gap in Ge is 0.72 eV. Given, \(h c=12400 \mathrm{eV}-Å\). The maximum wavelength of radiation that will generate electron-hole pair is
CorrectIncorrectHint
\(
\begin{aligned}
& \text { (c) Energy gap, } E_g=\frac{h c}{\lambda} \\
& \Rightarrow \quad \lambda=\frac{h c}{E_g}=\frac{12400 \mathrm{eV}-Å}{0.72 \mathrm{eV}}=17222 Å
\end{aligned}
\) -
Question 106 of 115
106. Question
The current in the circuit will be
 CorrectIncorrect
CorrectIncorrectHint
(b) \(D_1\) is reverse biased. Therefore, no current will flow through \(20 \Omega\) resistance. As, \(30 \Omega\) and \(20 \Omega\) resistance are in series, so total current will be \((5 / 50) \mathrm{A}\).
-
Question 107 of 115
107. Question
A Zener diode, having breakdown voltage equal to 15 V, is used in a voltage regulator circuit shown in the figure. The current through the diode is
 CorrectIncorrect
CorrectIncorrectHint
(b) Voltage across Zener diode is constant,

\(
\begin{gathered}
i_{1 \mathrm{k} \Omega}=\frac{15}{1 \times 10^3}=15 \mathrm{~mA} \\
i_{250 \Omega}=\frac{(20-15)}{250}=\frac{5}{250}=\frac{20}{1000}=20 \mathrm{~mA} \\
\therefore \quad i_{\text {Zener diode }}=(20-15)=5 \mathrm{~mA}
\end{gathered}
\) -
Question 108 of 115
108. Question
The logic circuit shown below has the input waveforms \(A\) and \(B\) as shown. Pick out the correct output waveform.
 CorrectIncorrect
CorrectIncorrectHint
\(
\text { (a) } Y=\overline{\bar{A}+\bar{B}}=A \cdot B \text {, i.e. it is a AND gate. }
\) -
Question 109 of 115
109. Question
A full wave rectifier circuit along with the input and output voltages is shown in the figure

The contribution to output voltage from \(D_2\) is
CorrectIncorrectHint
(b) In the positive half cycle of input AC signal diode, \(D_1\) is in forward biased and \(D_2\) is in reverse biased, so in the output voltage signal, \(A\) and \(C\) are due to diode \(D_1\). In negative half cycle of input AC signal, diode \(D_2\) is in forwards biased, hence it conducts, so output signals \(B\) and \(D\) are due to diode \(D_2\).
-
Question 110 of 115
110. Question
A researcher wants an alarm to sound when the temperature of air in his controlled research chamber rises above \(40^{\circ} \mathrm{C}\) or falls below \(20^{\circ} \mathrm{C}\). The alarm can be triggered by the output of a
CorrectIncorrectHint
(d) The alarm can be triggered by the output of an OR gate. For both the temperatures above \(40^{\circ} \mathrm{C}\) and below \(20^{\circ} \mathrm{C}\).
-
Question 111 of 115
111. Question
The graph given below represents the \(I-V\) characteristics of a Zener diode. Which part of the characteristics curve is most relevant for its operation as a voltage regulator?
 CorrectIncorrect
CorrectIncorrectHint
(d) If the reverse bias voltage is greater than the \(V_Z\), then there is breakdown condition. In breakdown region, i.e. \(V_i>V_Z\) and for a long range of load \(\left(R_L\right)\), the voltage is constant.
-
Question 112 of 115
112. Question
If the voltage between the terminals \(A\) and \(B\) is 17 V and Zener breakdown voltage is 9 V, then the potential across \(R\) is
 CorrectIncorrect
CorrectIncorrectHint
\(
\text { (b) The potential across } R=17 \mathrm{~V}-9 \mathrm{~V}=8 \mathrm{~V}
\) -
Question 113 of 115
113. Question
In the following circuit, the current flowing through \(1 \mathrm{k} \Omega\) resistor is
 CorrectIncorrect
CorrectIncorrectHint
(b) In the given circuit, the Zener diode is used as a voltage regulating device.
Hence, the voltage across \(1 \mathrm{k} \Omega\) is 5 V.
Current flowing through \(1 \mathrm{k} \Omega\) resistor,
\(
I=\frac{5}{1 \times 10^3}=5 \times 10^{-3} \mathrm{~A}=5 \mathrm{~mA}
\) -
Question 114 of 115
114. Question
In the given circuit

The current through the battery is
CorrectIncorrectHint
(c) In the given circuit, diode \(D_1\) is reverse biased, so it will not conduct. Diodes \(D_2\) and \(D_3\) are forward biased, so they will conduct. The corresponding equivalent circuit is as shown in the figure

The equivalent resistance of the circuit is
\(
R_{e q}=\frac{(5+5) \times 20}{(5+5)+20}=\frac{10 \times 20}{10+20}=\frac{200}{30}=\frac{20}{3} \Omega
\)
Current through the battery, \(I=\frac{10 \mathrm{~V}}{\frac{20}{3} \Omega}=1.5 \mathrm{~A}\) -
Question 115 of 115
115. Question
Identify the semiconductor devices whose characteristics are given below, in the order I, II, III, IV.
 CorrectIncorrect
CorrectIncorrectHint
(d) Simple diode, zener diode, solar cell, light dependent resistance