NCERT Exemplar Q & A
Quiz Summary
0 of 84 Questions completed
Questions:
Information
You have already completed the quiz before. Hence you can not start it again.
Quiz is loading…
You must sign in or sign up to start the quiz.
You must first complete the following:
Results
Results
0 of 84 Questions answered correctly
Your time:
Time has elapsed
You have reached 0 of 0 point(s), (0)
Earned Point(s): 0 of 0, (0)
0 Essay(s) Pending (Possible Point(s): 0)
Categories
- Not categorized 0%
- 1
- 2
- 3
- 4
- 5
- 6
- 7
- 8
- 9
- 10
- 11
- 12
- 13
- 14
- 15
- 16
- 17
- 18
- 19
- 20
- 21
- 22
- 23
- 24
- 25
- 26
- 27
- 28
- 29
- 30
- 31
- 32
- 33
- 34
- 35
- 36
- 37
- 38
- 39
- 40
- 41
- 42
- 43
- 44
- 45
- 46
- 47
- 48
- 49
- 50
- 51
- 52
- 53
- 54
- 55
- 56
- 57
- 58
- 59
- 60
- 61
- 62
- 63
- 64
- 65
- 66
- 67
- 68
- 69
- 70
- 71
- 72
- 73
- 74
- 75
- 76
- 77
- 78
- 79
- 80
- 81
- 82
- 83
- 84
- Current
- Review
- Answered
- Correct
- Incorrect
-
Question 1 of 84
1. Question
Consider a current carrying wire (current \(I\) ) in the shape of a circle. Note that as the current progresses along the wire, the direction of \(\mathbf{j}\) (current density) changes in an exact manner, while the current \(I\) remain unaffected. The agent that is essentially responsible for is
CorrectIncorrectHint
(b) Key concept: Current per unit area (taken normal to the current), \(I / A\), is called current density and is denoted by \(\vec{J}\).
The SI unit of the current density are \(\mathrm{A} / \mathrm{m}^2\). The current density is also directed along \(E\) and which is also a vector quantity and the relationship is given by
\(
\vec{J}=\sigma \vec{E}=\frac{\vec{E}}{\rho}
\)
where \(\sigma=\) conductivity and \(\rho=\) resistivity or specific resistance of the substance.
The \(\vec{J}\) changes due to the electric field produced by charges accumulated on the surface of wire. -
Question 2 of 84
2. Question
Two batteries of emf \(\varepsilon_1\) and \(\varepsilon_2\left(\varepsilon_2>\varepsilon_1\right)\) and internal resistances \(r_1\) and \(r_2\) respectively are connected in parallel as shown in Fig 3.1.
 CorrectIncorrect
CorrectIncorrectHint
(a) The equivalent emf of this combination is given by
\(
\varepsilon_{\text {eq }}=\frac{\frac{\varepsilon_1}{r_1}+\frac{\varepsilon_1}{r_2}}{\left(\frac{1}{r_1}+\frac{1}{r_2}\right)}=\frac{\varepsilon_1\left(\frac{1}{r_1}+\frac{\varepsilon_2 / \varepsilon_1}{r_2}\right)}{\left(\frac{1}{r_1}+\frac{1}{r_2}\right)}=\frac{\varepsilon_2\left(\frac{\varepsilon_1 / \varepsilon_2}{r_1}+\frac{1}{r_2}\right)}{\left(\frac{1}{r_1}+\frac{1}{r_2}\right)}
\)
\(
\text { As } \frac{\varepsilon_2}{\varepsilon_1}>1 \Rightarrow \Rightarrow \frac{\left(\frac{1}{r_1}+\frac{\varepsilon_2 / \varepsilon_1}{r_2}\right)}{\left(\frac{1}{r_1}+\frac{1}{r_2}\right)}>1 \text { or } \varepsilon_{\mathrm{eq}}>\varepsilon_1 \text { also } \frac{\varepsilon_1}{\varepsilon_2}<1 \Rightarrow \frac{\left(\frac{\varepsilon_1 / \varepsilon_2}{r_1}+\frac{1}{r_2}\right)}{\left(\frac{1}{r_1}+\frac{1}{r_2}\right)}<1 \text { or } \varepsilon_{\mathrm{eq}}<\varepsilon_2
\)
\(
\begin{aligned}
&\text { Hence }\\
&\varepsilon_1<\varepsilon_{\mathrm{eq}}<\varepsilon_2 .
\end{aligned}
\) -
Question 3 of 84
3. Question
A resistance \(R\) is to be measured using a meter bridge. Student chooses the standard resistance \(S\) to be \(100 \Omega\). He finds the null point at \(l_1=2.9 \mathrm{~cm}\). He is told to attempt to improve the accuracy. Which of the following is a useful way?
CorrectIncorrectHint
(c) The percentage error in \(R\) can be minimised by adjusting the balance point near the middle of the bridge, i.e., when \(l_1\) is close to 50 cm . This requires a suitable choice of \(S\).
Since,
\(
\frac{R}{S}=\frac{R l_1}{R\left(100-l_1\right)}=\frac{l_1}{100-l_4}
\)
Since here, \(R: S:: 2.9: 97.1\) imply that the \(S\) is nearly 33 times to that of \(R\). In orded to make this ratio \(1: 1\), it is necessary to reduce the value of \(S\) nearly \(\frac{1}{33}\) times i.e., nearly \(3 \Omega\). -
Question 4 of 84
4. Question
A metal rod of length 10 cm and a rectangular cross-section of \(1 \mathrm{~cm} \times \frac{1}{2} \mathrm{~cm}\) is connected to a battery across opposite faces. The resistance will be
CorrectIncorrectHint
(a) The resistance of wire is given by
\(
R=\rho \frac{l}{A}
\)
For greater value of \(R, l\) must be higher and \(A\) should be lower and it is possible only when the battery is connected across \(1 \mathrm{~cm} \times\left(\frac{1}{2}\right) \mathrm{cm}\) (area of cross-section \(A\) ). -
Question 5 of 84
5. Question
Which of the following characteristics of electrons determines the current in a conductor?
CorrectIncorrectHint
(a) The relationship between current and drift speed is given by
\(
I=n e A v_d
\)
Here, \(I\) is the current and \(v_d\) is the drift velocity.
So, \(I \propto v_d\)
Thus, only drift velocity determines the current in a conductor. -
Question 6 of 84
6. Question
Kirchhoff’s junction rule is a reflection of
CorrectIncorrectHint
(b, d)
Kirchhoff’s junction rule is also known as Kirchhoff’s current law which states that the algebraic sum of the currents flowing towards any point in an electric network is zero. i.e., charges are conserved in an electric network.
So, Kirchhoff’s junction rule is the reflection of conservation of charge. -
Question 7 of 84
7. Question
Consider a simple circuit shown in Fig 3.2. stands for a variable resistance \(R^{\prime} . R^{\prime}\) can vary from \(R_0\) to infinity. \(r\) is internal resistance of the battery \(\left(r \ll R \ll R_0\right)\).
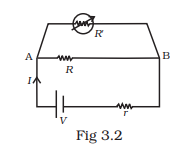 CorrectIncorrect
CorrectIncorrectHint
(a, d)
Here, the potential drop is taking place across \(A B\) and \(r\). Since the equivalent resistance of parallel combination of \(R\) and \(R^{\prime}\) is always less than \(R\), therefore \(I \geq \frac{V}{r+R}\) always.
Note In parallel combination of resistances, the equivalent resistance is smaller than smallest resistance present in combination. -
Question 8 of 84
8. Question
Temperature dependence of resistivity \(\rho(T)\) of semiconductors, insulators and metals is significantly based on the following factors:
CorrectIncorrectHint
(a, b) Resistivity is the intrinsic property of the substance.
For a metallic conductor, resistivity is given by
\(
\rho=\frac{m}{n e^2 \tau}
\)
where \(n\) is the number of charge carriers per unit volume (number density) which can change with temperature \(T\) and \(\tau\) is relaxation time (time interval between two successive collisions) which decreases with the increase of temperature \(\left(T \propto \frac{1}{\tau}\right)\). -
Question 9 of 84
9. Question
The measurement of an unknown resistance \(R\) is to be carried out using Wheatstones bridge (see Fig. below). Two students perform an experiment in two ways. The first students takes \(R_2=10 \Omega\) and \(R_1=5 \Omega\). The other student takes \(R_2\) \(=1000 \Omega\) and \(R_1=500 \Omega\). In the standard arm, both take \(R_3=5 \Omega\).
Both find \(R=\frac{R_2}{R_1} R_3=10 \Omega\) within errors.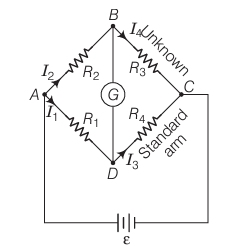 CorrectIncorrect
CorrectIncorrectHint
(b, c)
Given, for first student, \(R_2=10 \Omega, R_1=5 \Omega, R_3=5 \Omega\)
For second student, \(R_1=500 \Omega, R_3=5 \Omega\)
Now, according to Wheatstone bridge rule,
\(
\frac{R_2}{R}=\frac{R_1}{R_3} \Rightarrow R=R_3 \times \frac{R_2}{R_1} \dots(1)
\)
Now putting all the values in Eq. (i), we get \(R=10 \Omega\) for both students. Thus, we can analyse that the Wheatstone bridge is most sensitive and accurate if resistances are of same value.
Thus, the errors of measurement of the two students depend on the accuracy and sensitivity of the bridge, which inturn depends on the accuracy with which \(R_2\) and \(R_1\) can be measured.
When \(R_2\) and \(R_1\) are larger, the currents through the arms of bridge is very weak. This can make the determination of null point accurately more difficult. -
Question 10 of 84
10. Question
In a meter bridge the point D is a neutral point (Fig 3.3).
 CorrectIncorrect
CorrectIncorrectHint
(a, c) Key concept: Meter bridge: In case of meter bridge, the resistance wire AC is 100 cm long. Varying the position of tapping point \(B\), bridge is balanced. If in balanced position of bridge \(A B=l, B C=(100-l)\) so that \(Q / P=(100-l) / l\). Also \(P / Q=R / S=>S=\frac{100-l}{l} \times R\)
When there is no deflection in galvanometer there is no current across the galvanometer, then points B and D are at same potential. That point at which galvanometer shows no deflection is called null point, then potential at B and neutral point D are same. When the jockey contacts a point on the meter wire to the right of D , the potential drop across AD is more than potential drop across AB, which brings the potential of point D less than that of B, hence current flows from B to D in the galvanometer wire.
-
Question 11 of 84
11. Question
A metallic resistor is connected across a battery. If the number of collisions of the free electrons with the lattice is somehow decreased in the resistor (for example, by cooling it), the current will
CorrectIncorrectHint
(a) If the number of collisions of the free electrons with the lattice is decreased, then the drift velocity of the electrons increases.
Current \(i\) is directly proportional to the drift velocity ‘ \({V}_{{d}}\) ‘ and is given by the following relation: \({i}={neAV} {V}_{{d}}\), where ‘ \(n\) ‘ is the number density of electrons and ‘ \(A\) ‘ is the area of the cross-section of the conductor.
So, we can easily see that current increases with increase in drift velocity. -
Question 12 of 84
12. Question
Two resistors \(A\) and \(B\) have resistances \(R_A\) and \(R_B\) respectively with \(R_A<R_B\). The resistivities of their materials are \(\rho_A\) and \(\rho_B\).
CorrectIncorrectHint
(d) The resistance \(R\) of a conductor depends on its resistivity \(\rho\), length \(l\) and cross-sectional area \(A\). Thus,
\(
R=\rho \frac{l}{A}
\)
From the given comparison of resistances, we cannot derive the correct relation between the resistances. We also need to know the cross-sectional areas and the lengths of both the conductors before concluding about their resistivities. Only then can the relation between the resistivities be found.
The information is not sufficient to find the relation between \(\rho_A\) and \(\rho_B\). -
Question 13 of 84
13. Question
The product of resistivity and conductivity of a cylindrical conductor depends on
CorrectIncorrectHint
The relation between the resistivity \(\rho\) and conductivity \(\sigma\) of a material is given by
\(
\begin{aligned}
& \rho=\frac{1}{\sigma} \\
& \Rightarrow \rho \times \sigma=1
\end{aligned}
\)
The product of conductivity and resistivity is unity. So, this product does not depend on any of the given quantities. -
Question 14 of 84
14. Question
As the temperature of a metallic resistor is increased, the product of its resistivity and conductivity
CorrectIncorrectHint
(c) As the temperature of a metallic resistor increases, the product of its resistivity and conductivity remains constant.
Explanation: Resistivity and conductivity are inversely proportional to each other, meaning their product is always equal to 1, regardless of temperature changes.
\(
\begin{aligned}
& \rho=\frac{1}{\sigma} \\
& \Rightarrow \rho \times \sigma=1
\end{aligned}
\) -
Question 15 of 84
15. Question
In an electric circuit containing a battery, the charge (assumed positive) inside the battery
CorrectIncorrectHint
(b) In the study of electric current, the direction opposite the flow of electrons is regarded as the direction of flow of positive charge. In a battery, positive charge flows from the negative terminal to the positive terminal when it is discharging (connected to external circuit). But when the battery is charged, the positive charge flows from the positive terminal to the negative terminal.
-
Question 16 of 84
16. Question
A resistor of resistance \(R\) is connected to an ideal battery. If the value of \(R\) is decreased, the power dissipated in the resistor will
CorrectIncorrectHint
(a) As the resistance is connected to an ideal battery, it provides a constant potential difference across the two terminals.
The internal resistance of the battery is also zero.
The power dissipated in the resistor,
\(
\mathrm{P}=\frac{V^2}{R}
\)
\(V\) is constant; hence \(\mathrm{P} \propto \frac{1}{R}\)
Thus, if the value of the resistance is decreased, the power dissipated in the resistor will increase. -
Question 17 of 84
17. Question
A current passes through a resistor. Let \(K_1\) and \(K_2\) represent the average kinetic energy of the conduction electrons and the metal ions respectively.
CorrectIncorrectHint
(c) Kinetic energy ( \(K\) ) is proportional to the square of velocity ( \(v\) ) according to \(K=\frac{1}{2} m v^2\), where \(m\) is mass.
Since the velocity of conduction electrons is greater than that of the metal ions, their kinetic energy is also greater.
The average kinetic energy of conduction electrons ( \(K_1\) ) is greater than that of the metal ions ( \(\kappa_2\) ). -
Question 18 of 84
18. Question
Two resistors \(R\) and \(2 R\) are connected in series in an electric circuit. The thermal energy developed in \(R\) and \(2 R\) are in the ratio
CorrectIncorrectHint
The heat (thermal energy) developed in a resistor can be calculated using the formula:
\(
H=I^2 R T
\)
Where \(H\) is the heat developed, \(I\) is the current, \(R\) is the resistance, and \(T\) is the time for which the current flows.
For resistor \(R_1\) (which is \(R\) ):
\(
H_1=I^2 R T
\)
For resistor \(R_2\) (which is \(2 R\) ):
\(
H_2=I^2(2 R) T=2 I^2 R T
\)
\(
\begin{aligned}
&\frac{H_1}{H_2}=\frac{I^2 R T}{2 I^2 R T}\\
&\text { – Simplifying this gives: }\\
&\frac{H_1}{H_2}=\frac{1}{2}
\end{aligned}
\) -
Question 19 of 84
19. Question
Two resistances \(R\) and \(2 R\) are connected in parallel in an electric circuit. The thermal energy developed in \(R\) and \(2 R\) are in the ratio
CorrectIncorrectHint
(b) The thermal energy developed in resistor \(R\) is:
\(H_1=\frac{V^2}{R} t\)
The thermal energy developed in resistor \(2 R\) is:
\(\mathrm{H}_2=\frac{V^2}{2 R} t\)
The ratio of thermal energy developed in \(R\) and \(2 R\) is:
\(\frac{H_1}{H_2}=\frac{\frac{V^2}{R} t}{\frac{V^2}{2 R} t}\)
\(\frac{H_1}{H_2}=\frac{V^2}{R} t \cdot \frac{2 R}{V^2 t}\)
\(\frac{H_1}{H_2}=\frac{2}{1}\)
The ratio of thermal energy developed in \(R\) and \(2 R\) is \(2: 1\). -
Question 20 of 84
20. Question
A uniform wire of resistance \(50 \Omega\) is cut into 5 equal parts. These parts are now connected in parallel. The equivalent resistance of the combination is
CorrectIncorrectHint
(a) Resistance of a wire is directly proportional to its length.
So, when we cut the wire into 5 equal parts, the resistance of each part will be \(10 \Omega\).
On connecting these wires in parallel, the net resistance will be
\(
\begin{aligned}
& \frac{1}{R}=\frac{1}{10}+\frac{1}{10}+\frac{1}{10}+\frac{1}{10}+\frac{1}{10} \\
& \Rightarrow R=2 \Omega
\end{aligned}
\) -
Question 21 of 84
21. Question
Consider the following two statements:
(A) Kirchhoff’s junction law follows from conservation of charge.
(B) Kirchhoff’s loop law follows from conservative nature of electric field.CorrectIncorrectHint
(a) Kirchhoff’s junction law states that the current entering the junction is equal to the current leaving the junction, which indirectly means the charge entering the junction is equal charge leaving the junction. From the statement, it is clear that Kirchhoff’s junction law follows from the conservation of charge. Therefore, statement A is correct. Kirchhoff’s loop law states that the net potential drop in a loop is Zero \(\Sigma \mathbf{V}=\) \(\mathbf{0}\), which means potential changes around a closed loop is zero. Voltage is the measure of energy per unit charge in this context KVL can be restated as the total energy gained per unit charge must be equal to the amount of energy lost per unit charge (ie, Law of conservation of Energy). Therefore statement B is correct.
-
Question 22 of 84
22. Question
Two nonideal batteries are connected in series. Consider the following statements:
(A) The equivalent emf is larger than either of the two emfs.
(B) The equivalent internal resistance is smaller than either of the two internal resistances.CorrectIncorrectHint
(b) Let the emfs of the batteries be \(e_1\) and \(e_2\), and their respective resistances be \(r_1\) and \(r_2\).
Since the batteries are connected in series, the equivalent emf will be the sum of the emf of the two batteries ( \(\mathrm{e}=\mathrm{e}_1+\mathrm{e}_2\) ).
Thus, \(\mathrm{e}>\mathrm{e}_1\) and \(\mathrm{e}>\mathrm{e}_2\)
Thus, the equivalent emf is larger than either of the two emfs. Hence, statement A is correct.
Since the batteries are connected in series, the equivalent internal resistance (r) of the combination will be the sum of the internal resistance of the two batteries ( \(r=r_1+r_2\) ).
\(r>r_1[latex] and [latex]r>r_2\)
Thus, the equivalent internal resistance is greater that either of the two resistances. Hence, statement B is wrong. -
Question 23 of 84
23. Question
Two nonideal batteries are connected in parallel. Consider the following statements:
(A) The equivalent emf is smaller than either of the two emfs.
(B) The equivalent internal resistance is smaller than either of the two internal resistances.CorrectIncorrectHint
(c) For batteries in parallel, the equivalent emf \(\left(\epsilon_{e q}\right)\) can be calculated using the formula:
\(
\epsilon_{e q}=\frac{\epsilon_1 / r_1+\epsilon_2 / r_2}{1 / r_1+1 / r_2}
\)
Thus, the equivalent emf is greater than either of the emfs. Thus, statement A is wrong.
For the parallel combination of the batteries of internal resistance \(r_1\) and \(r_2\), the equivalent internal resistance \(r\) is given as
\(
r=\frac{r_1 r_2}{r_1+r_2}
\)
Thus, the value of the resultant resistance is even smaller than either resistance. -
Question 24 of 84
24. Question
The net resistance of an ammeter should be small to ensure that
CorrectIncorrectHint
(d) it does not appreciably change the current to be measured.
Explanation:
An ammeter is designed to measure the current flowing in a circuit. If the ammeter itself has a significant resistance, it will add to the overall resistance of the circuit, thereby reducing the current flowing through it. This means the measured current will not be the actual current flowing in the circuit without the ammeter. To minimize this interference and ensure an accurate measurement, the ammeter’s resistance should be as small as possible. -
Question 25 of 84
25. Question
The net resistance of a voltmeter should be large to ensure that
CorrectIncorrectHint
(d) It does not appreciably change the potential difference to be measured.
Explanation:
A voltmeter is designed to measure voltage without significantly affecting the circuit it’s connected to. If a voltmeter had a low resistance, it would draw a substantial current from the circuit, altering the voltage being measured. By having a very high resistance, the voltmeter draws minimal current, ensuring that the measured voltage is as close as possible to the actual voltage in the circuit. -
Question 26 of 84
26. Question
Consider a capacitor-charging circuit. Let \(Q_1\) be the charge given to the capacitor in a time interval of 10 ms and \(Q_2\) be the charge given in the next time interval of 10 ms. Let \(10 \mu \mathrm{C}\) charge be deposited in a time interval \(t_1\) and the next \(10 \mu \mathrm{C}\) charge is deposited in the next time interval \(t_2\).
CorrectIncorrectHint
(b) The charge \(Q\) on the plates of a capacitor at time \(t\) after connecting it in a charging circuit,
\(
Q=\epsilon C\left(1-e^{-t / R C}\right)
\)
where
\(
\epsilon=e m f \text { of the battery connected in the charging circuit }
\)
\(
\mathrm{C}=\text { capacitance of the given capacitor }
\)
\(
R=\text { resistance of the resistor connected in series with the capacitor }
\)
The charge developed on the plates of the capacitor in first 10 mili seconds is given by
\(
Q_1=\epsilon C\left(1-e^{-10 \times 10^{-3} / R C}\right)
\)
The charge developed on the plates of the capacitor in first 20 mili seconds is given by
\(
Q^{\prime}=\epsilon C\left(1-e^{-20 \times 10^{-3} / R C}\right)
\)
The charge developed at the plates of the capacitor in the interval \(t=10\) mili seconds to 20 mili seconds is given by
\(
\begin{aligned}
& Q_2=Q^{\prime}-Q_1 \\
& Q_2=\left[\epsilon C\left(1-e^{-20 \times 10^{-3} / R C}\right)\right]-\left[\epsilon C\left(1-e^{-10 \times 10^{-3} / R C}\right)\right] \\
& \Rightarrow Q_2=\left[\epsilon C\left(e^{-10 \times 10^{-3} / R C}-e^{-20 \times 10^{-3} / R C}\right)\right] \\
& \Rightarrow Q_2=\left[\epsilon C\left(e^{-10 \times 10^{-3} / R C}\right)\left(1-e^{-10 \times 10^{-3} / R C}\right)\right]
\end{aligned}
\)
Comparing \(\mathrm{Q}_1\) with \(\mathrm{Q}_2\)
\(
\begin{aligned}
& Q_1=\epsilon C\left(1-e^{-10 \times 10^{-3} / R C}\right), Q_2=\epsilon C e^{-10 \times 10^{-3} / R C}\left(1-e^{-10 \times 10^{-3} / R C}\right) \\
& \frac{Q_1}{Q_2}=\frac{1}{e^{-10 \times 10^{-3} / R C}} \\
& e^{-10 \times 10^{-3} / R C}<1 \\
& \therefore Q_1>Q_2
\end{aligned}
\)
For second part of the question
\(10 \mu \mathrm{C}\) charge be deposited in a time interval \(t_1\) and the next \(10 \mu \mathrm{C}\) charge is deposited in the next time interval \(\mathrm{t}_2\).
The time taken for \(10 \mu \mathrm{C}\) charge to develop on the plates of the capacitor is \(t_1\)
\(
10 \mu C=\epsilon C\left(1-e^{-t_1 / R C}\right) \dots(1)
\)
The time taken for \(20 \mu \mathrm{C}\) charge to develop on the plates of the capacitor is \(t^{\prime}\)
\(
20 \mu C=\epsilon C\left(1-e^{-t_2 / R C}\right) \dots(2)
\)
Dividing (2) by (1)
\(
\begin{aligned}
& \frac{20 \mu C}{10 \mu C}=\frac{\epsilon C\left(1-e^{-t_2 / R C}\right)}{\epsilon C\left(1-e^{-t_1 / R C}\right)} \\
& 2=\frac{\left(1-e^{-t_2 / R C}\right)}{\left(1-e^{-t_1 / R C}\right)} \\
& 2\left(1-e^{-t_1 / R C}\right)=\left(1-e^{-t_2 / R C}\right) \\
& e^{-t_2 / R C}=2 e^{-t_1 / R C}-1
\end{aligned}
\)
Taking natural log both side,
\(
\begin{aligned}
& \frac{t_2}{R C}=\ln (2)+\frac{t_1}{R C} \\
& \Rightarrow \mathrm{t}_1<\mathrm{t}_2
\end{aligned}
\) -
Question 27 of 84
27. Question
Electrons are emitted by a hot filament and are accelerated by an electric field as shown in the figure below. The two stops at the left ensure that the electron beam has a uniform cross-section.
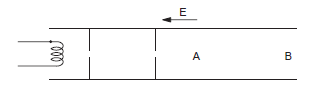 CorrectIncorrect
CorrectIncorrectHint
(a) Let the potentials at A and B be \(\mathrm{V}_{\mathrm{A}}\) and \(\mathrm{V}_{\mathrm{B}}\).
As potential,
\(
E=-\frac{d V}{d r}
\)
potential increases in the direction opposite to the direction of the electric field.
Thus, \(\mathrm{V}_{\mathrm{A}}<\mathrm{V}_{\mathrm{B}}\)
Potential energy of the electrons at points A and B :
\(
\mathrm{U}_{\mathrm{A}}=-\mathrm{eV}_{\mathrm{A}}
\)
\(
U_B=-e V_B
\)
Thus, \(\mathrm{U}_{\mathrm{A}}>\mathrm{U}_{\mathrm{B}}\)
Let the kinetic energy of an electron at points \(A\) and \(B[latex] be [latex]K_A\) and \(K_B\) respectively.
Applying the principle of conservation of mechanical energy, we get:
\(
\mathrm{U}_{\mathrm{A}}+\mathrm{K}_{\mathrm{A}}=\mathrm{U}_{\mathrm{B}}+\mathrm{K}_{\mathrm{B}}
\)
As, \(\mathrm{U}_{\mathrm{A}}>\mathrm{U}_{\mathrm{B}}\),
\(
\mathrm{K}_{\mathrm{A}}<\mathrm{K}_{\mathrm{B}}
\)
Therefore, the speed of the electrons is more at B than at A. -
Question 28 of 84
28. Question
A capacitor with no dielectric is connected to a battery at \(t=0\). Consider a point \(A\) in the connecting wires and a point \(B\) in between the plates.
CorrectIncorrectHint
(b, c) As the capacitor is connected to the battery at \(t=0\), current flows through the wire up to the time the capacitor is charged. As the capacitor is completely charged, the potential difference across the capacitor is equal to the terminal potential of the battery. So, the current stops flowing and does not pass through the point between the plates of the capacitor, as there is no medium for the flow of charge.
-
Question 29 of 84
29. Question
When no current is passed through a conductor,
CorrectIncorrectHint
(c, d) When no current passes through a conductor, free electrons do not move in a net direction. While they are constantly moving randomly due to thermal energy, there is no overall movement of electrons in a particular direction. When no current is passed through a conductor, the average of the velocities of all the free electrons at an instant is zero.
-
Question 30 of 84
30. Question
Which of the following quantities do not change when a resistor connected to a battery is heated due to the current?
CorrectIncorrectHint
(d) Resistance, resistivity and drift velocity varies with relaxation time which is dependent on temperature Number of free electrons in a conductor remain in variant even if temperature is changes.
-
Question 31 of 84
31. Question
As the temperature of a conductor increases, its resistivity and conductivity change. The ratio of resistivity to conductivity
CorrectIncorrectHint
(a) Resistivity \((\rho)\) of a conductor is the reciprocal of the its conductivity \((\sigma)\). Thus,
\(
\rho=\frac{1}{\sigma}
\)
The ratio of the resistivity and the conductivity,
\(
\frac{\rho}{\sigma}=\frac{\rho}{1 / \rho}=\rho^2
\)
As resistivity increases with temperature, the square of resistivity will also increase. Hence, the ratio of resistivity and conductivity will increase with increase in temperature. -
Question 32 of 84
32. Question
A current passes through a wire of nonuniform cross-section. Which of the following quantities are independent of the cross section?
CorrectIncorrectHint
(a, d) Drift speed and current density are inversely proportional to the area of cross-section of a wire. Thus, they are dependent on the cross-section. The charge crossing in a given time interval is independent of the area of cross-section of the wire. Free electron density is the total number of free electrons per unit volume of the wire. The density of free electrons depends on the distribution of the free electrons throughout the volume of the wire. It does not depend on the cross-section of the wire.
Note: Drift speed is the average speed of electrons in a conductor. It is related to the current density and the cross-sectional area through the equation \(\mathrm{J}=nev_d\) (where J is current density, n is free-electron density, e is electron charge, and \({v}_{d}\) is drift speed). Since current density depends on cross-section area, drift speed also depends on it.
-
Question 33 of 84
33. Question
Mark out the correct options.
CorrectIncorrectHint
(a, d)
The ammeter is connected in series in the circuit whose current is to be measured. If the net resistance of the ammeter is high, then the amount of current that the circuit draws will have error . So, we won’t be able to accurately measure the amount of current that the circuit draws from the voltage source. Thus, it should have a small resistance.
To measure the potential difference across any circuit element, the voltmeter is connected in parallel to that circuit element. If the resistance of the voltmeter is large, then maximum voltage drop occurs across the voltmeter and it will measure the correct value of the potential.
-
Question 34 of 84
34. Question
A capacitor of capacitance \(500 \mu \mathrm{~F}\) is connected to a battery through a \(10 \mathrm{k} \Omega\) resistor. The charge stored on the capacitor in the first 5 s is larger than the charge stored in the next
CorrectIncorrectHint
The charge \((Q)\) on the capacitor at any instant \(t\).
\(
Q=C V\left(1-e^{-t / R C}\right)
\)
where
C = capacitance of the given capacitance
\(R=\) resistance of the resistor connected in series with the capacitor
\(
\mathrm{RC}=\left(10 \times 10^3\right) \times\left(500 \times 10^{-6}\right)=5 \mathrm{~s}
\)
The charge on the capacitor in the first 5 seconds.
\(
Q_0=C V\left(1-e^{-5 / 5}\right)=C V \times 0.632
\)
The charge on the capacitor in the first 10 seconds.
\(
\begin{aligned}
& Q_1=C V\left(1-e^{-10 / 5}\right) \\
& Q_1=C V\left(1-e^{-2}\right)=0.864 \times C V
\end{aligned}
\)
Charge developed in the next 5 seconds.
\(
\begin{aligned}
& Q^{\prime}=Q_1-Q_0 \\
& Q^{\prime}=C V(0.864-0.632)=0.232 \mathrm{CV}
\end{aligned}
\)
The charge on the capacitor in the first 55 seconds.
\(
\begin{aligned}
& Q_2=C V\left(1-e^{-55 / 5}\right) \\
& Q_2=C V\left(1-e^{-11}\right)=0.99 \times C V
\end{aligned}
\)
Charge developed in the next 50 seconds.
\(
\begin{aligned}
& Q^{\prime}=Q_2-Q_0 \\
& Q^{\prime}=C V(0.99-0.632)=0.358 C V
\end{aligned}
\)
Charge developed in the first 505 seconds.
\(
Q_3=C V\left(1-e^{-500 / 5}\right)=C V\left(1-e^{-100}\right) \approx C V
\)
Charge developed in the next 500 seconds.
\(
Q^{\prime}=C V(1-0.632)=0.368 \mathrm{CV}
\)
Thus, the charge developed on the capacitor in the first 5 seconds is greater than the charge developed in the next \(5,50,500, 5000\) seconds. -
Question 35 of 84
35. Question
A capacitor \(C_1\) of capacitance \(1 \mu \mathrm{~F}\) and a capacitor \(C_2\) of capacitance \(2 \mu \mathrm{~F}\) are separately charged by a common battery for a long time. The two capacitors are then separately discharged through equal resistors. Both the discharge circuits are connected at \(t=0\).
CorrectIncorrectHint
(b, d) Let the voltage of the battery connected to the capacitors be V. Both the capacitors will charge up to the same potential (V).
The charge on the capacitors \(\mathrm{C}_1[latex] is [latex]\mathrm{C}_1 \mathrm{~V}=(1 \mu \mathrm{~F}) \times \mathrm{V}\)
The charge on the capacitors \(\mathrm{C}_2[latex] is [latex]\mathrm{C}_2 \mathrm{~V}=(2 \mu \mathrm{~F}) \times \mathrm{V}\)
The charge on the discharging circuit at an instant \(t\),
\(
Q=C V e^{-t / R C}
\)
The current through the discharging circuit,
\(
\frac{d Q}{d t}=-\frac{C V}{R C} e^{-t / R C}=\frac{V}{R} e^{-t / R C}
\)
At \(\mathrm{t}=0\), the current through the discharging circuit will be \(\frac{V}{R}\) for both the capacitors.
Let the time taken by the capacitor \(C_1\) to lose \(50 \%\) of the charge be \(t_1\).
\(
\begin{aligned}
& Q_1=\frac{C_1 V}{2} \\
& \frac{C_1 V}{2}=C_1 V e^{-t_1 / R C} \\
& \frac{1}{2}=e^{-t_1 / R C}
\end{aligned}
\)
Taking natural log on both sides, we get:
\(
\begin{aligned}
& -\ln 2=-\frac{t_1}{R C_1} \\
& t_1=R C_1 \ln 2
\end{aligned}
\)
Similarly,
Time taken for capacitor \(\mathrm{C}_2\):
\(
t_2=R C_2 \ln 2
\)
As, \(\mathrm{C}_1<\mathrm{C}_2, \mathrm{t}_1<\mathrm{t}_2\)
Thus, we can say that \(C_1\) loses \(50 \%\) of its initial charge sooner than \(C_2\) loses \(50 \%\) of its initial charge. -
Question 36 of 84
36. Question
The current through a wire depends on time as \(I=3 t^2+2 t+5\). The charge flowing through the cross-section of the wire in time interval between \(t=0\) to \(t=2 \mathrm{~s}\) is
CorrectIncorrectHint
(a) Current,
\(
\begin{aligned}
& I=\frac{d q}{d t}=3 t^2+2 t+5 \\
& q=\int_{t=0}^{t=2}\left(3 t^2+2 t+5\right) d t
\end{aligned}
\)
Charge, \(q=\left[t^3+t^2+5 t\right]_0^2=[8+4+10]=22 \mathrm{C}\) -
Question 37 of 84
37. Question
In a closed circuit, the current \(I\) (in ampere) at an instant of time \(t\) (in second) is given by \(I=4-0.08 t\). The number of electrons flowing in 50 s through the cross-section of the conductor is
CorrectIncorrectHint
\(
\begin{aligned}
&\text { Given, }\\
&\begin{aligned}
I & =4-0.08 t \\
\frac{d q}{d t} & =4-0.08 t \\
q & =\int_0^{50}(4-0.08 t) d t \\
n e & =\left[4 t-\frac{0.08 t^2}{2}\right]_0^{50}=100 \\
n & =\frac{100}{e}=\frac{100}{1.6 \times 10^{-19}}=6.25 \times 10^{20} \text { electrons }
\end{aligned}
\end{aligned}
\) -
Question 38 of 84
38. Question
Drift velocity \(v_d\) varies with the intensity of electric field as per the relation,
CorrectIncorrectHint
\(
\begin{aligned}
&\text { (a) Drift velocity, } v_d=\frac{e E \tau}{m}\\
&\therefore \quad v_d \propto E
\end{aligned}
\) -
Question 39 of 84
39. Question
The number density of free electron in a copper conductor is \(8.5 \times 10^{28} \mathrm{~m}^{-3}\). How long does an electron take to drift from one end of a wire, 3.0 m long to its other end? The area of cross-section of the wire is \(2.0 \times 10^{-6} \mathrm{~m}^2\) and it is carrying a current of 3.0 A.
CorrectIncorrectHint
\(
\begin{aligned}
&\text { (a) Drift velocity, }\\
&\begin{aligned}
& v_d=\frac{i}{n e A}=\frac{3}{8.5 \times 10^{28} \times 1.6 \times 10^{-19} \times 2 \times 10^{-6}} \\
& =0.11 \times 10^{-3} \mathrm{~ms}^{-1} \\
& \therefore \text { Time, } t=\frac{\text { length of the wire }}{v_d}=\frac{3}{0.11 \times 10^{-3}}=2.73 \times 10^4 \mathrm{~s}
\end{aligned}
\end{aligned}
\) -
Question 40 of 84
40. Question
Find the equivalent resistance between \(A\) and \(B\).
 CorrectIncorrect
CorrectIncorrectHint
(a) The points connected by a conducting wire are at same potential. Then, redraw the diagram by placing the points of same potential at one place and then solve for equivalent resistance.

Hence, from the new figure \(A\) and \(Y\) are at same potential; \(B\) and \(X\) are at same potential.
\(
\begin{aligned}
\Rightarrow \quad \frac{1}{R_{\text {eq }}} & =\frac{1}{6 R}+\frac{1}{9 R}+\frac{1}{12 R}=\frac{13}{36 R} \\
R_{\text {eq }} & =\frac{36 R}{13}=2.77 R
\end{aligned}
\) -
Question 41 of 84
41. Question
Find the equivalent resistance between \(P\) and \(Q\).
 CorrectIncorrect
CorrectIncorrectHint
(d) It can be seen that, this diagram is symmetrical about \(P Q\), so points on the perpendicular bisector of \(P Q\), i.e. \(X, Y\) and \(Z\) are at same potential. So, in this type of diagrams, to calculate the equivalent resistance, we can remove the resistances at the same potential, i.e. the resistances between \(X\) and \(Y, Y\) and \(Z\), are redundant and can be removed.
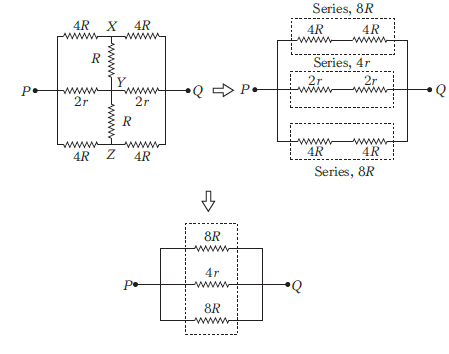
\(
\begin{aligned}
&\text { All resistances in the circuit are in parallel, }\\
&\begin{aligned}
& \frac{1}{R_{\text {eq }}}=\frac{1}{8 R}+\frac{1}{4 r}+\frac{1}{8 R}=\frac{R+r}{4 R r} \\
& R_{\text {eq }}=\frac{4 R r}{R+r} \Omega
\end{aligned}
\end{aligned}
\) -
Question 42 of 84
42. Question
Find the equivalent resistance between \(A\) and \(B\).
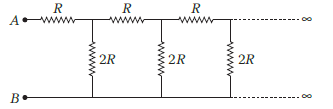 CorrectIncorrect
CorrectIncorrectHint
(a) Here, we have infinite pairs of \(R\) and \(2 R\). Suppose, the equivalent resistance is \(R_0\) between \(C\) and \(D\), i.e. excluding one pair near \(A B\) (since, pairs are infinite, equivalent resistance will remain same, if we include pair near \(A B\) ).

\(
\begin{gathered}
R_{\text {eq }}=R_0=R+\frac{2 R R_0}{2 R+R_0} \\
\left(R_0-R\right)\left(2 R+R_0\right)=2 R R_0 \\
2 R R_0-2 R^2+R_0^2-R R_0=2 R R_0 \\
R_0^2-R R_0-2 R^2=0 \\
R_0=\frac{R \pm \sqrt{R^2+8 R^2}}{2}=\frac{R \pm 3 R}{2}=2 R \text { or }-R
\end{gathered}
\)
Equivalent resistance between \(A\) and \(B=2 R(\because\) equivalent resistance cannot be negative). -
Question 43 of 84
43. Question
If \(e, \tau\) and \(m\) respectively, represent the electron density, relaxation time and mass of the electron, then the resistance \(R\) of a wire of length \(l\) and area of cross-section \(A\) will be
CorrectIncorrectHint
\(
\begin{aligned}
&\text { (a) Resistance, } R=\rho \frac{l}{A} \text { and resistivity, } \rho=\frac{m}{n e^2 \tau}\\
&\therefore \quad R=\frac{m l}{n e^2 \tau A}
\end{aligned}
\) -
Question 44 of 84
44. Question
Four wires are made of the same material and are at the same temperature. Which one of them has highest electrical resistance?
CorrectIncorrectHint
(a) The resistance \(R\) of a wire is given by \(R=\rho \frac{l}{A}\), where \(\rho\) is the resistivity of the material, \(l\) is the length, and \(A\) is the cross-sectional area.
The area of a circle is given by \(A=\pi r^2=\pi\left(\frac{d}{2}\right)^2=\frac{\pi d^2}{4}\), where \(r\) is the radius and \(d\) is the diameter.
Since, wires are made of same material, so resistivity \(\rho\) of all wires will be same.
Therefore, \(R \propto \frac{l}{A}\)
\(
\begin{aligned}
\Rightarrow \quad & R \propto \frac{l}{\pi r^2} \\
& R \propto \frac{l}{\left(\frac{d}{2}\right)^2}
\end{aligned}
\)
For length \(=50 \mathrm{~cm}\) and diameter \(=0.5 \mathrm{~mm}\)
Resistance will be maximum. -
Question 45 of 84
45. Question
The potential difference between points \(A\) and \(B\) of the following figure is
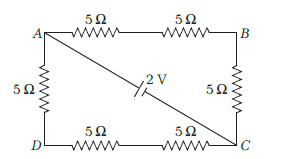 CorrectIncorrect
CorrectIncorrectHint
(c) Given, circuit can be redrawn as follows
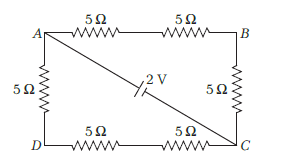
Here, \(I_1=\frac{I}{2}=\frac{V}{2 R_{\text {eq }}}=\frac{2}{2 \times\left(\frac{15}{2}\right)}=\frac{2}{15} \mathrm{~A}\)
So, potential across each resistance,
\(
V^{\prime}=I_1 R=\frac{2}{15} \times 5=\frac{2}{3} \mathrm{~V}
\)
\(\therefore[latex] Potential difference across,
[latex]
\mathrm{AB}=\frac{2}{3}+\frac{2}{3}=\frac{4}{3} \mathrm{~V}
\) -
Question 46 of 84
46. Question
In the figure given below, the current passing through \(6 \Omega\) resistor is
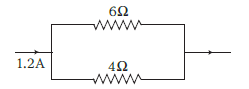 CorrectIncorrect
CorrectIncorrectHint
(b)
\(
\text { From current division rule, } i_1=i\left(\frac{R_2}{R_1+R_2}\right)
\)
\(
=1.2\left(\frac{4}{6+4}\right)=1.2\left(\frac{4}{10}\right)=0.48 \mathrm{~A}
\) -
Question 47 of 84
47. Question
Current \(i\) as shown in the circuit will be
 CorrectIncorrect
CorrectIncorrectHint
(b) Two resistances are short circuited.
So, only third resistance will be considered and hence,
\(
i=V / R=10 / 3 \mathrm{~A}
\) -
Question 48 of 84
48. Question
In the circuit shown, the point \(B\) is earthed. The potential at the point \(A\) is
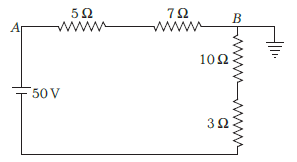 CorrectIncorrect
CorrectIncorrectHint
(b) As \(B\) is connected to the earth, so potential at \(B\) is \(V_B=0\).
Now, current in the given circuit,
\(
i=\frac{V}{R_{\text {net }}}=\frac{50}{5+7+10+3}=2 \mathrm{~A}
\)
Potential difference between \(A\) and \(B\) is
\(
\begin{aligned}
V_A-V_B & =2 \times 12 \\
V_A-0 & =24 \\
V_A & =24 \mathrm{~V}
\end{aligned}
\) -
Question 49 of 84
49. Question
A current of 2 A passes through a cell of emf 1.5 V having internal resistance of \(0.15 \Omega\). The potential difference measured in volt, across both the ends of the cell will be
CorrectIncorrectHint
\(
\begin{aligned}
&\text { (d) We have, } V=E \text { – ir }\\
&\begin{aligned}
& =1.5-2 \times 0.15 \\
V & =1.20 \mathrm{~V}
\end{aligned}
\end{aligned}
\) -
Question 50 of 84
50. Question
If \(V_{A B}\) is 4 V in the given figure, then resistance \(X\) will be
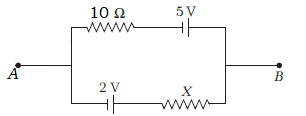 CorrectIncorrect
CorrectIncorrectHint
\(
\begin{aligned}
&\text { (d) Potential difference between } A \text { and } B \text { is given by }\\
&\begin{aligned}
V_A-V_B & =\frac{E_1 r_2+E_2 r_1}{r_1+r_2} \\
4 & =\frac{5 X+2 \times 10}{X+10} \\
X & =20 \Omega
\end{aligned}
\end{aligned}
\) -
Question 51 of 84
51. Question
Two batteries of emf 4 V and 8 V with internal resistances \(1 \Omega\) and \(2 \Omega\) are connected in a circuit with a resistance of \(9 \Omega\) as shown in figure. The current and potential difference between the points \(P\) and \(Q\) are
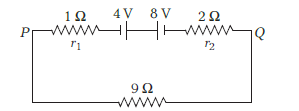 CorrectIncorrect
CorrectIncorrectHint
(a)

\(
E_1=4 \mathrm{~V} \text { and } E_2=8 \mathrm{~V}
\)
As, \(E_2>E_1\), so current flows from \(Q\) to \(P\).
\(
\therefore \quad i=\frac{8-4}{12}=\frac{1}{3} \mathrm{~A}
\)
\(\therefore\) Potential difference across \(P Q=\frac{1}{3} \times 9=3 \mathrm{~V}\) -
Question 52 of 84
52. Question
The current flowing through \(5 \Omega\) resistance is
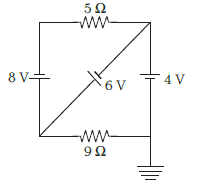 CorrectIncorrect
CorrectIncorrectHint
(d) The potentials of different points are as shown in figure below

\(
\begin{aligned}
&\text { Current through } 5 \Omega \text { resistance }\\
&\begin{aligned}
& =\frac{\text { Potential difference }}{\text { Resistance }} \\
& =\frac{(4-2)}{5}=0.4 \mathrm{~A}
\end{aligned}
\end{aligned}
\) -
Question 53 of 84
53. Question
The figure shows a network of currents. The current \(i\) will be
 CorrectIncorrect
CorrectIncorrectHint
(c)
\(
\begin{aligned}
&\text { Applying Kirchhoff’s first law (current law) at junction } A, B, C, D\\
&\begin{array}{lc}
\text { At } A, & i_{A B}=15+8=23 \mathrm{~A} \\
\text { At } B, & i_{B C}=23+3=26 \mathrm{~A} \\
\text { At } D, & i_{C D}=8-5=3 \mathrm{~A} \\
\text { At } C, & i_{C D}+i=i_{B C} \\
\text { or } & 3+i=26 \\
\therefore & i=23 \mathrm{~A}
\end{array}
\end{aligned}
\) -
Question 54 of 84
54. Question
In the circuit element given here, if the potential difference at point \(B, V_B=0\), then the potential difference between \(A\) and \(D\) are
 CorrectIncorrect
CorrectIncorrectHint
(d) Potential difference between \(A\) and \(B\),
\(
\begin{aligned}
& & V_A-V_B & =1 \times 1.5 \text { or } V_A-0=1.5 \quad \left(\because V_B=0, \text { given }\right)\\
\therefore & & V_A & =1.5 \mathrm{~V}
\end{aligned}
\)
Now, potential difference between \(B\) and \(C\),
\(
\begin{array}{llrl}
& & V_B-V_C & =1 \times 2.5=2.5 \mathrm{~V} \\
\therefore & & 0-V_C & =2.5 \mathrm{~V} \\
\Rightarrow & & V_C & =-2.5 \mathrm{~V}
\end{array}
\)
Hence, potential difference between \(C\) and \(D\),
\(
V_C-V_D=-2 \mathrm{~V} \text { or }-2.5-V_D=-2 \text { or } V_D=-0.5 \mathrm{~V}
\) -
Question 55 of 84
55. Question
Three resistances are connected to form a \(T\)-shape as shown in the figure. Then, the current \(i\) in the \(2 \Omega\) resistor is
 CorrectIncorrect
CorrectIncorrectHint
\(
\text { (b) Let at junction } C \text {, potential is } V \text {. }
\)
\(
\begin{aligned}
& \frac{V_A-V_C}{2}+\frac{V_B-V_C}{4}=\frac{V_C-0}{2} \\
& \frac{20-V}{2}+\frac{5-V}{4}=\frac{V-0}{2} \\
& V=9 \mathrm{~V}
\end{aligned}
\)
\(
\text { Current, } i_3=i=\frac{9}{2}=4.5 \mathrm{~A}
\) -
Question 56 of 84
56. Question
How much work is required to carry a \(6 \mu \mathrm{C}\) charge from the negative terminal to the positive terminal of a 9 V battery?
CorrectIncorrectHint
\(
\text { (b) Work done, } W=q V=6 \times 10^{-6} \times 9=54 \times 10^{-6} \mathrm{~J}
\) -
Question 57 of 84
57. Question
Two resistors \(R\) and \(2 R\) are connected in series in an electric circuit. The thermal energy developed in \(R\) and \(2 R\) are in the ratio
CorrectIncorrectHint
\(
\begin{aligned}
&\text { (a) In series, } i \text { is same, so } H=i^2 R t \text {. Therefore, } H \propto R \text {. }\\
&\begin{array}{ll}
\therefore & \frac{H_1}{H_2}=\frac{R}{2 R}=\frac{1}{2} \\
\Rightarrow & H_1: H_2=1: 2
\end{array}
\end{aligned}
\) -
Question 58 of 84
58. Question
The resistor of resistance \(R\) is connected to 25 V supply and heat produced in it is \(25 \mathrm{Js}^{-1}\). The value of \(R\) is
CorrectIncorrectHint
(c) The heat produced by a resistor is given by the formula \(H=\frac{V^2}{R}\), where \(V\) is the voltage and \(R\) is the resistance.
\(
25=\frac{25^2}{R}
\)
\(
R=25 \Omega
\) -
Question 59 of 84
59. Question
Just as electricity is supplied at 220 V for domestic use in India, it is supplied at 110 V in USA. If the resistance of 60 W bulb for use in India is \(R\), then that of 60 W bulb for use in USA will be
CorrectIncorrectHint
\(
\begin{aligned}
&\text { (a) Resistance, } R=\frac{V^2}{P} \text { or } R \propto V^2\\
&\Rightarrow \frac{R_1}{R_2}=\frac{V_1^2}{V_2^2} \Rightarrow \frac{R}{R_2}=\left(\frac{220}{110}\right)^2=4 \Rightarrow R_2=\frac{R}{4}
\end{aligned}
\) -
Question 60 of 84
60. Question
If \(R_1\) and \(R_2\) are respectively, the filament resistances of 200 W bulb and 100 W bulb designed to operate on the same voltage, then
CorrectIncorrectHint
(b) Power, \(P=\frac{V^2}{R}\)
\(
\therefore \quad P \propto \frac{1}{R}
\)
So,
\(
\begin{aligned}
\frac{P_1}{P_2} & =\frac{R_2}{R_1} \\
\frac{200}{100} & =\frac{R_2}{R_1} \\
R_2 & =2 R_1
\end{aligned}
\) -
Question 61 of 84
61. Question
The electric bulb have tungsten filaments of same length. If one of them gives 60 W and other 100 W, then
CorrectIncorrectHint
(a) Power, \(P=\frac{V^2}{R}\)
\(
\therefore \quad P \propto \frac{1}{R}
\)
Also, resistance of wire \(\propto \frac{1}{(\text { radius of filament })^2}\)
\(\therefore P \propto(\text { radius of filament })^2\)
So, 100 W bulb has thicker filament. -
Question 62 of 84
62. Question
How many calories of heat will be produced approximately in 210 W electric bulb in 5 min?
CorrectIncorrectHint
\(
\text { (d) } H=\frac{V^2 t}{R \times J}=\frac{P t}{J}=\frac{210 \times 5 \times 60}{4.2}=15000 \mathrm{cal}
\)Note: \(1 \text { calorie = } 4.2 \text { joules. }\)
-
Question 63 of 84
63. Question
A wire when connected to 220 V mains supply has power dissipation \(P_1\). Now, the wire is cut into two equal pieces, which are connected in parallel to the same supply. Power dissipation in this case is \(P_{2}\). Then, \(P_2: P_1\) is
CorrectIncorrectHint
\(
\begin{aligned}
& \text { (b) } R=\frac{R}{4} \text { and } P=\frac{V^2}{R} \\
& \therefore \quad P_2=4 P_1 \Rightarrow \frac{P_2}{P_1}=4
\end{aligned}
\) -
Question 64 of 84
64. Question
Two electric bulbs, one of \(200 \mathrm{~V}-40 \mathrm{~W}\) and other \(200 \mathrm{~V}-100\) W are connected in series to a 200 V line, then the potential drop across
CorrectIncorrectHint
(c) \(R_{40}>R_{100}\). In series, potential difference distributes in direct ratio of resistance.
-
Question 65 of 84
65. Question
Three identical bulbs are connected in series and these together dissipate a power \(P\). Now, if the bulbs are connected in parallel, then the power dissipated will be
CorrectIncorrectHint
(c) When bulbs are in series, \(P=\frac{V^2}{3 R} \dots(i)\)
When bulbs are connected in parallel,
\(
\begin{aligned}
P^{\prime} & =\frac{V^2}{(R / 3)}=\frac{3 V^2}{R}=3 \times 3 P \quad \text { [from Eq. (i)] } \\
& =9 P
\end{aligned}
\) -
Question 66 of 84
66. Question
\(A\) and \(B\) are two bulbs connected in parallel. If \(A\) is glowing brighter than \(B\), then the relation between \(R_A\) and \(R_B\) is
CorrectIncorrectHint
(b) It is known that in parallel combination,
\(
P_{\text {consumed }} \propto \text { Brigntness } \propto \frac{1}{R}
\)
According to question, \(P_A>P_B\) (given), therefore \(R_B>R_A\) -
Question 67 of 84
67. Question
Some electric bulbs are connected in series across a 220 V supply in a room. If one bulb is fused, then remaining bulbs are connected again in series (after removing the fused bulb) across the same supply. The illumination in the room will
CorrectIncorrectHint
\(
\text { (a) } P=\frac{V^2}{R} \text {. As, } R_{\text {net }} \text { will decrease, so } P \text { will increase. }
\) -
Question 68 of 84
68. Question
Electric bulbs of \(50 \mathrm{~W}-100 \mathrm{~V}\) glowing at full power are to be used in parallel with battery \(120 \mathrm{~V}, 10 \Omega\). Maximum number of bulbs that can be connected, so that they glow in full power is
CorrectIncorrectHint
(c) When each bulb is glowing at full power.
Current from each bulb, \(i=\frac{50}{100}=\frac{1}{2} \mathrm{~A}\)
So, main current, \(i=\frac{n}{2} \mathrm{~A}\) (for parallel circuit)
\(
\text { Also, } \begin{aligned}
E & =V+i r \\
120 & =100+\left(\frac{n}{2}\right) \times 10 \\
n & =4
\end{aligned}
\) -
Question 69 of 84
69. Question
In the circuit shown, \(A\) and \(V\) are ideal ammeter and voltmeter, respectively. Reading of the voltmeter will be
 CorrectIncorrect
CorrectIncorrectHint
(d) Ammeter is parallel with voltmeter, therefore its reading will be zero.
Explanation: An ideal ammeter has zero resistance and an ideal voltmeter has infinite resistance.
How to solve?
Since the voltmeter is connected across the ammeter and the ammeter has zero resistance, there will be no voltage drop across the ammeter.
Step 1: Since an ideal ammeter has zero resistance, there will be no voltage drop across it.
Step 2: The voltmeter reads the voltage across the ammeter, which is zero.The reading of the voltmeter will be zero.
-
Question 70 of 84
70. Question
The net resistance of a voltmeter should be large to ensure that
CorrectIncorrectHint
(d) It does not appreciably change the potential difference to be measured.
Explanation:
A voltmeter is designed to measure voltage without significantly affecting the circuit it’s connected to. If a voltmeter had a low resistance, it would draw a substantial current from the circuit, altering the voltage being measured. This would give an inaccurate reading. By having a high resistance, the voltmeter draws very little current, ensuring that the measured voltage is essentially the same as the original voltage in the circuit. -
Question 71 of 84
71. Question
Two galvanometers \(A\) and \(B\) require 3 mA and 6 mA, respectively, to produce the same deflection of 10 divisions. Then,
CorrectIncorrectHint
(a) Sensitivity of a galvanometer is inversely proportional to the current required for a given deflection.
Higher current means lower sensitivity (more current is needed to produce the same deflection).How to solve?
Compare the currents required for the same deflection to determine which galvanometer is more sensitive.Step 1: Calculate the sensitivity of Galvanometer \(A\)
Sensitivity, \(S_A\), is inversely proportional to the current, \(I_A\) :
\(S_A \propto \frac{1}{I_A}\)
Sensitivity \(S_A=\frac{1}{3 \mathrm{~mA}}\)Step 2: Calculate the sensitivity of Galvanometer B
Sensitivity, \(S_B\), is inversely proportional to the current, \(I_B\) :
\(S_B \propto \frac{1}{I_B}\)
Sensitivity \(S_B=\frac{1}{6 \mathrm{~mA}}\)Step 3: Compare the sensitivities
Compare \(S_A\) and \(S_B\) :
\(\frac{S_A}{S_B}=\frac{\frac{1}{3 \mathrm{~mA}}}{\frac{1}{6 \mathrm{~mA}}}=\frac{6}{3}=2\)
Therefore, \(S_A=2 S_B\)Step 4: Determine which galvanometer is more sensitive
Since \(S_A=2 S_{B^{\prime}}\) Galvanometer \(A\) is more sensitive than Galvanometer \(B\).
Galvanometer \(A\) is more sensitive than Galvanometer \(B\). -
Question 72 of 84
72. Question
An ammeter \(A\), a voltmeter \(V\) and a resistance \(R\) are connected as shown in the figure. If the voltmeter reading is 1.6 V and the ammeter reading is 0.4 A, then \(R\) is
 CorrectIncorrect
CorrectIncorrectHint
(b) If the voltmeter is ideal, then \(R\) should be \(\frac{1.6}{0.4}=4 \Omega\). If it is non-ideal, \(R\) should be greater than \(4 \Omega\).
-
Question 73 of 84
73. Question
In the following circuit, the emf of the cell is 2 V and the internal resistance is negligible. The resistance of the voltmeter is \(80 \Omega\). The reading of the voltmeter will be
 CorrectIncorrect
CorrectIncorrectHint
(c) Total resistance of given circuit
\(
=\frac{80}{1+1}+20=40+20=60 \Omega
\)
\(\therefore\) Main current, \(i=\frac{2}{60}=\frac{1}{30} \mathrm{~A}\)
Now, in parallel, there are two resistances of \(80 \Omega\) each (one of voltmeter and other \(80 \Omega\) resistance). So, current is equally distributed in \(80 \Omega\) resistance and voltmeter, i.e. \(\frac{1}{60} \mathrm{~A}\) of current flows through each.
\(\therefore\) Potential difference across \(80 \Omega\) resistance or voltmeter reading \(=\frac{1}{60} \times 80=1.33 \mathrm{~V}\) -
Question 74 of 84
74. Question
What is the reading of voltmeter in the figure?
 CorrectIncorrect
CorrectIncorrectHint
(d) Equivalent resistance of circuit,
\(
R_{\mathrm{eq}}=500+\frac{1000}{3}=\frac{2500}{3} \Omega
\)
\(\therefore\) Current drawn from the cell,
\(
\begin{gathered}
i=\frac{10}{2500 / 3}=\frac{3}{250} \mathrm{~A} \\
\text { Reading of voltmeter }=\frac{3}{250} \times \frac{1000}{3}=4 \mathrm{~V}
\end{gathered}
\) -
Question 75 of 84
75. Question
A galvanometer of \(25 \Omega\) and having full scale deflection for a current of 10 mA is changed into voltmeter of range 100 V by connecting a resistance \(R\) in series with the galvanometer. The resistance \(R\) (in ohm) is
CorrectIncorrectHint
(d) \(V=I R\)
\(
100=\left(10 \times 10^{-3}\right)(25+R)
\)
\(\therefore\) Resistance, \(R=9975 \Omega\) -
Question 76 of 84
76. Question
An ammeter and a voltmeter are joined in series to a cell. Their readings are \(A\) and \(V\), respectively. If a resistance is now joined in parallel with the voltmeter, then
CorrectIncorrectHint
(d) When some resistance is connected in parallel with voltmeter the effective resistance get decreased. So, \(A\) will increase and \(V\) will decrease.
-
Question 77 of 84
77. Question
In the circuit shown in the figure, the voltmeter reading is
 CorrectIncorrect
CorrectIncorrectHint
(d) From the definition of voltmeter \(R\) of \(V\) is very high \(R \simeq \infty\). So entire 6 V will appear across the voltmater.
-
Question 78 of 84
78. Question
To send \(10 \%\) of the main current through a moving coil galvanometer of resistance \(99 \Omega\), the shunt required is
CorrectIncorrectHint
(c) We have, \(i_g G=\left(i-i_g\right) S\)
\(
\begin{aligned}
10 \times 99 & =(90) S \\
S & =\frac{10 \times 99}{90}
\end{aligned}
\)
\(\therefore\) Shunt, \(S=11 \Omega\) -
Question 79 of 84
79. Question
The potential difference across the \(100 \Omega\) resistance in the circuit is measured by a voltmeter of \(900 \Omega\) resistance. The percentage error made in reading the potential difference is
 CorrectIncorrect
CorrectIncorrectHint
(c) Before connecting the voltmeter, let the potential difference across \(100 \Omega\) is \(V_1\).
\(
\begin{aligned}
&\therefore \quad V_1=\left(\frac{100}{100+10}\right) \times V=\frac{10}{11} V\\
&\text { After connecting the voltmeter across } 100 \Omega \text {, }
\end{aligned}
\)
\(
\begin{aligned}
&\text { Equivalent resistance }=\frac{100 \times 900}{100+900}=90 \Omega\\
&\text { Let this time potential difference is } V_2 \text {. }\\
&\therefore \quad V_2=\left(\frac{90}{90+10}\right) V=\frac{9}{10} V
\end{aligned}
\)
\(
\text { Magnitude of \% error }=\frac{\frac{10}{11} V-\frac{9}{10} V}{\frac{10}{11} V} \times 100=1.0
\) -
Question 80 of 84
80. Question
A microammeter has a resistance of \(100 \Omega\) and a full scale range of \(50 \mu \mathrm{~A}\). It can be used as a voltmeter or as a higher range ammeter provided a resistance is added to it. Pick the correct range and resistance combinations.
CorrectIncorrectHint
(b) How to solve?
Calculate the series resistance for voltmeter ranges using Ohm’s law.
Calculate the parallel resistance for ammeter ranges using the current divider rule.
Compare the calculated resistances with the given options.Step 1: Calculate the series resistance for the 50 V range
Use Ohm’s law to find the total resistance:
\(R_t=\frac{V}{I_m}=\frac{50 \mathrm{~V}}{50 \times 10^{-6} \mathrm{~A}}=10^6 \Omega\)Calculate the series resistance:
\(R_s=R_t-R_m=10^6 \Omega-100 \Omega=999900 \Omega \approx 10^6 \Omega=1000 \mathrm{k} \Omega\)Step 2: Calculate the series resistance for the 10 V range
Use Ohm’s law to find the total resistance:
\(R_t=\frac{V}{I_m}=\frac{10 \mathrm{~V}}{50 \times 10^{-6} \mathrm{~A}}=2 \times 10^5 \Omega\)Calculate the series resistance:
\(R_s=R_t-R_m=2 \times 10^5 \Omega-100 \Omega=199900 \Omega \approx 200 \mathrm{k} \Omega\)Step 3: Calculate the parallel resistance for the 10 mA range
Use the current divider rule:
\(I_m R_m=\left(I-I_m\right) R_p\)
\(R_p=\frac{I_m R_m}{I-I_m}=\frac{50 \times 10^{-6} \mathrm{~A} \times 100 \Omega}{10 \times 10^{-3} \mathrm{~A}-50 \times 10^{-6} \mathrm{~A}}=\frac{5 \times 10^{-3} \Omega}{9.95 \times 10^{-3}} \approx 0.5025 \Omega \approx 0.5 \Omega\)Step 4: Compare the calculated values with the given options
Option (a): 50 V range with \(10 \mathrm{k} \Omega\) resistance in series is incorrect.
Option (b): 10 V range with \(200 \mathrm{k} \Omega\) resistance in series is correct.
Option (c): 10 mA range with \(1 \Omega\) resistance in parallel is incorrect.
The correct range and resistance combination is option (b). -
Question 81 of 84
81. Question
The percentage error in measuring resistance with a meter bridge can be minimised by adjusting the balancing point close to
CorrectIncorrectHint
(b) What’s given in the problem
A meter bridge is used to measure resistance.
The meter bridge works on the principle of the Wheatstone bridge.
The percentage error is minimized when the balancing point is near the middle of the wire.How to solve?
Choose the balancing point closest to the middle of the meter bridge wire.Step 1: Identify the optimal balancing point
The optimal balancing point is at \(\frac{100}{2} \mathrm{~cm}=50 \mathrm{~cm}\).Step 2: Choose the closest option
The option closest to 50 cm is 50 cm.The percentage error is minimized when the balancing point is close to 50 cm.
-
Question 82 of 84
82. Question
When an additional resistance of \(1980 \Omega\) is connected in series with a voltmeter, then the scale division reads 100 times larger value. Resistance of the voltmeter is
CorrectIncorrectHint
\(
\begin{aligned}
& (b)\left(i_g R_V\right)(100)=i_g\left(R_V+1980\right) \\
& \therefore \text { Resistance, } R_V=20 \Omega
\end{aligned}
\) -
Question 83 of 84
83. Question
In the given circuit, it is observed that the current \(I\) is independent of the value of the resistance \(R_6\). Then, the resis tance values must satisfy
 CorrectIncorrect
CorrectIncorrectHint
(c) \(\frac{R_3}{R_4}=\frac{R_1}{R_2}\) (balanced Wheatstone bridge)
\(
R_1 R_4=R_2 R_3
\) -
Question 84 of 84
84. Question
\(A B\) is a wire of uniform resistance. The galvanometer \(G\) shows no current when the length \(A C=20 \mathrm{~cm}\) and \(C B=80 \mathrm{~cm}\). The resistance \(R\) is equal to
 CorrectIncorrect
CorrectIncorrectHint
(c) The galvanometer shows no current, it means this is a balanced Wheatstone bridge, so
\(
\frac{R}{80}=\frac{A C}{B C}=\frac{20}{80}
\)
\(\therefore\) Resistance, \(R=20 \Omega\)