NCERT Exemplar Q & A
Quiz Summary
0 of 106 Questions completed
Questions:
Information
You have already completed the quiz before. Hence you can not start it again.
Quiz is loading…
You must sign in or sign up to start the quiz.
You must first complete the following:
Results
Results
0 of 106 Questions answered correctly
Your time:
Time has elapsed
You have reached 0 of 0 point(s), (0)
Earned Point(s): 0 of 0, (0)
0 Essay(s) Pending (Possible Point(s): 0)
Categories
- Not categorized 0%
- 1
- 2
- 3
- 4
- 5
- 6
- 7
- 8
- 9
- 10
- 11
- 12
- 13
- 14
- 15
- 16
- 17
- 18
- 19
- 20
- 21
- 22
- 23
- 24
- 25
- 26
- 27
- 28
- 29
- 30
- 31
- 32
- 33
- 34
- 35
- 36
- 37
- 38
- 39
- 40
- 41
- 42
- 43
- 44
- 45
- 46
- 47
- 48
- 49
- 50
- 51
- 52
- 53
- 54
- 55
- 56
- 57
- 58
- 59
- 60
- 61
- 62
- 63
- 64
- 65
- 66
- 67
- 68
- 69
- 70
- 71
- 72
- 73
- 74
- 75
- 76
- 77
- 78
- 79
- 80
- 81
- 82
- 83
- 84
- 85
- 86
- 87
- 88
- 89
- 90
- 91
- 92
- 93
- 94
- 95
- 96
- 97
- 98
- 99
- 100
- 101
- 102
- 103
- 104
- 105
- 106
- Current
- Review
- Answered
- Correct
- Incorrect
-
Question 1 of 106
1. Question
A capacitor of \(4 \mu \mathrm{~F}\) is connected as shown in the circuit (Fig. 2.1). The internal resistance of the battery is \(0.5 \Omega\). The amount of charge on the capacitor plates will be
 CorrectIncorrect
CorrectIncorrectHint
(d) Key concept: A capacitor offers zero resistance in a circuit when it is uncharged, i.e., it can be assumed as short circuited and it offers infinite resistance when it is fully charged.

At steady state the capacitor offers infinite resistance in DC circuit and acts as open circuit as shown in figure, therefore no current flows through the capacitor and \(10 \Omega\) resistance, leaving zero potential difference across \(10 \Omega\) resistance. Hence potential difference across capacitor will be the potential difference across A and B .
The potential difference across lower and middle branch of circuit is equal to the potential difference across capacitor of upper branch of circuit.
Current flows through \(2 \Omega\) resistance from left to right, is given by \(I=\mathrm{V} / (\mathrm{R}+\mathrm{r})=1 \mathrm{~A}\). The potential difference across \(2 \Omega\) resistance, \(\mathrm{V}=\mathrm{IR}=1 \times 2=2 \mathrm{~V}\) Hence potential difference across capacitor is also 2 V. The charge on capacitor is \(\mathrm{q}=\mathrm{CV}=(2 \mu \mathrm{~F}) \times 2 \mathrm{~V}=8 \mu \mathrm{C}\). -
Question 2 of 106
2. Question
A positively charged particle is released from rest in an uniform electric field. The electric potential energy of the charge
CorrectIncorrectHint
(c) Key concept: Electric potential decreases in the direction of electric field. The direction of electric field is always perpendicular to one equipotential surface maintained at high electrostatic potential to other equipotential surface maintained at low electrostatic potential.
The positively charged particle experiences electrostatic force along the direction of electric field, hence moves in the direction of electric field. Thus, positive work is done by the electric field on the charge. We know
\(
W_{\text {electrical }}=-\Delta U=-q \Delta V=q\left(V_{\text {initial }}-V_{\text {final }}\right)
\)
Hence electrostatic potential energy of the positive charge decreases. -
Question 3 of 106
3. Question
Figure 2.2 shows some equipotential lines distributed in space. A charged object is moved from point \(A\) to point \(B\).
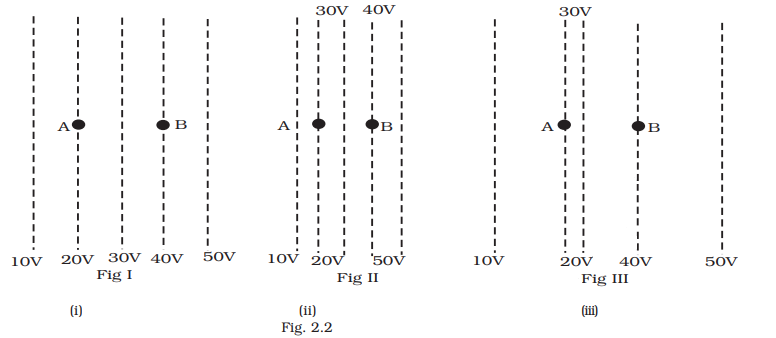 CorrectIncorrect
CorrectIncorrectHint
(c) As the direction of electric field is always perpendicular to one equipotential surface maintained at high electrostatic potential than other equipotential surface maintained at low electrostatic potential. Hence direction of electric field is from \(B\) to \(A\) in all three cases.
The positively charged particle experiences electrostatic force along the direction of electric field, hence moves in the direction opposite to electric field. Thus, the work done by the electric field on the charge will be negative. We know
\(
W_{\text {electrical }}=-\Delta U=-q \Delta V=q\left(V_{\text {initial }}-V_{\text {final }}\right)
\)
Here initial and final potentials are same in all three cases and same charge is moved, so work done is same in all three cases. -
Question 4 of 106
4. Question
The electrostatic potential on the surface of a charged conducting sphere is 100 V . Two statments are made in this regard:
\(\mathrm{S}_1\) : At any point inside the sphere, electric intensity is zero.
\(\mathrm{S}_2\) : At any point inside the sphere, the electrostatic potential is 100V.
Which of the following is a correct statement?CorrectIncorrectHint
(c) We know, the electric field intensity E and electric potential V are related
\(\mathrm{E}=\mathrm{dV} / \mathrm{dr}\)
If electric field intensity \(\mathrm{E}=0\), then \(\mathrm{dV} / \mathrm{dr}=0\). It means, \(\mathrm{E}=0\) inside the charged conducting sphere causes uniform potential inside the sphere. Hence uniform electrostatic potential 100 V will be at any point inside the sphere.
Important points:The electric field zero does not necessary imply that electric potential is zero. E.g., the electric field intensity at any point inside the charged spherical shell is zero but there may exist non-zero electric potential.
If two charged particles of same magnitude but opposite sign are placed, the electric potential at the midpoint will be zero but electric field is not equal to zero. -
Question 5 of 106
5. Question
Equipotentials at a great distance from a collection of charges whose total sum is not zero are approximately
CorrectIncorrectHint
(a) The collection of charges, whose total sum is not zero, with regard to great distance can be considered as a single point charge. The equipotential surfaces due to a point charge are spherical.
Important point:
The electric potential due to point charge \(q\) is given by \(V=q / 4 \pi \epsilon_0 r\)
It means electric potential due to point charge is same for all equidistant points. The locus of these equidistant points, which are at same potential, form spherical surface. -
Question 6 of 106
6. Question
A parallel plate capacitor is made of two dielectric blocks in series. One of the blocks has thickness \(d_1\) and dielectric constant \(k_1\) and the other has thickness \(d_2\) and dielectric constant \(k_2\) as shown in Fig. 2.3. This arrangement can be thought as a dielectric slab of thickness \(d\left(=d_1+d_2\right)\) and effective dielectric constant \(k\). The \(k\) is
 CorrectIncorrect
CorrectIncorrectHint
(c) Here the system can be considered as two capacitors \(\mathrm{C}_1\) and \(\mathrm{C}_2\) connected in series as shown in figure.

The capacitance of parallel plate capacitor filled with dielectric block has thickness \(d_1\) and dielectric constant \(\mathrm{K}_2\) is given by
\(
\begin{aligned}
& \frac{1}{C_{\mathrm{eq}}}=\frac{1}{C_1}+\frac{1}{C_2} \Rightarrow C_{\mathrm{eq}}=\frac{C_1 C_2}{C_1+C_2} \\
& C_{\mathrm{eq}}=\frac{\frac{K_1 \varepsilon_0 A}{d_1}}{\frac{K_1 \varepsilon_0 A}{d_1}+\frac{K_2 \varepsilon_0 A}{d_2}} \frac{K_2 \varepsilon_0 A}{d_2}=\frac{K_1 K_2 \varepsilon_0 A}{K_1 d_2+K_2 d_1} \dots(i)
\end{aligned}
\)
We can write the equivalent capacitance as
\(
C=\frac{K \varepsilon_0 A}{d_1+d_2} \dots(ii)
\)
On comparing (i) and (ii) we have
\(
K=\frac{K_1 K_2\left(d_1+d_2\right)}{K_1 d_2+K_2 d_1}
\) -
Question 7 of 106
7. Question
Consider a uniform electric field in the \(\hat{\mathbf{z}}\) direction. The potential is a constant
CorrectIncorrectHint
(b c, d) We know, the electric field intensity E and electric potential V are
\(
E=-\frac{d V}{d r}
\)
Electric potential decreases inf the direction of electric field. The direction of electric field is always perpendicular to one equipotential surface maintained at high electrostatic potential to other equipotential surface maintained at low electrostatic potential.
The electric field in z-direction suggest that equipotential surfaces are in \(x-y\) plane. Therefore the potential is a constant for any x for a given z , for any y for a given z and on the \(x-y\) plane for a given z . -
Question 8 of 106
8. Question
Equipotential surfaces
CorrectIncorrectHint
(a, b, c) The density of the equipotential lines gives an idea about the magnitude of electric field. Higher the density, larger the field strength.
We know, the electric field intensity E and electric potential V are related as a, b and c
We know that on any two points of equipotential surface, potential difference is zero or of equal potential.
\(
\because E=\frac{-d V}{d r}
\)
So the electric field intensity is inversely proportional to the separation between equipotential surfaces.
So equipotential surfaces are closer in regions of large electric. Thus, it verifies answer a
The electric field is larger near the sharp edge, due to larger charge density as a is very small
\(
\because \sigma=\frac{q}{A}
\)
So equipotential surfaces are closer or crowded. It verifies answer \(b\).
As the electric field \(E=\frac{k q}{r^2}\) and potential or field decreases as size of the body increases or vice-versa (case of the earth), so the equipotential surfaces will be more crowded if the charge density \(\sigma=\frac{q}{A}\) increases. It verifies the answer c .
As the equipotential surface depends on distance r by \(E=\frac{-d V}{r}\) and \(V=\frac{k q}{r}\). Equipotential surface depends on charge density at that place which is different at a different place, so equipotential surface are not equispaced all over.
Hence the electric field intensity E is inversely proportional to the separation between equipotential surfaces. So, equipotential surfaces are closer in regions of large electric fields. As electric field intensities is large near sharp edges of charged conductor and near regions of large charge densities. Therefore, equipotential surfaces are closer at such places. -
Question 9 of 106
9. Question
The work done to move a charge along an equipotential from A to B
CorrectIncorrectHint
(b, c) Key concept: The work done by the external agent in shifting the test charge along the dashed line from 1 to 2 is
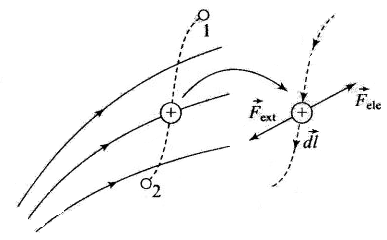
The external agent does a work \(W=-q \int_1^2 \vec{E} \cdot d \vec{l}\) in transporting the test charge \(q\) slowly from the positions 1 to 2 in the static electric field.
\(
W_{\mathrm{ext}}=\int_1^2 \vec{F}_{\mathrm{ext}} \cdot \overrightarrow{d l}=\int_1^2(-q \vec{E}) \cdot \overrightarrow{d l}=-q \int_1^2 \vec{E} \cdot \overrightarrow{d l}
\)
We know \(V_A-V_B=-\int_A^B \vec{E} \cdot \overrightarrow{d l} \dots(i)\)
\(W_{\text {electrical }}=-\Delta U=-q \Delta V=q\left(V_A-V_B\right) \dots(ii)\)
Hence from (i) and (ii), \(W_{\text {electrical }}=q\left(V_A-V_B\right)=-q \int_A^B \vec{E} \cdot \overrightarrow{d l}\)
If we want to calculate the work done to move a charge along an equipotential from \(A\) to \(B\),
For equipotential surface \(V_{A}=V_B\), hence \(W=0\).
Also electric field is perpendicular to equipotential surface, hence
\(
\vec{E} \cdot \overrightarrow{d l} \Rightarrow W_{\text {electrical }}=0
\) -
Question 10 of 106
10. Question
In a region of constant potential
CorrectIncorrectHint
(b, c) We know, the electric field intensity E and electric potential V are dV
related as \(E=-d V / d r\)
or we can write \(|E|=\Delta V / \Delta r\)
The electric field intensity E and electric potential V are related as \(\mathrm{E}=0\) and for \(\mathrm{V}=\mathrm{constant} \mathrm{dV} / \mathrm{dr}=0,\), this imply that electric field intensity \(\mathrm{E}=0\).
If some charge is present inside the region then electric field cannot be zero at that region, for this \(\mathrm{V}=\) constant is not valid. -
Question 11 of 106
11. Question
In the circuit shown in Fig. 2.4. initially key \(\mathrm{K}_1\) is closed and key \(K_2\) is open. Then \(K_1\) is opened and \(K_2\) is closed (order is important). [Take \(\mathrm{Q}_1{ }^{\prime}\) and \(\mathrm{Q}_2{ }^{\prime}\) as charges on \(\mathrm{C}_1\) and \(\mathrm{C}_2\) and \(V_1\) and \(V_2\) as voltage respectively.]

Then
CorrectIncorrectHint
(a, d) Initially key \(K_1\) is closed and key \(K_2\) is open, the capacitor \(\mathrm{C}_1\) is charged by battery and capacitor \(\mathrm{C}_2\) is still uncharged. Now \(\mathrm{K}_1\) is opened and \(\mathrm{K}_2\) is closed, the capacitors \(\mathrm{C}_1\) and \(\mathrm{C}_2\) both are connected in parallel. The charge stored by capacitor \(\mathrm{C}_1\), gets redistributed between \(\mathrm{C}_1\) and \(\mathrm{C}_2\) till their potentials become same, i.e., \(\mathrm{V}_2=\mathrm{V}_1\).
By law of conservation of charge, the charge stored in capacitor Cx is equal to sum of charges on capacitors \(\mathrm{C}_1\) and \(\mathrm{C}_2\) when \(\mathrm{K}_1\) is opened and \(\mathrm{K}_2\) is closed, i.e.,
\(
Q_1^{\prime}+Q_2^{\prime}=Q
\) -
Question 12 of 106
12. Question
If a conductor has a potential \(V \neq 0\) and there are no charges anywhere else outside, then
CorrectIncorrectHint
(a, b) The potential of a body is due to charge of the body and due to the charge of surrounding. If tfiere are no charges anywhere else outside, then the potential of the body will be due to its own charge. If there is a cavity inside a conducting body, then charge can be placed inside the body. Hence there must be charges on its surface or inside itself. Hence option (a) is correct. The charge resides on the outer surface of a closed charged conductor. Hence there cannot be any charge in the body of the conductor. Hence option (b) is correct.
-
Question 13 of 106
13. Question
A parallel plate capacitor is connected to a battery as shown in Fig. 2.5. Consider two situations:
A: Key K is kept closed and plates of capacitors are moved apart using insulating handle.
B: Key K is opened and plates of capacitors are moved apart using insulating handle.
Choose the correct option(s). CorrectIncorrect
CorrectIncorrectHint
(c, d) The battery maintains the potential difference across connected capacitor in every circumstance. However, charge stored by disconnected charged capacitor remains conserved.
Case A: When key K is kept closed and plates of capacitors are moved apart using insulating handle. The battery maintains the potential difference across connected capacitor in every circumstance. The separation between two plates increases which in turn decreases its capacitance ( \(C=\epsilon_0 A / d\) )and potential difference across
connected capacitor continue to be the same as capacitor is still connected with battery. Hence, the charge stored decreases as \(\mathrm{Q}=\mathrm{CV}\).
Case B: When key K is opened and plates of capacitors are moved apart using insulating handle. The charge stored by isolated charged capacitor remains conserved. The separation between two plates is increasing which in turn decreases its capacitance with the decrease of capacitance, potential difference V increases as \(V=Q / C\). -
Question 14 of 106
14. Question
A capacitor of capacitance \(C\) is charged to a potential \(V\). The flux of the electric field through a closed surface enclosing the capacitor is
CorrectIncorrectHint
(d) The capacitor has charges \(+Q\) and \(-Q\) on its plates.
The total charge enclosed is the sum of these charges:
\(Q_{\text {enc }}=+Q+(-Q)=0\)
The electric flux \(\Phi_E\) through the closed surface is given by:
\(\Phi_E=\frac{Q_{\text {enc }}}{\varepsilon_0}\)
Substitute \(Q_{\text {enc }}=0\) :
\(\Phi_E=\frac{0}{\varepsilon_0}=0\)
The electric flux through the closed surface is zero. -
Question 15 of 106
15. Question
Two capacitors, each having capacitance \(C\) and breakdown voltage \(V\) are joined in series. The capacitance and the breakdown voltage of the combination will be
CorrectIncorrectHint
(d) The formula for equivalent capacitance in series is:
\(\frac{1}{C_{e q}}=\frac{1}{C_1}+\frac{1}{C_2}\)
Since \(C_1=C_2=C\) :
\(\frac{1}{C_{e q}}=\frac{1}{C}+\frac{1}{C}=\frac{2}{C}\)
Therefore:
\(C_{e q}=\frac{C}{2}\)
The breakdown voltage for capacitors in series is the sum of individual breakdown voltages:
\(V_{e q}=V_1+V_2\)
Since \(V_1=V_2=V\) :
\(V_{e q}=V+V=2 V\)
The equivalent capacitance is \(\frac{C}{2}\) and the breakdown voltage is \(2 V\). -
Question 16 of 106
16. Question
If the capacitors in the previous question are joined in parallel, the capacitance and the breakdown voltage of the combination will be
CorrectIncorrectHint
The equivalent capacitance of capacitors in parallel is the sum of their individual capacitances.
\(
\begin{aligned}
& C_{e q}=C+C \\
& C_{e q}=2 C
\end{aligned}
\)
In a parallel connection, the voltage across each capacitor is the same and is equal to the applied voltage \(V\). Therefore, the breakdown voltage of the combination remains the same as that of each capacitor:
\(
V_{\text {breakdown }}=V
\)
The equivalent capacitance is \(2 C\) and the breakdown voltage is \(V\). -
Question 17 of 106
17. Question
The equivalent capacitance of the combination shown in the figure below is
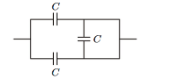 CorrectIncorrect
CorrectIncorrectHint
(b) Two capacitors are in parallel combination.
Hence equivalent capacitance \(C_{\text {eq }}=C_1+C_2=C+C=2 C\) -
Question 18 of 106
18. Question
A dielectric slab is inserted between the plates of an isolated capacitor. The force between the plates will
CorrectIncorrectHint
(c) Step 1: An isolated capacitor has a fixed charge \((Q)\) on its plates. The force between the plates of a capacitor can be expressed using the formula:
\(
F=\frac{Q^2}{2 A \epsilon_0}
\)
where:
\(F\) is the force between the plates,
\(Q\) is the charge on the plates,
\(A\) is the area of the plates,
\(\epsilon_0\) is the permittivity of free space.
Step 2: Insert the Dielectric Slab
When a dielectric slab is inserted between the plates of the capacitor, it affects the electric field between the plates. However, since the capacitor is isolated, the charge \((Q)\) on the plates remains constant.
Step 3: Analyze the Effect of the Dielectric
The presence of the dielectric slab increases the capacitance of the capacitor. The new capacitance \(C^{\prime}\) can be expressed as:
\(
C^{\prime}=K \cdot C
\)
where \(K\) is the dielectric constant of the material and \(C\) is the original capacitance without the dielectric.
Step 4: Determine the New Force
Even though the capacitance increases, the charge remains constant. The force between the plates can still be expressed using the original formula:
\(
F=\frac{Q^2}{2 A \epsilon_0}
\)
Since \(Q[latex] and [latex]A\) are constant and \(\epsilon_0\) does not change, the force remains unchanged.
Thus, the force between the plates of an isolated capacitor with a dielectric slab inserted remains the same. -
Question 19 of 106
19. Question
The energy density in the electric field created by a point charge falls off with the distance from the point charge as
CorrectIncorrectHint
(d) The electric field due to a point charge is given by \(E=\frac{k Q}{r^2}\).
The energy density of an electric field is given by \(u=\frac{1}{2} \epsilon_0 E^2\).
The energy density is:
\(u=\frac{1}{2} \epsilon_0 E^2\)
The electric field of a point charge is:
\(E=\frac{k Q}{r^2}\)
Substitute \(E\) into the energy density equation:
\(u=\frac{1}{2} \epsilon_0\left(\frac{k Q}{r^2}\right)^2\)
\(
u=\frac{1}{2} \epsilon_0 \frac{k^2 Q^2}{r^4}
\)
Since \(\frac{1}{2} \epsilon_0 k^2 Q^2\) is constant, the energy density is proportional to \(\frac{1}{r^4}\) :
\(u \propto \frac{1}{r^4}\) -
Question 20 of 106
20. Question
A parallel-plate capacitor has plates of unequal area. The larger plate is connected to the positive terminal of the battery and the smaller plate to its negative terminal. Let \(Q_{+}\)and \(Q_{-}\)be the charges appearing on the positive and negative plates respectively.
CorrectIncorrectHint
(b) Step 1: We have a parallel-plate capacitor with two plates of unequal area. The larger plate is connected to the positive terminal of a battery, while the smaller plate is connected to the negative terminal.
Hint: Visualize the capacitor setup and identify which plate is connected to which terminal of the battery.
Step 2: Identify the Charges
When a capacitor is connected to a battery, the plate connected to the positive terminal accumulates a positive charge (\((Q+)\)) and the plate connected to the negative terminal accumulates a negative charge (\((Q-)\)).
Hint: Remember that the convention is that the positive charge appears on the plate connected to the positive terminal.
Step 3: Relate Charge to Voltage
The relationship between the charge \((Q)\) on the plates of a capacitor and the voltage \((\mathrm{V})\) across the capacitor is given by the equation:
\(
Q=C \cdot V
[latex]
where C is the capacitance of the capacitor.
Hint: Capacitance (C) is a property of the capacitor and does not depend on the area of the plates in this context.
Step 4: Analyze the Capacitance
For a parallel-plate capacitor, the capacitance is given by:
[latex]
C=\frac{\varepsilon_0 \cdot A}{d}
\)
where \(\varepsilon_0\) is the permittivity of free space, \(\boldsymbol{A}\) is the area of the plates, and \(d\) is the distance between the plates. However, this does not affect the relationship between the charges on the plates.
Hint: Focus on the fact that the capacitance relates to the geometry of the plates but does not change the fundamental charge relationship.
Step 5: Conclude the Relationship Between Charges Since the charges on the plates of a capacitor are equal in magnitude but opposite in sign, we can state:
\(
|Q+|=|Q-|
\)
This means that the positive charge on the larger plate \((Q+)\) is equal in magnitude to the negative charge on the smaller plate (\((Q-)\)).
Hint: Remember that in a capacitor, the total charge is conserved, leading to the conclusion that the magnitudes of the charges are equal.
Thus, we conclude that:
\(
Q+=Q-
\) -
Question 21 of 106
21. Question
A thin metal plate \(P\) is inserted between the plates of a parallel-plate capacitor of capacitance \(C\) in such a way that its edges touch the two plates (figure below). The capacitance now becomes
 CorrectIncorrect
CorrectIncorrectHint
(d) The capacitance of a parallel plate capacitor is:
\(C=\frac{\epsilon_0 A}{d}\)
where:
\(C\) is the capacitance,
\(\epsilon_0\) is the permittivity of free space,
\(A\) is the area of one of the plates,
\(d\) is the distance between the plates.
When the metal plate touches both plates, the distance between the plates effectively becomes zero.
\(d=0\)
Substituting \(d=0\) into the capacitance formula:
\(C^{\prime}=\frac{\epsilon_0 A}{0}\)
\(C^{\prime}=\infty\)
However, in a real circuit, this results in a short circuit.
The capacitor discharges instantly.
The capacitance is undefined or indeterminate. -
Question 22 of 106
22. Question
Figure (below) shows two capacitors connected in series and joined to a battery. The graph shows the variation in potential as one moves from left to right on the branch containing the capacitors.
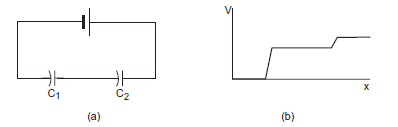 CorrectIncorrect
CorrectIncorrectHint
(c)
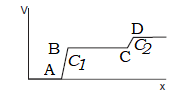
Region AB shows the potential difference across capacitor \(C_1\) and region CD shows the potential difference across capacitor \(C_2\). Now, we can see from the graph that region \(A B\) is greater than region CD. Therefore, the potential difference across capacitor \(C_1\) is greater than that across capacitor \(C_2\). \(\because\) Capacitance, \(\mathrm{C}=\frac{Q}{V}\)
\(\therefore \mathrm{C}_1<\mathrm{C}_2 \quad\) ( \(Q\) remains the same in series connection) -
Question 23 of 106
23. Question
Two metal plates having charges \(Q,-Q\) face each other at some separation and are dipped into an oil tank. If the oil is pumped out, the electric field between the plates will
CorrectIncorrectHint
(a) increase.
Explanation:
When the plates are dipped in oil, the oil acts as a dielectric material between the plates. This dielectric material reduces the electric field between the plates compared to the air. The electric field is inversely proportional to the dielectric constant of the material between the plates. Since the dielectric constant of oil is higher than that of air, the electric field decreases when the plates are dipped in oil.Oil between the plates of the capacitor acts as a dielectric. We know that the electric field decreases by a factor of \(\frac{1}{K}\) of the original field when we insert a dielectric between the plates of a capacitor (\(K\) is the dielectric constant of the dielectric). So, if the oil is pumped out, the electric field between the plates will increase, as the dielectric has been removed.
-
Question 24 of 106
24. Question
Two metal spheres of capacitances \(C_1\) and \(C_2\) carry some charges. They are put in contact and then separated. The final charges \(Q_1\) and \(Q_2\) on them will satisfy
CorrectIncorrectHint
(b) When the spheres are connected, charges flow between them until they both acquire the same common potential \(V\).
The final charges on the spheres are given by
\(
\begin{aligned}
& \mathrm{Q}_1=\mathrm{C}_1 \mathrm{~V} \text { and } \mathrm{Q}_2=\mathrm{C}_2 \mathrm{~V} \\
& \therefore \frac{Q_1}{Q_2}=\frac{C_1 V}{C_2 V}=\frac{C_1}{C_2}
\end{aligned}
\) -
Question 25 of 106
25. Question
Three capacitors of capacitances \(6 \mu \mathrm{~F}\) each are available. The minimum and maximum capacitances, which may be obtained, are
CorrectIncorrectHint
(d) The formula for the equivalent capacitance of capacitors in series is:
\(\frac{1}{C_s}=\frac{1}{C_1}+\frac{1}{C_2}+\frac{1}{C_3}\)
Since all capacitors are equal, \(C_1=C_2=C_3=C=6 \mu \mathrm{~F}\) :
\(\frac{1}{C_s}=\frac{1}{6}+\frac{1}{6}+\frac{1}{6}=\frac{3}{6}=\frac{1}{2}\)
Therefore, the equivalent capacitance in series is:
\(C_s=2 \mu \mathrm{~F}\)
The formula for the equivalent capacitance of capacitors in parallel is:
\(C_p=C_1+C_2+C_3\)
Since all capacitors are equal, \(C_1=C_2=C_3=C=6 \mu \mathrm{~F}\) :
\(C_p=6+6+6=18 \mu \mathrm{~F}\) -
Question 26 of 106
26. Question
The capacitance of a capacitor does not depend on
CorrectIncorrectHint
(c) The capacitance of a capacitor is given by \(C=\frac{\epsilon_0 A}{d}\)
Here, \(A\) is the area of the plates of the capacitor and \(d\) is the distance between the plates.
So, we can clearly see that the capacitance of a capacitor does depend on the size and shape of the plates and the separation between the plates; it does not depend on the charges on the plates. -
Question 27 of 106
27. Question
A dielectric slab is inserted between the plates of an isolated charged capacitor. Which of the following quantities will remain the same?
CorrectIncorrectHint
(b) When a dielectric slab is inserted between the plates of an isolated charged capacitor, the charge on the capacitor remains the same.
Explanation:
We know, conservation of charge states that total electric charge in an isolated system never changes. Here, the capacitor is kept isolated from the surroundings, hence charge cannot change by any means. So, the total charge of the system will be still the same as before inserting the dielectric slab.
Capacitance increases:
The capacitance of the capacitor increases when a dielectric is added (capacitor increases \(K\) times). This means that for the same amount of charge, the potential difference across the plates can decrease.Electric field decreases:
When we insert a dielectric between the plates of a capacitor, induced charges of opposite polarity appear on the face of the dielectric. They build an electric field inside the dielectric, directed opposite to the original field of the capacitor. Thus, the net effect is a reduced electric field. The electric field potential difference of capacitor reduce to \(\frac{1}{K}[latex] times.Potential difference decreases:
We know that the potential difference of a parallel plate charged capacitor is given by [latex]V=\frac{Q d}{\varepsilon A}\), where \(A\) is the area of the each parallel plate.
Since, total charge, the distance between the plates \(d\) and area \(A\) is constant then only changing the dielectric constant will change the potential difference.
Hence, inserting the dielectric slab will change the potential difference between the plates.Stored energy decreases:
The stored energy in the capacitor decreases because the potential difference decreases, even though the charge remains the same.
We know that the electrostatic energy stored in a parallel plate capacitor with charge \(Q[latex] is given by, [latex]E=\frac{1}{2} C V^2\), where \(V\) is the potential difference between the plates and \(C\) is the capacitance. Since, the capacitance is given by \(C=\frac{\varepsilon A}{d}\),changes with changing the dielectric constant. Also the potential difference between the plates changes, if we put the value of these two we get the energy term as,
\(
E=\frac{1}{2} \frac{\varepsilon A}{d}\left(\frac{Q d}{\varepsilon A}\right)^2
\)
\(\Rightarrow E=\frac{1}{2} \frac{Q^2 d}{\varepsilon A}\), Which changes with the change in the dielectric constant.Note: Here K is the dielectric constant of dielectric.
-
Question 28 of 106
28. Question
A dielectric slab is inserted between the plates of a capacitor. The charge on the capacitor is \(Q\) and the magnitude of the induced charge on each surface of the dielectric is \(Q^{\prime}\).
CorrectIncorrectHint
(d) We have a parallel plate capacitor with charge \(Q\) on one plate and \(-Q\) on the other plate.
When a dielectric slab with dielectric constant \(K\) is inserted, it affects the electric field between the plates.
Electric Field Without Dielectric:
The electric field \(E_0\) in the absence of the dielectric is given by:
\(
E_0=\frac{Q}{A \epsilon_0}
\)
where \(A\) is the area of the plates and \(\epsilon_0\) is the permittivity of free space.
Electric Field With Dielectric:
When the dielectric is inserted, the electric field \(E\) becomes:
\(
E=\frac{Q}{A \epsilon_0 K}
\)
This is because the dielectric reduces the electric field by a factor of \(K\).
Induced Charge on the Dielectric:
The dielectric slab will have induced charges on its surfaces due to the electric field. The charge induced on the positive surface of the dielectric is \(Q^{\prime}\).
Relation Between Charges:
The induced charge \(Q^{\prime}\) can be related to the electric field due to polarization. The electric field due to the induced charge is:
\(
E_p=\frac{Q^{\prime}}{A \epsilon_0}
\)
The net electric field \(E\) between the plates can be expressed as:
\(
E=E_0-E_p
\)
Setting Up the Equation:
Substituting the expressions for \(E_0[latex] and [latex]E_p\) :
\(
\frac{Q}{A \epsilon_0 K}=\frac{Q}{A \epsilon_0}-\frac{Q^{\prime}}{A \epsilon_0}
\)
Multiplying through by \(A \epsilon_0\) to eliminate the denominators:
\(
\frac{Q}{K}=Q-Q^{\prime}
\)
Solving for Induced Charge \(Q^{\prime}\) :
Rearranging the equation gives:
\(
Q^{\prime}=Q-\frac{Q}{K}
\)
Factoring out \(Q\) :
\(
Q^{\prime}=Q\left(1-\frac{1}{K}\right)
\)
Analyzing the Result:
Since \(K>1\) (the dielectric constant is always greater than 1 ), the term \(\left(1-\frac{1}{K}\right)\) is positive but less than 1. Therefore:
\(
Q^{\prime}<Q
\) -
Question 29 of 106
29. Question
Each plate of a parallel plate capacitor has a charge \(q\) on it. The capacitor is now connected to a battery. Now,
CorrectIncorrectHint
(a, c, d) Each plate of a parallel plate capacitor has a charge \(q\) on it (before the battery is connected). The capacitor is now connected to a battery, which will add \(q{\prime}\) charge on +ve side of the plate. So total charge becomes \(q+q{\prime}\). Similarly, on the other plate it will be \(q-q{\prime}\). That means the total charge on the two plates are not equal.
-
Question 30 of 106
30. Question
The separation between the plates of a charged parallel-plate capacitor is increased. Which of the following quantities will change?
CorrectIncorrectHint
(b, c) Because the charge always remains conserved in an isolated system, it will remain the same.
Charge and potential difference:
Since \(Q=C V\) (where \(Q\) is charge, \(C\) is capacitance, and \(V\) is potential difference), if the capacitance decreases while the charge remains constant (because the capacitor is disconnected from the battery), then the potential difference must increase to maintain the same charge.Energy:
The energy stored in a capacitor is given by \(U=\frac{1}{2} C V^2\). Since capacitance decreases and potential difference increases when the plates are separated, the energy stored in the capacitor also increases. -
Question 31 of 106
31. Question
A parallel-plate capacitor is connected to a battery. A metal sheet of negligible thickness is placed between the plates. The sheet remains parallel to the plates of the capacitor.
CorrectIncorrectHint
(d) Step 1: Understand the Configuration
We have a parallel-plate capacitor connected to a battery. The capacitor has two plates separated by a distance \(d\). A metal sheet of negligible thickness is inserted between the plates, remaining parallel to them.
Hint: Visualize the capacitor and the metal sheet to understand how they interact.
Step 2: Analyze the Effect of the Metal Sheet
When a metal sheet is placed between the plates of the capacitor, it will affect the electric field and the charge distribution. The metal sheet will induce charges on its surfaces due to the electric field between the capacitor plates.
Hint: Remember that conductors in an electric field will always have charges induced on their surfaces.
Step 3: Charge Induction on the Metal Sheet
The metal sheet will have equal and opposite charges induced on its two faces. The face of the metal sheet that is closer to the positive plate of the capacitor will acquire a negative charge, while the face closer to the negative plate will acquire a positive charge.
Hint: Think about how charges redistribute themselves in response to an electric field.
Step 4: Effect on Capacitance
The introduction of the metal sheet does not change the potential difference across the capacitor plates because the battery maintains a constant voltage. The capacitance \(C\) of a capacitor is given by the formula:
\(
C=\frac{Q}{V}
\)
Since the potential difference \(V\) remains constant and the charge \(Q\) does not change, the capacitance \(C\) also remains
unchanged.
Hint: Recall that capacitance depends on the physical configuration and the dielectric properties of the materials between the plates.
Step 5: Effect on Charge
Since the battery is connected, it will maintain the same potential difference across the capacitor. Therefore, the total charge \(Q\) on the capacitor remains constant. The presence of the metal sheet does not allow the capacitor to store more charge.
Hint: Consider how the battery regulates the charge on the capacitor.
Step 6: Conclusion
Based on the analysis:
The capacitance does not increase.
The potential difference does not change.
The charge does not increase.
Equal and opposite charges appear on the two faces of the metal sheet.
Thus, the correct conclusion is that equal and opposite charges will appear on the two faces of the metal sheet.Alternate:
The capacitance of the capacitor in which a dielectric slab of dielectric constant K , area A and thickness \(t\) is inserted between the plates of the capacitor of area \(A\) and separated by a distance \(d\) is given by \(C=\frac{\in_0 A}{(d-t)+\left(\frac{t}{K}\right)}\)
Since it is given that the thickness of the sheet is negligible, the above formula reduces to \(\mathrm{C}=\frac{\in_0 A}{d}\). In other words, there will not be any change in the electric field, potential or charge. -
Question 32 of 106
32. Question
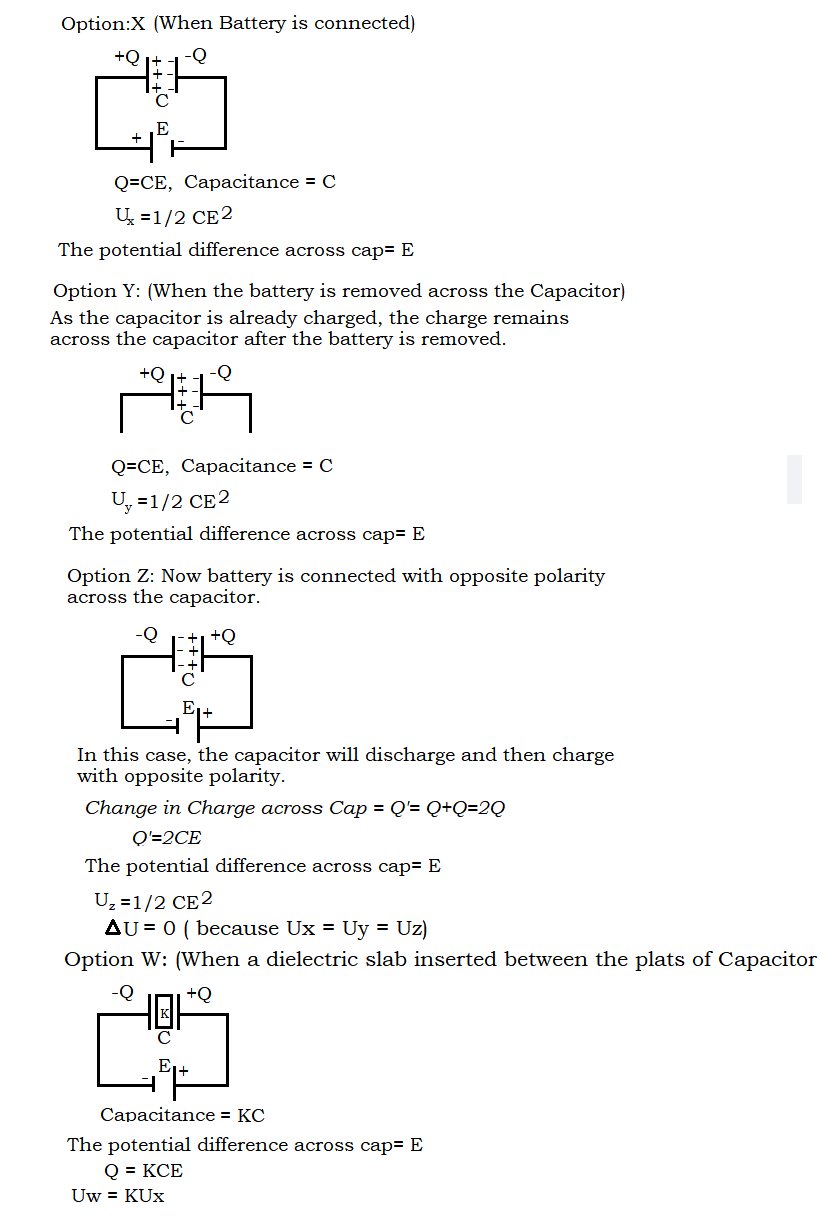
The following operations can be performed on a capacitor:
\(X\) – connect the capacitor to a battery of emf \(\varepsilon\).
\(Y\) – disconnect the battery.
\(Z\) – reconnect the battery with polarity reversed.
\(W\) – insert a dielectric slab in the capacitor.
(a) In \(X Y Z\) (perform \(X\), then \(Y\), then \(Z\) ) the stored electric energy remains unchanged and no thermal energy is developed.
(b) The charge appearing on the capacitor is greater after the action \(X W Y\) than after the action \(X Y W\).
(c) The electric energy stored in the capacitor is greater after the action \(W X Y\) than after the action \(X Y W\).
(d) The electric field in the capacitor after the action \(X W\) is the same as that after \(W X\).CorrectIncorrectHint
(b) (b,c,d) Justification of option
-
Question 33 of 106
33. Question
Find the work done by some external force in moving a charge \(q=4 \mu \mathrm{C}\) from infinity to a point, where electric potential is \(10^4 \mathrm{~V}\).
CorrectIncorrectHint
(a) Using the relation, \(W=q V_A\) where, \(V_A\) is the electric potential at point \(A\). We have, \(W=\left(4 \times 10^{-6}\right)\left(10^4\right)=4 \times 10^{-2} \mathrm{~J}\)
-
Question 34 of 106
34. Question
Equal charges are given to two spheres of different radii. The potential will
CorrectIncorrectHint
(a) The potential will be more on the smaller sphere.
Explanation: The potential \((\mathrm{V})\) of a sphere is given by the formula \(\mathrm{V}=\mathrm{kQ} / \mathrm{r}\), where \(k\) is Coulomb’s constant, \(Q\) is the charge, and \(r\) is the radius of the sphere. Since the charge \(({Q})\) is the same for both spheres, the potential is inversely proportional to the radius \((\mathrm{V} \propto 1 / \mathrm{r})\). Therefore, the smaller the radius, the higher the potential. -
Question 35 of 106
35. Question
The electric potential at a point in free space due to a charge \(Q\) coulomb is \(Q \times 10^{11}\) volts. The electric field at the point is
CorrectIncorrectHint
(c) Electric potential at a distance \(r\) due to point charge \(Q\) is \(V=\frac{k Q}{r}\) and electric field at same point is \(E=\frac{k Q}{r^2}\)
\(
\begin{array}{r}
\Rightarrow \quad E=\frac{k Q}{(k Q / V)^2}=\frac{V^2}{k Q}=\frac{\left(Q \times 10^{11}\right)^2}{k Q}=4 \pi \varepsilon_0 Q \times 10^{22} \mathrm{~V} / \mathrm{m} \\
\\
\left(\because k=\frac{1}{4 \pi \varepsilon_0}\right)
\end{array}
\) -
Question 36 of 106
36. Question
In an hydrogen atom, the electron revolves around the nucleus in an orbit of radius \(0.53 \times 10^{-10} \mathrm{~m}\). Then, the electrical potential produced by the nucleus at the position of the electron is
CorrectIncorrectHint
(c) The electrical potential produced by the nucleus at the position of the electron,
\(
\begin{aligned}
V & =9 \times 10^9 \times \frac{q}{r} \\
& =9 \times 10^9 \times \frac{\left(+1.6 \times 10^{-19}\right)}{0.53 \times 10^{-10}}=27.2 \mathrm{~V}
\end{aligned}
\) -
Question 37 of 106
37. Question
Three charges \(2 q,-q,-q\) are located at the vertices of an equilateral triangle. At the centre of the triangle,
CorrectIncorrectHint
(b) Obviously, from charge configuration, at the centre electric field is non-zero. Potential at the centre due to charge \(2 q, V_{2 q}=\frac{2 q}{r}\) and potential due to \(-q\) charge, \(V_{-q}=-\frac{q}{r}\) (where, \(r=\) distance of centre point)
\(\therefore\) Total potential, \(V=V_{2 q}+V_{-q}+V_{-q}=0\)
-
Question 38 of 106
38. Question
In a region of constant potential,
CorrectIncorrectHint
(b) The electric field intensity \(E\) and electric potential \(V\) are related as \(E=-\frac{d V}{d r}\) and for \(V=\) constant, \(\frac{d V}{d r}=0\) This imply that electric field intensity, \(E=0\).
-
Question 39 of 106
39. Question
The work done in bringing a 20 C charge from point \(A\) to point \(B\) for distance 0.2 m is 2 J. The potential difference between the two points will be (in volt)
CorrectIncorrectHint
\(
\text { (c) Potential difference, } \Delta V=\frac{W}{q}=\frac{2}{20}=0.1 \mathrm{~V}
\) -
Question 40 of 106
40. Question
As shown in the figure, charges \(+q\) and \(-q\) are placed at the vertices \(B\) and \(C\) of an isosceles triangle. The potential at the vertex \(A\) is
 CorrectIncorrect
CorrectIncorrectHint
\(
\begin{aligned}
&\text { (c) Potential at } A=\text { Potential due to }(+q) \text { charge }+ \text { Potential }\\
&=\frac{1}{4 \pi \varepsilon_0} \cdot \frac{q}{\sqrt{a^2+b^2}}+\frac{1}{4 \pi \varepsilon_0} \frac{(-q)}{\sqrt{a^2+b^2}}=0
\end{aligned}
\) -
Question 41 of 106
41. Question
In the rectangle shown below, the two corners have charges \(q_1=-5 \mu \mathrm{C}\) and \(q_2=+2.0 \mu \mathrm{C}\). The work done in moving a charge \(+3.0 \mu \mathrm{C}\) from \(B\) to \(A\) is (take, \(k=10^{10} \mathrm{~N}-\mathrm{m}^2 / \mathrm{C}^2\) )
 CorrectIncorrect
CorrectIncorrectHint
\(
\begin{aligned}
\text { (a) } V_A & =10^{10}\left[\frac{-5 \times 10^{-6}}{15 \times 10^{-2}}+\frac{2 \times 10^{-6}}{5 \times 10^{-2}}\right]=\frac{1}{15} \times 10^6 \mathrm{~V} \\
V_B & =10^{10}\left[\frac{2 \times 10^{-6}}{15 \times 10^{-2}}-\frac{5 \times 10^{-6}}{5 \times 10^{-2}}\right]=-\frac{13}{15} \times 10^6 \mathrm{~V} \\
\therefore W & =q\left(V_A-V_B\right)=3 \times 10^{-6}\left[\frac{1}{15} \times 10^6-\left(-\frac{13}{15} \times 10^6\right)\right]=2.8 \mathrm{~J}
\end{aligned}
\) -
Question 42 of 106
42. Question
Two point charges \(-q\) and \(+q\) are located at points \((0,0,-a)\) and \((0,0, a)\), respectively. The potential at a point \((0,0, z)\), where \(z>a\) is
CorrectIncorrectHint
(c) Potential at \(P\) due to \((+q)\) charge
\(
V_1=\frac{1}{4 \pi \varepsilon_0} \cdot \frac{q}{(z-a)}
\)
Potential at \(P\) due to \((-q)\) charge, \(V_2=\frac{1}{4 \pi \varepsilon_0} \cdot \frac{-q}{(z+a)}\)
Total potential at \(P\) due \((A B)\) electric dipole,
\(
\begin{aligned}
V & =V_1+V_2 \\
& =\frac{1}{4 \pi \varepsilon_0} \cdot \frac{q}{(z-a)}-\frac{1}{4 \pi \varepsilon_0} \frac{q}{(z+a)} \\
& =\frac{2 q a}{4 \pi \varepsilon_0\left(z^2-a^2\right)}
\end{aligned}
\) -
Question 43 of 106
43. Question
Two plates are 2 cm apart and a potential difference of 10 V is applied between them, then the electric field between the plates is
CorrectIncorrectHint
\(
\text { (b) Electric field, } E=\frac{V}{d}=\frac{10}{2 \times 10^{-2}}=500 \mathrm{~N} / \mathrm{C}
\) -
Question 44 of 106
44. Question
At a certain distance from a point charge the electric field is \(500 \mathrm{Vm}^{-1}\) and the potential is 3000 V. What is this distance?
CorrectIncorrectHint
\(
\begin{aligned}
&\text { (a) We have, } V=E \times d\\
&\Rightarrow \text { Distance, } d=\frac{V}{E}=\frac{3000}{500}=6 \mathrm{~m}
\end{aligned}
\) -
Question 45 of 106
45. Question
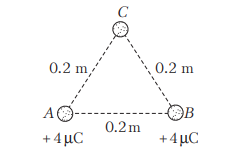
Two charges of \(4 \mu \mathrm{C}\) each are placed at the corners \(A\) and \(B\) of an equilateral triangle of side length 0.2 m in air. The electric potential at \(C\) is \(\left[\frac{1}{4 \pi \varepsilon_0}=9 \times 10^9 \mathrm{~N}-\mathrm{m}^2 \mathrm{C}^{-2}\right]\)
CorrectIncorrectHint
\(
\begin{aligned}
&\text { (c) Potential at }\\
&\begin{aligned}
C, V & =2\left(\frac{1}{4 \pi \varepsilon_0} \cdot \frac{q}{r}\right) \\
& =\left(9 \times 10^9 \times \frac{4 \times 10^{-6}}{0.2}\right) \times 2=36 \times 10^4 \mathrm{~V}
\end{aligned}
\end{aligned}
\)
-
Question 46 of 106
46. Question
The electric potential \(V\) at any point \(O(x, y, z\) all in metres) in space is given by \(V=4 x^2 \mathrm{~V}\). The electric field at the point \((1 \mathrm{~m}, 0,2 \mathrm{~m})\) in volt metre \(^{-1}\) is
CorrectIncorrectHint
(a) The electric potential \(V(x, y, z)=4 x^2 \mathrm{~V}\)
Now, \(\mathbf{E}=-\left(\hat{\mathbf{i}} \frac{\partial V}{\partial x}+\hat{\mathbf{j}} \frac{\partial V}{\partial y}+\hat{\mathbf{k}} \frac{\partial V}{\partial z}\right)\)
Now, \(\frac{\partial V}{\partial x}=8 x, \frac{\partial V}{\partial y}=0\) and \(\frac{\partial V}{\partial z}=0\)
Hence, \(\mathbf{E}=-8 x \hat{\mathbf{i}} \mathrm{~V} / \mathrm{m}\). So, at point \((1 \mathrm{~m}, 0,2 \mathrm{~m})\)
\(\mathbf{E}=-8 \hat{\mathbf{i}} \mathrm{~V} / \mathrm{m}\) or 8 , along negative \(X\)-axis. -
Question 47 of 106
47. Question
The electric potential \(V\) is given as a function of distance \(x\) (metre) by \(V=\left(5 x^2+10 x-9\right) \mathrm{V}\). Value of electric field at \(x=1\) is
CorrectIncorrectHint
\(
\begin{aligned}
&\text { (a) We have, electric field, }\\
&E=-\frac{d V}{d x}=-\frac{d}{d x}\left(5 x^2+10 x-9\right)=-10 x-10
\end{aligned}
\)
\(
\therefore \quad(E)_{x=1}=-10 \times 1-10=-20 \mathrm{~V} / \mathrm{m}
\) -
Question 48 of 106
48. Question
The work done to move a charge along an equipotential from \(A\) to \(B\)
CorrectIncorrectHint
(c) Work done in displacing a charge particle is given by \(W_{A B}=q\left(V_B-V_A\right)\) and the line integral of electrical field from point \(A\) to \(B\) gives potential difference \(V_B-V_A=-\int_A^B E \cdot d l\) For equipotential surface, \(V_B-V_A=0\) and hence \(W=0\).
-
Question 49 of 106
49. Question
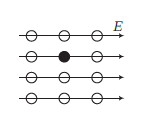
There is a uniform electric field of intensity \(E\) which is as shown. How many labelled points have the same electric potential as the fully shaded point?
 CorrectIncorrect
CorrectIncorrectHint
(c) Equipotential line always perpendicular to electric field lines
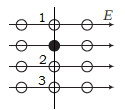
We can see that three more points (1, 2 and 3) are perpendicular to the electric field apart from the shaded point.
-
Question 50 of 106
50. Question
The electric potential at the surface of an atomic nucleus ( \(\mathrm{Z}=50\) ) of radius \(9.0 \times 10^{-15} \mathrm{~cm}\)
CorrectIncorrectHint
\(
\begin{aligned}
&\text { (b) Potential, }\\
&\begin{aligned}
V=\frac{K q}{r}=\frac{K Z e}{r} & =\frac{9 \times 10^9 \times 50 \times 16 \times 10^{-19}}{9 \times 10^{-15}} \\
& =8 \times 10^6 \mathrm{~V}
\end{aligned}
\end{aligned}
\) -
Question 51 of 106
51. Question
A hollow metal sphere of radius 5 cm is charged, so that the potential on its surface is 10 V. The potential at the centre of the sphere is
CorrectIncorrectHint
(b) Since, potential inside the hollow sphere is same as that on the surface.
-
Question 52 of 106
52. Question
Two charged spheres of radii \(R_1\) and \(R_2\) having equal surface charge density. The ratio of their potential is
CorrectIncorrectHint
(a) Let \(Q_1\) and \(Q_2\) are the charges on sphere of radii \(R_1\) and \(R_2\), respectively.

Surface charge density, \(\sigma=\frac{\text { charge }}{\text { area }}\)
According to given problem, \(\sigma_1=\sigma_2\)
\(
\begin{aligned}
& \frac{Q_1}{4 \pi R_1^2} & =\frac{Q_2}{4 \pi R_2^2} \\
\therefore & \frac{Q_1}{Q_2} & =\frac{R_1^2}{R_2^2} \dots(i)
\end{aligned}
\)
\(
\begin{aligned}
&\text { In case of a charged sphere, }\\
&V_s=\frac{1}{4 \pi \varepsilon_0} \frac{Q}{R}
\end{aligned}
\)
\(
\begin{aligned}
V_1 & =\frac{1}{4 \pi \varepsilon_0} \frac{Q_1}{R_1}, V_2=\frac{1}{4 \pi \varepsilon_0} \frac{Q_2}{R_2} \\
\frac{V_1}{V_2} & =\frac{Q_1}{R_1} \times \frac{R_2}{Q_2}=\frac{Q_1}{Q_2} \times \frac{R_2}{R_1} \\
& =\left(\frac{R_1}{R_2}\right)^2 \times\left(\frac{R_2}{R_1}\right)=\frac{R_1}{R_2} \text { [using Eq. (i)] }
\end{aligned}
\) -
Question 53 of 106
53. Question
The radii of two concentric spherical conducting shells are \(r_1\) and \(r_2\left(>r_1\right)\). The charge on the outer shell is \(q\). The charge on the inner shell which is connected to the earth is
CorrectIncorrectHint
(c) Let \(q^{\prime}\) be the charge on the inner shell. Then, the potential of the inner is \(V=\) potential due to its own \(q^{\prime}+\) potential due to the outer charge
\(
=\frac{1}{4 \pi \varepsilon}\left(\frac{q}{r_1}+\frac{q}{r_2}\right)
\)
But \(V=0\), because the inner shell is earthed.
\(
\therefore \quad \frac{q_1}{r_1}+\frac{q_2}{r_2}=0 \quad q^{\prime}=-q\left(\frac{r_1}{r_2}\right)
\) -
Question 54 of 106
54. Question
If a charged spherical conductor of radius 10 cm has potential \(V\) at a point distant 5 cm from its centre, then the potential at a point distant 15 cm from the centre will be
CorrectIncorrectHint
(b) Since the point at 5 cm is inside the conductor, the potential at this point is equal to the potential at the surface.
Therefore, the potential at the surface is \(v\).
The potential at the surface of the sphere is given by \(V=\frac{k Q}{r}\), where \(r\) is the radius of the sphere.
Solving for \(Q[latex], we get [latex]Q=\frac{V r}{k}\).
Substituting \(r=10 \mathrm{~cm}=0.1 \mathrm{~m}\), we get \(Q=\frac{V \times 0.1}{k}\).
The potential at a distance \(r^{\prime}=15 \mathrm{~cm}=0.15 \mathrm{~m}\) from the center is given by \(V^{\prime}=\frac{k Q}{r^{\prime}}\). Substituting \(Q=\frac{0.1 V}{k}\), we get \(V^{\prime}=\frac{k}{0.15} \times \frac{0.1 V}{k}\).
Simplifying, we get \(V^{\prime}=\frac{0.1 V}{0.15}=\frac{1}{1.5} V=\frac{2}{3} V\).
The potential at a point distant 15 cm from the center is \(\frac{2}{3} V\). -
Question 55 of 106
55. Question
A hollow conducting sphere of radius \(R\) has a charge \((+q)\) on its surface. What is the electric potential within the sphere at a distance \(r=R / 3\) from its centre
CorrectIncorrectHint
(c) Inside a conducting body, potential is same everywhere and equals to the potential at its surface.
The electric potential \(\boldsymbol{V}\) on the surface of the sphere is given by:
\(V=\frac{1}{4 \pi \epsilon_0} \frac{q}{R}\)Since the sphere is a conductor, the electric potential inside is constant and equal to the potential on the surface.
Therefore, the electric potential at \(r=\frac{R}{3}\) is the same as on the surface.
\(V_{\text {inside }}=V_{\text {surface }}=\frac{1}{4 \pi \epsilon_0} \frac{q}{R}\)
The electric potential within the sphere at a distance \(r=\frac{R}{3}\) from its center is \(\frac{1}{4 \pi \epsilon_0} \frac{q}{R}\). -
Question 56 of 106
56. Question
A spherical conductor of radius 2 m is charged to a potential of 120 V. It is now placed inside another hollow spherical conductor of radius 6 m. Calculate the potential to which the bigger sphere would be raised.
CorrectIncorrectHint
(d) If charge acquired by the smaller sphere is \(q\), then its potential, \(120=\frac{k q}{2} \dots(i)\).
Whole charge comes to outer sphere.
Also, potential of the outer sphere,
\(
V=\frac{k q}{6} \dots(ii)
\)
From Eq. (i) and (ii), we get
\(
V=40 \mathrm{~V}
\) -
Question 57 of 106
57. Question
Three concentric spherical shells have radii \(a, b\) and \(c(a<b<c)\) and have surface charge densities \(\sigma,-\sigma\) and \(\sigma\), respectively. If \(V_A, V_B\) and \(V_C\) denote the potentials of the three shell, … for \(c=a+b\), we have
CorrectIncorrectHint
\(
\text { (a) } V_A=\frac{\sigma}{\varepsilon_0}(a-b+c)
\)
\(
\begin{aligned}
&\begin{aligned}
V_B & =\frac{\sigma}{\varepsilon_0}\left(\frac{a^2}{c}-b+c\right) \\
\Rightarrow \quad V_C & =\frac{\sigma}{\varepsilon_0}\left(\frac{a^2}{c}-\frac{b^2}{c}+c\right)
\end{aligned}\\
&\text { On putting } c=a+b \Rightarrow V_A=V_C \neq V_B
\end{aligned}
\) -
Question 58 of 106
58. Question
The electrostatic potential of a uniformly charged thin spherical shell of charge \(Q\) and radius \(R\) at a distance \(r\) from the centre is
CorrectIncorrectHint
(a) If charge on a conducting sphere of radius \(R\) is \(Q\) then potential outside the sphere,
\(
V_{\text {out }}=\frac{1}{4 \pi \varepsilon_0} \frac{Q}{r}
\)
At the surface of sphere
\(
V_s=\frac{1}{4 \pi \varepsilon_0} \frac{Q}{R}
\) -
Question 59 of 106
59. Question
The diameter of a hollow metallic sphere is 60 cm and the sphere carries a charge of \(500 \mu \mathrm{C}\). The potential at a distance of 100 cm from the centre of the sphere will be
CorrectIncorrectHint
(c) Potential, \(V=\frac{1}{4 \pi \varepsilon_0} \cdot \frac{q}{r}=9 \times 10^9 \times \frac{500 \times 10^{-6}}{1.0}\) \(=4.5 \times 10^6 \mathrm{~V}\)
-
Question 60 of 106
60. Question
Obtain the energy in joule acquired by an electron beam when accelerated through a potential difference of 2000 V. How much speed will the electron gain?
CorrectIncorrectHint
(a) The kinetic energy gained by a charge of \(q\) coulomb through a potential difference of \(V\) volt is
\(
\begin{aligned}
& K=q V \mathrm{~J} \\
& K=\left(1.6 \times 10^{-19}\right)(2000)=3.2 \times 10^{-16} \mathrm{~J}
\end{aligned}
\)
We have,
\(
\begin{aligned}
& K=\frac{1}{2} m v^2 \\
& v=\sqrt{\frac{2 K}{m}}=\frac{2 \times 3.2 \times 10^{-16}}{9 \times 10^{-31}}=\frac{8}{3} \times 10^7 \mathrm{~m} / \mathrm{s}
\end{aligned}
\) -
Question 61 of 106
61. Question
A particle \(A\) has charge \(+16 q\) and a particle \(B\) has charge \(+4 q\) with each of them having the same mass \(m\). When allowed to fall from rest through the same electric potential difference, the ratio of their speed \(\frac{v_A}{v_B}\) will becomes
CorrectIncorrectHint
\(
\begin{aligned}
&\text { (a) Speed of the particle, } v=\sqrt{\frac{2 Q V}{m}}\\
&\Rightarrow \quad v \propto \sqrt{Q} \Rightarrow \frac{v_A}{v_B}=\sqrt{\frac{Q_A}{Q_B}}=\sqrt{\frac{16 q}{4 q}}=\frac{2}{1}
\end{aligned}
\) -
Question 62 of 106
62. Question
What potential difference must be applied to produce an electric field that can accelerate an electron to one-tength the velocity of light?
CorrectIncorrectHint
\(
\begin{aligned}
&\text { (c) The kinetic energy of the electron, }\\
&K=e V=\frac{1}{2} m v^2
\end{aligned}
\)
\(
V=\frac{1}{2} \frac{m v^2}{e}
\)
\(
\text { Here, } \quad v=\frac{c}{10}=\frac{3 \times 10^8}{10}=3 \times 10^7 \mathrm{~m} / \mathrm{s}
\)
\(
V=\frac{1}{2} \frac{\left(9.0 \times 10^{-31}\right)\left(3.0 \times 10^{-7}\right)^2}{\left(1.6 \times 10^{-19}\right)}
\)
\(
\text { Potential, } \quad V=2531 \mathrm{~V}
\) -
Question 63 of 106
63. Question
When a positive charge \(q\) is taken from lower potential to a higher potential point, then its potential energy will
CorrectIncorrectHint
(b) \(\Delta \mathrm{PE}=\) Work done by external agent \(=\left(V_f q-V_i q\right), V_f>V_i \Rightarrow \Delta \mathrm{PE}>0\), i.e. PE will increase.
-
Question 64 of 106
64. Question
When one electron is taken towards the other electron, then the electric potential energy of the system
CorrectIncorrectHint
(b) Potential energy of the system will be given by
\(
=\frac{(-e)(-e)}{4 \pi \varepsilon_0 r}=\frac{e^2}{4 \pi \varepsilon_0 r}
\)
As, \(r\) decreases, potential energy increases. -
Question 65 of 106
65. Question
Two positive point charges of \(12 \mu \mathrm{C}\) and \(8 \mu \mathrm{C}\) are 10 cm apart. The work done in bringing them 4 cm is
CorrectIncorrectHint
\(
\begin{aligned}
& \text { (c) Work done, } W=U_f-U_i=9 \times 10^9 \times Q_1 Q_2\left[\frac{1}{r_2}-\frac{1}{r_1}\right] \\
& \begin{aligned}
& \Rightarrow W=9 \times 10^9 \times 12 \times 10^{-6} \times 8 \times 10^{-6}\left[\frac{1}{4 \times 10^{-2}}-\frac{1}{10 \times 10^{-2}}\right] \\
& \quad=12.96 \mathrm{~J} \approx 13 \mathrm{~J}
\end{aligned}
\end{aligned}
\) -
Question 66 of 106
66. Question
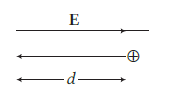
A proton moves a distance \(d\) in a uniform electric field \(\mathbf{E}\) as shown in the figure. Does the electric field do a positive or negative work on the proton? Does the electric potential energy of the proton increase or decrease?
 CorrectIncorrect
CorrectIncorrectHint
(a) Since, the proton is moving against the direction of electric field, so work is done by the proton against electric field. It implies that electric field does negative work on the proton. Again, proton is moving in electric field from low potential region to high potential region, hence its potential energy increases.
-
Question 67 of 106
67. Question
The electrostatic potential energy between proton and electron separated by a distance \(1 Å\) is
CorrectIncorrectHint
(c) Electric potential energy, \(U=\frac{1}{4 \pi \varepsilon_0} \frac{q_1 q_2}{d}\)
\(
\begin{aligned}
\therefore \quad U & =\frac{\left(9 \times 10^9\right) \times\left(1.6 \times 10^{-19}\right) \times\left(-1.6 \times 10^{-19}\right) \mathrm{J}}{10^{-10}} \\
& =-9 \times 10^9 \times 1.6 \times 10^{-9} \mathrm{eV}=-14.4 \mathrm{eV}
\end{aligned}
\)
Note In the solution given all the values are positive. It is important to mention the sign. -
Question 68 of 106
68. Question
Identify the wrong statement.
CorrectIncorrectHint
(c) Potential energy as well as force are positive, if there is repulsion between the particles and negative, if there is attraction.
We take only the magnitude of values when discussing decrease or increase of energy.
As, \(\quad U=\frac{Q_1 Q_2}{4 \pi \varepsilon_0 r}\)
Plus or minus i.e., whether both are of the same sign or different, if \(r\) decreases, the value increase. Therefore, option (c) is wrong. -
Question 69 of 106
69. Question
Two positive point charges of 12 and 5 microcoulombs are placed 10 cm apart in air. The work needed to bring them 4 cm closer is
CorrectIncorrectHint
(b) Potential energy of charges \(Q_1\) and \(Q_2\) at 10 cm apart,
\(
U_i=\frac{1}{4 \pi \varepsilon_0} \frac{12 \times 10^{-6} \times 5 \times 10^{-6}}{0.1}
\)
\(
=\frac{9 \times 10^9 \times 60 \times 10^{-12}}{0.1}=54 \times 10^{-1}=5.4 \mathrm{~J}
\)
Potential energy of charge \(Q_1\) and \(Q_2\) at 6 cm apart,
\(
U_2=\frac{9 \times 10^9 \times 60 \times 10^{-12}}{0.06}=9 \mathrm{~J}
\)
\(\therefore\) Work done \(=(9-5.4) \mathrm{J}=3.6 \mathrm{~J}\) -
Question 70 of 106
70. Question
Three identical charges each of \(2 \mu \mathrm{C}\) are placed at the vertices of a triangle \(A B C\) as shown in the figure.

If \(A B+A C=12 \mathrm{~cm}\) and \(A B \cdot A C=32 \mathrm{~cm}^2\), the potential energy of the charge at \(A\) is
CorrectIncorrectHint
\(
\begin{gathered}
\text { (d) We have, } A B+A C=12 \mathrm{~cm} \dots(i) \\
A B \cdot A C=32 \mathrm{~cm}^2
\end{gathered}
\)
\(
\begin{aligned}
\therefore A B-A C & =\sqrt{(A B+A C)^2-4 A B \cdot A C} \\
& A B-A C \dots(ii)
\end{aligned}=4 \mathrm{~cm}
\)
From Eqs. (i) and (ii), we get
\(
A B=8 \mathrm{~cm}, A C=4 \mathrm{~cm}
\)
Potential energy at point \(A\),
\(
\begin{aligned}
U_A & =\frac{1}{4 \pi \varepsilon_0}\left[\frac{q_A q_B}{A B}+\frac{q_A q_C}{A C}\right] \\
& =\frac{9 \times 10^9 \times 4 \times 10^{-12}}{10^{-2}}\left(\frac{1}{8}+\frac{1}{4}\right)=1.35 \mathrm{~J}
\end{aligned}
\) -
Question 71 of 106
71. Question
If three charges are placed at the vertices of equilateral triangle of charge \(q\) each, what is the net potential energy, if the side of equilateral triangle is \(l \mathrm{~cm}\)?
CorrectIncorrectHint
(c) Potential energy, \(U=\frac{1}{4 \pi \varepsilon_0}\left[\frac{Q_1 Q_2}{r_1}+\frac{Q_2 Q_3}{r_2}+\frac{Q_1 Q_3}{r_3}\right]\)
Net potential energy, \(U_{\text {net }}=\frac{3}{4 \pi \varepsilon_0} \cdot \frac{q^2}{l}\) -
Question 72 of 106
72. Question
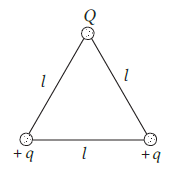
Three charges \(Q+q\) and \(+q\) are placed at the vertices of an equilateral triangle of side \(l\) as shown in the figure. If the net electrostatic energy of the system is zero, then \(Q\) is equal to
 CorrectIncorrect
CorrectIncorrectHint
\(
\begin{aligned}
&\text { (a) Potential energy of the system, }\\
&\begin{gathered}
U=K \frac{Q q}{l}+\frac{K q^2}{l}+\frac{K q Q}{l}=0 \\
\Rightarrow \quad \frac{K q}{l}(Q+q+Q)=0 \Rightarrow Q=-\frac{q}{2}
\end{gathered}
\end{aligned}
\) -
Question 73 of 106
73. Question
If identical charges \((-q)\) are placed at each corner of a cube of side \(b\), then electric potential energy of charge \((+q)\) which is placed at centre of the cube will be
CorrectIncorrectHint
(d) Length of the diagonal of a cube having each side \(b\) is \(\sqrt{3} b\). So, the distance of centre of cube from each vertex is \(\frac{\sqrt{3} b}{2}\). Hence, the potential energy of the given system of charge is
\(
U=8 \times\left[\frac{1}{4 \pi \varepsilon_0} \cdot \frac{(-q)(q)}{\sqrt{3} b / 2}\right]=\frac{-4 q^2}{\sqrt{3} \pi \varepsilon_0 b}
\) -
Question 74 of 106
74. Question
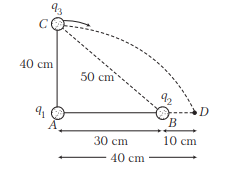
Two charges \(q_1\) and \(q_2\) are placed 30 cm apart as shown in the figure. A third charge \(q_3\) is moved along the arc of a circle of radius 40 cm from \(C\) to \(D\). The change in the potential energy of the system is \(\frac{q_3}{4 \pi \varepsilon_0} k\), where \(k\) is
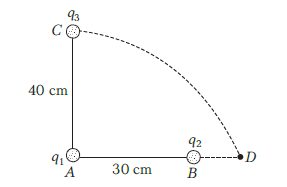 CorrectIncorrect
CorrectIncorrectHint
\(
\text { (a) Change in potential energy }(\Delta U)=U_f-U_i
\)
\(
\begin{aligned}
&\text { Potential energy, }\\
&\begin{aligned}
\Delta U & =\frac{1}{4 \pi \varepsilon_0}\left[\left(\frac{q_1 q_3}{0.4}+\frac{q_2 q_3}{0.1}\right)-\left(\frac{q_1 q_3}{0.4}+\frac{q_2 q_3}{0.5}\right)\right] \\
\Delta U & =\frac{1}{4 \pi \varepsilon_0}\left[8 q_2 q_3\right]=\frac{q_3}{4 \pi \varepsilon_0}\left(8 q_2\right) \\
k & =8 q_2
\end{aligned}
\end{aligned}
\) -
Question 75 of 106
75. Question
For dipole \(q=2 \times 10^{-6} \mathrm{C}\) and \(d=0.01 \mathrm{~m}\), calculate the maximum torque for this dipole if \(E=5 \times 10^5 \mathrm{~N} / \mathrm{C}\).
CorrectIncorrectHint
(c) Torque, \(\begin{aligned} \tau_{\max } & =p E=q(2 l) E=2 \times 10^{-6} \times 0.01 \times 5 \times 10^5 \\ & =10 \times 10^{-3} \mathrm{~N}-\mathrm{m}\end{aligned}\)
-
Question 76 of 106
76. Question
A molecule with a dipole moment \(p\) is placed in a electric field of strength \(E\). Initially, the dipole is aligned parallel to the field. If the dipole is to be rotated to be anti-parallel to the field, then the work required to be done by an external agency is
CorrectIncorrectHint
\(
\begin{gathered}
\text { (d) Work done, } W=p E(1-\cos \theta)=p E\left(1-\cos 180^{\circ}\right) \\
W=p E[1-(-1)]=2 p E
\end{gathered}
\) -
Question 77 of 106
77. Question
Three point charges of \(1 \mathrm{C}, 2 \mathrm{C}\) and \(3 C\) are placed at the corners of an equilateral triangle of side 100 cm. The work done to move these charges to the corners of a similar equilateral triangle of side 50 cm , will be
CorrectIncorrectHint
(a) Given, \(q_1=1 \mathrm{C}, q_2=2 \mathrm{C}, q_3=3 \mathrm{C}\) and \(r_1=100 \mathrm{~cm}=1 \mathrm{~m}\) Initial PE of system, \(U_1=\frac{1}{4 \pi \varepsilon_0 r_1}\left(q_1 q_2+q_2 q_3+q_3 q_1\right)\)
\(
\begin{aligned}
& =\frac{9 \times 10^9}{1}(1 \times 2+2 \times 3+3 \times 1) \\
& =99 \times 10^9 \mathrm{~J}
\end{aligned}
\)
When \(r_2=50 \mathrm{~cm}=0.5 \mathrm{~m}\)
Final PE of system, \(U_2=\frac{1}{4 \pi \varepsilon_0 r_2}\left(q_1 q_2+q_2 q_3+q_3 q_1\right)\)
\(
=\frac{9 \times 10^9}{0.5}(1 \times 2+2 \times 3+3 \times 1)=2 \times 99 \times 10^9 \mathrm{~J}
\)
Work done,
\(
\begin{aligned}
W & =U_2-U_1=2 \times 99 \times 10^9-99 \times 10^9 \\
& =99 \times 10^9 \mathrm{~J}=9.9 \times 10^{10} \mathrm{~J}
\end{aligned}
\) -
Question 78 of 106
78. Question
Identify the false statement.
CorrectIncorrectHint
\(
\text { (d) } \mathrm{H}_2, \mathrm{O}_2, \mathrm{~N}_2 \text { etc., are not polar dielectrics. }
\) -
Question 79 of 106
79. Question
Eight drops of mercury of equal radii possessing equal charges combine to form a big drop. Then, the capacitance of bigger drop compared to each individual small drop is
CorrectIncorrectHint
(c) Volume of 8 small drops \(=\) Volume of big drop
\(
8 \times \frac{4}{3} \pi r^3=\frac{4}{3} \pi R^3 \Rightarrow R=2 r
\)
As, capacity is proportional to \(r\), hence capacity become 2 times. -
Question 80 of 106
80. Question
The capacitance of a spherical conductor is
CorrectIncorrectHint
(c) The capacitance of an isolated spherical conductor is given by \(C=\frac{Q}{V}\), where \(Q\) is the charge on the sphere and \(V\) is the potential at the surface. The potential at the surface of a sphere with charge \(Q\) is given by \(V=\frac{Q}{4 \pi \epsilon_0 R}\). Therefore, the capacitance is \(C=\frac{Q}{Q /\left(4 \pi \epsilon_0 R\right)}=4 \pi \epsilon_0 R\).
-
Question 81 of 106
81. Question
The earth has volume \(V\) and surface area \(A\), then capacitance would be
CorrectIncorrectHint
(c) The capacitance of the Earth, modeled as a sphere, is given by \(C=4 \pi \varepsilon_0 r\), where \(r\) is the radius. Since the volume of a sphere is \(V=(4 / 3) \pi r^3\) and the surface area is \(A=4 \pi r^2\), we can express the radius in terms of volume and surface area.
From \(V=(4 / 3) \pi r^3\), we have \(r=\sqrt{\frac{3 V}{4 \pi}}\).
From \(A=4 \pi r^2\), we have \(r=\sqrt{\frac{A}{4 \pi}}\).
Using the relationship between surface area and volume, we can write:
\(
\begin{aligned}
& r^2=\frac{A}{4 \pi} \\
& r^3=\frac{3 V}{4 \pi}
\end{aligned}
\)
Dividing the second equation by the first, we get:
\(
\begin{aligned}
& \frac{r^3}{r^2}=\frac{\frac{3 V}{4 \pi}}{\frac{A}{4 \pi}} \\
& r=\frac{3 V}{A}
\end{aligned}
\)
Substituting this value of \(r\) into the capacitance equation:
\(
C=4 \pi \varepsilon_0 r=4 \pi \varepsilon_0 \frac{3 V}{A}=12 \pi \varepsilon_0 \frac{V}{A}
\) -
Question 82 of 106
82. Question
If the circumference of a sphere is 2 m, then capacitance of sphere in water would be
CorrectIncorrectHint
(d) Given, \(2 \pi R=2 \Rightarrow R=\frac{1}{\pi}\)
For sphere \(C=4 \pi \varepsilon_0 K R\)
\(\Rightarrow \quad C=\frac{1}{9 \times 10^9} \times \frac{1}{\pi} \times 80 \quad\) (for water \(K=80[latex] )
Capacitance of sphere, [latex]C=282828 \mathrm{pF} \approx 2800 \mathrm{pF}\) -
Question 83 of 106
83. Question
The capacitance of parallel plate condenser depends on
CorrectIncorrectHint
(d) We have, \(C=\frac{\varepsilon_0 A}{d} \Rightarrow C \propto \frac{1}{d}\)
Therefore, the capacitance of parallel plate condenser depends on the separation between the plates. -
Question 84 of 106
84. Question
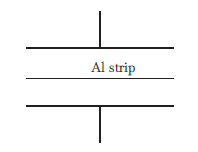
As shown in the figure, a very thin sheet of aluminium is placed in between the plates of the condenser. Then, the capacity will
 CorrectIncorrect
CorrectIncorrectHint
(c) Since, aluminium is a metal and very thin, therefore field inside this will be zero. Hence, it would not affect the field in between the two plates, so capacity \(=\frac{q}{V}=\frac{q}{E d}\), remains unchanged.
Explanation:
No change in field:
Since the aluminium sheet acts as a conductor, it doesn’t allow any electric field to penetrate through it, and thus the electric field between the plates of the capacitor remains the same as before the sheet was introduced.No change in capacitance:
The capacitance of a capacitor is primarily determined by the geometric factors like the area of the plates and the distance between them, along with the dielectric constant of the material between the plates. Since the sheet doesn’t change these geometric parameters or the dielectric constant (air or vacuum is used in this context), the capacitance remains unchanged. -
Question 85 of 106
85. Question
The potentials of the two plates of capacitor are +10 V and -10 V. The charge on one of the plates is 40 C. The capacitance of the capacitor is
CorrectIncorrectHint
(a) The potential difference across the parallel plate capacitor,
\(
\begin{aligned}
V & =10-(-10)=20 \mathrm{volt} \\
\therefore \text { Capacitance } & =\frac{Q}{V}=\frac{40}{20}=2 \mathrm{~F}
\end{aligned}
\) -
Question 86 of 106
86. Question
The capacitance of a parallel plate capacitor is \(12 \mu \mathrm{~F}\). If the distance between the plates is doubled and area is halved, then new capacitance will be
CorrectIncorrectHint
(d) \(C=\frac{\varepsilon_0 A}{d}\).
As \(A \rightarrow \frac{1}{2}\) times and \(d \rightarrow 2\) times So, \(C \rightarrow \frac{1}{4}[latex] times, i.e. [latex]C^{\prime}=\frac{1}{4} C=\frac{12}{4}=3 \mu \mathrm{~F}\) -
Question 87 of 106
87. Question
A parallel plate condenser has a capacitance \(50 \mu \mathrm{~F}\) in air and \(110 \mu \mathrm{~F}\) when immersed in an oil. The dielectric constant \(K\) of the oil is
CorrectIncorrectHint
\(
\begin{aligned}
& \text { (d) Capacitance, } C_{\text {medium }}=K C_{\text {air }} \\
& \Rightarrow \quad K=\frac{C_{\text {medium }}}{C_{\text {air }}}=\frac{110}{50}=2.20
\end{aligned}
\) -
Question 88 of 106
88. Question
A \(500 \mu \mathrm{~F}\) capacitor is charged at a steady rate of \(100 \mu \mathrm{C} / \mathrm{s}\). The potential difference across the capacitor will be 10 V after an interval of
CorrectIncorrectHint
(d) Capacitance: \(C=500 \mu F=500 \times 10^{-6} F\)
Charging rate: \(\frac{d Q}{d t}=100 \mu \mathrm{C} / \mathrm{s}=100 \times 10^{-6} \mathrm{C} / \mathrm{s}\)
Target voltage: \(V=10 \mathrm{~V}\)
Use the formula \(Q=C V\).
Substitute the given values:
\(Q=\left(500 \times 10^{-6} F\right) \times(10 V)\)
\(Q=5000 \times 10^{-6} C\)
\(Q=5 \times 10^{-3} C\)
Use the formula \(t=\frac{Q}{\frac{d Q}{d t}}\).
Substitute the values:
\(t=\frac{5 \times 10^{-3} \mathrm{C}}{100 \times 10^{-6} \mathrm{C} / \mathrm{s}}\)
\(t=\frac{5 \times 10^{-3}}{10^{-4}} \mathrm{~s}\)
\(t=50 \mathrm{~s}\)
The time it takes for the capacitor to reach 10 V is 50 s. -
Question 89 of 106
89. Question
There is an air-filled 1 pF parallel plate capacitor. When the plate separation is doubled and the space is filled with wax, the capacitance increases to 2 pF. The dielectric constant of wax is
CorrectIncorrectHint
\(
\begin{aligned}
& \text { (b) } C=\frac{\varepsilon_0 A}{d}=1 \mathrm{pF} \text { and } C=\frac{K \varepsilon_0 A}{2 d}=2 \mathrm{pF} \\
& \therefore K=4 .
\end{aligned}
\) -
Question 90 of 106
90. Question
A parallel plate capacitor with air between the plates has a capacitance of 9 pF . The separation between its plates is \(d\). The space between the plates is now filled with two dielectrics. One of the dielectrics has dielectric constant \(K_1=3\) and thickness \(d / 3\) while the other one has dielectric constant \(K_2=6\) and thickness \(2 d / 3\). Capacitance of the capacitor is now
CorrectIncorrectHint
(b)
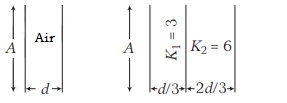
Capacitance in air, \(C_{\text {air }}=\frac{\varepsilon_0 A}{d}=9\)
Capacitance in medium,
\(
\begin{aligned}
\frac{1}{C_{\mathrm{med}}} & =\frac{1}{C_1}+\frac{1}{C_2}=\frac{d_1}{K_1 \varepsilon_0 A}+\frac{d}{K_2 \varepsilon_0 A} \\
\Rightarrow \quad C_{\mathrm{med}} & =\frac{K_1 K_2 \varepsilon_0 A}{K_1 d_2+K_2 d_1} \\
& =\frac{3 \times 6 \times \varepsilon_0 A}{3 \times 2 d / 3+6 \times d / 3}=\frac{18}{4} \times 9=40.5 \mathrm{pF}
\end{aligned}
\) -
Question 91 of 106
91. Question
Two parallel plate of area \(A\) are separated by two different dielectrics as shown in figure. The net capacitance is
 CorrectIncorrect
CorrectIncorrectHint
(a) Parallel plate capacitor, \(C=K \varepsilon_0 A / d\)
As, given in the figure, for series combination,
\(
\frac{1}{C^{\prime}}=\frac{1}{\frac{\varepsilon_0 A}{\frac{d}{2}}}+\frac{1}{\frac{2 \varepsilon_0 A}{\frac{d}{2}}} \Rightarrow {C^{\prime}}=\frac{4}{3} \frac{\varepsilon_0 A}{d}
\) -
Question 92 of 106
92. Question
Three capacitors each of capacitance \(C\) and of breakdown voltage \(V\) are joined in series. The capacitance and breakdown voltage of the combination will be
CorrectIncorrectHint
(c) Capacitor are in series, \(\frac{1}{C^{\prime}}=\frac{1}{C}+\frac{1}{C}+\frac{1}{C}=\frac{3}{C}\)
\(\therefore\) Capacitance, \(C^{\prime}=\frac{C}{3}\)
Total voltage of the series combination,
\(
V^{\prime}=V_1+V_2+V_3=V+V+V=3 V
\) -
Question 93 of 106
93. Question
Three condensers each of capacitance 2 F are put in series. The resultant capacitance is
CorrectIncorrectHint
(c) In series, \(\frac{1}{C}=\frac{1}{2}+\frac{1}{2}+\frac{1}{2}\) \(\Rightarrow\) Capacitance, \(C=\frac{2}{3} \mathrm{~F}\)
-
Question 94 of 106
94. Question
Two capacitors of capacitance \(2 \mu \mathrm{~F}\) and \(3 \mu \mathrm{~F}\) are joined in series. Outer plate of first capacitor is at 1000 V and outer plate of second capacitor is earthed (grounded). Now the potential on inner plate of each capacitor will be
CorrectIncorrectHint
(d) Equivalent capacitance \(=\frac{2 \times 3}{2+3}=\frac{6}{5} \mu \mathrm{~F}\)
Total charge by \(Q=C V=\frac{6}{5} \times 1000=1200 \mu \mathrm{C}\)
Potential \((V)\) across \(2 \mu \mathrm{~F}\) is \(V=\frac{Q}{C}=\frac{1200}{2}=600 \mathrm{~V}\)
\(\therefore\) Potential on inner plates \(=1000-600=400 \mathrm{~V}\) -
Question 95 of 106
95. Question
A series combination of three capacitors of capacities \(1 \mu \mathrm{~F}\) \(2 \mu \mathrm{~F}\) and \(8 \mu \mathrm{~F}\) is connected to a battery of emf 13 V. The potential difference across the plates of \(2 \mu \mathrm{~F}\) capacitor will be
CorrectIncorrectHint
(c) Capacitors are in series, \(\frac{1}{C_{\mathrm{eq}}}=\frac{1}{1}+\frac{1}{2}+\frac{1}{8}\)
\(
\Rightarrow \quad C_{\mathrm{eq}}=\frac{8}{13} \mu \mathrm{~F}
\)
Total charge, \(Q=C_{\mathrm{eq}} V=\frac{8}{13} \times 13=8 \mu \mathrm{C}\)
Potential difference across \(2 \mu \mathrm{~F}\) capacitor \(=\frac{8}{2}=4 \mathrm{~V}\) -
Question 96 of 106
96. Question
Four capacitors of equal capacitance have an equivalent capacitance \(C_1\) when connected in series and an equivalent capacitance \(C_2\) when connected in parallel. The ratio \(\frac{C_1}{C_2}\) is
CorrectIncorrectHint
(b) Capacitance, \(C_1=\frac{C}{4}\) (series)
Capacitance, \(C_2=4 C\) (parallel)
The ratio of capacitance,
\(
\therefore \quad \frac{C_1}{C_2}=\frac{C / 4}{4 C}=\frac{1}{16}
\) -
Question 97 of 106
97. Question
Three capacitors each of capacity \(4 \mu \mathrm{~F}\) are to be connected in such a way that the effective capacitance is \(6 \mu \mathrm{~F}\). This can be done by
CorrectIncorrectHint
(b) The given circuit can be drawn as follows
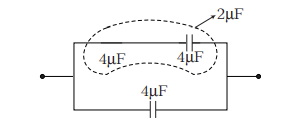
\(
\text { Effective capacitance, } C_{A B}=2+4=6 \mu \mathrm{~F}
\) -
Question 98 of 106
98. Question
In the figure shown, the effective capacitance between the points \(A\) and \(B\), if each has capacitance \(C\), is
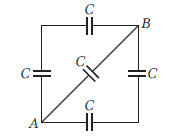 CorrectIncorrect
CorrectIncorrectHint
(a)
\(
\begin{aligned}
&\text { Equivalent capacitance between } A \text { and } B \text { is }\\
&C_{A B}=\frac{C}{2}+\frac{C}{2}+C=2 C
\end{aligned}
\) -
Question 99 of 106
99. Question
In the circuit as shown in the figure, the effective capacitance between \(A\) and \(B\) is
 CorrectIncorrect
CorrectIncorrectHint
(c) The given circuit can be simplified as follows
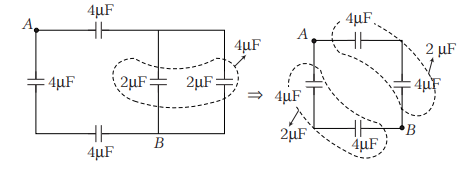
\(
\text { Equivalent capacitance between } A \text { and } B \text { is } C_{A B}=2+2=4 \mu \mathrm{~F}
\) -
Question 100 of 106
100. Question
The charge on anyone of the \(2 \mu \mathrm{~F}\) capacitors and \(1 \mu \mathrm{~F}\) capacitor will be given respectively (in \(\mu \mathrm{C}\) ) as
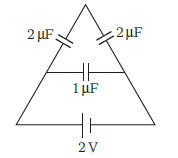 CorrectIncorrect
CorrectIncorrectHint
(d) Potential difference across both the lines is same, i.e. 2 V . Hence, charge flowing in line (2).
\(
Q=\left(\frac{2 \times 2}{2+2}\right)=2 \mu \mathrm{C}
\)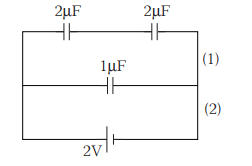
So, charge on each capacitor in line (2) is \(2 \mu \mathrm{C}\) and charge in line (1) is \(Q=2 \times 1=2 \mu \mathrm{C}\).
-
Question 101 of 106
101. Question
Equivalent capacitance between \(A\) and \(B\) is
 CorrectIncorrect
CorrectIncorrectHint
(a)
Therefore, capacitor \(2 \mu \mathrm{~F}, 4 \mu \mathrm{~F}\) and \(2 \mu \mathrm{~F}\) are in parallel.
So, equivalent capacitance between \(A\) and \(B\)
\(
C_{A B}=2+4+2=8 \mu \mathrm{~F}
\) -
Question 102 of 106
102. Question
The energy stored in a capacitor of capacitance \(100 \mu \mathrm{~F}\) is 50 J. Its potential difference is
CorrectIncorrectHint
(c) The energy stored in a capacitor is
\(
U=\frac{1}{2} C V^2
\)
So, potential difference, \(V=\sqrt{\frac{2 U}{C}}=\sqrt{\frac{2 \times 50}{100 \times 10^{-6}}}=1000 \mathrm{~V}\) -
Question 103 of 106
103. Question
A series combination of \(n_1\) capacitors, each of value \(C_1\), is charged by a source of potential difference 4 V. When another parallel combination of \(n_2\) capacitors, each of value \(C_2\), is charged by a source of potential difference \(V\), it has the same (total) energy stored in it, as the first combination has. The value of \(C_2\), in terms of \(C_1\) is, then
CorrectIncorrectHint
(a) In series capacitance, \(C^{\prime}=\left(\frac{C_1}{n_1}\right)\) and \(V^{\prime}=4 V\) Energy, \(U^{\prime}=\frac{1}{2} C^{\prime} V^{\prime 2}=\frac{1}{2}\left(\frac{C_1}{n_1}\right)(4 V)^2\)
In parallel capacitance, \(C^{\prime \prime}=n_2 C_2\) and \(V^{\prime \prime}=V\)
\(
U^{\prime \prime}=\frac{1}{2} C^{\prime \prime} V^{\prime \prime 2}=\frac{1}{2}\left(n_2 C_2\right) V^2
\)
Given, \(\frac{1}{2}\left(\frac{C_1}{n_1}\right)(4 V)^2=\frac{1}{2}\left(n_2 C_2\right) V^2\)
\(
\Rightarrow \quad C_2=\frac{16 C_1}{n_1 n_2}
\) -
Question 104 of 106
104. Question
If the charge on a capacitor is increased by 2 C, then the energy stored in it increases by \(21 \%\). The original charge on the capacitor is
CorrectIncorrectHint
(b) Energy of capacitor, \(U=\frac{1}{2} \frac{Q^2}{C}\)
\(
\begin{array}{ll}
\therefore & 1.21 U=\frac{1}{2} \frac{(Q+2)^2}{C} \\
\therefore & \frac{1.21}{1}=\frac{(Q+2)^2}{Q^2} \\
\Rightarrow & \sqrt{\frac{1.21}{1}}=\frac{Q+2}{Q} \\
\Rightarrow & 1.1 Q=Q+2
\end{array}
\)
Charge on the capacitor, \(Q=20 \mathrm{C}\) -
Question 105 of 106
105. Question
A capacitor of capacitance value \(1 \mu \mathrm{~F}\) is charged to 30 V and the battery is then disconnected. If it is connected across a \(2 \mu \mathrm{~F}\) capacitor, then the energy lost by the system is
CorrectIncorrectHint
\(
\begin{aligned}
& \text { (a) Initial energy }=\frac{1}{2} \times 1 \times 10^{-6} \times(30)^2=450 \times 10^{-6} \mathrm{~J} \\
& \text { Final energy }=\frac{1}{2}\left(C_1+C_2\right) V^2 \quad\left(\because V=\frac{V_1 C_1+V_2 C_2}{C_1+C_2}\right)
\end{aligned}
\)
\(
\begin{gathered}
=\frac{1}{2} \times 3 \times 10^{-6} \times(10)^2=150 \times 10^{-6} \mathrm{~J} \\
\text { Loss of energy }=(450-150) \times 10^{-6} \mathrm{~J}=300 \times 10^{-6}=300 \mu \mathrm{~J}
\end{gathered}
\) -
Question 106 of 106
106. Question
A parallel plate capacitor is charged to a potential difference of 50 V. It is then discharged through a resistance for 2 s and its potential drops by 10 V.. Calculate the fraction of energy stored in the capacitance
CorrectIncorrectHint
(d) Initial energy stored in the capacitor,
\(
U_i=\frac{1}{2} C V^2=\frac{1}{2} C \times(50)^2=\frac{1}{2} C(50)^2 \dots(i)
\)
After 2 s , when the potential drops by 10 V , the final potential is 40 V .
Final energy stored in the capacitor, \(U_f=\frac{1}{2} C(40)^2 \dots(ii)\)
Fraction of energy stored \(=\frac{U_f}{U_i}=\frac{\frac{1}{2} C(40)^2}{\frac{1}{2} C(50)^2}=\left(\frac{40}{50}\right)^2=0.64\)