Q2. An inductor of inductance L, a capacitor of capacitance C, and a resistor of resistance R are connected in series to an ac source of potential difference V volts as shown in the figure. The potential difference across L, C, and R is 40V, 10V, and 40V, respectively. The amplitude of the current flowing through the LCR series circuit is A. The impedance of the circuit is :

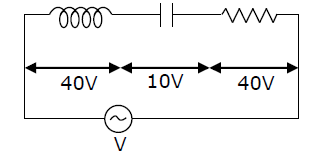
(1) \(5 \Omega\)

(2) \(4 \sqrt{2} \Omega\)

(3) \(5 / \sqrt{2} \Omega\)

(4) \(4 \Omega\)

Answer: (1)

Q3. A body is executing simple harmonic motion with frequency ‘n’, the frequency of its potential energy is :

(1) 4n

(2) n

(3) 2n

(4) 3n

Answer: (3)

Q4. A thick current-carrying cable of radius ‘R’ carries current ‘I’ uniformly distributed across its cross-section. The variation of magnetic field B(r) due to the cable with the distance ‘r’ from the axis of the cable is represented by:

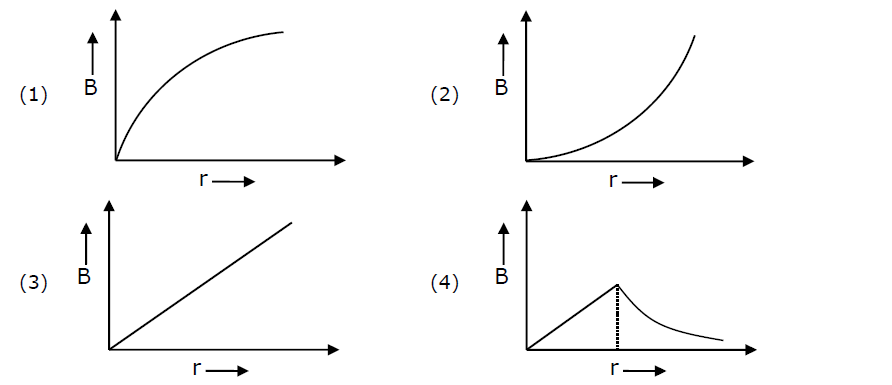
Answer: (4)

Q5. A nucleus with mass number 240 breaks into two fragments each of mass number 120, the binding per nucleon of unfragmented nuclei is 7.6MeV while that of fragments is 8.5MeV. The total gain in the Binding Energy in the process is :

(1) 216MeV

(2) 0.9MeV

(3) 9.4MeV

(4) 804MeV

Answer: (1)

Q6. A parallel plate capacitor has a uniform electric field in the space between the plates. If the distance between the plates is “d” and the area of each plate is “A”, the energy stored in the capacitor is: (= permittivity of free space).

(1) \(\frac{E^{2} A d}{\varepsilon_{0}}\)

(2) \(\frac{1}{2} \varepsilon_{0} \mathrm{E}^{2}\)

(3) \(\varepsilon_{0} \mathrm{EAd}\)

(4) \(\frac{1}{2} \varepsilon_{0} \mathrm{E}^{2} \mathrm{Ad}\)

Answer: 4

Q7. The number of photons per second on an average emitted by the source of monochromatic light of wavelength \(600 \mathrm{~nm}\), when it delivers the power of \(3.3 \times 10^{-3}\) watt will be: \(\left(\mathrm{h}=6.6 \times 10^{-34} \mathrm{Js}\right)\).

(1) \(10^{15}\)

(2) \(10^{18}\)

(3) \(10^{17}\)

(4) \(10^{16}\)

Answer: (4)

Q8. Polar molecules are the molecules:

(1) having a permanent electric dipole moment

(2) having zero dipole moment

(3) acquire a dipole moment only in the presence of an electric field due to displacement of charges

(4) acquire a dipole moment only when the magnetic field is absent

Answer: (1)

Q9. The half life of a radioactive nuclide is 100 hours. The fraction of original activity that will remain after 150 hours would be :

(1) \(\frac{2}{3 \sqrt{2}}\)

(2) \(\frac{1}{2}\)

(3) \(\frac{1}{2 \sqrt{2}}\)

(4) \(\frac{2}{3}\)

Answer: (3)

Q10. A capacitor of capacitance ‘C’, is connected across an ac source of voltage V, given by \(\mathrm{V}=\mathrm{V}_{0} \sin \omega \mathrm{t}\)
The displacement current between the plates of the capacitor, would then be given by :

(1) \(I_{d}=V_{0} \omega C \sin \omega t\)

(2) \(I_{d}=V_{0} \omega C \cos \omega t\)

(3) \(I_{d}=\frac{V_{0}}{\omega C} \cos \omega t\)

(4) \(I_{d}=\frac{V_{0}}{\omega C} \sin \omega t\)

Answer: (2)

Q11. A screw gauge gives the following readings when used to measure the diameter of a wire Main scale reading : 0 mm; Circular scale reading: 52 divisions; Given that 1 mm on the main scale corresponds to 100 divisions on the circular scale. The diameter of the wire from the above data is :

(1) 0.052 cm

(2) 0.52 cm

(3) 0.026 cm

(4) 0.26 cm

Answer: (1)

Q12. Find the value of the angle of emergence from the prism. The Refractive index of the glass is

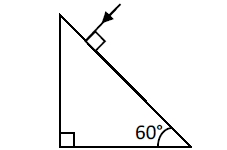
(1) 90°

(2) 60°

(3) 30°

(4) 45°

Answer: (2)

Q13. In a potentiometer circuit, a cell of EMF 1.5 V gives a balance point at 36 cm length of wire. If another cell of EMF 2.5 V replaces the first cell, then at what length of the wire, the balance point occurs?

(1) 62 cm

(2) 60 cm

(3) 21.6 cm

(4) 64 cm

Answer: (2)

Q14. A particle is released from height S from the surface of the Earth. At a certain height, its kinetic energy is three times its potential energy. The height from the surface of the earth and the speed of the particle at that instant are respectively

(1) \(\frac{S}{4}, \sqrt{\frac{3 g S}{2}}\)

(2) \(\frac{S}{4}, \frac{3 g S}{2}\)

(3) \(\frac{S}{4}, \frac{\sqrt{3 g S}}{2}\)

(4) \(\frac{S}{2}, \frac{\sqrt{3 g S}}{2}\)

Answer: (1)

Q15. The effective resistance of a parallel connection that consists of four wires of equal length, equal area of cross-section, and the same material is 0.25Ω. What will be the effective resistance if they are connected in series?
(1) 4 Ω

(2) 0.25 Ω

(3) 0.5 Ω

(4) 1 Ω

Answer: (1)

Q16. Waterfalls from a height of 60m at the rate of 15 kg/s to operate a turbine. The losses due to frictional force are 10% of the input energy. How much power is generated by the turbine?
(g = 10 m/)

(1) 7.0 kW

(2) 10.2kW

(3) 8.1 kW

(4) 12.3 kW

Answer: (3)

Q17. An infinitely long straight conductor carries a current of \(5 \mathrm{~A}\) as shown. An electron is moving with a speed of \(10^{5} \mathrm{~m} / \mathrm{s}\) parallel to the conductor. The perpendicular distance between the electron and the conductor is 20 cm at an instant. Calculate the magnitude of the force experienced by the electron at that instant.

(1) \(8 \times 10^{-20} \mathrm{~N}\)

(2) \(4 \times 10^{-20} \mathrm{~N}\)

(3) \(8 \pi \times 10^{-20} \mathrm{~N}\)

(4) \(4 \pi \times 10^{-20} \mathrm{~N}\)

Answer: (1)

Q18. Consider the following statements (A) and (B) and identify the correct answer.

(A) A Zener diode is connected in reverse bias when used as a voltage regulator.
(B) The potential barrier of the p-n junction lies between 0.1 V to 0.3 V.

(1) (A) is incorrect but (B) is correct.

(2) (A) and (B) both are correct.

(3) (A) and (B) both are incorrect.

(4) (A) is correct and (B) is incorrect.

Answer: (4)

Q19. A spring is stretched by 5 cm by a force of 10 N. The time period of the oscillations when a mass of 2 kg is suspended by it is

(1) 0.628 s

(2) 0.0628 s

(3) 6.28 s

(4) 3.14 s

Answer: (1)

Q20. The electron concentration in an n-type semiconductor is the same as hole concentration in a p-type semiconductor. An external field (electric) is applied across each of them. Compare the currents in them.

(1) No current will flow in p-type, current will only flow in n-type

(2) Current in n-type = current in p-type

(3) current in p-type > current in n-type

(4) current in n-type > current in p-type.

Answer: (4)

Q21. A dipole is placed in an electric field as shown. In which direction will it move?

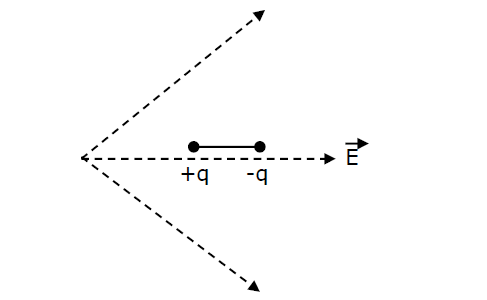
(1) towards the right as its potential energy will increase.

(2) towards the left as its potential energy will increase.

(3) towards the right as its potential energy will decrease.

(4) towards the left as its potential energy will decrease.

Answer: (3)

Q22. A convex lens ‘A’ of focal length 20 cm and a concave lens ‘B’ of focal length 5 cm are kept along the same axis with a distance ‘d’ between them. If a parallel beam of light falling on ‘A’ leaves ‘B’ as a parallel beam, then the distance ‘d’ in cm will be:

(1) 30

(2) 25

(3) 15

(4) 50

Answer: (3)

Q23. The escape velocity from the Earth’s surface is \(v\). The escape velocity from the surface of another planet having a radius, four times that of Earth and the same mass density is:

(1) \(4 v\)

(2) \(v\)

(3) \(2 v\)

(4) \(3 v\)

Answer: (1)

Q24. An electromagnetic wave of wavelength ‘\(\lambda\)‘ is incident on a photosensitive surface of negligible work function. If ‘\(\mathrm{m}^{\prime}\) mass is of photoelectron emitted from the surface has de-Broglie wavelength \(\lambda_{d}\), then:

(1) \(\lambda=\left(\frac{2 \mathrm{~h}}{\mathrm{mc}}\right) \lambda_{\mathrm{d}}{ }^{2}\)

(2) \(\lambda=\left(\frac{2 \mathrm{~m}}{\mathrm{hc}}\right) \lambda_{\mathrm{d}}{ }^{2}\)

(3) \(\lambda_{d}=\left(\frac{2 m c}{h}\right) \lambda^{2}\)

(4) \(\lambda=\left(\frac{2 \mathrm{mc}}{\mathrm{h}}\right) \lambda_{\mathrm{d}}^{2}\)

Answer: (4)

Q25. A lens of large focal length and large aperture is best suited as an objective of an astronomical telescope since:

(1) a large aperture contributes to the quality and visibility of the images.

(2) a large area of the objective ensures better light-gathering power.

(3) a large aperture provides a better resolution.

(4) All the above.

Answer: (4)

Back to Lesson

Next Lesson