8.10 Quantitative Analysis

Quantitative analysis of compounds is very important in organic chemistry. It helps chemists in the determination of mass per cent of elements present in a compound. You have learnt in Unit-1 that mass per cent of elements is required for the determination of emperical and molecular formula.

The percentage composition of elements present in an organic compound is determined by the following methods:

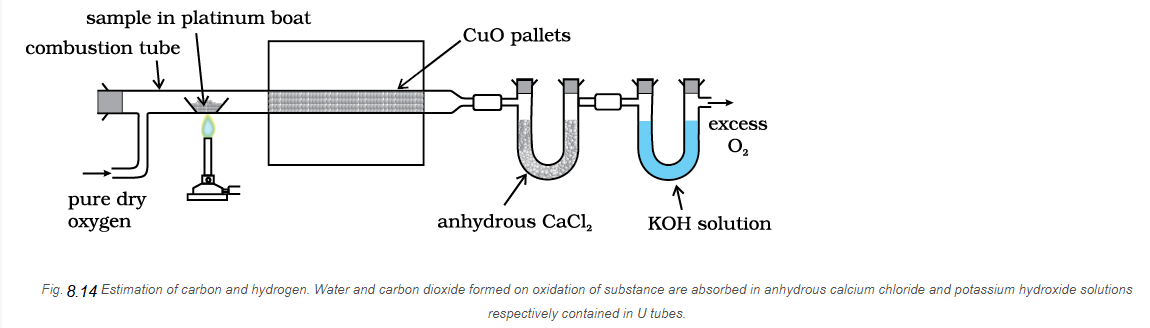
Both carbon and hydrogen are estimated in one experiment. A known mass of an organic compound is burnt in the presence of excess of oxygen and copper(II) oxide. Carbon and hydrogen in the compound are oxidised to carbon dioxide and water respectively.
\(
\mathrm{C}_{\mathrm{x}} \mathrm{H}_{\mathrm{y}}+(\mathrm{x}+\mathrm{y} / 4) \mathrm{O}_2 \longrightarrow \mathrm{x} \mathrm{CO}_2+(\mathrm{y} / 2) \mathrm{H}_2 \mathrm{O}
\)
The mass of water produced is determined by passing the mixture through a weighed U-tube containing anhydrous calcium chloride. Carbon dioxide is absorbed in another U-tube containing concentrated solution of potassium hydroxide. These tubes are connected in series (Fig. 8.14). The increase in masses of calcium chloride and potassium hydroxide gives the amounts of water and carbon dioxide from which the percentages of carbon and hydrogen are calculated.

Let the mass of organic compound be \(\mathrm{m} \mathrm{g}\), mass of water and carbon dioxide produced be \(m_1\) and \(m_2\) g respectively;
\(
\begin{aligned}
& \text { Percentage of carbon }=\frac{12 \times m_2 \times 100}{44 \times m} \\
& \text { Percentage of hydrogen }=\frac{2 \times m_1 \times 100}{18 \times m}
\end{aligned}
\)

Example 8.20: On complete combustion, \(0.246 \mathrm{~g}\) of an organic compound gave \(0.198 \mathrm{~g}\) of carbon dioxide and \(0.1014 \mathrm{~g}\) of water. Determine the percentage composition of carbon and hydrogen in the compound.

Answer:

\(
\text { Percentage of carbon }=\frac{12 \times 0.198 \times 100}{44 \times 0.246}=21.95 \%
\)
\(
\text { Percentage of hydrogen }=\frac{2 \times 0.1014 \times 100}{18 \times 0.246}=4.58 \%
\)

Nitrogen

There are two methods for estimation of nitrogen: (i) Dumas method and (ii) Kjeldahl’s method.
(i) Dumas method: The nitrogen containing organic compound, when heated with copper oxide in an atmosphere of carbon dioxide, yields free nitrogen in addition to carbon dioxide and water.

\(
\mathrm{C}_{\mathrm{x}} \mathrm{H}_{\mathrm{y}} \mathrm{N}_{\mathrm{z}}+(2 \mathrm{x}+\mathrm{y} / 2) \mathrm{CuO} \longrightarrow \mathrm{x} \mathrm{CO}_2+\mathrm{y} / 2 \mathrm{H}_2 \mathrm{O}+\mathrm{z} / 2 \mathrm{~N}_2+(2 \mathrm{x}+\mathrm{y} / 2) \mathrm{Cu}
\)
Traces of nitrogen oxides formed, if any, are reduced to nitrogen by passing the gaseous mixture over a heated copper gauze. The mixture of gases so produced is collected over an aqueous solution of potassium hydroxide which absorbs carbon dioxide. Nitrogen is collected in the upper part of the graduated tube (Fig.8.15).

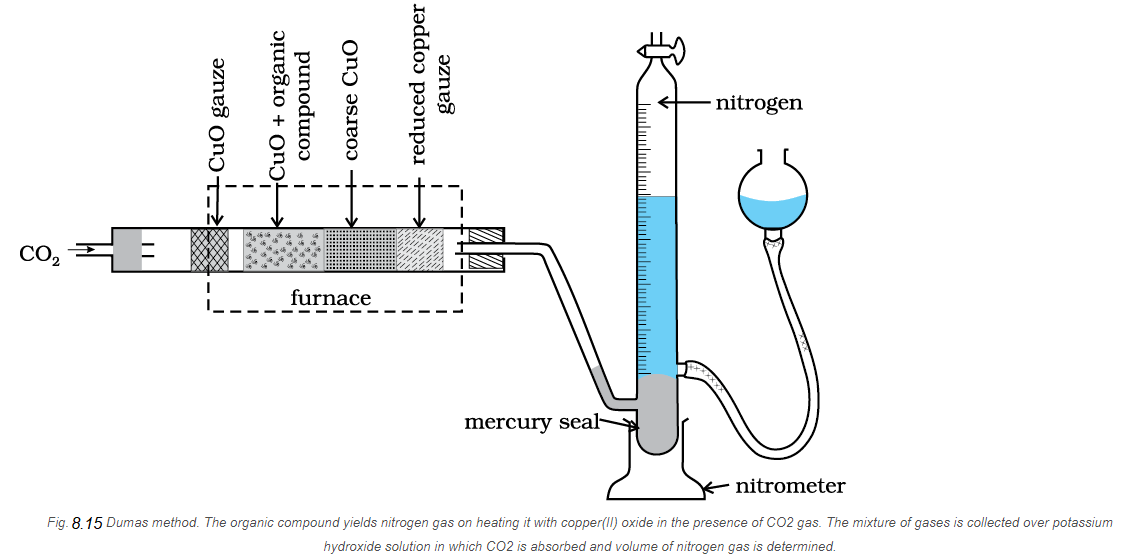
Let the mass of organic compound \(=m \mathrm{~g}\) Volume of nitrogen collected \(=V_1 \mathrm{~mL}\) Room temperature \(=T_1 \mathrm{~K}\)
\(
\text { Volume of nitrogen at } \mathrm{STP}=\frac{P_1 V_1 \times 273}{760 \times T_1} \text { (Let it be } V \mathrm{~mL} \text { ) }
\)
Where \(p_1\) and \(V_1\) are the pressure and volume of nitrogen, \(p_1\) is different from the atmospheric pressure at which nitrogen gas is collected. The value of \(p_1\) is obtained by the relation;
\(p_1=\) Atmospheric pressure – Aqueous tension \(22400 \mathrm{~mL} \mathrm{~N}_2\) at STP weighs \(28 \mathrm{~g}\).
\(
\begin{aligned}
& V \mathrm{~mL} \mathrm{~N}_2 \text { at STP weighs }=\frac{28 \times V}{22400} \mathrm{~g} \\
& \text { Percentage of nitrogen }=\frac{28 \times V \times 100}{22400 \times m}
\end{aligned}
\)

Example 8.21: In Dumas’ method for estimation of nitrogen, \(0.3 \mathrm{~g}\) of an organic compound gave \(50 \mathrm{~mL}\) of nitrogen collected at \(300 \mathrm{~K}\) temperature and \(715 \mathrm{~mm}\) pressure. Calculate the percentage composition of nitrogen in the compound. (Aqueous tension at \(300 \mathrm{~K}=15 \mathrm{~mm}\) )

Answer: Volume of nitrogen collected at \(300 \mathrm{~K}\) and \(715 \mathrm{~mm}\) pressure is \(50 \mathrm{~mL}\) Actual pressure \(=715-15=700 \mathrm{~mm}\)
Volume of nitrogen at STP = \(\frac{273 \times 700 \times 50}{300 \times 760}\) = \(41.9 \mathrm{~mL}\)
\(22,400 \mathrm{~mL}\) of \(\mathrm{N}_2\) at STP weighs \(=28 \mathrm{~g}\)
\(
\begin{aligned}
& 41.9 \mathrm{~mL} \text { of nitrogen weighs }=\frac{28 \times 41.9}{22400} \mathrm{~g} \\
& \begin{aligned}
\text { Percentage of nitrogen } & =\frac{28 \times 41.9 \times 100}{22400 \times 0.3} \\
& =17.46 \%
\end{aligned}
\end{aligned}
\)

(ii) Kjeldahl’s method: The compound containing nitrogen is heated with concentrated sulphuric acid. Nitrogen in the compound gets converted to ammonium sulphate (Fig. 8.16). The resulting acid mixture is then heated with excess of sodium hydroxide. The liberated ammonia gas is absorbed in an excess of standard solution of sulphuric acid. The amount of ammonia produced is determined by estimating the amount of sulphuric acid consumed in the reaction. It is done by estimating unreacted sulphuric acid left after the absorption of ammonia by titrating it with standard alkali solution. The difference between the initial amount of acid taken and that left after the reaction gives the amount of acid reacted with ammonia.

\(
\text { Organic compound }+\mathrm{H}_2 \mathrm{SO}_4 \longrightarrow\left(\mathrm{NH}_4\right)_2 \mathrm{SO}_4 \xrightarrow{2 \mathrm{NaOH}} \mathrm{Na}_2 \mathrm{SO}_4+2 \mathrm{NH}_3+2 \mathrm{H}_2 \mathrm{O}
\)
\(
2 \mathrm{NH}_3+\mathrm{H}_2 \mathrm{SO}_4 \longrightarrow\left(\mathrm{NH}_4\right)_2 \mathrm{SO}_4
\)
Let the mass of organic compound taken \(=\mathrm{mg}\) Volume of \(\mathrm{H}_2 \mathrm{SO}_4\) of molarity, M, taken \(=\mathrm{VmL}\)
Volume of \(\mathrm{NaOH}\) of molarity, M, used for titration of excess of \(\mathrm{H}_2 \mathrm{SO}_4=V_1 \mathrm{~mL}\) \(V_1 \mathrm{~mL}\) of \(\mathrm{NaOH}\) of molarity \(\mathrm{M}\)
\(=V_1 / 2 \mathrm{~mL}\) of \(\mathrm{H}_2 \mathrm{SO}_4\) of molarity \(\mathrm{M}\)

Volume of \(\mathrm{H}_2 \mathrm{SO}_4\) of molarity \(M\) unused \(=\left(V-V_1 / 2\right) \mathrm{mL}\)
\(\left(V-V_1 / 2\right) \mathrm{mL}\) of \(\mathrm{H}_2 \mathrm{SO}_4\) of molarity \(\mathrm{M}\)
\(=2\left(V-V_1 / 2\right) \mathrm{mL}\) of \(\mathrm{NH}_3\) solution of molarity M.
\(1000 \mathrm{~mL}\) of \(1 \mathrm{M} \mathrm{NH}_3\) solution contains \(17 \mathrm{~g} \mathrm{NH}_3\) or \(14 \mathrm{~g}\) of \(\mathrm{N}\)
\(2\left(V-V_1 / 2\right) \mathrm{mL}\) of \(\mathrm{NH}_3\) solution of molarity \(\mathrm{M}\) contains:
\(
\frac{14 \times \mathrm{M} \times 2\left(\mathrm{~V}-\mathrm{V}_1 / 2\right)}{1000} \mathrm{~g} \mathrm{~N}
\)
\(
\text { Percentage of } \mathrm{N}=\frac{14 \times \mathrm{M} \times 2\left(\mathrm{~V}-\mathrm{V}_1 / 2\right)}{1000} \times \frac{100}{m}=\frac{1.4 \times \mathrm{M} \times 2(\mathrm{~V}-\mathrm{V_1} / 2)}{m}
\)
Kjeldahl method is not applicable to compounds containing nitrogen in nitro and azo groups and nitrogen present in the ring (e.g. pyridine) as nitrogen of these compounds does not change to ammonium sulphate under these conditions.

Example 8.22: During estimation of nitrogen present in an organic compound by Kjeldahl’s method, the ammonia evolved from \(0.5 \mathrm{~g}\) of the compound in Kjeldahl’s estimation of nitrogen, neutralized \(10 \mathrm{~mL}\) of \(1 \mathrm{M} \mathrm{H}_2 \mathrm{SO}_4\). Find out the percentage of nitrogen in the compound.

Answer: \(1 \mathrm{M}\) of \(10 \mathrm{~mL} \mathrm{H}_2 \mathrm{SO}_4=1 \mathrm{M}\) of \(20 \mathrm{~mL} \mathrm{NH}_3\)
\(1000 \mathrm{~mL}\) of \(1 \mathrm{M}\) ammonia contains \(14 \mathrm{~g}\) nitrogen
\(20 \mathrm{~mL}\) of \(1 \mathrm{M}\) ammonia contains \(\frac{14 \times 20}{1000} \mathrm{~g}\) nitrogen
Percentage of nitrogen \(=\frac{14 \times 20 \times 100}{1000 \times 0.5}=56.0 \%\)

Halogens

Carius method: A known mass of an organic compound is heated with fuming nitric acid in the presence of silver nitrate contained in a hard glass tube known as Carius tube, (Fig.8.17) 

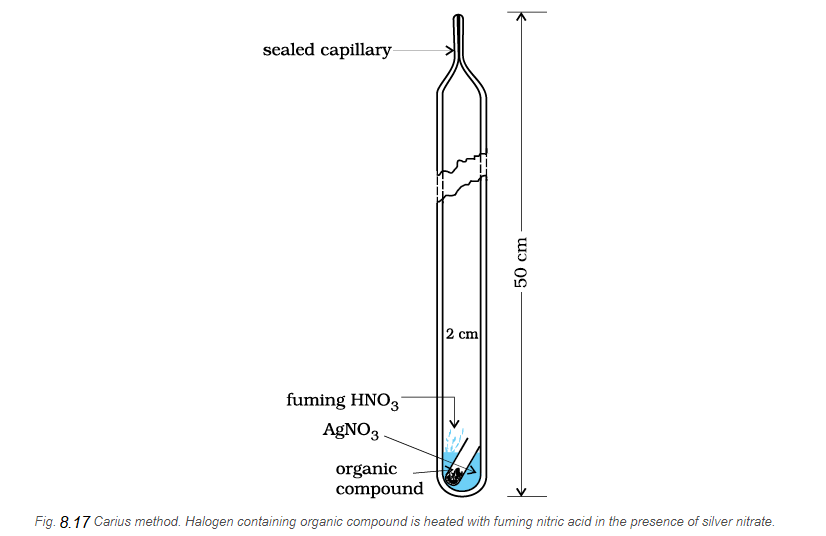
in a furnace. Carbon and hydrogen present in the compound are oxidised to carbon dioxide and water. The halogen present forms the corresponding silver halide (AgX). It is filtered, washed, dried and weighed.
Let the mass of organic compound taken \(=\mathrm{m} \mathrm{g}\) Mass of \(\mathrm{AgX}\) formed \(=\mathrm{m}_1 \mathrm{~g}\) \(1 \mathrm{~mol}\) of AgX contains \(1 \mathrm{~mol}\) of \(\mathrm{X}\)
\(
\begin{array}{r}
\text { Mass of halogen in } \mathrm{m}_1 \mathrm{~g} \text { of } \mathrm{AgX} \\
=\frac{\text { atomic mass of } \mathrm{X} \times \mathrm{m}_1 \mathrm{~g}}{\text { molecular mass of } \mathrm{AgX}}
\end{array}
\)
Percentage of halogen
\(
=\frac{\text { atomic mass of } \mathrm{X} \times \mathrm{m}_1 \mathrm{~g}}{\text { molecular mass of } \mathrm{AgX}}
\)

Example 8.23: In Carius method of estimation of halogen, \(0.15 \mathrm{~g}\) of an organic compound gave \(0.12 \mathrm{~g}\) of \(\mathrm{AgBr}\). Find out the percentage of bromine in the compound.

Answer:

\(
\text { Molar mass of } \mathrm{AgBr}=108+80=188 \mathrm{~g} \mathrm{~mol}^{-1}
\)
\(188 \mathrm{~g} \mathrm{AgBr}\) contains \(80 \mathrm{~g}\) bromine
\(0.12 \mathrm{~g} \mathrm{AgBr}\) contains \(\frac{80 \times 0.12}{188} \mathrm{~g}\) bromine
\(
\text { Percentage of bromine }=\frac{80 \times 0.12 \times 100}{188 \times 0.15}=34.04 \%
\)

Sulphur

A known mass of an organic compound is heated in a Carius tube with sodium peroxide or fuming nitric acid. Sulphur present in the compound is oxidised to sulphuric acid. It is precipitated as barium sulphate by adding excess of barium chloride solution in water. The precipitate is filtered, washed, dried and weighed. The percentage of sulphur can be calculated from the mass of barium sulphate.

Let the mass of organic compound taken \(=\mathrm{mg}\)
and the mass of barium sulphate formed \(=\mathrm{m}_1 \mathrm{~g}\)
\(
1 \mathrm{~mol} \text { of } \mathrm{BaSO}_4=233 \mathrm{~g} \mathrm{~BaSO}_4=32 \mathrm{~g} \text { sulphur }
\)
\(
\mathrm{m}_1 \mathrm{~g} \mathrm{BaSO}_4 \text { contains } \frac{32 \times m_1}{233} \mathrm{~g} \text { sulphur }
\)
\(
\text { Percentage of sulphur }=\frac{32 \times m_1 \times 100}{233 \times m}
\)

Example 8.24: In sulphur estimation, \(0.157 \mathrm{~g}\) of an organic compound gave \(0.4813 \mathrm{~g}\) of barium sulphate. What is the percentage of sulphur in the compound?

Answer: Molecular mass of \(\mathrm{BaSO}_4=137+32+64 =233 \mathrm{~g}\)
\(
233 \mathrm{~g} \mathrm{BaSO}_4 \text { contains } 32 \mathrm{~g} \text { sulphur }
\)
\(
0.4813 \mathrm{~g} \mathrm{BaSO}_4 \text { contains } \frac{32 \times 0.4813}{233} \mathrm{~g} \text { sulphur }
\)
Percentage of sulphur \(=\frac{32 \times 0.4813 \times 100}{233 \times 0.157}=42.10 \%\)

Phosphorus

A known mass of an organic compound is heated with fuming nitric acid whereupon phosphorus present in the compound is oxidised to phosphoric acid. It is precipitated as ammonium phosphomolybdate, \(\left(\mathrm{NH}_4\right)_3\) \(\mathrm{PO}_4 \cdot 12 \mathrm{MoO}_3\), by adding ammonia and ammonium molybdate. Alternatively, phosphoric acid may be precipitated as \(\mathrm{MgNH}_4 \mathrm{PO}_4\) by adding magnesia mixture which on ignition yields \(\mathrm{Mg}_2 \mathrm{P}_2 \mathrm{O}_7\).
Let the mass of organic compound taken \(=\mathrm{mg}\) and mass of ammonium phospho molydate \(=\mathrm{m}_1 \mathrm{~g}\)
Molar mass of \(\left(\mathrm{NH}_4\right)_3 \mathrm{PO}_4 \cdot 12 \mathrm{MoO}_3=1877 \mathrm{~g}\)
Percentage of phosphorus \(=\frac{31 \times m_1 \times 100}{1877 \times m} \%\)
If phosphorus is estimated as \(\mathrm{Mg}_2 \mathrm{P}_2 \mathrm{O}_7\),
Percentage of phosphorus \(=\frac{62 \times m_1 \times 100}{222}\)
where, \(222 \mathrm{u}\) is the molar mass of \(\mathrm{Mg}_2 \mathrm{P}_2 \mathrm{O}_7\), \(\mathrm{m}\), the mass of organic compound taken, \(m_1\), the mass of \(\mathrm{Mg}_2 \mathrm{P}_2 \mathrm{O}_7\) formed and 62 , the mass of two phosphorus atoms present in the compound \(\mathrm{Mg}_2 \mathrm{P}_2 \mathrm{O}_7\).

Oxygen

The percentage of oxygen in an organic compound is usually found by difference between the total percentage composition (100) and the sum of the percentages of all other elements. However, oxygen can also be estimated directly as follows:

A definite mass of an organic compound is decomposed by heating in a stream of nitrogen gas. The mixture of gaseous products containing oxygen is passed over red-hot coke when all the oxygen is converted to carbon monoxide. This mixture is passed through warm iodine pentoxide \(\left(\mathrm{I}_2 \mathrm{O}_5\right)\) when carbon monoxide is oxidised to carpon dioxide producing iodine.
\(
\text { Compound } \xrightarrow{\text { heat }}\mathrm{O}_2+\text { other gaseous products}
\)
\(
2 \mathrm{C}+\mathrm{O}_2 \xrightarrow{1373 \mathrm{~K}} 2 \mathrm{CO}] \times 5 \dots(A)
\)
\(
\mathrm{I}_2 \mathrm{O}_5+5 \mathrm{CO} \longrightarrow \mathrm{I}_2+\left.5 \mathrm{CO}_2\right] \times 2 \dots(B)
\)
On making the amount of \(\mathrm{CO}\) produced in equation (A) equal to the amount of \(\mathrm{CO}\) used in equation (B) by multiplying the equations (A) and (B) by 5 and 2 respectively; we find that each mole of oxygen liberated from the compound will produce two moles of carbondioxide.
Thus \(88 \mathrm{~g}\) carbon dioxide is obtained if \(32 \mathrm{~g}\) oxygen is liberated.
Let the mass of organic compound taken be \(\mathrm{mg}\) Mass of carbon dioxide produced be \(m_1 g\)
\(\therefore \mathrm{m}_1 \mathrm{~g}\) carbon dioxide is obtained from
\(
\frac{32 \times m_1}{88} \mathrm{gO}_2
\)
\(
\therefore \text { Percentage of oxygen }=\frac{32 \times m_1 \times 100}{88 \times m} \%
\)
The percentage of oxygen can be derived from the amount of iodine produced also.

Presently, the estimation of elements in an organic compound is carried out by using microquantities of substances and automatic experimental techniques. The elements, carbon, hydrogen and nitrogen present in a compound are determined by an apparatus known as CHN elemental analyser. The analyser requires only a very small amount of the substance (1-3 \(\mathrm{mg}\) ) and displays the values on a screen within a short time.