7.6 Exercise Problems and Solutions
Exercises
Q1. Assign oxidation number to the underlined elements in each of the following species:
(a) NaH2P_O4
(b) NaHS_O4
(c) H4P_2O7
(d) K2Mn_O4
(e) CaO_2
(f) NaB_H4
(g) H2S_2O7
(h) KAl(S_O4)2⋅12H2O
Answer: Let the oxidation no. of underlined element in all the given compounds =x
(a) We know that, Oxidation number of Na=+1
Oxidation number of H=+1
Oxidation number of O=−2
+1+1x−2
Na H2 PO4
Since the sum of oxidation number of various atoms in NaH2PO4 (neutral) is zero.
Thus, the oxidation number of P in NaH2PO4 =+5.
(b) +1+1x−2
In NaHSO4:1(+1)+1(+1)+x+4(−2)=0
Therefore, x=+6
Thus, the oxidation number of S in NaHSO4=+6.
(c) +1x−2
In H4P2O7:4(+1)+2(x)+7(−2)=0
Therefore, x=+5
Thus, the oxidation number of P in H4P2O7=+5.
(d) +1x−2
In K2MnO4:2(+1)+1(x)+4(−2)=0
Therefore, x=+6
Thus, the oxidation number of Mn in K2MnO4 =+6.
(e) +2x
In CaO2:2+2x=0 or x=−1
Thus, the oxidation number of oxygen in CaO2=−1
(f) In NaBH4, hydrogen is present as hydride ion. Therefore, its oxidation number is -1 . Thus,
+1x−1
In NaBH4:1(+1)+x+4(−1)=0 or x=+3
Thus, the oxidation number of B in NaBH4=+3
(g) +1x−2
lnH2 S2O7:2(+1)+2(x)+7(−2)=0
Therefore, x=+6
Thus, the oxidation number of S in H2 S2O7=+6.
(h) +1+3x−2
In KAl(SO4)2⋅12H2O :
+1+3+2x+8(−2)+12×0=0 or x=+6
Thus, the oxidation number of S in
KAl(SO4)2⋅12H2O=+6
Q2. What are the oxidation number of the underlined elements in each of the following and how do you rationalise your results ?
(a) KI_3
(b) H2S_4O6
(c) Fe_3O4
(d) C_H3C_H2OH
(e) C_H3C_OOH
Answer: (a) In KI3, since the oxidation number of K is +1 , therefore, the average oxidation number of iodine =−1/3. In the structure, K+[I−I<−I]−, a coordinate bond is formed between I2 molecule and I ion. The oxidation number of two iodine atoms forming the 12 molecule is zero while that of iodine ion forming the coordinate bond is -1 . Thus, the O.N. of three iodine atoms in Kl3 are 0,0 and -1 respectively.
(b) The structure of H2 S4O6 is shown below:
The O.N. of each of the S atoms linked with each other in the middle is zero while that of each of the remaining two S-atoms is +5.
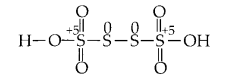
(c) x−2
Fe3O4
Let O.N. of Fe=x, then 3x+4(−2)=0 or x=+83 (average)
By stoichiometry Fe3O4 is
+2−2+3−2
Fe O⋅Fe2O3.
Thus, Fe has O.N. of +2 and +3.
(d)

In this molecule, C−2 is attached to three H-atoms (less electronegative than carbon) and one CH2OH group (more electronegativity than carbon).
Therefore O.N. of C-2 =3(+1)+x+1(−1)=0 or x=−2.
C−1 is however, attached to one OH (charge =−1 ) and one CH3 (charge =+1 ), and two H-atoms, O.N. of +1 .
Therefore, O.N. of C-1 =1(+1)+2(+1)+x+1(−1)=0 or x=−2
(e)

In this molecule, C−2 is attached to three H-atoms (less electronegative than carbon) and one-COOHgroup(more electronegativity than carbon).
Therefore, O.N. of C-2 =3(+1)+x+1(−1)=0 or x=−2.
C-1 is, however, attached to one oxygen atom by a double bond, one OH (charge =−1 ) and one CH3 (charge =+1 ) group, therefore, O.N. of C-1 = 1(+1)+x+1(−2)+1(−1)=0 or x=+2.
Q3. Justify that the following reactions are redox reactions:
(a) CuO(s)+H2( g)→Cu(s)+H2O(g)
(b) Fe2O3( s)+3CO(g)→2Fe(s)+3CO2( g)
(c) 4BCl3( g)+3LiAlH4( s)→2 B2H6( g)+3LiCl(s)+3AlCl3( s)
(d) 2 K( s)+F2( g)→2 K+F−(s)
(e) 4NH3( g)+5O2( g)→4NO(g)+6H2O(g)
Answer: (a)
+2−200+2−2
CuO(s)+H2(g)→Cu(s)+H2O(g)
O.N. of Cu decreases from +2 in CuO to 0 in Cu but that of H increases from 0 in H2 to +1 in H2O. Therefore, CuO is reduced to Cu but H2 is oxidised to H2O. Thus, this is a redox reaction.
(b)
+3−2+20+4
Fe2O3(s)+3CO(g)→2Fe(s)+3CO2(g)
O.N. of Fe decreases from +3 in Fe2O3 to 0 in Fe while that of C increases from +2 in CO to +4 in CO2. Thus, Fe2O3 is reduced and CO is oxidised. Thus, this is a redox reaction.
(c)
+3−1+1+3−1−3+1+1−1+3−1
4BCl3(g)+3LiAlH4(s)→2 B2H6(g)+3LiCl(s)+3AlCl3(s)
O.N. of B decreases from +3 in BCl3 to -3 in B2H6 while that of H increases from -1 in LiAlH4 to +1 in B2H6. Therefore, BCl3 is reduced while LiAlH4 is oxidised. Thus, this is a redox reaction.
(d)
2 K(s)+F2(g)→2 K+F−(s)
Each K atom has lost one electron to form K+ while F2 has gained two electrons to form two F−ions. Therefore, K is oxidised while F2 is reduced. Thus, it is a redox reaction.
(e)
−3+10+2−2+1−2
4NH3(g)+5O2(g)→4NO(g)+6H2O(g)
O.N. of N increases from -3 in NH3 to +2 in NO while that of O decreases from 0 in O2 to -2 in NO or H2O. Therefore, NH3 is oxidised while O2 is reduced. Thus, it is a redox reaction.
Q4. Fluorine reacts with ice and results in the change:
H2O(s)+F2( g)→HF(g)+HOF(g)
Justify that this reaction is a redox reaction.
Answer:
+1−20+1−1+1−2+1
H2O(s)+F2( g)→HF(g)+HOF(g)
Since fluorine can undergo oxidation as well as reduction hence, it is an example of redox reaction.
Q5. Calculate the oxidation number of sulphur, chromium and nitrogen in H2SO5. Cr2O2−7 and NO−3. Suggest structure of these compounds. Count for the fallacy.
Answer: Oxidation number of sulphur in H2SO5 :
Let the oxidation number of S=x then (+1)×2+x+(−2)×5=0 or 2+x−10=0
⇒x−8=0∴x=+8
The maximum O.N. of S cannot be more than 6 since it has only 6 electrons in the valence shell. This fallacy is overcome if we calculate the O.N. of sulphur by chemical bonding method. The structure of H2SO5 is
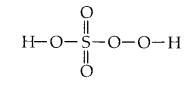
It has two peroxide oxygen with O.N. =−1 and three oxygens with O.N. =−2
Thus, 2×(+1)+x+2(−1)+3×(−2)=0 +2+x−2−6=0⇒x−6=0⇒x=+6 Thus, O.N. of sulphur in H2SO5=+6
Oxidtion number of chromium in Cr2O2−7 :
Let the oxidation number of chromium =x
∴2x+7(−2)=−2⇒2x−14=−2⇒2x=−2+14⇒2x=+12⇒x=+6
Thus, the oxidation number of chromium =+6
Oxidation number of nitrogen in NO−3:
Let the oxidation number of nitrogen =x then x+(−2)×3=−1⇒x−6=−1 ⇒x=−1+6⇒x=+5 Thus, the oxidation number of nitrogen =+5
Q6. Write formulas for the following compounds:
(a) Mercury(II) chloride
(b) Nickel(II) sulphate
(c) Tin{IV}) oxide
(d) Thallium(I) sulphate
(e) Iron(III) sulphate
(f) Chromium(III) oxide
Answer: (a) HgCl2
(b) NiSO4
(c) SnO2
(d) Tl2SO4
(e) Fe2(SO4)3
(f) Cr2O3
Q7. Suggest a list of the substances where carbon can exhibit oxidation states from -4 to +4 and nitrogen from -3 to +5 .
Answer:
Compound O.N. of Carbon Compound O.N. of Nitrogen CH4−4NH3NH4Cl−3CH3−CH3−3NH2−NH2−2CH2=CH2 or CH3Cl−2NH=NH,NH2OH−1CH≡CH−1 N≡N0C6H12O60 N2O+1C6Cl6+1 NO +2CHCl3+2N2O3HNO2+3(COOH)2+3 N2O4+4CCl4,CO2+4N2O5HNO3+5Q8. While sulphur dioxide and hydrogen peroxide can act as oxidising as well as reducing agents in their reactions, ozone and nitric acid act only as oxidants. Why ?
Answer: The oxidation state of sulphur in sulphur dioxide is +4 . It can be oxidised to +6 oxidation state or reduced to +2. Therefore, sulphur dioxide acts as a reducing agent as well as oxidising agent. Similarly, the oxidation state of oxygen in hydrogen peroxide is – 1 . It can be oxidised to O2 (zero oxidation state) or reduced to H2O or OH−(−2 oxidation state) and therefore, acts as reducing as well as oxidising agents. However, both ozone and nitric acid can only decrease their oxidation number and therefore, act only as oxidising agents.

NH=NH,NH2
Q9. Consider the reactions:
(a) 6CO2 (g) +6H2O(l)→C6H12O6(aq)+6O2( g)
(b) O3 (g) +H2O2(l)→H2O(l)+2O2 (g)
Why it is more approprlate to write these reactions as :
(a) 6CO2( g)+12H2O(l)→C6H12O6(aq)+6H2O(l)+6O2( g)
(b) O3( g)+H2O2(l)→H2O(l)+O2( g)+O2( g)
Also suggest a technique to investigate the path of the above (a) and (b) redox reactions.
Answer: (a) Most accepted mechanism of photosynthesis is supposed to occur in two steps. In the first step, H2O decomposes to give H2 and O2 in presence of chlorophyll and the H2 thus produced reduces CO2 to C6H12C6
and H2O molecules are also produced as shown below:
12H2O(l)→12H2(g)+6O2(g)…(i)
6CO2(g)+12H2(g)→C6H12O6(s)+6H2O(l)…(ii)
¯6CO2(g)+12H2O(l)→C6H12O6(s)+6H2O(l)+6O2(g)…(iii)
Therefore, it is more appropriate to write the equation for photosynthesis as (iii) because it emphasises that 12 molecules of H2O are used per molecule of carbohydrate formed and 6 molecules of H2O are produced during the process.
(b) The purpose of writing O2 two times suggests that O2 is being obtained from each of the two reactants.
O3(g)⟶O2(g)+O(g)H2O2(g)+O(g)⟶H2O(l)+O2(g)O3(g)+H2O2(l)⟶H2O(l)+O2(g)+O2(g)
The path of reactions (a) and (b) can be determined by tracer technique method using labelled H or O,H2O18 or H2O in reaction (a) or by using H2O182 or O183 in reaction (b).
Q10. The compound AgF2 is unstable compound. However, if formed, the compound acts as a very strong oxidising agent. Why ?
Answer: The oxidation state of Ag in AgF2 is +2 . But, +2 is an unstable oxidation state of Ag. Therefore, whenever AgF2 is formed, silver readily accepts an electron to form Ag+.
Ag2++e−→Ag+
This helps to bring the oxidation state of Ag down from +2 to a more stable state of +1 . As a result, AgF2 acts as a very strong oxidizing agent.
Q11. Whenever a reaction between an oxidising agent and a reducing agent is carried out, a compound of lower oxddation state is formed if the reducing agent is in excess and a compound of higher oxidation state is formed if the oxidising agent is in excess. Justify this statement giving three illustrations.
Answer: (i) C is a reducing agent while O2 is an oxidising agent. If excess of carbon is burnt in a limited supply of O2, CO is formed in which oxidation state of C is +2 but when O2 is in excess CO formed gets oxidised to CO2 in which oxidation state of C is +4.
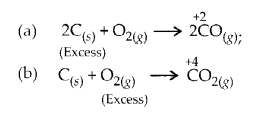
(ii) P4 is a reducing agent while Cl2 is an oxidising agent. When excess of P4 is used, PCl3 is formed in which the oxidation state of P is +3 . When excess of Cl2 is used, the initially formed PCl3 reacts further to form PCl5 in which the oxidation state of P is +5.
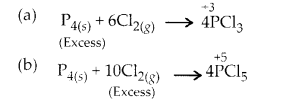
(iii) Na is a reducing agent and O2 is an oxidising agent. When excess of Na is used, sodium oxide is formed in which the oxidation state of O is -2 . If, excess of O2 is used, Na2O2 is formed in which the oxidation state of O is -1.
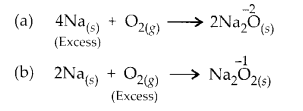
Q12. How do you count for the following observations?
(a) Though alkaline potassium permanganate and acidic potassium permanganate both are used as oxidants, yet in the manufacture of benzoic acid from toluene we use alcoholic potassium permanganate as an oxidant. Why ? Write a balanced redox equation for the reaction.
(b) When concentrated sulphuric acid is added to an inorganic mixture containing chloride, we get colourless pungent smelling gas HCl, but if the mixture contains bromide then we get red vapour of bromine. Why ?
Answer:(a) In neutral medium, KMnO4 acts as an oxidant as follows:
MnO4−+2H2O+3e−→MnO2+40H−
In laboratory, alkaline KMnO4 is used to oxidise toluene to benzoic acid.
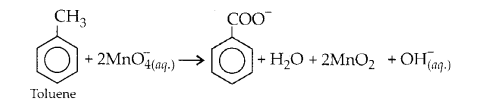
In industry alcoholic KMnO4 is preferred due to following reasons :
1. Cost of adding a base or an acid is avoided as a base ( OH−ions) is produced during the reaction itself.
2. An organic polar solvent, ethyl alcohol, helps in mixing of two reactants KMnO4 (due to its polar nature) and toluene (because, an organic compound).
(b) When a chloride such as NaCl is heated with conc. H2SO4,HCl is evolved which is not a good reducing agent and is not oxidised with conc. H2SO4
NaCl+H2SO4Δ⟶NaHSO4+HCl
But when NaBr is heated with conc. H2SO4, HBr produced being a good reducing agent is oxidised by conc. H2SO4 to give Br2 (red vapours).
H2SO4(aq) heat →SO2(g)+H2O(l)+[O]
NaBr(s)+H2SO4(aq) heat →NaHSO4(aq)+HBr(g)
2HBr(g)+[O]⟶H2O(l)+Br2(g)
Q13. Identify the substance oxidised reduced, oxidising agent and reducing agent for each of the following reactions:
(a) 2AgBr (s) +C6H6O2 (aq) →2Ag (s) +2HBr (aq) +C6H4O2 (aq)
(b) HCHO(l)+2[Ag(NH3)2]+(aq)+3OH−(aq)→2Ag(s)+HCOO−(aq)+4NH3(aq)+2H2O(l)
(c) HCHO(l)+2Cu2+(aq)+5OH−(aq)→Cu2O(s)+HCOO−(aq)+3H2O(l)
(d) N2H4(l)+2H2O2(l)→N2( g)+4H2O(l)
(e) Pb (s) +PbO2 (s) +2H2SO4 (aq) →2PbSO4 (s) +2H2O(l)
Answer:
Reducing agent (Substance oxidised) Oxidising agent (Substance reduced) (a) C6H6O2 (aq) AgBr(s) (b) HCHO(l)[Ag(NH3)2]+(aq) (c) HCHO(l)Cu2+(aq) (d) N2H4(l)H2O2(l) (e) Pb(s)PbO2(s)Oxidising agent (Substance
Q14. Consider the reactions :
2 S2O2−3(aq)+I2( s)→S4O2−6(aq)+2I−(aq)
S2O2−3(aq)+2Br2(l)+5H2O(l)→2SO2−4(aq)+4Br−(aq)+10H+(aq)
Why does the same reductant, thiosulphate react differently with lodine and bromine?
Answer: The average O.N. of S in S2O2−3 is +2 while in S4O2−6 it is +2.5 . The O.N. of S in SO2−4 is +6 . Since Br2 is a stronger oxidising agent, it oxidises S of S2O2−3 to a higher oxidation state of +6 and hence forms SO2−4 ion. I2, however, being a weaker oxidising agent oxidises S of S2O2−3 ion to a lower oxidation state of +2.5 in S4O2−6 ion.
Q15. Justify giving reactions that among halogens, fluorine is the best oxidant and among hydrohalic compounds, hydroiodic acid is the best reductant.
Answer: The halogens (X2) have strong electron accepting tendency and have positive standard oxidation potential values. They are therefore, powerful oxidising agents. The decreasing order of oxidising powers of halogens is:
X2:F2>Cl2>Br2>I2
E⊖:+2.87 V+1.36 V+1.09 V+0.54 V
Fluorine is the strongest oxidising agent (oxidant) because it can liberate the other halogens from their respective compounds. For example,
2KCl+F2⟶2KF+Cl22KBr+F2⟶2KF+Br22KI+F2⟶2KF+I2
Among halogen acids (HX), the HI is the strongest reducing agent or reductant because it has minimum bond dissociation energy:
Halogen acid: HFHClHBrHI
Bond Dissociation: enthalpy ( kJmol−1) 566431366299
The iodination of methane is of reversible nature because HI produced in the reaction being a reducing agent converts iodomethane back to methane.
CH4+I2⟶CH3I+HICH3I+HI⟶CH4+I2
Net reaction: ¯CH4+I2⇌CH3I+HI
Q16. Why does the following reaction occur?
XeO4−6(aq)+2 F−(aq)+6H+(aq)→XeO3( g)+F2( g)+3H2O(l)
What conclusion about the compound Na4XeO6 (of which XeO4−6 is a part) can be drawn from the reaction.
Answer:
XeO4−6(aq)+2 F−(aq)+6H+(aq)→XeO3( g)+F2( g)+3H2O(l)
In this reaction, O.N. of Xe decreases from +8 in XeO4−6(perxenate ion) to +6 in XeO3 while that of F increases from -1 in F−to 0 in F2. Therefore, XeO4−6 is reduced while F−is oxidised. From this reaction it is concluded that Na4XeO6 is a stronger oxidising agent than F2.
Q17. Consider the reactions:
(a) H3PO2(aq)+4AgNO3(aq)+2H2O(l)→H3PO4(aq)+4Ag(s)+4HNO3(aq)
(b) H3PO2(aq)+2CuSO4(aq)+2H2O(l)→H3PO4(aq)+2Cu(s)+H2SO4(aq)
(c) C6H5CHO(l)+2[Ag(NH3)2]+(aq)+3OH−(aq)→C6H5COO−(aq)+2Ag(s)+4NH3(aq)+2H2O(l)
(d) C6H5CHO(l)+2Cu2+(aq)+5OH−(aq)→ No change observed.
What inference do you draw about the behaviour of Ag+and Cu2+ from these reactions?
Answer: Reactions (a) and (b) indicate that both AgNO3 and CuSO4 oxidise H3PO2 to H3PO4. Hence, both are oxidising agents. Reactions (c) and (d) suggest that [Ag(NH3)2]+ oxidises C6H5CHO (benzaldehyde) to C6H5COO (benzoate ion) but Cu2+ ions cannot oxidise C6H5CHO to C6H5COO−. Therefore, from the above reactions, we infer that Ag+ion is a stronger oxidising agent than Cu2+ ion.
Q18. Balance the following redox reactions by ion – electron method :
(a) MnO−4(aq)+I−(aq)→MnO2 (s) +I2( s) (in basic medium)
(b) MnO−4(aq) +SO2 (g) →Mn2+ (aq) +HSO−4(aq) (in acidic solution)
(c) H2O2 (aq) +Fe2+(aq)→Fe3+(aq)+H2O (l) (in acidic solution)
(d) Cr2O2−7+SO2( g)→Cr3+(aq)+SO2−4 (aq) (in acidic solution)
Answer: (a) The skeleton equation is:
MnO−4(aq)+I−(aq)→MnO2( s)+I2( s)
(i) The O.N. of the atoms involved in the equation is:
+7−2(MnO−4)+−1I−⟶+4−2MnO2+0I2
(ii) The species involved in the oxidation and reduction half reaction :
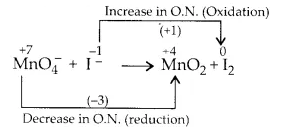
(iii) Oxidation half reaction : I−⟶I2
Reduction half reaction : MnO−4⟶MnO2
(iv) Balancing the oxidation half reaction
2I−⟶I2+2e−…(i)
(v) Balancing the reduction half reaction
(1) As the decrease in O.N. is 3, therefore, adding 3e−on the reactant side
MnO−4+3e−⟶MnO2
(2) To balance the oxygen atoms, add two H2O molecules on the product side
MnO−4+3e−⟶MnO2+2H2O
(3) To balance the charges, add 4OH−on the product side. Then to balance H atoms, add four H2O molecules on the reactant side.
MnO−4+3e−+4H2O⟶MnO2+4OH−+2H2OMnO−4+3e−+2H2O⟶MnO2+4OH−…(ii)
Thus, the reduction half reaction is balanced.
(vi) Adding the two half reactions In order to equate the electrons, multiply eqn. (i) by 3 and eqn. (ii) by 2 . Add the two equations.
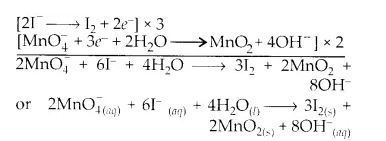
(b) The skeleton equation is:
MnO−4(aq)+SO2( g)→Mn2+(aq)+HSO−4(aq)
(i) The O.N. of atoms involved in the equation is:
+7−2+4−2+2−1+6−2
MnO−4+SO2⟶Mn2++HSO−4
(ii) The species involved in the oxidation and reduction half reactions:
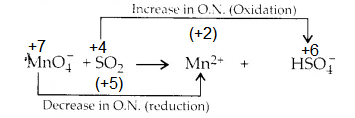
(iii) Oxidation half reaction : SO2⟶HSO−4
Reduction half reaction : MnO−4⟶Mn2+
(iv) Balancing the oxidation half reaction (1) As the increase in O.N. is 2, therefore, add two electrons on the product side to balance change in O.N.
SO2⟶HSO−4+2e−
(2). In order to balance the number of oxygen atoms, add two H2O molecules on the reactant side and then to balance H atoms add 3H+on the product side.
SO2+2H2O⟶HSO−4+3H++2e−…(i)
(v) Balancing the reduction half reaction
The reduction half reaction is :
MnO−4⟶Mn2+
(1) As the decrease in O.N. is 5, therefore add 5e−on the reactant side,
MnO−4+5e−⟶Mn2+
(2) In order to balance the no. of oxygen atoms, add four H2O molecules on the product side and then to balance H atoms add 8H+on the reactant side.
MnO−4+8H++5e−⟶Mn2++4H2O…(ii)
(vi) Adding the two half, reactions In order to equate the electrons, multiply eqn.
(i) by 5 and eqn. (ii) by 2 . Add the two eqns.

(c) Oxidation half equation :
Fe2+(aq)⟶Fe3+(aq)+e−…(i)
Reduction half equation :
H2O2(aq)+2H+(aq)+2e−⟶2H2O(l)…(ii)
Multiplying eqn. (i) by 2 and adding it to eqn. (ii), we get
H2O2(aq)+2Fe2+(aq)+2H+(aq)⟶2Fe3+(aq)+2H2O(l)
(d) Oxidation half equation :
SO2(g)+2H2O(l)⟶SO2−4(aq)+4H+(aq)+2e−…(i)
Reduction half equation :
Cr2O2−7(aq)+14H+(aq)+6e−⟶2Cr3+(aq)+7H2Ol…(ii)
Multiplying eqn. (i) by 3 and adding to eqn. (ii) we get,
Cr2O2−7(aq)+3SO2(g)+2H+(aq)⟶2Cr3+(aq)+3SO2−4(aq)+H2O(l)
Q19. Balance the following equations in basic medium by lon-electron method and oxidation number methods and identify the oxidising agent and the reducing agent.
(a) P4( s)+OH−(aq)→PH3( g)+H2PO−2(aq)
(b) N2H4(l)+ClO−3(aq)→NO(g)+Cl−(g)
(c) Cl2O7( g)+H2O2(aq)→ClO−2(aq)+O2( g)+H+
Answer: (a) Oxidation number method :
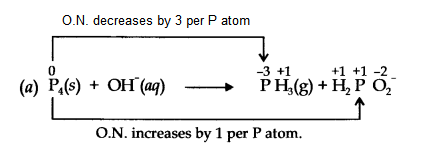
P4 acts both as an oxidising as well as a reducing agent.
Oxidation number method:
Total decrease in O.N. of P4 in PH3=3×4=12
Total increase in O.N. of P4 in H2PO−2=1×4=4
Therefore, to balance increases decreases in O.N. multiply PH3 by 1 and H2PO−2 by 3 , we have,P4( s)+OH−(aq)⟶PH3(g)+3H2PO−2(aq)
To balance O atoms, multiply OH−by 6 , we have,
P4( s)+6OH−(aq)⟶PH3(g)+3H2PO−2(aq)
To balance H atoms, add 3H2O to L.H.S. and 3OH−to the R.H.S., we have,
P4(s)+6OH−(aq)+3H2O(l)⟶PH3(g)+3H2PO−2(aq)+3OH−(aq)
P4(s)+3OH−(aq)+3H2O(l)⟶PH3(g)+3H2PO−2(aq)…(i)
Thus, Eq. (i) represents the correct balanced equation.
Ion electron method. The two half reactions are: Oxidation half reaction:
P4(s)⟶H2PO−2(aq)…(ii)
Balancing P atoms, we have,
0P4(s)⟶4H2+1PO−2(aq)
Balance O.N. by adding electrons,
P4(s)⟶4H2PO−2(aq)+4e−
Balance charge by adding 8OH−ions,
P4( s)+8OH−(aq)⟶4H2PO−2(aq)+4e−…(iii)
O and H get automatically balanced. Thus, Eq. (iii) represents the balanced oxidation half reaction.
Reduction half reaction:
0P4( s)⟶−3PH3( g)
Balancing P atoms, we have,
P4( s)⟶4PH3( g)
Balance O.N. by adding electrons,
P4( s)+12e−⟶4PH3( g)
Balance charge by adding 12OH−ions,
P4( s)+12e−⟶4PH3(g)+12OH−(aq)
Balance O atoms, by adding 12H2O to L.H.S. of above equation.
P4( s)+12H2O(l)+12e−⟶4PH3(g)+12OH−(aq)…(v)
To cancel out electrons, multiply Eq. (iii) by 3 and add it to Eq. (v), we have,
4P4( s)+24OH−(aq)+12H2O(l)⟶4PH3(aq)+12H2PO−2(aq)+12H2O(l)+12OH−(aq)
P4(g)+3OH−(aq)+3H2O(l)⟶PH3(aq)+3H2PO−2(aq)…(vi)
Thus, Eq. (vi) represents the correct balanced equation.
(b)
N2H4(l)+ClO−3(aq)→NO(g)+Cl−(g)
Oxidation number method :
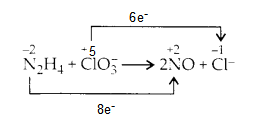
Total increase in O.N. of N=2×4=8
Total decreases in O.N. of Cl=1×6=6
Therefore, to balance increase/decrease in O.N. multiply N2H4 by 3 and ClO−3 by 4 , we have,
3 N2H4(l)+4ClO−3(aq)⟶NO(g)+Cl−(aq)
To balance N and Cl atoms, multiply NO by 6 and Cl−by 4 , we have,
3 N2H4(l)+4ClO−3(aq)⟶6NO(g)+4Cl−(aq)
Balance O atoms by adding 6H2O,
3 N2H4(l)+4ClO−3(aq)⟶6NO(g)+4Cl−(aq)+6H2O(l)…(i)
H atoms get automatically balanced and thus Eq. (i) represents the correct balanced equation.
Ion-electron method :
Oxidation half-reaction :
[N2H4+8OH−⟶2NO+8e−+6H2O]×6
Reduction half-reaction :
[ClO−3+6e−+3H2O⟶Cl−+6OH−]×8
Net reaction is
6 N2H4+8ClO−3⟶12NO+8Cl−+12H2O3 N2H4+4ClO−3⟶6NO+4Cl−+6H2O
Reductant : N2H4; Oxidant : ClO−3
(c) Cl2O7(g)+H2O2(aq))⟶ClO−2(aq)+O2(g)+H+(aq)

Cl2O7( g)+H2O2(aq)→ClO−2(aq)+O2( g)+H+
Oxidation number method :
Net reaction is
Cl2O7+4H2O2+2OH−⟶2ClO−2+5H2O+4O2
Ion-electron method:
Oxidation half-reaction :
[H2O2+2OH−⟶O2+2e−+2H2O]×4
Reduction half-reaction :
Cl2O7+8e−+3H2O⟶2ClO−2+6OH−
Net reaction is
Cl2O7+4H2O2+2OH−⟶2ClO−2+5H2O+4O2 Reductant : H2O2; Oxidant : Cl2O7
Q20. What sorts of informations can you draw from the following reaction?
(CN)2( g)+2OH−(aq)→CN−(aq)+CNO−(aq)+H2O(l)
Answer: Let x be the oxidation number of C.
∴ O.N. of C in cyanogen, (CN)2=2(x−3)=0 or x=+3
or x=+3
O.N. of C in cyanide ion, CN−=x−3=−1
or x=+2
O.N. of C in cyanate ion, CNO−=x−3−2=−1
or x=+4
The four informations about the reaction are :
(i) The reaction involves decomposition of cyanogen, (CN)2 in the alkaline medium to cyanide ion, CN−and cyanate ion, CNO−.
(ii) The O.N. of C decreases from +3 in (CN)2 to +2 in CN−ion and increases from +3 in (CN)2 to +4 in CNO−ion. Thus, cyanogen is simultaneously reduced to cyanide ion and oxidised to cyanate ion.
(iii) It is an example of disproportionation redox reaction.
(iv) Cyanogen is a pseudo halogen while cyanide ion is a pseudo halide ion.
Q21. The Mn3+ ion is unstable in solution and undergoes disproportionation to give Mn2+,MnO2, and H+ion. Write a balanced ionic equation for the reaction.
Answer: The skeletal equation is:
Mn3+(aq)⟶Mn2+(aq)+MnO2(s)+H+(aq).
Oxidation half equation:
+3Mn3+(aq)⟶+4MnO2(s)
Balance O.N. by adding electrons,
Mn3+(aq)⟶MnO2( s)+e−
Balance charge by adding 4H+ions,
Mn3+(aq)⟶MnO2(s)+4H+(aq)+e−
Balance O atoms by adding 2H2O :
3+Mn(aq)+2H2O(l)⟶MnO2(s)+4H+(aq)+e−…(i)
Reduction half equation:
+3Mn3+⟶+2Mn2+
Balance O.N. by adding electrons:
Mn3+(aq)+e−⟶Mn2+(aq)…(ii)
Adding Eq. (i) and Eq. (ii), the balanced equation for the disproportionation reaction is
2Mn3+(aq)+2H2O(l)⟶MnO2( s)+Mn2+(aq)+4H+(aq)
Q22. Consider the elements :
Cs, Ne, I and F
(a) Identify the element that exhibits only negative oxidation state.
(b) Identify the element that exhibits only postive oxidation state.
(c) Identify the element that exhibits both positive and negattve oxidation states.
(d) Identify the element which exhibits neither the negative nor does the positive oxidation state.
Answer: (a) F: Fluorine is the most electronegative element and shows only -1 oxidation state.
(b) Cs: Because of the presence of single electron in the valence shell, (alkali metals) Cs exhibits an oxidation state of +1 only.
(c) I: Because of the presence of seven electrons in the valence shell, I shows an oxidation state of- 1 in compounds with more electropositive elements (such as H,Na,K,Ca, etc.) and oxidation states of +3,+5, +7 in compounds of I with more electronegative elements (such as, O, F, etc.)
(d) Ne: It is an inert gas (with high ionization enthalpy and highly positive electron gain enthalpy) and hence, it exhibits neither negative nor positive oxidation states.
Q23. Chlorine is used to purify drinking water. Excess of chlorine is harmful. The excess of chlorine is removed by treating with sulphur dioxide. Present a balanced equation for this redox change taking place in water.
Answer: The skeletal equation is:
Cl2(aq)+SO2(aq)+H2O(l)⟶Cl−(aq)+SO2−4(aq)
Reduction half equation:
Cl2(aq)⟶Cl−(aq)
Balance Cl atoms,
0Cl2(aq)⟶−12Cl(aq)
Balance O.N. by adding electrons:
Cl2(aq)+2e−⟶2Cl−(aq)…(i)
Oxidation half equation:
+4SO2(aq)⟶+6SO2−4(aq)+2e−
Balance O.N. by adding electrons:
SO2(aq)⟶SO2−4(aq)+2e−
Balance charge by adding 4H+ions:
SO2(aq)⟶SO2−4(aq)+4H+(aq)+2e−
Balance O atoms by adding 2H2O,
SO2(aq)+2H2O(l)⟶SO2−4(aq)+4H+(aq)+2e−…(ii)
Adding Eq. (i) and Eq. (ii), we have,
Cl2(aq)+SO2(aq)+2H2O(l)⟶2Cl−(aq)+SO2−4(aq)+4H+(aq)
This represents the balanced redox reaction.
Q24. Refer to the periodic table given in your book and now answer the following questions:
(a) Select the possible non metals that can show disproportionation reaction.
(b) Select three metals that can show disproportionation reaction.
Answer: (a) The non-metals which show disproportionation reaction are N,P,S,Cl,Br and I.
(i) 2NO2(aq)+2OH−(aq)⟶NO−2(aq)+NO−3(aq)+H2O(l)
(ii) P4(s)+3OH−(aq)+3H2O(l)⟶PH3( g)+3H2PO−2(aq)
(iii) S8(s)+12OH−(aq)⟶4 S2−(aq)+2S2O2−3(aq)+6H2O(l)+6H2O(l)
(iv) Cl2( g)+2OH−(aq)⟶Cl−(aq)+ClO−(aq)+H2O(l)
Bromine and iodine give similar reactions as chlorine.
(b) The metals which undergo disproportionation reaction are:
Cu+,Ga+,In+, etc.
(i) 2Cu+(aq)⟶Cu2+(aq)+Cu(s)
(ii) 3Ga+(aq )⟶Ga3+(aq)+2Ga(s)
(iii) 3In+(aq)⟶In3+(aq)+2In(s)
Q25. In Ostwald’s process for the manufacture of nitric acid, the first step involves the oxidation of ammonia gas by oxygen gas to give nitric oxide gas and steam. What is the maximum weight of nitric oxide that can be obtained starting only with 10.00 g. of ammonia and 20.00 g of oxygen?
Answer: The reaction involved in the manufacturing process is:
∴68 g of NH3 will react with 160 g of O2 to produce 120 g of NO(g)
But oxygen which is actually available (20.0 g) is less than the amount which is required. Therefore, oxygen is the limiting reactant.
Now 160 g of O2 will form NO=120 g 20 g of O2 will form NO=(120)(160)×(20)=15 g
Q26. Using the standard electrode potentials, predict if the reaction between the following is feasible:
(a) Fe3+(aq) and I−(aq)
(b) Ag+(aq) and Cu(s)
(c) Fe3+ (aq) and Cu(s)
(d) Ag (s) and Fe3+ (aq)
(e) Br2 (aq) and Fe2+ (aq).
Answer: (a) The possible reaction between Fe3+(aq) and I−(aq)is
2Fe3+(aq)+2I−(aq)⟶2Fe2+(aq))+I2(s)
The above redox reaction can be split into the following two half reactions,
Oxidation :
2I−(aq)⟶I2(s)+2e−;E⊖oxi=−0.54 V…(i)
Reduction :
[Fe3+(aq)+e−⟶Fe2+(aq)]×2;E⊖red=+0.77 V…(ii)
Overall reaction :
2Fe3+(aq))+2I−(aq)⟶2Fe2+(aq)+I2(s);E⊖=+0.23 V
Since the EMF for the above reaction is positive, therefore, the above reaction is feasible.
(b) The possible reaction between Ag+(aq) and Cu(s) is
Cu(s)+2Ag+(aq)⟶Cu2+(aq)+2Ag(s)
The above redox reaction can be split into the following two half reactions, Oxidation :
Cu(s)⟶Cu2+(aq)+2e−;E⊖oxi=−0.34 V
Reduction :
[Ag−(aq)+e−⟶Ag(s)]×2;E⊖red=+0.80 V
Overall reaction :
Cu(s)+2Ag+(aq)⟶Cu2+(aq)+2Ag(s);E⊖=+0.46 V
Since the EMF for the above reaction comes out to be positive, therefore, the above reaction is feasible.
(c) There are two probabilities for reaction between Cu and Fe3+. The reaction between Fe3+ (aq) and Cu(s) occurs according to the following equation
(i) Cu(s)+2Fe3+(aq)⟶Cu2+(aq)+2Fe2+(aq)
The above reaction can be split into the following two half reactions, Oxidation:
Cu(s)⟶Cu2+(aq)+2e−;E⊖oxi=−0.34 V
Reduction :
[Fe3−(aq)+e−⟶Fe2−(aq)]×2;E⊖red=+0.77 V
Overall reaction :
Cu(s)+2Fe3+(aq)⟶Cu2+(aq)+2Fe2+(aq);E⊖=+0.43 V
Since the EMF for the above reaction is positive, therefore, the above reaction is feasible.
(ii) If the reaction between Fe3+(aq) and Cu(s) occurs according to the following equation,
3Cu(s)+2Fe3+(aq)⟶3Cu2+(aq)+2Fe(s)
The EMF of the reaction comes out to be negative, i.e., −0.376 V(−0.34 V−0.036 V) and hence, this reaction is not feasible.
(d) There are two possibilities for reaction between Ag and Fe3+ ion.
(i) If the reaction occurs by following equation,
Ag(s)+Fe3+(aq)⟶Ag+(aq)+Fe2+(aq)
The reaction can be split into the following two half reactions,
Oxidation :
Ag(s)⟶Ag+(aq)+e−;E⊖oxi=−0.80 V
Reduction:
Fe3+(aq)+e−⟶Fe2+(aq);E⊖red=+0.77 V
Overall reaction :
Ag(s)+Fe3+(aq)⟶Ag+(aq)+Fe2+(aq);;E⊖=−0.03 V
Since the EMF for the above reaction is negative, therefore, the above reaction is not feasible.
(ii) The reaction between Ag(s) and Fe3+(aq) may occur according to the following equation,
3Ag(s)+Fe3+(aq)⟶3Ag+(aq)+Fe(s)
EMF of this reaction comes to be even more negative i.e., −0.836 V, and hence this redox reaction is also not feasible.
(e) The reaction between Br2(aq) and Fe2+(aq) occurs according to the following equation:
Br2(aq)+2Fe2+(aq)⟶2Br−(aq)+2Fe3+(aq)
The above reaction can be split into the following two half reactions.
Oxidation :
[Fe2+(aq)⟶Fe3+(aq)+e−]×2;E⊖oxi=−0.77 V
Reduction :
Br2(aq)+2e−⟶2Br−(aq))−;E⊖red =+1.09 V
Overall reaction :
2Fe2+(aq)+Br2(aq)⟶2Fe3+(aq)+2Br−(aq);E⊖=+0.32 V
Since the EMF for the above reaction is positive, therefore, the above reaction is feasible.
Q27. Predict the products of electrolysis in each of the following:
(i) An aqueous solution of AgNO3 with silver electrodes
(ii) An aqueous solution AgNO3 with platinum electrodes
(iii) A dilute solution of H2SO4 with platinum electrodes
(iv) An aqueous solution of CuCl2 with platinum electrodes.
Answer: (i) An aqueous solution of AgNO3 using platinum electrodes :(P.I.S.A. Based)
Both AgNO3 and water will ionise in aqueous solution
AgNO3(s)(aq)⟶Ag+(aq)+NO−3(aq)H2O(aq)⇌H+(aq)+HO−(aq)
At cathode: Ag+ions with less discharge potential are reduced in preference to H+ions which will remain in solution. As a result, silver will be deposited at cathode.
Ag+(aq)+e−→Ag (deposited)
At anode : An equivalent amount of silver will be oxidised to Ag+ions by releasing electrons.
Ag(s)(aq)⟶Ag+(aq)+e−
As result of electrolysis, Ag from silver anode dissolves as Ag+(aq) ions while an equivalent amount of Ag+ (aq) ions from the aqueous AgNO3 solution get deposited on the cathode.
(ii) An aqueous solution of AgNO3 using platinum electrodes:
In the case, the platinum electrodes are the non-attackable electrodes. On passing current the following changes will occur at the electrodes.
At cathode : Ag+ions will be reduced to Ag which will get deposited at the cathode.
At anode : Both NO−3and OH−ions will migrate. But OH−ions with less discharge potential will be oxidised in preference to NO3 ions which will remain in solution.
OH−(aq)→OH+e−;4OH→2H2O(l)+O2( g)
Thus, as a result of electrolysis, silver is deposited on the cathode while O2 is evolved at the anode. The solution will be acidic due to the presence of HNO3.
(iii) A dilute solution of H2SO4 using platinum electrodes :
On passing current, both acid and water will ionise as follows :
H2SO4(l)(aq)⟶2H+(aq)+SO2−4(aq)
H2O(aq)⇌H+(aq)+OH−(aq)
At cathode :
H+(aq) ions will migrate to the cathode and will be reduced to H2.
H+(aq)+e−→H;H+H→H2( g)
Thus, H2( g) will evolve at cathode.
At anode: OH ions will be released in preference to SO2−4 ions because their discharge potential is less. They will be oxidised as follows :
OH−(aq)→OH+e−;4OH→2H2O(l)+O2( g)
Thus, O2( g) will be evolved at anode. The solution will be acidic and will contain H2SO4.
(iv) An aqueous solution of CuCl2 using platinum electrodes:
The electrolysis proceeds in the same manner as discussed in the case of AgNO3 solution. Both CuCl2 and H2O will ionise as follows :
CuCl2(aq)⟶Cu2+(aq)+2Cl−(aq)
H2O(aq)⇌H+(aq)+OH−(aq)
At cathode: Cu2+ ions will be reduced in preference to H+ions and copper will be deposited at cathode.
Cu2+(aq)+2e−→Cu( deposited)
At anode: Cl−ions will be discharged in preference to OH−ions which will remain in solution.
Cl−→Cl+e−;Cl+Cl→Cl2 (g) (evolved)
Thus, Cl2 will evolve at anode.
Q28. Arrange the following metals in the order in which they displace each other from the solution of their salts.
Al,Cu,Fe,Mg and Zn.
Answer: We know that,
E⊖Al3+/Al=−1.66 V,E⊖Cu2+/Cu=+0.34 V,
E⊖Fe2+/Fe=−0.44 V,E⊖Mg2+/Mg=−2.36 V and E⊖Zn2+/Zn=−0.76 V
Since a metal with lower electrode potential is a stronger reducing agent, therefore, Mg can displace all the above metals from their aqueous solutions, Al can displace all metals except Mg from the aqueous solutions of their salts. Zn can displace all metals except Mg and Al from the aqueous solutions of their salts while Fe can displace only Cu from the aqueous solution of its salts. Thus, the order in which they can displace each other from the solution of their salts is Mg,Al,Zn,Fe,Cu.
Q29. Given the standard electrode potentials,
K+/K=−2.93 V,Ag+/Ag=0.80 VHg2+/Hg=0.79 VMg2+/Mg=−2.37 V.Cr3+/Cr=−0.74 V
arrange these metals in their increasing order of reducing power.
Answer: Lower the electrode potential, better is the reducing power. Since the electrode potentials increase in the order;
K+/K(−2.93 V),
Mg2+/Mg(−2.37 V),Cr3+/Cr(−0.74 V),
Hg2+/Hg(0.79 V),Ag+/Ag(0.80 V),
therefore, reducing power of metals increases in the order, i.e., Ag<Hg<Cr<Mg<K.
Q30. Depict the galvanic cell in which the reaction Zn(s)+2Ag+(aq)→Zn2+(aq)+2Ag(s) takes place, Further show:
(i) which of the electrode is negatively charged,
(ii) the carriers of the current in the cell, and
(iii) Individual reaction at each electrode.
Answer: The given redox reaction is
Zn(s)+2Ag+(aq)→Zn2+(aq)+2Ag(s)
Since Zn gets oxidized to Zn2+ions, and Ag− gets reduced to Ag+ metal, therefore, oxidation occurs at zinc electrode and reduction occurs at the silver electrode.
Thus, galvanic cell corresponding to the above redox reaction may be depicted as :
Zn|Zn2+(aq)‖Ag+(aq)|Ag
(i) Since oxidation occurs at the zinc electrode, therefore, electrons accumulate on the zinc electrode and hence, zinc electrode is negatively charged.
(ii) The ions carry current. The electrons flow from Zn to Ag electrode while the current flows from Ag to Zn electrode.
(iii) The reactions occurring at the two electrodes are:
At Anode: Zn(s)⟶Zn2+(aq)+2e−
At Cathode: Ag+(aq)+e−⟶Ag(s)
Exemplar
Q31. The reaction Cl2( g)+2OH−(aq)⟶ClO−(aq)+Cl−(aq)+H2O(l)
represents the process of bleaching. Identify and name the species that bleaches the substances due to its oxidising action.
Answer:
0Cl2(g)+−2+12OH−(aq)→+1−2ClO−(aq)+−1Cl−(aq)++1−2H2O(l)
In this reaction, O.N. of Cl increases from 0 (in Cl2 ) to +1 (in ClO−) and decreases to – 1 (in Cl−). Therefore, Cl2 is both oxidized to ClO−and reduced to Cl−. Since Cl−ion cannot act as an oxidizing agent (because it cannot decrease its O.N. lower than -1), therefore, Cl2 bleaches substances due to oxidizing action of hypochlorite, ClO ion.
Q32. MnO2−4 undergoes disproportionation reaction in acidic medium but MnO−4 does not. Give reason.
Answer: In MnO4−,Mn is in the highest oxidation state of +7 (i.e.. cannot be oxidized further) and hence it cannot undergo disproportionation.
In contrast, the O.N. of Mn in MnO42− is +6. Therefore, it can increase its O. N. MnO42− from +6 to +7 in MnO−4 and decreases to +4 in MnO2. Thus, MnO42− undergoes disproportionation according to the following reaction.
Q33. PbO and PbO2 react with HCl according to following chemical equations :
2PbO+4HCl⟶2PbCl2+2H2OPbO2+4HCl⟶PbCl2+Cl2+2H2O
Why do these compounds differ in their reactivity?
Answer: (i) +22PbO+4HCl→+2PbCl2+2H2O
(ii) +4PbO2+4HCl→+2PbCl2+0Cl2+2H2O
In reaction (i), O.N. of none of the atoms undergo a change. Therefore, it is not a redox reaction. It is an acidbase reaction, because PbO is a basic oxide which reacts with HCl acid.
The reaction (ii) is a redox reaction in which PbO2 gets reduced and acts as an oxidizing agent.
Q34. Nitric acid is an oxidising agent and reacts with PbO but it does not react with PbO2. Explain why?
Answer: Nitric acid is an oxidizing agent and reacts with PbO to give a simple acid- base reaction without any change in oxidation state. In PbO2, Pb is in +4 oxidation state and cannot be oxidized further, hence no reaction takes place between PbO2 and HNO3.
2PbO+4HNO3→2 Pb Salt (NO3)2+2H2O (Acid base reaction)
Q35. Write balanced chemical equation for the following reactions:
(i) Permanganate ion (MnO−4)reacts with sulphur dioxide gas in acidic medium to produce Mn2+ and hydrogensulphate ion.
(Balance by ion electron method)
(ii) Reaction of liquid hydrazine (N2H4) with chlorate ion (ClO−3)in basic medium produces nitric oxide gas and chloride ion in gaseous state.
(Balance by oxidation number method)
(iii) Dichlorine heptaoxide (Cl2O7) in gaseous state combines with an aqueous solution of hydrogen peroxide in acidic medium to give chlorite ion (ClO−2)and oxygen gas. (Balance by ion electron method)
Answer:
(i) (2MnO−4+5SO2+2H2O+H+→5HSO−4+2Mn2+
Balancing by ion-electron method:
+7−2MnO−4++4−2SO2⟶+2Mn2+++1+6−2HSO−4(Skeletal equation)
Oxidation half: SO2⟶HSO−4
Reduction half: MnO−4⟶Mn2+
Oxidation half: SO2⟶HSO−4+2e−
SO2+2H2O⟶HSO−4+3H++2e−…(i)
(Add 2H2O molecules to balance O atoms)
Reduction half:
MnO−4+5e−⟶Mn2+MnO−4+8H++5e−⟶Mn2++4H2O…(ii)
(Add 4H2O molecules to balance O atoms and H atoms) Add oxidation and reduction half
[SO2+2H2O⟶HSO−4+3H++2e−]×5[MnO−4+8H++5e−⟶Mn2++4H2O]×22MnO−4+5SO2+2H2O+H+⟶5HSO−4+2Mn2+
(ii) 3 N2H4+4ClO−3→6NO+4Cl−+6H2O
Balancing by oxidation number method:
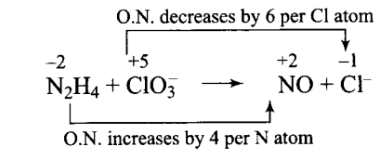
6 N2H4+8ClO−3→12NO+8Cl−+12H2O
(iii) Cl2O7( g)+4H2O2(aq)⟶2ClO−2(aq)+3H2O(l)+4O2( g)+2H+(aq)
Balancing by ion-electron method:
Oxidation half:
H2O2⟶O2+2e−
Adding 2H+on right side to balance H atoms and charge.
H2O2⟶O2+2H++2e−
Reduction half:
Cl2O7+8e−⟶2ClO−2
Adding H2O and H+to balance H and O atoms
Cl2O7+8e−+6H+⟶2ClO−2+3H2O
Adding oxidation and reduction half
[H2O2→O2+2e−+2H+]×4Cl2O7+8e−+6H+→2ClO−2+3H2O¯Cl2O7+4H2O2→2ClO−2+3H2O+4O2+2H+
Q36. Calculate the oxidation number of phosphorus in the following species.
(a) HPO2−3 and
(b) PO3−4
Answer: (a) Let the oxidation number of P in HPO32− be x.
So, +1+x+(−6)=−2;x=+3
(b) Let the oxidation number of P in PO3−4 be x.
So, x+(−8)=−3; x=+5
Q37. Calculate the oxidation number of each sulphur atom in the following compounds:
(a) Na2 S2O3
(b) Na2 S4O6
(c) Na2SO3
(d) Na2SO4
Answer:(a) Structure of Na2 S2O3 is
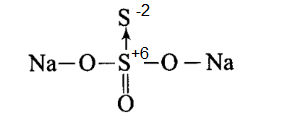
Let the oxidation number of sulphur atom is x.
Na2 S2O32×(+1)+2x+(−2)×3=02+2x−6=02x−4=02x=4x=+2
By conventional method, we can’t find the oxidation number of each sulphur atom. Thus, the oxidation states of two S atoms in Na2 S2O3 are −2 and +6.
(b) Structure of Na2 S4O6 is
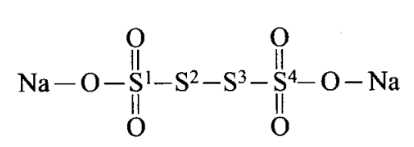
From the left, S1=(−2)+(−2)+(−1)=+5
S2=0 S3=0 S4=+5
(iii)
Na2SO32×(+1)+x+3×(−2)=0x=+4
(iv)
Q38. Balance the following equations by the oxidation number method.
(i) Fe2++H++Cr2O2−7⟶Cr3++Fe3++H2O
(ii) I2+NO−3⟶NO2+IO−3
(iii) I2+S2O2−3⟶I−+S4O2−6
(iv) MnO2+C2O2−4⟶Mn2++CO2
Answer: (i) Fe2++H++Cr2O2−7⟶Cr3++Fe3++H2O
+2Fe2++H+++6−2Cr2O2−7⟶+3Cr3+++3Fe3++H2O
(a) Balance the increase and decrease in O.N.
+26Fe2++H+++6−2Cr2O2−7⟶+32Cr3+++36Fe3++H2O
(b) Balancing H and O atoms by adding H+and H2O molecules
6Fe2++14H++Cr2O2−7⟶2Cr3++6Fe3++7H2O
(ii) I2+NO−3⟶NO2+IO−3
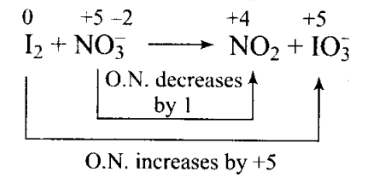
Total increase in O.N. =5×2=10
Total decrease in O.N. =1
To equalize O.N. multiply NO−3, by 10
I2+10NO−3⟶10NO2+IO−3
Balancing atoms other than O and H
I2+10NO−3⟶10NO2+2IO−3
Balancing O and H
I2+10NO−3+8H+→10NO2+2IO−3+4H2O
(iii) I2+S2O2−3⟶I−+S4O2−6
Balance all atoms other than H and O.
I2+2 S2O2−3→2I−+S4O2−6
The oxidation number of I changes from 0 to -1 . The change in the oxidation number of one I atom is 1.
Total change in the oxidation number for 2I atoms is 2.
The oxidation number of S changes from 2 to 2.5. The change in the oxidation number of one S atom is 0.5.
Total change in the oxidation number for 4 S atoms is 2.
The increase in the oxidation number is balanced with decrease in the oxidation number.
O atoms are balanced. This is the balanced chemical equation.
(iv) MnO2+C2O2−4⟶Mn2++CO2
Total increase in O.N. =1×2=2
Total decrease in O.N. =2
Now, in order to equalize O.N., we will multiply CO2 with 2 to give us,
MnO2+C2O2−4→Mn2++2CO2
Now, we will balance H and O by adding 2H2O on the right side, and 4H+on the left side of equation.
MnO2+C2O2−4+4H+→Mn2++2CO2+2H2O
Q39. Identify the redox reactions out of the following reactions and identify the oxidising and reducing agents in them.
(i) 3HCl(aq)+HNO3(aq)⟶Cl2( g)+NOCl(g)+2H2O(l)
(ii) HgCl2 (aq) +2KI (aq) ⟶HgI2 (s) +2KCl (aq)
(iii) Fe2O3 (s) +3CO (g) Δ→2Fe (s) +3CO2 (g)
(iv) PCl3 (l) +3H2O(l)⟶3HCl (aq) +H3PO3 (aq)
(v) 4NH3+3O2( g)⟶2 N2( g)+6H2O(g)
Answer:(i) Writing the O.N. of on each atom,
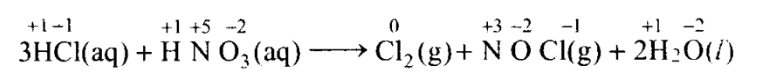
Here, O.N. of Cl increases from -1 (in HCl ) to 0 (in Cl2 ). Therefore, Cl−is oxidized and hence HCl acts as a reducing agent.
The O.N. of N decreases from +5 (in HNO3 ) to +3 (in NOCl) and therefore, HNO3 acts as an oxidizing agent. Thus, reaction (i) is a redox reaction.
(ii) Here O.N. of none of the atoms undergo a change and therefore, this is not a redox reaction.
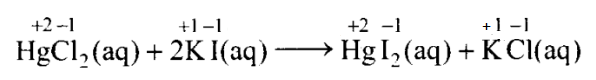
(iii) Here, O.N. of Fe decreases from +3 (in Fe2O3 ) to 0 (in Fe ) and therefore, Fe2O3 acts as an oxidizing agent. O.N. of C increases from +2 (in CO ) to +4 (in CO2 ) and therefore, CO acts as a reducing agent. Thus, this is a redox reaction.

(iv) Here O.N. of none of the atoms undergo a change and therefore, it is not a redox reaction.
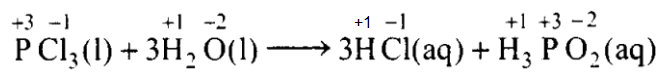
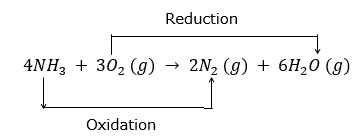
(v) In the below reaction, oxidising agent is O2 and the reducing agent is NH3.
Here. O.N. of N increases from -3 (in NH3 ) to 0 in ( N2 ) and therefore, NH3 acts as a reducing agent. O.N. of O decreases from 0 (in O2 ) to -2 (in H2,O ) and therefore, O2 acts as an oxidizing agent.
Thus, reaction (v) is a redox reaction.
Q40. Balance the following ionic equations
(i) Cr2O2−7+H++I−⟶Cr3++I2+H2O
(ii) Cr2O2−7+Fe2++H+⟶Cr3++Fe3++H2O
(iii) MnO−4+SO2−3+H+⟶Mn2++SO2−4+H2O
(iv) MnO−4+H++Br−⟶Mn2++Br2+H2O
Answer:

Dividing the equation into two half reactions:
Oxidation half reaction: I−→I2
Reduction half reaction: Cr2O2−7→Cr3+
Balancing oxidation and reduction half reactions separately as:
Oxidation half reaction
I−⟶I22I−⟶I22I−⟶I2+2e−…(i)
Reduction half reaction
Cr2O2−7⟶Cr3+Cr2O2−7⟶2Cr3+Cr2O2−7+6e−⟶2Cr3+
Cr2O2−7+14H++6e−⟶2Cr3++7H2O…(ii)
(acidic medium)
To balance the electrons, multiply eq. (i) by 3 and add to eq. (ii)
Cr2O2−7+14H++6I−⟶2Cr3++3I2+7H2O
(ii) Step-1: Separate the equation into two half reactions.
The oxidation number of various atoms are shown below:

In this case, chromium undergoes reduction, oxidation number decreases from +6 (in Cr2O2−7 ) to +3 (in Cr3+ )
Fe2+(O.N.=+2) changes to Fe3+(O⋅N.=+3). The species undergoing oxidation and reduction are:
Oxidation: Fe2+⟶Fe3+
Reduction: Cr2O2−7⟶Cr3+
Step-2: Balance each half reaction separately as:
Fe2+⟶Fe3+
(a) Balance all atoms other than H and O. This step is not needed, because, it is already balanced.
(b) The oxidation number on left is +2 and on right is +3 . To account for the difference, the electron is added to the right as:
Fe2+→Fe3++e−
(c) Charge is already balanced.
(d) No need to add H or O.
The balanced half equation is:
Fe2+→Fe3++e−…(i)
Consider the second half equation Cr2O2−7→Cr3+
(a) Balance the atoms other than H and O.
Cr2O2−7→2Cr3+
(b) The oxidation number of chromium on the left is +6 and on the right is +3 . Each chromium atom must gain three electrons. Since there are two Cr atoms, add 6e−on the left.
Cr2O2−7+6e−→2Cr3+
(c) Since the reaction takes place in acidic medium add 14H+on the left to equate the net charge on both sides.
Cr2O2−7+6e−+14H+→2Cr3+
(d) To balance FI atoms, add 7H2O molecules on the right.
Cr2O2−7+6e−+14H+→2Cr3++7H2O…(ii)
This is the balanced half equation.
Step-3: Now add up the two half equations. Multiply eq. (i) by 6 so that electrons are balanced.
[Fe2+⟶Fe3++e−]×6Cr2O2−7+6e−+14H+⟶2Cr3++7H2O¯6Fe2++Cr2O2−7+14H+⟶6Fe3++2Cr3++7H2O
The balanced equation is:
6Fe2++Cr2O2−7+14H+⟶6Fe3++2Cr3++7H2O
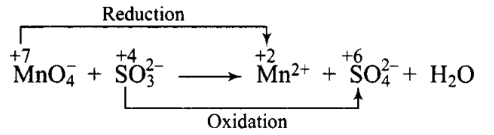
(iii) Dividing the equation into two half reactions: Oxidation half reaction: SO2−3⟶SO2−4
Reduction half reaction: MnO−4⟶Mn2+
As step (i), we will be generating the unbalanced skeleton i.e.,
MnO−4+SO2−3+H+→Mn2++SO2−4+H2O
Here in Mn undergoes reduction and S undergoes oxidation.
As step (ii) we will have to Identify the reactants which will get oxidized or reduced, and then write the half-cell reaction for them. This will be done along with the electron transfers as it will carefully be making the equal numbers of atoms of oxidized and reduced redox couples.
Thus, oxidation is SO2−3→SO2−4+2e−and reduction is MnO−4+5e−→Mn2+
As step (iii) we will balance the number of atoms in the oxidized and reduced redox couples. But as we can see that the atoms are balanced, so the reaction will be the same as the previous step.
Thus, oxidation is SO2−3→SO2−4+2e−and reduction is MnO−4+5e−→Mn2+
Now, as step (iv) for acidic solutions, we will balance the charge by adding H+ on the required side of the given equation.
Thus, oxidation is SO2−3→SO2−4+2e−+2H+and reduction is MnO−4+5e−+8H+→Mn2+
As part of the step (v) we will balance the oxygen atoms. As there are seven oxygen atoms in reduction reaction, so we will be adding water molecule to RHS of the reaction for balancing the oxygen.
Thus, oxidation is SO2−3+H2O→SO2−4+2e−+2H+ and
reduction is MnO−4+5e−+8H+→Mn2++4H2O
Now as part of the step (vi) we will be making the electron lost and gain, equal in both the reduction and oxidation reaction.
Thus, oxidation is 5SO2−3+5H2O→5SO2−4+10e−+10H+and
reduction is 2MnO−4+10e−+16H+→2Mn2++8H2O
Now, as part of the step (vii) we will combine the two given equations by adding them. This will ensure that all the products and reactants remain on the same side.
2MnO−4+10e−+16H++5SO2−3+5H2O→2Mn2++8H2O+5SO2−4+10e−+10H+
Now, as step (viii) we will simplify the equation. This will be done by eliminating the similar terms present on both of the sides of the equation.
2MnO−4+6H++5SO2−3→2Mn2++3H2O+5SO2−4
We will now verify whether all the charges are balanced.
So, LHS =2×−1+6+5×−2=−6 and the RHS =2×+2+3×0+5×−2=−6
Equal charges on both sides imply towards a balanced equation.
(iv)
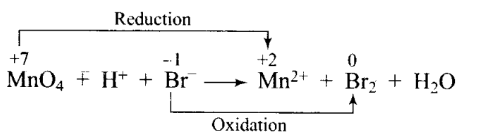
Dividing the equation into two half reactions:
Oxidation half reaction: Br⟶Br2
Reduction half reaction: MnO−4⟶Mn2+
Balancing oxidation and reduction half reactions separately as:
Oxidation half reaction
Br−⟶Br22Br−⟶Br22Br−⟶Br2+2e−…(i)
Reduction half reaction
MnO−4⟶Mn2+MnO−4+5e⟶Mn2+
MnO−4+8H++5e−⟶Mn2+
MnO−4+8H++5e−⟶Mn2++4H2O…(ii)
To balance the electrons, multiply eq. (i) by 5 and eq. (ii) by 2 and add
2MnO−4+10Br−+16H+⟶2Mn2++5Br2+8H2O
Q41. Explain redox reactions on the basis of electron transfer. Give suitable examples.
Answer:As we know that, the reactions
2Na(s)+Cl2( g)→2NaCl(s)4Na(s)+O2( g)→2Na2O(s)
are redox reactions because in each of these reactions sodium is oxidised due to the addition of either oxygen or more electronegative element to sodium. From our knowledge of chemical bonding we also know that, sodium chloride and sodium oxide are ionic compounds and perhaps better written as
Na+Cl−(s) and (Na+)2O2−(s).
Develpment of charges on the species produced suggests us to rewrite the above reaction in the following manner

For convenience, each of the above processes can be considered as two separate steps, one involving the loss of electrons and other the gain of electrons. As an illustration, we may further elaborate one of these, say, the formation of sodium chloride.
2Na(s)→2Na+(g)+2e−Cl2( g)+2e−→2Cl−(g)
Each of the above steps is called a half reaction, which explicitly shows involvement of electrons. Sum of the half reactions gives the overall reaction:
2Na(s)+Cl2( g)→2Na+Cl−(s) or 2NaCl(s)
The given reactions suggest that half reactions that involved loss of electrons are oxidation reactions. Similarly, the half reactions that involve gain of electrons are called reduction reactions.
It may be out of context to mention here that the new way of defining oxidation and reduction has been achieved only by establishing a correlation between the behaviour of species as per the classical idea and their interplay in electron-transfer change.
In the given reaction, sodium, which is oxidised, acts a reducing agent because it donates electron to each of the elements interacting with it and thus helps in reducing them. Chlorine and oxygen are reduced and act as oxidising agents because these accept electrons from sodium.
To summarise, we may mention that
Oxidation: Loss of electron(s) by any species.
Reduction: Gain of electron(s) by any species.
Oxidising agent: Acceptor of electron(s).
Reducing agent: Donor of electron(s).
Q42. On the basis of standard electrode potential values, suggest which of the following reactions would take place? (Consult the book for E⊖ value).
(i) Cu+Zn2+⟶Cu2++Zn
(ii) Mg+Fe2+⟶Mg2++Fe
(iii) Br2+2Cl−⟶Cl2+2Br−
(iv) Fe+Cd2+⟶Cd+Fe2+
Answer: As we know that,
E⊖Cu2+/Cu=0.34 V,E⊖Zn2+/Zn=−0.76 V,E⊖Mg2+/Mg=−2.37 V,E⊖Fe2+/Fe=−0.74 V,E⊖Br2/Br−=+1.08 V,E⊖Cl2/Cl−=+1.36 VE⊖Fe2+/Fe=−0.74 V,E⊖Cd2+/Cd=−0.44 V
(a)
E⊖Cu2+/Cu=0.34 V and E⊖Zn2+/Zn=−0.76 VCu+Zn2+→Cu2++Zn
In the given cell reaction, Cu is oxidised to Cu2+, therefore, Cu2+/Cu couple acts as anode and Zn2+ is reduced to Zn, therefore, Zn2+/Zn couple acts as cathode.
E⊖ cell =E∘ cathode −E∘ anode E⊖ cell =−0.74−(+0.34)=−1.10 V
Positive value of E⊖ cell indicates that the reaction will not occur.
(b) Mg+Fe2+→Mg2++Fe
E⊖Mg2+/Mg=−2.37 V and E⊖Fe2+/Fe=−0.74 V
In the given cell reaction, Mg is oxidised to Mg2+ hence, Mg2+/Mg couple acts as anode and Fe2+ is reduced to Fe hence, Fe2+/Fe couple acts as cathode.
E⊖cell =E⊖cathode −E⊖anode E⊖cell =−0.74−(−2.37)=+1.63 V
Positive value of E⊖ cell 5 indicates that the reaction will occur.
(c) Br2+2Cl−→Cl2+2Br−
E⊖Br2/Br−=+1.08 V and E⊖Cl2/Cl−=+1.36 V
In the given cell reaction, Cl−is oxidised to Cl2 hence, Cl−/Cl2 couple acts as anode and Br2 is reduced to Br−hence; Br−/Br2 couple acts as cathode.
E⊖ cell =E⊖ cathode −E⊖ anode E⊖cell =+1.08−(+1.36)=−0.28 V
Negative value of E⊖ cell indicates that the reaction will occur.
(d)
Fe+Cd2+→Cd+Fe2+E⊖Fe2+/Fe=−0.74 V and E⊖Cd2+/Cd=−0.44 V
In the given cell reaction, Fe is oxidised to Fe2+ hence, Fe2+/Fe couple acts as anode and Cd2+ is reduced to Cd hence, Cd2+/Cd−couple acts as cathode.
E⊖ cell =E⊖cathode −E⊖anode E⊖ cell =−0.44−(−0.74)=+0.30 V
Positive value E⊖ cell indicates that the reaction will occur.
Q43. Why does fluorine not show disproportionation reaction?
Answer:
In a disproportionation reaction, the same species is simultaneously oxidised as well as reduced. Therefore, for such a redox reaction to occur, the reacting species must contain an element which has atleast three oxidation states.
The element, in reacting species, is present in an intermediate state while lower and higher oxidation states are available for reduction and oxidation to occur (respectively).
Fluorine is the strongest oxidising agent. It does not show positive oxidation state. That is why fluorine does not show disproportionation reaction.
Q44. Write redox couples involved in the reactions (i) to (iv) given below.
(i) Cu+Zn2+⟶Cu2++Zn
(ii) Mg+Fe2+⟶Mg2++Fe
(iii) Br2+2Cl−⟶Cl2+2Br−
(iv) Fe+Cd2+⟶Cd+Fe2+
Answer: (i) Cu2+/Cu and Zn2+/Zn
(ii) Mg2+/Mg and Fe2+/Fe
(iii) Br2/Br−and Cl2/Cl−
(iv) Fe2+/Fe and Cd2+/Cd
Q45. Find out the oxidation number of chlorine in the following compounds and arrange them in increasing order of oxidation number of chlorine.
NaClO4,NaClO3,NaClO,KClO2,Cl2O7,ClO3,Cl2O,NaCl,Cl2,ClO2.
Which oxidation state is not present in any of the above compounds?
Answer: Suppose that the oxidation number of chlorine in these compounds be x.
O.N. of Cl in NaClO4∴+1+x+4(−2)=0 or, x=+7
O.N. of Cl in NaClO3∴+1+x+3(−2)=0 or, x=+5
O.N. of Cl in NaClO∴+1+x+1(−2)=0 or, x=+1
O.N. of Cl in KClO2∴+1+x+2(−2)=0 or, x=+3
O.N. of Cl in Cl2O7∴+2x+7(−2)=0 or, x=+7
O.N. of Cl in ClO3∴x+3(−2)=0 or, x=+6
O.N. of Cl in Cl2O∴2x+1(−2)=0 or, x=+1
O.N. of Cl in NaCl∴+1+x=0 or, x=−1
O.N. of Cl in Cl2∴2x=0 or, x=0
O.N. of Cl in ClO2∴x+2(−2)=0 or, x=+4
None of these compounds have an oxidation number of +2 .
Increasing order of oxidation number of chlorine is : −1,0,+1,+3,+4,+5,+6,+7
Therefore, the increasing order of oxidation number of Cl in compounds is
NaCl<Cl2<NaClO<KClO2<ClO2<NaClO3<ClO3<Cl2O7
Q46. Which method can be used to find out strength of reductant/oxidant in a solution? Explain with an example.
Answer:Measure the electrode potential of the given species by connecting the redox couple of the given species with standard hydrogen electrode. If it is positive, the electrode of the given species acts as reductant and if it negative, it acts as an oxidant.
Find the electrode potentials of the other given species in the same way, compare the values and determine their comparative strength as a reductant or oxidant.
e.g., measurement of standard electrode potential of Zn2+/Zn electrode using SHE as a reference electrode.
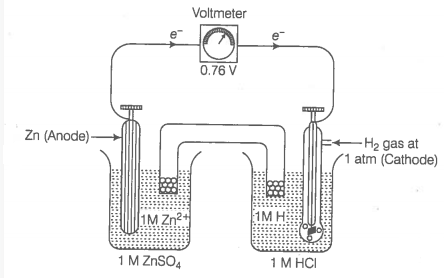
The EMF of the cell comes out to be 0.76 V. (reading of voltmeter is 0.76 V ). Zn2+/Zn couple acts as anode and SHE acts as cathode.
∴E⊖cell =0.76=E⊖cathode −E⊖anode 0.76=0−E⊖ anode E⊖anode =−0.76 VE⊖Zn2+/Zn=−0.76 V.