6.8 Factors Affecting Equilibria

Le Chatelier’s Principle and Factors Affecting Equilibria

One of the principal goals of chemical synthesis is to maximise the conversion of the reactants to products while minimizing the expenditure of energy. This implies maximum yield of products at mild temperature and pressure conditions. If it does not happen, then the experimental conditions need to be adjusted. For example, in the Haber process for the synthesis of ammonia from \(\mathrm{N}_2\) and \(\mathrm{H}_2\), the choice of experimental conditions is of real economic importance. Annual world production of ammonia is about hundred million tones, primarily for use as fertilizers.

Equilibrium constant, \(K_c\) is independent of initial concentrations. But if a system at equilibrium is subjected to a change in the concentration of one or more of the reacting substances, then the system is no longer at equilibrium; and net reaction takes place in some direction until the system returns to equilibrium once again. Similarly, a change in temperature or pressure of the system may also alter the equilibrium. In order to decide what course the reaction adopts and make a qualitative prediction about the effect of a change in conditions on equilibrium we use Le Chatelier’s principle.

It states that a change in any of the factors that determine the equilibrium conditions of a system will cause the system to change in such a manner so as to reduce or to counteract the effect of the change. This is applicable to all physical and chemical equilibria.

We shall now be discussing factors which can influence the equilibrium.

(i) Effect of Concentration Change

In general, when equilibrium is disturbed by the addition/removal of any reactant/ products, Le Chatelier’s principle predicts that:

• The concentration stress of an added reactant/product is relieved by net reaction in the direction that consumes the added substance.
• The concentration stress of a removed reactant/product is relieved by net reaction in the direction that replenishes the removed substance. or in other words, “When the concentration of any of the reactants or products in a reaction at equilibrium is changed, the composition of the equilibrium mixture changes so as to minimize the effect of concentration changes”.
  Let us take the reaction, \(\mathrm{H}_2(\mathrm{~g})+\mathrm{I}_2(\mathrm{~g}) \rightleftharpoons 2 \mathrm{HI}(\mathrm{g})\)
  If \(\mathrm{H}_2\) is added to the reaction mixture at equilibrium, then the equilibrium of the reaction is disturbed. In order to restore it, the reaction proceeds in a direction wherein \(\mathrm{H}_2\) is consumed, i.e., more of \(\mathrm{H}_2\) and \(\mathrm{I}_2\) react to form \(\mathrm{HI}\) and finally the equilibrium shifts in right (forward) direction (Fig.7.8). This is in accordance with the Le Chatelier’s principle which implies that in case of addition of a reactant/product, a new equilibrium will be set up in which the concentration of the reactant/product should be less than what it was after the addition but more than what it was in the original mixture.

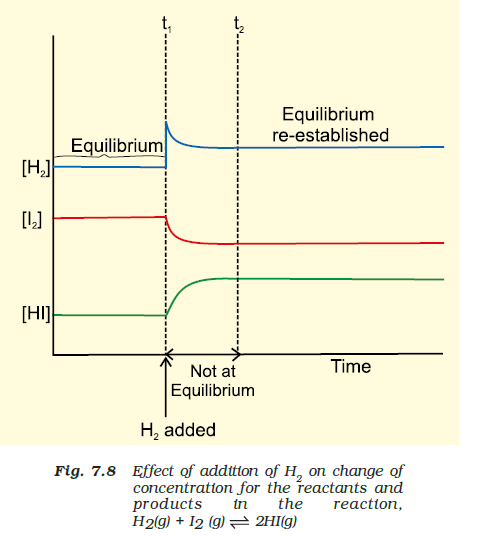
The same point can be explained in terms of the reaction quotient, \(Q_{c}\)
\(
Q_c=[\mathrm{HI}]^2 /\left[\mathrm{H}_2\right]\left[\mathrm{I}_2\right]
\)
The addition of hydrogen at equilibrium results in value of \(Q_c\) being less than \(K_c\). Thus, in order to attain equilibrium again reaction moves in the forward direction. Similarly, we can say that removal of a product also boosts the forward reaction and increases the concentration of the products and this has great commercial application in cases of reactions, where the product is a gas or a volatile substance.

In the case of the manufacture of ammonia, ammonia is liquified and removed from the reaction mixture so that the reaction keeps moving in the forward direction. Similarly, in the large-scale production of \(\mathrm{CaO}\) (used as important building material) from \(\mathrm{CaCO}_3\), constant removal of \(\mathrm{CO}_2\) from the kiln drives the reaction to completion. It should be remembered that continuous removal of a product maintains \(Q_c\) at a value less than \(K_c\) and the reaction continues to move in the forward direction.

Effect of Concentration – An experiment

This can be demonstrated by the following reaction:
\(
\mathrm{Fe}^{3+}(\mathrm{aq})+\mathrm{SCN}^{-}(\mathrm{aq}) \rightleftharpoons[\mathrm{Fe}(\mathrm{SCN})]^{2+}(\mathrm{aq}) \dots(7.24)
\)
\(
\text { yellow          colourless              deep red }
\)
\(
K_c=\frac{\left[\mathrm{Fe}(\mathrm{SCN})^{2+}(\mathrm{aq})\right]}{\left[\mathrm{Fe}^{3+}(\mathrm{aq})\right]\left[\mathrm{SCN}^{-}(\mathrm{aq})\right]} \dots(7.25)
\)
A reddish colour appears on adding two drops of \(0.002 \mathrm{M}\) potassium thiocynate solution to \(1 \mathrm{~mL}\) of \(0.2 \mathrm{M}\) iron(III) nitrate solution due to the formation of \([\mathrm{Fe}(\mathrm{SCN})]^{2+}\). The intensity of the red colour becomes constant on attaining equilibrium. This equilibrium can be shifted in either forward or reverse directions depending on our choice of adding a reactant or a product. The equilibrium can be shifted in the opposite direction by adding reagents that remove \(\mathrm{Fe}^{3+}\) or \(\mathrm{SCN}^{-}\)ions. For example, oxalic acid \(\left(\mathrm{H}_2 \mathrm{C}_2 \mathrm{O}_4\right)\), reacts with \(\mathrm{Fe}^{3+}\) ions to form the stable complex ion \(\left[\mathrm{Fe}\left(\mathrm{C}_2 \mathrm{O}_4\right)_3\right]^{3-}\), thus decreasing the concentration of free \(\mathrm{Fe}^{3+}(\mathrm{aq})\). In accordance with the Le Chatelier’s principle, the concentration stress of removed \(\mathrm{Fe}^{3+}\) is relieved by dissociation of \([\mathrm{Fe}(\mathrm{SCN})]^{2+}\) to replenish the \(\mathrm{Fe}^{3+}\) ions. Because the concentration of \([\mathrm{Fe}(\mathrm{SCN})]^{2+}\) decreases, the intensity of red colour decreases.

Addition of aq. \(\mathrm{HgCl}_2\) also decreases red colour because \(\mathrm{Hg}^{2+}\) reacts with \(\mathrm{SCN}^{-}\)ions to form stable complex ion \(\left[\mathrm{Hg}(\mathrm{SCN})_4\right]^{2-}\). Removal of free \(\mathrm{SCN}^{-}\)(aq) shifts the equilibrium in equation (7.24) from right to left to replenish \(\mathrm{SCN}^{-}\)ions. The addition of potassium thiocyanate on the other hand increases the colour intensity of the solution as it shift the equilibrium to the right.

(ii) Effect of Pressure Change

A pressure change obtained by changing the volume can affect the yield of products in the case of a gaseous reaction where the total number of moles of gaseous reactants and total number of moles of gaseous products are different. In applying Le Chatelier’s principle to a heterogeneous equilibrium the effect of pressure changes on solids and liquids can be ignored because the volume (and concentration) of a solution/liquid is nearly independent of pressure.
Consider the reaction,
\(
\mathrm{CO}(\mathrm{g})+3 \mathrm{H}_2(\mathrm{~g}) \rightleftharpoons \mathrm{CH}_4(\mathrm{~g})+\mathrm{H}_2 \mathrm{O}(\mathrm{g})
\)
Here, \(4 \mathrm{~mol}\) of gaseous reactants \(\left(\mathrm{CO}+3 \mathrm{H}_2\right)\) become \(2 \mathrm{~mol}\) of gaseous products \(\left(\mathrm{CH}_4+\right.\) \(\mathrm{H}_2 \mathrm{O}\) ). Suppose the equilibrium mixture (for the above reaction) kept in a cylinder fitted with a piston at constant temperature is compressed to one-half of its original volume. Then, total pressure will be doubled (according to \(p V=\) constant).

The partial pressure and therefore, concentration of reactants and products have changed and the mixture is no longer at equilibrium. The direction in which the reaction goes to re-establish equilibrium can be predicted by applying the Le Chatelier’s principle. Since pressure has doubled, the equilibrium now shifts in the forward direction, a direction in which the number of moles of the gas or pressure decreases (we know the pressure is proportional to the moles of the gas). This can also be understood by using the reaction quotient, \(Q_{c}\). Let \([\mathrm{CO}],\left[\mathrm{H}_2\right],\left[\mathrm{CH}_4\right]\) and \(\left[\mathrm{H}_2 \mathrm{O}\right]\) be the molar concentrations at equilibrium for methanation reaction. When the volume of the reaction mixture is halved, the partial pressure and the concentration are doubled. We obtain the reaction quotient by replacing each equilibrium concentration by double its value.
\(
Q_c=\frac{\left[\mathrm{CH}_4(\mathrm{~g})\right]\left[\mathrm{H}_2 \mathrm{O}(\mathrm{g})\right]}{[\mathrm{CO}(\mathrm{g})]\left[\mathrm{H}_2(\mathrm{~g})\right]^3}
\)
As \(Q_c<K_c\), the reaction proceeds in the forward direction.
In reaction \(\mathrm{C}(\mathrm{s})+\mathrm{CO}_2(\mathrm{~g}) \rightleftharpoons 2 \mathrm{CO}(\mathrm{g})\), when pressure is increased, the reaction goes in the reverse direction because the number of moles of gas increases in the forward direction.

(iii) Effect of Inert Gas Addition

If the volume is kept constant and an inert gas such as argon is added which does not take part in the reaction, the equilibrium remains undisturbed. This is because the addition of an inert gas at constant volume does not change the partial pressures or the molar concentrations of the substance involved in the reaction. The reaction quotient changes only if the added gas is a reactant or product involved in the reaction.

(iv) Effect of Temperature Change

Whenever an equilibrium is disturbed by a change in the concentration, pressure or volume, the composition of the equilibrium mixture changes because the reaction quotient, \(Q_{\mathrm{c}}\) no longer equals the equilibrium constant, \(K_{\mathrm{c}}\). However, when a change in temperature occurs, the value of the equilibrium constant, \(K_c\) is changed.

In general, the temperature dependence of the equilibrium constant depends on the sign of \(\Delta H\) for the reaction.

• The equilibrium constant for an exothermic reaction (negative \(\Delta H\) ) decreases as the temperature increases.
• The equilibrium constant for an endothermic reaction (positive \(\Delta H\) ) increases as the temperature increases.
  Temperature changes affect the equilibrium constant and rates of reactions.
  Production of ammonia according to the reaction,
  \(
  \mathrm{N}_2(\mathrm{~g})+3 \mathrm{H}_2(\mathrm{~g}) \rightleftharpoons 2 \mathrm{NH}_3(\mathrm{~g}) ;\Delta H=-92.38 \mathrm{~kJ} \mathrm{~mol}^{-1}
  \)
  is an exothermic process. According to Le Chatelier’s principle, raising the temperature shifts the equilibrium to the left and decreases the equilibrium concentration of ammonia. In other words, low temperature is favourable for high yield of ammonia, but practically very low temperatures slow down the reaction and thus a catalyst is used.

Effect of Temperature – An experiment

Effect of temperature on equilibrium can be demonstrated by taking \(\mathrm{NO}_2\) gas (brown in colour) which dimerises into \(\mathrm{N}_2 \mathrm{O}_4\) gas (colourless).
\(
2 \mathrm{NO}_2(\mathrm{~g}) \rightleftharpoons \mathrm{N}_2 \mathrm{O}_4(\mathrm{~g}) ; \Delta H=-57.2 \mathrm{~kJ} \mathrm{~mol}^{-1}
\)
\(\mathrm{NO}_2\) gas prepared by addition of \(\mathrm{Cu}\) turnings to conc. \(\mathrm{HNO}_3\) is collected in two \(5 \mathrm{~mL}\) test tubes (ensuring the same intensity of colour of gas in each tube) and stopper sealed with araldite. Three \(250 \mathrm{~mL}\) beakers 1, 2, and 3 containing freezing mixture, water at room temperature and hot water (363K), respectively, are taken (Fig. 7.9).

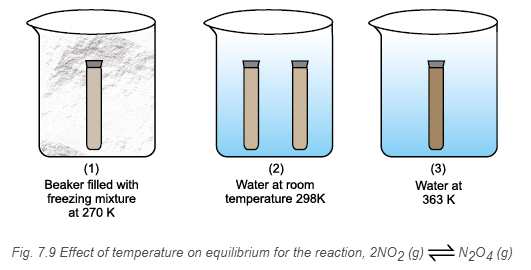
Both the test tubes are placed in beaker 2 for 8 – 10 minutes. After this one is placed in beaker 1 and the other in beaker 3. The effect of temperature on the direction of the reaction is depicted very well in this experiment. At low temperatures in beaker 1, the forward reaction of formation of \(\mathrm{N}_2 \mathrm{O}_4\) is preferred, as the reaction is exothermic, and thus, the intensity of the brown colour due to \(\mathrm{NO}_2\) decreases. While in beaker 3, high temperature favours the reverse reaction of formation of \(\mathrm{NO}_2\) and thus, the brown colour intensifies
The effect of temperature can also be seen in an endothermic reaction,
\(
\left[\mathrm{Co}\left(\mathrm{H}_2 \mathrm{O}\right)_6\right]^{3+}(\mathrm{aq})+4 \mathrm{Cl}^{-}(\mathrm{aq}) \rightleftharpoons\left[\mathrm{CoCl}_4\right]^{2-}(\mathrm{aq})+6 \mathrm{H}_2 \mathrm{O}(l)
\)
\(\text { pink colourless blue }\)
At room temperature, the equilibrium mixture is blue due to \(\left[\mathrm{CoCl}_4\right]^{2-}\). When cooled in a freezing mixture, the colour of the mixture turns pink due to \(\left[\mathrm{Co}\left(\mathrm{H}_2 \mathrm{O}\right)_6\right]^{3+}\).

(v) Effect of a Catalyst

A catalyst increases the rate of the chemical reaction by making available a new low-energy pathway for the conversion of reactants to products. It increases the rate of forward and reverse reactions that pass through the same transition state and does not affect equilibrium. Catalyst lowers the activation energy for the forward and reverse reactions by exactly the same amount. Catalyst does not affect the equilibrium composition of a reaction mixture. It does not appear in the balanced chemical equation or in the equilibrium constant expression.

Let us consider the formation of \(\mathrm{NH}_3\) from dinitrogen and dihydrogen which is a highly exothermic reaction and proceeds with the decrease in the total number of moles formed as compared to the reactants. The equilibrium constant decreases with an increase in temperature. At low temperatures, rate decreases and it takes a long time to reach equilibrium, whereas high temperatures give satisfactory rates but poor yields.

German chemist, Fritz Haber discovered that a catalyst consisting of iron catalyse the reaction to occur at a satisfactory rate at temperatures, where the equilibrium concentration of \(\mathrm{NH}_3\) is reasonably favourable. Since the number of moles formed in the reaction is less than those of reactants, the yield of \(\mathrm{NH}_3\) can be improved by increasing the pressure.

Optimum conditions of temperature and pressure for the synthesis of \(\mathrm{NH}_3\) using catalyst are around \(500^{\circ} \mathrm{C}\) and \(200 \mathrm{~atm}\).
Similarly, in the manufacture of sulphuric acid by contact process,
\(
2 \mathrm{SO}_2(\mathrm{~g})+\mathrm{O}_2(\mathrm{~g}) \rightleftharpoons 2 \mathrm{SO}_3(\mathrm{~g}) ; K_c=1.7 \times 10^{26}
\)
though the value of \(K\) is suggestive of the reaction going to completion, but practically the oxidation of \(\mathrm{SO}_2\) to \(\mathrm{SO}_3\) is very slow. Thus, platinum or divanadium penta-oxide \(\left(\mathrm{V}_2 \mathrm{O}_5\right)\) is used as catalyst to increase the rate of the reaction.

Note: If a reaction has an exceedingly small \(K\), a catalyst would be of little help.