6.6 Applications of Equilibrium Constants

Before considering the applications of equilibrium constants, let us summarise the important features of equilibrium constants as follows:

• Expression for equilibrium constant is applicable only when concentrations of the reactants and products have attained constant value at equilibrium state.
• The value of the equilibrium constant is independent of the initial concentrations of the reactants and products.
• Equilibrium constant is temperature dependent having one unique value for a particular reaction represented by a balanced equation at a given temperature.
• The equilibrium constant for the reverse reaction is equal to the inverse of the equilibrium constant for the forward reaction.
• The equilibrium constant \(K\) for a reaction is related to the equilibrium constant of the corresponding reaction, whose equation is obtained by multiplying or dividing the equation for the original reaction by a small integer.

Let us consider applications of equilibrium constant to:

• predict the extent of a reaction on the basis of its magnitude,
• predict the direction of the reaction, and
• calculate equilibrium concentrations.

Predicting the Extent of a Reaction

The numerical value of the equilibrium constant for a reaction indicates the extent of the reaction. But it is important to note that an equilibrium constant does not give any information about the rate at which the equilibrium is reached. The magnitude of \(K_{\mathrm{c}}\) or \(K_p\) is directly proportional to the concentrations of products (as these appear in the numerator of equilibrium constant expression) and inversely proportional to the concentrations of the reactants (these appear in the denominator). This implies that a high value of \(K\) is suggestive of a high concentration of products and vice-versa.
We can make the following generalizations concerning the composition of equilibrium mixtures:

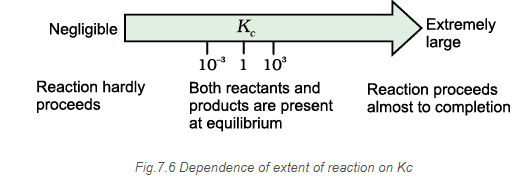
• If \(K_{\mathrm{c}}>10^3\), products predominate over reactants, i.e., if \(K_{\mathrm{c}}\) is very large, the reaction proceeds nearly to completion. Consider the following examples:
  • The reaction of \(\mathrm{H}_2\) with \(\mathrm{O}_2\) at \(500 \mathrm{~K}\) has a
    very large equilibrium constant,
    \(
    K_{\mathrm{c}}=2.4 \times 10^{47}
    \)
  • \(\mathrm{H}_2(\mathrm{~g})+\mathrm{Cl}_2(\mathrm{~g}) \rightleftharpoons 2 \mathrm{HCl}(\mathrm{g})\) at \(300 \mathrm{~K}\) has \(K_{\mathrm{c}}=4.0 \times 10^{31}\).
  • \(
    \begin{aligned}
    & \mathrm{H}_2(\mathrm{~g})+\mathrm{Br}_2(\mathrm{~g}) \rightleftharpoons 2 \mathrm{HBr}(\mathrm{g}) \text { at } 300 \mathrm{~K}, \\
    & K_c=5.4 \times 10^{18}
    \end{aligned}
    \)
• If \(K_c<10^{-3}\), reactants predominate over products, i.e., if \(K_c\) is very small, the reaction proceeds rarely. Consider the following examples:
  • The decomposition of \(\mathrm{H}_2 \mathrm{O}\) into \(\mathrm{H}_2\) and \(\mathrm{O}_2\) at \(500 \mathrm{~K}\) has a very small equilibrium constant, \(K_c=4.1 \times 10^{-48}\)
  • The decomposition of \(\mathrm{H}_2 \mathrm{O}\) into \(\mathrm{H}_2\) and \(\mathrm{O}_2\) at \(500 \mathrm{~K}\) has a very small equilibrium constant, \(K_c=4.1 \times 10^{-48}\)
  • \(
    \mathrm{N}_2(\mathrm{~g})+\mathrm{O}_2(\mathrm{~g}) \rightleftharpoons 2 \mathrm{NO}(\mathrm{g})
    \)
    at \(298 \mathrm{~K}\) has \(K_c=4.8 \times 10^{-31}\).
• If \(K_c\) is in the range of \(10^{-3}\) to \(10^3\), appreciable concentrations of both reactants and products are present. Consider the following examples:
  • For reaction of \(\mathrm{H}_2\) with \(\mathrm{I}_2\) to give \(\mathrm{HI}\), \(K_c=57.0\) at \(700 \mathrm{~K}\).
  • Also, gas phase decomposition of \(\mathrm{N}_2 \mathrm{O}_4\) to \(\mathrm{NO}_2\) is another reaction with a value of \(K_c=4.64 \times 10^{-3}\) at \(25^{\circ} \mathrm{C}\) which is neither too small nor too large. Hence, equilibrium mixtures contain appreciable concentrations of both \(\mathrm{N}_2 \mathrm{O}_4\) and \(\mathrm{NO}_2\).

These generalisations are illustrated in Fig. 7.6

Predicting the Direction of the Reaction

The equilibrium constant helps in predicting the direction in which a given reaction will proceed at any stage. For this purpose, we calculate the reaction quotient \(\boldsymbol{Q}\). The reaction quotient, \(Q\left(Q_c\right.\) with molar concentrations and \(Q_p\) with partial pressures) is defined in the same way as the equilibrium constant \(K_c\) except that the concentrations in \(Q_c\) are not necessarily equilibrium values. For a general reaction:
\(
\begin{aligned}
& \mathrm{aA}+\mathrm{b} \mathrm{B} \rightleftharpoons \mathrm{c} \mathrm{C}+\mathrm{d} \mathrm{D} \dots(7.19) \\
& Q_{\mathrm{c}}=[\mathrm{C}]^{\mathrm{c}}[\mathrm{D}]^{\mathrm{d}} /[\mathrm{A}]^{\mathrm{a}}[\mathrm{B}]^{\mathrm{b}} \dots(7.20)
\end{aligned}
\)
Then,
If \(Q_c>K_c\), the reaction will proceed in the direction of reactants (reverse reaction).
If \(Q_c<K_c\), the reaction will proceed in the direction of the products (forward reaction).
If \(Q_c=K_c\), the reaction mixture is already at equilibrium.

Consider the gaseous reaction of \(\mathrm{H}_2\) with \(\mathrm{I}_2\),
\(
\mathrm{H}_2(\mathrm{~g})+\mathrm{I}_2(\mathrm{~g}) \rightleftharpoons 2 \mathrm{HI}(\mathrm{g}) ; K_c=57.0 \text { at } 700 \mathrm{~K} \text {. }
\)
Suppose we have molar concentrations \(\left[\mathrm{H}_2\right]_{\mathrm{t}}=0.10 \mathrm{M},\left[\mathrm{I}_2\right]_{\mathrm{t}}=0.20 \mathrm{M}\) and \([\mathrm{HI}]_{\mathrm{t}}=0.40 \mathrm{M}\). (the subscript \(t\) on the concentration symbols means that the concentrations were measured at some arbitrary time \(\mathrm{t}\), not necessarily at equilibrium).
Thus, the reaction quotient, \(Q_c\) at this stage of the reaction is given by,
\(
\begin{aligned}
Q_c=[\mathrm{HI}]_{\mathrm{t}}^2 /\left[\mathrm{H}_2\right]_{\mathrm{t}}\left[\mathrm{I}_2\right]_{\mathrm{t}} & =(0.40)^2 /(0.10) \times(0.20) =8.0 \\
\end{aligned}
\)
Now, in this case, \(Q_c(8.0)\) does not equal \(K_c(57.0)\), so the mixture of \(\mathrm{H}_2(\mathrm{~g}), \mathrm{I}_2(\mathrm{~g})\) and \(\mathrm{HI}(\mathrm{g})\) is not at equilibrium; that is, more \(\mathrm{H}_2(\mathrm{~g})\) and \(\mathrm{I}_2(\mathrm{~g})\) will react to form more \(\mathrm{HI}(\mathrm{g})\) and their concentrations will decrease till \(Q_c=K_{c}\).
The reaction quotient, \(B_c\) is useful in predicting the direction of the reaction by comparing the values of \(Q_c\) and \(K_c\).
Thus, we can make the following generalisations concerning the direction of the reaction (Fig. 7.7) :

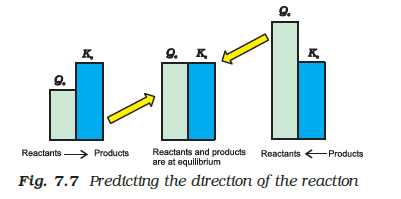
• If \(Q_c<K_c\), net reaction goes from left to right
• If \(Q_{\mathrm{c}}>K_c\), net reaction goes from right to left.
• If \(Q_c=K_c\), no net reaction occurs.

Example 7.7: The value of \(K_c\) for the reaction \(2 \mathrm{~A} \rightleftharpoons \mathrm{B}+\mathrm{C}\) is \(2 \times 10^{-3}\). At a given time, the composition of the reaction mixture is \([\mathrm{A}]=[\mathrm{B}]=[\mathrm{C}]=3 \times 10^{-4} \mathrm{M}\). In which direction the reaction will proceed?

Answer: For the reaction, the reaction quotient \(Q_c\) is given by,
\(
\begin{aligned}
& Q_c=[\mathrm{B}][\mathrm{C}] /[\mathrm{A}]^2 \\
& \text { as }[\mathrm{A}]=[\mathrm{B}]=[\mathrm{C}]=3 \times 10^{-4} \mathrm{M} \\
& Q_c=\left(3 \times 10^{-4}\right)\left(3 \times 10^{-4}\right) /\left(3 \times 10^{-4}\right)^2=1
\end{aligned}
\)
as \(Q_c>K_c\) so the reaction will proceed in the reverse direction.

Calculating Equilibrium Concentrations

In case of a problem in which we know the initial concentrations but do not know any of the equilibrium concentrations, the following three steps shall be followed:
step 1: Write the balanced equation for the reaction.
Step 2: Under the balanced equation, make a table that lists for each substance involved in the reaction:
(a) the initial concentration,
(b) the change in concentration on going to equilibrium, and
(c) the equilibrium concentration.
In constructing the table, define \(\mathrm{x}\) as the concentration (mol/L) of one of the substances that reacts on going to equilibrium, then use the stoichiometry of the reaction to determine the concentrations of the other substances in terms of \(\mathrm{x}\).
Step 3: Substitute the equilibrium| concentrations into the equilibrium equation for the reaction and solve for \(\mathrm{x}[latex]. If you are to solve a quadratic equation choose the mathematical solution that makes chemical sense.
Step 4: Calculate the equilibrium concentrations from the calculated value of [latex]x\).
Step 5: Check your results by substituting them into the equilibrium equation.

Example 7.8: \(13.8 \mathrm{~g}\) of \(\mathrm{N}_2 \mathrm{O}_4\) was placed in a \(1 \mathrm{~L}\) reaction vessel at \(400 \mathrm{~K}\) and allowed to attain equilibrium
\(
\mathrm{N}_2 \mathrm{O}_4(\mathrm{~g}) \rightleftharpoons 2 \mathrm{NO}_2(\mathrm{~g})
\)
The total pressure at equilibrium was found to be 9.15 bar. Calculate \(K_c, K_p\) and partial pressure at equilibrium.

Answer: We know \(p V=n \mathrm{R} T\)
Total volume \((V)=1 \mathrm{~L}\)
Molecular mass of \(\mathrm{N}_2 \mathrm{O}_4=92 \mathrm{~g}\)
Number of moles \(=13.8 \mathrm{~g} / 92 \mathrm{~g}=0.15\)
of the gas ( \(n\) )
Gas constant \((\mathrm{R})=0.083\) bar \(\mathrm{L} \mathrm{mol}^{-1} \mathrm{~K}^{-1}\)
Temperature \((T)=400 \mathrm{~K}\)
\(p V=n \mathrm{R} T\)
\(
p \times 1 \mathrm{~L}=0.15 \mathrm{~mol} \times 0.083 \mathrm{bar} \mathrm{L} \mathrm{~mol}^{-1} \mathrm{~K}^{-1} \times 400 \mathrm{~K}
\)
\(p=4.98\) bar
\(
\quad \quad \quad \quad \quad \quad \quad \mathrm{N}_2 \mathrm{O}_4 \rightleftharpoons 2 \mathrm{NO}_2
\)
\(
\text { Initial pressure: } 4.98 \text { bar } \quad 0
\)
At equilibrium: \((4.98-\mathrm{x})\) bar \(2 \mathrm{x}\) bar Hence,
\(
\begin{aligned}
& p_{\text {total }} \text { at equilibrium }=p_{\mathrm{N}_2 \mathrm{O}_4}+p_{\mathrm{NO}_2} \\
& 9.15=(4.98-\mathrm{x})+2 \mathrm{x} \\
& 9.15=4.98+\mathrm{x}
\end{aligned}
\)
\(
\mathrm{x}=9.15-4.98=4.17 \mathrm{bar}
\)
Partial pressures at equilibrium are,
\(
\begin{aligned}
& p_{\mathrm{N}_2 \mathrm{O}_4}=4.98-4.17=0.81 \mathrm{bar} \\
& p_{\mathrm{NO}_2}=2 \mathrm{x}=2 \times 4.17=8.34 \mathrm{bar}
\end{aligned}
\)
\(
K_p=\left(p_{N_2}\right)^2 / p_{N_2 O_4}
\)
\(
=(8.34)^2 / 0.81=85.87
\)
\(
\begin{aligned}
& K_p=K_c(\mathrm{R} T)^{\Delta n} \\
& 85.87=K_c(0.083 \times 400)^1 \\
& K_c=2.586=2.6
\end{aligned}
\)

Example 7.9: \(3.00 \mathrm{~mol}\) of \(\mathrm{PCl}_5\) kept in \(1 \mathrm{~L}\) closed reaction vessel was allowed to attain equilibrium at \(380 \mathrm{~K}\). Calculate the composition of the mixture at equilibrium. \(K_c=1.80\)

Answer:

\(
\quad \quad \quad \quad \quad \quad \quad \quad \mathrm{PCl}_5 \rightleftharpoons \mathrm{PCl}_3+\mathrm{Cl}_2
\)
Initial concentration: \(\quad 3.0 \quad \quad \quad 0 \quad \quad 0\)
Let \(\mathrm{x} \mathrm{~mol} \mathrm{~per} \mathrm{~litre} \mathrm{~of} \mathrm{~PCl}_5\) be dissociated,
At equilibrium:\(\quad \quad \quad \quad (3-x) \quad x \quad x\)
\(
K_c=\left[\mathrm{PCl}_3\right]\left[\mathrm{Cl}_2\right] /\left[\mathrm{PCl}_5\right]
\)
\(
\begin{aligned}
& 1.8=x^2 /(3-x) \\
& x^2+1.8 x-5.4=0
\end{aligned}
\)
\(
\begin{aligned}
& x=\frac{-1.8 \pm \sqrt{(1.8)^2-4 5 \times 4}}{2}\\
& x=[-1.8 \pm 4.98] / 2 \\
& x=[-1.8+4.98] / 2=1.59
\end{aligned}
\)
\(
\begin{aligned}
& {\left[\mathrm{PCl}_5\right]=3.0-\mathrm{x}=3-1.59=1.41 \mathrm{M}} \\
& {\left[\mathrm{PCl}_3\right]=\left[\mathrm{Cl}_2\right]=\mathrm{x}=1.59 \mathrm{M}}
\end{aligned}
\)