6.2 Equilibrium in Chemical Processes – Dynamic Equilibrium

Chemical equilibrium

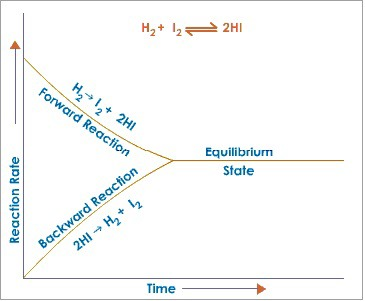
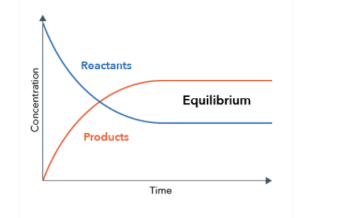
Chemical reactions can occur both in forward and backward directions. When the rates of the forward and reverse reactions become equal, the concentrations of the reactants and the products remain constant. This is the stage of chemical equilibrium. This equilibrium is dynamic in nature as it consists of a forward reaction in which the reactants give product(s) and reverse reaction in which product(s) gives the original reactants.

For a better comprehension, let us consider a general case of a reversible reaction,
\(
\mathrm{A}+\mathrm{B} \rightleftharpoons \mathrm{C}+\mathrm{D}
\)
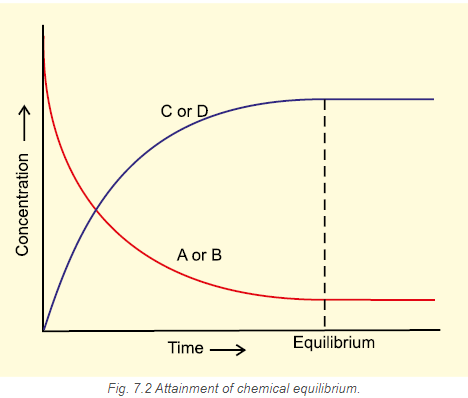
With the passage of time, there is an accumulation of the products \(\mathrm{C}\) and \(\mathrm{D}\) and depletion of the reactants A and B (Fig. 7.2). This leads to a decrease in the rate of forward reaction and an increase in the rate of the reverse reaction,. Eventually, the two reactions occur at the same rate and the system reaches a state of equilibrium.

Similarly, the reaction can reach the state of equilibrium even if we start with only \(\mathrm{C}\) and \(\mathrm{D}\); that is, no \(\mathrm{A}\) and \(\mathrm{B}\) being present initially, as the equilibrium can be reached from either direction.

Characteristics of Equilibrium states

• Equilibrium state can only be achieved if a reversible reaction is carried out in a closed space.
• Chemical equilibrium at a given temperature is characterised by the constancy of certain properties such as pressure, concentration, density or colour.
• At equilibrium each reactant and each product have a fixed concentration and it is independent of the fact whether we start the reaction with the reactants or with the products.

\(
\begin{aligned}
& 2 \mathrm{HI} \leftrightharpoons \mathrm{H}_2+\mathrm{I}_2 \\
& \mathrm{H}_2+\mathrm{I}_2 \leftrightharpoons 2 \mathrm{HI}
\end{aligned}
\)

• An equilibrium state is attained in a shorter time by the use of a positive catalyst.
• It is dynamic in nature. However, the reaction seems to have come to a standstill because the concentration of reactants and products does not change.

Why Is Chemical Equilibrium Called Dynamic Equilibrium?

Chemical reactions can either go in both directions (forward and reverse) or only in one direction. The ones that go in two directions are known as reversible reactions, and you can identify them by the arrows going in two directions, like the example below.
\(
\mathrm{H} 2 \mathrm{O}(\mathrm{l}) \rightleftharpoons \mathrm{H}^{+}(\mathrm{aq})+\mathrm{OH}^{-}(\mathrm{aq})
\)
Dynamic equilibrium only occurs in reversible reactions, and it’s when the rate of the forward reaction is equal to the rate of the reverse reaction. These equations are dynamic because the forward and reverse reactions are still occurring, but the two rates are equal and unchanging, so they’re also at equilibrium.

Dynamic equilibrium is an example of a system in a steady state. This means the variables in the equation are unchanging over time (since the rates of reaction are equal). If you look at a reaction in dynamic equilibrium, it’ll look like nothing is happening since the concentrations of each substance stay constant. However, reactions are actually continuously occurring.

Dynamic equilibrium doesn’t just occur in chemistry labs though; you’ve witnessed a dynamic equilibrium example every time you’ve had a soda. In a sealed bottle of soda, carbon dioxide is present in both the liquid/aqueous phase and the gaseous phase (bubbles). The two phases of carbon dioxide are in dynamic equilibrium inside the sealed soda bottle since the gaseous carbon dioxide is dissolving into the liquid form at the same rate that the liquid form of carbon dioxide is being converted back to its gaseous form.

The equation looks like this: \(\mathrm{CO}_2(\mathrm{~g}) \rightleftharpoons \mathrm{CO}_2(\mathrm{aq})\).

Changing the temperature, pressure, or concentration of a reaction can shift the equilibrium of an equation and knock it out of dynamic equilibrium. This is why, if you open a soda can and leave it out for a long time, eventually it’ll become “flat” and there will be no more bubbles. This is because the soda can is no longer a closed system and the carbon dioxide can interact with the atmosphere. This moves it out of dynamic equilibrium and releases the gaseous form of carbon dioxide until there are no more bubbles.

Example: Closed soda bottle of pop

• \(\mathrm{CO}_2\) gas leaving dissolved state and entering gas state
• \(\mathrm{CO}_2\) gas also leaving gas state and entering liquid state
• No visible change
  \(
  \mathrm{CO}_{2(\mathrm{~g})} \rightleftharpoons \mathrm{CO}_{2(\mathrm{aq})}
  \)

Differences between dynamic and Static equilibrium

\(
\begin{array}{|l|c|}
\hline \text { Dynamic Equilibrium } & \text { Static Equilibrium } \\
\hline \text { Reversible } & \text { Irreversible } \\
\hline \text { Reaction is still occurring } & \text { Reaction has stopped } \\
\hline \text { Rate of forward reaction = rate of reverse reaction } & \text { Both reaction rates are zero } \\
\hline \text { Occurs in a closed system } & \text { Can occur in an open or closed system } \\
\hline
\end{array}
\)

Dynamic equilibrium: synthesis of ammonia by Haber’s process

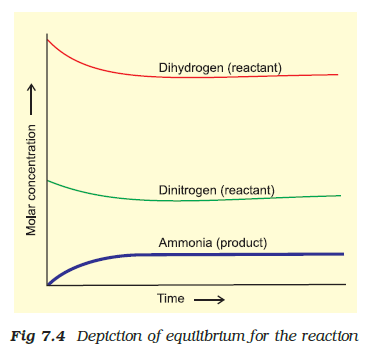
The dynamic nature of chemical equilibrium can be demonstrated in the synthesis of ammonia by Haber’s process. In a series of experiments, Haber started with known amounts of dinitrogen and dihydrogen maintained at high temperature and pressure and at regular intervals determined the amount of ammonia present. He was successful in determining also the concentration of unreacted dihydrogen and dinitrogen. Fig. 7.4 shows that after a certain time the composition of the mixture remains the same even though some of the reactants are still present. This constancy in composition indicates that the reaction has reached equilibrium.

In order to understand the dynamic nature of the reaction, synthesis of ammonia is carried out with exactly the same starting conditions (of partial pressure and temperature) but using \(\mathrm{D}_2\) (deuterium) in place of \(\mathrm{H}_2\). The reaction mixtures starting either with \(\mathrm{H}_2\) or \(\mathrm{D}_2\) reach equilibrium with the same composition, except that \(\mathrm{D}_2\) and \(\mathrm{ND}_3\) are present instead of \(\mathrm{H}_2\) and \(\mathrm{NH}_3\). After equilibrium is attained, these two mixtures
\(
\mathrm{N}_2(\mathrm{~g})+3 \mathrm{H}_2(\mathrm{~g}) \rightleftharpoons 2 \mathrm{NH}_3(\mathrm{~g})
\)
\(\left(\mathrm{H}_2, \mathrm{~N}_2, \mathrm{NH}_3\right.\) and \(\left.\mathrm{D}_2, \mathrm{~N}_2, \mathrm{ND}_3\right)\) are mixed together and left for a while. Later, when this mixture is analysed, it is found that the concentration of ammonia is just the same as before. However, when this mixture is analysed by a mass spectrometer, it is found that ammonia and all deuterium-containing forms of ammonia \(\left(\mathrm{NH}_3, \mathrm{NH}_2 \mathrm{D}, \mathrm{NHD}_2\right.\) and \(\left.\mathrm{ND}_3\right)\) and dihydrogen and its deutrated forms \(\left(\mathrm{H}_2, \mathrm{HD}\right.\) and \(\left.\mathrm{D}_2\right)\) are present. Thus one can conclude that scrambling of \(\mathrm{H}\) and \(\mathrm{D}\) atoms in the molecules must result from a continuation of the forward and reverse reactions in the mixture. If the reaction had simply stopped when they reached equilibrium, then there would have been no mixing of isotopes in this way.

The use of isotope (deuterium) in the formation of ammonia clearly indicates that chemical reactions reach a state of dynamic equilibrium in which the rates of forward and reverse reactions are equal and there is no net change in composition.

Equilibrium can be attained from both sides, whether we start reaction by taking, \(\mathrm{H}_2(\mathrm{~g})\) and \(\mathrm{N}_2(\mathrm{~g})\) and get \(\mathrm{NH}_3(\mathrm{~g})\) or by taking \(\mathrm{NH}_3(\mathrm{~g})\) and decomposing it into \(\mathrm{N}_2(\mathrm{~g})\) and \(\mathrm{H}_2\) (g).
\(
\mathrm{N}_2(\mathrm{~g})+3 \mathrm{H}_2(\mathrm{~g}) \rightleftharpoons 2 \mathrm{NH}_3(\mathrm{~g})
\)
\(
2 \mathrm{NH}_3(\mathrm{~g}) \rightleftharpoons \mathrm{N}_2(\mathrm{~g})+3 \mathrm{H}_2(\mathrm{~g})
\)

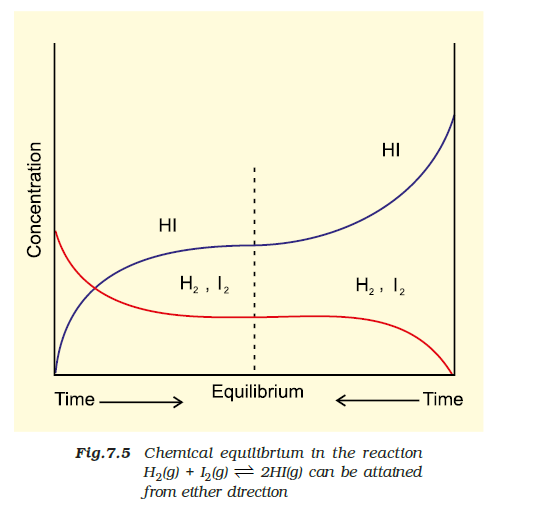
Similarly let us consider the reaction, \(\mathrm{H}_2(\mathrm{~g})+\mathrm{I}_2(\mathrm{~g}) \rightleftharpoons 2 \mathrm{HI}(\mathrm{g})\). If we start with equal initial concentration of \(\mathrm{H}_2\) and \(\mathrm{I}_2\), the reaction proceeds in the forward direction and the concentration of \(\mathrm{H}_2\) and \(\mathrm{I}_2\) decreases while that of \(\mathrm{HI}\) increases, until all of these become constant at equilibrium (Fig. 7.5). We can also start with \(\mathrm{HI}\) alone and make the reaction to proceed in the reverse direction; the concentration of \(\mathrm{HI}\) will decrease and concentration of \(\mathrm{H}_2\) and \(\mathrm{I}_2\) will increase until they all become constant when equilibrium is reached (Fig.7.5). If total number of \(\mathrm{H}\) and \(\mathrm{I}\) atoms are same in a given volume, the same equilibrium mixture is obtained whether we start it from pure reactants or pure product.