6.1 Equilibrium in Physical Processes

The characteristics of system at equilibrium are better understood if we examine some physical processes. The most familiar examples are phase transformation processes, e.g.,

\(
\begin{aligned}
& \text { solid } \rightleftharpoons \text { liquid } \\
& \text { liquid } \rightleftharpoons \text { gas } \\
& \text { solid } \rightleftharpoons \text { gas }
\end{aligned}
\)

Solid-Liquid Equilibrium

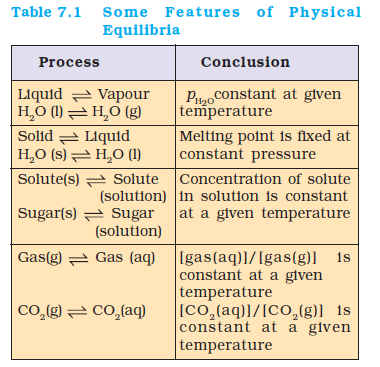
At \(0^{\circ} \mathrm{C}\)(\(273 \mathrm{~K}\)), the number of water molecules becoming ice is equal to the water molecules as the ice melts to form liquid water. The rate of freezing of water is equal to the rate of melting of ice. Thus, there is an equilibrium between solid ice and liquid water.
\(
\text { Ice }(s) \rightleftharpoons \text { Water }(\mathrm{l})
\)
The number of molecules of a liquid becoming vapour will be equal to the number of molecules condensing into liquid in a closed container. The rate of evaporation of liquid water is equal to the rate of condensation of water vapour. The liquid phase is in equilibrium with its own vapour phase.
\(
\text { Water }(\mathrm{l}) \rightleftharpoons \text { Water }(\mathrm{g})
\)

It is obvious that ice and water are in equilibrium only at particular temperature and pressure. For any pure substance at atmospheric pressure, the temperature at which the solid and liquid phases are at equilibrium is called the normal melting point or normal freezing point of the substance. The system here is in dynamic equilibrium and we can infer the following:

• Both the opposing processes occur simultaneously.
• Both the processes occur at the same rate so that the amount of ice and water remains constant.

Liquid-Vapour Equilibrium

At equilibrium, rate of evaporation = rate of condensation
\(
\mathrm{H}_2 \mathrm{O}(\mathrm{l}) \rightleftharpoons \mathrm{H}_2 \mathrm{O} \text { (vap) }
\)
At equilibrium the pressure exerted by the water molecules at a given temperature remains constant and is called the equilibrium vapour pressure of water (or just vapour pressure of water);vapour pressure of water increases with temperature.

Water and water vapour are in equilibrium position at atmospheric pressure (1.013 bar) and at \(100^{\circ} \mathrm{C}\) in a closed vessel. The boiling point of water is \(100^{\circ} \mathrm{C}\) at 1.013 bar pressure. For any pure liquid at one atmospheric pressure (1.013 bar), the temperature at which the liquid and vapours are at equilibrium is called normal boiling point of the liquid. Boiling point of the liquid depends on the atmospheric pressure. It depends on the altitude of the place; at high altitude the boiling point decreases.

Solid – Vapour Equilibrium

Let us now consider the systems where solids sublime to vapour phase. If we place solid iodine in a closed vessel, after some time the vessel gets filled up with violet vapour and the intensity of colour increases with time. After certain time the intensity of colour becomes constant and at this stage equilibrium is attained. Hence solid iodine sublimes to give iodine vapour and the iodine vapour condenses to give solid iodine. The equilibrium can be represented as,
\(
\mathrm{I}_2(\mathrm{~s}) \rightleftharpoons \mathrm{I}_2 \text { (Vapour) }
\)
At equilibrium, the rate of sublimation \(=\) The rate of deposition
Other examples showing this kind of equilibrium are,
Camphor (solid) \(\rightleftharpoons\) Camphor (vapour)
\(\mathrm{NH}_4 \mathrm{Cl}\) (solid) \(\rightleftharpoons \mathrm{NH}_4 \mathrm{Cl}\) (vapour)

Equilibrium Involving Dissolution of Solid or Gases in Liquids

Solids in liquids

Let’s consider the dissolution of sugar in water as an example. The concentration of the solute in a saturated solution depends upon the temperature. In a saturated solution, a dynamic equilibrium exits between the solute molecules in the solid state and in the solution:
\(
\text { Sugar (solution) } \rightleftharpoons \text { Sugar (solid) },
\)
and the rate of dissolution of sugar = rate of crystallisation of sugar.

Gases in liquids

When a soda water bottle is opened, some of the carbon dioxide gas dissolved in it fizzes out rapidly. The phenomenon arises due to the difference in solubility of carbon dioxide at different pressures. There is an equilibrium between the molecules in the gaseous state and the molecules dissolved in the liquid under pressure i.e.,
\(
\mathrm{CO}_2 \text { (gas) } \rightleftharpoons \mathrm{CO}_2 \text { (in solution) }
\)
This equilibrium is governed by Henry’s law, which states that the mass of a gas dissolved in a given mass of a solvent at any temperature is proportional to the pressure of the gas above the solvent. This amount decreases with increase of temperature. The soda water bottle is sealed under pressure of gas when its solubility in water is high. As soon as the bottle is opened, some of the dissolved carbon dioxide gas escapes to reach a new equilibrium condition required for the lower pressure, namely its partial pressure in the atmosphere. This is how the soda water in bottle when left open to the air for some time, turns ‘flat’. It can be generalised that:

• For solid \(\rightleftharpoons\) liquid equilibrium, there is only one temperature (melting point) at \(1 \mathrm{~atm}\) (1.013 bar) at which the two phases can coexist. If there is no exchange of heat with the surroundings, the mass of the two phases remains constant.
• For liquid \(\rightleftharpoons\) vapour equilibrium, the vapour pressure is constant at a given temperature.
• For dissolution of solids in liquids, the solubility is constant at a given temperature.
• For dissolution of gases in liquids, the concentration of a gas in liquid is proportional to the pressure (concentration) of the gas over the liquid. These observations are summarised in Table 7.1

General Characteristics of Equilibria Involving Physical Processes

For the physical processes discussed above, the following characteristics are common to the system at equilibrium:

• Equilibrium is possible only in a closed system at a given temperature.
• Both the opposing processes occur at the same rate and there is a dynamic but stable condition.
• All measurable properties of the system remain constant.
• When equilibrium is attained for a physical process, it is characterised by a constant value of one of its parameters at a given temperature. Table 7.1 lists such quantities.
• The magnitude of such quantities at any stage indicates the extent to which the physical process has proceeded before reaching equilibrium.