5.9 Exercise Problems and Solutions

Q1. Choose the correct answer. A thermodynamic state function is a quantity
(i) used to determine heat changes
(ii) whose value is independent of path
(iii) used to determine pressure-volume work
(iv) whose value depends on temperature only.

Answer: A thermodynamic state function is a quantity whose value is independent of a path. Functions like \(p, V, T\), etc. depend only on the state of a system and not on the path. Hence, alternative (ii) is correct.

Q2. For the process to occur under adiabatic conditions, the correct condition is:
(i) \(\Delta T=0\)
(ii) \(\Delta p=0\)
(iii) \(q=0\)
(iv) \(\mathrm{w}=0\)

Answer: A system is said to be under adiabatic conditions if there is no exchange of heat between the system and its surroundings. Hence, under adiabatic conditions, \(\mathrm{q}=0\).
Therefore, alternative (iii) is correct.

Q3. The enthalpies of all elements in their standard states are:
(i) unity
(ii) zero
(iii) \(<0\)
(iv) different for each element

Answer: The enthalpy of all elements in their standard state is zero. Therefore, alternative (ii) is correct.

Q4. \(\Delta U^{\ominus}\) of combustion of methane is \(-\mathrm{X} \mathrm{~kJ} \mathrm{~mol}{ }^{-1}\). The value of \(\Delta H^{\ominus}\) is
(i) \(=\Delta U^{\ominus}\)
(ii) \(>\Delta U^{\ominus}\)
(iii) \(<\Delta U^{\ominus}\)
(iv) \(=0\)

Answer: The balanced chemical equation for the combustion reaction is :
\(
\mathrm{CH}_4(g)+2 \mathrm{O}_2(g) \longrightarrow \mathrm{CO}_2(g)+2 \mathrm{H}_2 \mathrm{O}(l)
\)
\(
\Delta_{n g}=1-3=-2
\)
\(
\Delta \mathrm{H}^{\ominus}=\Delta \mathrm{U}^{\ominus}+\Delta_{n g} \mathrm{R} T=\Delta \mathrm{U}^{\ominus}-2 \mathrm{R} T
\)
\(
\therefore \Delta \mathrm{H}^{\ominus}<\Delta \mathrm{U}^{\ominus} \text { or (iii) is the correct answer. }
\)

Q5. The enthalpy of combustion of methane, graphite and dihydrogen at \(298 \mathrm{~K}\) are, \(-890.3 \mathrm{~kJ} \mathrm{~mol}^{-1}, -393.5 \mathrm{~kJ} \mathrm{~mol}^{-1}\) and \(-285.8 \mathrm{~kJ} \mathrm{~mol}^{-1}\) respectively. Enthalpy of formation of \(\mathrm{CH}_4(\mathrm{~g})\) will be
(i) \(-74.8 \mathrm{~kJ} \mathrm{~mol}^{-1}\)
(ii) \(-52.27 \mathrm{~kJ} \mathrm{~mol}^{-1}\)
(iii) \(+74.8 \mathrm{~kJ} \mathrm{~mol}^{-1}\)
(iv) \(+52.26 \mathrm{~kJ} \mathrm{~mol}^{-1}\).

Answer: As per the available data :
(i) \(\quad \mathrm{CH}_4(g)+2 \mathrm{O}_2(g) \longrightarrow \mathrm{CO}_2(g)+2 \mathrm{H}_2 \mathrm{O}(l) ; \quad \Delta_{\mathrm{C}} \mathrm{H}^{\ominus}=-890.3 \mathrm{~kJ} \mathrm{~mol}^{-1}\)
\(
\text { (ii) } \quad \mathrm{C}(\mathrm{s})+\mathrm{O}_2(\mathrm{~g}) \longrightarrow \mathrm{CO}_2(\mathrm{~g}) ; \Delta_c \mathrm{H}^{\ominus}=-393.5 \mathrm{~kJ} \mathrm{~mol}^{-1}
\)
\(
\text { (iii) } \quad \mathrm{H}_2(g)+1 / 2 \mathrm{O}_2(g) \longrightarrow \mathrm{H}_2 \mathrm{O}(l) ; \quad \Delta_c \mathrm{H}^{\ominus}=-285.8 \mathrm{~kJ} \mathrm{~mol}^{-1}
\)
The equation we aim at:
\(
\mathrm{C}(\mathrm{s})+2 \mathrm{H}_2(\mathrm{~g}) \longrightarrow \mathrm{CH}_4(\mathrm{~g}) ; \quad \Delta_f \mathrm{H}^{\ominus}=?
\)
Eqn. (ii) \(+2 \times\) Eqn. (iii) – Eqn. (i) and the correct \(\Delta_f \mathrm{H}^{\ominus}\) value is:
\(
=(-393.5)+2 \times(-285.8)-(-890.3)=-74.8 \mathrm{~kJ} \mathrm{~mol}^{-1}
\)
\(\therefore(i)\) is the correct answer.

Q6. A reaction, \(A+B \rightarrow C+D+q\) is found to have a positive entropy change. The reaction will be
(i) Possible at high temperature
(ii) possible only at low temperature
(iii) not possible at any temperature
(iv) possible at any temperature

Answer: For a reaction to be spontaneous, \(\Delta \mathrm{G}\) should be negative.
\(
\Delta \mathrm{G}=\Delta \mathrm{H}-\mathrm{T} \Delta \mathrm{S}
\)
According to the question, for the given reaction,
\(
\begin{aligned}
& \Delta \mathrm{S}=\text { positive } \\
& \Delta \mathrm{H}=\text { negative (since heat is evolved) } \\
& \Rightarrow \Delta \mathrm{G}=\text { negative }
\end{aligned}
\)
Therefore, the reaction is spontaneous at any temperature. Hence, alternative (iv) is correct.

Q7. In a process, \(701 \mathrm{~J}\) of heat is absorbed by a system and \(394 \mathrm{~J}\) of work is done by the system. What is the change in internal energy for the process?

Answer: According to the first law of thermodynamics,
\(\Delta U=q+W \dots(i)\)
where, \(\Delta U=\) change in internal energy for a process, q = heat
\(W=\) work Given,
\(q=+701 \mathrm{~J}\) (Since heat is absorbed)
\(\mathrm{W}=-394 \mathrm{~J}\) (Since work is done by the system)
Substituting the values in expression (i), we get
\(
\begin{aligned}
& \Delta U=701 \mathrm{~J}+(-394 \mathrm{~J}) \\
& \Delta U=307 \mathrm{~J}
\end{aligned}
\)
Hence, the change in internal energy for the given process is \(307 \mathrm{~J}\).

Q8. The reaction of cyanamide, \(\mathrm{NH}_2 \mathrm{CN}\) (s), with dioxygen, was carried out in a bomb calorimeter, and \(\Delta U\) was found to be \(-742.7 \mathrm{~kJ} \mathrm{~mol}^{-1}\) at \(298 \mathrm{~K}\). Calculate the enthalpy change for the reaction at \(298 \mathrm{~K}\).
\(
\mathrm{NH}_2 \mathrm{CN}(\mathrm{g})+\frac{3}{2} \mathrm{O}_2(\mathrm{~g}) \rightarrow \mathrm{N}_2(\mathrm{~g})+\mathrm{CO}_2(\mathrm{~g})+\mathrm{H}_2 \mathrm{O}(\mathrm{l})
\)

Answer: Enthalpy change for a reaction \((\Delta \mathrm{H})\) is given by the expression,
\(
\Delta \mathrm{H}=\Delta \mathrm{U}+\Delta n_g R T
\)
Where,
\(\Delta \mathrm{U}=\) change in internal energy
\(\Delta n_g=\) change in number of moles
For the given reaction,
\(
\begin{aligned}
& \Delta \mathrm{ng}=\sum n_g \text { (products) }-\sum n_g \text { (reactants) } \\
& =(2-2.5) \text { moles } \\
& \Delta \mathrm{ng}=-0.5 \text { moles }
\end{aligned}
\)
And,
\(
\begin{aligned}
& \Delta \mathrm{U}=-742.7 \mathrm{~kJ} \mathrm{~mol}^{-1} \\
& \mathrm{~T}=298 \mathrm{~K} \\
& \mathrm{R}=8.314 \times 10^{-3} \mathrm{~kJ} \mathrm{~mol}^{-1} \mathrm{~K}
\end{aligned}
\)
Substituting the values in the expression of \(\Delta \mathrm{H}\) :
\(
\begin{aligned}
& \Delta \mathrm{H}=\left(-742.7 \mathrm{~kJ} \mathrm{~mol}^{-1}\right)+(-0.5 \mathrm{~mol})(298 \mathrm{~K})\left(8.314 \times 10^{-3} \mathrm{~kJ} \mathrm{~mol}^{-1} \mathrm{~K}^{-1}\right)=-742.7-1.2 \\
& \Delta \mathrm{H}=-743.9 \mathrm{~kJ} \mathrm{~mol}^{-1}
\end{aligned}
\)

Q9. Calculate the number of \(\mathrm{kJ}\) of heat necessary to raise the temperature of \(60.0 \mathrm{~g}\) of aluminum from \(35^{\circ} \mathrm{C}\) to \(55^{\circ} \mathrm{C}\). Molar heat capacity of \(\mathrm{Al}\) is \(24 \mathrm{~J} \mathrm{~mol}^{-1} \mathrm{~K}^{-1}\).

Answer:

\(
\begin{aligned}
& \text {No. of moles of } \mathrm{Al}(\mathrm{m})=(60 \mathrm{~g}) /\left(27 \mathrm{~g} \mathrm{~mol}^{-1}\right)=2.22 \mathrm{~mol} \\
& \text { Molar heat capacity }(\mathrm{C})=24 \mathrm{~J} \mathrm{~mol}^{-1} \mathrm{~K}^{-1} \text {. } \\
& \text { Rise in temperature }(\Delta \mathrm{T})=55-35=20^{\circ} \mathrm{C}=20 \mathrm{~K} \\
& \text { Heat evolved }(\mathrm{q})=\mathrm{C} \times \mathrm{m} \times {\Delta \mathrm{T}}=\left(24 \mathrm{~J} \mathrm{~mol}^{-1} \mathrm{~K}^{-1}\right) \times(2.22 \mathrm{~mol}) \times(20 \mathrm{~K}) \\
& =1065.6 \mathrm{~J}=1.067 \mathrm{kJ}
\end{aligned}
\)

Q10. Calculate the enthalpy change on freezing of \(1.0 \mathrm{~mol}\) of water at \(10.0^{\circ} \mathrm{C}\) to ice at \(-10.0^{\circ} \mathrm{C}\). \(\Delta_{\text {fus }} H=6.03 \mathrm{~kJ} \mathrm{~mol}^{-1}\) at \(0^{\circ} \mathrm{C}\).
\(
\begin{aligned}
& C_p\left[\mathrm{H}_2 \mathrm{O}(l)\right]=75.3 \mathrm{~J} \mathrm{~mol}^{-1} \mathrm{~K}^{-1} \\
& C_p\left[\mathrm{H}_2 \mathrm{O}(\mathrm{s})\right]=36.8 \mathrm{~J} \mathrm{~mol}^{-1} \mathrm{~K}^{-1}
\end{aligned}
\)

Answer: The total enthalpy change involved in the transformation is the sum of the following changes:
(a) Energy change involved in the transformation of \(1 \mathrm{~mol}\) of water at \(10^{\circ} \mathrm{C}\) to \(1 \mathrm{~mol}\) of water at \(0^{\circ} \mathrm{C}\).
(b) Energy change involved in the transformation of \(1 \mathrm{~mol}\) of water at \(0^{\circ}\) to \(1 \mathrm{~mol}\) of ice at \(0^{\circ} \mathrm{C}\).
(c) Energy change involved in the transformation of \(1 \mathrm{~mol}\) of ice at \(0^{\circ} \mathrm{C}\) to \(1 \mathrm{~mol}\) of ice at \(-10^{\circ} \mathrm{C}\).
\(
\text { Total } \Delta H=C_p\left[H_2 \mathrm{OCl}\right] \Delta T+\Delta H_{\text {freezing }}+C_p\left[H_2 O_{(s)}\right] \Delta T
\)
\(
=\left(75.3 \mathrm{~J} \mathrm{~mol}^{-1} \mathrm{~K}^{-1}\right)(0-10) \mathrm{K}+\left(-6.03 \times 103 \mathrm{~J} \mathrm{~mol}^{-1}\right)+\left(36.8 \mathrm{~J} \mathrm{~mol}^{-1} \mathrm{~K}^{-1}\right)(-10-0) \mathrm{K}
\)
\(
\begin{aligned}
& =-753 \mathrm{~J} \mathrm{~mol}^{-1}-6030 \mathrm{~J} \mathrm{~mol}^{-1}-368 \mathrm{~J} \mathrm{~mol}^{-1} \\
& =-7151 \mathrm{~J} \mathrm{~mol}^{-1} \\
& =-7.151 \mathrm{~kJ} \mathrm{~mol}^{-1}
\end{aligned}
\)
Hence, the enthalpy change involved in the transformation is \(-7.151 \mathrm{~kJ} \mathrm{~mol}^{-1}\).

Q11. Enthalpy of combustion of carbon to \(\mathrm{CO}_2\) is \(-393.5 \mathrm{~kJ} \mathrm{~mol}^{-1}\). Calculate the heat released upon formation of \(35.2 \mathrm{~g}\) of \(\mathrm{CO}_2\) from carbon and dioxygen gas.

Answer: Formation of \(\mathrm{CO}_2\) from carbon and dioxygen gas can be represented using the combustion equation
\(
C_{(s)}+O_{2(g)} \rightarrow \mathrm{CO}_{2(g)}
\)
\(
\Delta_c H=-393.5 k J \mathrm{~mol}^{-1}
\)
\(
(1 \mathrm{~mole}=44 \mathrm{~g})
\)
Heat released on formation of \(44 \mathrm{g} \mathrm{~CO}_2=-393.5 \mathrm{~kJ} \mathrm{~mol}^{-1}\)
\(\therefore\) Heat released on formation of \(35.2 \mathrm{g} \mathrm{~CO}_2\)
\(
\begin{aligned}
& =\frac{-393.5 \mathrm{~kJ} \mathrm{~mol}^{-1}}{44 \mathrm{~g}} \times 35.2 \mathrm{~g} \\
& =-314.8 \mathrm{~kJ} \mathrm{~mol}^{-1}
\end{aligned}
\)

Q12. Enthalpies of formation of \(\mathrm{CO}(\mathrm{g}), \mathrm{CO}_2(\mathrm{~g}), \mathrm{N}_2 \mathrm{O}(\mathrm{g})\) and \(\mathrm{N}_2 \mathrm{O}_4(\mathrm{~g})\) are \(-110,-393,81\) and \(9.7 \mathrm{~kJ} \mathrm{~mol}^{-1}\) respectively. Find the value of \(\Delta_{\mathrm{r}} H\) for the reaction:
\(
\mathrm{N}_2 \mathrm{O}_4(\mathrm{~g})+3 \mathrm{CO}(\mathrm{g}) \rightarrow \mathrm{N}_2 \mathrm{O}(\mathrm{g})+3 \mathrm{CO}_2(\mathrm{~g})
\)

Answer: \(\Delta_r \mathrm{H}\) for a reaction is defined as the difference between \(\Delta_f H\) value of products and \(\Delta_f H\) value of reactants.
\(
\Delta_{\mathrm{r}} \mathrm{H}=\text { (Sum of enthalpy of formation of products) – (sum of enthalpy of reactants) }
\)
\(
\Delta_r H=\sum \Delta_f H \text { (products) }-\sum \Delta_f H \text { (reactants) }
\)
For the given reaction,
\(
\mathrm{N}_2 \mathrm{O}_{4(g)}+3 \mathrm{CO}_{(g)} \longrightarrow \mathrm{N}_2 \mathrm{O}_{(g)}+3 \mathrm{CO}_{2(g)}
\)
Given
\(
\Delta_f \mathrm{H} \text { of } \mathrm{CO}(\mathrm{g})=-110 \mathrm{~KJ} / \mathrm{mol}
\)
\(
\Delta_f \mathrm{H} \text { of } \mathrm{CO_2}(\mathrm{g})=-393 \mathrm{~KJ} / \mathrm{mol}
\)
\(
\Delta_f \mathrm{H} \text { of } \mathrm{N}_2 \mathrm{O}(\mathrm{g})=81 \mathrm{~KJ} / \mathrm{mol}
\)
\(
\Delta_f \mathrm{H} \text { of } \mathrm{N}_2 \mathrm{O}_4(\mathrm{~g})=9.7 \mathrm{KJ} / \mathrm{mol}
\)
Products are \(\mathrm{N}_2 \mathrm{O}\) and \(\mathrm{CO}_2\)
Reactants are \(\mathrm{N}_2 \mathrm{O}_4\) and \(\mathrm{CO}\)
\(
\therefore \Delta_{\mathrm{r}} \mathrm{H}=\left[\Delta_{\mathrm{f}} \mathrm{H}\left(\mathrm{N}_2 \mathrm{O}\right)+3 \Delta_{\mathrm{f}} \mathrm{H}\left(\mathrm{CO}_2\right)\right]-\left[\Delta_{\mathrm{f}} \mathrm{H}\left(\mathrm{N}_2 \mathrm{O}_4\right)+3 \Delta_{\mathrm{f}} \mathrm{H}(\mathrm{CO})\right]
\)
By substituting the values given, we get
\(
\begin{aligned}
& \Rightarrow \Delta_{\mathrm{r}} \mathrm{H}=[81 \mathrm{~kJ} / \mathrm{mol}+3 \times(-393 \mathrm{~kJ} / \mathrm{mol})]-[9.7 \mathrm{~kJ} / \mathrm{mol}+3 \times(-110 \mathrm{~kJ} / \mathrm{mol})] \\
& \Rightarrow \Delta_{\mathrm{r}} \mathrm{H}=-777.7 \mathrm{~kJ} / \mathrm{mol}=-778 \mathrm{~kJ} \mathrm{~mol}^{-1}
\end{aligned}
\)
Thus, the value of \(\Delta_{\mathrm{r}} \mathrm{H}\) for the reaction is \(-778 \mathrm{~kJ} / \mathrm{mol}\).
Note: Enthalpy of reaction \(\left(\Delta_{\mathrm{r}} \mathrm{H}\right)\) is actually the difference between the enthalpies of the products and the reactants when the quantities of the reactants indicated by the chemical equation have completely reacted.

Q13. Given
\(
\mathrm{N}_2(\mathrm{~g})+3 \mathrm{H}_2(\mathrm{~g}) \rightarrow 2 \mathrm{NH}_3(\mathrm{~g}) ; \Delta_{\mathrm{r}} H^{\ominus}=-92.4 \mathrm{~kJ} \mathrm{~mol}^{-1}
\)
What is the standard enthalpy of formation of \(\mathrm{NH}_3\) gas?

Answer: Standard enthalpy of formation of a compound is the change in enthalpy that takes place during the formation of 1 mole of a substance in its standard form from its constituent elements in their standard state.
Re-writing the given equation for 1 mole of \(\mathrm{NH}_{3(g)}\),
\(
\frac{1}{2} N_{2(g)}+\frac{3}{2} H_{2(g)} \rightarrow N H_{3(g)}
\)
\(\therefore\) Standard enthalpy of formation of \(\mathrm{NH}_3(\mathrm{~g})\)
\(
\begin{aligned}
& =1 / 2 \Delta_r H^\theta \\
& =1 / 2\left(-92.4 k J \mathrm{~mol}^{-1}\right) \\
& =-46.2 \mathrm{~kJ} \mathrm{~mol}^{-1}
\end{aligned}
\)

Q14. Calculate the standard enthalpy of formation of \(\mathrm{CH}_3 \mathrm{OH}(\mathrm{l})\) from the following data:
\(
\mathrm{CH}_3 \mathrm{OH}(\mathrm{l})+\frac{3}{2} \mathrm{O}_2(\mathrm{~g}) \rightarrow \mathrm{CO}_2(\mathrm{~g})+2 \mathrm{H}_2 \mathrm{O}(\mathrm{l}) ; \Delta_r H^{\ominus}=-726 \mathrm{~kJ} \mathrm{~mol}^{-1} \dots(i)
\)
\(
\mathrm{C} \text { (graphite) }+\mathrm{O}_2(\mathrm{~g}) \rightarrow \mathrm{CO}_2(\mathrm{~g}) ; \Delta_c H^{\ominus}=-393 \mathrm{~kJ} \mathrm{~mol}^{-1} \dots(ii)
\)
\(
\mathrm{H}_2(\mathrm{~g})+\frac{1}{2} \mathrm{O}_2(\mathrm{~g}) \rightarrow \mathrm{H}_2 \mathrm{O}(\mathrm{l}) ; \Delta_f H^{\ominus}=-286 \mathrm{~kJ} \mathrm{~mol}^{-1} \dots(iii)
\)

Answer:

\(
\text { The reaction takes place during the formation of } \mathrm{CH}_3 \mathrm{OH}(\mathrm{l}) \text { can be written as: }
\)
\(\mathrm{C}(\mathrm{s})+2 \mathrm{H}_2(\mathrm{~g})+\mathrm{1} / 2 \mathrm{O}_2\) (g) \(—>\mathrm{CH}_3 \mathrm{OH}(\mathrm{l}) ;; \Delta_f H^{\ominus}= \pm ? \ldots\) (iv)
The reaction (iv) can be obtained from the given reactions by following the algebraic calculations as Equation (ii) \(+2 \times\) equation (iii) – equation (i)
\(
\Delta_f H^\theta\left[\mathrm{CH}_3 \mathrm{OH}(\mathrm{l})\right]=\Delta_c H^\theta+2 \Delta_f H^\theta\left[H_2 O{(l)}\right]-\Delta_r H^\theta
\)
\(
=\left(-393 \mathrm{~kJ} \mathrm{~mol}^{-1}\right)+2\left(-286 \mathrm{~kJ} \mathrm{~mol}^{-1}\right)-\left(-726 \mathrm{~kJ} \mathrm{~mol}^{-1}\right)
\)
\(
=(-393-572+726) \mathrm{kJ} \mathrm{mol}^{-1}
\)
\(
\therefore \Delta_f H^\theta\left[\mathrm{CH}_3 \mathrm{OH}(\mathrm{l})\right]=-239 \mathrm{~kJ} \mathrm{~mol}^{-1}
\)

Q15. Calculate the enthalpy change for the process
\(
\mathrm{CCl}_4(\mathrm{~g}) \rightarrow \mathrm{C}(\mathrm{g})+4 \mathrm{Cl}(\mathrm{g})
\)
and calculate bond enthalpy of \(\mathrm{C}-\mathrm{Cl}\) in \(\mathrm{CCl}_4(\mathrm{~g})\).
\(
\begin{aligned}
& \Delta_{\text {vap }} H^{\ominus}\left(\mathrm{CCl}_4\right)=30.5 \mathrm{~kJ} \mathrm{~mol}^{-1} . \\
& \Delta_f H^{\ominus}\left(\mathrm{CCl}_4\right)=-135.5 \mathrm{~kJ} \mathrm{~mol}^{-1} . \\
& \Delta_a H^{\ominus}(\mathrm{C})=715.0 \mathrm{~kJ} \mathrm{~mol}^{-1}, \text { where } \Delta_a H^{\ominus} \text { is enthalpy of atomisation } \\
& \Delta_a H^{\ominus}\left(\mathrm{Cl}_2\right)=242 \mathrm{~kJ} \mathrm{~mol}^{-1}
\end{aligned}
\)

Answer: The available data is:
\(
\text { (i) } \quad \mathrm{CCl}_4(l) \longrightarrow \mathrm{CCl}_4(g), \quad \Delta_{\mathrm{vap}} \mathrm{H}^{\ominus}=30.5 \mathrm{~kJ} \mathrm{~mol}^{-1}
\)
\(
\text { (ii) } \quad \mathrm{C}(\mathrm{s})+2 \mathrm{Cl}_2(\mathrm{~g}) \longrightarrow \mathrm{CCl}_4(l), \Delta_f \mathrm{H}^{\ominus}=-135.5 \mathrm{~kJ} \mathrm{~mol}^{-1}
\)
\(
\text { (iii) } \quad \mathrm{C}(\mathrm{s}) \longrightarrow \mathrm{C}(g), \quad \Delta_a \mathrm{H}^{\ominus}=715.0 \mathrm{~kJ} \mathrm{~mol}^{-1}
\)
\(
\text { (iv) } \quad \mathrm{Cl}_2(\mathrm{~g}) \longrightarrow 2 \mathrm{Cl}(\mathrm{g}), \quad \Delta_a \mathrm{H}^{\ominus}=242 \mathrm{~kJ} \mathrm{~mol}^{-1}
\)
The equation we aim at is:
\(
\mathrm{CCl}_4(\mathrm{~g}) \longrightarrow \mathrm{C}(\mathrm{g})+4 \mathrm{Cl}(\mathrm{g}) ; \Delta \mathrm{H}=\text { ? }
\)
Eqn. (iii) \(+2 \times\) Eqn. (iv) – Eqn. (i) – Eqn. (ii) gives the required equation with
\(
\begin{aligned}
\Delta \mathrm{H} & =715.0+2(242)-30.5-(-135.5) \mathrm{kJ} \mathrm{mol}^{-1} \\
& =1304 \mathrm{~kJ} \mathrm{~mol}^{-1}
\end{aligned}
\)
\(
\text { Bond enthalpy of } \mathrm{C}-\mathrm{Cl} \text { in } \mathrm{CCl}_4 \text { (average value) }=\frac{1304}{4}=326 \mathrm{~kJ} \mathrm{~mol}^{-1} \text {. }
\)

Q16. For an isolated system, \(\Delta U=0\), what will be \(\Delta S\) ?

Answer: \(\Delta \mathrm{S}\) will be positive i.e., greater than zero Since \(\Delta U=0, \Delta S\) will be positive and the reaction will be spontaneous.

Q17. For the reaction at \(298 \mathrm{~K}\),
\(
\begin{aligned}
& 2 \mathrm{~A}+\mathrm{B} \rightarrow \mathrm{C} \\
& \Delta H=400 \mathrm{~kJ} \mathrm{~mol}^{-1} \text { and } \Delta \mathrm{S}=0.2 \mathrm{~kJ} \mathrm{~K}^{-1} \mathrm{~mol}^{-1}
\end{aligned}
\)
At what temperature will the reaction become spontaneous considering \(\Delta H\) and \(\Delta S\) to be constant over the temperature range?

Answer: As per the Gibbs Helmholtz equation:
\(
\Delta \mathrm{G}=\Delta \mathrm{H}-\mathrm{T} \Delta \mathrm{S}
\)
Assuming the reaction at equilibrium, T for the reaction would be:
\(
\begin{aligned}
& T=(\Delta H-\Delta G) \frac{1}{\Delta S} \\
& =\frac{\Delta H}{\Delta S} \quad(\Delta G=0 \text { at equilibrium }) \\
& T=\frac{400 \mathrm{~kJ} \mathrm{~mol}^{-1}}{0.2 \mathrm{~kJ} \mathrm{~K}^{-1} \mathrm{~mol}^{-1}} \\
& T=2000 \mathrm{~K}
\end{aligned}
\)
Thus, the reaction will be in a state of equilibrium at \(2000 \mathrm{~K}\) and will be spontaneous above this temperature. For the reaction to be spontaneous, \(\Delta \mathrm{G}\) must be negative. Hence, for the given reaction to be spontaneous, \(\mathrm{T}\) should be greater than \(2000 \mathrm{~K}\).

Q18. For the reaction, \(2 \mathrm{Cl}(\mathrm{g}) \rightarrow \mathrm{Cl}_2(\mathrm{~g})\), what are the signs of \(\Delta H\) and \(\Delta \mathrm{S}\) ?

Answer: \(\Delta \mathrm{H}\) is negative (bond energy is released) and \(\Delta \mathrm{S}\) is negative (There is less randomness among the molecules than among the atoms)
Explanation: \(\Delta \mathrm{H}\) and \(\Delta \mathrm{S}\) are negative
The given reaction represents the formation of chlorine molecules from chlorine atoms.
Here, bond formation is taking place. Therefore, energy is being released. Hence, \(\Delta \mathrm{H}\) is negative.
Also, two moles of atoms have more randomness than one mole of a molecule. Since spontaneity is decreased, \(\Delta S\) is negative for the given reaction.

Q19. For the reaction
\(
\begin{aligned}
& 2 \mathrm{~A}(\mathrm{~g})+\mathrm{B}(\mathrm{g}) \rightarrow 2 \mathrm{D}(\mathrm{g}) \\
& \Delta U^{\ominus}=-10.5 \mathrm{~kJ} \text { and } \Delta \mathrm{S}^{\ominus}=-44.1 \mathrm{JK}^{-1} .
\end{aligned}
\)
Calculate \(\Delta G^{\ominus}\) for the reaction, and predict whether the reaction may occur spontaneously.

Answer: For the reaction
\(
2 \mathrm{~A}(\mathrm{~g})+\mathrm{B}(\mathrm{g}) \rightarrow 2 \mathrm{D}(\mathrm{g})
\)
\(
\begin{aligned}
& \Delta n_g=2-3 \\
& =-1 \text { mole }
\end{aligned}
\)
\(
\Delta \mathrm{H}^{\ominus}=\Delta \mathrm{U}^{\ominus}+\Delta n_g R T
\)
\(
\Delta \mathrm{U}^{\ominus}=-10.5 \mathrm{~kJ}
\)
\(
R=8.314 \times 10^{-3} \mathrm{~kJ} \mathrm{~K}^{-1} \mathrm{~mol}^{-1} ; \mathrm{T}=298 \mathrm{~K}
\)
\(
\begin{aligned}
\Delta \mathrm{H}^{\ominus} & =(-10.5 \mathrm{~kJ})+\left[(-1 \mathrm{~mol}) \times\left(8.314 \times 10^{-3} \mathrm{~kJ} \mathrm{~K}^{-1} \mathrm{~mol}^{-1}\right) \times(298 \mathrm{~K})\right] \\
& =-10.5 \mathrm{~kJ}-2.478 \mathrm{~kJ}=-12.978 \mathrm{~kJ}
\end{aligned}
\)
According to Gibbs Helmholtz equation:
\(
\Delta \mathrm{G}^{\ominus}=\Delta \mathrm{H}^{\ominus}-T \Delta S^{\ominus}
\)
\(\Delta \mathrm{G}^{\ominus}=(-12.978 \mathrm{~kJ})-(298 \mathrm{~K}) \times\left(-0.0441 \mathrm{~kJ} \mathrm{~K}^{-1}\right)\)
\(
=-12.978+13.112=-12.978+13.142=\mathbf{0 . 1 6 4} \mathbf{k J}
\)
Since \(\Delta G^\theta\) for the reaction is positive, the reaction will not occur spontaneously.

Q20. The equilibrium constant for a reaction is 10. What will be the value of \(\Delta G^{\ominus}\)?
\(
\mathrm{R}=8.314 \mathrm{JK}^{-1} \mathrm{~mol}^{-1}, \mathrm{~T}=300 \mathrm{~K} .
\)

Answer:

\(
\Delta \mathrm{G}^{\ominus}=-\mathrm{R} T \ln \mathrm{K}=-2.303 \mathrm{R} T \log \mathrm{K}
\)
\(
\mathrm{R}=8.314 \mathrm{JK}^{-1} \mathrm{~mol}^{-1} ; \mathrm{T}=300 \mathrm{~K} ; \mathrm{K}=10
\)
\(
\Delta \mathrm{G}^{\ominus}=-2.303 \times 8.314 \mathrm{JK}^{-1} \mathrm{~mol}^{-1} \times(300 \mathrm{~K}) \times \log 10=-5.744 \mathrm{~kJ} \mathrm{~mol}^{-1}
\)

Q21. Comment on the thermodynamic stability of \(\mathrm{NO}(\mathrm{g})\), given
\(
\frac{1}{2} \mathrm{~N}_2(\mathrm{~g})+\frac{1}{2} \mathrm{O}_2(\mathrm{~g}) \rightarrow \mathrm{NO}(\mathrm{g}) \quad ; \quad \Delta_{\mathrm{r}} H^{\ominus}=90 \mathrm{~kJ} \mathrm{~mol}^{-1}
\)
\(
\mathrm{NO}(\mathrm{g})+\frac{1}{2} \mathrm{O}_2(\mathrm{~g}) \rightarrow \mathrm{NO}_2(\mathrm{~g}): \Delta_{\mathrm{r}} H^{\ominus}=-74 \mathrm{~kJ} \mathrm{~mol}^{-1}
\)

Answer: For \(\mathrm{NO}(g) ; \Delta_r \mathrm{H}^{\ominus}=+\) ve : Unstable in nature
For \(\mathrm{NO}_2(g) ; \Delta_r \mathrm{H}^{\ominus}=-\) ve : Stable in nature (is formed)
Explanation:
The positive value of \(\Delta_r \mathrm{H}\) indicates that heat is absorbed during the formation of \(\mathrm{NO}(\mathrm{g})\). This means that \(\mathrm{NO}(\mathrm{g})\) has higher energy than the reactants \(\left(\mathrm{N}_2\right.\) and \(\left.\mathrm{O}_2\right)\). Hence, \(\mathrm{NO}(\mathrm{g})\) is unstable. The negative value of \(\Delta_r \mathrm{H}\) indicates that heat is evolved during the formation of \(\mathrm{NO_2} (\mathrm{~g})\) from \(\mathrm{NO}(\mathrm{g})\) and \(\mathrm{O}_2(\mathrm{~g})\). The product, \(\mathrm{NO}_2(\mathrm{~g})\) is stabilized with minimum energy. Hence, unstable \(\mathrm{NO}(\mathrm{g})\) changes to unstable \(\mathrm{NO}_2(g)\).

Q22. Calculate the entropy change in surroundings when \(1.00 \mathrm{~mol} \mathrm{~of} \mathrm{~H}_2 \mathrm{O}(l)\) is formed under standard conditions. \(\Delta_f H^{\ominus}=-286 \mathrm{~kJ} \mathrm{~mol}^{-1}\).

Answer: It is given that \(286 \mathrm{~kJ} \mathrm{~mol}^{-1}\) of heat is evolved on the formation of \(1 \mathrm{~mol}\) of \(\mathrm{H}_2 \mathrm{O}(\mathrm{l})\). Thus, an equal amount of heat will be absorbed by the surroundings.
\(
q_{\mathrm{rev}}=\left(-\Delta_f \mathrm{H}^{\ominus}\right)=-286 \mathrm{~kJ} \mathrm{~mol}^{-1}=286000 \mathrm{~J} \mathrm{~mol}^{-1}=+286 \mathrm{~kJ} \mathrm{~mol}^{-1}
\)
\(
\Delta S_{\text {(Surroundings) }}=\frac{q_{\text {rev }}}{\mathrm{T}}=\frac{\left(286000 \mathrm{~J} \mathrm{~mol}^{-1}\right)}{298 \mathrm{~K}}=959.73 \mathrm{~J} \mathrm{~K}^{-1} \mathrm{~mol}^{-1}
\)

Exemplar Problems

Q23. \(18.0 \mathrm{~g}\) of water completely vapourises at \(100^{\circ} \mathrm{C}\) and \(1 \mathrm{bar}\) pressure and the enthalpy change in the process is \(40.79 \mathrm{~kJ} \mathrm{~mol}^{-1}\). What will be the enthalpy change for vapourising two moles of water under the same conditions? What is the standard enthalpy of vapourisation for water?

Answer: Given that, quantity of water \(=18.0 \mathrm{~g}\), pressure \(=1 \mathrm{bar}\)
As we know that, \(18.0 \mathrm{~g} \mathrm{~H}_2 \mathrm{O}=1\) mole \(H_2 \mathrm{O}\)
Enthalpy change for vaporising 1 mole of \(\mathrm{H}_2 \mathrm{O}=40.79 \mathrm{~kJ} \mathrm{~mol}^{-1}\)
\(\therefore\) Enthalpy change for vaporising 2 moles of \(\mathrm{H}_2 \mathrm{O}=2 \times 40.79 \mathrm{~kJ}=81.358 \mathrm{~kJ}\)
Standard enthalpy of vaporisation at \(100^{\circ} \mathrm{C}\) and 1 bar pressure, \(\Delta_{\text {vap }} H^{\ominus}=+40.79 \mathrm{~kJ} \mathrm{~mol}^{-1}\)

Q24. One mole of acetone requires less heat to vapourise than \(1 \mathrm{~mol}\) of water. Which of the two liquids has a higher enthalpy of vapourisation?

Answer: One mole of acetone requires less heat to vaporise than 1 mole of water. Hence, acetone has less enthalpy of vaporisation and water has higher enthalpy of vaporisation. It can be represented as \(
\left(\Delta \mathrm{H}_r\right)_{\text {water }}>\left(\Delta \mathrm{H}_r\right)_{\text {acetone }}\)

Q25. Standard molar enthalpy of formation, \(\Delta_f H^{\ominus}\) is just a special case of enthalpy of reaction, \(\Delta_r H^{\ominus}\). Is the \(\Delta_r H^{\ominus}\) for the following reaction same as \(\Delta_f H^{\ominus}\)? Give reason for your answer.
\(
\mathrm{CaO}(\mathrm{s})+\mathrm{CO}_2(\mathrm{~g}) \rightarrow \mathrm{CaCO}_3(\mathrm{~s}) ; \quad \Delta_f H^{\ominus}=-178.3 \mathrm{~kJ} \mathrm{~mol}^{-1}
\)

Answer: No, since \(\mathrm{CaCO}_3\) has been formed from other compounds and not from its constituent elements.
Standard molar enthalpy of formation is the enthalpy of the reaction when 1 mole of compound is formed from its constituent elements.
\(
\mathrm{Ca}(\mathrm{s})+\mathrm{C}(\mathrm{s})+\frac{3}{2} \mathrm{O}_2(\mathrm{~g}) \rightarrow \mathrm{CaCO}_3(\mathrm{~s}) ; \quad \Delta_f H^{\ominus}
\)
As the close reaction is different from the given reaction, \(\therefore \quad \Delta_r H^{\ominus} \neq \Delta_f H^{\ominus}\)

Q26. The value of \(\Delta_f H^{\ominus}\) for \(\mathrm{NH}_3\) is \(-91.8 \mathrm{~kJ} \mathrm{~mol}^{-1}\). Calculate the enthalpy change for the following reaction :
\(
2 \mathrm{NH}_3(\mathrm{~g}) \rightarrow \mathrm{N}_2(\mathrm{~g})+3 \mathrm{H}_2(\mathrm{~g})
\)

Answer:

\(
\text { Given, } \frac{1}{2} \mathrm{~N}_2(\mathrm{~g})+\frac{3}{2} \mathrm{H}_2(\mathrm{~g}) \rightarrow \mathrm{NH}_3(\mathrm{~g}) ; \Delta_{\mathrm{f}} \mathrm{H}^{\ominus}=-91.8 \mathrm{~kJ} \mathrm{~mol}^{-1}
\)
\(
\left(\Delta_{\mathrm{f}} \mathrm{H}^{\ominus} \text { means enthalpy of formation of } 1 \text { mole of } \mathrm{NH}_3\right. \text { ) }
\)
\(
\therefore \text { Enthalpy change for the formation of } 2 \text { moles of } \mathrm{NH}_3
\)
\(
\mathrm{N}_2(\mathrm{~g})+3 \mathrm{H}_2(\mathrm{~g}) \rightarrow 2 \mathrm{NH}_3(\mathrm{~g}) ; \Delta_{\mathrm{f}} \mathrm{H}^{\ominus}=2 \times-91.8=-183.6 \mathrm{~kJ} \mathrm{~mol}^{-1}
\)
And for the reverse reaction,
\(
2 \mathrm{NH}_3(\mathrm{~g}) \rightarrow \mathrm{N}_2(\mathrm{~g})+3 \mathrm{H}_2(\mathrm{~g}) ; \Delta_{\mathrm{r}} \mathrm{H}^{\ominus}=+183.6 \mathrm{~kJ} \mathrm{~mol}^{-1}
\)
\(
\text { Hence, the value of } \Delta_{\mathrm{r}} \mathrm{H}^{\ominus} \text { for } \mathrm{NH}_3 \text { is }+183.6 \mathrm{~kJ} \mathrm{~mol}^{-1}
\)

Q27. Enthalpy is an extensive property. In general, if the enthalpy of an overall reaction A \(\rightarrow \mathrm{B}\) along one route is \(\Delta_r H\) and \(\Delta_r H_1, \Delta_r H_2, \Delta_r H_3 \ldots .\). represent enthalpies of intermediate reactions leading to product \(\mathrm{B}\). What will be the relation between \(\Delta_r H\) for overall reaction and \(\Delta_r H_1, \Delta_r H_2 \ldots \ldots\) etc. for intermediate reactions?

Answer: In general, if the enthalpy of an overall reaction \(\mathrm{A} \rightarrow \mathrm{B}\) along one route is \(\Delta_{\mathrm{r}} \mathrm{H}\) and \(\Delta_{\mathrm{r}} \mathrm{H}_1, \Delta_{\mathrm{r}} \mathrm{H}_2, \Delta_{\mathrm{r}} \mathrm{H}_3 \ldots \ldots\). representing enthalpies of reaction leading to same product \(\mathrm{B}\) along another route, then we have by Hess’s law
\(
\Delta_{\mathrm{r}} \mathrm{H}=\Delta_{\mathrm{r}} \mathrm{H}_1+\Delta_{\mathrm{r}} \mathrm{H}_2+\Delta_{\mathrm{r}} \mathrm{H}_3 \ldots \ldots \ldots
\)

Q28. The enthalpy of atomisation for the reaction \(\mathrm{CH}_4(\mathrm{~g}) \rightarrow \mathrm{C}(\mathrm{g})+4 \mathrm{H}(\mathrm{g})\) is \(1665 \mathrm{~kJ} \mathrm{~mol}^{-1}\). What is the bond energy of \(\mathrm{C}-\mathrm{H}\) bond?

Answer: In \(\mathrm{CH}_4\), there are four \(\mathrm{C}-\mathrm{H}\) bonds. The enthalpy of atomisation of 1 mole of \(\mathrm{CH}_4\) means dissociation of four moles of \(\mathrm{C}\) – \(\mathrm{H}\) bond. \(\therefore \mathrm{C}-\mathrm{H}\) bond energy per mol \(=\frac{1665 \mathrm{~kJ}}{4 \mathrm{~mol}}=416.25 \mathrm{~kJ} \mathrm{~mol}^{-1}\)

Q29. Use the following data to calculate \(\Delta_{\text {lattice }} H^{\ominus}\) for NaBr.
\(\Delta_{\text {sub }} H^{\ominus}\) for sodium metal \(=108.4 \mathrm{~kJ} \mathrm{~mol}^{-1}\)
Ionization enthalpy of sodium \(=496 \mathrm{~kJ} \mathrm{~mol}^{-1}\)
Electron gain enthalpy of bromine \(=-325 \mathrm{~kJ} \mathrm{~mol}^{-1}\)
Bond dissociation enthalpy of bromine \(=192 \mathrm{~kJ} \mathrm{~mol}^{-1}\)
\(\Delta_f H^{\ominus}\) for \(\mathrm{NaBr}(\mathrm{s})=-360.1 \mathrm{~kJ} \mathrm{~mol}^{-1}\)

Answer: \(
\text { Given that, } \Delta_{\text {sub }} \mathrm{H}^{\ominus} \text { for } \mathrm{Na} \text { metal }=108.4 \mathrm{k} \mathrm{J} \mathrm{mol}^{-1}
\)
\(
\text { IE of } \mathrm{Na}=496 \mathrm{k} \mathrm{J} \mathrm{~mol}^{-1}
\)
\(
\Delta_{\mathrm{eg}} \mathrm{H}^{\ominus} \text { of } \mathrm{Br}=-325 \mathrm{k} \mathrm{J} \mathrm{~mol}^{-1}
\)
\(
\Delta_{\text {diss }} \mathrm{H}^{\ominus} \text { of } \mathrm{Br}=192 \mathrm{~kJ} \mathrm{~mol}^{-1}
\)
\(
\Delta_{\mathrm{f}} \mathrm{H}^{\ominus} \text { for } \mathrm{NaBr}=-360.1 \mathrm{k} \mathrm{J} \mathrm{~mol}^{-1}
\)
Born-Haber cycle for the formation of \(\mathrm{NaBr}\) is as

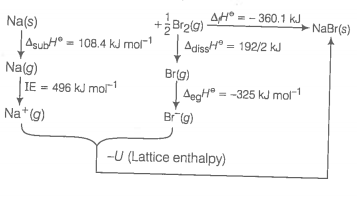
By applying Hess’s law,
\(
\begin{aligned}
& \Delta_{\mathrm{f}} \mathrm{H}^{\ominus}=\Delta_{\mathrm{sub}} \mathrm{H}^{\ominus}+\mathrm{IE}+\Delta_{\text {diss }} \mathrm{H}^{\ominus}+\Delta_{\text {eg }} \mathrm{H}^{\ominus}+\mathrm{U} \\
& -360.1=108.4+496+96+(-325)-\mathrm{U} \\
& \mathrm{U}=+735.5 \mathrm{~kJ} \mathrm{~mol}^{-1}
\end{aligned}
\)

Q30. Given that \(\Delta H=0\) for mixing of two gases. Explain whether the diffusion of these gases into each other in a closed container is a spontaneous process or not.

Answer: It is a spontaneous process. Although enthalpy change is zero but randomness or disorder ( \(\Delta S\) ) increases and \(\Delta S\) is positive. Therefore, in the equation, \(\Delta G=\Delta H-T \Delta S\), the term \(T \Delta S\) will be negative. Hence \(\Delta G\) will be negative.

Q31. Heat has randomising influence on a system and temperature is the measure of the average chaotic motion of particles in the system. Write the mathematical relation that relates these three parameters.

Answer: Heat has randomising influence on a system and temperature is the measure of the average chaotic motion of particles in the system. The mathematical relation which relates these three parameters is \(\Delta S=\frac{\mathrm{q}_{\mathrm{rev}}}{\mathrm{T}}\)
Here, \(\Delta \mathrm{S}=\) change in entropy \(\mathrm{q}_{\mathrm{rev}}=\) heat of reversible reaction \(\mathrm{T}=\) temperature

Q32. Increase in the enthalpy of the surroundings is equal to decrease in the enthalpy of the system. Will the temperature of the system and surroundings be the same when they are in thermal equilibrium?

Answer: Yes, when the system and the surroundings are in thermal equilibrium, their temperatures are the same.

Q33. At \(298 \mathrm{~K} . K_p\) for the reaction \(\mathrm{N}_2 \mathrm{O}_4(\mathrm{~g}) \rightleftharpoons 2 \mathrm{NO}_2(\mathrm{~g})\) is 0.98 . Predict whether the reaction is spontaneous or not.

Answer: \(\Delta_{\mathrm{r}} \mathrm{G}^{\ominus}=-R T \ln K_p\)
\(=-R T \ln (0.98)\)
Since \(\ln (0.98)\) is negative
\(\therefore \Delta_{\mathrm{r}} \mathrm{G}^{\ominus}\) is positive, hence the reaction is non-spontaneous.

Q34. A sample of \(1.0 \mathrm{~mol}\) of a monoatomic ideal gas is taken through a cyclic process of expansion and compression as shown in Figure below. What will be the value of \(\Delta H\) for the cycle as a whole?

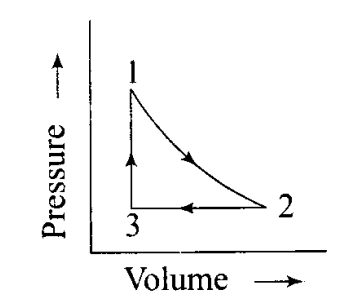
Answer: The net enthalpy change, \(\Delta \mathrm{H}\) for a cyclic process is zero as enthalpy change is a state function, i.e., \(\Delta \mathrm{H}\) (cycle) \(=0\)

Q35. The standard molar entropy of \(\mathrm{H}_2 \mathrm{O}(l)\) is \(70 \mathrm{~J} \mathrm{~K}^{-1} \mathrm{~mol}^{-1}\). Will the standard molar entropy of \(\mathrm{H}_2 \mathrm{O}(\mathrm{s})\) be more, or less than \(70 \mathrm{~J} \mathrm{~K}^{-1} \mathrm{~mol}^{-1}\) ?

Answer: Less, because ice is more ordered than \(\mathrm{H}_2 \mathrm{O}(l)\).
The standard molar entropy of \(\mathrm{H}_2 \mathrm{O}(l)\) is \(70 \mathrm{~J} \mathrm{~K}^{-1} \mathrm{~mol}^{-1}\). The solid form of \(\mathrm{H}_2 \mathrm{O}\) is ice. In ice, molecules of \(\mathrm{H}_2 \mathrm{O}\) are less random than in liquid water. Thus, molar entropy of \(\mathrm{H}_2 \mathrm{O}(\mathrm{s})<\) molar entropy of \(\mathrm{H}_2 \mathrm{O}\) (l). The standard molar entropy of \(\mathrm{H}_2 \mathrm{O}(\mathrm{s})\) is less than \(70 \mathrm{~J} \mathrm{~K}^1 \mathrm{~mol}^{-1}\).

Q36. Identify the state functions and path functions out of the following: enthalpy, entropy, heat, temperature, work, and free energy.

Answer: State functions are those values that depend only on the state of the system and not on how it is reached e.g., enthalpy, entropy, temperature and free energy. Path functions are those values that depend on the path of the system. e.g., heat and work.

Q37. The molar enthalpy of vapourisation of acetone is less than that of water. Why?

Answer: Amount of heat required to vaporise one mole of a liquid at constant temperature and under standard pressure \((1\) bar \()\) is called its molar enthalpy of vaporisation \(\Delta_{\mathrm{vap}} \mathrm{H}^{\ominus}\). The molar enthalpy of vaporisation of water is more than that of acetone because there is strong hydrogen bonding in \(\mathrm{H}_2 \mathrm{O}\) formula.

Q38. Which quantity out of \(\Delta_r \mathrm{G}\) and \(\Delta_r \mathrm{G}^{\ominus}\) will be zero at equilibrium?

Answer: Gibbs energy for a reaction in which all reactants and products are in standard state. \(\Delta_{\mathrm{r}} \mathrm{G}^{\ominus}\) is related to the equilibrium constant of the reaction as follows \(\Delta_{\mathrm{r}} \mathrm{G}=\Delta_{\mathrm{r}} \mathrm{G}^{\ominus}+\mathrm{RTlnK}\) 
At equilibrium, \(0=\Delta_{\mathrm{r}} \mathrm{G}^{\ominus}+\mathrm{RTlnK}\) \(\quad\left(\therefore \Delta_{\mathrm{r}} \mathrm{G}=0\right)\)
or \(\Delta_{\mathrm{r}} \mathrm{G}^{\ominus}=-\mathrm{RTlnK}\) 
\(\Delta_{\mathrm{r}} \mathrm{G}^{\ominus}=0\), when \(\mathrm{K}=1\)
For all other values of K. \(\Delta_{\mathrm{r}} \mathrm{G}^{\ominus}\) will be non-zero.

Q39. Predict the change in internal energy for an isolated system at constant volume.

Answer: For isolated systems, there is no transfer of energy as heat or as work i.e., \(\mathrm{w}=0\) and \(\mathrm{q}=0\). According to the first law of thermodynamics.
\(
\begin{aligned}
\Delta U & =q+\mathrm{w} \\
& =0+0=0 \\
\therefore \quad \Delta U & =0
\end{aligned}
\)

Q40. Although heat is a path function but heat absorbed by the system under certain specific conditions is independent of the path. What are those conditions? Explain.

Answer: The two conditions under which heat becomes independent of path are
(i) when volume remains constant
(ii) when pressure remains constant
At constant volume
By the first law of thermodynamics:
\(
\begin{aligned}
q & =\Delta U+(-\mathrm{w}) \\
(-\mathrm{w}) & =\mathrm{p} \Delta V \\
\therefore \quad q & =\Delta U+\mathrm{p} \Delta V \\
\Delta V & =0, \text { since volume is constant. } \\
\therefore \quad q_{v} & =\Delta U+0 \\
\Rightarrow \quad q_{v} & =\Delta U=\text { change in internal energy }
\end{aligned}
\)
At constant pressure
\(
q_p=\Delta U+p \Delta V
\)
But, \(\Delta U+p \Delta V=\Delta H\)
\(
\therefore \quad q_p=\Delta H=\text { change in enthalpy. }
\)
So, at a constant volume and at constant pressure heat change is a state function because it is equal to change in internal energy and change in enthalpy respectively which are state functions.

Q41. Expansion of a gas in vacuum is called free expansion. Calculate the work done and the change in internal energy when 1 litre of ideal gas expands isothermally into vacuum until its total volume is 5 litre?

Answer: Work done of a gas in vacuum, \(\mathrm{w}=-\mathrm{p}_{\text {ext }}\left(\mathrm{V}_2-\mathrm{V}_1\right)\).
\(
(-\mathrm{w})=p_{\text {ext }}\left(V_2-V_1\right)=0 \times(5-1)=0
\)
For isothermal expansion \(q=0\) By first law of thermodynamics
\(
\begin{aligned}
q & =\Delta U+(-\mathrm{w}) \\
\Rightarrow \quad 0 & =\Delta U+0 \text { so } \Delta U=0
\end{aligned}
\)

Q42. Heat capacity \(\left(C_p\right)\) is an extensive property but specific heat (c) is an intensive property. What will be the relation between \(C_p\) and \(\mathrm{c}\) for \(1 \mathrm{~mol}\) of water?

Answer: For water, heat capacity \(=18 \times\) specific heat or \(\mathrm{C}_p=18 \times \mathrm{c}\)
Specific heat \(=\mathrm{c}=4.18 \mathrm{Jg}^{-1} \mathrm{~K}^{-1}\)
Heat capacity \(=\mathrm{C}_p=18 \times 4.18 \mathrm{JK}^{-1}=75.3 \mathrm{~J} \mathrm{~K}^{-1}\)

Q43. The difference between \(C_p\) and \(C_V\) can be derived using the empirical relation \(H=U+p V\). Calculate the difference between \(\mathrm{C}_p\) and \(\mathrm{C}_V\) for 10 moles of an ideal gas.

Answer: Given that, \(\mathrm{C}_{\mathrm{v}}=\) heat capacity at constant volume, \(\mathrm{C}_{\mathrm{p}}=\) heat capacity at constant pressure Difference between \(\mathrm{C}_{\mathrm{p}}\) and \(\mathrm{C}_{\mathrm{v}}\) is equal to gas constant (R).
\(
\begin{aligned}
& \therefore \mathrm{C}_{\mathrm{p}}-\mathrm{C}_{\mathrm{v}}=\mathrm{nR} \quad \text { (where, } \mathrm{n}=\text { no. of moles) } \\
& =10 \times 4.184 \mathrm{~J} \\
& =41.84 \mathrm{~J}
\end{aligned}
\)
(where, \(\mathrm{n}=\) no. of moles)

Q44. If the combustion of \(1 \mathrm{~g}\) of graphite produces \(20.7 \mathrm{~kJ}\) of heat, what will be molar enthalpy change? Give the significance of the sign also.

Answer: Molar enthalpy change for graphite

\((\Delta H)=\text { enthalpy change for } 1 \mathrm{~g} \text { carbon } \times \text { molar mass of carbon }\)
\(
\begin{aligned}
& \Delta \mathrm{H}=-20.7 \mathrm{kJg}^{-1} \times 12 \mathrm{~g} \mathrm{~mol}^{-1} \\
& \Delta \mathrm{H}=-2.48 \times 10^2 \mathrm{~kJ} \mathrm{~mol}^{-1}
\end{aligned}
\)
Negative value of \(\Delta H \Rightarrow\) exothermic reaction.

Q45. The net enthalpy change of a reaction is the amount of energy required to break all the bonds in reactant molecules minus the amount of energy required to form all the bonds in the product molecules. What will be the enthalpy change for the following reaction?
\(
\mathrm{H}_2(\mathrm{~g})+\mathrm{Br}_2(\mathrm{~g}) \rightarrow 2 \mathrm{HBr}(\mathrm{g})
\)
Given that Bond energy of \(\mathrm{H}_2, \mathrm{Br}_2\) and \(\mathrm{HBr}\) is \(435 \mathrm{~kJ} \mathrm{~mol}^{-1}, 192 \mathrm{~kJ} \mathrm{~mol}^{-1}\) and \(368 \mathrm{~kJ} \mathrm{~mol}^{-1}\) respectively.

Answer:

\(
\Delta_r H^{\ominus}=\Sigma \mathrm{B} \cdot \mathrm{E}_{\text {(reactants) }}-\Sigma \mathrm{B} \cdot \mathrm{E}_{\text {(products) }}
\)
\(
\Delta_r H^{\ominus}=\text { Bond energy of } \mathrm{H}_2+\text { Bond energy of } \mathrm{Br}_2-2 \times \text { Bond energy of } \mathrm{HBr}
\)
\(
\begin{aligned}
& =435+192-(2 \times 368) \mathrm{kJ} \mathrm{mol}^{-1} \\
\Rightarrow \Delta_r H^{\ominus} & =-109 \mathrm{~kJ} \mathrm{~mol}^{-1}
\end{aligned}
\)

Q46. The enthalpy of vapourisation of \(\mathrm{CCl}_4\) is \(30.5 \mathrm{~kJ} \mathrm{~mol}^{-1}\). Calculate the heat required for the vapourisation of \(284 \mathrm{~g}\) of \(\mathrm{CCl}_4\) at constant pressure. (Molar mass of \(\left.\mathrm{CCl}_4=154 \mathrm{~g} \mathrm{~mol}^{-1}\right)\).

Answer: Given that, \(1 \mathrm{~mol}\) of \(\mathrm{CCl}_4=154 \mathrm{~g}\)
\(
\mathrm{q}_p=\Delta \mathrm{H}=30.5 \mathrm{~kJ} \mathrm{~mol}^{-1}
\)
\(\therefore\) Heat required for vapourisation of \(284 \mathrm{~g} \mathrm{of} \mathrm{CCl}_4=\frac{284 \mathrm{~g}^{-1}}{154 \mathrm{~g} \mathrm{~mol}^{-1}} \times 30.5 \mathrm{~kJ} \mathrm{~mol}^{-1}\)
\(
=56.2 \mathrm{~kJ}
\)

Q47. The enthalpy of reaction for the reaction : \(2 \mathrm{H}_2(\mathrm{~g})+\mathrm{O}_2(\mathrm{~g}) \rightarrow 2 \mathrm{H}_2 \mathrm{O}(l)\) is \(\Delta_{\mathrm{r}} \mathrm{H}^{\ominus}=-572 \mathrm{~kJ} \mathrm{~mol}^{-1}\).
What will be the standard enthalpy of formation of \(\mathrm{H}_2 \mathrm{O}(l)?\)

Answer: According to the definition of standard enthalpy of formation, the enthalpy change for the following reaction will be standard enthalpy of formation of \(\mathrm{H}_2 \mathrm{O}({l})\)
\(
\mathrm{H}_2(\mathrm{~g})+\frac{1}{2} \mathrm{O}_2(\mathrm{~g}) \rightarrow \mathrm{H}_2 \mathrm{O}(l)
\)
or the standard enthalpy of formation of \(\mathrm{H}_2 \mathrm{O}(l)\) will be half of the enthalpy of the given equation i.e., \(\Delta_r H^{\ominus}\) is also halved.
\(
\Delta_f H_{\mathrm{H}_2 \mathrm{O}}^{\ominus}(l)=\frac{1}{2} \times_{\mathrm{r}} H^{\ominus}=\frac{-572 \mathrm{~kJ} \mathrm{~mol}^{-1}}{2}=-286 \mathrm{~kJ} / \mathrm{mol}
\)

Q48. What will be the work done on an ideal gas enclosed in a cylinder, when it is compressed by a constant external pressure, \(p_{\text {ext }}\) in a single step as shown in Figure below. Explain graphically.

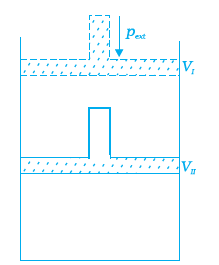
Answer:

Suppose the total volume of the gases is \(V_i\) and the pressure of the gas inside the cylinder is \(p\). After compression by constant external pressure, ( \(p_{\text {ext }}\) ) in a single tap, the final volume of the gas becomes \(\mathrm{V}_{\mathrm{f}}\).
Then volume change, \(\Delta \mathrm{V}=\left(\mathrm{V}_{\mathrm{f}}-\mathrm{V}_{\mathrm{i}}\right)\)
If \(\mathrm{W}\) is the work done on the system by the movement of the piston, then
\(
\begin{aligned}
& \mathrm{W}=\mathrm{p}_{\text {ext }}(-\Delta \mathrm{V}) \\
& \mathrm{W}=-\mathrm{p}_{\text {ext }}\left(\mathrm{V}_{\mathrm{f}}-\mathrm{V}_{\mathrm{i}}\right)
\end{aligned}
\)
This can be calculated from \(\mathrm{p}-\mathrm{V}\) graph as shown in the figure. Work done is equal to the shaded area \(\mathrm{ABV}_{\mathrm{f}} \mathrm{V}_{\mathrm{i}}\)

The negative sign in this expression is required to obtain a conventional sign for \(\mathrm{W}\) which will be positive. Because in case of compression, work is done on the system, so \(\Delta V\) will be negative.

Q49. How will you calculate work done on an ideal gas in a compression, when change in pressure is carried out in infinite steps?

Answer: The work done can be calculated with the help of \(p-V\) plot. A \(p-V\) plot of the work of compression which is carried out by change in pressure in infinite steps, is given in Figure below. The shaded area represents the work done on the gas.

Q50. Represent the potential energy/enthalpy change in the following processes graphically.
(a) Throwing a stone from the ground to the roof.
(b)
\(
\frac{1}{2} \mathrm{H}_2(\mathrm{~g})+\frac{1}{2} \mathrm{Cl}_2(\mathrm{~g}) \rightleftharpoons \mathrm{HCl}(\mathrm{g}) \quad \Delta_r H^{\ominus}=-92.32 \mathrm{~kJ} \mathrm{~mol}^{-1}
\)
In which of the processes potential energy/enthalpy change is contributing factor to the spontaneity?

Answer: Representation of potential energy/enthalpy change in the following processes
(a) Throwing a stone from the ground to the roof.

\(
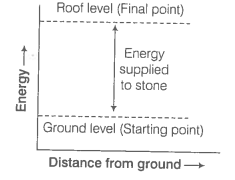
\text { (b) } \frac{1}{2} \mathrm{H}_2(\mathrm{~g})+\frac{1}{2} \mathrm{Cl}_2(\mathrm{~g}) \rightleftharpoons \mathrm{HCl}(\mathrm{g}) ; \Delta_{\mathrm{r}} \mathrm{H}^{\ominus}=-93.32 \mathrm{~kJ} \mathrm{~mol}^{-1}
\)
Energy increases in (a) and it decreases in (b) process. Hence, in the process (b), enthalpy change is the contributing factor to the spontaneity.

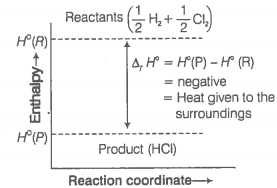
Q51. The enthalpy diagram for a particular reaction is given in Figure below. Is it possible to decide the spontaneity of a reaction from given diagram? Explain.

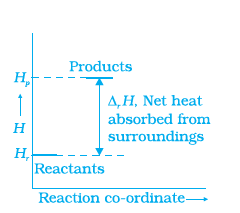
Answer: No, Enthalpy is one of the contributory factors in deciding spontaneity but it is not the only factor. One must look for the contribution of another factor i.e., entropy also, for getting the correct result.

Q52. \(1.0 \mathrm{~mol}\) of a monoatomic ideal gas is expanded from state (1) to state (2) as shown in Figure below. Calculate the work done for the expansion of gas from state (1) to state (2) at \(298 \mathrm{~K}\).

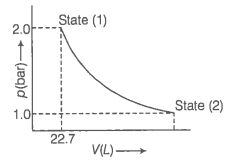
Answer: It is clear from the figure that the process has been carried out in infinite steps, hence it is isothermal reversible expansion.
\(
\mathrm{w}=-2.303 n R T \log \frac{V_2}{V_1}
\)
\(
\text { But, } \mathrm{p}_1 V_1=\mathrm{p}_2 V_2 \Rightarrow \frac{V_2}{V_1}=\frac{p_1}{p_2}=\frac{2}{1}=2
\)
\(
\begin{aligned}
\therefore \quad \mathrm{w} & =-2.303 \mathrm{nRT} \log \frac{p_1}{p_2} \\
& =-2.303 \times 1 \mathrm{~mol} \times 8.314 \mathrm{~J} \mathrm{~mol}^{-1} \mathrm{~K}^{-1} \times 298 \mathrm{~K}^{-1} \times \log 2 \\
& =-2.303 \times 8.314 \times 298 \times 0.3010 \mathrm{~J}=-1717.46 \mathrm{~J}
\end{aligned}
\)

Q53. An ideal gas is allowed to expand against a constant pressure of 2 bar from \(10 \mathrm{~L}\) to \(50 \mathrm{~L}\) in one step. Calculate the amount of work done by the gas. If the same expansion were carried out reversibly, will the work done be higher or lower than the earlier case?
\((\) Given that \(1 \mathrm{~L}\) bar \(=100 \mathrm{~J}\) )

Answer: In the first case, as the expression is against constant external pressure
\(
\mathrm{w}=-\mathrm{p}_{\mathrm{ex}}\left(V_f-V_t\right)=-2 \times 40=-80 \mathrm{Lbar}=-8 \mathrm{~kJ}
\)
The negative sign shows that work is done by the system on the surroundings. Work done will be more in the reversible expansion because internal pressure and external pressure are almost the same at every step.

Q54. Derive the relationship between \(\Delta H\) and \(\Delta U\) for an ideal gas. Explain each term involved in the equation.

Answer: From the first law of thermodynamics, \(q=\Delta \mathrm{U}+\mathrm{p} \Delta \mathrm{V}\) If the process carried out at constant volume, \(\Delta \mathrm{V}=0\)
Hence, \(\mathrm{q}_{\mathrm{v}}=\Delta \mathrm{U}\)
[Here, \(\mathrm{q}_{\mathrm{v}}=\) Heat absorbed at constant volume, \(\Delta \mathrm{U}=\) change in internal energy]
Similarly, \(\mathrm{q}_{\mathrm{p}}=\Delta \mathrm{H}\)
Here, \(\mathrm{q}_{\mathrm{p}}=\) heat absorbed at constant pressure
\(\Delta \mathrm{H}=\) enthalpy change of the system.
Enthalpy change of a system is equal to the heat absorbed or evolved by the system at constant pressure. As we know that at constant pressure, \(\Delta \mathrm{H}=\Delta \mathrm{U}+\mathrm{p} \Delta \mathrm{V}\) where \(\Delta \mathrm{V}\) is the change in volume.
\(
\text { This equation can be rewritten as } \Delta \mathrm{H}=\Delta \mathrm{U}+\mathrm{p}\left(\mathrm{V}_{\mathrm{f}}-\mathrm{V}_{\mathrm{i}}\right)=\Delta \mathrm{U}+\left(\mathrm{pV}_{\mathrm{f}}-\mathrm{pV}_{\mathrm{i}}\right) \ldots \ldots \text { (i) }
\)
where, \(V_i=\) initial volume of the system, \(V_f=\) final volume of the system But for the ideal gases, \(\mathrm{pV}=\mathrm{nRT}\)
so that \(\mathrm{pV}_1=\mathrm{n}_1 \mathrm{RT}\)
and \(\mathrm{pV}_2=\mathrm{n}_2 \mathrm{RT}\)
where \(n=\) number of moles of the gaseous reactants Substituting these values in Eq. (i), we get
\(
\begin{aligned}
& \Delta \mathrm{H}=\Delta \mathrm{U}+\left(\mathrm{n}_2 \mathrm{RT}-\mathrm{n}_1 \mathrm{RT}\right) \\
& \Delta \mathrm{H}=\Delta \mathrm{U}+\left(\mathrm{n}_2-\mathrm{n}_1\right) \mathrm{RT} \\
& \text { or } \Delta \mathrm{H}=\Delta \mathrm{U}+\Delta \mathrm{n}_{\mathrm{g}} \mathrm{RT}
\end{aligned}
\)
where \(\Delta \mathrm{n}_{\mathrm{g}}=\mathrm{n}_2-\mathrm{n}_1\) is the difference between the number of moles of the gaseous products and gaseous reactants. Putting the values of \(\Delta H\) and \(\Delta \mathrm{U}\) we get
\(
\mathrm{q}_{\mathrm{p}}=\mathrm{q}_{\mathrm{v}}+\Delta \mathrm{n}_{\mathrm{g}} \mathrm{RT}
\)

Q55. Extensive properties depend on the quantity of matter but intensive properties do not. Explain whether the following properties are extensive or intensive. Mass, internal energy, pressure, heat capacity, molar heat capacity, density, mole fraction, specific heat, temperature and molarity.

Answer: Extensive properties Those properties whose value depends on the quantity or size of matter present in the system is known as extensive properties. e.g., mass, internal energy, heat capacity.
Intensive properties Those properties which do not depend on the quantity or size of matter present are known as intensive properties. e.g., pressure, molar heat capacity, density, mole fraction, specific heat, temperature and molarity.
The mole fraction or molarity of a solution is the same whether we take a small amount of solution or large amount of the solution.
The ratio of two extensive properties is always intensive.
\(\frac{\text { Extensive }}{\text { Extensive }}=\) Intensive
So, mole fraction and molarity are intensive properties.
e.g., mole fraction \(=\frac{\text { Moles of the component }}{\text { Total no. of mole }}=\frac{\text { Extensive }}{\text { Extensive }}\)
and molarity \(=\frac{\text { Mole }}{\text { Volume }}=\frac{\text { Extensive }}{\text { Extensive }}\)

Q56. The lattice enthalpy of an ionic compound is the enthalpy when one mole of an ionic compound present in its gaseous state, dissociates into its ions. It is impossible to determine it directly by experiment. Suggest and explain an indirect method to measure lattice enthalpy of \(\mathrm{NaCl}(\mathrm{s})\).

Answer: 

• \(
  \mathrm{Na}(\mathrm{s})+\frac{1}{2} \mathrm{Cl}_2(\mathrm{~g}) \rightarrow \mathrm{Na}^{+}(\mathrm{g})+\mathrm{Cl}^{-}(\mathrm{g}) ; \quad \Delta_{\text {lattlce }} H^{\ominus}
  \)
• Born – Haber Cycle
• Steps to measure lattice enthalpy from the Born – Haber cycle
• Sublimation of sodium metal
  (1)
  \(
  \mathrm{Na}(\mathrm{s}) \rightarrow \mathrm{Na}(\mathrm{g}) ; \quad \Delta_{\text {sub }} H^{\ominus}
  \)
  (2) Ionisation of sodium atoms
  \(\mathrm{Na}(\mathrm{g}) \rightarrow \mathrm{Na}^{+}(\mathrm{g})+\mathrm{e}^{-}(\mathrm{g}) ; \quad \Delta_t H^{\ominus}\) i.e., ionisation enthalpy
  (3) Dissociation of chlorine molecule
  \(\frac{1}{2} \mathrm{Cl}_2(\mathrm{~g}) \rightarrow \mathrm{Cl}(\mathrm{g}) ; \quad \frac{1}{2} \Delta_{\text {bond }} H^{\ominus} \quad\) i.e., One-half of bond dissociation enthalpy.
  \(
  \text { (4) } \mathrm{Cl}(\mathrm{g})+\mathrm{e}^{-}(\mathrm{g}) \rightarrow \mathrm{Cl}^{-}(\mathrm{g}) ; \quad \Delta_{\mathrm{eg}} H^{\ominus} \quad \text { i.e., electron gain enthalpy. }
  \)

Q57. \(\Delta G\) is net energy available to do useful work and is thus a measure of “free energy”. Show mathematically that \(\Delta G\) is a measure of free energy. Find the unit of \(\Delta G\). If a reaction has positive enthalpy change and positive entropy change, under what condition will the reaction be spontaneous?

Answer:

\(
\begin{aligned}
& \Delta S_{\text {Total }}=\Delta S_{\text {sys }}+\Delta S_{\text {surr }} \\
& \Delta S_{\text {Total }}=\Delta S_{\text {sys }}+\frac{-\Delta H_{\text {sys }}}{T} \\
& T \Delta S_{\text {Total }}=T \Delta S_{\text {sys }}-\Delta H_{\text {sys }}
\end{aligned}
\)
For spontaneous change, \(\Delta S_{\text {total }}>0\)
\(
\begin{aligned}
& \therefore \quad T \Delta S_{\text {sys }}-\Delta H_{s y s}>0 \\
& \Rightarrow \quad-\left(\Delta H_{s y s}-T \Delta S_{s y s}\right)>0
\end{aligned}
\)
\(
\text { But, } \quad \Delta H_{\text {sys }}-T \Delta S_{\text {sys }}=\Delta G_{\text {sys }}
\)
\(
\therefore \quad-\Delta G_{s y s}>0
\)
\(
\Rightarrow \Delta G_{s y s}=\Delta H_{s y s}-T \Delta S_{s y s}<0
\)
\(\Delta H_{\text {sys }}=\) Enthalpy change of a reaction.
\(T \Delta S_{\text {sys }}=\) Energy which is not available to do useful work.
\(\Delta G_{\text {sys }}=\) Energy available for doing useful work.
Unit of \(\Delta G\) is Joule
The reaction will be spontaneous at high temperatures.

Q58. Graphically show the total work done in an expansion when the state of an ideal gas is changed reversibly and isothermally from \(\left(\mathrm{p}_{\mathrm{i}}, V_i\right)\) to \(\left(\mathrm{p}_f, V_f\right)\). With the help of a \(p V\) plot compare the work done in the above case with that carried out against a constant external pressure \(\mathrm{p}_f\).

Answer: A process or change is said to be reversible, if a change is brought out in such a way that the process could, at any moment, be reversed by an infinitesimal change. A reversible process proceeds infinitely slowly by a series of equilibrium states such that the system and the surroundings are always in near equilibrium with each other.

(i) Reversible Work is represented by the combined areas (horizontal line area + Yellow color area)
(ii) Work against constant pressure, \(p_f\) is represented by the area highlighted in yellow.
Work (i) \(>\) Work (ii)