5.6 Spontaneity

According to the First Law of Thermodynamics, the total energy of an isolated system always remains constant. The first law explains the relationship between the work done by the system or by the system and the heat absorbed without putting any limitation on the direction of heat flow.

The Second Law of Thermodynamics states that no matter what process takes place inside the container, its entropy must increase or remain the same within the limit of a reversible process. This article will help you to know more about entropy and spontaneity.

A spontaneous process is an irreversible process and may only be reversed by some external agency.

The tendency for a process to occur depends upon two factors:

• the tendency for minimum energy
• the tendency for maximum randomness

The resultant of the above two tendencies which gives the overall tendency for a process to occur is called the driving force of the process.

(a) Is Decrease in Enthalpy a Criterion for spontaneity?

If we examine the phenomenon like the flow of water downhill or the fall of a stone onto the ground, we find that there is a net decrease in potential energy in the direction of change. By analogy, we may be tempted to state that a chemical reaction is spontaneous in a given direction because the decrease in energy has taken place, as in the case of exothermic reactions. For example:

\(
\frac{1}{2} \mathrm{~N}_2(\mathrm{~g})+\frac{3}{2} \mathrm{H}_2(\mathrm{~g})=\mathrm{NH}_3(\mathrm{~g}) \text {; }\Delta_r H^{\ominus}=-46.1 \mathrm{~kJ} \mathrm{~mol}^{-1}
\)
\(
\frac{1}{2} \mathrm{H}_2(\mathrm{~g})+\frac{1}{2} \mathrm{Cl}_2(\mathrm{~g})=\mathrm{HCl}(\mathrm{g}) \text {; }\Delta_r H^{\ominus}=-92.32 \mathrm{~kJ} \mathrm{~mol}^{-1}
\)
\(
\mathrm{H}_2(\mathrm{~g})+\frac{1}{2} \mathrm{O}_2(\mathrm{~g}) \rightarrow \mathrm{H}_2 \mathrm{O}(\mathrm{l}) ;\Delta_r H^{\ominus}=-285.8 \mathrm{~kJ} \mathrm{~mol}^{-1}
\)
The decrease in enthalpy in passing from reactants to products may be shown for any exothermic reaction on an enthalpy diagram as shown in Fig. 6.10(a).
Thus, the postulate that the driving force for a chemical reaction may be due to decrease in energy sounds ‘reasonable’ as the basis of evidence so far!
Now let us examine the following reactions:
\(
\frac{1}{2} \mathrm{~N}_2(\mathrm{~g})+\mathrm{O}_2(\mathrm{~g}) \rightarrow \mathrm{NO}_2(\mathrm{~g}); \Delta_r H^{\ominus}=+33.2 \mathrm{~kJ} \mathrm{~mol}^{-1}
\)
\(
\mathrm{C} \text { (graphite, } \mathrm{s})+2 \mathrm{~S}(\mathrm{l}) \rightarrow \mathrm{CS}_2(\mathrm{l}); \Delta_r H^{\ominus}=+128.5 \mathrm{~kJ} \mathrm{~mol}^{-1}
\)

These reactions though endothermic, are spontaneous. The increase in enthalpy may be represented on an enthalpy diagram as shown in Fig. 6.10(b).

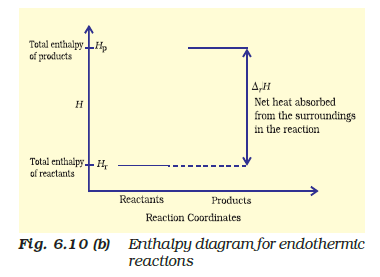
Therefore, it becomes obvious that while decrease in enthalpy may be a contributory factor for spontaneity, but it is not true for all cases.

(b) Entropy and Spontaneity

Spontaneity:

Let us examine such a case in which \(\Delta H=0\) i.e., there is no change in enthalpy, but still, the process is spontaneous. Let us consider diffusion of two gases into each other in a closed container which is isolated from the surroundings as shown in Fig. 6.11. The two gases, say, gas A and gas B are represented by black dots and white dots respectively and separated by a movable partition [Fig. 6.11 (a)]. When the partition is withdrawn [Fig.6.11(b)], the gases begin to diffuse into each other and after a period of time, diffusion will be complete.

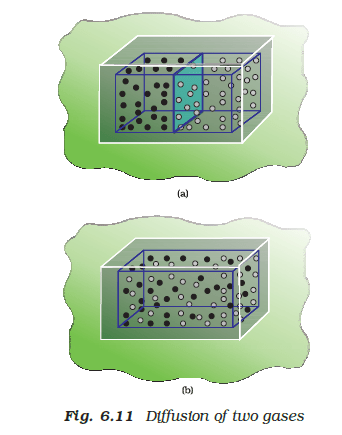
Let us examine the process. Before partition, if we were to pick up the gas molecules from the left container, we would be sure that these will be molecules of gas A and similarly, if we were to pick up the gas molecules from the right container, we would be sure that these will be molecules of gas \(\mathrm{B}\). But, if we were to pick up molecules from the container when the partition is removed, we are not sure whether the molecules picked are of gas \(\mathrm{A}\) or gas B. We say that the system has become less predictable or more chaotic.

We may now formulate another postulate: in an isolated system, there is always a tendency for the systems’ energy to become more disordered or chaotic and this could be a criterion for spontaneous change !

Entropy: It is a measure of the degree of randomness or disorder in the system. The greater the disorder in an isolated system, the higher is the entropy. Unit of entropy is \(\mathrm{JK}^{-1} \mathrm{~mol}^{-1}\). Entropy, like any other thermodynamic property such as internal energy \(U\) and enthalpy \(H\) is a state function and \(\Delta S\) is independent of path.

Whenever heat is added to the system, it increases molecular motions causing increased randomness in the system. Thus heat \((q)\) has randomising influence on the system. We know that the distribution of heat also depends on the temperature at which heat is added to the system. A system at higher temperature has greater randomness in it than one at lower temperature. Thus, temperature is the measure of average chaotic motion of particles in the system. Heat added to a system at lower temperature causes greater randomness than when the same quantity of heat is added to it at higher temperature. This suggests that the entropy change is inversely proportional to the temperature.
Entropy change during a process is given by:
\(
\Delta \mathrm{S}=\mathrm{S}_2-\mathrm{S}_1=\Sigma \mathrm{S}_{\text {product }}-\Sigma \mathrm{S}_{\text {reactant }}
\)
\(\Delta \mathrm{S}\) is related to \(\mathrm{q}\) and \(\mathrm{T}\) for a reversible process as follows:
\(
\Delta \mathrm{S}=\frac{q_{\text {rev }}}{T} \dots(6.18)
\)

In any process: 

The total entropy change ( \(\Delta S_{\text {total }}\) ) for the system and surroundings of a spontaneous process is given by
\(
\Delta S_{\text {total }}=\Delta S_{\text {system }}+\Delta S_{\text {surr }}>0 \dots(6.19)
\)
When a system is in equilibrium, the entropy is maximum, and the change in entropy, \(\Delta S=0\).
We can say that entropy for a spontaneous process increases till it reaches maximum and at equilibrium the change in entropy is zero. Since entropy is a state property, we can calculate the change in entropy of a reversible process by
\(
\Delta \mathrm{S}_{\mathrm{sys}}=\frac{q_{\text {sys }, \text { rev }}}{T}
\)
We find that both for reversible and irreversible expansion for an ideal gas, under isothermal conditions, \(\Delta U=0\), but \(\Delta S_{\text {total }}\) i.e., \(\Delta S_{\text {sys }}+\Delta S_{\text {sum }}\) is not zero for irreversible process. Thus, \(\Delta U\) does not discriminate between reversible and irreversible process, whereas \(\Delta S\) does.

Example 6.10: Predict in which of the following, entropy increases/decreases:
(i) A liquid crystallizes into a solid.
(ii) The temperature of a crystalline solid is raised from \(0 \mathrm{~K}\) to \(115 \mathrm{~K}\).
(iii)
\(
\begin{array}{r}
2 \mathrm{NaHCO}_3(\mathrm{~s}) \rightarrow \mathrm{Na}_2 \mathrm{CO}_3(\mathrm{~s})+\mathrm{CO}_2(\mathrm{~g})+\mathrm{H}_2 \mathrm{O}(\mathrm{g})
\end{array}
\)
(iv) \(\mathrm{H}_2(\mathrm{~g}) \rightarrow 2 \mathrm{H}(\mathrm{g})\)

Answer:
(i) After freezing, the molecules attain an ordered state, and therefore, entropy decreases.
(ii) At \(0 \mathrm{~K}\), the constituent particles are static and entropy is minimum. If the temperature is raised to \(115 \mathrm{~K}\), these begin to move and oscillate about their equilibrium positions in the lattice and the system becomes more disordered, therefore entropy increases.
(iii) Reactant, \(\mathrm{NaHCO}_3\) is a solid and it has low entropy. Among products, there are one solid and two gases. Therefore, the products represent a condition of higher entropy.
(iv) Here one molecule gives two atoms i.e., the number of particles increases leading to more disordered state. Two moles of \(\mathrm{H}\) atoms have higher entropy than one mole of dihydrogen molecule.

Example 6.11: For oxidation of iron, 

\(
4 \mathrm{Fe}(\mathrm{s})+3 \mathrm{O}_2(\mathrm{~g}) \rightarrow 2 \mathrm{Fe}_2 \mathrm{O}_3(\mathrm{~s})
\)
entropy change is \(-549.4 \mathrm{JK}^{-1} \mathrm{~mol}^{-1}\) at \(298 \mathrm{~K}\). In spite of the negative entropy change of this reaction, why is the reaction spontaneous?
\(
\begin{aligned}
& \left(\Delta_r H^{\ominus}\right. \text { for this reaction is } \left.-1648 \times 10^3 \mathrm{~J} \mathrm{~mol}^{-1}\right)
\end{aligned}
\)

Answer:

One decides the spontaneity of a reaction by considering
\(
\Delta S_{\text {total }}\left(\Delta S_{\text {sys }}+\Delta S_{\text {surr }}\right)
\)
For calculating \(\Delta S_{\text {surr }}\), we have to consider the heat absorbed by the surroundings which is equal to \(-\Delta_{\mathrm{r}} H^{\ominus}\). At temperature \(\mathrm{T}\), entropy change of the surroundings is
\(
\left.\Delta \mathrm{S}_{\text {surr }}=-\frac{\Delta_r H^{\ominus}}{T} \text { (at constant pressure }\right)
\)
\(
=-\frac{\left(-1648 \times 10^3 \mathrm{~J} \mathrm{~mol}^{-1}\right)}{298 \mathrm{~K}}
\)
\(
=5530 \mathrm{JK}^{-1} \mathrm{~mol}^{-1}
\)

Thus, the total entropy change for this reaction
\(
\begin{aligned}
\Delta_r S_{\text {total }}= & 5530 \mathrm{JK}^{-1} \mathrm{~mol}^{-1}+ \\
& \left(-549.4 \mathrm{JK}^{-1} \mathrm{~mol}^{-1}\right)
\end{aligned}
\)
\(
=4980.6 \mathrm{JK}^{-1} \mathrm{~mol}^{-1}
\)
This shows that the above reaction is spontaneous.

(c) Gibbs Energy and Spontaneity

We have seen that for a system, it is the total entropy change, \(\Delta \boldsymbol{S}_{\text {total }}\) which decides the spontaneity of the process. But most of the chemical reactions fall into the category of either closed systems or open systems. Therefore, for most of the chemical reactions, there are changes in both enthalpy and entropy. It is clear from the discussion in previous sections that neither decrease in enthalpy nor increase in entropy alone can determine the direction of spontaneous change for these systems.
For this purpose, we define a new thermodynamic function the Gibbs energy or Gibbs function, \(G\), as
\(
G=H-T S \dots(6.20)
\)
Gibbs function, \(G\) is an extensive property and a state function.

The change in Gibbs energy for the system, \(\Delta G_{\text {sys }}\) can be written as
\(
\Delta G_{\text {sys }}=\Delta H_{\text {sys }}-T \Delta S_{\text {sys }}-S_{\text {sys }} \Delta T
\)

At constant temperature, \(\Delta T=0\)
\(
\therefore \Delta G_{\text {sys }}=\Delta H_{\text {sys }}-T \Delta S_{\text {sys }}
\)

Usually the subscript ‘system’ is dropped and we simply write this equation as
\(
\Delta G=\Delta H-T \Delta S \dots(6.21)
\)
Thus, Gibbs energy change \(=\) enthalpy change – temperature \(\times\) entropy change, and is referred to as the Gibbs equation, one of the most important equations in chemistry.

Here, we have considered both terms together for spontaneity: energy (in terms of \(\Delta H\) ) and entropy ( \(\Delta S\), a measure of disorder) as indicated earlier. Dimensionally if we analyse, we find that \(\Delta G\) has units of energy because, both \(\Delta H\) and the \(T \Delta S\) are energy terms, since \(T \Delta S=(\mathrm{K})(\mathrm{J} / \mathrm{K})=\mathrm{J}\).
Now let us consider how \(\Delta G\) is related to reaction spontaneity.
We know,
\(
\Delta S_{\text {total }}=\Delta S_{\text {sys }}+\Delta S_{\text {surr }}
\)
If the system is in thermal equilibrium with the surroundings, then the temperature of the surroundings is the same as that of the system. Also, an increase in the enthalpy of the surrounding is equal to a decrease in the enthalpy of the system. Therefore, entropy change of surroundings,
\(
\begin{aligned}
& \Delta S_{\text {surt }}=\frac{\Delta H_{\text {surt }}}{T}=-\frac{\Delta H_{\text {sys }}}{T} \\
& \Delta S_{\text {total }}=\Delta S_{\text {sys }}+\left(-\frac{\Delta H_{\text {sys }}}{T}\right)
\end{aligned}
\)
Rearranging the above equation:
\(
T \Delta S_{\text {total }}=T \Delta S_{\text {sys }}-\Delta H_{\text {sys }}
\)

For spontaneous process, \(\Delta S_{\text {total }}>0\), so
\(
\begin{aligned}
T \Delta S_{s y s}-\Delta H_{s y s} & >0 \\
\Rightarrow-\left(\Delta H_{s y s}-T \Delta S_{s y s}\right) & >0
\end{aligned}
\)
Using equation 6.21, the above equation can be written as
\(
\begin{aligned}
& -\Delta G>0 \\
& \Delta G=\Delta H-T \Delta S<0 \dots(6.22)
\end{aligned}
\)
\(\Delta H_{\text {sys }}\) is the enthalpy change of a reaction, \(T \Delta S_{\text {sys }}\) is the energy which is not available to do useful work. So \(\Delta G\) is the net energy available to do useful work and is thus a measure of the ‘free energy’. For this reason, it is also known as the free energy of the reaction.
\(\Delta G\) gives a criteria for spontaneity at constant pressure and temperature.

• If \(\Delta G\) is negative \((<0)\), the process is spontaneous.
• If \(\Delta G\) is positive ( \(>0\) ), the process is non spontaneous.

Note: If a reaction has a positive enthalpy change and positive entropy change, it can be spontaneous when \(T \Delta S\) is large enough to outweigh \(\Delta H\). This can happen in two ways;
(a) The positive entropy change of the system can be ‘small’ in which case \(T\) must be large.
(b) The positive entropy change of the system can be ‘large’, in which case \(T\) may be small. The former is one of the reasons why reactions are often carried out at high temperatures. Table 6.4 summarises the effect of temperature on the spontaneity of reactions.

(d) Entropy and Second Law of Thermodynamics

It states that the energy of universe is constant whereas the entropy of universe is continuously increasing and tends to a maximum.

We know that for an isolated system the change in energy remains constant. Therefore, increase in entropy in such systems is the natural direction of a spontaneous change. This, in fact, is the second law of thermodynamics. The second law of thermodynamics explains why spontaneous exothermic reactions are so common. In exothermic reactions heat released by the reaction increases the disorder of the surroundings and overall entropy change is positive which makes the reaction spontaneous.

(e) Absolute Entropy and the Third Law of Thermodynamics

Molecules of a substance may move in a straight line in any direction, they may spin like a top and the bonds in the molecules may stretch and compress. These motions of the molecule are called translational, rotational and vibrational motion respectively. When the temperature of the system rises, these motions become more vigorous and entropy increases. On the other hand, when temperature is lowered, the entropy decreases.

The entropy of any pure crystalline substance approaches zero as the temperature approaches absolute zero. This is called third law of thermodynamics.

This is so because there is perfect order in a crystal at absolute zero. The statement is confined to pure crystalline solids because theoretical arguments and practical evidences have shown that the entropy of solutions and supercooled liquids is not zero at \(0 \mathrm{~K}\). The importance of the third law lies in the fact that it permits the calculation of absolute values of entropy of pure substance from thermal data alone. For a pure substance, this can be done by summing \(\frac{q_{r e v}}{T}\) increments from \(0 \mathrm{~K}\) to 298 K. Standard entropies can be used to calculate standard entropy changes by a Hess’s law type of calculation.