5.3 Measurement of ΔU and ΔH: Calorimetry

We can measure energy changes associated with chemical or physical processes by an experimental technique called calorimetry. In calorimetry, the process is carried out in a vessel called calorimeter, which is immersed in a known volume of a liquid. Knowing the heat capacity of the liquid in which calorimeter is immersed and the heat capacity of calorimeter, it is possible to determine the heat evolved in the process by measuring temperature changes. Measurements are made under two different conditions:

• at constant volume, \(q_v\)
• at constant pressure, \(q_p\)

(a) \(\Delta U\) Measurements

For chemical reactions, heat absorbed at constant volume is measured in a bomb calorimeter (Fig. 6.7). Here, a steel vessel (the bomb) is immersed in a water bath. The whole device is called calorimeter. The steel vessel is immersed in water bath to ensure that no heat is lost to the surroundings. A combustible substance is burnt in pure dioxygen supplied in the steel bomb. The heat evolved during the reaction is transferred to the water around the bomb and its temperature is monitored. Since the bomb calorimeter is sealed, its volume does not change i.e., the energy changes associated with reactions are measured at constant volume. Under these conditions, no work is done as the reaction is carried out at constant volume in the bomb calorimeter. Even for reactions involving gases, there is no work done as \(\Delta V=0\). Temperature change of the calorimeter produced by the completed reaction is then converted to \(q_V\), by using the known heat capacity of the calorimeter with the help of equation 6.11.

(b) \(\Delta H\) Measurements

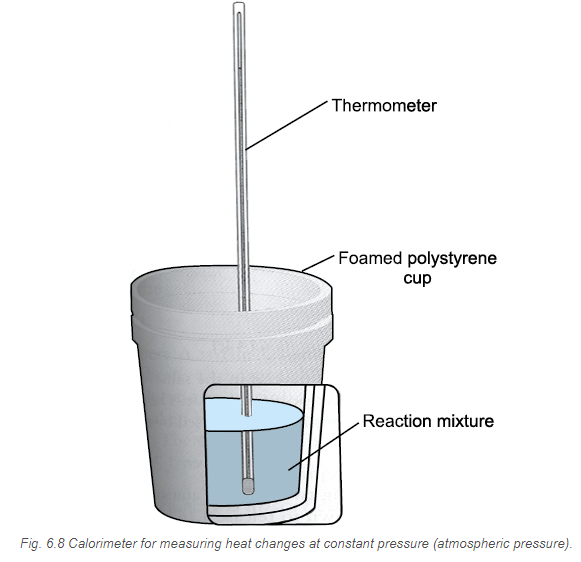
Measurement of heat change at constant pressure (generally under atmospheric pressure) can be done in a calorimeter shown in Fig. 6.8. We know that \(\Delta H=q_p\) (at constant \(p\) ) and, therefore, heat absorbed or evolved, \(q_p\) at constant pressure is also called the heat of reaction or enthalpy of reaction, \(\Delta_r H\).

In an exothermic reaction, heat is evolved, and the system loses heat to the surroundings. Therefore, \(q_p\) will be negative and \(\Delta_r H\) will also be negative. Similarly in an endothermic reaction, heat is absorbed, \(q_p\) is positive and \(\Delta_r H\) will be positive.

Example 6.6: 1 g of graphite is burnt in a bomb calorimeter in excess of oxygen at \(298 \mathrm{~K}\) and 1 atmospheric pressure according to the equation
\(
\mathrm{C} \text { (graphite) }+\mathrm{O}_2(\mathrm{~g}) \rightarrow \mathrm{CO}_2(\mathrm{~g})
\)
During the reaction, the temperature rises from \(298 \mathrm{~K}\) to \(299 \mathrm{~K}\). If the heat capacity of the bomb calorimeter is \(20.7 \mathrm{~kJ} / \mathrm{K}\), what is the enthalpy change for the above reaction at \(298 \mathrm{~K}\) and \(1 \mathrm{~atm}\)?

Answer:Suppose \(q\) is the quantity of heat from the reaction mixture and \(C_V\) is the heat capacity of the calorimeter, then the quantity of heat absorbed by the calorimeter.
\(
q=C_V \times \Delta T
\)

The quantity of heat from the reaction will have the same magnitude but opposite sign because the heat lost by the system (reaction mixture) is equal to the heat gained by the calorimeter.
\(
\begin{aligned}
q=-C_V \times \Delta T & =-20.7 \mathrm{~kJ} / \mathrm{K} \times(299-298) \mathrm{K} \\
& =-20.7 \mathrm{~kJ}
\end{aligned}
\)
(Here, the negative sign indicates the exothermic nature of the reaction)

Thus, \(\Delta U\) for the combustion of the \(\lg\) of graphite \(=-20.7 \mathrm{kJK}^{-1}\)

For combustion of \(1 \mathrm{~mol}\) of graphite,
\(
\begin{aligned}
& =\frac{12.0 \mathrm{~g} \mathrm{~mol}^{-1} \times(-20.7 \mathrm{~kJ})}{\lg } \\
& =-2.48 \times 10^2 \mathrm{~kJ} \mathrm{~mol}^{-1}, \quad \text { Since } \Delta n_g=0, \\
& \Delta H=\Delta U=-2.48 \times 10^2 \mathrm{~kJ} \mathrm{~mol}^{-1}
\end{aligned}
\)