4.6 Hybridisation
In order to explain the characteristic geometrical shapes of polyatomic molecules like \(\mathrm{CH}_4, \mathrm{NH}_3\) and \(\mathrm{H}_2 \mathrm{O}\), etc., Pauling introduced the concept of hybridisation.
- According to him, the atomic orbitals combine to form new set of equivalent orbitals known as hybrid orbitals. Unlike pure orbitals, the hybrid orbitals are used in bond formation. The phenomenon is known as hybridisation.
- Hybridisation can be defined as the process of intermixing of the orbitals of slightly different energies so as to redistribute their energies, resulting in the formation of new set of orbitals of equivalent energies and shape. For example, when one \(2s\) and three \(2 p\)-orbitals of carbon hybridise, there is the formation of four new \(s p^3\) hybrid orbitals.
Salient features of hybridisation: The main features of hybridisation are as under:
- The number of hybrid orbitals is equal to the number of the atomic orbitals that get hybridised.
- The hybridised orbitals are always equivalent in energy and shape.
- The hybrid orbitals are more effective in forming stable bonds than the pure atomic orbitals.
- These hybrid orbitals are directed in space in some preferred direction to have minimum repulsion between electron pairs and thus a stable arrangement. Therefore, the type of hybridisation indicates the geometry of the molecules.
Important conditions for hybridisation
- The orbitals present in the valence shell of the atom are hybridised.
- The orbitals undergoing hybridisation should have almost equal energy.
- Promotion of electron is not essential condition prior to hybridisation.
- It is not necessary that only half-filled orbitals participate in hybridisation. In some cases, even filled orbitals of valence shell take part in hybridisation.
Types of Hybridisation
There are various types of hybridisation involving \(s, p\) and \(d\) orbitals. The different types of hybridisation are as under:
(I) sp hybridisation
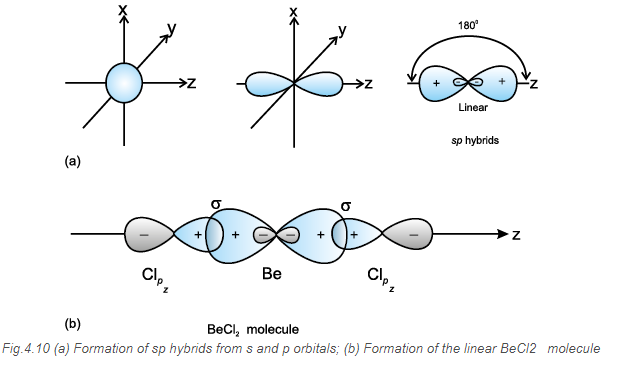
This type of hybridisation involves the mixing of one \(s\) and one \(p\) orbital resulting in the formation of two equivalent \(s p\) hybrid orbitals. The suitable orbitals for \(s p\) hybridisation are \(s\) and \(p_z\), if the hybrid orbitals are to lie along the \(z\)-axis. Each \(s p\) hybrid orbitals has \(50 \% s\)-character and \(50 \% p\)-character. Such a molecule in which the central atom is \(s p\)-hybridised and linked directly to two other central atoms possesses linear geometry. This type of hybridisation is also known as diagonal hybridisation.
The two \(s p\) hybrids point in the opposite direction along the \(\mathrm{z}\)-axis with projecting positive lobes and very small negative lobes, which provides more effective overlapping resulting in the formation of stronger bonds.
Example of molecule having \(s p\) hybridisation
\(\mathbf{B e C l}_2\) : The ground state electronic configuration of \(\mathrm{Be}\) is \(1 s^2 2 s^2\). In the exited state one of the \(2 s\)-electrons is promoted to vacant \(2 p\) orbital to account for its bivalency. One \(2 s\) and one \(2 p\)-orbital gets hybridised to form two \(s p\) hybridised orbitals. These two \(s p\) hybrid orbitals are oriented in opposite direction forming an angle of \(180^{\circ}\). Each of the \(s p\) hybridised orbital overlaps with the \(2 p\)-orbital of chlorine axially and form two Be\(\mathrm{Cl}\) sigma bonds. This is shown in Fig. 4.10.
(II) \(s p^2\) hybridisation
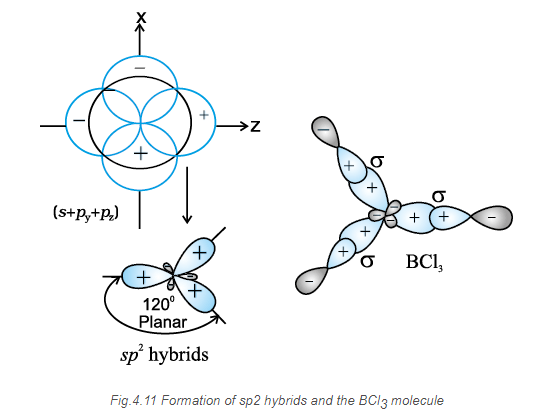
In this hybridisation there is involvement of one \(s\) and two \(p\)-orbitals in order to form three equivalent \(s p^2\) hybridised orbitals. For example, in \(\mathrm{BCl}_3\) molecule, the ground state electronic configuration of central boron atom is \(1 s^2 2 s^2 2 p^1\). In the excited state, one of the \(2 s\) electrons is promoted to vacant \(2 p\) orbital as a result boron has three unpaired electrons. These three orbitals (one \(2 s\) and two \(2 p\) ) hybridise to form three \(s p^2\) hybrid orbitals. The three hybrid orbitals so formed are oriented in a trigonal planar arrangement and overlap with \(2 p\) orbitals of chlorine to form three \(\mathrm{B}-\mathrm{Cl}\) bonds. Therefore, in \(\mathrm{BCl}_3\) (Fig. 4.11), the geometry is trigonal planar with \(\mathrm{ClBCl}\) bond angle of \(120^{\circ}\).
(III) \(s p^3\) hybridisation
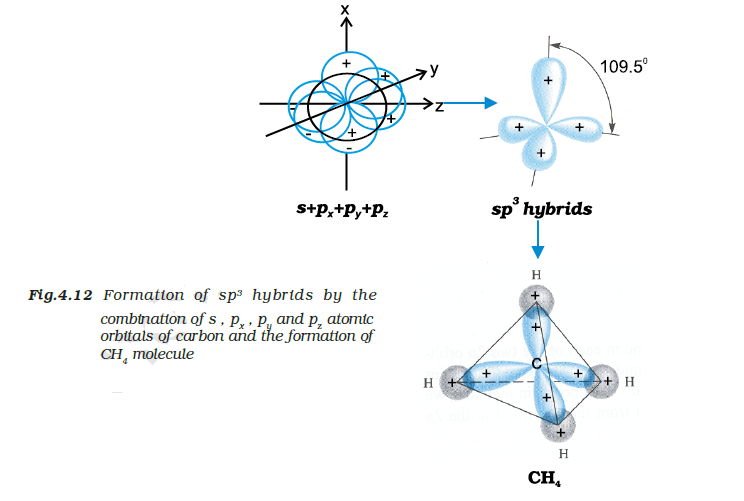
This type of hybridisation can be explained by taking the example of \(\mathrm{CH}_4\) molecule in which there is mixing of one s-orbital and three \(p\)-orbitals of the valence shell to form four \(s p^3\) hybrid orbital of equivalent energies and shape. There is \(25 \%\) \(s\)-character and \(75 \% p\)-character in each \(s p^3\) hybrid orbital. The four \(s p^3\) hybrid orbitals so formed are directed towards the four corners of the tetrahedron. The angle between \(s p^3\) hybrid orbital is \(109.5^{\circ}\) as shown in Fig. 4.12.
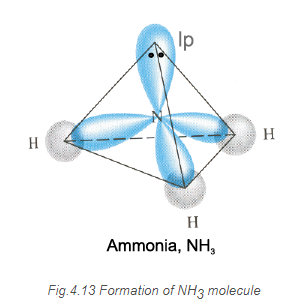
The structure of \(\mathrm{NH}_3\) and \(\mathrm{H}_2 \mathrm{O}\) molecules can also be explained with the help of \(s p^3\) hybridisation. In \(\mathrm{NH}_3\), the valence shell (outer) electronic configuration of nitrogen in the ground state is \(2 s^2 2 p_x^1 2 p_y^1 2 p_z^1\) having three unpaired electrons in the \(s p^3\) hybrid orbitals and a lone pair of electrons is present in the fourth one. These three hybrid orbitals overlap with ls orbitals of hydrogen atoms to form three N-H sigma bonds. We know that the force of repulsion between a lone pair and a bond pair is more than the force of repulsion between two bond pairs of electrons. The molecule thus gets distorted and the bond angle is reduced to \(107^{\circ}\) from \(109.5^{\circ}\). The geometry of such a molecule will be pyramidal as shown in Fig. 4.13.
In case of \(\mathrm{H}_2 \mathrm{O}\) molecule, the four oxygen orbitals (one \(2 s\) and three \(2 p\) ) undergo \(s p^3\) hybridisation forming four \(s p^3\) hybrid orbitals out of which two contain one electron each and the other two contain a pair of electrons. These four \(s p^3\) hybrid orbitals acquire a tetrahedral geometry| with two corners occupied by hydrogen atoms while the other two by the lone pairs. The bond angle in this case is reduced to \(104.5^{\circ}\) from \(109.5^{\circ}\) (Fig. 4.14) and the molecule thus acquires a V-shape or angular geometry.

Other Examples of \(s p^3, s p^2\) and \(s p\) Hybridisation
\({sp}^3\) Hybridisation in \({C}_2 {H}_6\) molecule:
In ethane molecule both the carbon atoms assume \(s p^3\) hybrid state. One of the four \(s p^3\) hybrid orbitals of carbon atom overlaps axially with similar orbitals of other atom to form \(s p^3-s p^3\) sigma bond while the other three hybrid orbitals of each carbon atom are used in forming \(s p^3-s\) sigma bonds with hydrogen atoms as discussed earlier. Therefore in ethane \(\mathrm{C}-\mathrm{C}\) bond length is 154 \(\mathrm{pm}\) and each \(\mathrm{C}-\mathrm{H}\) bond length is \(109 \mathrm{pm}\).
\(s p^2\) Hybridisation in \({C}_2 {H}_4\) :
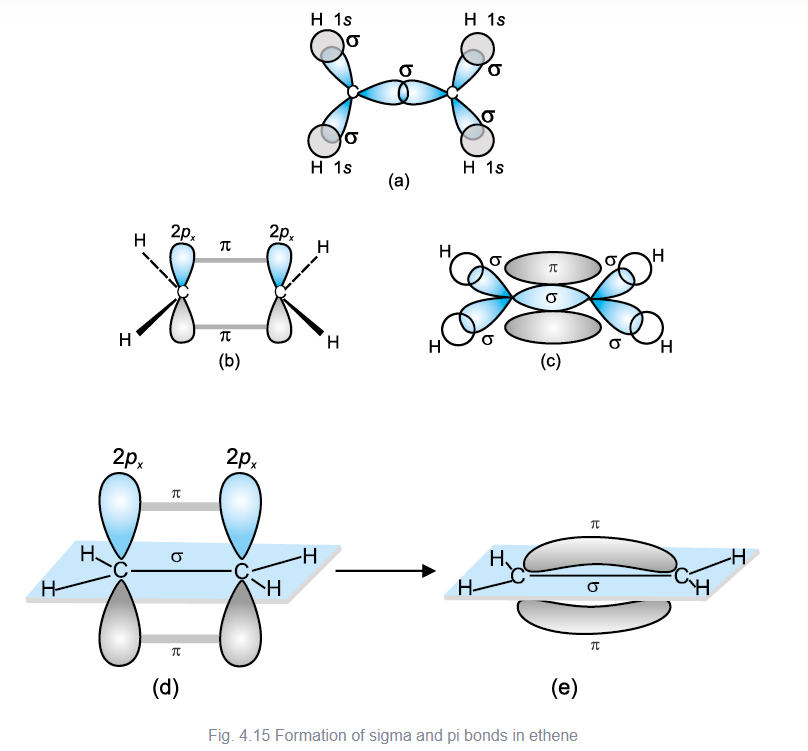
In the formation of the ethene molecule, one of the \(s p^2\) hybrid orbitals of carbon atom overlaps axially with \(s p^2\) hybridised orbital of another carbon atom to form C-C sigma bond. While the other two \(s p^2\) hybrid orbitals of each carbon atom are used for making \(s p^2-s\) sigma bond with two hydrogen atoms. The unhybridised orbital \(\left(2 p_{x}\right.\) or \(2p_{y}\) ) of one carbon atom overlaps sidewise with the similar orbital of the other carbon atom to form weak \(\pi\) bond, which consists of two equal electron clouds distributed above and below the plane of carbon and hydrogen atoms.
Thus, in ethene molecule, the carboncarbon bond consists of one \(s p^2-s p^2\) sigma bond and one pi \((\pi)\) bond between \(p\) orbitals which are not used in the hybridisation and are perpendicular to the plane of molecule; the bond length \(134 \mathrm{pm}\). The \(\mathrm{C}-\mathrm{H}\) bond is \(s p^2-s\) sigma with bond length \(108 \mathrm{pm}\). The \(\mathrm{H}-\mathrm{C}-\mathrm{H}\) bond angle is \(117.6^{\circ}\) whlile the \(\mathrm{H}-\mathrm{C}-\mathrm{C}\) angle is \(121^{\circ}\). The formation of sigma and pi bonds in ethene is shown in Fig. 4.15.
sp Hybridisation in \({C}_2 {H}_2\):
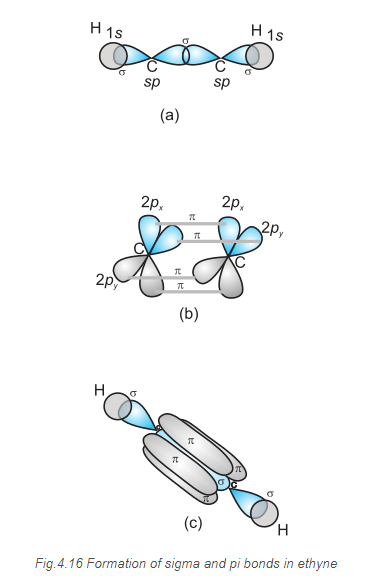
In the formation of ethyne molecule, both the carbon atoms undergo sp-hybridisation having two unhybridised orbital i.e., \(2 p_{\mathrm{y}}\) and \(2 p_{\mathrm{x}}\).
One \(s p\) hybrid orbital of one carbon atom overlaps axially with \(s p\) hybrid orbital of the other carbon atom to form \(\mathrm{C}-\mathrm{C}\) sigma bond, while the other hybridised orbital of each carbon atom overlaps axially with the half filled \(s\) orbital of hydrogen atoms forming \(\sigma\) bonds. Each of the two unhybridised p orbitals of both the carbon atoms overlaps sidewise to form two \(\pi\) bonds between the carbon atoms. So the triple bond between the two carbon atoms is made up of one sigma and two pi bonds as shown in Fig. 4,16.
Hybridisation of Elements involving \(d\) Orbitals
The elements present in the third period contain \(d\) orbitals in addition to \(s\) and \(p\) orbitals. The energy of the \(3 d\) orbitals are comparable to the energy of the \(3 s\) and \(3 p\) orbitals. The energy of \(3 d\) orbitals are also comparable to those of \(4 s\) and \(4 p\) orbitals. As a consequence the hybridisation involving either \(3 s, 3 p\) and \(3 d\) or \(3 d, 4 s\) and \(4 p\) is possible. However, since the difference in energies of \(3 p\) and \(4 s\) orbitals is significant, no hybridisation involving \(3 p, 3 d\) and \(4 s\) orbitals is possible.
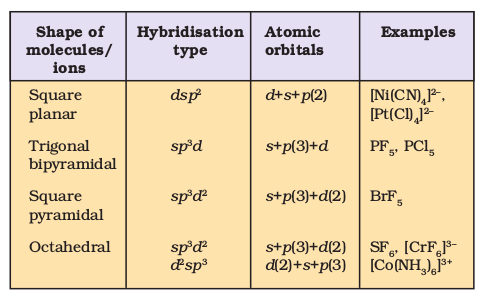
The important hybridisation schemes involving \(s, p\) and \(d\) orbitals are summarised below:
(i) Formation of \({PCl}_5\) (\({sp}^3 {d}\) hybridisation): The ground state and the excited state outer electronic configurations of phosphorus \((Z=15)\) are represented below.
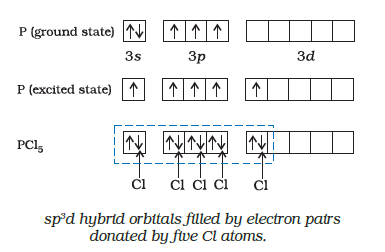
Now the five orbitals (i.e., one \(s\), three \(p\) and one \(d\) orbitals) are available for hybridisation to yield a set of five \(s p^3 d\) hybrid orbitals which are directed towards the five corners of a trigonal bipyramidal as depicted in Fig. 4. 17.

It should be noted that all the bond angles in trigonal bipyramidal geometry are not equivalent. In \(\mathrm{PCl}_5\) the five \(s p^3 d\) orbitals of phosphorus overlap with the singly occupied \(p\) orbitals of chlorine atoms to form five \(\mathrm{P}-\mathrm{Cl}\) sigma bonds. Three \(\mathrm{P}-\mathrm{Cl}\) bond lie in one plane and make an angle of \(120^{\circ}\) with each other; these bonds are termed as equatorial bonds. The remaining two \(\mathrm{P}-\mathrm{Cl}\) bonds-one lying above and the other lying below the equatorial plane, make an angle of \(90^{\circ}\) with the plane. These bonds are called axial bonds. As the axial bond pairs suffer more repulsive interaction from the equatorial bond pairs, therefore axial bonds have been found to be slightly longer and hence slightly weaker than the equatorial bonds; which makes \(\mathrm{PCl}_5\) molecule more reactive.
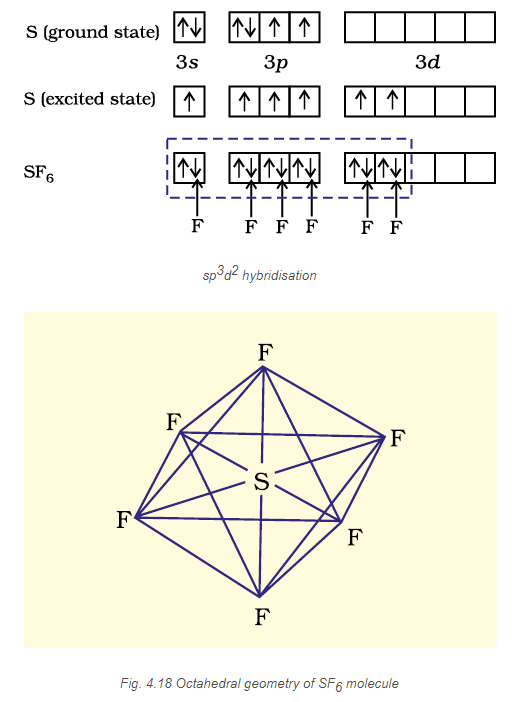
(ii) Formation of \(\mathbf{S F}_6\left(s p^3 d^2\right.\) hybridisation): In \(\mathrm{SF}_6\) the central sulphur atom has the ground state outer electronic configuration \(3 s^2 3 p^4\). In the exited state the available six orbitals i.e., one \(s\), three \(p\) and two \(d\) are singly occupied by electrons. These orbitals hybridise to form six new \(s p^3 d^2\) hybrid orbitals, which are projected towards the six corners of a regular octahedron in \(\mathrm{SF}_6\). These six \(s p^3 d^2\) hybrid orbitals overlap with singly occupied orbitals of fluorine atoms to form six S-F sigma bonds. Thus \(\mathrm{SF}_6\) molecule has a regular octahedral geometry as shown in Fig. 4.18.