4.5 Valence Bond Theory

As we know that Lewis approach helps in writing the structure of molecules but it fails to explain the formation of chemical bond. It also does not give any reason for the difference in bond dissociation enthalpies and bond lengths in molecules like \(\mathrm{H}_2\left(435.8 \mathrm{~kJ} \mathrm{~mol}^{-1}\right.\), although in both cases a single covalent bond is formed by the sharing of an electron pair between the respective atoms. It also gives no idea about the shapes of polyatomic molecules.

Similarly, the VSEPR theory gives the geometry of simple molecules but theoretically, it does not explain them and also it has limited applications. To overcome these limitations the two important theories based on quantum mechanical principles are introduced. These are valence bond (VB) theory and molecular orbital (MO) theory.

Valence bond theory was introduced by Heitler and London (1927) and developed further by Pauling and others.

• Valence bond theory is based on the knowledge of atomic orbitals, electronic configurations of elements, the overlap criteria of atomic orbitals, the hybridization of atomic orbitals and the principles of variation and superposition.

To start with, let us consider the formation of hydrogen molecule which is the simplest of all molecules.

Consider two hydrogen atoms \(\mathrm{A}\) and \(\mathrm{B}\) approaching each other having nuclei \(\mathrm{N}_{\mathrm{A}}\) and \(\mathrm{N}_B\) and electrons present in them are represented by \(e_A\) and \(e_B\). When the two atoms are at a large distance from each other, there is no interaction between them. As these two atoms approach each other, new attractive and repulsive forces begin to operate.
Attractive forces arise between:
(i) nucleus of one atom and its own electron that is \(\mathrm{N}_{\mathrm{A}}-\mathrm{e}_{\mathrm{A}}\) and \(\mathrm{N}_{\mathrm{B}}-\mathrm{e}_{\mathrm{B}}\).
(ii) nucleus of one atom and electron of other atom i.e., \(\mathrm{N}_{\mathrm{A}}-\mathrm{e}_{\mathrm{B}}, \mathrm{N}_{\mathrm{B}}-\mathrm{e}_{\mathrm{A}}\).
Similarly repulsive forces arise between
(i) electrons of two atoms like \(e_A-e_B\),
(ii) nuclei of two atoms \(\mathrm{N}_{\mathrm{A}}-\mathrm{N}_{\mathrm{B}}\).
Attractive forces tend to bring the two atoms close to each other whereas repulsive forces tend to push them apart (Fig. 4.7).

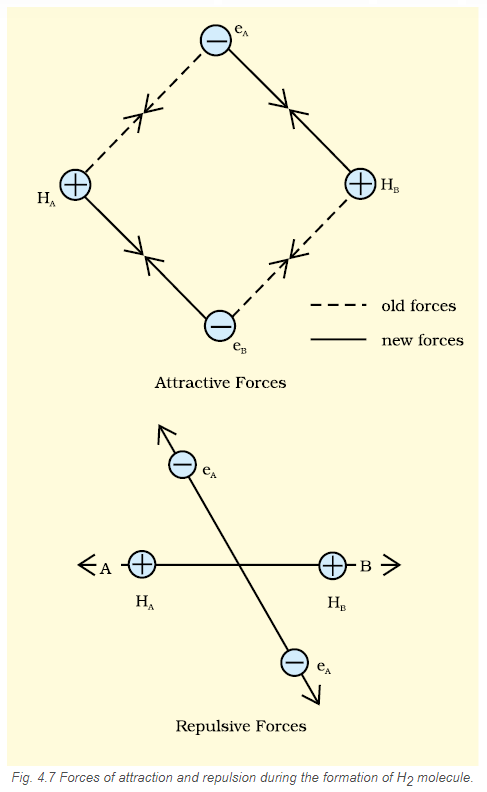
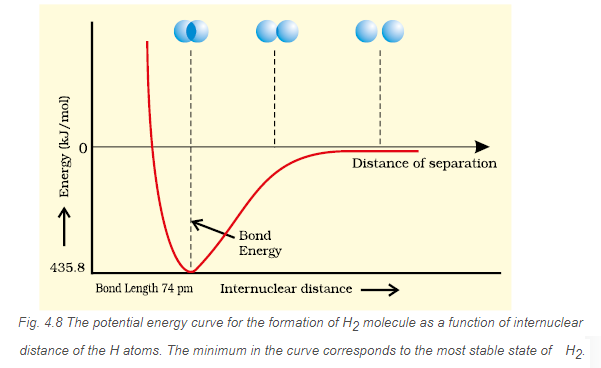
Experimentally it has been found that the magnitude of new attractive force is more than the new repulsive forces. As a result, two atoms approach each other and potential energy decreases. Ultimately a stage is reached where the net force of attraction balances the force of repulsion and the system acquires minimum energy. At this stage two hydrogen atoms are said to be bonded together to form a stable molecule having the bond length of \(74 \mathrm{pm}\).

Since the energy gets released when the bond is formed between two hydrogen atoms, the hydrogen molecule is more stable than that of isolated hydrogen atoms. The energy so released is called as bond enthalpy, which corresponds to the minimum in the curve depicted in Fig. 4.8. Conversely, \(435.8 \mathrm{~kJ}\) of energy is required to dissociate one mole of \(\mathrm{H}_2\) molecule.
\(
\mathrm{H}_2(\mathrm{~g})+435.8 \mathrm{~kJ} \mathrm{~mol}^{-1} \rightarrow \mathrm{H}(\mathrm{g})+\mathrm{H}(\mathrm{g})
\)

Orbital Overlap Concept

In the formation of hydrogen molecule, there is a minimum energy state when two hydrogen atoms are so near that their atomic orbitals undergo partial interpenetration. This partial merging of atomic orbitals is called overlapping of atomic orbitals which results in the pairing of electrons. The extent of overlap decides the strength of a covalent bond. In general, the greater the overlap the stronger is the bond formed between two atoms.

• Therefore, according to the orbital overlap concept, the formation of a covalent bond between two atoms results by pairing of electrons present in the valence shell having opposite spins.

Directional Properties of Bonds

• The covalent bond is formed by overlapping of atomic orbitals. The molecule of hydrogen is formed due to the overlap of 1 s-orbitals of two \(\mathrm{H}\) atoms.
• In the case of polyatomic molecules like \(\mathrm{CH}_4\), \(\mathrm{NH}_3\) and \(\mathrm{H}_2 \mathrm{O}\), the geometry of the molecules is also important in addition to the bond formation. For example why is it so that \(\mathrm{CH}_4\) molecule has tetrahedral shape and \(\mathrm{HCH}\) bond angles are \(109.5^{\circ}\) ? Why is the shape of \(\mathrm{NH}_3\) molecule pyramidal?
• The valence bond theory explains the shape, the formation and directional properties of bonds in polyatomic molecules like \(\mathrm{CH}_4, \mathrm{NH}_3\) and \(\mathrm{H}_2 \mathrm{O}\), etc. in terms of overlap and hybridisation of atomic orbitals.

Overlapping of Atomic Orbitals

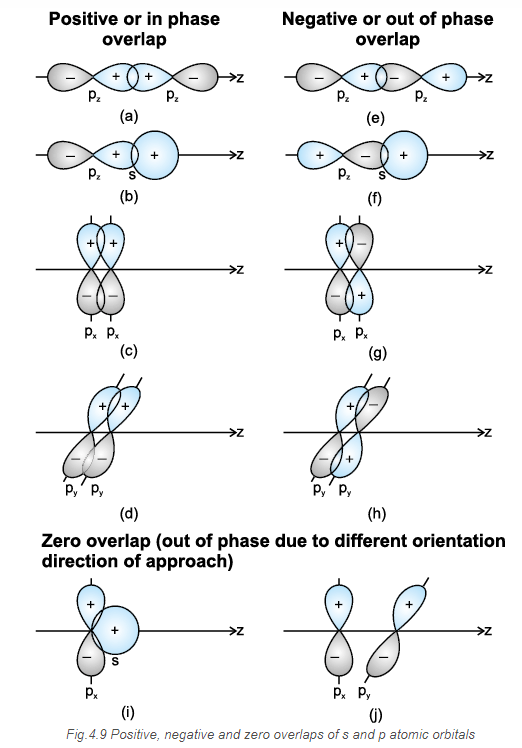
When orbitals of two atoms come close to form bond, their overlap may be positive, negative or zero depending upon the sign (phase) and direction of orientation of amplitude of orbital wave function in space (Fig. 4.9). Positive and negative sign on boundary surface diagrams in the Fig. 4.9 show the sign (phase) of the orbital wave function and are not related to charge. Orbitals forming bond should have same sign (phase) and orientation in space. This is called positive overlap. Various overlaps of \(s\) and \(p\) orbitals are depicted in Fig. 4.9.

The criterion of overlap, as the main factor for the formation of covalent bonds applies uniformly to the homonuclear/heteronuclear diatomic molecules and polyatomic molecules. We know that the shapes of \(\mathrm{CH}_4, \mathrm{NH}_3\), and \(\mathrm{H}_2 \mathrm{O}\) molecules are tetrahedral, pyramidal and bent respectively. It would be therefore interesting to use VB (Valence Band) theory to find out if these geometrical shapes can be explained in terms of the orbital overlaps.

Let us first consider the \(\mathrm{CH}_4\) (methane) molecule. The electronic configuration of carbon in its ground state is [He] \(2 s^2 2 p^2\) which in the excited state becomes [He] \(2 s^1 2 p_x{ }^1 2 p_y{ }^1\) \(2 p_z{ }^1\). The energy required for this excitation is compensated by the release of energy due to overlap between the orbitals of carbon and the hydrogen. The four atomic orbitals of carbon, each with an unpaired electron can overlap with the \(1 s\) orbitals of the four \(\mathrm{H}\) atoms which are also singly occupied. This will result in the formation of four C-H bonds. It will, however, be observed that while the three p orbitals of carbon are at \(90^{\circ}\) to one another, the \(\mathrm{HCH}\) angle for these will also be \(90^{\circ}\). That is three \(\mathrm{C}-\mathrm{H}\) bonds will be oriented at \(90^{\circ}\) to one another. The \(2 s\) orbital of carbon and the \(1 s\) orbital of \(\mathrm{H}\) are spherically symmetrical and they can overlap in any direction. Therefore the direction of the fourth \(\mathrm{C}-\mathrm{H}\) bond cannot be ascertained. This description does not fit in with the tetrahedral \(\mathrm{HCH}\) angles of \(109.5^{\circ}\). Clearly, it follows that simple atomic orbital overlap does not account for the directional characteristics of bonds in \(\mathrm{CH}_4\). Using similar procedure and arguments, it can be seen that in the case of \(\mathrm{NH}_3\) and \(\mathrm{H}_2 \mathrm{O}\) molecules, the \(\mathrm{HNH}\) and \(\mathrm{HOH}\) angles should be \(90^{\circ}\). This is in disagreement with the actual bond angles of \(107^{\circ}\) and \(104.5^{\circ}\) in the \(\mathrm{NH}_3\) and \(\mathrm{H}_2 \mathrm{O}\) molecules respectively.

Types of Overlapping and Nature of Covalent Bonds

The covalent bond may be classified into two types depending upon the types of overlapping:

• \(\operatorname{Sigma}(\sigma)\) bond, and
• \(\mathrm{pi}(\pi)\) bond

Sigma( \(\sigma)\) bond: This type of covalent bond is formed by the end-to-end (head-on) overlap of bonding orbitals along the internuclear axis. This is called as head-on overlap or axial overlap. This can be formed by any one of the following types of combinations of atomic orbitals.

• \(s-s\) overlapping: In this case, there is overlap of two half-filled s-orbitals along the internuclear axis as shown below:

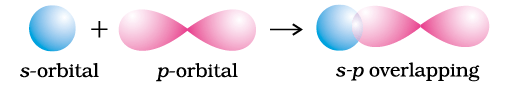
• \(\boldsymbol{s}-\boldsymbol{p}\) overlapping: This type of overlap occurs between half-filled s-orbitals of one atom and half-filled \(p\)-orbitals of another atom.

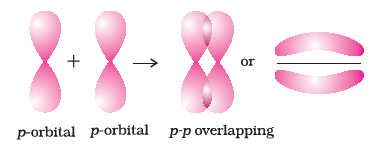
• \(p-p\) overlapping: This type of overlap takes place between half-filled \(p\)-orbitals of the two approaching atoms.

• pi \((\pi)\) bond: In the formation of \(\pi\) bond the atomic orbitals overlap in such a way that their axes remain parallel to each other and perpendicular to the internuclear axis. The orbitals formed due to sidewise overlapping consist of two saucer-type charged clouds above and below the plane of the participating atoms.

Strength of Sigma and pi Bonds

Basically, the strength of a bond depends upon the extent of overlapping. In the case of sigma bond, the overlapping of orbitals takes place to a larger extent. Hence, it is stronger as compared to the pi bond where the extent of overlapping occurs to a smaller extent. Further, it is important to note that in the formation of multiple bonds between two atoms of a molecule, pi bond(s) is formed in addition to a sigma bond.

Comparision between Sigma and pi Bond

\(
\begin{array}{|l|l|}
\hline {\text { Sigma bond }} & {\text { Pi bond }} \\
\hline \begin{array}{l}
\text { They are formed by head-on overlap } \\
\text { between orbitals. }
\end{array} & \text { Formed by lateral overlapping. } \\
\hline \text { It is more powerful. } & \begin{array}{l}
\text { It’s a weak link because there’s a lot of } \\
\text { overlapping, but there’s also a lot of minor } \\
\text { overlapping. }
\end{array} \\
\hline \text { There is greater bond energy } & \text { The bond energy is lower. } \\
\hline \begin{array}{l}
\text { On the internuclear axis, it results in a high } \\
\text { electron density between two nuclei. }
\end{array} & \begin{array}{l}
\text { It causes increased electron density above } \\
\text { and below the internuclear axis, but not } \\
\text { along the nuclear axis. }
\end{array} \\
\hline \begin{array}{l}
\text { Around the internuclear axis, the bond is } \\
\text { rotationally symmetrical. }
\end{array} & \begin{array}{l}
\text { The bond is not symmetrical in rotation } \\
\text { around the internuclear axis. }
\end{array} \\
\hline \begin{array}{l}
\text { It can only be created between any two } \\
\text { orbitals, namely the } s-s, s-p \text { or } p-p \\
\text { and so forth. }
\end{array} & \text { It involves only } p \text { orbitals. } \\
\hline \begin{array}{l}
\text { This bond results by the involvement of } \\
\text { pure and hybrid orbitals. }
\end{array} & \begin{array}{l}
\text { These are formed by the involvement of } \\
\text { only pure orbitals. }
\end{array} \\
\hline
\end{array}
\)