4.3 Bond Parameters

Bond Length

Bond length is defined as the equilibrium distance between the nuclei of two bonded atoms in a molecule. Bond lengths are measured by spectroscopic, X-ray diffraction and electron-diffraction techniques. Each atom of the bonded pair contributes to the bond length (Fig. 4.1). In the case of a covalent bond, the contribution from each atom is called the covalent radius of that atom.

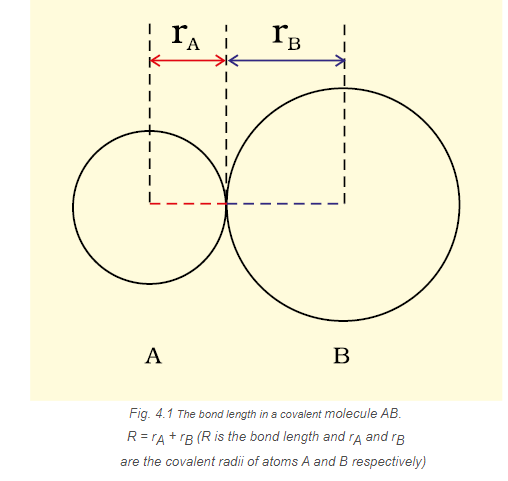
The covalent radius is half of the distance from the centre of nucleus of one atom to the centre of nucleus of the other atom provided
bonded atoms will be of the same element in a molecule. The covalent radius is half of the distance between two similar atoms joined by a covalent bond in the same molecule.

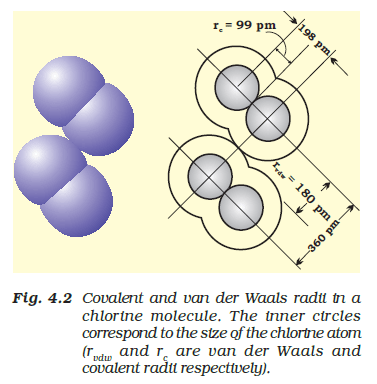
The van der Waals radius represents the overall size of the atom which includes its valence shell in a non-bonded situation. Further, the van der Waals radius is half of the distance between two similar atoms in separate molecules in a solid. Covalent and van der Waals radii of chlorine are depicted in Fig. 4.2
Van der Waal’s radius \(>\) Covalent radius

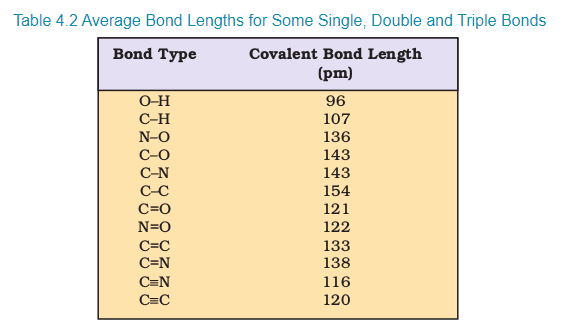
Some typical average bond lengths for single, double and triple bonds are shown in Table 4.2.

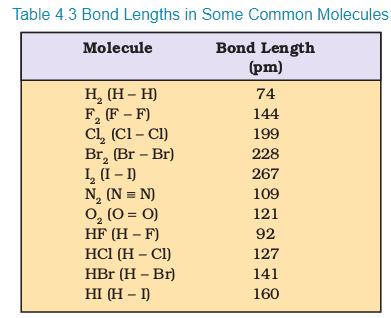
Bond lengths for some common molecules are given in Table 4.3.

The covalent radii of some common elements are listed in Table 4.4.

Bond Angle

It is defined as the angle between the orbitals containing bonding electron pairs around the central atom in a molecule/complex ion.

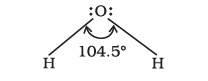
Bond angle is expressed in degree which can be experimentally determined by spectroscopic methods. It gives some idea regarding the distribution of orbitals around the central atom in a molecule/complex ion and hence it helps us in determining its shape. For example \(\mathrm{H}-\mathrm{O}-\mathrm{H}\) bond angle in water can be represented as under:

Bond Enthalpy

It is defined as the amount of energy required to break one mole of bonds of a particular type between two atoms in a gaseous state. The unit of bond enthalpy is \(\mathrm{kJ} \mathrm{mol}^{-1}\).

For example, the \(\mathrm{H}-\mathrm{H}\) bond enthalpy in hydrogen molecule is \(435.8 \mathrm{~kJ} \mathrm{~mol}^{-1}\).

\(
\mathrm{H}_2(\mathrm{~g}) \rightarrow \mathrm{H}(\mathrm{g})+\mathrm{H}(\mathrm{g}) ; \Delta_{\mathrm{a}} H^{\ominus}=435.8 \mathrm{~kJ} \mathrm{~mol}^{-1}
\)
Similarly the bond enthalpy for molecules containing multiple bonds, for example \(\mathrm{O}_2\) and \(\mathrm{N}_2\) will be as under :
\(
\mathrm{O}_2(\mathrm{O}=\mathrm{O})(\mathrm{g}) \rightarrow \mathrm{O}(\mathrm{g})+\mathrm{O}(\mathrm{g}) ;\Delta_{\mathrm{a}} H^{\ominus}=498 \mathrm{~kJ} \mathrm{~mol}^{-1}
\)
\(
\mathrm{N}_2(\mathrm{~N} \equiv \mathrm{N})(\mathrm{g}) \rightarrow \mathrm{N}(\mathrm{g})+\mathrm{N}(\mathrm{g}); \Delta_{\mathrm{a}} H^{\ominus}=946.0 \mathrm{~kJ} \mathrm{~mol}^{-1}
\)
It is important that larger the bond dissociation enthalpy, stronger will be the bond in the molecule. For a heteronuclear diatomic molecules like \(\mathrm{HCl}\), we have
\(
\mathrm{HCl}(\mathrm{g}) \rightarrow \mathrm{H}(\mathrm{g})+\mathrm{Cl}(\mathrm{g}) ; \Delta_{\mathrm{a}} H^{\ominus}=431.0 \mathrm{~kJ} \mathrm{~mol}{ }^{-1}
\)
In case of polyatomic molecules, the measurement of bond strength is more complicated. For example in case of \(\mathrm{H}_2 \mathrm{O}\) molecule, the enthalpy needed to break the two \(\mathrm{O}-\mathrm{H}\) bonds is not the same.
\(
\begin{aligned}
& \mathrm{H}_2 \mathrm{O}(\mathrm{g}) \rightarrow \mathrm{H}(\mathrm{g})+\mathrm{OH}(\mathrm{g}) ; \Delta_{\mathrm{a}} H_1^{\ominus}=502 \mathrm{~kJ} \mathrm{~mol}^{-1} \\
& \mathrm{OH}(\mathrm{g}) \rightarrow \mathrm{H}(\mathrm{g})+\mathrm{O}(\mathrm{g}) ; \Delta_{\mathrm{a}} H_2^{\ominus}=427 \mathrm{~kJ} \mathrm{~mol}{ }^{-1}
\end{aligned}
\)
The difference in the \(\Delta_{\mathrm{a}} H^{\ominus}\) value shows that the second \(\mathrm{O}-\mathrm{H}\) bond undergoes some change because of changed chemical environment. This is the reason for some difference in energy of the same \(\mathrm{O}-\mathrm{H}\) bond in different molecules like \(\mathrm{C}_2 \mathrm{H}_5 \mathrm{OH}\) (ethanol) and water. Therefore in polyatomic molecules the term mean or average bond enthalpy is used. It is obtained by dividing total bond dissociation enthalpy by the number of bonds broken as explained below in the case of water molecule,
\(
\begin{aligned}
\text { Average bond enthalpy } & =\frac{502+427}{2} \\
& =464.5 \mathrm{~kJ} \mathrm{~mol}^{-1}
\end{aligned}
\)

Bond Order

In the Lewis description of covalent bond, the Bond Order is given by the number of bonds between the two atoms in a molecule.

The bond order, for example in \(\mathrm{H}_2\) (with a single shared electron pair), in \(\mathrm{O}_2\) (with two shared electron pairs) and in \(\mathrm{N}_2\) (with three shared electron pairs) is \(1,2,3\) respectively. Similarly in CO (three shared electron pairs between \(\mathrm{C}\) and \(\mathrm{O}\) ) the bond order is 3. For \(\mathrm{N}_2\), bond order is 3 and its \(\Delta_{\mathrm{a}} H^{\ominus}\) is \(946 \mathrm{~kJ} \mathrm{~mol}^{-1}\); being one of the highest for a diatomic molecule.

Isoelectronic molecules and ions have identical bond orders; for example, \(\mathrm{F}_2\) and \(\mathrm{O}_2^{2-}\) have bond order \(1 . \mathrm{N}_2, \mathrm{CO}\) and \(\mathrm{NO}^{+}\) have bond order 3.

A general correlation useful for understanding the stablities of molecules is that: with the increase in bond order, bond enthalpy increases and bond length decreases.

Bond order \(\propto\) Bond Enthalpy \(\propto \frac{1}{\text { Bond length }}\)

Resonance Structures

It has been found that the observed properties of certain molecules cannot be satisfactorily explained by one structure. The molecule is then supposed to have many structures, each of which can explain most of the properties but not all. This phenomenon is called resonance.

It is often observed that a single Lewis structure is inadequate for the representation of a molecule in conformity with its experimentally determined parameters. For example, the ozone, \(\mathrm{O}_3\) molecule can be equally represented by the structures I and II shown below:

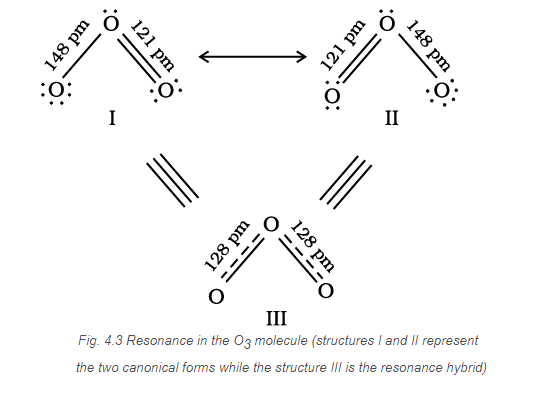
In both structures, we have a \(\mathrm{O}-\mathrm{O}\) single bond and a \(\mathrm{O}=\mathrm{O}\) double bond. The normal \(\mathrm{O}-\mathrm{O}\) and \(\mathrm{O}=\mathrm{O}\) bond lengths are \(148 \mathrm{pm}\) and \(121 \mathrm{pm}\) respectively. Experimentally determined oxygen-oxygen bond lengths in the \(\mathrm{O}_3\) molecule are same \((128 \mathrm{pm})\). Thus the oxygen-oxygen bonds in the \(\mathrm{O}_3\) molecule are intermediate between a double and a single bond. Obviously, this cannot be represented by either of the two Lewis structures shown above.

The concept of resonance was introduced to deal with the type of difficulty experienced in the depiction of accurate structures of molecules like \(\mathrm{O}_3\). According to the concept of resonance, whenever a single Lewis structure cannot describe a molecule accurately, a number of structures with similar energy, positions of nuclei, bonding and non-bonding pairs of electrons are taken as the canonical structures of the hybrid which describes the molecule accurately. Thus for \(\mathrm{O}_3\), the two structures shown above constitute the canonical structures or resonance structures and their hybrid i.e., the III structure represents the structure of \(\mathrm{O}_3\) more accurately. This is also called resonance hybrid. Resonance is represented by a double-headed arrow.
Some of the other examples of resonance structures are provided by the carbonate ion and the carbon dioxide molecule.

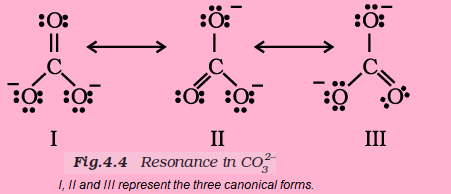
Example 4.3: Explain the structure of \(\mathrm{CO}_3^{2-}\) ion in terms of resonance.

Solution: The single Lewis structure based on the presence of two single bonds and one double bond between carbon and oxygen atoms is inadequate to represent the molecule accurately as it represents unequal bonds. According to the experimental findings, all carbon to oxygen bonds in \(\mathrm{CO}_3^{2-}\) are equivalent. Therefore the carbonate ion is best described as a resonance hybrid of the canonical forms I, II, and III shown below.

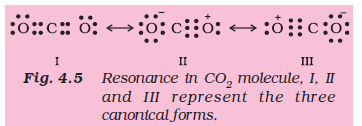
Example 4.4: Explain the structure of \(\mathrm{CO}_2\) molecule.

Solution: The experimentally determined carbon to oxygen bond length in \(\mathrm{CO}_2\) is \(115 \mathrm{pm}\). The lengths of a normal carbon to oxygen double bond \((\mathrm{C}=\mathrm{O})\) and carbon to oxygen triple bond \((\mathrm{C} \equiv \mathrm{O})\) are \(121 \mathrm{pm}\) and \(110 \mathrm{pm}\) respectively. The carbon-oxygen bond lengths in \(\mathrm{CO}_2(115 \mathrm{pm})\) lie between the values for \(\mathrm{C}=\mathrm{O}\) and \(\mathrm{C} \equiv \mathrm{O}\). Obviously, a single Lewis structure cannot depict this position and it becomes necessary to write more than one Lewis structures and to consider that the structure of \(\mathrm{CO}_2\) is best described as a hybrid of the canonical or resonance forms I, II and III.

Resonance Facts

In general, it may be stated that

• Resonance stabilizes the molecule as the energy of the resonance hybrid is less than the energy of any single cannonical structure; and,
• Resonance averages the bond characteristics as a whole.

Thus the energy of the \(\mathrm{O}_3\) resonance hybrid is lower than either of the two cannonical froms I and II (Fig 4.3).

Misconceptions about resonance

Many misconceptions are associated with resonance and the same need to be dispelled. You should remember that:

• The cannonical forms have no real existence.
• The molecule does not exist for a certain fraction of time in one cannonical form and for other fractions of time in other cannonical forms.
• There is no such equilibrium between the cannonical forms as we have between tautomeric forms (keto and enol) in tautomerism.
• The molecule as such has a single structure which is the resonance hybrid of the cannonical forms and which cannot as such be depicted by a single Lewis structure.

Polarity Bonds

The existence of a hundred percent ionic or covalent bond represents an ideal situation. In reality, no bond or a compound is either completely covalent or ionic. Even in the case of covalent bond between two hydrogen atoms, there is some ionic character.

When covalent bond is formed between two similar atoms, for example in \(\mathrm{H}_2, \mathrm{O}_2, \mathrm{Cl}_2\), \(\mathrm{N}_2\) or \(\mathrm{F}_2\), the shared pair of electrons is equally attracted by the two atoms. As a result electron pair is situated exactly between the two identical nuclei. The bond so formed is called nonpolar covalent bond. Contrary to this in the case of a heteronuclear molecule like \(\mathrm{HF}\), the shared electron pair between the two atoms gets displaced more towards fluorine since the electronegativity of fluorine is far greater than that of hydrogen. The resultant covalent bond is a polar covalent bond.

As a result of polarisation, the molecule possesses the dipole moment (depicted below) which can be defined as the product of the magnitude of the charge and the distance between the centres of positive and negative charge. It is usually designated by a Greek letter ‘ \(\mu\) ‘. Mathematically, it is expressed as follows :
Dipole moment \((\mu)=\) charge \((\mathrm{Q}) \times\) distance of separation (r)
Dipole moment is usually expressed in Debye units (D). The conversion factor is
\(
1 \mathrm{~D}=3.33564 \times 10^{-30} \mathrm{C} \mathrm{m}
\)
where \(\mathrm{C}\) is coulomb and \(\mathrm{m}\) is meter.
Further dipole moment is a vector quantity and by convention it is depicted by a small arrow with tail on the negative centre and head pointing towards the positive centre. But in chemistry presence of dipole moment is represented by the crossed arrow put on Lewis structure of the molecule. The cross is on positive end and arrowhead is on the negative end. For example, the dipole moment of HF may be represented as:

This arrow symbolises the direction of the shift of electron density in the molecule. Note that the direction of crossed arrow is opposite to the conventional direction of dipole moment vector.

Dipole Moment

In the case of polyatomic molecules the dipole moment not only depend upon the individual dipole moments of bonds known as bond dipoles but also on the spatial arrangement of various bonds in the molecule. In such case, the dipole moment of a molecule is the vector sum of the dipole moments of various bonds. For example in \(\mathrm{H}_2 \mathrm{O}\) molecule, which has a bent structure, the two \(\mathrm{O}-\mathrm{H}\) bonds are oriented at an angle of \(104.5^{\circ}\). Net dipole moment of 6.17 \(\times 10^{-30} \mathrm{C} \mathrm{m}\left(1 \mathrm{D}=3.33564 \times 10^{-30} \mathrm{C} \mathrm{m}\right)\) is the resultant of the dipole moments of two \(\mathrm{O}-\mathrm{H}\) bonds.

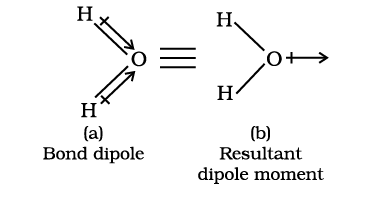
Net Dipole moment, \(\mu=1.85 \mathrm{D}\)
\(
=1.85 \times 3.33564 \times 10^{-30} \mathrm{C} \mathrm{m}=6.17 \times 10^{-30} \mathrm{C} \mathrm{m}
\)
The dipole moment in the case of \(\mathrm{BeF}_2\) is zero. This is because the two equal bond dipoles point in opposite directions and cancel the effect of each other.

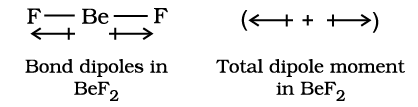
In tetra-atomic molecule, for example in \(\mathrm{BF}_3\), the dipole moment is zero although the \(\mathrm{B}-\mathrm{F}\) bonds are oriented at an angle of \(120^{\circ}\) to one another, the three bond moments give a net sum of zero as the resultant of any two is equal and opposite to the third.

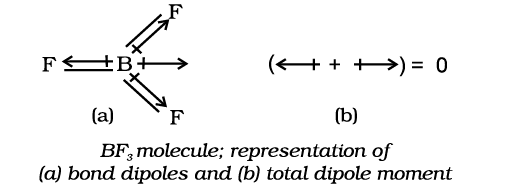
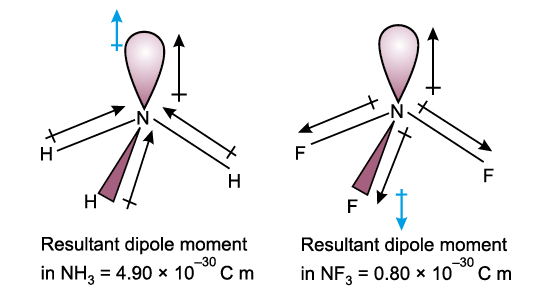
Let us study an interesting case of \(\mathrm{NH}_3\) and \(\mathrm{NF}_3\) molecule. Both the molecules have pyramidal shape with a lone pair of electrons on nitrogen atom. Although fluorine is more electronegative than nitrogen, the resultant dipole moment of \(\mathrm{NH}_3\left(4.90 \times 10^{-30} \mathrm{C} \mathrm{m}\right)\) is greater than that of \(\mathrm{NF}_3\left(0.8 \times 10^{-30} \mathrm{C} \mathrm{m}\right)\). This is because, in case of \(\mathrm{NH}_3\) the orbital dipole due to lone pair is in the same direction as the resultant dipole moment of the \(\mathrm{N}-\mathrm{H}\) bonds, whereas in \(\mathrm{NF}_3\) the orbital dipole is in the direction opposite to the resultant dipole moment of the three N-F bonds. The orbital dipole because of lone pair decreases the effect of the resultant \(\mathrm{N}-\mathrm{F}\) bond moments, which results in the low dipole moment of \(\mathrm{NF}_3\) as represented below :

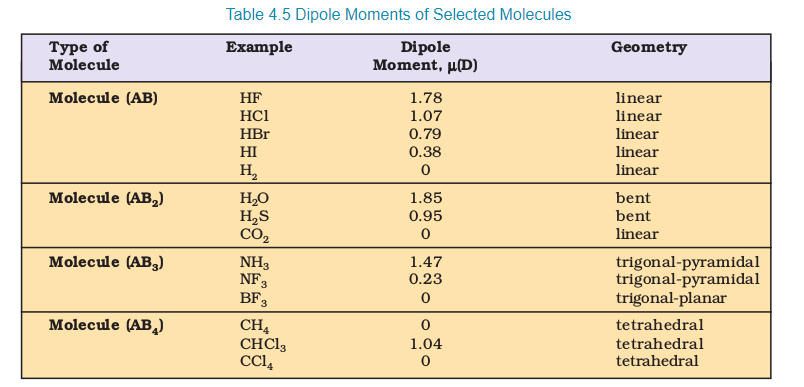
Dipole moments of some molecules are shown in Table 4.5.

Just as all the covalent bonds have some partial ionic character, the ionic bonds also have partial covalent character. The partial covalent character of ionic bonds was discussed by Fajans in terms of the following rules:

• The smaller the size of the cation and the larger the size of the anion, the greater the covalent character of an ionic bond.
• The greater the charge on the cation, the greater the covalent character of the ionic bond.
• For cations of the same size and charge, the one, with electronic configuration \((n-1) d^{\mathrm{n}} n s^0\), typical of transition metals, is more polarising than the one with a noble gas configuration, \(n s^2 n p^6\), typical of alkali and alkaline earth metal cations.
  The cation polarises the anion, pulling the electronic charge toward itself and thereby increasing the electronic charge between the two. This is precisely what happens in a covalent bond, i.e., buildup of electron charge density between the nuclei. The polarising power of the cation, the polarisability of the anion and the extent of distortion (polarisation) of anion are the factors, which determine the per cent covalent character of the ionic bond.

Note: Peter Debye, the Dutch chemist received the Nobel prize in 1936 for his work on X-ray diffraction and dipole moments. The magnitude of the dipole moment is given in Debye units in order to honour him.