3.9 Exercise Problems and Solutions

Q1. What is the basic theme of organisation in the periodic table?

Answer: The basic theme of organization of elements in the periodic table is to classify the elements in periods and groups according to their properties. This arrangement makes the study of elements and their compounds simple and systematic. In the periodic table, elements with similar properties are placed in the same group. This has made the study simple because the properties of elements are now studied in the form of groups rather than individually.

Q2. Which important property did Mendeleev use to classify the elements in his periodic table and did he stick to that?

Answer: Mendeleev arranged the elements in his periodic table ordered by atomic weight or mass. He arranged the elements in periods and groups in order of their increasing atomic weight. He placed the elements with similar properties in the same group. However, he did not stick to this arrangement for long. He found out that if the elements were arranged strictly in order of their increasing atomic weights, then some elements did not fit within this scheme of classification. Therefore, he ignored the order of atomic weights in some cases. For example, the atomic weight of iodine is lower than that of tellurium. Still, Mendeleev placed tellurium (in Group VI) before iodine (in Group VII) simply because iodine’s properties are so similar tofluorine, chlorine, and bromine.

Q3. What is the basic difference in approach between Mendeleev’s Periodic Law and the Modern Periodic Law?

Answer: The basic difference in approach between Mendeleev’s Periodic Law and Modem Periodic Law is the change in basis of the classification of elements from atomic weight to atomic number.

Q4. On the basis of quantum numbers, justify that the sixth period of the periodic table should have 32 elements.

Answer: The sixth period corresponds to the sixth shell. The orbitals present in this shell are \(6 \mathrm{~s}, 4 \mathrm{f}, 5 \mathrm{d}\), and \(6 \mathrm{~p}\). The maximum number of electrons which can be present in these sub-shell is \(2+14+6+10=32\). Since the number of elements in a period corresponds to the number of electrons in the shells, therefore, sixth period should have a maximum of 32 elements.

Q5. In terms of period and group where would you locate the element with \(Z=114\)?

Answer: Elements with atomic numbers from \(Z=87\) to \(Z=114\) are present in the 7th period of the periodic table. Thus, the element with \(\mathrm{Z}=114\) is present in the 7th period of the periodic table. In the 7 th period, first two elements with \(\mathrm{Z}=87\) and \(\mathrm{Z}=88\) are s-block elements, the next 14 elements excluding \(Z=89\) i.e., those with \(Z=90-103\) are \(f\) – block elements, ten elements with \(Z=89\) and \(Z=104-112\) are \(d-\) block elements, and the elements with \(Z=113-118\) are \(p-\) block elements. Therefore, the element with \(Z=114\) is the second \(\mathrm{p}\) – block element in the 7th period. Thus, the element with \(\mathrm{Z}=\mathbf{1 1 4}\) is present in the 7th period and 14th group of the periodic table.

Q6. Write the atomic number of the element present in the third period and seventeenth group of the periodic table.

Answer:

Periodic table:

• The periodic table is an arrangement of all the elements known to man in accordance with their increasing atomic number and recurring chemical properties.
• It consists of horizontal rows called Periods. There are a total of 7 periods.
• Vertical columns are called groups. There are a total of 18 groups.

Identifying the element present in the third period and the seventeenth group of the periodic table:

• The first period of the periodic table has a total of 2 elements.
• The second period of the periodic table has a total of 8 elements.
• The third period also consists of 8 elements.
• The third period begins with the element having an atomic number, \(Z=11\).
• In the third period, the first two elements( with \(Z=11 \& 12\) ) fall in group 1 and 2 .
• Elements with \(Z=13\) Onwards are placed in group 13 onwards.
• As a result of this, the element with atomic number \(Z=17\) is present in group seventeenth and third period of the periodic table.
• The element is Chlorine having atomic number 17.

Q7. Which element do you think would have been named by
(i) Lawrence Berkeley Laboratory
(ii) Seaborg’s group?

Answer: (i) Lawrencium ( \(\mathrm{Lr}\) ) with \(\mathrm{Z}=103\) and Berkelium (Bk) with \(\mathrm{Z}=97\)
(ii) Seaborgium (Sg) with Z = 106

Q8. Why do elements in the same group have similar physical and chemical properties?

Answer: The physical and chemical properties of elements depend on the number of valence electrons. Elements present in the same group have the same number of valence electrons. Therefore, elements present in the same group have similar physical and chemical properties.

Q9. What does atomic radius and ionic radius really mean to you?

Answer: Atomic radius: The distance from the centre of nucleus to the outermost shell electrons in the atom of any element is called its atomic radius. It refers to both covalen or metallic radius depending on whether the element is a non-metal or a metal.

Ionic radius: The lonic radii can be estimated by measuring the distances between cations and anions in ionic crystals.

Explanation: Atomic radius is the radius of an atom. It measures the size of an atom. If the element is a metal, then the atomic radius refers to the metallic radius, and if the element is a nonmetal, then it refers to the covalent radius. Metallic radius is calculated as half the internuclear distance separating the metal cores in the metallic crystal. For example, the internuclear distance between two adjacent copper atoms in solid copper is \(256 \mathrm{pm}\). Thus, the metallic radius of copper is taken as. \(\frac{256}{2} \mathrm{pm}=128 \mathrm{pm}\). Covalent radius is measured as the distance between two atoms when they are found together by a single bond in a covalent molecule. For example, the distance between two chlorine atoms in a chlorine molecule is \(198 \mathrm{pm}\). Thus, the covalent radius of chlorine is taken as \(\frac{198}{2} \mathrm{pm}=99 \mathrm{pm}\)

lonic radius means the radius of an ion (cation or anion). The ionic radii can be calculated by measuring the distances between the cations and anions in ionic crystals. Since a cation is formed by removing an electron from an atom, the cation has fewer electrons than the parent atom resulting in an increase in the effective nuclear charge. Thus, a cation is smaller than the parent atom. For example, the ionic radius of ion is \(95 \mathrm{pm}\), whereas the atomic radius of \(\mathrm{Na}\) atom is \(186 \mathrm{pm}\). On the other hand, an anion is larger in size than its parent atom. This is because an anion has the same nuclear charge, but more electrons than the parent atom resulting in an increased repulsion among the electrons and a decrease in the effective nuclear charge. For example, the ionic radius of \(\mathrm{F}\) ion is \(136 \mathrm{pm}\), whereas the atomic radius of \(\mathrm{F}\) atom is \(64 \mathrm{pm}\).

Q10. How do atomic radius vary in a period and in a group? How do you explain the variation?

Answer: Within a group Atomic radius increases down the group.
Reason: This is due to continuous increases in the number of electronic shells or orbit numbers in the structure of atoms of the elements down a group.
Variation across period: Atomic Radii. From left to right across a period atomic radii generally decreases due to increase in effective nuclear charge from left to right across a period.

Q11. What do you understand by isoelectronic species? Name a species that will be 1soelectronic with each of the following atoms or ions.
(i) \(\mathrm{F}^{-}\)
(ii) \(\mathrm{Ar}\)
(iii) \(\mathrm{Mg}^{2+}\)
(iv) \(\mathrm{Rb}^{+}\)

Answer: Atoms and ions having the same number of electrons are called isoelectronic species.
(i) \(\mathrm{F}^{-}\)ion has \(9+1=10\) electrons. Thus, the species isoelectronic with it will also have 10 electrons. Some of its isoelectronic species are \(\mathrm{Na}^{+}\)ion \((11-1=10\) electrons), \(\mathrm{Ne}\) (10 electrons), \(\mathrm{O}^{2-}\) ion ( \(8+2=10\) electrons), and \(\mathrm{Al}^{3+}\) ion \((13-3\) \(=10\) electrons).
(ii) Ar has 18 electrons. Thus, the species isoelectronic with it will also have 18 electrons. Some of its isoelectronic species are \(\mathrm{S}^{2-}\) ion \((16+2=18\) electrons), \(\mathrm{Cl}^{-}\)ion \((17+1=18\) electrons \(), \mathrm{K}^{+}\)ion ( \(19-1=18\) electrons \()\), and \(\mathrm{Ca}^{2+}\) ion \((20-2\) \(=18\) electrons).
(iii) \(\mathrm{Mg}^{2+}\) ion has \(12-2=10\) electrons. Thus, the species isoelectronic with it will also have 10 electrons. Some of its isoelectronic species are \(\mathrm{F}^{-}\)ion \((9+1=\) 10 electrons), \(\mathrm{Ne}\) (10 electrons), \(\mathrm{O}^{2-}\) ion \(\left(8+2=10\right.\) electrons), and \(\mathrm{Al}^{3+}\) ion \((13-\) \(3=10\) electrons).
(iv) \(\mathrm{Rb}^{+}\)ion has \(37-1=36\) electrons. Thus, the species isoelectronic with it will also have 36 electrons. Some of its isoelectronic species are \(\mathrm{Br}^{-}\)ion \((35+1=36\) electrons), \(\mathrm{Kr}\) (36 electrons), and \(\mathrm{Sr}^{2+}\) ion ( \(38-2=36\) electrons).

Q12. Consider the following species :
\(\mathrm{N}^{3-}, \mathrm{O}^{2-}, \mathrm{F}^{-}, \mathrm{Na}^{+}, \mathrm{Mg}^{2+}\) and \(\mathrm{Al}^{3+}\)
(a) What is common in them?
(b) Arrange them in the order of increasing ionic radii.

Answer: (a) All of them are isoelectronic in nature and have 10 electrons each.
(b) In isoelectronic species, the greater the nuclear charge, the lesser will be the atomic or ionic radius. \(\mathrm{Al}^{3+}<\mathrm{Mg}^{2+}<\mathrm{Na}^{+}<\mathrm{F}^{-}<\mathrm{O}^{2-}<\mathrm{N}^{3-}\)

Q13. Explain why cation are smaller and anions larger in radii than their parent atoms.

Answer: A cation is smaller than the parent atom because it has fewer electrons while its nuclear charge remains the same. The size of the anion will be larger than that of the parent atom because the addition of one or more electrons would result in increased repulsion among the electrons and a decrease in effective nuclear charge.

Q14. What is the significance of the terms – ‘isolated gaseous atom’ and ‘ground state’ while defining the ionization enthalpy and electron gain enthalpy?

Answer: Significance of the term ‘isolated gaseous atom’: The atoms in the gaseous state are far separated in the sense that they do not have any mutual attractive and repulsive interactions. These are therefore regarded as isolated atoms. In this state the value of ionization enthalpy and electron gain enthalpy are not influenced by the presence of the other atoms. It is not possible to express these when the atoms are in the; liquid or solid state due to the presence of inter-atomic forces.
Significance of ground state: Ground state of the atom represents the normal – energy state of an atom. It means electrons in a particular atom are in the lowest energy state and they neither lose nor gain electrons. Both ionisation enthalpy and I electron gain enthalpy are generally expressed with respect to the ground state of an atom only.

Q15. The energy of an electron in the ground state of the hydrogen atom is \(-2.18 \times 10^{-18} \mathrm{~J}\). Calculate the ionization enthalpy of atomic hydrogen in terms of \(\mathrm{J} \mathrm{mol}^{-1}\).

Answer: The energy of an electron in the ground state of the hydrogen atom is \(-2.18 \times\) \(10^{-18} \mathrm{~J}\). Therefore, the energy required to remove that electron from the ground state of the hydrogen atom is \(2.18 \times 10^{-18} \mathrm{~J}\).
\(\therefore\) Ionization enthalpy of atomic hydrogen \(=2.18 \times 10^{-18} \mathrm{~J}\)
Hence, ionization enthalpy of atomic hydrogen in terms of \(\mathrm{J} \mathrm{mol}^{-1}\) \(=2.18 \times 10^{-18} \times 6.02 \times 10^{23} \mathrm{~J} \mathrm{~mol}^{-1}=1.31 \times 10^6 \mathrm{~J} \mathrm{~mol}^{-1}\)

Q16. Among the second period elements the actual ionization enthalpies are in the order \(\mathrm{Li} <\mathrm{B}<\mathrm{Be}<\mathrm{C}<\mathrm{O}<\mathrm{N}<\mathrm{F}<\mathrm{Ne}\).
Explain why
(i) Be has higher \(\Delta_i H\) than B
(ii) O has lower \(\Delta_{{i}} H\) than \(\mathrm{N}\) and \(\mathrm{F}\)?

Answer: (i) In case of \(\mathrm{Be}\left(1 s^2 2 s^2\right)\) the outermost electron is present in \(2 \mathrm{~s}\)-orbital while in \(\mathrm{B}\left(1 \mathrm{~s}^2 2 \mathrm{~s}^2 2 \mathrm{p}^1\right)\) it is present in \(2 p\)-orbital.
During the process of ionization, the electron to be removed from the beryllium atom is a \(2 \mathrm{~s}\)-electron, whereas the electron to be removed from the boron atom is a \(2 p\)-electron. Now, \(2 \mathrm{~s}\)-electrons are more strongly attached to the nucleus than \(2 p\)-electrons. Therefore, more energy is required to remove a \(2 \mathrm{~s}\)-electron of beryllium than that required to remove a \(2 p\)-electron of boron. Hence, beryllium has higher \(\Delta_i H\) than boron.
(ii)In nitrogen, the three \(2 p\)-electrons of nitrogen occupy three different atomic orbitals. However, in oxygen, two of the four \(2 p\)-electrons of oxygen occupy the same \(2 \mathrm{p}\)-orbital. This results in increased electron-electron repulsion in the oxygen atom. As a result, the energy required to remove the fourth \(2 p\)-electron from oxygen is less as compared to the energy required to remove one of the three \(2 \mathrm{p}\)-electrons from nitrogen. Hence, oxygen has lower \(\Delta_i H\) than nitrogen.
Fluorine contains one electron and one proton more than oxygen. As the electron is being added to the same shell, the increase in nuclear attraction (due to the addition of a proton) is more than the increase in electronic repulsion (due to the addition of an electron). Therefore, the valence electrons in the fluorine atom experience a more effective nuclear charge than that experienced by the electrons present in oxygen. As a result, more energy is required to remove an electron from a fluorine atom than that required to remove an electron from an oxygen atom. Hence, oxygen has lower \(\Delta_i H\) than fluorine.

Q17. How would you explain the fact that the first ionization enthalpy of sodium is lower than that of magnesium but its second ionization enthalpy is higher than that of magnesium?

Answer: Electronic configuration of \(\mathrm{Na}\) and \(\mathrm{Mg}\) are
\(
\begin{aligned}
& \mathrm{Na}=1 s^2 2 s^2 2 p^6 3 s^1 \\
& \mathrm{Mg}=1 s^2 2 s^2 2 p^6 3 s^2
\end{aligned}
\)
The first electron in both cases has to be removed from 3s-orbital but the nuclear charge of \(\mathrm{Na}(+11)\) is lower than that of \(\mathrm{Mg}(+12)\) therefore first ionization energy of sodium is lower than that of magnesium. After the loss of the first electron, the electronic configuration of
\(
\begin{aligned}
& \mathrm{Na}^{+}=1 s^2 2 s^2 2 p^6 \\
& \mathrm{Mg}^{+}=1 s^2 2 s^2 2 p^6 3 s^1
\end{aligned}
\)
Here electron is to be removed from an inert (neon) gas configuration which is very stable and hence removal of the second electron requires more energy in comparison to \(\mathrm{Mg}\).
Therefore, the second ionization enthalpy of sodium is higher than that of magnesium.

Q18. What are the various factors due to which the ionization enthalpy of the main group elements tends to decrease down a group?

Answer: Atomic size: With the increase in atomic size, the number of electron shells increases. Therefore, the force that binds the electrons with the nucleus decreases. The ionization enthalpy thus decreases with the increase in atomic size.
Screening or shielding effect of inner shell electron: With the addition of new shells, the number of inner electron shells which shield the valence electrons increases. As a result, the force of attraction of the nucleus for the valence electrons further decreases and hence the ionization enthalpy decreases. 

Alternate Explanation: The factors responsible for the ionization enthalpy of the main group elements to decrease down a group are listed below:
(i) Increase in the atomic size of elements: As we move down a group, the number of shells increases. As a result, the atomic size also increases gradually on moving down a group. As the distance of the valence electrons from the nucleus increases, the electrons are not held very strongly. Thus, they can be removed easily. Hence, on moving down a group, ionization energy decreases.
(ii) Increase in the shielding effect: The number of inner shells of electrons increases on moving down a group. Therefore, the shielding of the valence electrons from the nucleus by the inner core electrons increases down a group. As a result, the valence electrons are not held very tightly by the nucleus. Hence, the energy required to remove a valence electron decreases down a group.

Q19. The first ionization enthalpy values \(\text { (in } \mathrm{kJ} \mathrm{mol}^{-1} \text { ) }\) of group 13 elements are :
\(\begin{array}{lllll}\text { B} & \text { Al} & \text { Ga } & \text { In } & \text { Tl } & \\ 801 & 577 & 579 & 558 & 589\end{array}\)
How would you explain this deviation from the general trend?

Answer: Down the group ( \(\downarrow\) ), from \(B\) to \(A l\), the ionisation enthalpy decreases due to an increase in size and screening effect. The ionisation enthalpy of \(G a\) is slightly higher than \(A l, G a\), and In. These deviations from the general trend can be explained on the basis of electronic configuration.
\(
\begin{array}{|l|c|c|c|c|c|}
\hline {\text { Element }} & \mathrm{B} & \mathrm{Al} & \mathrm{Ga} & \mathrm{In} & \mathrm{Tl} \\
\hline \begin{array}{l}
\text { Electronic } \\
\text { configuration }
\end{array} & {[\mathrm{He}] 2 s^2} & {[\mathrm{Ne}] 3 s^2} & {[\mathrm{Ar}] 3 d^{10}} & {[\mathrm{Kr}] 4 d^{10}} & {[\mathrm{Xe}] 4 f^{14} 5 d^{10}} \\
& 2 p^1 & 3 p^1 & 4 s^2 4 p^1 & 5 s^2 5 p^1 & 6 s^2 6 p^1 \\
\hline
\end{array}
\)

Explanation: On moving down a group, ionization enthalpy generally decreases due to an increase in the atomic size and shielding. Thus, on moving down group 13, ionization enthalpy decreases from B to Al. But, Ga has higher ionization enthalpy than Al. Al follows immediately after s-block elements, whereas Ga follows after d-block elements. The shielding provided by d-electrons is not very effective. These electrons do not shield the valence electrons very effectively. As a result, the valence electrons of Ga experience a greater effective nuclear charge than those of Al. Further, moving from Ga to In, the ionization enthalpy decreases due to an increase in the atomic size and shielding. But, on moving from In to TI, the ionization enthalpy again increases. In the periodic table, TI follows after \(4 \mathrm{f}\) and \(5 \mathrm{~d}\) electrons. The shielding provided by the electrons in both these orbitals is not very effective. Therefore, the valence electron is held quite strongly by the nucleus. Hence, the ionization energy of \(\mathrm{TI}\) is on the higher side

Q20. Which of the following pairs of elements would have a more negative electron gain enthalpy?
(i) \(\mathrm{O}\) or \(\mathrm{F}\)
(i) \(\mathrm{F}\) or \(\mathrm{Cl}\)

Answer: (i) \(\mathrm{O}\) or \(\mathrm{F}\). Both \(\mathrm{O}\) and \(\mathrm{F}\) lie in 2 nd period. As we move from \(\mathrm{O}\) to \(\mathrm{F}\) the atomic size decreases.
Due to the smaller size of \(\mathrm{F}\) nuclear charge increases.
Further, the gain of one electron by
\(\mathrm{F} \rightarrow \mathrm{F}^{-}\)
\(\mathrm{F}\) -ion has an inert gas configuration, While the gain of one electron by
\(
O->O^{-}
\)
gives \(\mathrm{CT}\) ion which does not have a stable inert gas configuration, consequently, the energy released is much higher in going from \(\mathrm{F} \rightarrow \mathrm{F}^{-}\) than going from \(\mathrm{O} \rightarrow \mathrm{O}^{-}\) In other words electron gain enthalpy of \(\mathrm{F}\) is much more negative than that of oxygen.
(ii) The negative electron gain enthalpy of \(\mathrm{Cl}(\Delta \mathrm{eg} \mathrm{~H}=-349 \mathrm{~kJ} \mathrm{mol}^{-1}\) is more than that of \(\mathrm{F}(\Delta \mathrm{eg} \mathrm{~H}=-328 \mathrm{~kJ} \mathrm{mol}^{-1}\)
The reason for the deviation is due to the smaller size of F. Due to its small size, the electron repulsions in the relatively compact 2p-subshell are comparatively large and hence the attraction for incoming electrons is less as in the case of \(\mathrm{Cl}\).

Explanation: (i) \(\mathrm{O}\) and \(\mathrm{F}\) are present in the same period of the periodic table. An \(\mathrm{F}\) atom has one proton and one electron more than \(\mathrm{O}\) and as an electron is being added to the same shell, the atomic size of \(\mathrm{F}\) is smaller than that of \(\mathrm{O}\). As \(\mathrm{F}\) contains one proton more than \(\mathrm{O}\), its nucleus can attract the incoming electron more strongly in comparison to the nucleus of \(\mathrm{O}\) atom. Also, \(\mathrm{F}\) needs only one more electron to attain the stable noble gas configuration. Hence, the electron gain enthalpy of \(F\) is more negative than that of \(O\).
(ii) \(\mathrm{F}\) and \(\mathrm{Cl}\) belong to the same group of the periodic table. The electron gain enthalpy usually becomes less negative on moving down a group. However, in this case, the value of the electron gain enthalpy of \(\mathrm{Cl}\) is more negative than that of \(\mathrm{F}\). This is because the atomic size of \(\mathrm{F}\) is smaller than that of \(\mathrm{Cl}\). In \(\mathrm{F}\), the electron will be added to quantum level \(\mathrm{n}=2\), but in \(\mathrm{Cl}\), the electron is added to quantum level \(\mathrm{n}=3\). Therefore, there are less electron-electron repulsions in \(\mathrm{Cl}\) and an additional electron can be accommodated easily. Hence, the electron gain enthalpy of \(\mathrm{Cl}\) is more negative than that of \(\mathrm{F}\).

Q21. Would you expect the second electron gain enthalpy of \(\mathrm{O}\) as positive, more negative or less negative than the first? Justify your answer.

Answer:

\(
\begin{aligned}
& \mathrm{O}(\mathrm{g})+\mathrm{e}^{-} \rightarrow \mathrm{O}^{-}(\mathrm{g})\left(\Delta_{\mathrm{eg}} \mathrm{H}=-141 \mathrm{~kJ} \mathrm{~mol}^{-1}\right) \\
& \mathrm{O}^{-}(\mathrm{g})+\mathrm{e}^{-} \rightarrow \mathrm{O}^{2-}(\mathrm{g})\left(\Delta_{\mathrm{eg}} \mathrm{H}=+780 \mathrm{~kJ} \mathrm{~mol}^{-1}\right)
\end{aligned}
\)
The first electron gain enthalpy of oxygen is negative because energy is released when a gaseous atom accepts an electron to form a monovalent anion. The second electron gain enthalpy is positive because energy is needed to overcome the force of repulsion between the monovalent anion and the second incoming electron.

Q22. What is the basic difference between the terms electron gain enthalpy and electronegativity?

Answer: Electron gain enthalpy refers to the tendency of an isolated gaseous atom to accept an additional electron to form a negative ion. Whereas electronegativity refers to the tendency of the atom of an element to attract a shared pair of electrons towards it in a covalent bond.

Q23. How would you react to the statement that the electronegativity of \(\mathrm{N}\) on the Pauling scale is \(3.0 \mathrm{~in}\) all the nitrogen compounds?

Answer: On the Pauling scale, the electronegativity of nitrogen, (3.0) indicates that it is sufficiently electronegative. However it is not correct to say that the electronegativity of nitrogen in all the compounds is 3. It depends upon its state of hybridisation in a particular compound, the greater the percentage of s-character, the more will be the electronegativity of the element. Thus, the electronegativity of nitrogen increases in moving from \(\mathrm{SP}^3\) hybridised orbitals to \(\mathrm{SP}\) hybridised orbitals i.e., as \(\mathrm{SP}^3<\mathrm{SP}^2<\mathrm{SP}\).

Q24. Describe the theory associated with the radius of an atom as it
(a) gains an electron
(b) loses an electron

Answer: (a) Gain of an electron leads to the formation of an anion. The size of an anion will be larger than that of the parent atom because the addition of one or more electrons would result in increased repulsion among electrons and a decrease in effective nuclear charge.
This the ionic radius of fluoride ion \(\left(\mathrm{F}^{-}\right)\)is \(136 \mathrm{pm}\) whereas atomic radius of Fluorine \((\mathrm{F})\) is only \(64 \mathrm{pm}\).
(b) Loss of an electron from an atom results in the formation of a cation. A cation is smaller than its parent atom because it has former electrons while its nuclear charge remains the same. For example, The atomic radius of sodium \((\mathrm{Na})\) is \(186 \mathrm{pm}\) and atomic radius of sodium ion \(\left(\mathrm{Na}^{+}\right)=95 \mathrm{pm}\).

Q25. Would you expect the first ionization enthalpies for two isotopes of the same element to be the same or different? Justify your answer.

Answer: Ionization enthalpy, among other things, depends upon the electronic configuration (number of electrons) and nuclear charge (number of protons). Since isotopes of an element have the same electronic configuration and same nuclear charge, they have the same ionization enthalpy.

Q26. What are the major differences between metals and non-metals?

Answer: 

\(
\begin{array}{|c|c|}
\hline \text { Metals } & \text { Non-Metals } \\
\hline \begin{array}{l}
\text { 1. Have a strong tendency to lose electrons to } \\
\text { form cations. }
\end{array} & \begin{array}{l}
\text { 1. Non-metals have a strong tendency to accept } \\
\text { electrons to form anions. }
\end{array} \\
\hline \text { 2. Metals are strong reducing agents. } & \text { 2. Non-metals are strong oxidising agent. } \\
\hline \text { 3. Metals have low ionization enthalpies. } & \text { 3. Non-metals have high ionization enthalpies. } \\
\hline \begin{array}{l}
\text { 4. Metals form basic oxides and ionic } \\
\text { compounds. }
\end{array} & \begin{array}{l}
\text { 4. Non-metals form acidic oxides and covalent } \\
\text { compounds. }
\end{array} \\
\hline
\end{array}
\)

Q27. Use the periodic table to answer the following questions.
(a) Identify an element with five electrons in the outer subshell.
(b) Identify an element that would tend to lose two electrons.
(c) Identify an element that would tend to gain two electrons.
(d) Identify the group having metal, non-metal, liquid as well as gas at the room temperature.

Answer: (a) The electronic configuration of an element having 5 electrons in its outermost subshell should be \(\mathrm{ns}^2 \mathrm{np}^5\)
. This is the electronic configuration of the halogen group. Thus, the element can be \(\mathrm{F}, \mathrm{Cl}, \mathrm{Br}\), I, or At.
(b) An element having two valence electrons will lose two electrons easily to attain the stable noble gas configuration. The general electronic configuration of such an element will be \(\mathrm{ns}^2\). This is the electronic configuration of group 2 elements. The elements present in group 2 are \(\mathrm{Be}, \mathrm{Mg}, \mathrm{Ca}, \mathrm{Sr}, \mathrm{Ba}\).
(c) An element is likely to gain two electrons if it needs only two electrons to attain the stable noble gas configuration. Thus, the general electronic configuration of such an element should be \(\mathrm{ns}^2 \mathrm{np}^4\). This is the electronic configuration of the oxygen family.
(d) Group 17 has metalloid, non-metal, liquid as well as gas at room temperature. \(\mathrm{F}\) and \(\mathrm{Cl}\) are the gaseous states, \(\mathrm{Br}\) is liquid state and I is solid state. Here, the \(\mathrm{F}, \mathrm{Cl}, \mathrm{Br}\) and I are non-metals whereas, At is metalloid.

Q28. The increasing order of reactivity among group 1 elements is \(\mathrm{L} i<\mathrm{Na}<\mathrm{K}<\mathrm{Rb}<\mathrm{Cs}\) whereas that among group 17 elements is \(\mathrm{F}>\mathrm{Cl}>\mathrm{Br}>\mathrm{I}\). Explain.

Answer: The elements present in group 1 have only 1 valence electron, which they tend to lose. Group 17 elements, on the other hand, need only one electron to attain the noble gas configuration. On moving down group 1 , the ionization enthalpies decrease. This means that the energy required to lose the valence electron decreases. Thus, reactivity increases on moving down a group. Thus, the increasing order of reactivity among group 1 elements is as follows: \(\mathrm{Li}<\mathrm{Na}<\mathrm{K}<\mathrm{Rb}<\mathrm{Cs}\)
In group 17, as we move down the group from \(\mathrm{Cl}\) to \(\mathrm{I}\), the electron gain enthalpy becomes less negative i.e., its tendency to gain electrons decreases down group 17. Thus, reactivity decreases down a group. The electron gain enthalpy of \(\mathrm{F}\) is less negative than \(\mathrm{Cl}\). Still, it is the most reactive halogen. This is because of its low bond dissociation energy. Thus, the decreasing order of reactivity among group 17 elements is as follows: \(\mathrm{F}>\mathrm{Cl}>\mathrm{Br}>\mathrm{I}\)

Q29. Write the general outer electronic configuration of \(s-, p\)-, \(d\) – and \(f\)-block elements.

Answer: Electronic configuration: Electronic configuration is the distribution of the electrons in orbitals of atoms using some basic principles like the Pauli exclusion principle and the Aufbau principle.

s-block elements:

• The general electronic configuration of \(\mathrm{s}\)-block element is \({ns}^{(1-2)}, \text { where } n=2-7 \text {. }\).
• The s-block elements are at the left corner of the periodic table and those elements have their outer electrons in \(\mathbf{s}\)-orbitals.
• The group 1 & 2 elements are called s-block elements.

p-block elements:

• The general electronic configuration of \(\mathrm{p}\)-block elements is \(n s^2 n p^{1-6}, \text { where } n=2-6 \text {. }\).
• The \(\mathrm{p}\)-block elements are in the right corner of the periodic table and those elements have their outer electrons in \(\mathrm{p}\)-orbitals.
• The group \(13-18\) includes \(\mathrm{p}\)-block elements.

d-block elements:

• The general electronic configuration of \(\mathrm{d}-\) block element is
  \(
  (\mathrm{n}-1) \mathrm{d}^{(1-10)} \mathrm{ns}^{(1-2)} \text { where } n=4,5,6,7
  \)
• The d-block elements are in the middle of the periodic table and those elements have their outer electrons in \(\mathrm{d}\)-orbitals.
• The group in the middle are \(\mathrm{d}\)-block elements.

f-block elements:

• The general electronic configuration of \(\mathrm{f}\)-block element is \((n-2) f^{0-14}(n-1) d^{(0-10)} n s^2 \text { where } n=6,7\).
• The \(\mathrm{f}\)-block elements are at the bottom of the periodic table and those elements have their outer electrons in \(\mathrm{f}\)-orbitals.
• These elements are at the bottom of the periodic table.

Q30. Assign the position of the element having outer electronic configuration (i) \(n s^2 n p^4\) for \(n=3\) (ii) \((n-1) d^2 n s^2\) for \(n=4\), and (iii) (n-2) \(f^7(n-1) d^1 n s^2\) for \(n=6\), in the periodic table.

Answer: (i)

\(n=3\)
Thus element belongs to the 3rd period, the p-block element.
Since the valence shell contains \(=6\) electrons, group \(\mathrm{No}=10+6=16\) configuration \(=1 \mathrm{~s}^2 2 \mathrm{~s}^2 2 \mathrm{p}^6 3 \mathrm{~s}^2\)
\(3 p^4\) element name is sulphur.
(ii) \(n=4\)
This means the element belongs to 4 4th period belongs to group 4 as in the valence shell \((2+2)=4\) electrons.
Electronic configuration. \(=1 s^2 2 s^2 2 p^6 3 s^2 3 p^6 3 d^2 4 s^2\), and the element name is Titanium \(\left(T_i\right)\).
(iii) \(\mathrm{n}=6\)
“Means the element belongs to the 6th period. The last electron goes to the f-orbital, element is from f-block.
\(
\text { group }=3
\)
The element is gadolinium \((z=64)\)
Complete electronic configuration \(=\left[\mathrm{X}_{\mathrm{e}}\right] 4 \mathrm{f}^7 5 \mathrm{~d}^1 6 \mathrm{~s}^2\). Thus, its atomic number is 54+7+2+1=64. Hence, the element is Gadolinium.

Q31. The first \(\left(\Delta_i H_1\right)\) and the second \(\left(\Delta_i H_2\right)\) ionization enthalples \(\left(\mathrm{in} \mathrm{~kJ} \mathrm{~mol} \mathrm{k}^{-1}\right. \text { ) }\) and the \(\left(\Delta_{e g} H\right)\) electron gain enthalpy \(\left(\mathrm{in} \mathrm{~kJ} \mathrm{~mol} \mathrm{k}^{-1}\right. \text { ) }\) of a few elements are given below:
\(
\begin{array}{llll}
\text { Elements } & \Delta H_1 & \Delta H_2 & \Delta_{e g} H \\
\text { I } & 520 & 7300 & -60 \\
\text { II } & 419 & 3051 & -48 \\
\text { III } & 1681 & 3374 & -328 \\
\text { IV } & 1008 & 1846 & -295 \\
\text { V } & 2372 & 5251 & +48 \\
\text { VI } & 738 & 1451 & -40
\end{array}
\)
Which of the above elements is likely to be :
(a) the least reactive element.
(b) the most reactive metal.
(c) the most reactive non-metal.
(d) the least reactive non-metal.
(e) the metal which can form a stable binary halide of the formula \(\mathrm{MX}_2(\mathrm{X}=\) halogen \()\).
(f) the metal which can form a predominantly stable covalent halide of the formula \(\mathrm{MX}(\mathrm{X}=\) halogen \()\)?

Answer: (a) The element \(V\) has highest first ionization enthalpy \(\left(\Delta_i H_1\right)\) and positive electron gain enthalpy \(\left(\Delta_{e g} H\right)\) and hence it is the least reactive element. Since inert gases have positive \(\Delta_{e g} H\), therefore, the element- \(V\) must be an inert gas. The values of \(\Delta_i H_1, \Delta_i H_2\) and \(\Delta_{\mathrm{eg}} \mathrm{H}\) match that of \(\mathrm{He}\).
(b) The element II which has the least first ionization enthalpy \(\left(\Delta_{\mathrm{i}} \mathrm{H}_1\right)\) and a low negative electron gain enthalpy \(\left(\Delta_{e g} \mathrm{H}\right)\) is the most reactive metal. The values of \(\Delta_i \mathrm{H}_1, \Delta_i \mathrm{H}_2\) and \(\Delta_{e g} \mathrm{H}\) match that of \(\mathrm{K}\) (potassium).
(c) The element III which has high first ionization enthalpy \(\left(\Delta_{\mathrm{i}} \mathrm{H}_1\right)\) and a very high negative electron gain enthalpy \(\left(\Delta_{\mathrm{eg}} \mathrm{H}\right)\) is the most reactive non-metal. The values of \(\Delta_{\mathrm{i}} \mathrm{H}_1, \Delta_{\mathrm{i}} \mathrm{H}_2\) and \(\Delta_{\mathrm{eg}} \mathrm{H}\) match that of \(\mathrm{F}\) (fluorine).
(d) The element IV has a high negative electron gain enthalpy \(\left(\Delta_{\mathrm{eg}} \mathrm{H}\right)\) but not so high first ionization enthalpy \(\left(\Delta_{\mathrm{eg}} H\right)\). Therefore, it is the least reactive non-metal. The values of \(\Delta_i H_1, \Delta_i H_2\) and \(\Delta_{\mathrm{eg}} H\) match that of \(\mathrm{I}\) (lodine).
(e) The element \(\mathrm{VI}\) has low first ionization enthalpy \(\left(\Delta_i \mathrm{H}_1\right)\) but higher than that of alkali metals. Therefore, it appears that the element is an alkaline earth metal and hence will form binary halide of the formula \(\mathrm{MX}_2\) (where \(\mathrm{X}=\) halogen). The values of \(\Delta_i \mathrm{H}_1, \Delta_i \mathrm{H}_2\) and \(\Delta_{\mathrm{eg}} \mathrm{H}\) match that of \(\mathrm{Mg}\) (magnesium).
(f) The element I has low first ionization \(\left(\Delta_{\mathrm{i}} \mathrm{H}_1\right)\) but a very high second ionization enthalpy \(\left(\Delta_{\mathrm{i}} \mathrm{H}_2\right)\), therefore, it must be an alkali metal. Since the metal forms a predominantly stable covalent halide of the formula MX (\(\mathrm{X}=\) halogen), therefore, the alkali metal must be the least reactive. The values of \(\Delta_i H_1, \Delta_i H_2\) and \(\Delta_{\text {eg }} H\) match that of Li (lithium).

Q32. Predict the formulas of the stable binary compounds that would be formed by the combination of the following pairs of elements.
(a) Lithium and oxygen
(b) Magnesium and nitrogen
(c) Alumintum and todine
(d) Silicon and oxygen
(e) Phosphorus and fluorine
(f) Element 71 and fluorine

Answer:

\(
\text { (a) } \mathrm{LiO}_2 \text { (Lithium oxide) (b) } \mathrm{Mg}_3 \mathrm{~N}_2 \text { (Magnesium nitride) }
\)
\(
\text { (c) } \mathrm{AlI}_3 \text { (Aluminium iodide) (d) } \mathrm{SiO}_2 \text { (Silicon dioxide) }
\)
\(
\text { (e) Phosphorous and fluorine (f) Z = } 71
\)
The element is Lutenium \((\mathrm{Lu})\). Electronic configuration \(\left[\mathrm{X}_{\mathrm{e}}\right] 4 \mathrm{f}^7 5 \mathrm{~d}^1 6 \mathrm{~s}^2\). with fluorine, it will form a binary compound \(=Lu F 3\)

Q33. In the modern periodic table, the period indicates the value of :
(a) atomic number
(b) atomic mass
(c) principal quantum number
(d) azimuthal quantum number.

Answer: In the modern periodic table, each period begins with the filling of a new shell. Therefore, the period indicates the value of the principal quantum number. Thus, option (c) is correct.

Q34. Which of the following statements related to the modern periodic table is incorrect?
(a) The \(p\)-block has 6 columns because a maximum of 6 electrons can occupy all the orbitals in a \(p\)-shell.
(b) The \(d\)-block has 8 columns because a maximum of 8 electrons can occupy all the orbitals in a \(d\)-subshell.
(c) Each block contains a number of columns equal to the number of electrons that can occupy that subshell.
(d) The block indicates the value of the azimuthal quantum number ( \(l\) ) for the last subshell that received electrons in building up the electronic configuration.

Answer: The d-block has 5 columns because a maximum of 10 electrons can occupy all the orbitals in a d subshell. (b) is the correct answer.

Q35. Anything that influences the valence electrons will affect the chemistry of the element. Which one of the following factors does not affect the valence shell?
(a) Valence principal quantum number \((n)\)
(b) Nuclear charge \((Z)\)
(c) Nuclear mass
(d) Number of core electrons.

Answer: Nuclear mass does not affect the valence electrons. (c) is the correct answer.

Q36. The size of isoelectronic species- \(\mathrm{F}^{-}, \mathrm{Ne}\) and \(\mathrm{Na}^{+}\)is affected by
(a) nuclear charge \((Z)\)
(b) valence principal quantum number \((n)\)
(c) electron-electron interaction in the outer orbitals
(d) none of the factors because their size is the same.

Answer: The size of an isoelectronic species increases with a decrease in the nuclear charge (Z). For example, the order of the increasing nuclear charge of \(\mathrm{F}^{-}\) , \(\mathrm{Ne}\), and \(\mathrm{Na}^{+}\) is as follows:
\(
\begin{aligned}
& \mathrm{F}^{-}<\mathrm{Ne}<\mathrm{Na}^{+} \\
& Z \quad 9 \quad 10 \quad 11
\end{aligned}
\)
Therefore, the order of the increasing size of \(\mathrm{F}^{-}, \mathrm{Ne}\) and \(\mathrm{Na}^{+}\)is as follows :
\(
\mathrm{Na}^{+}<\mathrm{Ne}<\mathrm{F}^{-}
\)

Q37. Which one of the following statements is incorrect in relation to ionization enthalpy?
(a) Ionization enthalpy increases for each successive electron.
(b) The greatest increase in ionization enthalpy is experienced on the removal of electrons from the core noble gas configuration.
(c) The end of valence electrons is marked by a big jump in ionization enthalpy.
(d) Removal of the electron from orbitals bearing lower \(n\) value is easier than from orbital having higher \({n}\) value.

Answer: Electrons in orbitals bearing a lower \(\mathrm{n}\) value are more attracted to the nucleus than electrons in orbitals bearing a higher \(\mathrm{n}\) value. Hence, the removal of electrons from orbitals bearing a higher \(n\) value is easier than the removal of electrons from orbitals having a lower \(\mathrm{n}\) value. (d) is incorrect.

Q38. Considering the elements \(\mathrm{B}, \mathrm{Al}, \mathrm{Mg}\), and \(\mathrm{K}\), the correct order of their metallic character is:
(a) \(\mathrm{B}>\mathrm{Al}>\mathrm{Mg}>\mathrm{K}\)
(b) \(\mathrm{Al}>\mathrm{Mg}>\mathrm{B}>\mathrm{K}\)
(c) \(\mathrm{Mg}>\mathrm{Al}>\mathrm{K}>\mathrm{B}\)
(d) \(\mathrm{K}>\mathrm{Mg}>\mathrm{Al}>\mathrm{B}\)

Answer: In a period, metallic character decreases as we move from left to right. Therefore, metallic character of \(\mathrm{K}, \mathrm{Mg}\) and \(\mathrm{Al}\) decreases in the order: \(\mathrm{K}>\mathrm{Mg}>\mathrm{Al}\). However, within a group, the metallic character increases from top to bottom. Thus, Al is more metallic than B. Therefore, the correct sequence of decreasing metallic character is: \(\mathrm{K}>\mathrm{Mg}>\mathrm{Al}>\mathrm{B}\), i.e., option (d) is correct.

Q39. Considering the elements B, C, N, F, and Si, the correct order of their non-metallic character is :
(a) B \(>\) C \(>\) Si \(>\) N \(>\) F
(b) Si \(>\) C \(>\) B \(>\) N \(>\) F
(c) F \(>\) N \(>\) C \(>\) B \(>\) Si
(d) F \(>\) N \(>\) C \(>\) Si \(>\) B

Answer: In a period, the non-metallic character increases from left to right. Thus, among B, C, N and F, nonmetallic character decreases in the order: \(\mathrm{F}>\mathrm{N}>\mathrm{C}>\mathrm{B}\). However, within a group, non-metallic character decreases from top to bottom. Thus, \(\mathrm{C}\) is more non-metallic than \(\mathrm{Si}\). Therefore, the correct sequence of decreasing non-metallic character is: \(\mathrm{F}>\mathrm{N}>\mathrm{C}>\mathrm{B}>\mathrm{Si}\), i.e., option (c) is correct.

Q40. Considering the elements \(\mathrm{F}, \mathrm{Cl}, \mathrm{O}\) and \(\mathrm{N}\), the correct order of their chemical reactivity in terms of oxidizing property is :
\(
\text { (a) } \mathrm{F}>\mathrm{Cl}>\mathrm{O}>\mathrm{N}
\)
\(
\text { (b) } \mathrm{F}>\mathrm{O}>\mathrm{Cl}>\mathrm{N}
\)
\(
\text { (c) } \mathrm{Cl}>\mathrm{F}>\mathrm{O}>\mathrm{N}
\)
\(
\text { (d) } \mathrm{O}>\mathrm{F}>\mathrm{N}>\mathrm{Cl}
\)

Answer: Within a period, the oxidising character increases from left to right. Therefore, among \(\mathrm{F}\), \(\mathrm{O}\) and \(\mathrm{N}\), oxidising power decreases in the order: \(\mathrm{F}>\mathrm{O}>\mathrm{N}\). However, within a group, oxidising power decreases from top to bottom. Thus, \(\mathrm{F}\) is a stronger oxidising agent than \(\mathrm{Cl}\). Further because \(\mathrm{O}\) is more electronegative than \(\mathrm{Cl}\), therefore, \(\mathrm{O}\) is a stronger oxidising agent than \(\mathrm{Cl}\). Thus, the overall decreasing order of oxidising power is: \(\mathrm{F}\) > \(\mathrm{O}>\mathrm{Cl}>\mathrm{N}\), i.e., option (b) is correct.

Exemplar

Q41. Explain why the electron gain enthalpy of fluorine is less negative than that of chlorine.

Answer: In fluorine, the new electron to be added goes to \(2 \mathrm{p}\)-subshell while in chlorine, the added electron goes to \(3 p\)-subshell. Since the \(2 p\)-subshell is relatively small as compared to \(3 p\)-subshell, the added electron in small \(2 p\)-subshell experiences strong interelectronic repulsions in comparison to that in \(3 p\)-subshell in \(\mathrm{Cl}\). As a result, the incoming electron does not feel much attraction from the nucleus and therefore, the electron gain enthalpy of \(\mathrm{F}\) is less negative than that of \(\mathrm{Cl}\).

Q42. All transition elements are \(d\)-block elements, but all \(d\)-block elements are not transition elements. Explain.

Answer: Elements in which the last electron enters in the d-orbitals, are called d-block elements or transition elements. These elements have the general outer electronic configuration \((n-1) d^{1-10} n s^{0-2}. Z n, C d\) and \(\mathrm{Hg}\) having the electronic configuration \((n-1) d^{10} n s^2\) do not show most of the properties of transition elements.

Explanation: All the elements in between s- and p-block, i.e., between group 2 and 13 are called transition elements. Elements in which the last electron enters the d-orbitals of their respective penultimate shells are called \(d\) block elements. According to this definition, \(\mathrm{Zn}, \mathrm{Cd}\) and \(\mathrm{Hg}\) cannot be regarded as d-block elements because the last electron in these elements enters the s-orbital of their outermost shells rather than d-orbital of their penultimate shells. Therefore, these elements should not be regarded as d-block elements. Therefore, to make the study of the periodic classification of elements more rational, these are studied along with d-block elements. Thus, on the basis of properties, all the transition elements are d-block elements but on the basis of electronic configuration, all d-block elements are not transition elements.

Q43. Identify the group and valency of the element having atomic number 119. Also, predict the outermost electronic configuration and write the general formula of its oxide.

Answer: The present setup of the Long form of the periodic table can accommodate maximum 118 elements. Thus, in accordance with the Aufbau principle, the filling of \(8 \mathrm{~s}\)-orbital will occur. In other words, the 119th electron will enter \(8 \mathrm{~s}\)-orbital. As such its outmost electronic configuration will be \(8 \mathrm{~s}^1\). Since, it has only one electron in the valence shell, i.e., 8 s, therefore, its valency will be 1 and it will lie in group 1 along with alkali metals and the formula of its oxide will be \(\mathrm{M}_2 \mathrm{O}\) where \(\mathrm{M}\) represents the element.

Q44. Ionisation enthalpies of elements of the second period are given below:
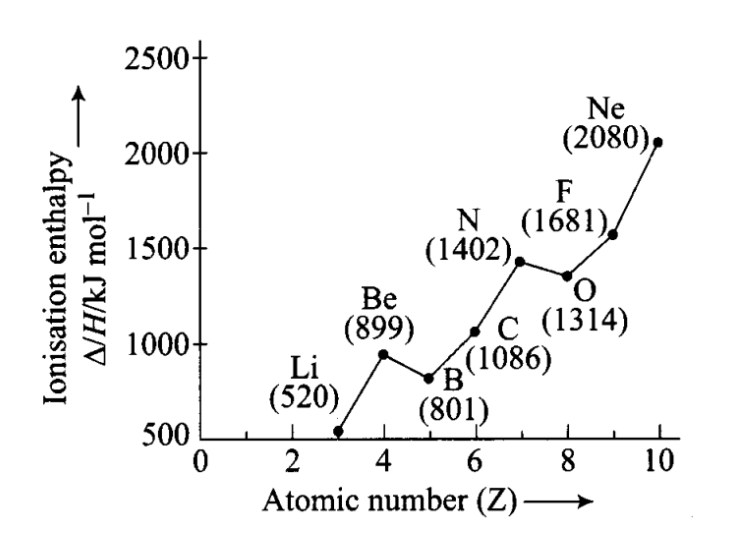
Ionisation enthalpy/ \(\mathrm{k}\) cal mol\({ }^{-1}: 520,899,801,1086,1402,1314,1681,2080\). Match the correct enthalpy with the elements and complete the graph given in Fig. 3.1. Also write symbols of elements with their atomic number.

Answer: To match the correct enthalpy with the elements and to complete the graph the following points are taken into consideration. As we move from left to right across a period, the ionisation enthalpy keeps on increasing due to increased nuclear charge and a simultaneous decrease in atomic radius. However, there are some exceptions given below.
(a) In spite of increased nuclear charge, the first ionisation enthalpy of B is lower than that of Be. This is due to the presence of fully filled \(2 \mathrm{~s}\) orbital of Be \(\left[1 s^2 2 s^2\right]\) which is a stable electromagnetic arrangement. Thus, higher energy is required to knock out the electron from fully filled \(2 \mathrm{~s}\) orbitals. While \(B\) \(\left[1 \mathrm{~s}^2 2 \mathrm{~s}^2 2 p^1\right]\) contains valence electrons in \(2 \mathrm{~s}\) and \(2 \mathrm{p}\) orbitals. It can easily lose its one \(\mathrm{e}^{-}\)from \(2 \mathrm{p}\) orbital in order to achieve noble gas configuration. Thus, the first ionisation enthalpy of \(\mathrm{B}\) is lower than that of \(\mathrm{Be}\).
Since the electrons in \(2 \mathrm{~s}\)-orbital are more tightly held by the nucleus than these present in \(2 \mathrm{p}\)-orbital therefore, ionisation enthalpy of \(\mathrm{B}\) is lower than that of Be.
(b) The first ionisation enthalpy of \(\mathrm{N}\) is higher than that of \(\mathrm{O}\) through the nuclear charge of \(\mathrm{O}\) is higher than that of \(\mathrm{N}\). This is due to the reason that in the case of \(\mathrm{N}\), the electron is to be removed from a more stable exactly half-filled electronic configuration \(\left(1 \mathrm{~s}^2 2 \mathrm{~s}^2 2 \mathrm{p}_{\mathrm{x}}^1 2 \mathrm{p}_{\mathrm{y}}^1 2 \mathrm{p}_{\mathrm{z}}^1\right)\) which is not present in \(\mathrm{O}\) \(\left(1 \mathrm{~s}^2 2 \mathrm{~s}^2 2 \mathrm{p}_{\mathrm{x}}^2 2 \mathrm{p}_{\mathrm{y}}^1 2 \mathrm{p}_{\mathrm{z}}^1\right)\).
Therefore, the first ionisation enthalpy of \(\mathrm{N}\) is higher than that of \(\mathrm{O}\). The symbols of \(\mathrm{d}\)-elements along with their atomic numbers are given in the following graph.

Q45. Among the elements \(\mathrm{B}, \mathrm{Al}\), \(\mathrm{C}\) and \(\mathrm{Si}\),
(i) which element has the highest first ionisation enthalpy?
(ii) which element has the most metallic character?
Justify your answer in each case.

Answer: Arranging the elements into different groups and periods:
\(
\begin{array}{|l|l|l|}
\hline \text { Group } & 13 & 14 \\
\hline \text { Period 2 } & \text { B } & \text { C } \\
\hline \text { Period 3 } & \text { Al } & \text { Si } \\
\hline
\end{array}
\)

• Ionization enthalpy increases along a period and decreases down a group. Therefore, \(\mathrm{C}\) has the highest first ionization enthalpy.
• Metallic character decreases along a period and increases down a group. Therefore, Al has the most metallic character.

Q46. Write four characteristic properties of \(p\)-block elements.

Answer: The four important characteristic properties of \(\mathrm{p}\)-block elements are the following
(a) p-Block elements include both metals and non-metals but the number of non-metals is much higher than that of metals. Further, the metallic character increases from top to bottom within a group and the non-metallic character increases from left to right along a period on this block.
(b) Their ionisation enthalpies are relatively higher as compared to s-block elements.
(c) They mostly form covalent compounds.
(d) Some of them show more than one (variable) oxidation state in their compounds. Their oxidising character increases from left to right in a period and reducing character increases from top to bottom in a group.

Q47. Choose the correct order of atomic radii of fluorine and neon (in pm) out of the options given below and justify your answer.
(i) 72,160
(ii) 160,160
(iii) 72,72
(iv) 160,72

Answer: (i) Atomic radius decreases as we move from left to right in a period in the periodic table. Fluorine has the smallest atomic radius. As we move to neon in the same period, the atomic radius increases as it has van der Waals radius and van der Waals radii are bigger than covalent radii. Therefore, atomic radius of \(\mathrm{F}\) is smaller than atomic radius of \(\mathrm{Ne}(\mathrm{F}=72 \mathrm{pm}, \mathrm{Ne}=160 \mathrm{pm})\).

Q48. Illustrate by taking examples of transition elements and non-transition elements that oxidation states of elements are largely based on electronic configuration.

Answer: The oxidation state of an element depends upon the electrons present in the outermost shell or eight minus the number of valence shell electrons (outermost shell electrons). e.g.,
Alkali metals (Group 1 elements) General valence shell electronic configuration \(-ns^1\); Oxidation state \(=+1\)
Alkaline earth metals (Group 2 elements) General valence shell electronic configuration \(-n s^2\); Oxidation state \(=+2\).
Alkali metals and alkaline earth metals belong to s-block elements and elements of group 13 to group 18 are known as p-block elements.
Group 13 elements General valence shell electronic configuration \(-ns^2 n p^1\); Oxidation states \(=+3\) and +1.
Group 14 elements General valence shell electronic configuration \(-ns^2 n p^2\); Oxidation states \(=+4\) and +2.
Group 15 elements General valence shell electronic configuration \(-n s^2 n p^3\); Oxidation states \(=-3,+3\) and +5. Nitrogen shows \(+1,+2,+4\) oxidation states also.
Group 16 elements General valence shell electronic configuration \(-n s^2 n p^4\); Oxidation states \(=-2,+2,+4\) and +6.
Group 17 elements General valence shell electronic configuration \(-{ns}^2 {np}^5\); Oxidation states \(=-1; \mathrm{Cl}, \mathrm{Br}\) and \(\mathrm{I}\) also shows \(+1,+3,+5\) and +7 oxidation states.
Group 18 elements General valence shell configuration \(-n s^2 n p^6\). Oxidation state=zero.
Transition elements or d-block elements General electronic configuration – \((n-1) d^{1-10} n s^{1-2}\). These elements show variable oxidation states due to the involvement of not only ns electrons but \(d\) or \({f}\)-electrons (inner-transition elements) as well. Their most common oxidation states are +2 and +3.

Alternate: The oxidation state of an element is based on its electronic configuration. The various oxidation states of a transition metal are due to the involvement of \((n-1) d\) and outer \(ns\) electrons in bonding.
For example, \(\mathrm{Ti}\left(22\right.\), electronic configuration \(\left.[\mathrm{Ar}] 3 \mathrm{~d}^2 4 \mathrm{~s}^2\right)\) can show three oxidation states \((+2,+3\) and +4\()\) in various compounds like \(\mathrm{TiO}_2(+4), \mathrm{Ti}_2 \mathrm{O}_3(+3)\) and \(\mathrm{TiO}(+2)\).
The non-transition elements, mainly the p-block elements can show a number of oxidation states from \(+n\) to \((n-8)\) where \(n\) is the number of electrons present in the outermost shell. For example, phosphorus can show \(-3,+3\) and +5 oxidation states.
Lower oxidation states are ionic as the atom accepts the electron or electrons to achieve stable configuration while higher oxidation states are achieved by unpairing the paired electrons and shifting the electrons to vacant d-orbital.

Q49. Nitrogen has positive electron gain enthalpy whereas oxygen has negative. However, oxygen has lower ionisation enthalpy than nitrogen. Explain.

Answer: The outermost electronic configuration of nitrogen is \(\left(2 s^2 2 p_x^1 2 p_y^1 2 p_z^1\right)\). It is stable because it has exactly half-filled \(2 p\)-subshell. Therefore, it has no tendency to accept extra electrons and energy has to be supplied to add additional electrons. Thus, the electron gain enthalpy of nitrogen is slightly positive. On the other hand, the outermost electronic configuration of O is \(\left(2 s^2 2 p_x^2 2 p_y^1 2 p_z^1\right)\). It has higher positive charge \((+8)\) than nitrogen \((+7)\) and lower atomic size than \(\mathrm{N}\). Therefore, it has a tendency to accept an extra electron. Thus, the electron gain enthalpy of \(\mathrm{O}\) is negative. However, oxygen has four electrons in the \(2 p\) subshell and can lose one electron to acquire a stable half-filled configuration and therefore, it has low ionization enthalpy. Because of the stable configuration of \(\mathrm{N}\), it cannot readily lose electron and therefore, its ionization enthalpy is higher than that of \(\mathrm{O}\).

Q50. The first member of each group of representative elements (i.e., \(s\) and \(p\)-block elements) shows anomalous behaviour. Illustrate with two examples.

Answer: The first member of each group of s-and p-block elements shows anomalous behaviour due to the following reasons:
(i) Small size
(ii) High ionization enthalpy
(iii) High electronegativity
(iv) Absence of d-orbitals
Examples: Li in the first group shows different properties from the rest of the elements like the covalent nature of its compounds and, the formation of nitrides.
Similarly, beryllium, the first element of the second group differs from its own group in the following ways:

• Beryllium carbide reacts With water to produce methane gas while carbides of other elements give acetylene.
• Beryllium shows a coordination number of four while other elements show a coordination number of six.
• Compounds of lithium have a significant covalent character. While compounds of other alkali metals are predominantly ionic.
• Lithium reacts with nitrogen to form lithium nitride while other alkali metals do not form nitrides.

In p-block elements, the first member of each group has four orbitals, one 2 s-orbital and three \(2 p\)-orbitals in their valence shell. So, these elements show a maximum covalency of four while other members of the same group or different groups show a maximum covalency beyond four due to the availability of vacant d-orbitals.

Q51. Block elements form acidic, basic and amphoteric oxides. Explain each property by giving two examples and also write the reactions of these oxides with water.

Answer: The oxides of p-block elements show acidic, basic and amphoteric properties, due to the following factors:
1. Ionisation enthalpy: Higher the ionisation enthalpy of an element, the stronger will be the acid formed by that element. If ionisation enthalpy of an element is high, then its oxide will be acidic in nature, if low, then it will be basic in nature, and if intermediate, its oxide will be amphoteric in nature.
2. Electronegativity: The higher the electronegativity of the element, the more acidic is its oxide. For instance, \(\mathrm{N}_2 \mathrm{O}_3\) is more acidic than \(\mathrm{B}_2 \mathrm{O}_3\)
3. Oxidation states: The higher the oxidation state of the elements; the stronger will be its acid. For instance, \(\mathrm{SO}_3\) is a stronger acid than \(\mathrm{SO}_2\)
Reaction with water
\(
\mathrm{B}_2 \mathrm{O}_3+3 \mathrm{H}_2 \mathrm{O} \rightleftharpoons 2 \mathrm{H}_3 \mathrm{BO}_3
\)
Boron Trioxide Orthoboric acid
\(
\mathrm{B}(\mathrm{OH})_3+\mathrm{H}-\mathrm{OH} \rightarrow\left[\mathrm{B}(\mathrm{OH})_4\right]^{-}+\mathrm{H}^{+}
\)
\(\mathrm{Al}_2 \mathrm{O}_3\) is amphoteric in nature. It is insoluble in water but dissolves in alkalies and reacts with acids.
\(
\mathrm{Al}_2 \mathrm{O}_3+2 \mathrm{NaOH} \stackrel{\Delta}{\rightarrow} 2 \mathrm{NaAlO}_2+\mathrm{H}_2 \mathrm{O}
\)
Aluminium trioxide Sodium meta aluminate
\(
\mathrm{Al}_2 \mathrm{O}_3+6 \mathrm{HCl} \stackrel{\Delta}{\rightarrow} 2 \mathrm{AlCl}_3+3 \mathrm{H}_2 \mathrm{O}
\)
Aluminium Chloride
\(\mathrm{Ti}_2 \mathrm{O}\) is as basic as \(\mathrm{NaOH}\) due to its lower oxidation state (+1).
\(\mathrm{Ti}_2 \mathrm{O}+2 \mathrm{HCl} \rightarrow 2 \mathrm{TiCl}+\mathrm{H}_2 \mathrm{O}\)

Q52. How would you explain the fact that the first ionisation enthalpy of sodium is lower than that of magnesium but its second ionisation enthalpy is higher than that of magnesium?

Answer: Electronic configuration of \(\mathrm{Na}\) is \(1 s^2 2 s^2 2 \mathrm{p}^6 3 \mathrm{~s}^1\). After losing one electron from its outermost shell, sodium easily attains stable electronic configuration \(\left(1 s^2 2 s^2 2 p^6\right)\), while magnesium does not lose its electron easily due to the presence of two electrons in s-orbital \(\left( 1 s^2 2 s^2 2 p^6 3 s^2\right)\). Hence first ionisation energy of sodium is less than magnesium.
When one electron is removed from \(\mathrm{Na}\) and \(\mathrm{Mg}\), their configurations become \(1 s^2 2 s^2 2 p^6\) and \(1 s^2 2 s^2 2 p^6 3 s^1\) respectively. Now it is easier to remove one electron from \(3 s\) of \(\mathrm{Mg}^{+}\)than \(2 \mathrm{p}^6\) of \(\mathrm{Na}^{+}\). Hence, second ionisation energy of \(\mathrm{Mg}\) is less than \(\mathrm{Na}\).

Q53. What do you understand by exothermic reaction and endothermic reaction? Give one example of each type.

Answer: Exothermic reaction: The reaction that is accompanied with the evolution of heat is known as exothermic reaction.
\(
\mathrm{CaO}+\mathrm{CO}_2 \rightarrow \mathrm{CaCO}_3, \Delta \mathrm{H}=-178.3 \mathrm{~kJ} \mathrm{~mol}^{-1}
\)
Endothermic reaction: The reaction that is accompanied with the absorption of energy is known as endothermic reaction, e.g.,
\(
2 \mathrm{NH}_3 \rightarrow \mathrm{N}_2+3 \mathrm{H}_2 ; \Delta \mathrm{H}=+91.8 \mathrm{~kJ} \mathrm{~mol}^{-1}
\)

Q54. Arrange the elements \(\mathrm{N}, \mathrm{P}\), \(\mathrm{O}\) and \(\mathrm{S}\) in the order of-
(i) increasing first ionisation enthalpy.
(ii) increasing non-metallic character.
Give reason for the arrangement assigned.

Answer: (i) \(S<P<O<N\)
Ionisation enthalpy increases from left to right in a period and decreases down the group. \(N\) has higher ionisation enthalpy than O due to the extra stability of half-filled orbitals. Similarly, \(\mathrm{P}\) has higher ionisation enthalpy than \(S\) due to half-filled orbitals.
(ii) \(\mathrm{P}<\mathrm{S}<\mathrm{N}<\mathrm{O}\)
Non-metallic character decreases down the group and increases from left to right in a period.

Q55. Explain the deviation in ionisation enthalpy of some elements from the general trend by using Figure 3.2.

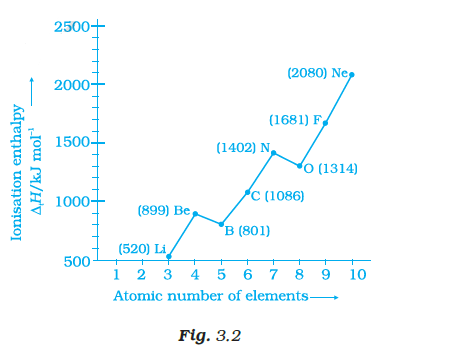
Answer: Ionisation enthalpy increases in a period with the increase in atomic number. The graph shows few exceptions and not the linear relationship. Ionisation enthalpy of \(\mathrm{Be}\) is greater than that of \(\mathrm{B}\) due to filled s-orbital in \(\mathrm{Be}\left(\mathrm{Be}-1\mathrm{s}^2 2 \mathrm{~s}^2; \mathrm{~B}-1 \mathrm{~s}^2 2 \mathrm{~s}^2 2 \mathrm{p}^1\right)\).
Ionisation enthalpy of \(\mathrm{N}\) is greater than that of \(\mathrm{O}\) due to half-filled p-orbitals in nitrogen. \((N-1 s^2 2 s^2 2 p^3\); \(\text { and } O=1 s^2 2 s^2 2 p^4\)

Q56. Explain the following:
(a) Electronegativity of elements increase on moving from left to right in the periodic table.
(b) Ionisation enthalpy decrease in a group from top to bottom?

Answer: (a) The electronegativity generally increases on moving across a period from left to right (e.g., from Li to F in the second period). This is due to a decrease in atomic size and an increase in effective nuclear charge. As a result of an increase in effective nuclear charge, the attraction for the outer electron and the nucleus increases in a period and therefore, electronegativity also increases e.g., the electronegativity of the elements of the 2 nd period increases regularly from left to right as follows \(\mathrm{Li}(1.0), \mathrm{Be}(1.5), \mathrm{B}(2.0), \mathrm{C}(2.5), \mathrm{N}(3.0), \mathrm{O}(3.5)\) (Decrease in size of atom and increase in nuclear charge).
(b) On moving down a group there is a gradual decrease in ionisation enthalpy. The decrease in ionisation enthalpy down a group can be explained in terms of the net effect of the following factors (Increase in atomic size.):
(i) In going from top to bottom in a group, the nuclear charge increases. On moving down a group from top to bottom, the atomic size increases gradually due to the addition of a new principal energy shell at each succeeding element. As a result, the distance between the nucleus and the valence shell increases.
In other words, the force of attraction of the nucleus for the valence electrons decreases and hence the ionisation enthalpy should decrease.
(ii) There is a gradual increase in atomic size due to an additional main energy shell ( \(n\) ). With the addition of new shells, the number of inner shell which shield the valence electrons from the nucleus increases. In other words, the shielding effect or the screening effect increases. As a result, the force of attraction of the nucleus for the valence electrons further decreases and hence the ionisation enthalpy should decrease.
(iii) There is an increase in the shielding effect on the outermost electron due to an increase in the number of inner electrons. Further, in a group from top to bottom nuclear charge increases with an increase in atomic number. As a result, the force of attraction of the nucleus for the valence electrons increases and hence the ionisation enthalpy should increase.
The effect of an increase in atomic size and the shielding effect is much more than the effect of an increase in nuclear charge. As a result, the electron becomes less and less firmly held to the nucleus as we move down the group. Hence, there is a gradual decrease in the ionization enthalpies in a group.

Q57. How does the metallic and non-metallic character vary on moving from left to right in a period?

Answer: As we move from left to right in a period, the number of valence electrons increases by one at each succeeding element but the number of shells remains the same. Due to this, the effective nuclear charge increases. More is the effective nuclear charge, more is the attraction between the nucleus and electron. Hence, the tendency of the element to lose electrons decreases. This results in decrease in metallic character. Furthermore, the tendency of an element to gain electrons increases with an increase in effective nuclear charge, so non-metallic character increases on moving from left to right in a period.

Alternate: Metallic character decreases and non-metallic character increases in moving from left to right in a period. It is due to increase in ionisation enthalpy and electron gain enthalpy.

Q58. The radius of \(\mathrm{Na}^{+}\)cation is less than that of \(\mathrm{Na}\) atom. Give reason.

Answer: When an atom loses an electron to form cation, its radius decreases. In a cation, per electron nuclear forces increase due to a decrease in the number of electrons. As a result of this, the effective nuclear charge increases and the radius of cation decreases. e.g., ionic radius of \(\mathrm{Na}^{+}\)is smaller than the radius of its parent atom \(\mathrm{Na}\).
\(
\mathrm{Na} \rightarrow \mathrm{Na}^{+}+1 \mathrm{e}^{-}
\)

The radius of \(\mathrm{Na}^{+}\)is less than \(\mathrm{Na}\) atom because \(\mathrm{Na}^{+}\)is formed by losing one energy shell (decrease of one shell).
\(
\begin{aligned}
& \mathrm{Na}-1 s^2 2 s^2 2 p^6 3 s^1 \\
& \mathrm{Na}^{+}-1 s^2 2 s^2 2 p^6
\end{aligned}
\)

Q59. Among alkali metals which element do you expect to be least electronegative and why?

Answer: Electronegativity decreases in a group from top to bottom. Thus, caesium is the least electronegative element.

Q60. Discuss the factors affecting electron gain enthalpy and the trend in its variation in the periodic table.

Answer: Factors affecting electron gain enthalpy:
(i) Nuclear charge: The electron gain enthalpy become more negative as the nuclear charge increases. This is due to the greater attraction for the incoming electron if the nuclear charge is high.
(ii) Size of the atom: With the increase in size of the atom, the distance between the nucleus and the incoming electron increases and this results in lesser attraction. Consequently, the electron gain enthalpy become less negative with an increase in size of the atom of the element.
(iii) Electronic configuration: The elements having stable electronic configurations of half-filled and completely filled valence subshells show a very small tendency to accept additional electrons and thus electron gain enthalpies are less negative.
Variation of electron gain enthalpies in the periodic table:
Electron gain enthalpy, in general, becomes more negative from left to right in a period and becomes less negative as we go from top to bottom in a group.
(a) Variation down a group: On moving down a group, the size and nuclear charge increases. But the effect of increase in atomic size is much more pronounced than that of nuclear charge and thus the additional electron feels less attraction by the large atom. Consequently, electron gain enthalpy becomes less negative. This is clear from decrease of electron gain enthalpy in going from chlorine to bromine and to iodine.
(b) Variation along a period: On moving across a period, the size of the atom decreases and nuclear charge increases. Both these factors result in greater attraction for the incoming electron, therefore, electron gain enthalpy, in general, becomes more negative in a period from left to right. However, certain irregularities are observed in the general trend. These are mainly due to the stable electronic configurations of certain atoms. Important Trends in Electron Gain Enthalpies:
There are some important features of electron gain enthalpies of elements. These are:
(i) Halogens have the highest negative electron gain enthalpies.
(ii) Electron gain enthalpy values of noble gases are positive while those of \(\mathrm{Be}, \mathrm{Mg}, \mathrm{N}\) and \(\mathrm{P}\) are almost zero.
(iii) Electron gain enthalpy of fluorine is unexpectedly less negative than that of chlorine.

Q61. Define ionisation enthalpy. Discuss the factors affecting ionisation enthalpy of the elements and its trends in the periodic table.

Answer: Ionisation enthalpy: It is defined as the minimum amount of energy required to remove the most loosely bound electron from an isolated gaseous atom.
\(
M(g) \rightarrow M^{+}(g)+1 e^{-}(I . E .)_1
\)
\(
M^{+}(g) \rightarrow M^{+2}(g)+1 e^{-}(I . E .)_2
\)
Factors on which Ionisation Enthalpy Depends:
(i) Size of the atom: The larger the atomic size, the smaller is the value of ionisation enthalpy. In a larger atom, the outer electrons are far away from the nucleus and thus force of attraction with which they are attracted by the nucleus is less and hence can be easily removed.
Ionisation enthalpy \(\propto 1 /\) Atomic size
(ii) Screening effect: The higher the screening effect, the lesser is the value of ionisation enthalpy as the screening effect reduces the force of attraction towards the nucleus and hence the outer electrons can be easily removed.
Ionisation enthalpy \(\propto 1 /\) Screening effect
(iii) Nuclear charge: As the nuclear charge increases among atoms having the same number of energy shells, the ionisation enthalpy increases because the force of attraction towards the nucleus increases.
Ionisation enthalpy \(\propto\) Nuclear charge
(iv) Half-filled and fully filled orbitals: The atoms having half-filled and fully filled orbitals are comparatively more stable, hence more energy is required to remove the electron from such atoms. The ionization enthalpy is rather higher than the expected value in the case of such an atom.
Ionisation enthalpy \(\propto\) Stable electronic configuration
(v) Shape of orbital: The s-orbital is more close to the nucleus than the p-orbital of the same orbit. Thus, it is easier to remove the electron from a p-orbital in comparison to s-orbital. In general, the ionisation enthalpy follows the following order
\((s>p>d>f)\) orbitals of the same orbit.
Variation of ionisation enthalpy in the periodic table
In general, the ionisation energy decreases down the group due to increase in atomic size. On the other hand, the ionisation energy increases across the period from left to right, again due to decrease in atomic size from left to right.

Q62. Justify the given statement with suitable examples- “the Properties of the elements are a periodic function of their atomic numbers”.

Answer: The similarities in the properties arise due to the same distribution of electrons in the outermost orbitals or electronic configuration which depends upon the atomic number. The elements present in a group or period exhibit similar chemical properties which depend upon the atomic number.

Q63. Write down the outermost electronic configuration of alkali metals. How will you justify their placement in group 1 of the periodic table?

Answer: There are numerous physical properties of elements such as melting points, boiling points, heats of fusion and vaporisation, energy of atomisation, etc. which show periodic variations.
The cause of periodicity in properties is the repetition of similar outer electronic configurations after certain regular intervals. e.g., all the elements of 1st group (alkali metals) have similar outer electronic configuration, i.e., ns \({ }^1\).
\(
\begin{aligned}
& { }_3 \mathrm{Li}=1 \mathrm{~s}^2, 2 \mathrm{~s}^1 \\
& { }_{11} \mathrm{Na}=1 \mathrm{~s}^2, 2 \mathrm{~s}^2, 2 \mathrm{p}^6, 3 \mathrm{~s}^1 \\
& { }_{19} \mathrm{~K}=1 \mathrm{~s}^2, 2 \mathrm{~s}^2, 2 \mathrm{p}^6, 3 \mathrm{~s}^2, 3 \mathrm{p}^6, 4 \mathrm{~s}^1 \\
& { }_{37} \mathrm{Rb}=1 \mathrm{~s}^2, 2 \mathrm{~s}^2, 2 \mathrm{p}^6, 3 \mathrm{~s}^2, 3 \mathrm{p}^6, 3 \mathrm{~d}^{10}, 4 \mathrm{~s}^2, 4 \mathrm{p}^6, 5 \mathrm{~s}^1 \\
& { }_5^{55} \mathrm{Cs}=1 \mathrm{~s}^2, 2 \mathrm{~s}^2, 2 \mathrm{p}^6, 3 \mathrm{~s}^2, 3 \mathrm{p}^6, 3 \mathrm{~d}^{10}, 4 \mathrm{~s}^2, 4 \mathrm{p}^6, 4 \mathrm{~d}^{10}, 5 \mathrm{~s}^2, 5 \mathrm{p}^6, 6 \mathrm{~s}^1 \\
& { }_{87} \mathrm{Fr}=1 \mathrm{~s}^2, 2 \mathrm{~s}^2, 2 \mathrm{p}^6, 3 \mathrm{~s}^2, 3 \mathrm{p}^6, 3 \mathrm{~d}^{10}, 4 \mathrm{~s}^2, 4 \mathrm{p}^6, 4 \mathrm{~d}^{10}, 4 \mathrm{f}^{14}, 5 \mathrm{~s}^2, 5 \mathrm{p}^6, 5 \mathrm{~d}^{10}, 6 \mathrm{~s}^2, 6 \mathrm{p}^6, 7 \mathrm{~s}^1
\end{aligned}
\)
Therefore, due to similar outermost shell electronic configuration, all alkali metals have similar properties. e.g., sodium and potassium both are soft and reactive metals. They all form basic oxides and their basic character increases down the group. They all form unipositive ion by the loss of one electron. Similarly, all the elements of 17 th group (halogens) have similar outermost shell electronic configurations, i.e.,  \({ns}^2 {~np}^5\) and thus possess similar properties.
\(
\begin{aligned}
& { }_9 \mathrm{~F}=1 \mathrm{~s}^2, 2 \mathrm{~s}^2, 2 \mathrm{p}^5 \\
& { }_{17} \mathrm{Cl}=1 \mathrm{~s}^2, 2 \mathrm{~s}^2, 2 \mathrm{p}^6, 3 \mathrm{~s}^2, 3 \mathrm{p}^5 \\
& { }_{35} \mathrm{Br}=1 \mathrm{~s}^2, 2 \mathrm{~s}^2, 2 \mathrm{p}^6, 3 \mathrm{~s}^2, 3 \mathrm{p}^6, 3 \mathrm{~d}^{10}, 4 \mathrm{~s}^2, 4 \mathrm{p}^5 \\
& { }_{35} \mathrm{I}=1 \mathrm{~s}^2, 2 \mathrm{~s}^2, 2 \mathrm{p}^6, 3 \mathrm{~s}^2, 3 \mathrm{p}^6, 3 \mathrm{~d}^{10}, 4 \mathrm{~s}^2, 4 \mathrm{p}^6, 4 \mathrm{~d}^{10}, 5 \mathrm{~s}^2, 5 \mathrm{p}^5 \\
& { }_{85} \mathrm{At}=1 \mathrm{~s}^2, 2 \mathrm{~s}^2, 2 \mathrm{p}^6, 3 \mathrm{~s}^2, 3 \mathrm{p}^6, 3 \mathrm{~d}^{10}, 4 \mathrm{~s}^2, 4 \mathrm{p}^6, 4 \mathrm{~d}^{10}, 4 \mathrm{f}^{14}, 5 \mathrm{~s}^2, 5 \mathrm{p}^6, 5 \mathrm{~d}^{10}, 6 \mathrm{~s}^2, 6 \mathrm{p}^5
\end{aligned}
\)

Q64.  Write the drawbacks in Mendeleev’s periodic table that led to its modification.

Answer: Drawbacks of Mendeleev’s periodic table:
1. Position of hydrogen: Hydrogen is placed in group I. However, it resembles the elements of group I (alkali metals) as well as the elements of group VILA, (halogens). Therefore, the position of hydrogen in the periodic table is not correctly defined.
2. Anomalous pairs: In certain pairs of elements, the increasing order of atomic masses was not obeyed. In these cases, Mendeleev placed elements according to similarities in their properties and not in increasing order of their atomic masses. For example, argon (Ar, atomic mass 39.9) is placed before potassium ( \(K\), atomic mass 39.1). Similarly, cobalt ( \(\mathrm{Co}\), atomic mass 58.9 ) is placed before nickel ( \(\mathrm{Ni}\), atomic mass 58.6 ) and tellurium ( \(\mathrm{Te}\), atomic mass 127.6) is placed before iodine (\(\mathrm{I}\), atomic mass 126.9). These positions were not justified.
3. Position of isotopes: Isotopes are the atoms of the same element having different atomic masses but the same atomic number. Therefore, according to Mendeleev’s classification, these should be placed at different places depending on their atomic masses. For example, isotopes of hydrogen with atomic masses 1,2 and 3 should be placed in three places. However, isotopes have not been given separate places in the periodic table.
4. Some similar elements are separated and dissimilar elements are grouped together: In Mendeleev’s periodic table, some similar elements were placed in different groups while some dissimilar elements had been grouped together. For example, copper and mercury resembled in their properties but they had been placed in different groups. At the same time, elements of group IA such as \(\mathrm{Li}, \mathrm{Na}\) and \(\mathrm{K}\) were grouped with copper \((\mathrm{Cu})\), silver \((\mathrm{Ag})\) and gold \((\mathrm{Au})\), though their properties are quite different.
5. Cause of periodicity: Mendeleev did not explain the cause of periodicity among the elements.
6. Position of lanthanoids (or lanthanides) and actinoids (or actinides):
The fourteen elements following lanthanum (known as lanthanoids, from atomic number 58-71) and the fourteen elements following actinium (known as actinoids, from atomic number \(90-103\) ) have not been given separate places in Mendeleev’s table.
In order to cover more elements, Mendeleev modified his periodic table.

Q65. In what manner is the long form of the periodic table better than Mendeleev’s periodic table? Explain with examples.

Answer: Superiority of the Long form of the Table over Mendeleev’s Table:
(i) This table is based on a more fundamental property, i.e., atomic number.
(ii) It correlates the position of the elements with their electronic configurations more clearly.
(iii) The completion of each period is more logical. In a period as the atomic number increases, the energy shells are gradually filled up until an inert gas configuration is reached. It eliminates the even and odd series of IV, V, VI and VII periods of Mendeleev’s periodic table.
(iv) The position of VIII th group is appropriate in this table. All the transition elements have been brought in the middle as the properties of transition elements are intermediate between s-block and p-block elements.
(v) Due to the separation of two subgroups, dissimilar elements do not fall together. One vertical column accommodates elements with the same electronic configuration thereby showing the same properties.
(vi) In this table, a complete separation between metals and non-metals has been achieved. The non-metals are present in the upper right corner of the periodic table.
(vii) There is a gradual change in properties of the elements with the increase in their atomic numbers, i.e., the periodicity of properties can be easily visualized. The same properties of recurrence in properties occur after the intervals of \(2,8,8,18,18\) and 32 elements which indicates the capacity of various periods of the table.
(viii) This arrangement of elements is easy to remember and reproduce.

Q66. Discuss and compare the trend in ionisation enthalpy of the elements of group 1 with those of group 17 elements.

Answer: The ionization enthalpies decrease regularly as we move down a group from one element to the other. This is evident from the values of the first ionisation enthalpies of the elements of group 1 (alkali metals) and group 17 (halogens) elements as given in the table and figure.
\(
\begin{array}{|c|c|c|c|}
\hline \text { Group } 1 & \text { First ionisation enthalpies }\left(\mathrm{kJ} \mathrm{moL}^{-1}\right) & \begin{array}{l}
\text { Group 17} \\
\end{array} & \text { First ionisation enthalpies }\left(\mathrm{kJ} \mathrm{mol}^{-1}\right) \\
\hline \mathrm{H} & 1312 & F & 1681 \\
\hline \mathrm{Li} & 520 & \mathrm{Cl} & 1255 \\
\hline \mathrm{Na} & 496 & \mathrm{Br} & 1142 \\
\hline \text { K } & 419 & 1 & 1009 \\
\hline \mathrm{Rb} & 403 & \text { At } & 917 \\
\hline \mathrm{Cs} & 374 & & \\
\hline
\end{array}
\)

The given trend can be easily explained on the basis of increasing atomic size and screening effect as follows

• On moving down the group, the atomic size increases gradually due to the addition of one new principal energy shell at each succeeding element. Hence, the distance of the valence electrons from the nucleus increases. Consequently, the force of attraction by the nucleus for the valence electrons decreases and hence the ionisation enthalpy should decrease.
• With the addition of new shells, the shielding or the screening effect increases. As a result, the force of attraction of the nucleus for the valence electrons further decreases and hence the ionisation enthalpy should decrease.
• Nuclear charge increases with an increase in atomic number. As a result, the force of attraction by the nucleus for the valence electrons should increase and accordingly, the ionisation enthalpy should increase. The combined effect of the increase in the atomic size and the screening effect more than compensates for the effect of the increased nuclear charges. Consequently, the valence electrons become less and less firmly held by the nuclear and hence the ionisation enthalpies gradually decrease as move down the group.