3.7 Periodic Trends in Properties of Elements

There are many observable patterns in the physical and chemical properties of elements as we descend in a group or move across a period in the Periodic Table.

For example, within a period, chemical reactivity tends to be high in Group 1 metals, lower in elements towards the middle of the table and increases to a maximum in the Group 17 non-metals. Likewise within a group of representative metals (say alkali metals) reactivity increases on moving down the group, whereas within a group of non-metals (say halogens), reactivity decreases down the group. To explain this trend and periodicity we must look into the theories of atomic structure and properties of the atom. In this section, we shall discuss the periodic trends in certain physical and chemical properties and try to explain them in terms of number of electrons and energy levels.

Trends in Physical Properties
There are numerous physical properties of elements such as melting and boiling points, heat of fusion and vaporization, energy of atomization, etc. which show periodic variations. However, we shall discuss the periodic trends with respect to atomic and ionic radii, ionization enthalpy, electron gain enthalpy and electronegativity.

(a) Atomic Radius

• Firstly, because the size of an atom \(\left(\sim 1.2 Å\right.\) i.e., \(1.2 \times 10^{-10} \mathrm{~m}\) in radius \()\) is very small. Secondly, since the electron cloud surrounding the atom does not have a sharp boundary, the determination of the atomic size cannot be precise. In other words, there is no practical way by which the size of an individual atom can be measured.
• It is defined as half the distance between the nuclei of two bonded atoms. It refers to both covalent and metallic radius depending on whether the element is metal or non-metal.
• One practical approach to estimate the size of an atom of a non-metallic element is to measure the distance between two atoms when they are bound together by a single bond in a covalent molecule and from this value, the “Covalent Radius” of the element can be calculated. For example, the bond distance in the chlorine molecule \(\left(\mathrm{Cl}_2\right)\) is \(198 \mathrm{pm}\) and half this distance \((99 \mathrm{pm})\), is taken as the atomic radius of chlorine.
• For metals, we define the term “Metallic Radius” which is taken as half the internuclear distance separating the metal cores in the metallic crystal. For example, the distance between two adjacent copper atoms in solid copper is \(256 \mathrm{pm}\); hence the metallic radius of copper is assigned a value of \(128 \mathrm{pm}\). For simplicity, in this book, we use the term Atomic Radius to refer to both covalent or metallic radius depending on whether the element is a non-metal or a metal.
• Atomic radii can be measured by \(X\)-ray or other spectroscopic methods.
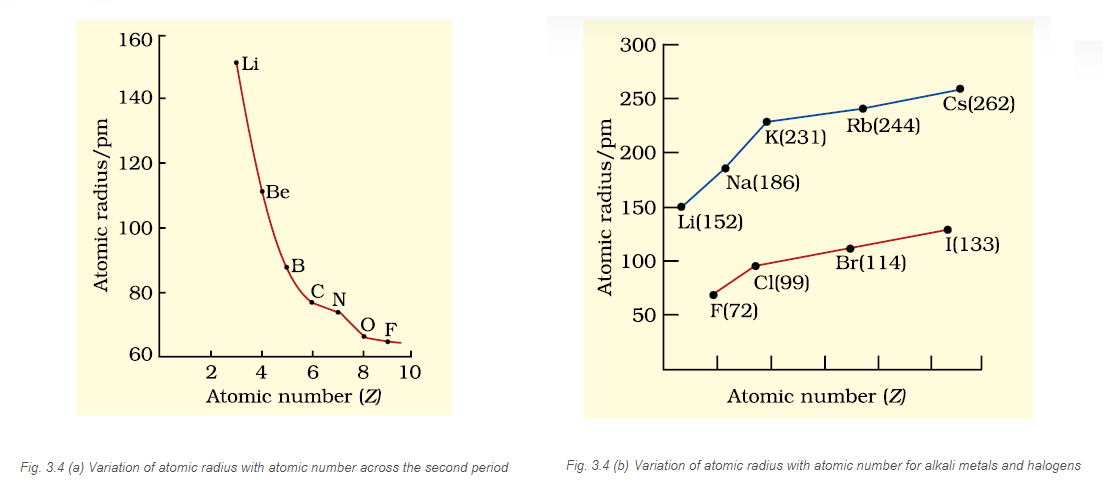
• The atomic radii of a few elements are listed in Table 3.6. Two trends are obvious. The atomic size generally decreases across a period as illustrated in Fig. 3.4(a) for the elements of the second period. It is because within the period the outer electrons are in the same valence shell and the effective nuclear charge increases as the atomic number increases resulting in the increased attraction of electrons to the nucleus.
• Within a family or vertical column of the periodic table, the atomic radius increases regularly with atomic number as illustrated in Fig. 3.4(b). For alkali metals and halogens, as we descend the groups, the principal quantum number ( \(n\) ) increases and the valence electrons are farther from the nucleus. This happens because the inner energy levels are filled with electrons, which serve to shield the outer electrons from the pull of the nucleus. Consequently, the size of the atom increases as reflected in the atomic radii.
• Note that the atomic radii of noble gases are not considered here. Being monoatomic, their (non-bonded radii) values are very large. In fact, radii of noble gases should be compared not with the covalent radii but with the van der Waals radii of other elements.

(b) Ionic Radius

• The removal of an electron from an atom results in the formation of a cation, whereas the gain of an electron leads to an anion.
• The ionic radii can be estimated by measuring the distances between cations and anions in ionic crystals.
• In general, the ionic radii of elements exhibit the same trend as the atomic radii.
• A cation is smaller than its parent atom because it has fewer electrons while its nuclear charge remains the same.
• The size of an anion will be larger than that of the parent atom because the addition of one or more electrons would result in increased repulsion among the electrons and a decrease in effective nuclear charge. For example, the ionic radius of fluoride ion \(\left(\mathrm{F}^{-}\right)\)is \(136 \mathrm{pm}\) whereas the atomic radius of fluorine is only \(64 \mathrm{pm}\). On the other hand, the atomic radius of sodium is \(186 \mathrm{pm}\) compared to the ionic radius of \(95 \mathrm{pm}\) for \(\mathrm{Na}^{+}\).
• When we find some atoms and ions that contain the same number of electrons, we call them isoelectronic species (Two or more species with same number of atoms, same number of valence electrons and same structure, regardless of the nature of elements involved.). For example, \(\mathrm{O}^{2-}, \mathrm{F}^{-}, \mathrm{Na}^{+}\)and \(\mathrm{Mg}^{2+}\) have the same number of electrons (10). Their radii would be different because of their different nuclear charges.
• The cation with the greater positive charge will have a smaller radius because of the greater attraction of the electrons to the nucleus.
• Anion with the greater negative charge will have the larger radius. In this case, the net repulsion of the electrons will outweigh the nuclear charge and the ion will expand in size.

Example 3.5: Which of the following species will have the largest and the smallest size?
\(\mathrm{Mg}, \mathrm{Mg}^{2+}, \mathrm{Al}, \mathrm{Al}^{3+}\).

Answer: Atomic radii decrease across a period. Cations are smaller than their parent atoms. Among isoelectronic species, the one with the larger positive nuclear charge will have a smaller radius.

Hence the largest species is Mg; the smallest one is \(\mathrm{Al}^{3+}\).

(c) Ionization Enthalpy (IE)

A quantitative measure of the tendency of an element to lose electron is given by its Ionization Enthalpy. It represents the energy required to remove an electron from an isolated gaseous atom \((\mathrm{X})\) in its ground state. In other words, the first ionization enthalpy for an element \(\mathrm{X}\) is the enthalpy change \(\left(\Delta_t H\right)\) for the reaction depicted in equation 3.1.
\(
\mathrm{X}(\mathrm{g}) \rightarrow \mathrm{X}^{+}(\mathrm{g})+\mathrm{e}^{-} \dots(3.1)
\)
The ionization enthalpy is expressed in units of \(\mathrm{kJ} \mathrm{mol}^{-1}\). We can define the second ionization enthalpy as the energy required to remove the second most loosely bound electron; it is the energy required to carry out the reaction shown in equation 3.2.
\(
\mathrm{X}^{+}(\mathrm{g}) \rightarrow \mathrm{X}^{2+}(\mathrm{g})+\mathrm{e}^{-} \dots(3.2)
\)

• Energy is always required to remove electrons from an atom and hence ionization enthalpies are always positive. The second ionization enthalpy will be higher than the first ionization enthalpy because it is more difficult to remove an electron from a positively charged ion than from a neutral atom. In the same way, the third ionization enthalpy will be higher than the second and so on. The term “ionization enthalpy”, if not qualified, is taken as the first ionization enthalpy.
• \(\mathrm{IE}_3>\mathrm{IE}_2>\mathrm{IE}_1\) This is because it is more difficult to remove an electron from a positively charged species than from a neutral atom. Down the group, atomic size increases and IE decreases. While across a period, atomic size decreases and IE increases.

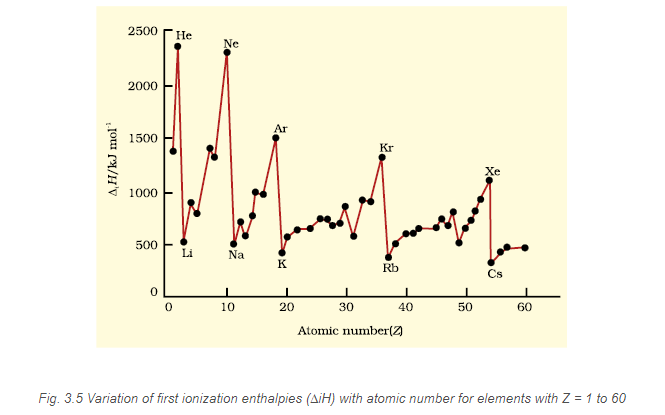
The first ionization enthalpies of elements having atomic numbers up to 60 are plotted in Fig. 3.5. The periodicity of the graph is quite striking. You will find maxima at the noble gases which have closed electron shells and very stable electron configurations. On the other hand, minima occur at the alkali metals and their low ionization enthalpies can be correlated with their high reactivity.

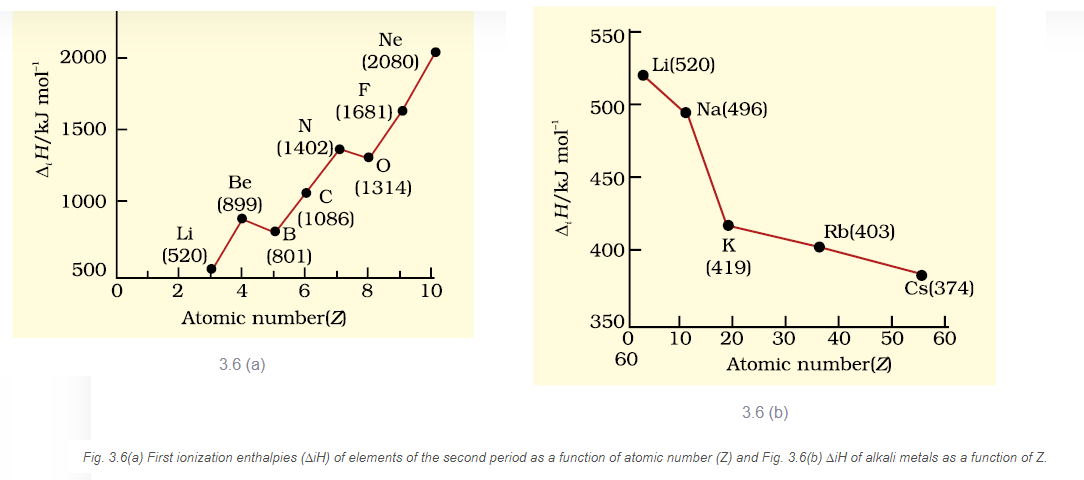
In addition, you will notice two trends the first ionization enthalpy generally increases as we go across a period and decreases as we descend in a group. These trends are illustrated in Figs. 3.6(a) and 3.6(b) respectively for the elements of the second period and the first group of the periodic table.

• The ionization enthalpy and atomic radius are closely related properties. To understand these trends, we have to consider two factors : (i) the attraction of electrons towards the nucleus, and (ii) the repulsion of electrons from each other. The effective nuclear charge experienced by a valence electron in an atom will be less than the actual charge on the nucleus because of “shielding” or “screening” of the valence electron from the nucleus by the intervening core electrons. For example, the 2 s electron in lithium is shielded from the nucleus by the inner core of \(1 s\) electrons. As a result, the valence electron experiences a net positive charge which is less than the actual charge of +3. In general, shielding is effective when the orbitals in the inner shells are completely filled. This situation occurs in the case of alkali metals which have single outermost ns-electron preceded by a noble gas electronic configuration.
• When we move from lithium to fluorine across the second period, successive electrons are added to orbitals in the same principal quantum level and the shielding of the nuclear charge by the inner core of electrons does not increase very much to compensate for the increased attraction of the electron to the nucleus. Thus, across a period, increasing nuclear charge outweighs the shielding. Consequently, the outermost electrons are held more and more tightly and the ionization enthalpy increases across a period.
• As we go down a group, the outermost electron being increasingly farther from the nucleus, there is an increased shielding of the nuclear charge by the electrons in the inner levels. In this case, the increase in shielding outweighs the increasing nuclear charge and the removal of the outermost electron requires less energy down a group.
• From Fig. 3.6(a), you will also notice that the first ionization enthalpy of boron \((Z=5)\) is slightly less than that of beryllium \((Z=4)\) even though the former has a greater nuclear charge. When we consider the same principal quantum level, an s-electron is attracted to the nucleus more than a p-electron.
• In beryllium, the electron removed during the ionization is an \(s\)-electron whereas the electron removed during ionization of boron is a \(p\)-electron. The penetration of a \(2 s\)-electron to the nucleus is more than that of a \(2 p\)-electron; hence the \(2 p\) electron of boron is more shielded from the nucleus by the inner core of electrons than the 2 s electrons of beryllium. Therefore, it is easier to remove the \(2 p\)-electron from boron compared to the removal of a \(2 \mathrm{~s}\) – electron from beryllium. Thus, boron has a smaller first ionization enthalpy than beryllium.
• Another “anomaly” is the smaller first ionization enthalpy of oxygen compared to nitrogen. This arises because in the nitrogen atom, three \(2 p\)-electrons reside in different atomic orbitals (Hund’s rule) whereas in the oxygen atom, two of the four \(2 p\)-electrons must occupy the same \(2 p\)-orbital resulting in an increased electron-electron repulsion. Consequently, it is easier to remove the fourth \(2 p\)-electron from oxygen than it is, to remove one of the three \(2 p\)-electrons from nitrogen.

Factors governing the Ionization energy

• Nuclear charge: IE increases with increases in nuclear charge.
• Atomic size: IE decreases as the atomic radius decreases.
• Penetrating effect of \(\mathrm{e}^{-1} \mathrm{~s}\) : IE increases as penetration effect of \(\mathrm{e}^{-1} \mathrm{~s}\) increases. Within the same shell, the penetration effect decreases in the order : \(s>p>d>f\). Thus, IE to knock out \(s-\mathrm{e}^{-}\)will be higher than \(p-\mathrm{e}^{-}\)of the same shell.
• Shielding or screening effect of inner shell \(\mathrm{e}^{-1} \mathrm{~s}\): As shielding or screening effect of inner \(\mathrm{e}^{-1} \mathrm{~s}\). increases, IE decreases.
• Effect of exactly half-filled or completely filled orbitals: The more stable the electronic configuration, the greater is the IE. This is because of extra stability associated with exactly half-filled or completely filled orbitals due to which more energy is required to remove the \(\mathrm{e}^{-}\). This is the reason why IE of \(\mathrm{N}\) is more than that of \(\mathrm{O}\).
• Noble gases, being stable with completely filled orbital have the highest IE in their respective periods.

Example 3.6: The first ionization enthalpy \(\left(\Delta_t H\right)\) values of the third-period elements, \(\mathrm{Na}, \mathrm{Mg}\) and Si are respectively 496, 737 and \(786 \mathrm{~kJ}\) \(\mathrm{mol}^{-1}\). Predict whether the first \(\Delta_t H\) value for \(\mathrm{Al}\) will be more close to 575 or \(760 \mathrm{~kJ}\) \(\mathrm{mol}^{-1}\)? Justify your answer.

Answer: It will be more close to \(575 \mathrm{~kJ} \mathrm{~mol}^{-1}\). The value for \(\mathrm{Al}\) should be lower than that of \(\mathrm{Mg}\) because of effective shielding of \(3 p\) electrons from the nucleus by 3s-electrons.

(d) Electron Gain Enthalpy

When an electron is added to a neutral gaseous atom \((\mathrm{X})\) to convert it into a negative ion, the enthalpy change accompanying the process is defined as the Electron Gain Enthalpy \(\left(\Delta_{e g} \boldsymbol{H}\right)\). Electron gain enthalpy provides a measure of the ease with which an atom adds an electron to form anion as represented by equation 3.3.
\(
\mathrm{X}(\mathrm{g})+\mathrm{e}^{-} \rightarrow \mathrm{X}^{-}(\mathrm{g}) \dots(3.3)
\)

• Depending on the element, the process of adding an electron to the atom can be either endothermic or exothermic.
• For many elements energy is released when an electron is added to the atom and the electron gain enthalpy is negative. For example, group 17 elements (the halogens) have very high negative electron gain enthalpies because they can attain stable noble gas electronic configurations by picking up an electron.
• On the other hand, noble gases have large positive electron gain enthalpies because the electron has to enter the next higher principal quantum level leading to a very unstable electronic configuration. It may be noted that electron gain enthalpies have large negative values toward the upper right of the periodic table preceding the noble gases.
• The variation in electron gain enthalpies of elements is less systematic than for ionization enthalpies. As a general rule, electron gain enthalpy becomes more negative with increase in the atomic number across a period.
• The effective nuclear charge increases from left to right across a period and consequently, it will be easier to add an electron to a smaller atom since the added electron on an average would be closer to the positively charged nucleus.
• We should also expect electron gain enthalpy to become less negative as we go down a group because the size of the atom increases and the added electron would be farther from the nucleus. This is generally the case (Table 3.7).

• However, the electron gain enthalpy of \(\mathrm{O}\) or \(\mathrm{F}\) is less negative than that of the succeeding element. This is because when an electron is added to \(\mathrm{O}\) or \(\mathrm{F}\), the added electron goes to the smaller \(n=2\) quantum level and suffers significant repulsion from the other electrons present in this level. For the \(n=3\) quantum level ( \(\mathrm{S}\) or \(\mathrm{Cl}\) ), the added electron occupies a larger region of space and the electron-electron repulsion is much less.

Factors on which EGE depends (EGE)

• Atomic size: As size increases EGE becomes less negative.
• Nuclear charge: As nuclear charge increases, EGE becomes more negative.
• Electronic configuration: Elements having exactly half-filled or completely filled orbitals are very stable and have large positive electron gain enthalpy because they do not accept additional \(\mathrm{e}^{-}\)easily.

Variation of EGE in the periodic table

• Down the group, atomic size increases, EGE becomes less negative. Across a period, atomic size decreases, nuclear charge increases and EGE becomes more negative.
• Halogens have very high negative EGE because they attain stable noble gas electronic configuration by accepting an \(\mathrm{e}^{-}\).
• Noble gases have large positive EGE because the \(\mathrm{e}^{-}\) (electron) has to enter the next higher shell leading to a very unstable electronic configuration.
• \(\mathrm{EGE}\) of \(\mathrm{O}\) or \(\mathrm{F}\) is less negative than the succeeding element \(\mathrm{S}\) or \(\mathrm{Cl}\). This is because when an \(\mathrm{e}^{-}\)is added to \(\mathrm{O}\) or \(\mathrm{F}\), the added \(\mathrm{e}^{-}\)goes to smaller \(\mathrm{n}=2\) quantum level and suffers significant repulsion from other \(\mathrm{e}^{-1} \mathrm{~s}\) in this level. For \(\mathrm{n}=3\) level ( \(\mathrm{S}\) or \(\mathrm{Cl}\) ), added \(\mathrm{e}^{-}\)occupies a larger region of space and \(\mathrm{e}^{-}-\mathrm{e}^{-}\)repulsion is much less.

Example 3.7: Which of the following will have the most negative electron gain enthalpy and which is the least negative?
\(
\mathrm{P}, \mathrm{S}, \mathrm{Cl}, \mathrm{F} \text {. }
\)
Explain your answer.

Answer: Electron gain enthalpy generally becomes more negative across a period as we move from left to right. Within a group, electron gain enthalpy becomes less negative down a group. However, adding an electron to the \(2 p\)-orbital leads to greater repulsion than adding an electron to the larger \(3 p\)-orbital. Hence the element with most negative electron gain enthalpy is chlorine; the one with the least negative electron gain enthalpy is phosphorus.

(e) Electronegativity

A qualitative measure of the ability of an atom in a chemical compound to attract shared electrons to itself is called electronegativity. Unlike ionization enthalpy and electron gain enthalpy, it is not a measurable quantity. However, a number of numerical scales of electronegativity of elements viz., Pauling scale, Mulliken-Jaffe scale, and Allred-Rochow scale have been developed. The one which is the most widely used is the Pauling scale. Linus Pauling, an American scientist, in 1922 assigned arbitrarily a value of 4.0 to fluorine, the element considered to have the greatest ability to attract electrons. Approximate values for the electronegativity of a few elements are given in Table 3.8(a) The electronegativity of any given element is not constant; it varies depending on the element to which it is bound.

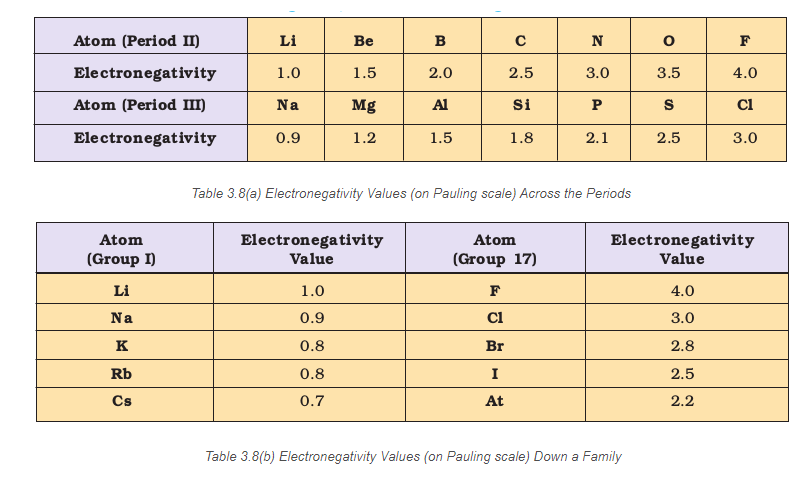
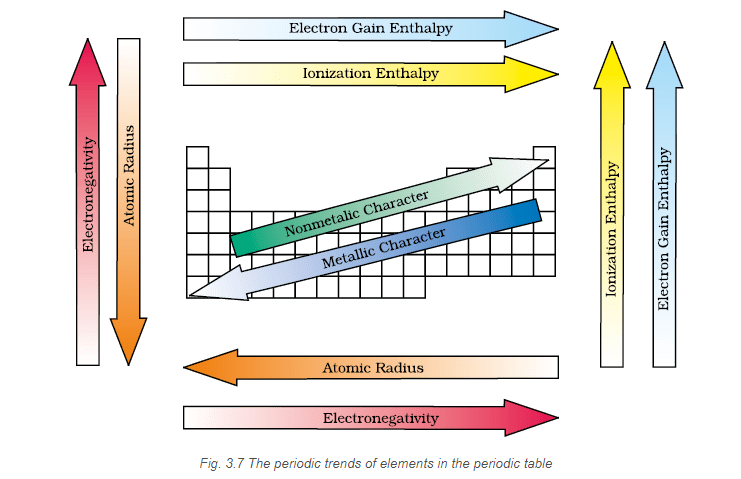
• Electronegativity generally increases across a period from left to right (say from lithium to fluorine) and decrease down a group (say from fluorine to astatine) in the periodic table.
• The attraction between the outer (or valence) electrons and the nucleus increases as the atomic radius decreases in a period. The electronegativity also increases. On the same account, electronegativity values decrease with the increase in atomic radii down a group. The trend is similar to that of ionization enthalpy.
• Non-metallic elements have strong tendency to gain electrons. Therefore, electronegativity is directly related to that non-metallic properties of elements. It can be further extended to say that the electronegativity is inversely related to the metallic properties of elements.
• Thus, the increase in electronegativities across a period is accompanied by an increase in non-metallic properties (or decrease in metallic properties) of elements. Similarly, the decrease in electronegativity down a group is accompanied by a decrease in non-metallic properties (or increase in metallic properties) of elements. All these periodic trends are summarised in Figure 3.7.

The electronegativity of any given element is not constant but depends on the following factors:

• State of hybridization: \(s p\)-hybridized carbon is more electronegative than \(\mathrm{sp}^2\) hybridized which in turn is more electronegative than \(\mathrm{sp}^3\) hybridized carbon.
• O.S. of the element: As O.S. of the element increases, electronegativity increases.
• Nature of substituents attached to the atom: For ex: \(\mathrm{C}\)-atom in \(\mathrm{CF}_3 \mathrm{I}\) is more electronegative than in \(\mathrm{CH}_3 \mathrm{I}\).

Variation of electronegativity in the periodic table

• Down the group, atomic radius increases, electronegativity decreases
• Across a period, atomic radius and nuclear charge increases, and electronegativity increases.
• \(\mathrm{F}\) is the most electronegative element and caesium is the least electronegative element.

Periodic Trends in Chemical Properties

In this section, we shall study the periodicity of the valence state shown by elements and the anomalous properties of the second-period elements (from lithium to fluorine). Chemical reactivity is highest at the two extremes of a period and is lowest in the centre.

(a) Periodicity of Valence or Oxidation States

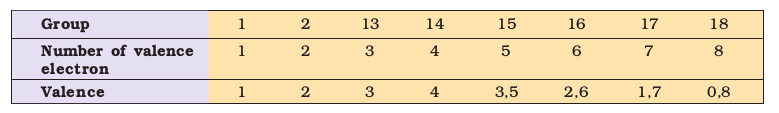
• The valence is the most characteristic property of the elements and can be understood in terms of their electronic configurations. The valence of representative elements is usually (though not necessarily) equal to the number of electrons in the outermost orbitals and/or equal to eight minus the number of outermost electrons as shown below.

• The oxidation state of an element in a particular compound can be defined as the charge acquired by its atom on the basis of electronegative consideration from other atoms in the molecule. Nowadays the term oxidation state is frequently used for valence. Consider the two oxygen containing compounds: \(\mathrm{OF}_2\) and \(\mathrm{Na}_2 \mathrm{O}\). The order of electronegativity of the three elements involved in these compounds is \(\mathrm{F}>\) \(\mathrm{O}>\mathrm{Na}\). Each of the atoms of fluorine, with outer electronic configuration \(2 s^2 2 p^5\), shares one electron with oxygen in the \(\mathrm{OF}_2\) molecule. Being the highest electronegative element, fluorine is given oxidation state -1. Since there are two fluorine atoms in this molecule, oxygen with outer electronic configuration \(2 s^2 2 p^4\) shares two electrons with fluorine atoms and thereby exhibits oxidation state +2. In \(\mathrm{Na}_2 \mathrm{O}\), oxygen being more electronegative accepts two electrons, one from each of the two sodium atoms and, thus, shows oxidation state -2. On the other hand sodium with electronic configuration \(3 s^1\) loses one electron to oxygen and is given oxidation state +1.
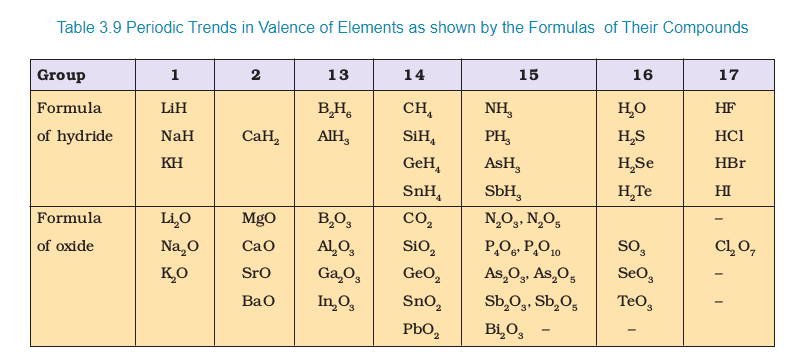
• Some periodic trends observed in the valence of elements (hydrides and oxides) are shown in Table 3.9. Other such periodic trends which occur in the chemical behaviour of the elements are discussed elsewhere in this book. There are many elements which exhibit variable valence. This is particularly characteristic of transition elements and actinoids, which we shall study later.

Variation of oxidation state in the periodic table

• Across a period, no. of valence \(\mathrm{e}^{-1} \mathrm{~s}\) increases from 1 to 8 . The valence of elements first increases from 1 to 4 and then decreases to zero.
• Down the group, no. of valence \(\mathrm{e}^{-1}\) s remain the same, and therefore, all elements in a group exhibit the same valence.
• Noble gases are zerovalent, i.e., their valence is zero because they are chemically inert.

Example 3.8: Using the Periodic Table, predict the formulas of compounds which might be formed by the following pairs of elements; (a) silicon and bromine (b) aluminium and sulphur.

Answer: (a) Silicon is group 14 element with a valence of 4; bromine belongs to the halogen family with a valence of 1. Hence the formula of the compound formed would be \(\mathrm{SiBr}_4\).
(b) Aluminium belongs to group 13 with a valence of 3; sulphur belongs to group 16 elements with a valence of 2. Hence, the formula of the compound formed would be \(\mathrm{Al}_2 \mathrm{~S}_3\).

(b) Anomalous Properties of Second-Period Elements

• The first element of each of the groups 1 (lithium) and 2 (beryllium) and groups 13-17 (boron to fluorine) differs in many respects from the other members of their respective group. For example, lithium unlike other alkali metals, and beryllium unlike other alkaline earth metals, form compounds with pronounced covalent character; the other members of these groups predominantly form ionic compounds. In fact, the behaviour of lithium and beryllium is more similar with the second element of the following group i.e., magnesium and aluminium, respectively. This sort of similarity is commonly referred to as diagonal relationship in the periodic properties.

• The anomalous behaviour is attributed to their (i) small size, (ii) large charge/ radius ratio, (iii) high electronegativity of the elements and (iv) non-availability of orbitals due to which they cannot expand their covalence beyond 4.
• In addition, the first member of group has only four valence orbitals ( \(2 s\) and \(2 p\) ) available for bonding, whereas the second member of the groups have nine valence orbitals \((3 s, 3 p, 3 d)\). As a consequence of this, the maximum covalency of the first member of each group is 4 (e.g., boron can only form \(\left[\mathrm{BF}_4\right]^{-}\), whereas the other members of the groups can expand their valence shell to accommodate more than four pairs of electrons e.g., aluminium \(\left[\mathrm{AlF}_6\right]^{3-}\) forms).
• Furthermore, the first member of \(p\)-block elements displays greater ability to form \(p_\pi-p_\pi\) multiple bonds to itself (e.g., \(\mathrm{C}=\mathrm{C}, \mathrm{C} \equiv \mathrm{C}, \mathrm{N}=\mathrm{N}\), \(\mathrm{N} \equiv \mathrm{N}\) ) and to other second period elements (e.g., \(\mathrm{C}=\mathrm{O}, \mathrm{C}=\mathrm{N}, \mathrm{C} \equiv \mathrm{N}\), \(\mathrm{N}=\mathrm{O}\) ) compared to subsequent members of the same group.

Example 3.9: Are the oxidation state and covalency of \(\mathrm{Al}\) in \(\left[\mathrm{AlCl}\left(\mathrm{H}_2 \mathrm{O}\right)_5\right]^{2+}\) same?

Answer: The oxidation state of \(\mathrm{Al}\) is +3 and the covalency is 6.

Periodic Trends and Chemical Reactivity

• We have observed the periodic trends in certain fundamental properties such as atomic and ionic radii, ionization enthalpy, electron gain enthalpy and valence. We know by now that the periodicity is related to electronic configuration. That is, all chemical and physical properties are a manifestation of the electronic configuration of elements.
• The atomic and ionic radii, as we know, generally decrease in a period from left to right. As a consequence, the ionization enthalpies generally increase (with some exceptions) and electron gain enthalpies become more negative across a period.
• In other words, the ionization enthalpy of the extreme left element in a period is the least and the electron gain enthalpy of the element on the extreme right is the highest negative (note: noble gases having completely filled shells have rather positive electron gain enthalpy values). This results into high chemical reactivity at the two extremes and the lowest in the centre.
• Thus, the maximum chemical reactivity at the extreme left (among alkali metals) is exhibited by the loss of an electron leading to the formation of a cation and at the extreme right (among halogens) shown by the gain of an electron forming an anion. This property can be related with the reducing and oxidizing behaviour of the elements which you will learn later.
• The metallic character of an element, which is highest at the extremely left decreases and the non-metallic character increases while moving from left to right across the period.
• The chemical reactivity of an element can be best shown by its reactions with oxygen and halogens. Here, we shall consider the reaction of the elements with oxygen only. Elements on two extremes of a period easily combine with oxygen to form oxides.
• The normal oxide formed by the element on extreme left is the most basic (e.g., \(\mathrm{Na}_2 \mathrm{O}\) ), whereas that formed by the element on extreme right is the most acidic (e.g., \(\mathrm{Cl}_2 \mathrm{O}_7\) ).
• Oxides of elements in the centre are amphoteric (e.g., \(\mathrm{Al}_2 \mathrm{O}_3, \mathrm{As}_2 \mathrm{O}_3\) ) or neutral (e.g., \(\mathrm{CO}, \mathrm{NO}, \mathrm{N}_2 \mathrm{O}\) ).
• Amphoteric oxides behave as acidic with bases and as basic with acids, whereas neutral oxides have no acidic or basic properties.
• Among transition metals ( \(3 d\) series), the change in atomic radii is much smaller as compared to those of representative elements across the period. The change in atomic radii is still smaller among inner-transition metals ( \(4 f\) series). The ionization enthalpies are intermediate between those of \(s\) – and \(p\)-blocks. As a consequence, they are less electropositive than group 1 and 2 metals.
• In a group, the increase in atomic and ionic radii with increase in atomic number generally results in a gradual decrease in ionization enthalpies and a regular decrease (with the exception in some third-period elements) in electron gain enthalpies in the case of main group elements. Thus, the metallic character increases down the group and non-metallic character decreases.
• In the case of transition elements, however, a reverse trend is observed. This can be explained in terms of atomic size and ionization enthalpy.

Example 3.10: Show by a chemical reaction with water that \(\mathrm{Na}_2 \mathrm{O}\) is a basic oxide and \(\mathrm{Cl}_2 \mathrm{O}_7\) is an acidic oxide.

Answer: 

\(\mathrm{Na}_2 \mathrm{O}\) with water forms a strong base whereas \(\mathrm{Cl}_2 \mathrm{O}_7\) forms strong acid.
\(
\begin{aligned}
& \mathrm{Na}_2 \mathrm{O}+\mathrm{H}_2 \mathrm{O} \rightarrow 2 \mathrm{NaOH} \\
& \mathrm{Cl}_2 \mathrm{O}_7+\mathrm{H}_2 \mathrm{O} \rightarrow 2 \mathrm{HClO}_4
\end{aligned}
\)
Their basic or acidic nature can be qualitatively tested with litmus paper.