Historically, results observed from the studies of interactions of radiations with matter have provided immense information regarding the structure of atoms and molecules. Neils Bohr utilised these results to improve upon the model proposed by Rutherford. Two developments played a major role in the formulation of Bohr’s model of atom. These were:

(i) Dual character of the electromagnetic radiation which means that radiations possess both wave like and particle like properties, and

(ii) Experimental results regarding atomic spectra.

First, we will discuss about the dual nature of electromagnetic radiations.

Wave Nature of Electromagnetic Radiation

James Maxwell (1870) was the first to give a comprehensive explanation about the interaction between the charged bodies and the behaviour of electrical and magnetic fields on macroscopic level (It was experimentally confirmed by German Physicist Heinrich Hertz). He suggested that when electrically charged particle moves under acceleration, alternating electrical and magnetic fields are produced and transmitted. These fields are transmitted in the forms of waves called electromagnetic waves or electromagnetic radiation. Maxwell was again the first to reveal that light waves are associated with oscillating electric and magnetic character (Fig. 2.6).

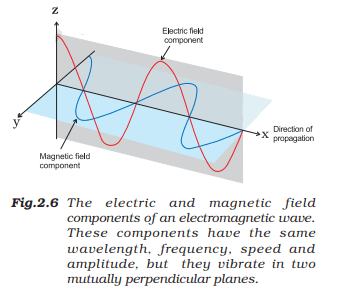
Although electromagnetic wave motion is complex in nature, we will consider here only a few simple properties of EM waves.

(i) The oscillating electric and magnetic fields produced by oscillating charged particles are perpendicular to each other and both are perpendicular to the direction of propagation of the wave. Simplified picture of electromagnetic wave is shown in Fig. 2.6.

(ii) Unlike sound waves or waves produced in water, electromagnetic waves do not require medium and can move in vacuum.

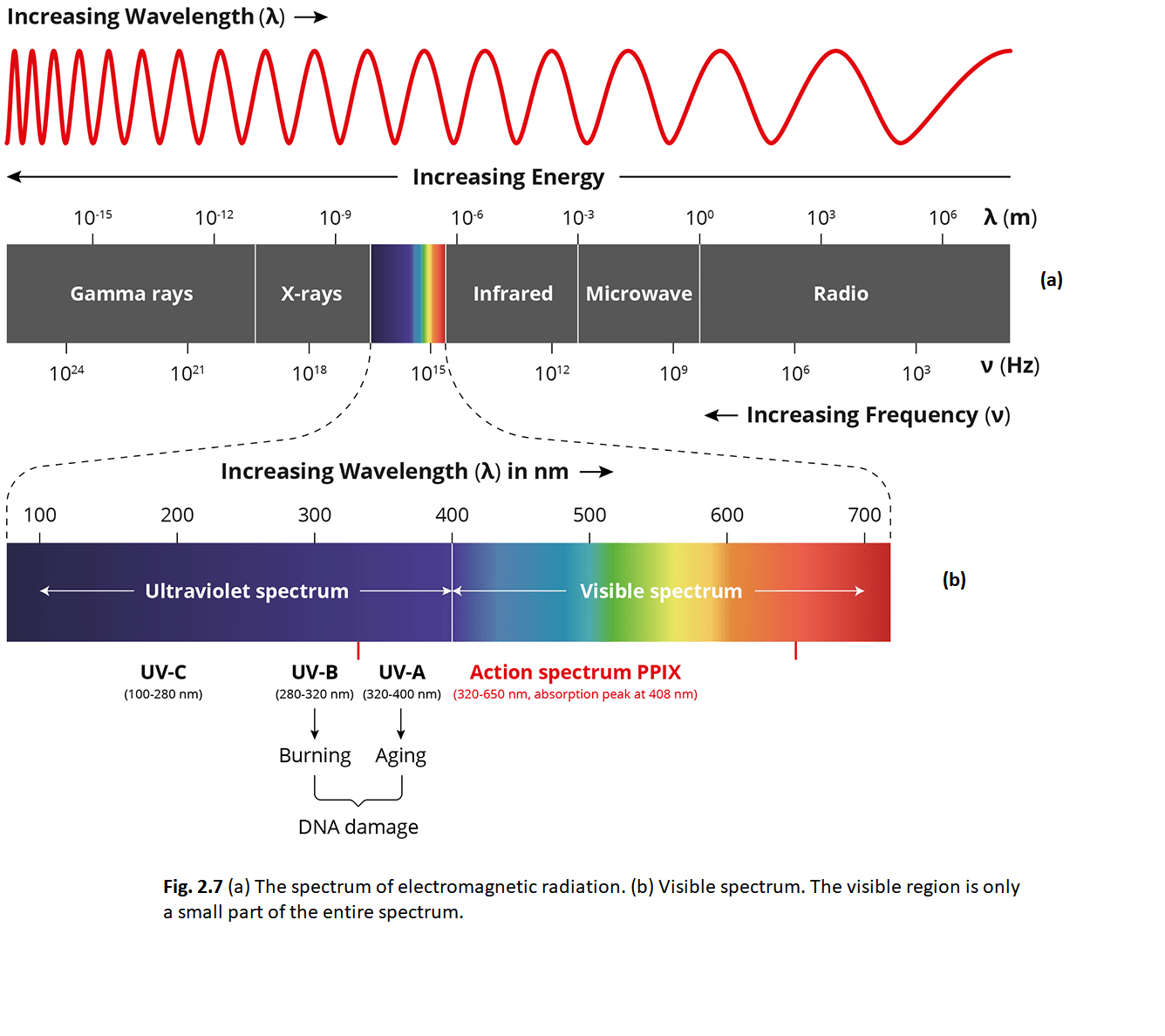
(iii) EM waves are of many different types, which differ from one another in wavelength (or frequency). These constitute what is called electromagnetic spectrum (Fig. 2.7). Different regions of the spectrum are identified by different names. Some examples are: radio frequency region around \(10^{6} \mathrm{~Hz}\), used for broadcasting; microwave region around \(10^{10} \mathrm{~Hz}\) used for radar; infrared region around \(10^{13} \mathrm{~Hz}\) used for heating; ultraviolet region around \(10^{16} \mathrm{~Hz}\) a component of sun’s radiation. The small portion around \(10^{15} \mathrm{~Hz}\), is what is ordinarily called visible light. It is only this part which our eyes can see (or detect). Special instruments are required to detect non-visible radiation.

(iv) Different kinds of units are used to represent electromagnetic radiation.

These radiations are characterised by the properties, namely, frequency \((\nu)\) ( or \(f\) ) and wavelength \((\lambda)\).

The SI unit for frequency \((f)\) is hertz (Hz, \(\left.\mathbf{s}^{-1}\right)\), after Heinrich Hertz. It is defined as the number of waves that pass a given point in one second.

Wavelength should have the units of length and as you know that the SI units of length is meter \((\mathrm{m})\). Since electromagnetic radiation consists of different kinds of waves of much smaller wavelengths, smaller units are used. Fig. \(2.7\) shows various types of electromagnetic radiations which differ from one another in wavelengths and frequencies.

Relation between Wavelength and Frequency 

In vaccum all types of electromagnetic radiations, regardless of wavelength, travel at the same speed, i.e., \(3.0 \times 10^{8} \mathrm{~m} \mathrm{~s}^{-1}(2.997925\) \(\times 10^{8} \mathrm{~m} \mathrm{~s}^{-1}\), to be precise). This is called speed (velocity) of light and is given the symbol ‘ \(\mathrm{c}\) ‘. The frequency \((f)\), wavelength \((\lambda)\) and velocity of light (c) are related by the equation (2.5).

\(c=f \lambda \dots ….(2.5)\)

Note: Wavelength and frequency of a wave are inversely proportional. As the wavelength increases, the frequency decreases and as the frequency increases wavelength decreases.

The other commonly used quantity specially in spectroscopy, is the wavenumber \(\tilde{\nu}\). It is defined as the number of wavelengths per unit length. Its units are reciprocal of wavelength unit, i.e., \(\mathrm{m}^{-1}\). However commonly used unit is \(\mathrm{cm}^{-1}\) (not SI unit).

\(\tilde{\nu}=\frac{1}{\lambda}\), where \(\lambda\) is the wavelength.

Problem 2.3

The Vividh Bharati station of All India Radio, Delhi, broadcasts on a frequency of \(1,368 \mathrm{kHz}\) (kilo hertz). Calculate the wavelength of the electromagnetic radiation emitted by the transmitter. Which part of the electromagnetic spectrum does it belong to?

Solution:

The wavelength, \(\lambda\), is equal to \(\mathrm{c} / f\), where \(c\) is the speed of electromagnetic radiation in vacuum and \(f\) is the frequency. Substituting the given values, we have
\(\lambda=\frac{c}{f}\)
\(=\frac{3.00 \times 10^{8} \mathrm{~ms}^{-1}}{1368 \mathrm{kHz}}\)
\(=\frac{3.00 \times 10^{8} \mathrm{~m} \mathrm{~s}^{-1}}{1368 \times 10^{3} \mathrm{~s}^{-1}}\)
\(=219.3 \mathrm{~m}\)
This is a characteristic radiowave wavelength.

Problem 2.4

The wavelength range of the visible spectrum extends from violet \((400 \mathrm{~nm}\) ) to red \((750 \mathrm{~nm})\). Express these wavelengths in frequencies \((\mathrm{Hz}) .\left(1 \mathrm{~nm}=10^{-9} \mathrm{~m}\right)\)

Solution:
Using equation 2.5,  frequency of violet light
\(f=\frac{\mathrm{c}}{\lambda}=\frac{3.00 \times 10^{8} \mathrm{~m} \mathrm{~s}^{-1}}{400 \times 10^{-9} \mathrm{~m}}\)
\(=7.50 \times 10^{14} \mathrm{~Hz}\)

Frequency of red light
\(
f=\frac{\mathrm{c}}{\lambda}=\frac{3.00 \times 10^{8} \mathrm{~ms}^{-1}}{750 \times 10^{-9} \mathrm{~m}}=4.00 \times 10^{14} \mathrm{~Hz}
\)
The range of visible spectrum is from \(4.0 \times 10^{14}\) to \(7.5 \times 10^{14} \mathrm{~Hz}\) in terms of frequency units.

Problem 2.5

Calculate (a) wavenumber and (b) frequency of yellow radiation having wavelength \(5800 \AA\).
Solution
(a) Calculation of wavenumber \((\tilde{\nu})\)
\(
\begin{aligned}
\lambda=5800 Å &=5800 \times 10^{-8} \mathrm{~cm} \\
&=5800 \times 10^{-10} \mathrm{~m}
\end{aligned}
\)
\(
\begin{aligned}
\tilde{\nu}=\frac{1}{\lambda} &=\frac{1}{5800 \times 10^{-10} \mathrm{~m}} \\
&=1.724 \times 10^{6} \mathrm{~m}^{-1} \\
&=1.724 \times 10^{4} \mathrm{~cm}^{-1}
\end{aligned}
\)

(b) Calculation of the frequency \((f)\)
\(
f=\frac{\mathrm{c}}{\lambda}=\frac{3 \times 10^{8} \mathrm{~ms}^{-1}}{5800 \times 10^{-10} \mathrm{~m}}=5.172 \times 10^{14} \mathrm{~s}^{-1}
\)

Particle Nature of Electromagnetic Radiation: Planck’s Quantum Theory

Some of the experimental phenomenon such as diffraction and interference can be explained by the wave nature of the electromagnetic radiation. However, following are some of the observations which could not be explained with the help of even the electromagentic theory of 19th century physics (known as classical physics):

(i) the nature of emission of radiation from hot bodies (black-body radiation)

(ii) ejection of electrons from metal surface when radiation strikes it (photoelectric effect)

(iii) variation of heat capacity of solids as a function of temperature

(iv) Line spectra of atoms with special reference to hydrogen.

These phenomena indicate that the system can take energy only in discrete amounts. All possible energies cannot be taken up or radiated. It is noteworthy that the first concrete explanation for the phenomenon of the black body radiation mentioned above was given by Max Planck in 1900. Let us first try to understand this phenomenon, which is given below:

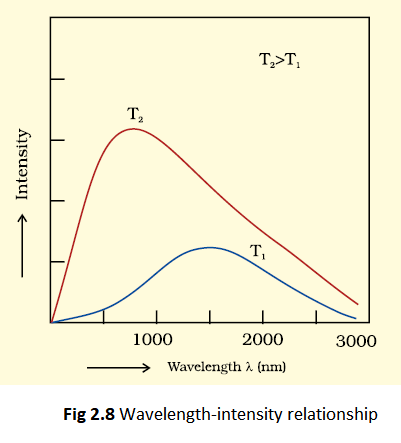
Hot objects emit electromagnetic radiations over a wide range of wavelengths. At high temperatures, an appreciable proportion of radiation is in the visible region of the spectrum. As the temperature is raised, a higher proportion of short wavelength (blue light) is generated. For example, when an iron rod is heated in a furnace, it first turns to dull red and then progressively becomes more and more red as the temperature increases. As this is heated further, the radiation emitted becomes white and then becomes blue as the temperature becomes very high. This means that red radiation is most intense at a particular temperature and the blue radiation is more intense at another temperature. This means intensities of radiations of different wavelengths emitted by hot body depend upon its temperature.

By late 1850’s it was known that objects made of different material and kept at different temperatures emit different amount of radiation. Also, when the surface of an object is irradiated with light (electromagnetic radiation), a part of radiant energy is generally reflected as such, a part is absorbed and a part of it is transmitted. The reason for incomplete absorption is that ordinary objects are as a rule imperfect absorbers of radiation. An ideal body, which emits and absorbs radiations of all frequencies uniformly, is called a black body, and the radiation emitted by such a body is called black body radiation. In practice, no such body exists. Carbon black approximates fairly closely to black body. A good physical approximation to a black body is a cavity with a tiny hole, which has no other opening. Any ray entering the hole will be reflected by the cavity walls and will be eventually absorbed by the walls. A black body is also a perfect radiator of radiant energy. Furthermore, a black body is in thermal equilibrium with its surroundings. It radiates same amount of energy per unit area as it absorbs from its surrounding in any given time. The amount of light emitted (intensity of radiation) from a black body and its spectral distribution depends only on its temperature. At a given temperature, intensity of radiation emitted increases with the increase of wavelength, reaches a maximum value at a given wavelength, and then starts decreasing with further increase of wavelength, as shown in Fig. 2.8. Also, as the temperature increases, maxima of the curve shifts to short wavelength. Several attempts were made to predict the intensity of radiation as a function of wavelength.

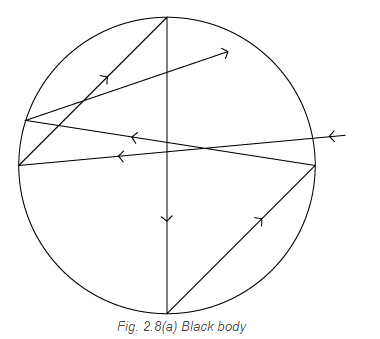
Max Planck arrived at a satisfactory relationship by making an assumption that absorption and emmission of radiation arises from oscillator i.e., atoms in the wall of black body. Their frequency of oscillation is changed by interaction with oscillators of electromagnetic radiation. Planck assumed that radiation could be sub-divided into discrete chunks of energy. He suggested that atoms and molecules could emit or absorb energy only in discrete quantities and not in a continuous manner. He gave the name quantum to the smallest quantity of energy that can be emitted or absorbed in the form of electromagnetic radiation. 

The energy \((E)\) of a quantum of radiation is proportional to its frequency \((f)\) and is expressed by equation (2.6).
\(E=h f \dots ….(2.6)\)
The proportlonality constant, ‘ \(h\) ‘ is known as Planck’s constant and has the value \(6.626 \times 10^{-34} \mathrm{~J} \mathrm{~s}\).

With this theory, Planck was able to explain the distribution of intensity in the radiation from black body as a function of frequency or wavelength at different temperatures.

Quantisation has been compared to standing on a staircase. A person can stand on any step of a staircase, but it is not possible for him/her to stand in between the two steps. The energy can take any one of the values from the following set, but cannot take on any values between them.
\(\mathrm{E}=0, h v, 2 h v, 3 h v \ldots . n h v \ldots . .\)

Photoelectric Effect

In 1887, H. Hertz performed a very interesting experiment in which electrons (or electric current) were ejected when certain metals (for
example potassium, rubidium, caesium, etc.) were exposed to a beam of light as shown in Fig.2.9. The phenomenon is called Photoelectric effect. The results observed in this experiment were:

(i) The electrons are ejected from the metal surface as soon as the beam of light strikes the surface, i.e., there is no time lag between the striking of light beam and the ejection of electrons from the metal surface.

(ii) The number of electrons ejected is proportional to the intensity or brightness of light.

(iii) For each metal, there is a characteristic minimum frequency, \(f_{0}\) (also known as threshold frequency) below which photoelectric effect is not observed. At a frequency \(f>f_{0}\), the ejected electrons come out with certain kinetic energy. The kinetic energies of these electrons increase with the increase of frequency of the light used.

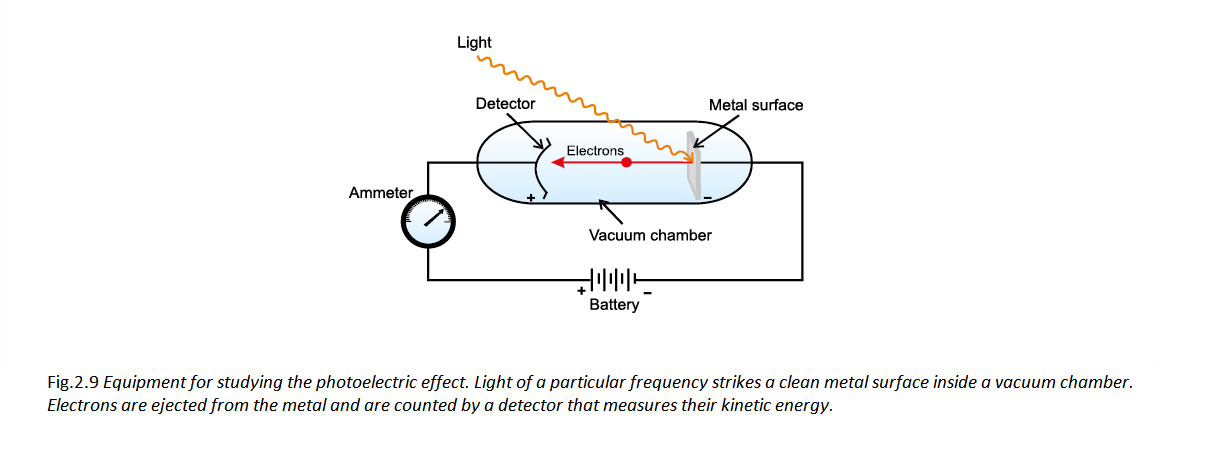
All the above results could not be explained on the basis of laws of classical physics. According to latter, the energy content of the beam of light depends upon the brightness of the light. In other words, number of electrons ejected and kinetic energy associated with them should depend on the brightness of light. It has been observed that though the number of electrons ejected does depend upon the brightness of light, the kinetic energy of the ejected electrons does not. For example, red light \(\left[f=(4.3\right.\) to \(\left.4.6) \times 10^{14} \mathrm{~Hz}\right]\) of any brightness (intensity) may shine on a plece of potassium metal for hours but no photoelectrons are ejected. But, as soon as even a very weak yellow light \(\left(f=5.1-5.2 \times 10^{14} \mathrm{~Hz}\right)\) shines on the potassium metal, the photoelectric effect is observed. The threshold frequency \(\left(f_{0}\right)\) for potassium metal is \(5.0 \times 10^{14} \mathrm{~Hz}\).

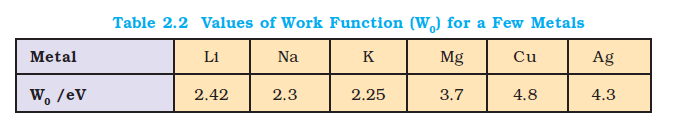
Einstein (1905) was able to explain the photoelectric effect using Planck’s quantum theory of electromagnetic radiation as a starting point. Shining a beam of light on to a metal surface can, therefore, be viewed as shooting a beam of particles, the photons. When a photon of sufficient energy strikes an electron in the atom of the metal, it transfers its energy instantaneously to the electron during the collision and the electron is ejected without any time lag or delay. Greater the energy possessed by the photon, greater will be transfer of energy to the electron and greater the kinetic energy of the ejected electron. In other words, kinetic energy of the ejected electron is proportional to the frequency of the electromagnetic radiation. Since the striking photon has energy equal to \(h f\) and the minimum energy required to eject the electron is \(h f_{0}\) (also called work function, \(\mathrm{W}_{0}\); Table 2.2), then the difference in energy \(\left(h f-h f_{0}\right.\) ) is transferred as the kinetic energy of the photoelectron. Following the conservation of energy principle, the kinetic energy of the ejected electron is given by the equation 2.7.
\(h f=h f_{0}+\frac{1}{2} m_{\mathrm{e}} \mathrm{v}^{2} \dots ….(2.7)\)
where \(m_{e}\) is the mass of the electron and \(v\) is the velocity associated with the ejected electron. Lastly, a more intense beam of light consists of larger number of photons, consequently, the number of electrons ejected is also larger as compared to that in an experiment in which a beam of weaker intensity of light is employed.

Dual Behaviour of Electromagnetic Radiation

The photoelectric effect could be explained considering that radiations consist of small packets of energy called quanta. These packets of energy can be treated as particles. With various experiments, it was concluded that light possesses both particle and wave-like properties, i.e., light has dual behaviour). Whenever radiation interacts with matter, it displays particle-like properties in contrast to the wavelike properties (interference and diffraction), which it exhibits when it propagates. 

• Particle nature
• Wave nature

Problem 2.6
Calculate the energy of one mole of photons of radiation whose frequency is \(5 \times 10^{14}\) Hz.

Solution:
Energy ( \(E\) ) of one photon is given by the expression
\(E=h f\)
\(h=6.626 \times 10^{-34} \mathrm{~J} \mathrm{~s}\)
\(f=5 \times 10^{14} \mathrm{~s}^{-1}\) (glven)
\(E=\left(6.626 \times 10^{-34} \mathrm{~J} \mathrm{~s}\right) \times\left(5 \times 10^{14} \mathrm{~s}^{-1}\right)\)
\(=3.313 \times 10^{-19} \mathrm{~J}\)
Energy of one mole of photons
\(
=\left(3.313 \times 10^{-19} \mathrm{~J}\right) \times\left(6.022 \times 10^{23} \mathrm{~mol}^{-1}\right)
\)
\(
=199.51 \mathrm{~kJ} \mathrm{~mol}^{-1}
\)

Problem 2.7
A 100-watt bulb emits monochromatic light of wavelength \(400 \mathrm{~nm}\). Calculate the number of photons emitted per second by the bulb.

Solution:
Power of the bulb \(=100\) watt
\(
=100 \mathrm{~J} \mathrm{~s}^{-1}
\)
Energy of one photon \(E=h f=h c / \lambda\)
\(
=\frac{6.626 \times 10^{-34} \mathrm{~J} \mathrm{~s} \times 3 \times 10^{8} \mathrm{~m} \mathrm{~s}^{-1}}{400 \times 10^{-9} \mathrm{~m}}
\)
\(
=4.969 \times 10^{-19} \mathrm{~J}
\)
Number of photons emitted
\(
\frac{100 \mathrm{~J} \mathrm{~s}^{-1}}{4.969 \times 10^{-19} \mathrm{~J}}=2.012 \times 10^{20} \mathrm{~s}^{-1}
\)

Problem 2.8
When electromagnetic radiation of wavelength \(300 \mathrm{~nm}\) falls on the surface of sodium, electrons are emitted with a kinetic energy of \(1.68 \times 10^{5} \mathrm{~J} \mathrm{~mol}^{-1}\). What is the minimum energy needed to remove an electron from sodium? What is the maximum wavelength that will cause a photoelectron to be emitted?

Solution: 

The energy \((E)\) of a \(300 \mathrm{~nm}\) photon is given by
\(
\begin{aligned}
E &=h c / \lambda \\
&=\frac{6.626 \times 10^{-34} \mathrm{~J} \mathrm{~s} \times 3.0 \times 10^{8} \mathrm{~m} \mathrm{~s}^{-1}}{300 \times 10^{-9} \mathrm{~m}} \\
&=6.626 \times 10^{-19} \mathrm{~J}
\end{aligned}
\)
The energy of one mole of photons
\(
\begin{aligned}
&=6.626 \times 10^{-19} \mathrm{~J} \times 6.022 \times 10^{23} \mathrm{~mol}^{-1} \\
&=3.99 \times 10^{5} \mathrm{~J} \mathrm{~mol}^{-1}
\end{aligned}
\)
The minimum energy needed to remove one mole of electrons from sodium
\(
=(3.99-1.68) 10^{5} \mathrm{~J} \mathrm{~mol}^{-1}
\)
\(=2.31 \times 10^{5} \mathrm{~J} \mathrm{~mol}^{-1}\)
The minimum energy for one electron
\(=3.84 \times 10^{-19} \mathrm{~J}\)
This corresponds to the wavelength
\(
\begin{aligned}
\therefore \lambda &=\frac{h c}{E} \\
&=\frac{6.626 \times 10^{-34} \mathrm{~J} \mathrm{~s} \times 3.0 \times 10^{8} \mathrm{~m} \mathrm{~s}^{-1}}{3.84 \times 10^{-19} \mathrm{~J}} \\
&=517 \mathrm{~nm}
\end{aligned}
\)
(This corresponds to green light)

Problem 2.9
The threshold frequency \(v_{0}\) for a metal is \(7.0 \times 10^{14} \mathrm{~s}^{-1}\). Calculate the kinetic energy of an electron emitted when radiation of frequency \(v=1.0 \times 10^{15} \mathrm{~s}^{-1}\) hits the metal.

Solution:
According to Einstein’s equation
Kinetic energy \(=1 / 2 m_{\mathrm{c}} \mathrm{v}^{2}=h\left(f-f_{0}\right)\)
\(
\begin{array}{r}
=\left(6.626 \times 10^{-34} \mathrm{~J} \mathrm{~s}\right)\left(1.0 \times 10^{15} \mathrm{~s}^{-1}-7.0\right. \left.\times 10^{14} \mathrm{~s}^{-1}\right) \\
=\left(6.626 \times 10^{-34} \mathrm{~J} \mathrm{~s}\right)\left(10.0 \times 10^{14} \mathrm{~s}^{-1}-7.0\right. \left.\times 10^{14} \mathrm{~s}^{-1}\right) \\
=\left(6.626 \times 10^{-34} \mathrm{~J} \mathrm{~s}\right) \times\left(3.0 \times 10^{14} \mathrm{~s}^{-1}\right) \\
=1.988 \times 10^{-19} \mathrm{~J}
\end{array}
\)

Evidence for the quantized Electronic Energy Levels: Atomic spectra

The speed of light depends upon the nature of the medium through which it passes. As a result, the beam of light is deviated or refracted from its original path as it passes from one medium to another. It is observed that when a ray of white light is passed through a prism, the wave with shorter wavelength bends more than the one with a longer wavelength. Since ordinary white light consists of waves with all the wavelengths in the visible range, a ray of white light is spread out into a series of coloured bands called spectrum. The light of red colour which has longest wavelength is deviated the least while the violet light, which has shortest wavelength is deviated the most. The spectrum of white light, that we can see, ranges from violet at \(7.50 \times 10^{14} \mathrm{~Hz}\) to red at \(4 \times 10^{14} \mathrm{~Hz}\). Such a spectrum is called continuous spectrum. Continuous because violet merges into blue, blue into green and so on. A similar spectrum is produced when a rainbow forms in the sky. Remember that visible light is just a small portion of the electromagnetic radiation (Fig.2.7). When electromagnetic radiation interacts with matter, atoms and molecules may absorb energy and reach to a higher energy state. With higher energy, these are in an unstable state. For returning to their normal (more stable, lower energy states) energy state, the atoms and molecules emit radiations in various regions of the electromagnetic spectrum.

Note: The restriction of any property to discrete values is called quantization.

Emission and Absorption Spectra

The spectrum of radiation emitted by a substance that has absorbed energy is called an emission spectrum. Atoms, molecules or ions that have absorbed radiation are said to be “excited”. To produce an emission spectrum, energy is supplied to a sample by heating it or irradiating it and the wavelength (or frequency) of the radiation emitted, as the sample gives up the absorbed energy, is recorded.

An absorption spectrum is like the photographic negative of an emission spectrum. A continuum of radiation is passed through a sample which absorbs radiation of certain wavelengths. The missing wavelength which corresponds to the radiation absorbed by the matter, leave dark spaces in the bright continuous spectrum.

spectroscopy

The study of emission or absorption spectra is referred to as spectroscopy. The spectrum of the visible light, as discussed above, was continuous as all wavelengths (red to violet) of the visible light are represented in the spectra. The emission spectra of atoms in the gas phase, on the other hand, do not show a continuous spread of wavelength from red to violet, rather they emit light only at specific wavelengths with dark spaces between them. Such spectra are called line spectra or atomic spectra because the emitted radiation is identified by the appearance of bright lines in the spectra (Fig. 2.10).

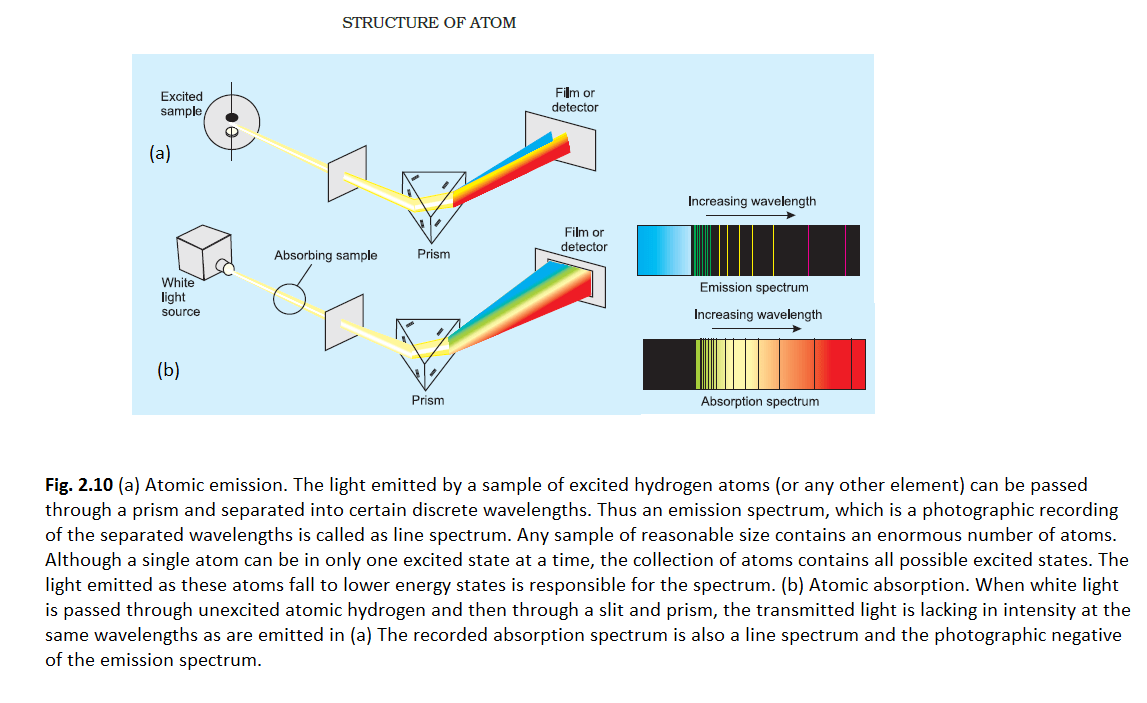
Line emission spectra are of great interest in the study of electronic structure. Each element has a unique line emission spectrum. The characteristic lines in atomic spectra can be used in chemical analysis to identify unknown atoms. German chemist, Robert Bunsen (1811-1899) was one of the first investigators to use line spectra to identify elements. Elements like rubidium (Rb), caesium (Cs) thallium (Tl), indium (In), gallium (Ga), and scandium \((\mathrm{Sc})\) were discovered when their minerals were analysed by spectroscopic methods. The element helium (He) was discovered in the sun by spectroscopic method.

Line Spectrum of Hydrogen

When an electric discharge is passed through gaseous hydrogen, the \(\mathrm{H}_{2}\) molecules dissociate and the energetically excited hydrogen atoms produced emit electromagnetic radiation of discrete frequencies. The hydrogen spectrum consists of several series of lines named after their discoverers. Balmer showed in 1885 on the basis of experimental observations that if spectral lines are expressed in terms of wavenumber \((\bar{\nu})\), then the visible lines of the hydrogen spectrum obey the following formula:
\(\bar{\nu}=109,677\left(\frac{1}{2^{2}}-\frac{1}{n^{2}}\right) \mathrm{cm}^{-1} \dots ….(2.8)\)
where \(n\) is an integer equal to or greater than 3 (i.e., \(n=3,4,5, \ldots)\)

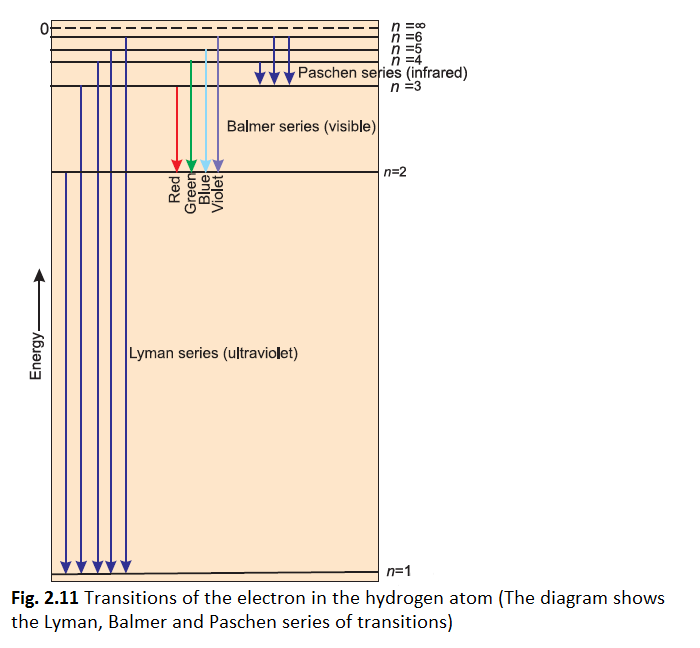
The series of lines described by this formula are called the Balmer series. The Balmer series of lines are the only lines in the hydrogen spectrum which appear in the visible region of the electromagnetic spectrum. The Swedish spectroscopist, Johannes Rydberg, noted that all series of lines in the hydrogen spectrum could be described by the following expression:

\(\bar{\nu}=109,677\left(\frac{1}{n_{1}^{2}}-\frac{1}{n_{2}^{2}}\right) \mathrm{cm}^{-1} \dots ….(2.9)\)
where \(n_{1}=1,2 \ldots \ldots . .\)
\(n_{2}=n_{1}+1, n_{1}+2 \ldots \ldots\)

The value \(109,677 \mathrm{~cm}^{-1}\) is called the Rydberg constant for hydrogen. The first five series of lines that correspond to \(n_{1}=1,2,3\), 4, 5 are known as Lyman, Balmer, Paschen, Bracket, and Pfund series, respectively, Table \(2.3\) shows these series of transitions in the hydrogen spectrum. Fig 2.11 shows the Lyman, Balmer, and Paschen series of transitions for hydrogen atom.

Of all the elements, hydrogen atom has the simplest line spectrum. Line spectrum becomes more and more complex for heavier atom. There are, however, certain features which are common to all line spectra, i.e., (i) line spectrum of element is unique and (ii) there is regularity in the line spectrum of each element.