1.12 Exercise Problems and Solutions
Q1. Calculate the molar mass of the following:
(i) \(\mathrm{H}_2 \mathrm{O}\)
(ii) \(\mathrm{CO}_2\)
(iii) \(\mathrm{CH}_4\)
Answer: (i) \(\mathrm{H}_2 \mathrm{O}\) :
The molecular mass of water, \(\mathrm{H}_2 \mathrm{O}\)
\(
\begin{aligned}
& =(2 \times \text { Atomic mass of hydrogen })+(1 \times \text { Atomic mass of oxygen }) \\
& =[2(1.0084 \mathrm{~u})+1(16.00 \mathrm{~u}) \\
& =18.02 \mathrm{~u}
\end{aligned}
\)
(ii) \(\mathrm{CO}_2\) :
The molecular mass of carbon dioxide, \(\mathrm{CO}_2\)
\(
\begin{aligned}
& =(1 \times \text { Atomic mass of carbon })+(2 \times \text { Atomic mass of oxygen }) \\
& =[1(12.011 \mathrm{~u})+2(16.00 \mathrm{~u})] \\
& =44.01 \mathrm{~u}
\end{aligned}
\)
(iii) \(\mathrm{CH}_4\) :
The molecular mass of methane, \(\mathrm{CH}_4\) \(=(1 \times\) Atomic mass of carbon \()+(4 \times\) Atomic mass of hydrogen \()\) \(=[1(12.011 \mathrm{~u})+4(1.008 \mathrm{~u})]\) \(=16.043 \mathrm{~u}\)
Q2. Calculate the mass percent of different elements present in sodium sulphate \(\left(\mathrm{Na}_2 \mathrm{SO}_4\right)\).
Answer: The molecular formula of sodium sulfate is \(\mathrm{Na}_2 \mathrm{SO}_4\).
Molar mass of \(\mathrm{Na}_2 \mathrm{SO}_4=[(2 \times 23.0)+(32.066)+4(16.00)]\) \(=142.066 \mathrm{~g}\)
The mass percent of an element \(=\frac{\text { Mass of thet element in the compound }}{\text { Molar mass of the compound }} \times 100\)
\(\therefore\) The mass percent of sodium:
\(=\frac{46.0 \mathrm{~g}}{142.066} \times 100\)
\(=32.379\)
\(=32.4 \%\)
The mass percent of sulphur:
\(
\begin{aligned}
& =\frac{32.066 \mathrm{~g}}{142.066} \times 100 \\
& =22.57 \\
& =22.6 \%
\end{aligned}
\)
The mass percent of oxygen:
\(
\begin{aligned}
& =\frac{64.0 \mathrm{~g}}{142.066} \times 100 \\
& =45.049 \\
& =45.05 \%
\end{aligned}
\)
Q3. Determine the empirical formula of an oxide of iron, which has \(69.9 \%\) iron and \(30.1 \%\) dioxygen by mass.
Answer: Step 1:
\(\%\) of iron by mass \(=69.9 \%\)
\(\%\) of oxygen by mass \(=30.1 \%\)
Relative moles of iron in iron oxide:
\(
\begin{aligned}
& =\frac{\% \text { of iron by mass }}{\text { Atomic mass of iron}} \\
& =\frac{69.9}{55.85}=1.25
\end{aligned}
\)
Relative moles of oxygen in iron oxide:
\(
\begin{aligned}
& =\frac{\% \text { of oxygen by mass }}{\text { Atomic mass of oxygen }} \\
& =\frac{30.1}{16.00}=1.88
\end{aligned}
\)
Step 2:
The simplest molar ratio of iron to oxygen:
\(
\begin{aligned}
& =1.25: 1.88 \\
& =1: 1.5 \\
& =2: 3
\end{aligned}
\)
\(\therefore\) The empirical formula of the iron oxide is \(\mathrm{Fe}_2 \mathrm{O}_3\)
Q4. Calculate the amount of carbon dioxide that could be produced when
(i) 1 mole of carbon is burnt in air.
(ii) 1 mole of carbon is burnt in \(16 \mathrm{~g}\) of dioxygen.
(iii) 2 moles of carbon are burnt in \(16 \mathrm{~g}\) of dioxygen.
Answer: The balanced reaction of combustion of carbon can be written as:
\(
\mathrm{C}(\mathrm{s})+\mathrm{O}_2(\mathrm{~g}) \rightarrow \mathrm{CO}_2(\mathrm{~g})
\)
(i) As per the balanced equation, 1 mole of carbon burns in 1 mole of dioxygen (air) to produce 44 gram of carbon dioxide.
(ii) Oxygen is acting as LIMITING REAGENT. it will react with 0.5 mole of carbon to give \(22 \mathrm{~g}\) of carbon dioxide.
(iii) According to the question, only \(16 \mathrm{~g}\) of dioxygen is available. It is a limiting reactant. Thus, \(16 \mathrm{~g}\) of dioxygen can combine with only \(0.5 \mathrm{~mole}\) of carbon to give \(22 \mathrm{~g}\) of carbon dioxide.
Q5. Calculate the mass of sodium acetate \(\left(\mathrm{CH}_3 \mathrm{COONa}\right)\) required to make \(500 \mathrm{~mL}\) of 0.375 molar aqueous solution. Molar mass of sodium acetate is \(82.0245 \mathrm{~g} \mathrm{~mol}^{-1}\).
Answer: \(0.375 \mathrm{M}\) aqueous solution of sodium acetate \(\equiv 1000 \mathrm{~mL}\) of solution containing 0.375 moles of sodium acetate Number of moles of sodium acetate in \(500 \mathrm{~mL}\) \(=\frac{0.375}{1000} \times 500\) \(=0.1857 \mathrm{~mole}\)
The molar mass of sodium acetate \(=82.0245 \mathrm{~g} \mathrm{~mol}^{-1}\) (Given) Required mass of sodium acetate \(=\left(82.0245 \mathrm{~g} \mathrm{~mol}^{-1}\right)(0.1875 \mathrm{~mole})\) \(=15.38 \mathrm{~g}\)
Q6. Calculate the concentration of nitric acid in moles per litre in a sample which has a density, \(1.41 \mathrm{~g} \mathrm{~mL}^{-1}\) and the mass percent of nitric acid in th being \(69 \%\).
Answer: The mass percent of nitric acid in the sample \(=69 \%\) Thus, \(100 \mathrm{~g}\) of nitric acid contains \(69 \mathrm{~g}\) of nitric acid by mass. Molar mass of nitric acid \(\mathrm{HNO_3}\)
\(
\begin{aligned}
& =\{1+14+3(16)\} \mathrm{g} \mathrm{~mL}^{-1} \\
& =1+14+48 \\
& =63 \mathrm{~g} \mathrm{~mL}^{-1}
\end{aligned}
\)
\(\therefore\) Number of moles in \(69 \mathrm{~g}\) of \(\mathrm{HNO_3}\)
\(
=\frac{69 \mathrm{~g}}{63 \mathrm{~g} \mathrm{~mol}^{-1}}=1.095 \mathrm{~mol}
\)
Volume of \(100 \mathrm{~g}\) of nitric acid solution
\(
\begin{aligned}
& =\frac{\text { Mass of solution }}{\text { density of solution }} \\
& =\frac{100 \mathrm{~g}}{1.41 \mathrm{gL}^{-1}} \\
& =70.92 \mathrm{~mL}=70.92 \times 10^{-3} \mathrm{~L}
\end{aligned}
\)
Concentration of nitric acid
\(
\begin{aligned}
& =\frac{1.095 \mathrm{~mole}}{70.92 \times 10^{-3} \mathrm{~L}} \\
& =15.44 \mathrm{~mol} / \mathrm{L} \\
& \therefore \text { Concentration of nitric acid }=15.44 \mathrm{~mol} / \mathrm{L}
\end{aligned}
\)
Q7. How much copper can be obtained from \(100 \mathrm{~g}\) of copper sulphate \(\left(\mathrm{CuSO}_4\right)\)?
Answer: 1 mole of \(\mathrm{CuSO}_4\) contains 1 mole of copper.
Molar mass of \(\mathrm{CuSO}_4=(63.5)+(32.00)+4(16.00)\)
\(
=63.5+32.00+64.00
\)
\(
=159.5 \mathrm{~g}
\)
\(159.5 \mathrm{~g}\) of \(\mathrm{CuSO}_4\) contains \(63.5 \mathrm{~g}\) of copper.
\(\Rightarrow 100 \mathrm{~g}\) of \(\mathrm{CuSO}_4\) will contain \(\frac{63.5 \times 100 \mathrm{~g}}{159.5}\) of copper.
Amount of copper that can be obtained from \(100 \mathrm{~g} \mathrm{CuSO} 4=\frac{63.05 \times 100}{159.5}\) \(=39.81 \mathrm{~g}\)
Q8. Determine the molecular formula of an oxide of iron, in which the mass percent of iron and oxygen are 69.9 and 30.1, respectively.
Answer: Mass percent of iron \((\mathrm{Fe})=69.9 \%\)
Mass percent of oxygen \((0)=30.1 \%\)
Number of moles of iron present in the oxide \(=\frac{69.90}{55.85}=1.25\)
A number of moles of oxygen present in the oxide \(=30.1 / 16.0\) \(=1.88\)
The ratio of iron to oxygen in the oxide,
\(
\begin{aligned}
& =1.25: 1.88 \\
& =\frac{1.25}{1.25}: \frac{1.88}{1.25} \\
& =1: 1.5 \\
& =2: 3
\end{aligned}
\)
\(\therefore\) The empirical formula of the oxide is \(\mathrm{Fe}_2 \mathrm{O}_3\).
Empirical formula mass of \(\mathrm{Fe} 2 \mathrm{O} 3=159.69 \mathrm{~g}\)
\(
\begin{aligned}
\therefore \mathrm{n} & =\frac{\text { Molar mass }}{\text { Empirical formula mass }}=\frac{159.69 \mathrm{~g}}{159.7 \mathrm{~g}} \\
& =0.999=1 \text { (approx) }
\end{aligned}
\)
The molecular formula of a compound is obtained by multiplying the empirical formula with \(\mathrm{n}\). Thus, the empirical formula of the given oxide is \(\mathrm{Fe}_2 \mathrm{O}_3\) and \(n\) is 1.
Hence, the molecular formula of the oxide is \(\mathrm{Fe}_2 \mathrm{O}_3\).
Q9.
\(\begin{aligned}
&\text { Calculate the atomic mass (average) of chlorine using the following data: }\\
&\begin{array}{ccc}
& \text { % Natural Abundance } & \text { Molar Mass } \\
{ }^{35} \mathrm{Cl} & 75.77 & 34.9689 \\
{ }^{37} \mathrm{Cl} & 24.23 & 36.9659
\end{array}
\end{aligned}
\)
Answer: The average atomic mass of chlorine
\(=[\) (Fractional abundance \()]\)
of \({ }_{35} \mathrm{Cl}(\) Molar mass \()\)
of \({ }_{35} \mathrm{Cl}+\) (Fractional)
abundance
of \({ }_{37} \mathrm{Cl}(\) Molar mass \()\)
of \({ }_{37} \mathrm{Cl}\)
\(
\begin{aligned}
& =\left[\left\{\left(\frac{75.77}{100}\right)(34.9689 \mathrm{u})\right\}+\left\{\left(\frac{24.23}{100}\right)(36.9659 \mathrm{u})\right\}\right] \\
& =26.4959+8.9568 \\
& =35.4527 \mathrm{u} \\
& \therefore \text { The average atomic mass of chlorine }=35.4527 \mathrm{u}
\end{aligned}
\)
Q10. In three moles of ethane \(\left(\mathrm{C}_2 \mathrm{H}_6\right)\), calculate the following:
(i) Number of moles of carbon atoms.
(ii) Number of moles of hydrogen atoms.
(iii) Number of molecules of ethane.
Answer: (i) 1 mole of \(\mathrm{C}_2 \mathrm{H}_6\) contains 2 moles of carbon atoms. Number of moles of carbon atoms in 3 moles of \(\mathrm{C}_2 \mathrm{H}_6\) \(=2 \times 3=6\)
(ii) 1 mole of \(\mathrm{C}_2 \mathrm{H}_6\) contains 6 moles of hydrogen atoms. Number of moles of carbon atoms in 3 moles of \(\mathrm{C}_2 \mathrm{H}_6\) \(=3 \times 6=18\)
(iii) 1 mole of \(\mathrm{C}_2 \mathrm{H}_6\) contains \(6.023 \times 10^{23}\) molecules of ethane.
Number of molecules in 3 moles of \(\mathrm{C}_2 \mathrm{H}_6\)
\(
=3 \times 6.023 \times 10^{23}=18.069 \times 10^{23}
\)
Q11. What is the concentration of sugar \(\left(\mathrm{C}_{12} \mathrm{H}_{22} \mathrm{O}_{11}\right)\) in mol \(\mathrm{L}^{-1}\) if its \(20 \mathrm{~g}\) are dissolved in enough water to make a final volume up to \(2 \mathrm{~L}\)?
Answer: The molarity (M) of a solution is given by,
\(
\begin{aligned}
& =\frac{\text { Number of moles of solute }}{\text { Volume of solution in liters }} \\
& =\frac{\text { Mass of sugar } / \text { molar mass of sugar }}{2 \mathrm{~L}} \\
& =\frac{20 \mathrm{~g} /[(12 \times 12)+(1 \times 22)+(11 \times 16) \mathrm{g}]}{2 \mathrm{~L}} \\
& =\frac{20 \mathrm{~g} / 342 \mathrm{~g}}{2 \mathrm{~L}} \\
& =\frac{0.0585 \mathrm{~mol}}{2 \mathrm{~L}} \\
& =0.02925 \mathrm{~mol} / \mathrm{L} \\
& \therefore \text { Molar concentration of sugar }=0.02925 \mathrm{~mol} / \mathrm{L}
\end{aligned}
\)
Q12. If the density of methanol is \(0.793 \mathrm{~kg} \mathrm{~L}^{-1}\), what is its volume needed for making \(2.5 \mathrm{~L}\) of its \(0.25 \mathrm{M}\) solution?
Answer:
\(
\begin{aligned}
& \text { Molar mass of methanol }\left(\mathrm{CH}_3 \mathrm{OH}\right)=(1 \times 12)+(4 \times 1)+(1 \times 16) \\
& =32 \mathrm{~g} \mathrm{~mol}^{-1} \\
& =0.032 \mathrm{~kg} \mathrm{~mol}^{-1}
\end{aligned}
\)
\(
\begin{aligned}
& \text { Molarity of methanol solution }=\frac{0.793 \mathrm{~kg} / \mathrm{L}}{0.032 \mathrm{~kg} / \mathrm{mol}} \\
& =24.78 \mathrm{~mol} / \mathrm{L}
\end{aligned}
\)
(Since density is mass per unit volume)
\(
\mathrm{M}_1 \mathrm{~V}_1=\mathrm{M}_2 \mathrm{~V}_2
\)
(Given solution) (Solution to be prepared)
\(
\begin{aligned}
& (24.78 \mathrm{~mol} / \mathrm{L}) \mathrm{V}_1=(0.25 \mathrm{~mol} / \mathrm{L})(2.5 \mathrm{~L}) \\
& \mathrm{V}_1=0.0252 \mathrm{~L} \\
& \mathrm{~V}_1=25.22 \mathrm{~mL}
\end{aligned}
\)
Q13. Pressure is determined as force per unit area of the surface. The SI unit of pressure, pascal is as shown below:
\(1 \mathrm{~Pa}=1 \mathrm{~N} \mathrm{~m}^{-2}\)
If the mass of air at sea level is \(1034 \mathrm{~g} \mathrm{~cm}^{-2}\), calculate the pressure in pascal.
Answer:
\(\begin{aligned}
& \text { Mass }=1034 \mathrm{~g}=1.034 \mathrm{~kg} \\
& \text { Force }=\mathrm{mg}=1.034 \times 9.81 \mathrm{~N}=10.143 \mathrm{~N} \\
& \text { area }=1 \mathrm{~cm}^2=1 \times 10^{-4} \mathrm{~m}^2 \\
& \text { Pressure }=\mathrm{F} / \mathrm{A}=10.134 / 1 \times 10^{-4} \mathrm{~N} / \mathrm{m}^2=1.013 \times 10^5 \mathrm{~N} / \mathrm{m}^2=1.013 \times 10^5 \mathrm{~Pa}
\end{aligned}
\)
Q14. What is the SI unit of mass? How is it defined?
Answer: The SI unit of mass is kilogram (kg). 1 Kilogram is defined as the mass equal to the mass of the international prototype of a kilogram.
Q15. Match the following prefixes with their multiples:
\(
\begin{array}{|l|l|}
\hline \text { Prefixes } & \text { Multiples } \\
\hline \text { 1. Micro } & 10^6 \\
\hline \text { 2. Deca } & 10^9 \\
\hline \text { 3. Mega } & 10^{-6} \\
\hline \text { 4. Giga } & 10^{-15} \\
\hline \text { 5. Femto } & 10 \\
\hline
\end{array}
\)
Answer:
\(\begin{array}{|l|l|}
\hline \text { Prefix } & \text { Multiples } \\
\hline \text { 1. micro } & 10^{-6} \\
\hline \text { 2. deca } & 10 \\
\hline \text { 3. mega } & 10^6 \\
\hline \text { 4. giga } & 10^9 \\
\hline \text { 5. femto } & 10^{-15} \\
\hline
\end{array}
\)
Q16. What do you mean by significant figures?
Answer: Significant figures are those meaningful digits that are known with certainty. ie, if \(15.6 \mathrm{~mL}\) is the result of an experiment, then 15 is certain while 6 is uncertain, and the total number of significant figures are 3. Hence, significant figures are defined as the total number of digits in a number including the last digit that represents the uncertainty of the result.
Q17. A sample of drinking water was found to be severely contaminated with chloroform, \(\mathrm{CHCl}_3\), supposed to be carcinogenic in nature. The level of contamination was 15 ppm (by mass).
(i) Express this in percent by mass.
(ii) Determine the molality of chloroform in the water sample.
Answer: \(15 \mathrm{ppm}\) means 15 parts in million \(\left(10^6\right)\) by mass in the solution
\(\therefore\) Percentage by mass \(=\frac{15}{10^6} \times 100=15 \times 10^{-4} \%\)
As only \(15 \mathrm{~g}\) of chloroform is present in \(10^6 \mathrm{~g}\) of the solution, mass of the solvent \(=10^6 \mathrm{~g}\)
Molar mass of \(\mathrm{CHCl}_3=12+1+3 \times 35 \cdot 5\)
\(=119 \cdot 5 \mathrm{~g} \mathrm{~mol}^{-1}\)
Moles of \(\mathrm{CHCl}_3=\frac{15}{119 \cdot 5}\)
\(\therefore\) Molarity \(=\frac{15 / 119 \cdot 5 \times 1000}{10^6}=1 \cdot 25 \times 10^{-4} \mathrm{~m}\)
Q18. Express the following in the scientific notation:
\(\begin{array}{ll}\text { (i) } & 0.0048 \\ \text { (ii) } & 234,000 \\ \text { (iii) } & 8008 \\ \text { (iv) } & 500.0 \\ \text { (v) } & 6.0012\end{array}\)
Answer: (i) \(0.0048=4.8 \times 10^{-3}\)
(ii) \(234,000=2.34 \times 10^5\)
(iii) \(8008=8.008 \times 10^3\)
(iv) \(500.0=5.000 \times 10^2\)
(v) \(6.0012=6.0012\)
Q19. How many significant figures are present in the following?
(i) 0.0025
(ii) 208
(iii) 5005
(iv) 126,000
(v) 500.0
(vi) 2.0034
Answer: (i) 0.0025
There are 2 significant figures.
(ii) 208
There are 3 significant figures.
(iii) 5005
There are 4 significant figures.
(iv) 126,000
There are 3 significant figures.
(v) 500.0
There are 4 significant figures.
(vi) 2.0034
There are 5 significant figures.
Q20. Round up the following upto three significant figures:
\(\begin{array}{ll}\text { (i) } & 34.216 \\ \text { (ii) } & 10.4107 \\ \text { (iii) } & 0.04597 \\ \text { (iv) } & 2808\end{array}\)
Answer: (i) 34.2
(ii) 10.4
(iii) 0.0460
(iv) 2810
Q21. The following data are obtained when dinitrogen and dioxygen react together to form different compounds:
\(\begin{array}{lcc}
& \text { Mass of dinitrogen } & \text { Mass of dioxygen } \\
\text { (1) } & 14 \mathrm{~g} & 16 \mathrm{~g} \\
\text { (11) } & 14 \mathrm{~g} & 32 \mathrm{~g} \\
\text { (111) } & 28 \mathrm{~g} & 32 \mathrm{~g} \\
\text { (1v) } & 28 \mathrm{~g} & 80 \mathrm{~g}
\end{array}
\)
(a) Which law of chemical combination is obeyed by the above experimental data? Give its statement.
(b) F1ll in the blanks in the following conversions:
(i) \(1 \mathrm{~km}=——\) \(\mathrm{mm}=——–\) \(\mathrm{pm}\)
(ii) \(1 \mathrm{mg}=——\) \(\mathrm{kg}=——-\) ng
(iii) \(1 \mathrm{~mL}=—-\) \(\mathrm{L}=———\) \(\mathrm{dm}^3\)
Answer: (a) for the fixed mass of dinitrogen at \(28 \mathrm{~g}\), then the masses of dioxygen that will combine with the fixed mass of dinitrogen are \(32 \mathrm{~g}, 64 \mathrm{~g}, 32 \mathrm{~g}\), and \(80 \mathrm{~g}\). The masses of dioxygen bear a whole number ratio of 1:2:2:5. Hence, the given experimental data obeys the law of multiple proportions. The law states that if two elements combine to form more than one compound, then the masses of one element that combine with the fixed mass of another element are in the ratio of small whole numbers.
(b)
\(
\begin{aligned}
& \text { (i) } 1 \mathrm{~km}=1 \mathrm{~km} \times \frac{1000 \mathrm{~m}}{1 \mathrm{~km}} \times \frac{100 \mathrm{~cm}}{1 \mathrm{~m}} \times \frac{10 \mathrm{~mm}}{1 \mathrm{~cm}} \\
& \therefore 1 \mathrm{~km}=10^6 \mathrm{~mm} \\
& 1 \mathrm{~km}=1 \mathrm{~km} \times \frac{1000 \mathrm{~m}}{1 \mathrm{~km}} \times \frac{1 \mathrm{pm}}{10^{-12} \mathrm{~m}} \\
& \therefore 1 \mathrm{~km}=10^{15} \mathrm{pm} \\
& \text { Hence, } 1 \mathrm{~km}=10^6 \mathrm{~mm}=10^{15} \mathrm{pm}
\end{aligned}
\)
\(
\begin{aligned}
& \text { (ii) } 1 \mathrm{mg}=1 \mathrm{mg} \times \frac{1 \mathrm{~g}}{1000 \mathrm{mg}} \times \frac{1 \mathrm{~kg}}{1000 \mathrm{~g}} \\
& \Rightarrow 1 \mathrm{mg}=10^{-6} \mathrm{~kg} \\
& 1 \mathrm{mg}=1 \mathrm{mg} \times \frac{1 \mathrm{~g}}{1000 \mathrm{mg}} \times \frac{1 \mathrm{ng}}{10^{-9} \mathrm{~g}} \\
& \Rightarrow 1 \mathrm{mg}=10^6 \mathrm{ng} \\
& \therefore 1 \mathrm{mg}=10^{-6} \mathrm{~kg}=10^6 \mathrm{ng}
\end{aligned}
\)
\(
\begin{aligned}
& \text { (iii) } 1 \mathrm{~mL}=1 \mathrm{~mL} \times \frac{1 \mathrm{~L}}{1000 \mathrm{~mL}} \\
& \Rightarrow 1 \mathrm{~mL}=10^{-3} \mathrm{~L} \\
& 1 \mathrm{~mL}=1 \mathrm{~cm}^3=1 \mathrm{~cm}^3 \frac{1 \mathrm{dm} \times 1 \mathrm{dm} \times 1 \mathrm{dm}}{10 \mathrm{~cm} \times 10 \mathrm{~cm} \times 10 \mathrm{~cm}} \\
& \Rightarrow 1 \mathrm{~mL}=10^{-3} \mathrm{dm}^3 \\
& \therefore 1 \mathrm{~mL}=10^{-3} \mathrm{~L}=10^{-3} \mathrm{dm}^3
\end{aligned}
\)
Q22. If the speed of light is \(3.0 \times 10^8 \mathrm{~m} \mathrm{~s}^{-1}\), calculate the distance covered by light in \(2.00 \mathrm{~ns}\).
Answer: According to the question:
Time is taken to cover the distance \(=2.00 \mathrm{~ns}\)
\(
=2.00 \times 10^{-9} \mathrm{~s}
\)
Speed of light \(=3.0 \times 10^8 \mathrm{~m} / \mathrm{s}\)
Distance traveled by light in \(2.00 \mathrm{~ns}\)
\(=\) Speed of light \(\times\) Time taken
\(
\begin{aligned}
& =(3.0 \times 108 \mathrm{~m} / \mathrm{s})\left(2.00 \times 10^{-9} \mathrm{~s}\right) \\
& =6.00 \times 10^{-1} \mathrm{~m} \\
& =0.600 \mathrm{~m}
\end{aligned}
\)
Q23. In a reaction
\(
\mathrm{A}+\mathrm{B}_2 \rightarrow \mathrm{AB}_2
\)
Identify the limiting reagent, if any, in the following reaction mixtures.
(i) 300 atoms of \(A+200\) molecules of \(B\)
(ii) \(2 \mathrm{~mol} \mathrm{~A}+3 \mathrm{~mol} \mathrm{~B}\)
(iii) 100 atoms of \(\mathrm{A}+100\) molecules of \(\mathrm{B}\)
(iv) \(5 \mathrm{~mol} \mathrm{~A}+2.5 \mathrm{~mol} \mathrm{~B}\)
(v) \(\quad 2.5 \mathrm{~mol} \mathrm{~A}+5 \mathrm{~mol} \mathrm{~B}\)
Answer: A limiting reagent determines the extent of a reaction.
(i) As per the given reaction, 1 atom of A reacts with 1 molecule of \(B\). Thus, 200 molecules of \(B\) will react with 200 atoms of A, thereby leaving 100 atoms of \(A\) unused. Hence, \(B\) is the limiting reagent.
(ii) As per given the reaction, 1 mole of A reacts with 1 mole of \(B\). Thus, 2 moles of A will react with only 2 moles of B. As a result, 1 mole of A will not be consumed. Hence, \(A\) is the limiting reagent.
(iii) According to the given reaction, 1 atom of A combines with 1 molecule of \(B\). Thus, all 100 atoms of \(A\) will combine with all 100 molecules of \(B\). Hence, the mixture is stoichiometric where no limiting reagent is present.
(iv) 1 mole of atom A combines with 1 mole of molecule B. Thus, 2.5 moles of B will combine with only 2.5 moles of A. As a result, 2.5 moles of A will be left as such. Hence, \(B\) is the limiting reagent.
(v) According to the reaction, 1 mole of atom A combines with 1 mole of molecule B. Thus, 2.5 mole of A will combine with only 2.5 mole of B and the remaining 2.5 mole of \(B\) will be left as such. Hence, \(A\) is the limiting reagent.
Q24. Dinitrogen and dihydrogen react with each other to produce ammonia according to the following chemical equation:
\(
\mathrm{N}_2(\mathrm{~g})+\mathrm{H}_2(\mathrm{~g}) \rightarrow 2 \mathrm{NH}_3(\mathrm{~g})
\)
(i) Calculate the mass of ammonia produced if \(2.00 \times 10^3 \mathrm{~g}\) dinitrogen reacts with \(1.00 \times 10^3 \mathrm{~g}\) of dihydrogen.
(ii) Will any of the two reactants remain unreacted?
(iii) If yes, which one and what would be its mass?
Answer:
(i) Balancing the given chemical equation, \(
\mathrm{N}_2(\mathrm{~g})+\mathrm{H}_2(\mathrm{~g}) \rightarrow 2 \mathrm{NH}_3(\mathrm{~g})
\)
\(
\text { From the equation, } 1 \text { mole ( } 28 \mathrm{~g} \text { ) of dinitrogen reacts with } 3 \text { moles }(6 \mathrm{~g}) \text { of dihydrogen to give } 2 \text { moles }(34 \mathrm{~g}) \text { of ammonia. }
\)
\(
\Rightarrow 2.00 \times 10^3 \mathrm{~g} \text { of dinitrogen will react with } \frac{6 \mathrm{~g}}{28 \mathrm{~g}} \times 2.00 \times 10^3 \mathrm{~g} \text { dihydrogen i.e., } 2.00 \times 10^3 \mathrm{~g} \text { of dinitrogen will react with } 428.6 \mathrm{~g} \text { of dihydrogen. }
\)
\(
\text { Given, the Amount of dihydrogen }=1.00 \times 10^3 \mathrm{~g} \text { Hence, }
\)
\(\mathrm{N_2}\) is the limiting reagent. \(28 \mathrm{~g}\) of \(\mathrm{N_2}\) produces \(34 \mathrm{~g}\) of \(\mathrm{NH}_3\).
Hence, the mass of ammonia produced by \(2000 \mathrm{~g}\) of \(\mathrm{N_2}\) \(\frac{34 \mathrm{~g}}{28 \mathrm{~g}} \times 2000 \mathrm{~g}=2428.57 \mathrm{~g}\)
(ii) \(\mathrm{N_2}\) is the limiting reagent and \(\mathrm{H_2}\) is the excess reagent. Hence, \(\mathrm{H_2}\) will remain unreacted.
(iii) Mass of dihydrogen left unreacted \(=1.00 \times 10^3 \mathrm{~g}-428.6 \mathrm{~g}=571.4 \mathrm{~g}\)
Q25. How are \(0.50 \mathrm{~mol} \mathrm{~Na}_2 \mathrm{CO}_3\) and \(0.50 \mathrm{~M} \mathrm{~Na}_2 \mathrm{CO}_3\) different?
Answer:
\(
\text { Molar mass of } \mathrm{Na}_2 \mathrm{CO}_3=(2 \times 23)+12.00+(3 \times 16)=106 \mathrm{~g} \mathrm{~mol}^{-1}
\)
\(
\text { Now, } 1 \text { mole of } \mathrm{Na}_2 \mathrm{CO}_3 \text { means } 106 \text { g of } \mathrm{Na}_2 \mathrm{CO}_3 \text {. }
\)
\(
0.5 \mathrm{~mol} \text { of } \mathrm{Na}_2 \mathrm{CO}_3=\frac{106 \mathrm{~g}}{1 \mathrm{~mole}} \times 0.5 \mathrm{~mol} \mathrm{~Na}_2 \mathrm{CO}_3
\)
\(
=53 \mathrm{~g} \mathrm{Na}_2 \mathrm{CO}_3
\)
\(
\Rightarrow 0.50 \mathrm{M} \text { of } \mathrm{Na}_2 \mathrm{CO}_3=0.50 \mathrm{~mol} / \mathrm{L} \mathrm{~Na}_2 \mathrm{CO}_3
\)
\(
\text { Hence, } 0.50 \mathrm{~mol} \text { of } \mathrm{Na}_2 \mathrm{CO}_3 \text { is present in } 1 \mathrm{~L} \text { of water or } 53 \mathrm{~g} \text { of } \mathrm{Na}_2 \mathrm{CO}_3 \text { is present in } 1 \mathrm{~L} \text { of water }
\)
Q26. If 10 volumes of dihydrogen gas reacts with five volumes of dioxygen gas, how many volumes of water vapour would be produced?
Answer: The reaction of dihydrogen with dioxygen can be written as:
\(
\mathrm{2H}_2(\mathrm{~g})+\mathrm{O}_2(\mathrm{~g}) \rightarrow 2 \mathrm{H_2O}(\mathrm{~g})
\)
Now, two volumes of dihydrogen react with one volume of dihydrogen to produce two volumes of water vapour. Hence, ten volumes of dihydrogen will react with five volumes of dioxygen to produce ten volumes of water vapour.
Q27.
\(\begin{aligned}
&\text { Convert the following into basic units: }\\
&\begin{array}{ll}
\text { (1) } & 28.7 \mathrm{pm} \\
\text { (11) } & 15.15 \mathrm{pm} \\
\text { (111) } & 25365 \mathrm{mg}
\end{array}
\end{aligned}
\)
Answer: (i) \(28.7 \mathrm{pm}\) :
\(
\begin{aligned}
& 1 \mathrm{pm}=10^{-12} \mathrm{~m} \\
& \therefore 28.7 \mathrm{pm}=28.7 \times 10^{-12} \mathrm{~m} \\
& =2.87 \times 10^{-11} \mathrm{~m}
\end{aligned}
\)
(ii) \(15.15 \mathrm{pm}\) :
\(
\begin{aligned}
& 1 \mathrm{pm}=10^{-12} \mathrm{~m} \\
& \therefore 15.15 \mathrm{pm}=15.15 \times 10^{-12} \mathrm{~m} \\
& =1.515 \times 10^{-11} \mathrm{~m}
\end{aligned}
\)
(iii) \(25365 \mathrm{mg}\) :
\(
\begin{aligned}
& 1 \mathrm{mg}=10^{-3} \mathrm{~g} \\
& 25365 \mathrm{mg}=2.5365 \times 104 \times 10^{-3} \mathrm{~g} \text { Since, } \\
& 1 \mathrm{~g}=10^{-3} \mathrm{~kg} \\
& 2.5365 \times 101 \mathrm{~g}=2.5365 \times 10^{-1} \times 10^{-3} \mathrm{~kg} \\
& \therefore 25365 \mathrm{mg}=2.5365 \times 10^{-2} \mathrm{~kg}
\end{aligned}
\)
Q28. Which one of the following will have the largest number of atoms?
(i) \(1 \mathrm{~g} \mathrm{Au}(\mathrm{s})\)
(ii) \(1 \mathrm{~g} \mathrm{Na}\) (s)
(iii) \(1 \mathrm{~g} \mathrm{Li}\) (s)
(iv) \(1 \mathrm{~g}\) of \(\mathrm{Cl}_2\) (g)
Answer:
\(
\begin{aligned}
& \text { (i). } 1 \mathrm{~g} \text { of } \mathrm{Au}(\mathrm{s})=1 / 197 \mathrm{~mol} \text { of } \mathrm{Au}(\mathrm{s}) \\
& =\frac{6.022 \times 10^{23}}{197} \text { atoms of } \mathrm{Au}(\mathrm{s}) \\
& =3.06 \times 10^{21} \text { atoms of } \mathrm{Au}(\mathrm{s})
\end{aligned}
\)
(ii). \(1 \mathrm{~g}\) of \(\mathrm{Na}(\mathrm{s})=1 / 23 \mathrm{~mol}\) of \(\mathrm{Na}(\mathrm{s})\) \(=\frac{6.022 \times 10^{23}}{23}\) atoms of \(\mathrm{Na}(\mathrm{s})\) \(=0.262 \times 10^{23}\) atoms of \(\mathrm{Na}(\mathrm{s})\) \(=26.2 \times 10^{21}\) atoms of \(\mathrm{Na}(\mathrm{s})\)
(iii). \(1 \mathrm{~g}\) of \(\mathrm{Li}(\mathrm{s})=1 / 7 \mathrm{~mol}\) of \(\mathrm{Li}(\mathrm{s})\) \(=\frac{6.022 \times 10^{23}}{7}\) atoms of \(\mathrm{Li}(\mathrm{s})\) \(=0.86 \times 10^{23}\) atoms of \(\mathrm{Li}(\mathrm{s})\) \(=86.0 \times 10^{21}\) atoms of \(\mathrm{Li}(\mathrm{s})\)
(iv). \(1 \mathrm{~g}\) of \(\mathrm{Cl_2}(\mathrm{~g})=1 / 71 \mathrm{~mol}\) of \(\mathrm{Cl_2}\) (g)
(Molar mass of \(\mathrm{Cl_2}\) molecule \(=35.5 \times 2=71 \mathrm{~g} \mathrm{~mol}^{-1}\) )
\(
\begin{aligned}
& =\frac{6.022 \times 10^{23}}{71} \text { atoms of } \mathrm{Cl_2}(\mathrm{~g}) \\
& =0.0848 \times 10^{23} \text { atoms of } \mathrm{Cl_2}(\mathrm{~g}) \\
& =8.48 \times 10^{21} \text { atoms of } \mathrm{Cl_2}(\mathrm{~g})
\end{aligned}
\)
Hence, \(1 \mathrm{~g}\) of \(\mathrm{Li}(\mathrm{s})\) will have the largest number of atoms.
Q29. Calculate the molarity of a solution of ethanol in water, in which the mole fraction of ethanol is 0.040 (assume the density of water to be one).
Answer:
\(
\text { Mole fraction of } \mathrm{C}_2 \mathrm{H}_5 \mathrm{OH}=\frac{\text { Number of moles of } \mathrm{C}_2 \mathrm{H}_5 \mathrm{OH}}{\text { Number of moles of solution }}
\)
\(
0.040=\frac{\mathrm{n}_{\mathrm{C}_2 \mathrm{H}_5 \mathrm{OH}}}{\mathrm{n}_{\mathrm{C}_2 \mathrm{H}_5} \mathrm{OH}+\mathrm{n}_{\mathrm{H}_2 \mathrm{O}}} \ldots . . \text { (i) }
\)
Number of moles present in \(1 \mathrm{~L}\) water :
\(
\begin{aligned}
& \mathrm{n}_{\mathrm{H}_2 \mathrm{O}}=\frac{1000 \mathrm{~g}}{18 \mathrm{~g} \mathrm{~mol}^{-1}} \\
& \mathrm{n}_{\mathrm{H}_2 \mathrm{O}}=55.55 \mathrm{~mol}
\end{aligned}
\)
Substituting the value of \(\mathrm{n}_{\mathrm{H}_2 \mathrm{O}}\) in equation (i),
\(
\frac{\mathrm{n}_{\mathrm{c}_2 \mathrm{H}_5 \mathrm{OH}}}{\mathrm{n}_{\mathrm{c}_2 \mathrm{H}_5 \mathrm{OH}}+55.55}=0.040
\)
\(
\mathrm{n}_{\mathrm{c}_2 \mathrm{H}_5 \mathrm{OH}}=0.040 \mathrm{n}_{\mathrm{c}_2 \mathrm{H}_5 \mathrm{OH}}+(0.404)(55.55)
\)
\(
\begin{aligned}
& 0.96 \mathrm{n}_{\mathrm{c}_2 \mathrm{H}_5 \mathrm{OH}}=2.222 \mathrm{~mol} \\
& \mathrm{n}_{\mathrm{c}_2 \mathrm{H}_5 \mathrm{OH}}=\frac{2.222}{0.96} \mathrm{~mol} \\
& \mathrm{n}_{\mathrm{c}_2 \mathrm{H}_5 \mathrm{OH}}=2.314 \mathrm{~mol} \\
& \therefore \text { Molarity of solution }=\frac{2.314 \mathrm{~mol}}{1 \mathrm{~L}} \\
& =2.314 \mathrm{M}
\end{aligned}
\)
Q30. What will be the mass of one \({ }^{12} \mathrm{C}\) atom in g?
Answer:
\(\begin{aligned}
& 1 \text { mole of carbon atoms }=6.023 \times 1023 \text { atoms of carbon } \\
& =12 \mathrm{~g} \text { of carbon } \\
& \therefore \text { Mass of one }{ }^{12} \mathrm{C} \text { atom }=\frac{12 \mathrm{~g}}{6.022 \times 10^{23}} \\
& =1.993 \times 10^{-23} \mathrm{~g}
\end{aligned}
\)
Q31. How many significant figures should be present in the answer of the following calculations?
(i) \(\frac{0.02856 \times 298.15 \times 0.112}{0.5785}\)
(ii) \(5 \times 5.364\)
(iii) \(\quad 0.0125+0.7864+0.0215\)
Answer: (i) \(\frac{0.02856 \times 298.15 \times 0.112}{0.5785}\)
The least precise number of calculations \(=0.112\)
Number of significant figures in the answer
\(=\) Number of significant figures in the least precise number \(=3\)
(ii) \(5 \times 5.364\)
The least precise number of calculations \(=5.364\)
Number of significant figures in the answer \(=\) Number of significant figures in 5.364 \(=4\)
(iii) \(0.0125+0.7864+0.0215\)
Since the least number of decimal places in each term is four, the number of significant figures in the answer is also 4.
Q32. Use the data given in the following table to calculate the molar mass of naturally occurring argon isotopes:
\(
\begin{array}{lll}
\text { Isotope } & \text { Isotopic molar mass } & \text { Abundance } \\
{ }^{36} \mathrm{Ar} & 35.96755 \mathrm{~g} \mathrm{~mol}^{-1} & 0.337 \% \\
{ }^{38} \mathrm{Ar} & 37.96272 \mathrm{~g} \mathrm{~mol}^{-1} & 0.063 \% \\
{ }^{40} \mathrm{Ar} & 39.9624 \mathrm{~g} \mathrm{~mol}^{-1} & 99.600 \%
\end{array}
\)
Answer: Molar mass of argon
\(
\begin{aligned}
& =\left[\left(35.96755 \times \frac{0.337}{100}\right)+\left(37.96272 \times \frac{0.063}{100}\right)+\left(39.9624 \times \frac{99.60}{100}\right)\right] \mathrm{gmol}^{-1} \\
& =[0.121+0.024+39.802] \mathrm{gmol}^{-1} \\
& =39.947 \mathrm{~g} \mathrm{mol}^{-1}
\end{aligned}
\)
Q33. Calculate the number of atoms in each of the following (i) 52 moles of Ar (ii) \(52 \mathrm{u}\) of \(\mathrm{He}(iii) 52 \mathrm{~g}\) of \(\mathrm{He}\).
Answer: (i) 1 mole of \(\mathrm{Ar}=6.022 \times 10^{23}\) atoms of \(\mathrm{Ar}\)
\(\therefore 52 \mathrm{~mol}\) of \(\mathrm{Ar}=52 \times 6.022 \times 10^{23}\) atoms of \(\mathrm{Ar}\)
\(=3.131 \times 10^{25}\) atoms of \(\mathrm{Ar}\)
(ii) 1 atom of \(\mathrm{He}=4 \mathrm{u}\) of \(\mathrm{He}\)
or,
\(4 \mathrm{u}\) of \(\mathrm{He}=1\) atom of \(\mathrm{He}\)
\(1 \mathrm{u}\) of \(\mathrm{He}=1 / 4\) atom of \(\mathrm{He}\)
\(52 \mathrm{u}\) of \(\mathrm{He}=52 / 4\) atom of \(\mathrm{He}\)
\(=13\) atoms of \(\mathrm{He}\)
(iii) \(4 \mathrm{~g}\) of \(\mathrm{He}=6.022 \times 1023\) atoms of \(\mathrm{He}\)
\(\therefore 52 \mathrm{~g}\) of He\(=\frac{6.0222 \times 10^{21} \times 52}{4}\) atoms of \(\mathrm{He}\)
\(=7.8286 \times 10^{24}\) atoms of \(\mathrm{He}\)
Q34. A welding fuel gas contains carbon and hydrogen only. Burning a small sample of it in oxygen gives \(3.38 \mathrm{~g}\) carbon dioxide, \(0.690 \mathrm{~g}\) of water and no other products. A volume of \(10.0 \mathrm{~L}\) (measured at STP) of this welding gas is found to weigh \(11.6 \mathrm{~g}\). Calculate (i) the empirical formula, (ii) the molar mass of the gas, and (iii) the molecular formula.
Answer: (i) 1 mole ( \(44 \mathrm{~g}\) ) of \(\mathrm{CO} 2\) contains \(12 \mathrm{~g}\) of carbon.
\(
\begin{aligned}
& \therefore 3.38 \mathrm{~g} \text { of } \mathrm{CO} 2 \text { will contain carbon }=\frac{12 \mathrm{~g}}{44 \mathrm{~g}} \times 3.38 \mathrm{~g} \\
& =0.9217 \mathrm{~g} \\
& 18 \mathrm{~g} \text { of water contains } 2 \mathrm{~g} \text { of hydrogen. } \\
& \therefore 0.690 \mathrm{~g} \text { of water will contain hydrogen }=\frac{2 \mathrm{~g}}{18 \mathrm{~g}} \times 0.690 \\
& =0.0767 \mathrm{~g}
\end{aligned}
\)
Since carbon and hydrogen are the only constituents of the compound, the total mass of the compound is:
\(
\begin{aligned}
& =0.9217 \mathrm{~g}+0.0767 \mathrm{~g} \\
& =0.9984 \mathrm{~g} \\
& \therefore \text { Percent of } \mathrm{C} \text { in the compound }=\frac{0.9217 \mathrm{~g}}{0.9984 \mathrm{~g}} \times 100 \\
& =92.32 \%
\end{aligned}
\)
Percent of \(\mathrm{H}\) in the compound \(=\frac{0.0767 \mathrm{~g}}{0.9984 \mathrm{~g}} \times 100\)
\(
=7.68 \%
\)
Moles of carbon in the compound \(=\frac{92.32}{12.00}\)
\(
=7.69
\)
Moles of hydrogen in the compound \(=\frac{7.68}{1}\)
\(
=7.68
\)
\(\therefore\) Ratio of carbon to hydrogen in the compound \(=7.69: 7.68=1: 1\)
Hence, the empirical formula of the gas is \(\mathrm{CH}\).
(ii) Given,
\(
\begin{aligned}
& \text { Weight of } 10.0 \mathrm{~L} \text { of the gas (at S.T.P) }=11.6 \mathrm{~g} \\
& \therefore \text { Weight of } 22.4 \mathrm{~L} \text { of gas at STP }=\frac{11.6 \mathrm{~g}}{10.0 \mathrm{~L}} \times 22.4 \\
& \approx 26.0 \mathrm{~g} \mathrm{~mol}^{-1}
\end{aligned}
\)
Hence,\(
\text { molar mass } 26.0 \mathrm{~g} \mathrm{~mol}^{-1}
\)
(iii) Empirical formula mass of \(\mathrm{CH}=12+1=13 \mathrm{~g}\)
\(
\begin{aligned}
& \mathbf{n}=\frac{\text { Molar mass of gas }}{\text { Empirical formula mass of gas }} \\
& =\frac{26 \mathrm{~g}}{13 \mathrm{~g}} \\
& \mathrm{n}=2 \\
& \therefore \text { Molecular formula of gas }=(\mathrm{CH})_{\mathrm{n}} \\
& =\mathrm{C}_2 \mathrm{H}_2
\end{aligned}
\)
Q35. Calcium carbonate reacts with aqueous \(\mathrm{HCl}\) to give \(\mathrm{CaCl}_2\) and \(\mathrm{CO}_2\) according to the reaction, \(\mathrm{CaCO}_3(\mathrm{~s})+2 \mathrm{HCl}(\mathrm{aq}) \rightarrow \mathrm{CaCl}_2(\mathrm{aq})+\mathrm{CO}_2(\mathrm{~g})+\mathrm{H}_2 \mathrm{O}(\mathrm{l})\)
\(
\text { What mass of } \mathrm{CaCO}_3 \text { is required to react completely with } 25 \mathrm{~mL} \text { of } 0.75 \mathrm{M} \mathrm{~HCl} \text { ? }
\)
Answer: The given equation is
\(
\mathrm{CaCO}_3(\mathrm{~s})+2 \mathrm{HCl}(\mathrm{aq}) \rightarrow \mathrm{CaCl}_2(\mathrm{aq})+\mathrm{CO}_2(\mathrm{~g})+\mathrm{H}_2 \mathrm{O}(\mathrm{l})
\)
Number of moles of \(\mathrm{HCl}=\) Molarity \(\times\) Volume
\(
=0.75 \mathrm{M} \times 0.025 \mathrm{~L}=0.01875 \mathrm{~mol}
\)
As, the mole ratio between \(\mathrm{CaCO}_3\) and \(\mathrm{HCl}\) is \(1: 2\). So, the number of moles of \(\mathrm{CaCO}_3\) should be half of the number of moles of \(\mathrm{HCl}\)
Number of moles of \(\mathrm{CaCO}_3=\frac{1}{2} \times 0.01875 \mathrm{~mol}=0.009375 \mathrm{~mol}\)
Molar mass of \(\mathrm{CaCO}_3=100 \mathrm{~g} / \mathrm{mol}\)
Mass of Calcium Carbonate \(=\) Moles \(\times\) Molar mass
\(=0.009375 \mathrm{~mol} \times 100 \mathrm{~g} / \mathrm{mol}=0.9375 \mathrm{~g} \approx 0.94 \mathrm{~g} \mathrm{~CaCO}_3\)
Q36. Chlorine is prepared in the laboratory by treating manganese dioxide \(\left(\mathrm{MnO}_2\right)\) with aqueous hydrochloric acid according to the reaction
\(
4 \mathrm{HCl}(\mathrm{aq})+\mathrm{MnO}_2(\mathrm{~s}) \rightarrow 2 \mathrm{H}_2 \mathrm{O}(\mathrm{l})+\mathrm{MnCl}_2(\mathrm{aq})+\mathrm{Cl}_2(\mathrm{~g})
\)
\(
\text { How many grams of } \mathrm{HCl} \text { react with } 5.0 \mathrm{~g} \text { of manganese dioxide? }
\)
Answer: \(1 \mathrm{~mol}[55+2 \times 16=87 \mathrm{~g}] \mathrm{MnO}_2\) reacts completely with \(4 \mathrm{~mol}[4 \times 36.5=146 \mathrm{~g}]\) of \(\mathrm{HCl}\).
\(5.0 \mathrm{~g}\) of \(\mathrm{MnO}_2\) will react with
\(
=\frac{146 \mathrm{~g}}{87 \mathrm{~g}} \times 5.0 \mathrm{~g} \text { of } \mathrm{HCl}
\)
\(
=8.4 \mathrm{~g} \text { of } \mathrm{HCl}
\)
Hence, \(8.4 \mathrm{~g}\) of \(\mathrm{HCl}\) will react completely with \(5.0 \mathrm{~g}\) of manganese dioxide.
Q37. Hydrogen gas is prepared in the laboratory by reacting dilute \(\mathrm{HCl}\) with granulated zinc. The following reaction takes place
\(
\mathrm{Zn}+2 \mathrm{HCl} \rightarrow \mathrm{ZnCl}_2+\mathrm{H}_2
\)
Calculate the volume of hydrogen gas liberated at STP when \(32.65 \mathrm{~g}\) of zinc reacts with \(\mathrm{HCl}\). 1 mol of a gas occupies \(22.7 \mathrm{~L}\) volume at STP; atomic mass of \(Z n=65.3 u\)
Answer: Given that, the mass of \(Z n=32.65 \mathrm{~g}\)
1 mole of gas occupies \(=22.7 \mathrm{~L}\) volume at STP
Atomic mass of \(\mathrm{Zn}=65.3 \mathrm{u}\)
The given equation is
\(
\underset{65.3 \mathrm{~g}}{\mathrm{Zn}}+2 \mathrm{HCl} \rightarrow \mathrm{ZnCl}_2+\underset{1 \text { mol=22.7 L at STP }}{\mathrm{H}_2}
\)
From the above equation, it is clear that \(65.3 \mathrm{~g} \mathrm{~Zn}\), when reacts with \(\mathrm{HCl}\), produces \(=22.7 \mathrm{~L}\) of \(\mathrm{H}_2\) at STP \(\therefore 32.65 \mathrm{~g} \mathrm{~Zn}\), when reacts with \(\mathrm{HCl}\), will produce \(=\frac{22.7 \times 32.65}{65.3}=11.35 \mathrm{~L}\) of \(\mathrm{H}_2\) at STP
Q38. The density of 3 molal solution of \(\mathrm{NaOH}\) is \(1.110 \mathrm{~g} \mathrm{~mL}^{-1}\). Calculate the molarity of the solution.
Answer: 3 molal solution of \(\mathrm{NaOH}\) means 3 moles of \(\mathrm{NaOH}\) are dissolved in \(1 \mathrm{~kg}\) solvent. So, the mass of solution \(=1000 \mathrm{~g}\) solvent \(+120 \mathrm{~g} \mathrm{NaOH}=1120 \mathrm{~g}\) solution (Molar mass of \(\mathrm{NaOH}=23+16+1=40 \mathrm{~g}\) and 3 moles of \(\mathrm{NaOH}=3 \times 40=120 \mathrm{~g}\) )
\(
\text { Volume of solution }=\frac{\text { Mass of solution }}{\text { Density of solution }}
\)
\(
\begin{aligned}
& \mathrm{V}=\frac{1120 \mathrm{~g}}{1.110 \mathrm{~g} \mathrm{~mL}^{-1}}=1009 \mathrm{~mL} \\
& \text { Molarity }=\frac{\text { Moles of solute } \times 1000}{\text { Volume of solution }(\mathrm{mL})} \\
& =\frac{3 \times 1000}{1009}=2.973 \mathrm{M} \approx 3 \mathrm{M}
\end{aligned}
\)
Q39. The Volume of a solution changes with change in temperature, then what will the molality of the solution be affected by temperature? Give a reason for your answer.
Answer: No, the molality of the solution does not change with temperature since mass remains unaffected with temperature.
Molality, \(\mathrm{m}=\frac{\text { moles of solute }}{\text { weight of solvent (in g) }} \times 1000\)
Q40. If \(4 \mathrm{~g}\) of \(\mathrm{NaOH}\) dissolves in \(36 \mathrm{~g}\) of \(\mathrm{H}_2 \mathrm{O}\), calculate the mole fraction of each component in the solution. Also, determine the molarity of the solution (specific gravity of solution is \(1 \mathrm{~g} \mathrm{~mL}^{-1}\) ).
Answer: Number of moles of \(\mathrm{NaOH}\),
\(
\mathrm{n}_{\mathrm{NaOH}}=\frac{4}{40}=0.1 \mathrm{~mol} \quad\left\{\because \mathrm{n}=\frac{\text { Mass }(\mathrm{g})}{\text { Molar mass }\left(\mathrm{g} \mathrm{mol}^{-1}\right)}\right\}
\)
\(
\text { Similarly, } \mathbf{n}_{\mathrm{H}_2 \mathrm{O}}=\frac{36}{18}=2 \mathrm{~mol}
\)
Mole fraction of \(\mathrm{NaOH}, \mathrm{X}_{\mathrm{NaOH}}=\frac{\text { moles of } \mathrm{NaOH}}{\text { moles of } \mathrm{NaOH}+\text { moles of } \mathrm{H}_2 \mathrm{O}}\) \(\mathrm{X}_{\mathrm{NaOH}}=\frac{0.1}{0.1+2}=0.0476\)
Similarly, \(\mathrm{X}_{\mathrm{H}_2 \mathrm{O}}=\frac{\mathrm{n}_{\mathrm{H}_2 \mathrm{O}}}{\mathrm{n}_{\mathrm{NaOH}}+\mathrm{n}_{\mathrm{H}_2 \mathrm{O}}}\) \(=\frac{2}{0.1+2}=0.9524\)
Total mass of solution \(=\) mass of solute + mass of solvent \(=4+36=40 \mathrm{~g}\)
Volume of solution \(=\frac{\text { Mass of solution }}{\text { specific gravity }}=\frac{40 \mathrm{~g}}{1 \mathrm{~g} \mathrm{~mL}^{-1}}=40 \mathrm{~mL}\)
Molarity \(=\frac{\text { Moles of solute } \times 1000}{\text { Volume of solution }(\mathrm{mL})}\)
\(=\frac{0.1 \times 1000}{40}=2.5 \mathrm{M}\)
Q41. The reactant which is entirely consumed in the reaction is known as a limiting reagent. In the reaction \(2 \mathrm{~A}+4 \mathrm{~B} \rightarrow 3 \mathrm{C}+4 \mathrm{D}\), when 5 moles of \(\mathrm{A}\) react with 6 moles of \(B\), then
(a) which is the limiting reagent?
(b) calculate the amount of \(\mathrm{C}\) formed.
Answer: The reactant that is entirely consumed in the reaction is known as limiting reagent. In the reaction
\(2 \mathrm{~A}+4 \mathrm{~B} \rightarrow 3 \mathrm{C}+4 \mathrm{D}
\)
According to the given reaction, 2 moles of A react with 4 moles of \(B\).
Hence, 5 moles of A will react with 10 moles of \(B\left(\frac{5 \times 4}{2}=10\right.\) moles \()\)
(a) It indicates that reactant B is limiting reagent as it will consume first in the reaction because we have only 6 moles of B.
(b) Limiting reagent decide the amount of product produced.
According to the reaction,
4 moles of \(B\) produces 3 moles of \(C\)
\(\therefore 6\) moles of \(B\) will produce \(\frac{3 \times 6}{4}=4.5\) moles of \(C\).
Q42. Match the following:
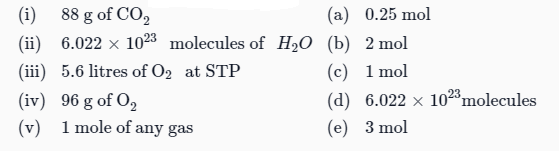
Answer: The correct answer is (i)-(b), (ii)-(c), (iii)-(a), (iv)-(e), (v)-(d)
\(88 \mathrm{~g} \mathrm{CO}_2=88 / 44 \mathrm{~mol}=2 \mathrm{~mol}\). Hence, (i)-(b).
\(6.022 \times 10^{23}\) molecules of \(\mathrm{H}_2 \mathrm{O}=1 \mathrm{~mol}\). Hence, (ii)-(b)
\(5.6 \mathrm{~L}\) of \(\mathrm{O}_2\) at \(\mathrm{STP}=5.6 / 22.4 \mathrm{~mol}=0.25 \mathrm{~mol}\). Hence, (iii)-(a)
96 go of \(\mathrm{O}_2=96 / 32 \mathrm{~mol}=3 \mathrm{~mol}\). Hence, (iv)-(e).
\(1 \mathrm{~mol}\) of any gas \(=6.022 \times 10^{23}\) molecules. Hence, \((\mathrm{v})-(\mathrm{d})\)
Q43. Match the following:
\(\begin{array}{|c|c|}
\hline \text { Physical quantity } & \text { UnIt } \\
\hline \text { (i) Molarity } & \text { (a) } \mathrm{g} \mathrm{~mL}^{-1} \\
\hline \text { (ii) Mole fraction } & \text { (b) mol } \\
\hline \text { (iii) Mole } & \text { (c) Pascal } \\
\hline \text { (iv) Molality } & \text { (d) Unitless } \\
\hline \text { (v) Pressure } & \text { (e) } \mathrm{mol} \mathrm{~L}^{-1} \\
\hline \text { (vi) Luminous intensity } & \text { (f) Candela } \\
\hline \text { (vii) Density } & \text { (g) } \mathrm{mol} \mathrm{~kg}^{-1} \\
\hline {\text { (viii) Mass }} & \text { (h) } \mathrm{Nm}^{-1} \\
\hline & \text { (i) } \mathrm{kg} \\
\hline
\end{array}
\)
Answer:
\(\begin{array}{|l|l|}
\hline {\text { Physical quantity }} & {\text { Unit }} \\
\hline \text { (i) Molarity } & \text { (e) } \mathrm{mol} \mathrm{~L}^{-1} \\
\hline \text { (ii) Mole fraction } & \text { (d) Unitless } \\
\hline \text { (iii) Mole } & \text { (b) } \mathrm{mol} \\
\hline \text { (iv) Molality } & \text { (g) } \mathrm{mol} \mathrm{~kg}^{-1} \\
\hline \text { (v) Pressure } & \text { (c) Pascal } \\
\hline \text { (vi) Luminous intensity } & \text { (f) Candela } \\
\hline \text { (vii) Density } & \text { (a) } \mathrm{g} \mathrm{~mL}^{-1} \\
\hline \text { (viii) Mass } & \text { (i) } \mathrm{kg} \\
\hline
\end{array}
\)
(i) It is defined as the number of moles of solute dissolved in 1 litre of solution. So, the unit of molarity is \(
\mathrm{mol} \mathrm{L}^{-1}\)
(ii) Mole fraction is defined as the number of moles of a constituent divided by the total number of moles. So, it is unitless.
(iii) Mole is the unit to measure the amount of an atom or molecule in SI system. it is symbolized as “mol”.
(iv) It is defined as the number of moles of solute dissolved in \(1 \mathrm{~kg}\) of solvent. so, the unit of morality is \(
\mathrm{mol} \mathrm{kg}^{-1}
\)
(v) It is defined as the force per unit area. so, the unit of pressure is Pascal.
(vi) It is the amount of visible light released per unit solid angle in unit time. so, it’s unit will ve candela.
(vii) It is defined as the mass of a substance (in \(\mathrm{kg}\) ) divided by the volume of that substance (in \(\mathrm{mL}\) ). The unit of density is \(\mathrm{g} \mathrm{mL}^{-1}\)
(viii) Mass is used to measure the amount of a substance or an object. The unit of mass is \(\mathrm{kg}\).
Q44. Assertion (A) The empirical mass of ethene is half of its molecular mass.
Reason \((R)\) The empirical formula represents the simplest whole number ratio of various atoms present in a compound.
(a) Both \(A\) and \(R\) are true and \(R\) is the correct explanation of \(A\).
(b) \(A\) is true but \(R\) is false.
(c) \(A\) is false but \(R\) is true.
(d) Both \(A\) and \(R\) are false.
Answer: (a) Hint: Molecular formula \(=\) Empirical formula \(\times 2\)
The empirical formula represents the simplest whole-number ratio of various atoms present in a compound. The molecular formula of ethene is \(\mathrm{C}_2 \mathrm{H}_4\). It can be written as \(\left(\mathrm{CH}_2\right)_2\). Hence, the value of \(n\) is 2. Thus, Molecular formula = Empirical formula \(\times 2\) Hence, both assertion and reason are true and the reason is the correct explanation of the assertion.
Q45. Assertion (A) One atomic mass unit is defined as one-twelfth of the mass of one carbon-12 atom.
Reason ( \(R\) ) Carbon-12 isotope is the most abundant isotope of carbon and has been chosen as the standard.
(a) Both \(\mathrm{A}\) and \(\mathrm{R}\) are true and \(\mathrm{R}\) is not the correct explanation of \(\mathrm{A}\).
(b) \(A\) is true but \(R\) is false.
(c) \(A\) is false but \(R\) is true.
(d) Both \(\mathrm{A}\) and \(\mathrm{R}\) are false.
Answer: (a) Hint: The carbon atom had been used as a reference while assigning atomic mass to the elements of the periodic table.
According to the standard definition of the atomic unit of mass, it is defined as accurately \(\frac{1}{12}\) of the mass of a carbon-12 atom. It is true Carbon-12 isotope is the most abundant isotope of carbon and has been chosen as the standard. The percentage of C- 12 isotope is \(98.93 \%\).
but the correct reason that carbon-12 is the standard while measuring the atomic masses. Because no other nuclides other than carbon-12 have exactly whole-number masses in this scale.
This is due to two factors:
[1] the different mass of neutrons and protons acting to change the total mass in nuclides with proton/neutron ratios other than the 1:1 ratio of carbon-12; and
[2] an exact whole number will not be located if there exists a loss/gain of mass to difference in mean binding energy relative to the mean binding energy for carbon-12
Hence, both assertion and reason are true and the reason is not the correct explanation of assertion.
Q46. Assertion (A) Significant figures for 0.200 is 3 whereas for 200 it is 1.
Reason \((R)\) Zero at the end or right of a number are significant provided they are not on the right side of the decimal point.
(a) Both \(A\) and \(R\) are true and \(R\) is the correct explanation of \(A\).
(b) \(A\) is true but \(R\) is false.
(c) \(A\) is false but \(R\) is true.
(d) Both A and R are false.
Answer: (b) Hint: Significant figures are all numbers that add to the meaning of the overall value of the number.
0.200 contains 3 while 200 contains only one significant figure because zero at the end or right of a number are significant provided they are on the right side of the decimal point.
Thus, The assertion is true but the reason is false
Q47. Assertion (A) Combustion of \(16 \mathrm{~g}\) of methane gives \(18 \mathrm{~g}\) of water.
Reason (R) In the combustion of methane, water is one of the products.
(a) Both \(\mathrm{A}\) and \(\mathrm{R}\) are true but \(\mathrm{R}\) is not the correct explanation of \(\mathrm{A}\).
(b) \(A\) is true but \(R\) is false.
(c) \(A\) is false but \(R\) is true.
(d) Both A and R are false.
Answer:(c)
\(
\text { Hint: } \mathrm{CH}_4+2 \mathrm{O}_2 \rightarrow \mathrm{CO}_2+2 \mathrm{H}_2 \mathrm{O}
\)
The combustion reaction of methane is as follows:
\(\mathrm{CH}_4\)
\(1 \mathrm{~mol}\)
\(
=16 \mathrm{~g}+2 \mathrm{O}_2 \rightarrow \mathrm{CO}_2+\underset{2 \mathrm{~mol}}{2 \mathrm{H}_2 \mathrm{O}}
\)
From the equation, it is clear that 1 mole or \(16 \mathrm{~g} \mathrm{CH}_4\) produces \(2 \mathrm{~mol}\) or \(36 \mathrm{~g}\) of \(\mathrm{H}_2 \mathrm{O}\)
Thus, the Assertion is false but the reason is true because the amount of water is \(36 \mathrm{~g}\), not \(18 \mathrm{~g}\). Water is one of the products.
Q48. A vessel contains \(1.6 \mathrm{~g}\) of dioxygen at STP \((273.15 \mathrm{~K}, 1 \mathrm{~atm}\) pressure). The gas is now transferred to another vessel at a constant temperature, where pressure becomes half of the original pressure. Calculate
(a) the volume of the new vessel.
(b) number of molecules of dioxygen.
Answer: (a) \(\mathrm{p}_1=1 \mathrm{~atm}, \mathrm{p}_2=\frac{1}{2}=0.5 \mathrm{~atm}, \mathrm{~T}_1=273.15, \mathrm{~V}_2=\) ?. \(\mathrm{V}_1=\) ?
\(32 \mathrm{~g}\) dioxygen occupies \(=22.4 \mathrm{~L}\) volume at \(\mathrm{STP}\)
\(\therefore 1.6 \mathrm{~g}\) dioxygen will occupy \(=\frac{22.4 \mathrm{~L} \times 1.6 \mathrm{~g}}{32 \mathrm{~g}}=1.12 \mathrm{~L}\) \(\mathrm{V}_1=1.12 \mathrm{~L}\)
From Boyle’s law (as temperature is constant),
\(
\begin{aligned}
& \mathrm{p}_1 \mathrm{~V}_1=\mathrm{p}_2 \mathrm{~V}_2 \\
& \mathrm{~V}_2=\frac{\mathrm{p}_1 \mathrm{~V}_1}{\mathrm{p}_2}
\end{aligned}
\)
(b) Number of moles of dioxygen \(=\frac{\text { Mass of dioxygen }}{\text { Molar mass of dioxygen }}\)
\(
\mathrm{n}_{\mathrm{O}_2}=\frac{16}{32}=0.05 \mathrm{~mol}
\)
\(1 \mathrm{~mol}\) of dioxygen contains \(=6.022 \times 10^{23}\) molecules of dioxygen \(\therefore 0.05 \mathrm{~mol}\) of dioxygen \(=6.022 \times 10^{23} \times 0.05\) molecules of \(\mathrm{O}_2\) \(=0.3011 \times 10^{23}\) molecules \(=3.011 \times 10^{22}\) molecules.
Q49. Calcium carbonate reacts with aqueous \(\mathrm{HCl}\) to give \(\mathrm{CaCl}_2\) and \(\mathrm{CO}_2\) according to the reaction given below
\(
\mathrm{CaCO}_3(\mathrm{~s})+2 \mathrm{HCl}(\mathrm{aq}) \rightarrow \mathrm{CaCl}_2(\mathrm{aq})+\mathrm{CO}_2(\mathrm{~g})+\mathrm{H}_2 \mathrm{O}(\mathrm{l})
\)
What mass of \(\mathrm{CaCl}_2\) will be formed when \(250 \mathrm{~mL}\) of \(0.76 \mathrm{M} \mathrm{HCl}\) reacts with \(1000 \mathrm{~g}\) of \(\mathrm{CaCO}_3\) ? Name the limiting reagent. Calculate the number of moles of \(\mathrm{CaCl}_2\) formed in the reaction.
Answer:
\(
\begin{aligned}
& \text { Molar mass of } \mathrm{CaCO}_3=40+12+3 \times 16=100 \mathrm{~g} \mathrm{~mol}^{-1} \\
& \text { Moles of } \mathrm{CaCO}_3 \text { in } 1000 \mathrm{~g}, \mathrm{n}_{\mathrm{CaCO}_3}=\frac{\text { Mass }(\mathrm{g})}{\text { Molar mass }} \\
& \mathrm{n}_{\mathrm{CaCO}_3}=\frac{1000 \mathrm{~g}}{100 \mathrm{~g} \mathrm{~mol}^{-1}}=10 \mathrm{~mol} \\
& \text { Molarity }=\frac{\text { Moles of solute }(\mathrm{HCl}) \times 1000}{\text { Volume of solution }}
\end{aligned}
\)
\(
\text { (It is given that moles of } \mathrm{HCl} \text { in } 250 \mathrm{~mL} \text { of } 0.76 \mathrm{M} \mathrm{HCl}=\mathrm{n}_{\mathrm{HCl}} \text { ) }
\)
\(
\begin{aligned}
& 0.76=\frac{\mathrm{n}_{\mathrm{HCl}} \times 1000}{250} \\
& \mathrm{n}_{\mathrm{HCl}}=\frac{0.76 \times 250}{1000}=0.19 \mathrm{~mol} .
\end{aligned}
\)
\(
\underset{1 \mathrm{~mol}}{\mathrm{CaCO}_3(\mathrm{~s})}+\underset{2 \mathrm{~mol}}{2 \mathrm{HCl}(\mathrm{aq})} \rightarrow \mathrm{CaCl}_2(\mathrm{aq})+\mathrm{CO}_2(\mathrm{~g})+\mathrm{H}_2 \mathrm{O}(\mathrm{l})
\)
According to the equation, 1 mole of \(\mathrm{CaCO}_3\) reacts with 2 moles of \(\mathrm{HCl}\) \(\therefore 10\) moles of \(\mathrm{CaCO}_3\) will react with \(\frac{10 \times 2}{1}=20\) moles \(\mathrm{HCl}\). But we have only 0.19 moles \(\mathrm{HCl}\), so \(\mathrm{HCl}\) is limiting reagnt and it limits the yield of \(\mathrm{CaCl}_2\). Since, 2 moles of \(\mathrm{HCl}\) produce 1 mole of \(\mathrm{CaCl}_2\) 0.19 mole of \(\mathrm{HCl}\) will produce \(\frac{1 \times 0.19}{2}=0.095 \mathrm{~mol} \mathrm{CaCl}_2\) Molar mass of \(\mathrm{CaCl}_2=40+(2 \times 35.5)=111 \mathrm{~g} \mathrm{~mol}^{-1}\) \(\therefore 0.095\) mole of \(\mathrm{CaCl}_2=0.095 \times 111=10.54 \mathrm{~g}\)
Q50. Define the law of multiple proportions. Explain it with two examples. How does this law point to the existence of atoms?
Answer: ‘Law of multiple proportions’ was first studied by Daltion in 1803 which may be defined as follows
When two elements combine to form two or more chemical compounds, then the masses of one of the elements which combine with a fixed mass of the other, bear a simple ratio to one another.
e.g., hydrogen combines with oxygen to form two compounds, namely, water and hydrogen peroxide.
\(
\underset{2 \mathrm{~g}}{\text { Hydrogen }}+\underset{16 \mathrm{~g}}{\text { Oxygen }} \rightarrow \underset{18 \mathrm{~g}}{\text { Water }}
\)
\(
\underset{2 \mathrm{~g}}{\text { Hydrogen }}+\underset{32 \mathrm{~g}}{\text { Oxygen }} \rightarrow \underset{34 \mathrm{~g}}{\text { Hydrogen peroxide }}
\)
Here, the masses of oxygen (i.e., \(16 \mathrm{~g}\) and \(32 \mathrm{~g}\) ) which will combine with a fixed mass of hydrogen ( \(2 \mathrm{~g}\) ) bear a simple ratio, i.e., \(16: 32 \mathrm{~or} ~1: 2\).
As we know that, when compounds are mixed in different proportionations, then they form different compounds. In the above examples, when hydrogen is mixed with different proportions of oxygen, then they form water or hydrogen peroxide.
It shows that there are constituents that combine in a definite proportion. These constituents may be atoms. Thus, the law of multiple proportions shows the existence of atoms that combine into molecules.
Q51. A box contains some identical red coloured balls, labelled as A, each weighing 2 grams. Another box contains identical blue coloured balls, labelled as B, each weighing \(5 \mathrm{gm}\). Consider the combinations \(\mathrm{AB}, \mathrm{AB}_2, \mathrm{~A}_2 \mathrm{~B}\) and \(\mathrm{A}_2 \mathrm{~B}_3\), show that law of multiple proportions is applicable.
Answer:
\(\begin{array}{c|c|c}
\hline \text { Combination } & \text { Mass of } \boldsymbol{A}(\boldsymbol{g}) & \text { Mass of } \boldsymbol{B}(\boldsymbol{g}) \\
\hline A B & 2 & 5 \\
A B_2 & 2 & 10 \\
A_2 B & 4 & 5 \\
A_2 B_3 & 4 & 15 \\
\hline
\end{array}
\)
Mass of B which is combined with a fixed mass of A (say \(1 \mathrm{~g}\) ) will be \(2.5 \mathrm{~g}, 5 \mathrm{~g}, 1.25 \mathrm{~g}\) and \(3.75 \mathrm{~g}\). They are in the ratio \(2: 4: 1: 3\) which is a simple whole number ratio. Hence, the law of multiple proportions is applicable.