NEET PYQs

Hint

(a) From the given equations,
\(
\begin{aligned}
&2 \mathrm{NH}_{3} \rightleftharpoons \mathrm{N}_{2}+3 \mathrm{H}_{2} ; \frac{1}{K_{1}} \dots(i) \\
&\mathrm{~N}_{2}+\mathrm{O}_{2} \rightleftharpoons 2 \mathrm{NO} ; K_{2} \dots(ii) \\
&3 \mathrm{H}_{2}+\frac{3}{2} \mathrm{O}_{2} \rightarrow 3 \mathrm{H}_{2} \mathrm{O} ; K_{3}^{3} \dots(iii)
\end{aligned}
\)
By adding equations (i), (ii) and (iii), we get
\(
2 \mathrm{NH}_{3}+\frac{5}{2} \mathrm{O}_{2} \stackrel{K}{\rightleftharpoons} 2 \mathrm{NO}+3 \mathrm{H}_{2} \mathrm{O}, K=\frac{K_{2} K_{3}^{3}}{K_{1}}
\)

Hint

(c) Let solubility of \(\mathrm{Ag}_{2} \mathrm{C}_{2} \mathrm{O}_{4}\) be \(s \mathrm{~mol} \mathrm{~L^{-1}}\)
\(
\begin{aligned}
&\mathrm{Ag}_{2} \mathrm{C}_{2} \mathrm{O}_{4(s)} \rightleftharpoons 2 \mathrm{Ag}_{(a q)}^{+}+\mathrm{C}_{2} \mathrm{O}_{4}{ }^{2-}(a q) \\
&\quad s \quad \quad \quad \quad \quad \quad 2s \quad \quad \quad \quad s \\
&K_{s p}=(2 s)^{2}(s) \Rightarrow 4 s^{3} \\
&K_{s p}=4 \times\left(1.1 \times 10^{-4}\right)^{3}\left(\because\left[\mathrm{Ag}^{+}\right]=2 s=2.2 \times 10^{-4}\right) \\
&K_{s p} \approx 5.3 \times 10^{-12}
\end{aligned}
\)

Hint

(d) \(\mathrm{SrCO}_{3(s)} \rightleftharpoons \mathrm{SrO}_{(s)}+\mathrm{CO}_{2(g)} ; K_{p}=1.6 \mathrm{~atm}\)
\(
\begin{aligned}
K_{p} &=\frac{p_{\mathrm{CO}_{2}} \times p_{\mathrm{SrO}}}{p_{\mathrm{SrCO}_{3}}} \\
\Rightarrow \quad 1.6 &=p_{\mathrm{CO}_{2}} \quad\left(\because p_{\mathrm{SrO}}=p_{\mathrm{SrCO}_{3}}=1\right)
\end{aligned}
\)
\(\therefore\) Maximum pressure of \(\mathrm{CO}_{2}=1.6 \mathrm{~atm}\)
Let the maximum volume of the container when pressure of \(\mathrm{CO}_{2}\) is \(1.6\) atm be \(V \mathrm{~L}\)
During the process, \(P V=\) constant
\(
\begin{aligned}
&\therefore \quad 0.4 \times 20=1.6 \times V \\
&\Rightarrow \quad V=\frac{0.4 \times 20}{1.6}=5 \mathrm{~L}
\end{aligned}
\)

Hint

(b) \(\mathrm{C}_{5} \mathrm{H}_{5} \mathrm{~N}+\mathrm{H}_{2} \mathrm{O} \rightleftharpoons \mathrm{C}_{5} \mathrm{H}_{5} \stackrel{+} {\mathrm{N}} \mathrm{H}+\mathrm{OH}^{-}\)
\(0.10 \mathrm{M}\)
\(
\alpha=\sqrt{\frac{K_{b}}{C}}=\sqrt{\frac{1.7 \times 10^{-9}}{0.10}}=1.30 \times 10^{-4}
\)
\(\therefore\) Percentage of pyridine that forms pyridinium ion \(=1.30 \times 10^{-4} \times 100=0.013 \%\)

Hint

(b) Let \(s\) be the solubility of \(\mathrm{AgCl}\) in moles per litre.
\(
\mathrm{AgCl}_{(a q)} \rightleftharpoons \mathrm{Ag}_{(a q)}^{+}+\mathrm{Cl}_{(a q)}^{-}
\)
\(
\begin{array}{lll}
s & & s & & (s+0.1)
\end{array}
\)
\((\because 0.1 \mathrm{~M} \mathrm{~NaCl}\) solution also provides \(0.1 \mathrm{~M} \mathrm{~Cl}^{-}\)ion)
\(
K_{s p}=\left[\mathrm{Ag}^{+}\right][\mathrm{Cl^{-}}] ; 1.6 \times 10^{-10}=s(s+0.1)
\)
\(
1.6 \times 10^{-10}=s(0.1) \quad(\because s<<<<0.1)
\)
\(
s=\frac{1.6 \times 10^{-10}}{0.1}=1.6 \times 10^{-9} \mathrm{M}
\)

Hint

(b) \(\mathrm{BF}_{3} \rightarrow\) Lewis acid (incomplete octet)
\(\mathrm{PF}_{3} \rightarrow\) Lewis base (presence of lone pair on \(\mathrm{P}\) atom)
\(\mathrm{CF}_{4} \rightarrow\) Complete octet
\(\mathrm{SiF}_{4} \rightarrow\) Lewis acid (empty \(d\)-orbital in Si-atom)

Hint

(d) For \(M Y: K_{s p}=s_{1}^{2}\)
\(\Rightarrow s_{1}=\sqrt{K_{s p}}=\sqrt{6.2 \times 10^{-13}}=7.87 \times 10^{-7} \mathrm{~mol} \mathrm{~L}^{-1}\)
For \(N Y_{3}: K_{s p}=27 s_{2}^{4}\)
\(\Rightarrow \quad s_{2}=\sqrt[4]{\frac{6.2 \times 10^{-13}}{27}}=3.89 \times 10^{-4} \mathrm{~mol} \mathrm{~L}^{-1}\)
Hence, molar solubility of \(M Y\) in water is less than that of \(\mathrm{NY}_{3}\)

Hint

(d) If the reaction is multiplied by \(\frac{1}{2}\), then new equilibrium constant, \(K^{\prime}=K^{1 / 2}\)

Hint

(d) One mole of \(\mathrm{NaOH}\) is completely neutralised by one mole of \(\mathrm{HCl}\).

Hence, \(0.01\) mole of \(\mathrm{NaOH}\) will be completely neutralised by \(0.01\) mole of \(\mathrm{~HCl}\).
\(\Rightarrow \mathrm{NaOH}\) left unneutralised \(=0.1-0.01=0.09 \mathrm{~mol}\)
As equal volumes of two solutions are mixed,
\(
\begin{aligned}
& {[\mathrm{OH}]^{-}=\frac{0.09}{2}=0.045 \mathrm{~M} } \\
\Rightarrow & \mathrm{pOH}=-\log (0.045)=1.35 \\
\therefore & \mathrm{pH}=14-1.35=12.65
\end{aligned}
\)

Hint

(a) \(\mathrm{HCl}\) is a strong acid and dissociates completely into ions in aqueous solution

Hint

(d) Acidic buffer is a mixture of a weak acid and its salt with a strong base. \(\mathrm{HClO}_{4}\) is a strong acid.

Hint

(b)
Salt \(\quad \quad \quad \quad K_{s p} \quad \quad \quad \quad \quad \quad \quad \) Solubility
\(\mathrm{Ag}_{2} \mathrm{CrO}_{4} \quad 1.1 \times 10^{-12}=4 s^{3} \quad s=\sqrt[3]{\frac{K_{s p}}{4}}=0.65 \times 10^{-4}\)
\(\mathrm{AgCl} \quad 1.8 \times 10^{-10}=s^{2} \quad \quad \quad s=\sqrt{K_{s p}}=1.34 \times 10^{-5}\)
\(\mathrm{AgBr} \quad 5 \times 10^{-13}=s^{2} \quad \quad \quad \quad s=\sqrt{K_{s p}}=0.71 \times 10^{-6}\)
AgI \(\quad \quad 8.3 \times 10^{-17}=s^{2} \quad \quad \quad \quad s=\sqrt{K_{s p}}=0.9 \times 10^{-8}\)
Solubility of \(\mathrm{Ag}_{2} \mathrm{CrO}_{4}\) is highest thus, it will be precipitated at last

Hint

(a)

\(
\Delta G=\Delta G^{\circ}+2.303 R T \log K
\)

At equilibrium, \(\Delta \mathrm{G}=0\)
\(0=\Delta G^{\circ}+2.303 R T \log K\)
\(\Delta G^{\circ}=-2.303 R T \log K\)

Hint

(a) The value of \(K\) is high which means reaction proceeds almost to completion i.e., the system will contain mostly products.

Explanation:

The value of the equilibrium constant \(\mathrm{K}\) is \(1.6 \times 10^{12}\) which is very high.
\(
\mathrm{K}=\frac{[\text { Products }]}{[\text { Reactants }]}
\)

This indicates the ratio of product concentration to reactant concentration is very high.
Hence, the reaction mixture mostly contains products.

Hint

(c) \(\mathrm{Na}_{2} \mathrm{CO}_{3}\) which is a salt of \(\mathrm{NaOH}\) (strong base) and \(\mathrm{H}_{2} \mathrm{CO}_{3}\) (weak acid) will produce a basic solution with \(\mathrm{pH}\) greater than 7.

Explanation:

The highest \(\mathrm{pH}\) refers to the basic solution containing \(\mathrm{OH}^{-}\)ions. Therefore, the basic salt releasing more \(\mathrm{OH}^{-}\) ions on hydrolysis will give highest \(p H\) in water.

Only the salt of strong base and weak acid would release more \(\mathrm{OH}^{-}\)ion on hydrolysis. Among the given salts, \(\mathrm{Na}_2 \mathrm{CO}_3\) corresponds to the basic salt as it is formed by the neutralisation of \(\mathrm{NaOH}\) [strong base] and \(\mathrm{H}_2 \mathrm{CO}_3\) [weak acid].
\(
\mathrm{CO}_3^{2-}+\mathrm{H}_2 \mathrm{O} \rightleftharpoons \mathrm{HCO}_3^{-}+\mathrm{OH}^{-}
\)

Hint

\(
\begin{aligned}
&\text { (b) }: \Delta G^{\circ}=-2.303 R T \log K_{s p} \\
&63.3 \times 10^{3} \mathrm{~J}=-2.303 \times 8.314 \times 298 \log K_{s p} \\
&63.3 \times 10^{3} \mathrm{~J}=-5705.84 \log K_{s p} \\
&\log K_{s p}=-\frac{63.3 \times 10^{3}}{5705.84}=-11.09 \\
&K_{s p}=\text { antilog }(-11.09)=8.128 \times 10^{-12}
\end{aligned}
\)

Hint

 (d)  As the forward reaction is exothermic and leads to lowering of pressure (produces lesser number of gaseous moles) hence, according to Le Chatelier’s principle, at high pressure and low temperature, the given reversible reaction will shift in forward direction to form more product.

Hint

(a) \(\log \frac{K_{p}^{\prime}}{K_{p}}=-\frac{\Delta H}{2.303 R}\left[\frac{1}{T_{2}}-\frac{1}{T_{1}}\right]\)
For exothermic reaction, \(\Delta H=-\) ve i.e. heat is evolved. The temperature \(T_{2}\) is higher than \(T_{1}\).
Thus, \(\left(\frac{1}{T_{2}}-\frac{1}{T_{1}}\right)\) is negative.
so, \(\log K_{p}^{\prime}-\log K_{p}=-\) ve or \(\log K_{p}>\log K_{p}^{\prime}\) or \(K_{p}>K_{p}^{\prime}\)

Hint

(a) \(\mathrm{HCl}\) and \(\mathrm{SO}_{2}\) are reducing agents. So they can reduce \(\mathrm{MnO}_{4}^{-}\). \(\mathrm{CO}_{2}\) is neither oxidising nor reducing agent, it will provide only acidic medium. It can shift the reaction in forward direction and the reaction can go to completion

Hint

\(
\text { (a) } \mathrm{BF}_{3} \text { is Lewis acid } \text { ( } e^{-} \text {pair acceptor). }
\)

Hint

(b) Degree of dissociation, \(\alpha=\frac{3.7}{100}=0.037\)
According to Ostwald’s formula,
\(
K_{a}=\alpha^{2} C=(0.037)^{2} \times 0.10=1.369 \times 10^{-4} \approx 1.4 \times 10^{-4}
\)

Hint

(d) We know that, at \(25^{\circ} \mathrm{C}, K_{w}=1 \times 10^{-14}\) At \(100^{\circ} \mathrm{C}, K_{w}=55 \times 10^{-14}\)
\(
\begin{aligned}
&\mathrm{H}^{+}=\sqrt{55 \times 10^{-14}} \\
&\mathrm{pH}=-\log \left[\mathrm{H}^{+}\right] \\
&\mathrm{pH}=-\log \left[\sqrt{55 \times 10^{-14}}\right]
\end{aligned}
\)

\(
\begin{array}{l}
=\frac{1}{2}\left[-\log \left(55 \times 10^{-14}\right)\right]=\frac{1}{2}[-\log 55+14 \log 10] \\
=\frac{1}{2}[-1.74+14]=\frac{1}{2}[12.26]=6.13
\end{array}
\)

Hint

(d) \(\mathrm{CaCO}_{3} \rightarrow \mathrm{Ca}^{2+}+\mathrm{CO}_{3}^{2-}\)
\(\quad \quad \quad \quad \quad \quad \quad x \quad x\)
\(\mathrm{CaC}_{2} \mathrm{O}_{4} \rightarrow \mathrm{Ca}^{2+}+\mathrm{C}_{2} \mathrm{O}_{4}^{2-}\)
\(\quad \quad \quad \quad \quad \quad \quad y \quad y\)
Now, \(\left[\mathrm{Ca}^{2+}\right]=x+y\)
and \(x(x+y)=4.7 \times 10^{-9} \dots(i)\)
\(
y(x+y)=1.3 \times 10^{-9} \dots(ii)
\)
Dividing equation (i) and (ii) we get
\(
\begin{aligned}
&\quad \frac{x}{y}=3.6 \\
&\therefore \quad x=3.6 y \\
&\text { Putting this value in equation (ii), we get } \\
&\qquad y(3.6 y+y)=1.3 \times 10^{-9} \\
&\text { On solving, we get } y=1.68 \times 10^{-5} \\
&\text { and } x=3.6 \times 1.68 \times 10^{-5}=6.048 \times 10^{-5} \\
&\therefore \quad\left[\mathrm{Ca}^{2+}\right]=(x+y)=\left(1.68 \times 10^{-5}\right)+\left(6.048 \times 10^{-5}\right) \\
&\therefore \quad\left[\mathrm{Ca}^{2+}\right]=7.728 \times 10^{-5} \mathrm{M}
\end{aligned}
\)

Hint

\(
\begin{aligned}
&\text { (b) } \mathrm{pH}=\mathrm{p} K_{a}+\log \frac{[\text { Salt }]}{[\text { Acid }]} \\
&5=-\log K_{a}+\log \frac{[\text { Salt }]}{[\text { Acid }]} \quad\left[\because \mathrm{p} K_{a}=-\log K_{a}\right] \\
&5=-\log \left[1 \times 10^{-4}\right]+\log \frac{[\text { Salt }]}{[\text { Acid }]} \\
&5=4+\log \frac{[\text { Salt }]}{[\text { Acid }]} \\
&5-4=\log \frac{[\text { Salt }]}{[\text { Acid }]} \\
&1=\log \frac{[\text { Salt }]}{[\text { Acid }]} \\
&\frac{[\text { Salt }]}{[\text { Acid }]}=10=10: 1
\end{aligned}
\)

Hint

\(
\begin{aligned}
&\text { (b) } \mathrm{pH} \text { of solution }=12 \\
&{\left[\mathrm{H}^{+}\right]=10^{-12}} \\
&{\left[\mathrm{OH}^{-}\right]=\frac{10^{-14}}{10^{-12}}=10^{-2}} \\
&\mathrm{Ba}(\mathrm{OH})_{2} \rightleftharpoons \mathrm{Ba}^{2+}+2 \mathrm{OH}^{-} \\
& \quad \quad \quad \quad \quad \quad s \quad \quad 2 s \\
&2 s=10^{-2} \Rightarrow s=\frac{10^{-2}}{2} \\
&K_{s p}=(s)(2 s)^{2}=4 s^{3}
\end{aligned}
\)
\(
=4 \times\left(\frac{10^{-2}}{2}\right)^3=\frac{4}{8} \times 10^{-6}=5 \times 10^{-7}
\)

Hint

(a) \(\mathrm{BaCl}_{2}\) is made up of \(\mathrm{Ba}(\mathrm{OH})_{2}\) and \(\mathrm{HCl}\). \(\mathrm{AlCl}_{3}\) is made up of \(\mathrm{Al}(\mathrm{OH})_{3}\) and \(\mathrm{HCl}\).
\(\mathrm{LiCl}\) is made up of \(\mathrm{LiOH}\) and \(\mathrm{HCl}\).
\(\mathrm{BeCl}_{2}\) is made up of \(\mathrm{Be}(\mathrm{OH})_{2}\) and \(\mathrm{HCl}\).
\(\mathrm{Ba}(\mathrm{OH})_{2}\) is strongest base among the given options thus have maximum \(\mathrm{pH}\)

Hint

(a)

If small amount of an acid or alkali is added to a buffer solution, it converts them into unionised acid or base.
Thus, its \(p H\) remains unaffected or in other words its acidity/alkalinity remains constant, e.g.
\(
\begin{array}{l}
\mathrm{H}_3 \mathrm{O}^{+}+\mathrm{A}^{-} \rightleftharpoons \mathrm{H}_2 \mathrm{O}+\mathrm{HA} \\
\mathrm{OH^{-}}+\mathrm{HA} \rightarrow \mathrm{H}_2 \mathrm{O}+\mathrm{A}^{-}
\end{array}
\)

If acid is added, it reacts with \(A^{-}\)to form undissociated \(HA\). Similarly, if base/alkali is added, \(\mathrm{OH}^{-}\)combines with HA to give \(\mathrm{H}_2 \mathrm{O}\) and \(\mathrm{A}^{-}\)and thus, maintains the acidity/ alkalinity of buffer solution.

Hint

(c) \(2 \mathrm{SO}_{2(g)}+\mathrm{O}_{2(g)} \rightleftharpoons 2 \mathrm{SO}_{3(g)} \dots(i)\)
\(K=278\)
By reversing the equation (i), we get
\(
2 \mathrm{SO}_{3(\mathrm{~g})} \rightleftharpoons 2 \mathrm{SO}_{2(\mathrm{~g})}+\mathrm{O}_{2(\mathrm{~g})} \dots(ii)
\)
Equilibrium constant for this reaction is
\(
K^{\prime}=\frac{1}{K}=\frac{1}{278}
\)
By dividing the equation (ii) by 2 , we get desired equation,
\(
\mathrm{SO}_{3(g)} \rightleftharpoons \mathrm{SO}_{2(g)}+\frac{1}{2} \mathrm{O}_{2(g)} \dots(iii)
\)
Equilibrium constant for this reaction
\(
K^{\prime \prime}=\sqrt{K^{\prime}}=\sqrt{\frac{1}{K}}=\sqrt{\frac{1}{278}}=0.0599 \approx 0.06 \text { or } 6 \times 10^{-2}
\)

Hint

\(
\begin{aligned}
&\text { (c) } A_{2(g)}+B_{2(g)} \rightleftharpoons 2 A B_{(g)} \\
&K_{c}=\frac{[A B]^{2}}{\left[A_{2}\right]\left[B_{2}\right]} \\
&=\frac{\left(2.8 \times 10^{-3}\right)^{2}}{\left(3.0 \times 10^{-3}\right)\left(4.2 \times 10^{-3}\right)}=\frac{2.8 \times 2.8}{4.2 \times 3.0}=0.62
\end{aligned}
\)

Hint

(d) \(X_{2(g)}+4 Y_{2(g)} \rightleftharpoons 2 X Y_{4(g)}\)
\(
\Delta n_{g}=-\mathrm{ve} \text { and } \Delta H=-\mathrm{ve}
\)
The reaction is favoured in forward direction at low temperature and high pressure.

Hint

(b) \(\left[\mathrm{NH}_{3}\right]=0.30 \mathrm{~M}, K_{b}=1.8 \times 10^{-5}\)
\(
\begin{aligned}
&{\left[\mathrm{NH}_{4}^{+}\right]=0.20 \mathrm{~M}} \\
&\mathrm{pOH}=\mathrm{p} K_{b}+\log \frac{[\text { salt }]}{[\text { base }]}=4.74+\log \frac{0.2}{0.3}=4.56 \\
&\mathrm{pH}=(14-4.56)=9.43
\end{aligned}
\)

Hint

(c) \(\mathrm{BF}_{3}\) is an electron deficient species and acts as Lewis base

Hint

\(
\begin{aligned}
&\text { (c) } \mathrm{N}_{2}+\mathrm{O}_{2} \rightleftharpoons 2 \mathrm{NO} ; K_{1} \\
&2 \mathrm{NO}+\mathrm{O}_{2} \rightleftharpoons 2 \mathrm{NO}_{2} ; K_{2} \\
&\mathrm{NO}_{2} \rightleftharpoons \frac{1}{2} \mathrm{~N}_{2}+\mathrm{O}_{2} ; K \\
&K_{1}=\frac{\left[\mathrm{NO}]^{2}\right.}{\left[\mathrm{N}_{2}\right]\left[\mathrm{O}_{2}\right]} ; K_{2}=\frac{\left[\mathrm{NO}_{2}\right]^{2}}{\left[\mathrm{NO}]^{2}\left[\mathrm{O}_{2}\right]\right.}
\end{aligned}
\)

\(
\begin{array}{l}
K=\frac{\left[\mathrm{N}_2\right]^{1 / 2}\left[\mathrm{O}_2\right]}{\left[\mathrm{NO}_2\right]}=\sqrt{\frac{\left[\mathrm{N}_2\right]\left[\mathrm{O}_2\right] \times\left[\mathrm{NO}]^2\left[\mathrm{O}_2\right]\right.}{\left[\mathrm{NO}]^2 \times\left[\mathrm{NO}_2\right]^2\right.}} \\
K=\sqrt{\frac{1}{\mathrm{~K}_1 \mathrm{~K}_2}}
\end{array}
\)

Hint

(c)

\(
K_{s p}[\mathrm{AgCl}]=\left[\mathrm{Ag}^{+}\right][\mathrm{Cl}^{-}]
\)
\(
\left[\mathrm{Ag}^{+}\right]=\frac{1.8 \times 10^{-10}}{10^{-1}}=1.8 \times 10^{-9} \mathrm{M}
\)
\(
K_{s p}\left[\mathrm{PbCl}_2\right]=\left[\mathrm{Pb}^{2+}\right][\mathrm{Cl}^{-}]^2
\)
\(
\left[\mathrm{Pb}^{2+}\right]=\frac{1.7 \times 10^{-5}}{10^{-1} \times 10^{-1}}=1.7 \times 10^{-3} \mathrm{M}
\)

Hint

\(
\begin{aligned}
&\text { (d) We Know, } \mathrm{pH}+\mathrm{pOH}=14\\
&\text { Here, } \quad 12+\mathrm{pOH}=14\\
&\mathrm{pOH}=2\\
&\left[\mathrm{OH}^{-}\right]=10^{-2}\\
&\mathrm{Ba}(\mathrm{OH})_{2} \rightleftharpoons \mathrm{Ba}^{2+}+2 \mathrm{OH}^{-}\\
&2 S=[\mathrm{OH}^{-}]=10^{-2}\\
&s=\frac{10^{-2}}{2}=5 \times 10^{-3} \mathrm{M}\\
&K_{s p}=\left[\mathrm{Ba}^{2+}\right]\left[\mathrm{OH}^{-}\right]^{2}=\left(5 \times 10^{-3}\right)\left(10^{-2}\right)^{2}\\
&K_{s p}=5 \times 10^{-7}
\end{aligned}
\)

Hint

(d) \(\mathrm{CH}_{3} \mathrm{COOH} \rightleftharpoons \mathrm{CH}_{3} \mathrm{COO}^{-}+\mathrm{H}^{+}\)
\(C-x \quad \quad \quad \quad \quad x \quad \quad \quad \quad x\)
\(\mathrm{CH}_{3} \mathrm{COONa} \rightarrow \mathrm{CH}_{3} \mathrm{COO}^{-}+\mathrm{Na}^{+}\)
\(0.2 \mathrm{~M} \quad \quad \quad \quad 0.2 \mathrm{~M} \quad \quad \quad 0.2 \mathrm{~M}\)
\({\left[\mathrm{CH}_{3} \mathrm{COOH}\right]=C-x \approx 0.1 \mathrm{M} }\) \({\left[\mathrm{CH}_{3} \mathrm{COO}^{-}\right]=0.2+x \approx 0.2 \mathrm{M} }\)\(\left\{\begin{array}{l}\text { acetic acid is a } \\ \text { weak acid so, } \\ \text { dissociation is } \\ \text { minimum. }\end{array}\right.\)
\(\therefore \quad\left[\mathrm{H}^{+}\right]=\frac{K_{a}\left[\mathrm{CH}_{3} \mathrm{COOH}\right]}{\left[\mathrm{CH}_{3} \mathrm{COO}^{-}\right]}\)
\(=\frac{1.8 \times 10^{-5} \times 10^{-1}}{2 \times 10^{-1}}=9 \times 10^{-6} \mathrm{M}\)

Hint

(d) \(K_{p}\) and \(K_{c}\) are related by the equation,
\(
K_{p}=K_{c}(R T)^{\Delta n_{g}}
\)
where \(\Delta n_{g}=\) difference in the no. of moles of products and reactants in the gaseous state. For
\(
\begin{gathered}
2 \mathrm{C}_{(s)}+\mathrm{O}_{2(g)} \rightleftharpoons 2 \mathrm{CO}_{2(g)} \\
\Delta n_{g}=2-(1)=1 \neq 0
\end{gathered}
\)

Hint

(d) We know, \(\mathrm{pOH}=\mathrm{p} K_{b}+\log \frac{\left[B^{-}\right]}{[\mathrm{H} B]}\)
Since, \(\left[B^{-}\right]=[H B]\) (given)
\(
\begin{aligned}
&\therefore \quad \mathrm{pOH}=\mathrm{p} K_{b} \Rightarrow \mathrm{pOH}=10 \\
&\therefore \quad \mathrm{pH}=14-10=4
\end{aligned}
\)

Hint

\(\begin{array}{lcccc}\text { (b) } & 2 A_{(g)}+ & B_{(g)} \rightleftharpoons & 3 C_{(g)} & +D_{(g)} \\ \text { Initial moles : } & 1 & 1 & 0 & 0 \\ \text { Moles at eq. : } & 1-(2 \times 0.25) & 1-0.25 & 3 \times 0.25 & 0.25 \\ & =0.5 & =0.75 & =0.75 & =0.25\end{array}\) Equilibrium constant, \(K=\frac{[C]^{3}[D]}{[A]^{2}[B]}\)
\(
\therefore \quad K=\frac{(0.75)^{3}(0.25)}{(0.5)^{2}(0.75)}
\)

Hint

(c) Given,
\(
\begin{aligned}
&\mathrm{CH}_{3} \mathrm{COOH} \rightleftharpoons \mathrm{CH}_{3} \mathrm{COO}^{-}+\mathrm{H}^{+} \\
&K_{1}=\frac{\left[\mathrm{CH}_{3} \mathrm{COO}^{-}\right]\left[\mathrm{H}^{+}\right]}{\left[\mathrm{CH}_{3} \mathrm{COOH}\right]}=1.5 \times 10^{-5} \\
&\mathrm{HCN} \rightleftharpoons \mathrm{H}^{+}+\mathrm{CN}^{-} \\
&K_{2}=\frac{\left[\mathrm{CN}^{-}\right]\left[\mathrm{H}^{+}\right]}{\left[\mathrm{HCN}\right]}=4.5 \times 10^{-10} \\
&\mathrm{CN}^{-}+\mathrm{CH}_{3} \mathrm{COOH} \rightleftharpoons \mathrm{HCN} + \mathrm{CH}_{3} \mathrm{COO}^{-} \\
&K=\frac{\left[\mathrm{HCN}\right]\left[\mathrm{CH}_{3} \mathrm{COO}^{-}\right]}{\left[\mathrm{CN}^{-}\right]\left[\mathrm{CH}_{3} \mathrm{COOH}\right]} \\
&K=\frac{K_{1}}{K_{2}}=\frac{1.5 \times 10^{-5}}{4.5 \times 10^{-10}} \approx 0.3 \times 10^{5}
\end{aligned}
\)

Hint

(d) Lewis acids are electron deficient compounds, since \(\left(\mathrm{CH}_{3}\right)_{3} \mathrm{~B}\) is electron deficient (due to incomplete octect of B), it acts as a Lewis acid

Hint

(d) \(\mathrm{NH}_{4} \mathrm{Cl}\) is a salt of strong acid and weak base, so hydrolysis constant is
\(
\begin{gathered}
K_{h}=\frac{K_{w}}{K_{b}} \\
\text { Given, } K_{b}\left(\mathrm{NH}_{4} \mathrm{OH}\right)=1.77 \times 10^{-5} \\
K_{w}=10^{-14} \\
\therefore \quad K_{h}=\frac{10^{-14}}{1.77 \times 10^{-5}}=0.565 \times 10^{-9} \\
\text { or } \quad K_{h}=5.65 \times 10^{-10}
\end{gathered}
\)

Hint

(d) Millimoles of \(\mathrm{H}^{+}\)produced \(=20 \times 0.05=1\)
Millimoles of \(\mathrm{OH}^{-}\)produced \(=30 \times 0.1 \times 2=6\)
( \(\because\) Each \(\mathrm{Ba}(\mathrm{OH})_2\) gives \(2 \mathrm{OH}^{-}\).)
\(\therefore\) Millimoles of \(\mathrm{OH}^{-}\) remaining in solution
\(
=6-1=5
\)

Total volume of solution \(=20+30=50 \mathrm{~mL}\)
\(
\therefore \quad\left[\mathrm{OH}^{-}\right]=\frac{5}{50}=0.1 \mathrm{M}
\)

Hint

(d)

\(
\begin{array}{cccr}
& 2 A B_{2(g)} \rightleftharpoons 2 A B_{(g)}+B_{2(g)} & \\
& 2 \quad \quad \quad 0 \quad \quad \quad \quad 0 & \text { (initially) } \\
& 2(1-x) \quad \quad 2 x \quad \quad \quad \quad x & \text { (at equilibrium) }
\end{array}
\)\(
\begin{aligned}
&\text { Amount of moles at equilibrium }=2(1-x)+2 x+x = 2 + x \\
&\qquad \begin{aligned}
K_{p}=& \frac{\left[p_{A B}\right]^{2}\left[p_{B_{2}}\right]}{\left[p_{A B_{2}}\right]^{2}} \\
K_{p}=\frac{\left(\frac{2 x}{2+x} \times P\right)^{2} \times\left(\frac{x}{2+x} \times P\right)}{\left(\frac{2(1-x)}{2+x} \times P\right)^{2}}=\frac{\frac{4 x^{3}}{2+x} \times P}{4(1-x)^{2}} \\
K_{p}=\frac{4 x^{3} \times P}{2} \times \frac{1}{4} \quad(\because 1-x \approx 1 \& 2+x \approx 2) \\
x=\left(\frac{8 K_{p}}{4 P}\right)^{1 / 3} \quad \Rightarrow x=\left(\frac{2 K_{p}}{P}\right)^{1 / 3}
\end{aligned}
\end{aligned}
\)

Hint

(a) \(\mathrm{Fe}(\mathrm{OH})_{3(s)} \rightleftharpoons \mathrm{Fe}_{(a q)}^{3+}+3 \mathrm{OH}_{(a q)}^{-}\)
\(
K=\frac{\left[\mathrm{Fe}^{3+}\right]\left[\mathrm{OH}^{-}\right]^{3}}{\left[\mathrm{Fe}(\mathrm{OH})_{3}\right]}
\)
\(K=\left[\mathrm{Fe}^{3+}\right]\left[\mathrm{OH}^{-}\right]^{3}\) (activity of solid is taken unity)
Concentration of \(\mathrm{OH}^{-}\)ion in the reaction is decreased by \(1 / 4\) times then equilibrium concentration of \(\mathrm{Fe}^{3+}\) will be increased by 64 times in order to keep the value of \(K\) constant

Hint

(d) \(\mathrm{pH}=-\log \left[\mathrm{H}^{+}\right]\)
or \(\left[\mathrm{H}^{+}\right]=10^{-\mathrm{pH}} ;\left[\mathrm{H}^{+}\right]\)of soln. \(1=10^{-3}\)
\(\left[\mathrm{H}^{+}\right]\)of \(\operatorname{soln} .2=10^{-4} ;\left[\mathrm{H}^{+}\right]\)of \(\operatorname{soln} .3=10^{-5}\)
Total concentration of \(\left[\mathrm{H}^{+}\right]\)
\(
\begin{gathered}
=10^{-3}\left(1+1 \times 10^{-1}+1 \times 10^{-2}\right) \\
\Rightarrow 10^{-3}\left(\frac{1}{1}+\frac{1}{10}+\frac{1}{100}\right) \Rightarrow 10^{-3}\left(\frac{100+10+1}{100}\right) \\
\Rightarrow 10^{-3}\left(\frac{111}{100}\right)=1.11 \times 10^{-3}
\end{gathered}
\)
So, \(\mathrm{H}^{+}\)ion concentration in mixture of equal volume of these acid solution \(=\frac{1.11 \times 10^{-3}}{3}=3.7 \times 10^{-4} \mathrm{M}\)

Hint

(d) \(\mathrm{HI}_{(\mathrm{g})} \rightleftharpoons 1 / 2 \mathrm{H}_{2(g)}+1 / 2 \mathrm{I}_{2(g)}\)
i.e. \(K=\frac{\left[\mathrm{H}_{2}\right]^{1 / 2}\left[\mathrm{I}_{2}\right]^{1 / 2}}{[\mathrm{HI}]}=8\)
\(
\begin{gathered}
\mathrm{H}_{2(\mathrm{~g})}+\mathrm{I}_{2(\mathrm{~g})} \rightleftharpoons 2 \mathrm{HI}_{(\mathrm{g})} \\
K^{\prime}=\frac{[\mathrm{HI}]^{2}}{\left[\mathrm{H}_{2}\right]\left[\mathrm{I}_{2}\right]}=\left(\frac{1}{8}\right)^{2} \Rightarrow K^{\prime}=\frac{1}{64}
\end{gathered}
\)

Hint

\(
\begin{array}{l}
X \rightleftharpoons Y+Z \dots(i) \\
A \rightleftharpoons 2 B \dots(ii) \\
X \rightleftharpoons Y+Z \\
\begin{array}{llll}
1 \quad \quad \quad 0 \quad \quad 0 & \text { Initially }
\end{array} \\
1-\alpha \quad \quad \alpha \quad \quad \alpha \quad \text { At equilibrium } \\
\end{array}
\)
Total no. of moles at equilibrium \(=1-\alpha+2 \alpha=1+\alpha\) Similarly,
\(
A \rightleftharpoons 2 B
\)
\(
\begin{array}{lll}
1 & 0 & \text { Initially } \\
1-\alpha & 2 \alpha & \text { At equilibrium }
\end{array}
\)
Total no. of moles at equilibrium \(=1-\alpha+2 \alpha=1+\alpha\)
\(
\therefore \quad K_{p_1}=\frac{p_Y \times p_Z}{p_X}=\frac{\frac{\alpha}{1+\alpha} \times P_1 \times \frac{\alpha}{1+\alpha} \times P_1}{\frac{1-\alpha}{1+\alpha} \times P_1}
\)
\(
K_{p_2}=\frac{\left(p_B\right)^2}{p_A}=\frac{\left(\frac{2 \alpha}{1+\alpha} \times P_2\right)^2}{\frac{1-\alpha}{1+\alpha} \times P_2}
\)
\(
\text { Now } \frac{K_{p_1}}{K_{p_2}}=\frac{P_1}{4 P_2} \Rightarrow \frac{P_1}{P_2}=\frac{36}{1}=36: 1
\)

Hint

(a) For a weak acid, degree of dissociation, \(\alpha=\sqrt{\frac{K_{a}}{C}}=\sqrt{\frac{1 \times 10^{-5}}{0.1}}=10^{-2}\) i.e. \(1.00 \%\)

Hint

(a) Given, \(\left[\mathrm{H}_{3} \mathrm{O}^{+}\right]=1 \times 10^{-10}\) or, \(\mathrm{pH}=10\)
Now at \(25^{\circ} \mathrm{C}, \mathrm{pH}+\mathrm{pOH}=\mathrm{p} K_{w}=14\)
or, \(\mathrm{pOH}=14-\mathrm{pH}=14-10=4\)

Hint

(c) \(\mathrm{CH}_{4(g)}+2 \mathrm{O}_{2(g)} \rightleftharpoons \mathrm{CO}_{2(g)}+2 \mathrm{H}_{2} \mathrm{O}_{(l)}\)
Now, \(K_p=\frac{\left[\mathrm{CO}_2\right]\left[\mathrm{H}_2 \mathrm{O}\right]^2}{\left[\mathrm{CH}_4\right]\left[\mathrm{O}_2\right]^2}\)
Now, \(\mathrm{H}_2 \mathrm{O}\) is pure liquid, so, \(\left[\mathrm{H}_2 \mathrm{O}\right]=1\)
\(
\Rightarrow K_C=\frac{\left[\mathrm{CO}_2\right]}{\left[\mathrm{CH}_4\right]\left[\mathrm{O}_2\right]^2}
\)
\(\because \Delta H r=-170.8 \mathrm{KJ} / \mathrm{mol}\) is negative, so reaction is exothermic by adding \(\mathrm{O}_2(g)\)
or \(\mathrm{CH}_4(\mathrm{~g})\) at equilibrium, by Le Chatelier’s principle, the equilibrium shift towards right side.

Hint

(b) \(\mathrm{HNO}_{2}\) and \(\mathrm{NaNO}_{2}\) are examples of acidic buffer

Hint

(c) \(10^{-8} \mathrm{~M} \mathrm{~HCl}=10^{-8} \mathrm{~M} \mathrm{~H}^{+}\)
Also from water, \(\left[\mathrm{H}^{+}\right]=10^{-7}\)
Total \(\left[\mathrm{H}^{+}\right]=10^{-7}+0.10 \times 10^{-7}=1.1 \times 10^{-7}\)

Hint

(d) \(C=0.01 \mathrm{M}\)
\(
K_{b}=1 \times 10^{-12} \text { at } 25^{\circ} \mathrm{C}
\)
\(\mathrm{BOH} \rightleftharpoons \mathrm{B}^{+}+\mathrm{OH}^{-}\)
\(
\begin{array}{lll}
C & & & 0 & & & 0
\end{array}
\)
\(
\text { at eq. } C-C \alpha \quad C \alpha \quad C \alpha
\)
\(
\begin{aligned}
{\left[\mathrm{OH}^{-}\right] } &=C \alpha \\
{\left[\mathrm{OH}^{-}\right] } &=\sqrt{K_{b} C}=\sqrt{1 \times 10^{-12} \times 10^{-2}} \\
{\left[\mathrm{OH}^{-}\right] } &=10^{-7} \mathrm{~mol} \mathrm{~L}
\end{aligned}
\)

Hint

(a) The cation of group II are precipitated as their sulphides.

Solubility product of sulphide of group II radicals are very low. Therefore, even with low conc. of \(\mathrm{S}^{2-}\) ions, the ionic product exceeds the value of their solubility product and the radical of group II gets precipitated. The low conc. of \(\mathrm{S}^{2-}\) ions is obtained by passing \(\mathrm{H}_2 \mathrm{S}\) gas through the solution of the salt in the presence of dil. \(\mathrm{HCl}\) which suppresses degree of ionisation of \(\mathrm{H}_2 \mathrm{S}\) by common ion effect.

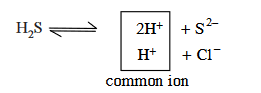
Note: Solubility product of group IV radicals are quite high.
It is necessary to suppress the conc. of \(\mathrm{S}^{2-}\) ions, otherwise radical of group IV will also get precipitated along with group II radicals.

Hint

(a) \( K_{1}=\frac{P_{\mathrm{NO}_{2}}}{P_{\mathrm{NO}} \cdot\left(P_{\mathrm{O}_{2}}\right)^{1 / 2}} \dots(1) \)
\(
K_{2}=\frac{\left(P_{\mathrm{NO}}\right)^{2} \cdot \mathrm{Po}_{2}}{\left(P_{\mathrm{NO}_{2}}\right)^{2}} \dots(2)
\)
taking square root on both sides in eq. 2
\(
\begin{aligned}
&\Rightarrow \sqrt{K_{2}}=\frac{P_{\mathrm{NO}} \cdot\left(\mathrm{Po}_{2}\right)^{1 / 2}}{P_{\mathrm{NO}_{2}}} \\
&\Rightarrow \sqrt{K_{2}}=\frac{1}{K_{1}} ; \Rightarrow K_{2}=\frac{1}{K_{1}^{2}}
\end{aligned}
\)

Hint

(c)

\(
K_{s p}=3.2 \times 10^{-11}
\)
\(
A X_2 \rightleftharpoons A^{2+}+2 X^{-}
\)
\(
\quad \quad \quad \quad \quad s \quad 2 s
\)
\(
K_{s p}=s \times(2 s)^2=4 s^3 ; \text { i.e., } 3.2 \times 10^{-11}=4 s^3
\)
\(
\begin{array}{ll}
\text { or, } & s^3=0.8 \times 10^{-11}=8 \times 10^{-12} \\
\therefore & s=2 \times 10^{-4}
\end{array}
\)

Hint

(d) Let us consider the formation of a salt of a weak acid and a strong base.
\(\mathrm{In}^{-}+\mathrm{H}_{2} \mathrm{O} \rightleftharpoons \mathrm{HIn}+\mathrm{OH}^{-}\)
\(
K_{h}=\frac{[\mathrm{HIn}]\left[\mathrm{OH}^{-}\right]}{\left[\mathrm{In}^{-}\right]} \dots(i)
\)
Other equations present in the solution are
\(\begin{aligned} \mathrm{HIn} & \rightleftharpoons \mathrm{H}^{+}+\mathrm{In}^{-} \\ \mathrm{H}_{2} \mathrm{O} & \rightleftharpoons \mathrm{H}^{+}+\mathrm{OH}^{-} \\ K_{\mathrm{In}} &=\frac{\left[\mathrm{H}^{+}\right]\left[\mathrm{In}^{-}\right]}{[\mathrm{HIn}]} \dots(ii) \\ K_{w}=& {\left[\mathrm{H}^{+}\right]\left[\mathrm{OH}^{-}\right] } \dots(iii) \\ \text {From (ii) and (iii) } \end{aligned}\)
\(
\begin{aligned}
&\frac{K_{w}}{K_{\mathrm{ln}}}=\frac{[\mathrm{HIn}]\left[\mathrm{OH}^{-}\right]}{\left[\mathrm{In}^{-}\right]}=K_{h} \dots(iv) \\
&{\left[\mathrm{OH}^{-}\right]=\frac{K_{w}}{K_{\mathrm{In}}} \frac{\left[\mathrm{In}^{-}\right]}{[\mathrm{HIn}]}}
\end{aligned}
\)

\(
\begin{array}{l}
\log \left[\mathrm{OH}^{-}\right]=\log K_w-\log K_{\text {In }}+\log \frac{\left[\mathrm{In}^{-}\right]}{[\mathrm{HIn}]} \\
-\mathrm{pOH}=-\mathrm{p} K_w+\mathrm{p} K_{\text {In }}+\log \frac{\left[\operatorname{In}^{-}\right]}{[\mathrm{HIn}]} \\
\mathrm{p} K_w-\mathrm{pOH}=\mathrm{p} K_{\text {In }}+\log \frac{\left[\operatorname{In}^{-}\right]}{[\mathrm{HIn}]} \\
\text { or, } \mathrm{pH}=\mathrm{p} K_{\text {In }}+\log \frac{\left[\operatorname{In}^{-}\right]}{[\mathrm{HIn}]} \\
\text { i.e. } \log \frac{\left[\operatorname{In}^{-}\right]}{[\mathrm{HIn}]}=\mathrm{pH}-\mathrm{p} K_{\text {In }} \\
\end{array}
\)

Hint

(b) \(3 \mathrm{H}_{2}+\mathrm{N}_{2} \rightarrow 2 \mathrm{NH}_{3}\)
\(\begin{array}{ccc}3 & & 1 & & 2 \\ 3 / 2 & & 1 / 2 & & 1\end{array}\)
\(10 \times \frac{3}{2} \quad 10 \times \frac{1}{2} \quad 10 \times 1\)
\(15 \quad \quad \quad 5 \quad \quad \quad 10\)
Composition of gaseous mixture under the aforesaid condition in the end
\(
\begin{aligned}
&\mathrm{H}_{2}=30-15=15 \text { litres } \\
&\mathrm{N}_{2}=30-5=25 \text { litres } \\
&\mathrm{NH}_{3}=10 \text { litres }
\end{aligned}
\)

Hint

(c) \(\mathrm{N}_{2(g)}+3 \mathrm{H}_{2(g)} \rightleftharpoons 2 \mathrm{NH}_{3(g)}\)
\(
K_{c}=\frac{\left[\mathrm{NH}_{3}\right]^{2}}{\left[\mathrm{~N}_{2}\right]\left[\mathrm{H}_{2}\right]^{3}} ; \quad \Delta n=2-4=-2
\)
Thus the reaction will shift in forward direction when \(Q>K_{c}\)

Hint

(d) Due to strong hydrogen-fluorine bond, proton is not given off easily and hence, \(\mathrm{HF}\) is weakest acid

Hint

(b) AgI \(\rightleftharpoons \mathrm{Ag}^{+}+\mathrm{I}^{-}\)
\(
1.0 \times 10^{-16}=s \times s
\)
Solubility of \(\mathrm{Ag}^{+}=1.0 \times 10^{-8} \mathrm{~mol} \mathrm{~L}^{-1}\)
Solubility of \(\mathrm{AgI}\) in \(\mathrm{KI}\) solution \(=1.0 \times 10^{-8} \times 10^{-4}\) \(=1.0 \times 10^{-12} \mathrm{~mol} \mathrm{~L}^{-1}\)

Hint

(c) Pressure of \(\mathrm{O}_{2}\) does not depend on concentration terms of other reactants (because both are in solid state), since this is an endothermic reaction. If the temperature be raised dissociation of \(\mathrm{BaO}_{2}\) would occur, more \(\mathrm{O}_{2}\) is produced at equilibrium, pressure of \(\mathrm{O}_{2}\) increases

Hint

(d) If \(s\) is the solubility of the electrolyte \(M X_{2}\)
\(
C_{M_{2+}}=s, C_{X-}=2 s
\)
Solubility product, \(K_{s p}=s \times(2 s)^{2}=4 s^{3}\);
\(
\begin{aligned}
&s=0.5 \times 10^{-4} \mathrm{~mole} / \mathrm{litre} \\
&\therefore \quad K_{s p}=4 \times\left(0.5 \times 10^{-4}\right)^{3} ; K_{s p}=5 \times 10^{-13}
\end{aligned}
\)

Hint

(b) \(\mathrm{NH}_{4} \mathrm{OH}\) is a weak base but \(\mathrm{HCl}\) is a strong acid in solution, so \(\mathrm{pH}\) of \(\mathrm{NH}_{4} \mathrm{Cl}\) solution is comparatively low.

\(\mathrm{NaNO}_3\) is a salt of strong base and strong acid, so \(\mathrm{pH}\) of the solution will be 7 .
Hydrolysis of potassium acetate (a salt of a weak acid and a strong alkali) gives a weakly alkaline solution, since the acetate ion acts as a weak base.
\(
\mathrm{CH}_3 \mathrm{COOK}+\mathrm{H}_2 \mathrm{O} \rightarrow \mathrm{CH}_3 \mathrm{COOH}+\mathrm{K}^{+}+\mathrm{OH}^{-}
\)
The \(\mathrm{pH}\) of this solution \(\approx 8.8\).
Hydrolysis of sodium carbonate (a salt of strong alkali and a weak acid) gives an alkaline solution
\(
\mathrm{Na}_2 \mathrm{CO}_3+2 \mathrm{H}_2 \mathrm{O} \rightarrow 2\left(\mathrm{Na}^{+}+\mathrm{OH}^{-}\right)+\mathrm{H}_2 \mathrm{CO}_3
\)
The \(\mathrm{pH}\) of this solution is \(>10\).

Hint

(b) Solution of \(0.1 \mathrm{~N} \mathrm{NH}_{4} \mathrm{OH}\) and \(0.1 \mathrm{NNH}_{4} \mathrm{Cl}\) is a buffer solution.

According to Henderson equation, the \(\mathrm{pH}\) of a basic buffer,
\(
\begin{aligned}
& \mathrm{pH}=14-\mathrm{p} K_{b}-\log \frac{C_{\text {salt }}}{C_{\text {base }}} \\
\Rightarrow & \mathrm{p} K_{b}=14-\mathrm{pH}-\log \frac{C_{\text {salt }}}{C_{\text {base }}} \\
\Rightarrow & \mathrm{p} K_{b}=14-9.25-\log \frac{0.1}{0.1} \\
\Rightarrow & \mathrm{p} K_{b}=14-9.25=4.75 \\
\therefore & \mathrm{p} K_{b} \text { of } \mathrm{NH}_{4} \mathrm{OH}=4.75
\end{aligned}
\)

Hint

(c) Proton accepting tendency is known as the strength of basicity.

In \(\mathrm{R}-\mathrm{NH}_{2}, \mathrm{~N}\) has lone pair of electron which intensify due to electron releasing \(R\)-group and increase the tendency to donate lone pair of electrons to \(\mathrm{H}^{+}\).

Secondly as the size of the ion increases there is less attraction for \(\mathrm{H}^{+}\)to form weaker bonds with \(\mathrm{H}\) – atom and are less basic. The order of the given series: \(\mathrm{R-NH}_{2}>\mathrm{NH}_{3}>\mathrm{HS}^{-}>\mathrm{I}^{-}\)

Hint

(d) \(\mathrm{CH}_{3} \mathrm{COOH} \rightleftharpoons \mathrm{CH}_{3} \mathrm{COO}^{-}+\mathrm{H}^{+}\)
\(
\begin{aligned}
&K_{\text {ion }}=\frac{\left[\mathrm{CH}_{3} \mathrm{COO}^{-}\right]\left[\mathrm{H}^{+}\right]}{\left[\mathrm{CH}_{3} \mathrm{COOH}]\right.} \\
&{\left[\mathrm{CH}_{3} \mathrm{COOH}\right]=\frac{3.4 \times 10^{-4} \times 3.4 \times 10^{-4}}{17 \times 10^{-5}}=6.8 \times 10^{-3}}
\end{aligned}
\)

Hint

(b) For reaction,
\(
M_{2} \mathrm{~S} \rightleftharpoons 2 M^{+}+\mathrm{S}^{2-}
\)
Solubility \(=3.5 \times 10^{-6}\)
Solubility product, \(K_{s p}=\left[M^{+}\right]^{2}\left[\mathrm{~S}^{2-}\right]\)
\(
=(2 s)^{2} s=4 s^{3}=4 \times\left(3.5 \times 10^{-6}\right)^{3}=1.7 \times 10^{-16}
\)

Hint

(b) (i) \(\mathrm{H}_2 A \stackrel{K_{a_1}}{\rightleftharpoons} \mathrm{H} A^{-}+\mathrm{H}^{+}\)
(ii) \(\mathrm{HA}^{-} \stackrel{K_{a_{2}}}{\rightleftharpoons} \mathrm{A}^{2-}+\mathrm{H}^{+}\)

In the \(1^{\text {st }}\) step \(\mathrm{H}^{+}\)ion comes from neutral molecule, while in the \(2^{\text {nd }}\) step the \(\mathrm{H}^{+}\)ion comes from negatively charged ions. The presence of -ve charge makes the removal \(\mathrm{H}^{+}\)ion difficult. Thus, \(K_{a_1}>K_{a_2}\).

Hint

(b) For a reaction, \(A+B \rightleftharpoons C+D\).
\(
K_{\mathrm{eq}}=\frac{[C][D]}{[A][B]}
\)
Increase in conc. of reactants will proceed the equilibrium in the forward direction giving more products. So that the equilibrium constant value remains constant and independent of concentration.

Hint

(d) \(\mathrm{NH}_{2}^{-}+\mathrm{H}^{+} \rightarrow \mathrm{NH}_{3}\) (conjugate acid)
Substance \(+ \mathrm{H}^{+} \rightarrow\) conjugate acid
Substance \(-\mathrm{H}^{+} \rightarrow\) conjugate base

Hint

(b) After mixing \(1 \mathrm{~N}\) solution of \(\mathrm{CH}_{3} \mathrm{COOH}\) (weak acid) and \(1 \mathrm{~N} \mathrm{~NaOH}\) (strong base), the resulting solution will have free \(\mathrm{OH}^{-}\)ions. Thus \(\mathrm{pH}\) will be higher than 7

Hint

(a) \(K_{p}=P_{\mathrm{CO}_{2}}\)
Solids do not exert pressure, so their partial pressure is taken as unity

Explanation:

\(
\begin{array}{l}
\mathrm{MgCO}_3(\mathrm{~s}) \rightleftharpoons \mathrm{MgO}(\mathrm{s})+\mathrm{CO}_2(\mathrm{~g}) \\
\text { Now, } \mathrm{K}_{\mathrm{P}}=\frac{\mathrm{P}_{\mathrm{CO}_2} \cdot \mathrm{P}_{\mathrm{MgO}}}{\mathrm{P}_{\mathrm{MgCO}_3}}
\end{array}
\)

But, \(\mathrm{MgCO}_3\) and \(\mathrm{MgO}\) are pure solids.
So, \(\mathrm{K}_{\mathrm{P}}=\mathrm{P}_{\mathrm{CO}_2}\).

Hint

(d) \(\mathrm{CH}_{3} \mathrm{COOH} \rightleftharpoons \mathrm{CH}_{3} \mathrm{COO}^{-}+\mathrm{H}^{+}\)
Weak acid                                    Conjugate base
As \(\mathrm{CH}_{3} \mathrm{COOH}\) is the weakest acid, so its conjugate base \(\left(\mathrm{CH}_{3} \mathrm{COO}^{-}\right)\) is the strongest base. \(\mathrm{H}_{2} \mathrm{SO}_{4}, \mathrm{HCl}\), \(\mathrm{HNO}_{3}\) are strong acids, so their conjugate bases are weak

Hint

(a) \(\left[\mathrm{H}^{+}\right]=\mathrm{C} \alpha=0.1 \times 0.02=2 \times 10^{-3} \mathrm{M}\) (As degree of dissociation \(=2 \%=0.02\) )

\(
\begin{array}{l}
\mathrm{K}_{\mathrm{W}}=\left[\mathrm{H}^{+}\right]\left[\mathrm{OH}^{-}\right]\\
10^{-14}=2 \times 10^{-3} \times\left[\mathrm{OH}^{-}\right]
\end{array}
\)
Hence \(\left[\mathrm{OH}^{-}\right]=\frac{10^{-14}}{2 \times 10^{-3}}=5 \times 10^{-12} \mathrm{M}\)

Hint

(d) For \(\mathrm{CaF}_{2}\), decomposition is as follows:
\(
\begin{aligned}
& \mathrm{CaF}_{2} \rightarrow \mathrm{Ca}^{2+}+2 \mathrm{~F}^{-} \\
& s \quad \quad \quad \quad s \quad \quad \quad 2 s \\
\Rightarrow & K_{s p}=\left[\mathrm{Ca}^{2+}\right]\left[\mathrm{F}^{-}\right]^{2}=s \times(2 s)^{2} \\
\text { or, } & K_{s p}=4 s^{3} \Rightarrow K_{s p}=4 s^{3}=4 \times\left(2 \times 10^{-4}\right)^{3} \\
\Rightarrow & K_{s p}=32 \times 10^{-12}
\end{aligned}
\)

Hint

(d) Given,
\(
\begin{aligned}
&\mathrm{XeF}_{6}+\mathrm{H}_{2} \mathrm{O} \Longrightarrow \mathrm{XeOF}_{4}+2 \mathrm{HF}, K_{\mathrm{eq}}=K_{1} \\
&\mathrm{XeOF}_{4}+2 \mathrm{HF} \rightleftharpoons \mathrm{XeF}_{6}+\mathrm{H}_{2} \mathrm{O}, K_{\text {eq }}=1 / K_{1} \dots(1)
\end{aligned}
\)
and \(\mathrm{XeO}_{4}+\mathrm{XeF}_{6} \rightleftharpoons \mathrm{XeOF}_{4}+\mathrm{XeO}_{3} \mathrm{~F}_{2}, K_{\text {eq }}=K_{2} \dots(2) \)
The reaction, \(\mathrm{XeO}_{4}+2 \mathrm{HF} \rightleftharpoons \mathrm{XeO}_{3} \mathrm{~F}_{2}+\mathrm{H}_{2} \mathrm{O}\), can be obtained by adding eq. (1) and eq.(2).

So, the equilibrium constant for the above reaction can be obtained by multiplying the equilibrium constants of eq. (1) and eq. (2)

\(
\text { Hence, the value is }=\frac{K_2}{K_1}
\)

Hint

(b) \( \mathrm{pH}=\mathrm{p} K_{a}+\log \frac{[\text { Salt }]}{[\text { Acid }]}\)
For small concentration of buffering agent and for maximum buffer capacity [Salt] \(/[\) Acid \(] \approx 1\) i.e., \(\mathrm{pH}=\mathrm{p} K_{a}\)

Hint

(d) \(\mathrm{NaH}+\mathrm{H}_{2} \mathrm{O} \rightarrow \mathrm{NaOH}+\mathrm{H}_{2}\)
or, \(\mathrm{H}_{(a q)}^{-}+\mathrm{H}_{2} \mathrm{O}_{(l)} \rightarrow \mathrm{OH}^{-}+\mathrm{H}_{2} \uparrow\)
Hydride ions will abstract proton from \(\mathrm{NaOH}\) and hydrogen gas will evolve as a result of it

Hint

(b) The greater the solubility product, the greater is the solubility.

For CuS & HgS \(Ksp=S^2\) (where \(\mathrm{s}=\) solubility)
For \(\mathrm{Ag}_2 \mathrm{~S} ; \mathrm{Ksp}=4 \mathrm{S}^3\) ( \(\mathrm{S}=\) solubility)
Now put the values of Ksp and check in which case the value of \(S\) is highest & lowest.

Solubility of CuS: \(\left(10^{-31}\right)^{1 / 2}=\left(10^{-31 / 2}\right)\);
\(
\mathrm{Ag}_2 \mathrm{~S}:\left(10^{-44}\right)^{1 / 3}=\left(10^{-44 / 3}\right) ;
\)
\(\mathrm{HgS}\);
\(
\left(10^{-54}\right)^{1 / 2}=\left(10^{-54 / 2}\right)=\left(10^{-27}\right) ;
\)

The order of solubility will be as per above values:
Hence, the order of solubllity is: \(\mathrm{Ag}_2 \mathrm{~S}>\mathrm{CuS}>\mathrm{HgS}\)

Hint

(c) The equilibrium constant for the reverse reaction will be \(1 / K\)

Hint

 (d) At high temperature, the value of ionic product increases.

Hint

\(
\text { (c)Total number of moles }=2(1-\alpha)+2 \alpha=2
\)

Hint

(b) Since \(\mathrm{NaOH}\) is a strong base, therefore it completely ionises. Thus, the hydroxyl ion concentration is equal to that of the base itself. We know that concentration of \(\mathrm{OH}^{-}\)in \(N / 10 \mathrm{~NaOH}=\) \(0.1=10^{-1}\). Therefore value of
\(
\begin{aligned}
&\mathrm{H}_{3} \mathrm{O}^{+}=\frac{K_{w}}{\left[\mathrm{OH}^{-}\right]}=\frac{1 \times 10^{-14}}{10^{-1}}=1 \times 10^{-13} \\
&\mathrm{pH}=-\log \left[\mathrm{H}_{3} \mathrm{O}^{+}\right]=-\log \left[1 \times 10^{-13}\right]=13
\end{aligned}
\)

Hint

(b) In \(\mathrm{BF}_{3}\) and \(\mathrm{FeCl}_{3}\) molecules, the central atoms have incomplete octet and in \(\mathrm{SiF}_{4}\), the central atom has empty \(d\)-orbitals. Hence, according to Lewis concept, these are Lewis acids.

Hint

(d) The \(\mathrm{pH}\) value of the blood is maintained constant by buffer solution present in the blood itself. Buffer solutions resist the change in \(\mathrm{pH}\) values.

The \(p \mathrm{H}\) of the blood does not appreciably change by small addition of acid or a base because blood contains serum protein which acts as a buffer.

Hint

(c) Since \(\mathrm{HCl}\) is a strong acid and it completely ionises, therefore \(\mathrm{H}_{3} \mathrm{O}^{+}\)ions concentration is equal that of the acid itself i.e. \(\left[\mathrm{H}_{3} \mathrm{O}^{+}\right]=[\mathrm{HCl}]=10 \mathrm{~M}\)

\(
\text { Therefore } \mathrm{pH}=-\log \left[\mathrm{H}_3 \mathrm{O}^{+}\right]=-\log [10]=-1
\)

Hint

(a) There are greater number of particles (i.e. ions) compared to others. Hence, solubility will be minimum.

Hint

(a)  Vapour pressure is directly related to temperature. Greater is the temperature, greater will be the vapour pressure. So to keep it constant, temperature should be constant.

Hint

(d) higher the value of he solubility product, greater is the solubility

Hint

(a) \(\left[\mathrm{H}_{3} \mathrm{O}^{+}\right]=[\mathrm{OH}^{-}]=1 \times 10^{-6}\) mole/litre
\(
K_{W}=\left[\mathrm{H}_{3} \mathrm{O}^{+}\right]\left[\mathrm{OH}^{-}\right]=\left[1 \times 10^{-6}\right] \times\left[1 \times 10^{-6}\right]=1 \times 10^{-12}
\)

Hint

(d) When solid and liquid are in equilibrium, the increase in temperature results in increase in volume of liquid or decrease in the amount of solid.
Solid \(\rightleftharpoons\) Liquid
With increase in temperature equilibrium shifts in forward direction.

Hint

(a) Sodium borate is a salt formed from strong base \((\mathrm{NaOH})\) and weak acid \(\left(\mathrm{H}_{3} \mathrm{BO}_{3}\right)\). Hence, sodium borate will act basic.

Hint

(d) According to Le Chateliers principle, if an equilibrium is subjected to change in concentration, pressure or temperature, etc. equilibrium shift in such a way so as to undo the effect of a change imposed.

Hint

\(
\text { (b) } \mathrm{CH}_{3} \mathrm{COOH}+\mathrm{H}_{2} \mathrm{O} \rightleftharpoons \mathrm{CH}_{3} \mathrm{COO}^{-}+\mathrm{H}_{3} \mathrm{O}^{+}
\)

Hint

(a)

\(
\begin{array}{ll}
\mathrm{N}_2+\mathrm{O}_2 \rightleftharpoons 2 \mathrm{NO}, & \mathrm{K}_1 \\
\mathrm{NO} \rightleftharpoons \frac{1}{2} \mathrm{~N}_2+\frac{1}{2} \mathrm{O}_2, & \mathrm{~K}_2
\end{array}
\)

The reaction is halved and reversed
\(
\mathrm{K}_2=\sqrt{\frac{1}{\mathrm{~K}_1}} \Rightarrow K_1=\left(\frac{1}{K_2}\right)^2
\)

Hint

(c) \(\mathrm{NH}_{4} \mathrm{Cl}\) and \(\mathrm{NaHCO}_{3}\) are acidic in nature and \(\mathrm{NaCl}\) is neutral. Only \(\mathrm{Na}_{2} \mathrm{CO}_{3}\) is basic and thus have highest \(\mathrm{pH}\).

Explanation:

\(\mathrm{NaCl}\) is a salt of strong acid and strong base hence its aqueous solution will be neutral ie \(\mathrm{pH}=7 . \mathrm{NaHCO}_3\) is an acidic salt hence \(\mathrm{pH}<7 . \mathrm{Na}_2 \mathrm{CO}_3\) is a salt of weak acid and strong base. Hence its aqueous solution will be strongly basic ie. \(\mathrm{pH}>7 . \mathrm{NH}_4 \mathrm{Cl}\) is salt of weak base and strong acid, hence its aqueous solution will be strongly acidic i.e. \(\mathrm{pH}<7\).

Hint

(d) \(\mathrm{HCl}\) cannot accept \(\mathrm{H}^{+}\)therefore cannot act as Bronsted base.

Hint

(b) When a proton is removed from an acid, we obtain its conjugate base.
\(
\begin{array}{l}
\mathrm{H}_2 \mathrm{O} \rightleftharpoons \mathrm{H}^{+}+\mathrm{OH}^{-} \\
\mathrm{HF} \rightleftharpoons \mathrm{H}^{+}+\mathrm{F}^{-}
\end{array}
\)

Hint

(c)

\(
\begin{array}{lcc}
\mathrm{CaF}_2 & \rightleftharpoons \quad \mathrm{Ca}^{2+}+2 \mathrm{~F}^{-} \\
t=0 & 0 \quad \quad  \quad \quad 0 \\
\text { At eqm. } & s \quad \quad \quad \quad 2 s
\end{array}
\)
\(
\underset{0.1}{\mathrm{NaF}} \longrightarrow \underset{0.1} {\mathrm{Na}^{+}}+\underset{0.1} {\mathrm{F}^{-}}
\)
Due to common ion effect of \(\mathrm{NaF}\), solubility of \(\mathrm{CaF}_2\) is further supressed. Therefore, the concentration of \(\mathrm{F}^{-}\)will be mainly due to \(\mathrm{NaF}\).
\(
\begin{array}{l}
K_{s p}=\left[\mathrm{Ca}^{2+}\right]\left[\mathrm{F}^{-}\right]_2 \\
K_{s p}=(\mathrm{s})(0.1+2 \mathrm{~s})^2 \quad 0.1+2 \mathrm{~s} \approx 0.1 \\
s=\frac{K_{\mathrm{sp}}}{(0.1)^2}=\frac{5.3 \times 10^{-11}}{(0.1)^2}=5.3 \times 10^{-9} \mathrm{~mol} \mathrm{~L}^{-1}
\end{array}
\)

Hint

(d)
\(
\underset{s} {\mathrm{Ni}(\mathrm{OH})^2} \rightleftharpoons \underset{s}{\mathrm{Ni}^{2+}}+\underset{2 s}{2 \mathrm{OH}^{-}}
\)
\(
\begin{array}{l}
\quad \mathrm{NaOH} \longrightarrow \mathrm{Na}+\mathrm{OH}^{-} \\
\text {Total }\left[\mathrm{OH}^{-}\right]=2 s+0.1 \approx 0.1 \\
\text { Ionic product }=[\mathrm{Ni}]^{2+}[\mathrm{OH}]^2 \\
2 \times 10^{-15}=s(0.1)^2 \\
s=2 \times 10^{-13} \\
\text { Solubility of } \mathrm{Ni}(\mathrm{OH})_2=2 \times 10^{-13} \mathrm{M}
\end{array}
\)

Hint

\(
\begin{array}{l}
\mathrm{K}_{\mathrm{a}}=\mathrm{C} \alpha^2 \\
\mathrm{~K}_{\mathrm{a}}=(0.1) \times(0.01)^2 \\
\mathrm{~K}_{\mathrm{a}}=1 \times 10^{-5}
\end{array}
\)

Hint

(a) Acidic buffer is prepared by mixing weak acid and its salt with strong base.

Hint

(b)

\(
\quad \quad \quad \quad \quad \quad \mathrm{A}+\mathrm{B} \rightleftharpoons \mathrm{C}+\mathrm{D}
\)
\(
\text { at equilibrium } \begin{array}{llll}
2 & 3 & 10 & 6
\end{array}
\)
\(
\begin{aligned}
K_{\text {eq }} & =[C][D]/[A][B] \\
K_{\text {eq }} & =\frac{10 \times 6}{2 \times 3}=10 \\
\Delta G^0 & =-R T \ln \mathrm{K} \\
& =-2.303 \mathrm{RT} \log \mathrm{K} \\
& =-2.303 \times 2 \times 300 \times \log 10 \\
& =-1381.8 \mathrm{~cal}
\end{aligned}
\)

Hint

(d) For weak acid (i.e. \(\mathrm{CH}_3 \mathrm{COOH}\) )
\(
\begin{array}{l}
{\left[H^{+}\right]=C \alpha} \\
=0.01 \times \frac{1}{100}=10^{-4} \mathrm{M} \\
p H=-\log H^{+}=-\log 10^{-4}=4
\end{array}
\)

Hint

(b) According to Henry’s Law,
\(
p=K_H X
\)
Where ‘ \(p\) ‘ is partial pressure of gas in vapour phase.
\(\mathrm{K}_{\mathrm{H}}\) is Henry’s Law constant.
‘ \(X\) ‘ is mole fraction of gas in liquid.

Higher the value of \(K_H\) at a given pressure, lower is the solubility of the gas in the liquid.
\(\therefore\) Solubility : \(\mathrm{Ar}<\mathrm{CO}_2<\mathrm{CH}_4<\mathrm{HCHO}\)

Hint

(b)

\(
\begin{array}{l}
\mathrm{CO}_2(\mathrm{~g})+\mathrm{C}(\mathrm{s}) \rightleftharpoons 2 \mathrm{CO}(\mathrm{g}) \\
\Delta \mathrm{n}_{\mathrm{g}}=2-1=1 \\
\mathrm{~K}_{\mathrm{p}}=\mathrm{K}_{\mathrm{c}}(\mathrm{RT})^{\Delta \mathrm{n}_{\mathrm{g}}} \\
\mathrm{K}_{\mathrm{p}}=\mathrm{K}_{\mathrm{c}}(\mathrm{RT}) \\
{\left[\because \mathrm{K}_{\mathrm{p}}=3\right]} \\
\mathrm{K}_{\mathrm{c}}=\frac{\mathrm{K}_{\mathrm{p}}}{\mathrm{RT}}=\frac{3}{0.083 \times 1000} \\
=0.036 \\
=3.6 \times 10^{-2}
\end{array}
\)

Hint

(a) It is a mixture of weak acid and salt of its conjugate base. Hence it is acidic buffer. Weak acid \(\left(\mathrm{CH}_3 \mathrm{COOH}\right)\) and salt of weak acid-strong base \(\left(\mathrm{CH}_3 \mathrm{COONa}\right)\) form an acidic buffer. Sodium acetate \(\left(\mathrm{CH}_3 \mathrm{COONa}\right)=0.10 \mathrm{M}\); Acetic acid \(\left(\mathrm{CH}_3 \mathrm{COOH}\right)=0.01 \mathrm{M}\); \(\mathrm{pH}\) of acidic buffer solution is given by
\(
\begin{array}{l}
p H=p K_a+\log \frac{[\text { Salt }]}{[\text { Acid }]} \\
=4.57+\log \left(\frac{0.1}{0.01}\right) \\
=4.57+1 \\
=5.57
\end{array}
\)

Hint

(d) \(
\begin{array}{l}
3 \mathrm{O}_2(\mathrm{~g}) \rightleftharpoons 2 \mathrm{O}_3(\mathrm{~g}) \\
\mathrm{K}_{\mathrm{c}}=\frac{\left[\mathrm{O}_3\right]^2}{\left[\mathrm{O}_2\right]^3} \\
3 \times 10^{-59}=\frac{\left[\mathrm{O}_3\right]^2}{\left(4 \times 10^{-2}\right)^3} \\
{\left[\mathrm{O}_3\right]^2=3 \times 10^{-59} \times 64 \times 10^{-6}} \\
=19.2 \times 10^{-64} \\
=4.38 \times 10^{-32} \mathrm{M}
\end{array}
\)

Hint

(d) \(\Delta G=\Delta G^{\circ}+R T \ln Q\)

At equilibrium \(\Delta G=0, Q=K_{\text {eq }}\)
So, \(\Delta_r G^{\circ}=-R T \ln K_{e q}\)
\(\Delta_r G^{\circ}=-8.314 \mathrm{~J} \mathrm{~mol}^{-1} \mathrm{~K}^{-1} \times 300 \mathrm{~K} \times \ln \left(2 \times 10^{13}\right)\)

Hint

(d)

\(
\begin{array}{l}
{\left[\mathrm{OH}^{-}\right]=0.01 \mathrm{M}=10^{-2} \mathrm{M}} \\
\mathrm{pOH}=-\log \left[\mathrm{OH}^{-}\right]=-\log \left(10^{-2}\right)=2 \\
\mathrm{pH}=14-\mathrm{pOH}=12
\end{array}
\)

Hint

(a)

\(
\mathrm{Ca}(\mathrm{OH})_2 \rightleftharpoons \mathrm{Ca}^{2+}+2 \mathrm{OH}^{-}
\)
\(
\begin{array}{l}
\mathrm{pH}=9, \mathrm{pOH}=14-9=5 \\
{[\mathrm{OH}^{-}]=10^{-5}} \\
{\left[\mathrm{Ca}^{2+}\right]=\frac{10^{-5}}{2}} \\
K_{s p}=\left[\mathrm{Ca}^{2+}\right]\left[\mathrm{OH}^{-}\right]_2=\left(\frac{10^{-5}}{2}\right) \times\left(10^{-5}\right)^2 \\
\quad=0.5 \times 10^{-15}
\end{array}
\)

Hint

(a) 

\(
\mathrm{A}_2(\mathrm{~g})+\mathrm{B}_2(\mathrm{~g}) \rightleftharpoons \mathrm{X}_2(\mathrm{~g}) \Delta_{\mathrm{r}} H=-X \mathrm{~kJ}
\)
On increasing pressure equilibrium shifts in a direction where number of moles decreases i.e. forward direction.
On decreasing temperature, equilibrium shifts in exothermic direction i.e., forward direction.
So, high pressure and low temperature favours maximum formation of product.

Hint

(c) 

\(
\text { Meq. of } \mathrm{HCl}=75 \times \frac{1}{5} \times 1=15
\)
\(
\begin{array}{l}
\text { Meq. of } \mathrm{NaOH}=25 \times \frac{1}{5} \times 1=5 \\
\text { Meq. of } \mathrm{HCl} \text { in resulting solution }=10 \\
\text { Molarity of }\left[\mathrm{H}^{+}\right] \text {in resulting mixture }=\frac{10}{100}=\frac{1}{10}
\end{array}
\)
\(
\mathrm{pH}=-\log \left[\mathrm{H}^{+}\right]=-\log \left[\frac{1}{10}\right]=1.0
\)

Hint

\(
\begin{array}{l}
\text { (a) Solubility of } \mathrm{BaSO}_4=2.42 \times 10^{-3} \mathrm{~gL}^{-1} \\
\therefore \quad s=\frac{2.42 \times 10^{-3}}{233}=1.038 \times 10^{-5} \mathrm{~mol} \mathrm{~L}^{-1} \\
K_{s p}=s^2=\left(1.038 \times 10^{-5}\right)^2=1.08 \times 10^{-10} \mathrm{~mol}^2 \mathrm{~L}^{-2}
\end{array}
\)

Hint

(d)

Dimethylammonium acetate is a salt of weak acid and weak base whose \(\mathrm{pH}\) can be calculated as
\(
\mathrm{pH}=7+\frac{1}{2}\left(\mathrm{pK}_{\mathrm{a}}-\mathrm{pK}_{\mathrm{b}}\right)
\)
\(\mathrm{pK}_{\mathrm{a}}\) of acetic acid \(=4.77\)
\(\mathrm{pK}_{\mathrm{b}}\) of dimethyl amine \(=3.27\)
\(
\mathrm{pH}=7+\frac{1}{2}(4.77-3.27)
\)
\(
=7.75
\)

Hint

To find the relationship between \(K _1\) and \(K _2\), let’s carefully analyze the given equilibrium reactions and their constants.

First, let’s write down the equilibrium reactions clearly:
Reaction 1: \(3 A_2+ B _2 \rightleftharpoons 2 A_3 B\) with equilibrium constant \(K _1\).
Reaction 2: \(A _3 B \rightleftharpoons \frac{3}{2} A_2+\frac{1}{2} B_2\) with equilibrium constant \(K _2\).
Now, understand that \(K_2\) represents the equilibrium constant of the reverse reaction of Reaction 1 but with both sides divided by 2 . We need to relate these constants. Here is the step-by-step process:
We know Reaction 1 is: \(3 A_2+ B _2 \rightleftharpoons 2 A_3 B\).
The equilibrium constant for Reaction 1 is: \(K _1=\frac{\left[ A _3 B\right]^2}{\left.\left.\left[A_2\right]^3\right]_2\right]}\).
For Reaction 2: \(A _3 B \rightleftharpoons \frac{3}{2} A_2+\frac{1}{2} B_2\), the equilibrium constant \(K _2\) can be expressed as the equilibrium constant of the reverse of Reaction 1 , with adjusted coefficients.
The equilibrium constant for the reverse reaction of Reaction 1 would be \(\frac{1}{K_1}\). Since Reaction 2 includes halving the coefficients, the equilibrium constant should be adjusted by taking the square root:
\(
K _2=\left(\frac{1}{K_1}\right)^{1 / 2}=\frac{1}{\sqrt{ K _1}}
\)
Therefore, the relationship between \(K _1\) and \(K _2\) is:
Option D: \(K _2=\frac{1}{\sqrt{ K _1}}\).

Hint

(d) The given chemical reaction is an example of the synthesis of ammonia, commonly known as the Haber process:
\(
N _2(g)+3 H _2(g) \rightleftharpoons 2 NH _3(g), \Delta H =- Q
\)
This reaction is exothermic, as indicated by the negative enthalpy change \((\Delta H =- Q )\). In order to determine which conditions favor the forward reaction, we need to consider Le Chatelier’s principle, which states that the system will adjust to counteract any changes imposed upon it.
Let’s analyze each option:
Option A: Use of catalyst
A catalyst does not favor the forward or reverse direction of a reaction. It only speeds up the rate at which equilibrium is achieved.
Option B: Decreasing concentration of \(N _2\)
Decreasing the concentration of \(N _2\) would shift the equilibrium to the left, favoring the reverse reaction to produce more \(N _2\) and \(H _2\).
Option C: Low pressure, high temperature, and high concentration of ammonia
Low pressure and high temperature would favor the reverse reaction. Moreover, a high concentration of ammonia would also shift the equilibrium to the left. Thus, this set of conditions does not favor the forward reaction.
Option D: High pressure, low temperature and higher concentration of \(H _2\)
High pressure favors the formation of ammonia because there are fewer moles of gas on the product side ( 2 moles) as compared to the reactant side ( 4 moles, i.e., 1 mole of \(N _2\) and 3 moles of \(H _2\) ). Low temperature favors the exothermic forward reaction. Additionally, a higher concentration of \(H _2\) will shift the equilibrium to the right, forming more ammonia.
Therefore, the correct answer is:
Option D: High pressure, low temperature and higher concentration of \(H _2\)

Hint

(a) To determine in which of the given equilibria \(K _p\) and \(K _{ c }\) are not equal, it’s important to understand the relationship between these two equilibrium constants. This relationship is expressed by the equation:
\(
K _p= K _c(R T)^{\Delta n}
\)
where \(R\) is the gas constant, \(T\) is the temperature in Kelvin, and \(\Delta n\) is the change in the number of moles of gas (number of moles of gaseous products minus number of moles of gaseous reactants).
If \(\Delta n=0\), then \(K _p\) and \(K _{ c }\) are equal because \((R T)^0=1\). However, if \(\Delta n \neq 0\), the constants will not be the same, and the degree to which they differ will depend on the temperature and the value of \(\Delta n\).

Now, let’s analyze each option:
Option A: \(PCl _{5(g)} \rightleftharpoons PCl _{3(g)}+ Cl _{2(g)}\)
Reactant side moles \(=1\), Product side moles \(=2 ; \Delta n=2-1=1\).
Option B: \(H _{2(g)}+ I _{2(g)} \rightleftharpoons 2 HI _{( g )}\)
Reactant side moles \(=2\), Product side moles \(=2 ; \Delta n=2-2=0\).
Option C: \(CO _{( g )}+ H _2 O _{( g )} \rightleftharpoons CO _{2(g)}+ H _{2(g)}\)
Reactant side moles \(=2\), Product side moles \(=2 ; \Delta n=2-2=0\).
Option D: \(2 BrCl _{( g )} \rightleftharpoons Br _{2(g)}+ Cl _{2(g)}\)
Reactant side moles \(=2\), Product side moles \(=2 ; \Delta n=2-2=0\).
From this analysis, it is evident that \(K _p\) and \(K _{ c }\) are not equal in Option A where \(\Delta n=1\). In all other options, since \(\Delta n=0, K_p\) is equal to \(K _{ c }\). Thus, the correct answer is Option A.

Hint

(c) To determine which option is correct regarding the reaction state and its direction, we need to calculate the reaction quotient \(Q_c\) and compare it to the equilibrium constant \(K_c\). The reaction given is:
\(
2 A \rightleftharpoons B + C
\)
The equilibrium constant expression \(K_c\) for this reaction is:
\(
K_c=\frac{[ B ][ C ]}{[ A ]^2}
\)
Given that \(K_c=4 \times 10^{-3}\) and the concentrations of \(A , B\), and C at this time are each \(2 \times 10^{-3} M\), we can substitute these values into the expression for \(K_c\) to calculate the reaction quotient \(Q_c\) :
\(
Q_c=\frac{\left(2 \times 10^{-3} M \right)\left(2 \times 10^{-3} M \right)}{\left(2 \times 10^{-3} M \right)^2}
\)
Simplifying, we find:
\(
Q_c=\frac{4 \times 10^{-6} M ^2}{4 \times 10^{-6} M ^2}=1
\)
Comparing \(Q_c\) with \(K_c\) :
\(
\begin{aligned}
Q_c & =1 \\
K_c & =4 \times 10^{-3}
\end{aligned}
\)
Since \(Q_c>K_c(1>0.004)\), the reaction quotient is greater than the equilibrium constant. This indicates that the concentration of products ( B and C ) is too high relative to the concentration of reactants (A) for the system to be at equilibrium under these conditions.
This means that the reaction has a tendency to move in the backward direction to reach equilibrium, reducing the concentration of the products ( B and C ) and increasing the concentration of the reactant (A). Therefore, the correct answer to the given question is:
Option C: Reaction has a tendency to go in backward direction.

Hint

(d)

\(
\begin{aligned}
& 2 NO _{( g )} \rightleftharpoons N _{2(g)}+ O _{2(g)} \\
& K _c=\frac{\left[ N _2\right]\left[ O _2\right]}{[ NO ]^2} \\
& =\frac{3 \times 10^{-3} \times 4.2 \times 10^{-3}}{2.8 \times 10^{-3} \times 2.8 \times 10^{-3}} \\
& =1.607
\end{aligned}
\)
\(
\quad \quad \quad 2 NO _{( g )} \rightleftharpoons N _{2(g)}+ O _{2(g)}
\)
\(
\begin{array}{llll}
t=0 & & 0.1 & & & 0 & & & 0
\end{array}
\)
\(
\quad \quad 0.1-0.1 \alpha \quad 0.05 \alpha \quad 0.05 \alpha
\)
\(
\begin{aligned}
& K _c=\frac{0.05 \alpha \times 0.05 \alpha}{(0.1-0.1 \alpha)^2} \\
& K_c=\frac{0.05 \alpha \times 0.05 \alpha}{0.01(1-\alpha)^2} \\
& 1.607=\frac{(0.05)^2 \alpha^2}{0.01(1-\alpha)^2} \\
& \frac{\alpha^2}{(1-\alpha)^2}=\frac{1.607 \times(0.1)^2}{(0.05)^2} \\
& \frac{\alpha}{1-\alpha}=\frac{1.27 \times 0.1}{0.05} \\
& \frac{\alpha}{1-\alpha}=2.54 \\
& \alpha=2.54-2.54 \alpha \\
& 3.54 \alpha=2.54 \\
& \alpha=\frac{2.54}{3.54}=0.717
\end{aligned}
\)

Hint

(c) Titration of Sodium Hydroxide \(( NaOH )\) against Oxalic Acid \(\left( H _2 C _2 O _4\right)\)
Sodium hydroxide is a strong base, and oxalic acid is a weak acid. The reaction between them is a neutralization reaction:
\(
2 NaOH + H _2 C _2 O _4 \longrightarrow Na _2 C _2 O _4+2 H _2 O
\)
Choosing the Right Indicator
An indicator is a substance that changes color at a specific pH range, signaling the endpoint of the titration. The ideal indicator for a titration should have a color change near the equivalence point of the reaction. The equivalence point is the point where the moles of acid and base are stoichiometrically equal.
In this case:
At the equivalence point, the solution will be slightly basic due to the formation of the sodium oxalate salt \(\left( Na _2 C _2 O _4\right)\), which is the conjugate base of a weak acid.
Phenolphthalein changes color in the slightly basic pH range (around 8.2 to 10.0), making it an appropriate indicator for this titration.
Color Change
Phenolphthalein is colorless in acidic solutions and turns pink in basic solutions. So, the color change at the endpoint of this titration will be:
Colorless (before equivalence point) \(\rightarrow\) Pink (at equivalence point)
Why Other Options Are Incorrect
Option A: Phenolphthalein does not change from pink to yellow, it changes from colorless to pink.
Option B: Alkaline \(KMnO _4\) is not a suitable indicator for this titration because it is not sensitive enough to detect the slight pH change at the equivalence point.
Option D: Methyl orange changes color in the acidic pH range (around 3.1 to 4.4 ) and is therefore not suitable for this titration.

Hint

(a) To find the ratio of solubility of AgCl in 0.1 M KCl solution to the solubility of AgCl in water, we first need to understand how common ion effect influences solubility.
Let’s denote the solubility product constant of AgCl as \(K_{\text {sp }}\).
Given \(K_{s p}\) of \(AgCl =10^{-10}\).
First, we calculate the solubility of AgCl in pure water:
In water, the dissociation of AgCl can be represented as:
\(
AgCl ( s ) \leftrightarrow Ag ^{+}( aq )+ Cl ^{-}( aq )
\)
If \(s\) is the solubility of AgCl in water, then
\(
\begin{aligned}
& {\left[ Ag ^{+}\right]=s} \\
& {\left[ Cl ^{-}\right]=s}
\end{aligned}
\)
Hence, the solubility product \(K_{s p}\) can be written as:
\(
\begin{aligned}
& K_{s p}=\left[A g^{+}\right]\left[ Cl ^{-}\right] \\
& K_{s p}=s \cdot s=s^2
\end{aligned}
\)
S0,
\(
\begin{aligned}
& s^2=10^{-10} \\
& s=10^{-5}
\end{aligned}
\)
Now, let’s consider the solubility of AgCl in 0.1 M KCl solution. Because of the common ion effect, the presence of \(Cl ^{-}\)ions from KCl will suppress the solubility of AgCl .
Here, \(\left[ Cl ^{-}\right]\)from KCl is 0.1 M . Let the new solubility of AgCl in this solution be \(s^{\prime}\).
Then,
\(
\begin{aligned}
& {\left[ Ag ^{+}\right]=s^{\prime}} \\
& {\left[ Cl ^{-}\right]=0.1+s^{\prime} \approx 0.1}
\end{aligned}
\)
Since \(s^{\prime}\) is much smaller than 0.1 M , we can approximate:
\(
\begin{aligned}
& K_{s p}=\left[ Ag ^{+}\right]\left[ Cl ^{-}\right] \\
& 10^{-10}=s^{\prime} \times 0.1 \\
& s^{\prime}=\frac{10^{-10}}{0.1} \\
& s^{\prime}=10^{-9}
\end{aligned}
\)
Finally, we find the ratio of solubility in 0.1 M KCl to that in pure water:
\(
\text { Ratio }=\frac{s^{\prime}}{s}=\frac{10^{-9}}{10^{-5}}=10^{-4}
\)