NEET PYQs
Quiz Summary
0 of 99 Questions completed
Questions:
Information
You have already completed the quiz before. Hence you can not start it again.
Quiz is loading…
You must sign in or sign up to start the quiz.
You must first complete the following:
Results
Results
Time has elapsed
Categories
- Not categorized 0%
- 1
- 2
- 3
- 4
- 5
- 6
- 7
- 8
- 9
- 10
- 11
- 12
- 13
- 14
- 15
- 16
- 17
- 18
- 19
- 20
- 21
- 22
- 23
- 24
- 25
- 26
- 27
- 28
- 29
- 30
- 31
- 32
- 33
- 34
- 35
- 36
- 37
- 38
- 39
- 40
- 41
- 42
- 43
- 44
- 45
- 46
- 47
- 48
- 49
- 50
- 51
- 52
- 53
- 54
- 55
- 56
- 57
- 58
- 59
- 60
- 61
- 62
- 63
- 64
- 65
- 66
- 67
- 68
- 69
- 70
- 71
- 72
- 73
- 74
- 75
- 76
- 77
- 78
- 79
- 80
- 81
- 82
- 83
- 84
- 85
- 86
- 87
- 88
- 89
- 90
- 91
- 92
- 93
- 94
- 95
- 96
- 97
- 98
- 99
- Current
- Review
- Answered
- Correct
- Incorrect
-
Question 1 of 99
1. Question
For a given reaction, \(\Delta H=35.5 \mathrm{~kJ} \mathrm{~mol}^{-1}\) and \(\Delta S=83.6 \mathrm{~J} \mathrm{~K}^{-1} \mathrm{~mol}^{-1}\). The reaction is spontaneous at (Assume that \(\Delta H\) and \(\Delta S\) do not vary with temperature.) [NEET 2017]
Hint
(a) For a spontaneous reaction, \(\Delta G<0\) i.e., \(\Delta H-T \Delta S<0\)
\(
\begin{aligned}
T &>\frac{\Delta H}{\Delta S} \\
& T>\left(\frac{35.5 \times 1000}{83.6}=424.6=425 \mathrm{~K}\right) \\
\therefore \quad T &>425 \mathrm{~K}
\end{aligned}
\) -
Question 2 of 99
2. Question
A gas is allowed to expand in a well insulated container against a constant external pressure of \(2.5 \mathrm{~atm}\) from an initial volume of \(2.50 \mathrm{~L}\) to a final volume of \(4.50 \mathrm{~L}\). The change in internal energy \(\Delta U\) of the gas in joules will be [NEET 2017]
Hint
(b) The system is in isolated state.
\(
\begin{array}{l}
\because \text { For an adiabatic process, } q=0 \\
\Delta U=q+w \\
\therefore \Delta U=w \\
=-p \Delta V \\
=-2.5 \mathrm{~atm} \times(4.5-2.5) \mathrm{L} \\
=-2.5 \times 2 \mathrm{~L}-\mathrm{atm} \\
=-5 \times 101.3 \mathrm{~J} \\
=-506.5 \mathrm{~J} \approx-505 \mathrm{~J} \\
\end{array}
\) -
Question 3 of 99
3. Question
For a sample of perfect gas when its pressure is changed isothermally from \(p_{i}\) to \(p_{f}\), the entropy change is given by [NEET-II 2016]
Hint
(b) For an ideal gas undergoing reversible expansion, when temperature changes from \(T_{i}\) to \(T_{f}\) and pressure changes from \(p_{i}\) to \(p_{f}\),
\(
\Delta S=n C_{p} \ln \frac{T_{f}}{T_{i}}+n R \ln \frac{p_{i}}{p_{f}}
\)
For an isothermal process, \(T_{i}=T_{f}\) so, \(\ln 1=0\)
\(
\therefore \quad \Delta S=n R \ln \frac{p_{i}}{p_{f}}
\) -
Question 4 of 99
4. Question
The correct thermodynamic conditions for the spontaneous reaction at all temperatures is [NEET-I 2016]
Hint
(a, c) \(\Delta G=\Delta H-T \cdot \Delta S\)
For a spontaneous reaction \(\Delta G=-\mathrm{ve}\) (always) which is possible only if \(\Delta H<0\) and \(\Delta S>0\)
\(\therefore\) spontaneous at all temperatures.If \(\Delta H<0\) and \(\Delta S=0\)
\(\Delta G=(-\mathrm{ve})-T(0)=-\mathrm{ve}\) at all temperatures. -
Question 5 of 99
5. Question
Consider the following liquid-vapour equilibrium.
Liquid \(\rightleftharpoons\) Vapour
Which of the following relations is correct? [NEET-I 2016]Hint
(b) This is Clausius-Clapeyron equation.
\(
\frac{\mathrm{d} \ln \mathrm{P}}{\mathrm{dT}}=\frac{\Delta \mathrm{H}_{\mathrm{V}}}{\mathrm{RT}^2}
\)According to this equation, the rate at which the natural logarithm of the vapor pressure of a liquid changes with temperature is determined by the molar enthalpy of vaporization of the liquid, the ideal gas constant, and the temperature of the system.
-
Question 6 of 99
6. Question
The heat of combustion of carbon to \(\mathrm{CO}_{2}\) is \(-393.5 \mathrm{~kJ} / \mathrm{mol}\). The heat released upon formation of \(35.2 \mathrm{~g}\) of \(\mathrm{CO}_{2}\) from carbon and oxygen gas is [NEET 2015]
Hint
(d) We can represent the formation of carbon dioxide from carbon and dioxygen by the equation:
\(
\mathrm{C}(\mathrm{s})+\mathrm{O}_2(g) \rightarrow \mathrm{CO}_2(g)
\)The data given in the question is:
\(\Delta_f H=-393.5 \mathrm{~kJ} / \mathrm{mol}\) that is 1 mole of \(\mathrm{CO}_2\) releases \(-393.5 \mathrm{~kJ} / \mathrm{mol}\) heat.To find the heat released upon formation of \(35.2 \mathrm{~g}\) of \(\mathrm{CO}_2\) from carbon and oxygen gas, we have to first find the mass of one molecule of carbon dioxide.
One molecule of carbon dioxide consists of 1 atom of carbon and 2 atoms of oxygen. We can calculate the mass of one molecule of carbon dioxide by adding the masses of 1 atom of carbon and 2 atoms of oxygen.
Mass of carbon atom \(=12\) grams.
Mass of oxygen atom \(=16\) grams.
Mass of one molecule of carbon dioxide \(=12+2(16)=44\) grams.
Heat released by,
\(1 \mathrm{~mole}\) of \(\mathrm{CO}_2 \rightarrow-393.5 \mathrm{~kJ} / \mathrm{mol}\)
44 grams of \(\mathrm{CO}_2 \rightarrow-393.5 \mathrm{~kJ} / \mathrm{mol}\)
1 gram of \(\mathrm{CO}_2 \rightarrow \frac{-393.5}{44} \mathrm{~kJ} / \mathrm{mol}\)
\(35.2 \mathrm{~g}\) of \(\mathrm{CO}_2 \rightarrow \frac{-393.5}{44} \times 35.2 \mathrm{~g}=-315 \mathrm{KJ} / \mathrm{mole}\)Note: Combustion of carbon to \(\mathrm{CO}_2\) is an exothermic reaction. It is denoted by a negative sign which indicates that the heat is released. In contrast, the positive sign denotes absorption of heat.
-
Question 7 of 99
7. Question
Which of the following statements is correct for the spontaneous adsorption of a gas?[NEET 2014]
Hint
(b) The accumulation of the molecules at the surface rather than the bulk of the solid or liquid is called adsorption. We will see the effect on thermodynamic parameters for the adsorption of a gas.
We know that during the process of adsorption, there is always a decrease in the residual forces of the surface. So, there is a decrease in the surface energy which appears as heat. Therefore, we can say that adsorption is an exothermic process.
Now, we know that in the case of spontaneous adsorption of a gas, the free energy \((\Delta G)\) of the reaction can be given as
\(
\Delta G=\Delta H-T \Delta S
\)Now, for a spontaneous reaction, the change in free energy \((\Delta G)\) needs to be negative.
Here, free energy change can be negative only if \(\Delta H\) is sufficiently negative which we can see in the equation.
Also, when a gas is adsorbed, its entropy decreases as the freedom of movement becomes restricted. So, for the adsorption of gas, \(\Delta S\) is negative.
Thus, we can conclude that for a spontaneous adsorption of gas, \(\Delta S\) is negative and therefore \(\Delta H\) should be highly negative.So, the correct answer is (b).
Note:Remember that for all the spontaneous processes, the change is free energy \((\Delta G)\) is negative. For all the non-spontaneous processes, the change in free energy \((\Delta G)\) is positive.
-
Question 8 of 99
8. Question
For the reaction, \(\mathrm{X}_{2} \mathrm{O}_{4(l)} \longrightarrow 2 \mathrm{XO}_{2(\mathrm{~g})}\)
\(\Delta U=2.1 \mathrm{~kcal}, \Delta S=20 \mathrm{~cal} \mathrm{~K}^{-1}\) at \(300 \mathrm{~K}\). Hence, \(\Delta G\) is [NEET 2014]Hint
(b) \(\Delta H=\Delta U+\Delta n_{g} R T\)
Given, \(\Delta U=2.1 \mathrm{~kcal}, \Delta n_{g}=2\),
\(
R=2 \times 10^{-3} \mathrm{~kcal}, T = 300 \mathrm{~K}
\)\(
\therefore \quad \Delta H=2.1+2 \times 2 \times 10^{-3} \times 300=3.3 \mathrm{~kcal}
\)Again, \(\Delta G=\Delta H-T \Delta S\)
Given, \(\Delta S=20 \times 10^{-3} \mathrm{~kcal} \mathrm{~K}^{-1}\)
On putting the values of \(\Delta H\) and \(\Delta S\) in the equation, we get
\(
\begin{aligned}
\Delta G & =3.3-300 \times 20 \times 10^{-3} \\
& =3.3-6 \times 10^3 \times 10^{-3}=-2.7 \mathrm{~kcal}
\end{aligned}
\) -
Question 9 of 99
9. Question
A reaction having equal energies of activation for forward and reverse reactions has [NEET 2013]
Hint
(a) Hint: The change in enthalpy for a reaction is actually the difference in energies in forward and backward reaction. When these energies are equal, the difference is zero.
Complete step by step solution:
The term \(\boldsymbol{\Delta H}\) represents enthalpy of a system, in chemistry. Enthalpy is the additional product of the internal energy of a system, along with the system’s volume and the pressure too. It is a state function and is used in a lot of measurements.
It shows the capacity of a system to do non-mechanical work, or radiate heat energy. In chemistry, change of enthalpy is calculated instead of the absolute enthalpy, because it is not feasible for us to know the initial point, rather the origin.
There is a condition while measuring the difference in enthalpy and that is, pressure should remain constant during the whole process.
It is to be noted that a chemical reaction that has equal energies of activation for both the forward as well as the reverse reaction has no difference of enthalpy. As the enthalpies are the same, there is no difference between them, so we can say that \(\boldsymbol{\Delta H}=0\) for the reaction.
Which gives our answer as option (a)
Note: Enthalpy itself is a very big topic. There are various types of enthalpies like enthalpy of atomisation, enthalpy of fusion, enthalpy of formation, enthalpy of adsorption, enthalpy of hydration, etc. In all these, the differences in enthalpies are taken from final state to the initial state. Enthalpy can never become a path function as it does not depend on the pathway of the reaction. -
Question 10 of 99
10. Question
When 5 litres of a gas mixture of methane and propane is perfectly combusted at \(0^{\circ} \mathrm{C}\) and 1 atmosphere, 16 litres of oxygen at the same temperature and pressure is consumed. The amount of heat released from this combustion in \(\mathrm{kJ}\left(\Delta H_{\text {comb. }}\left(\mathrm{CH}_{4}\right)=890 \mathrm{~kJ} \mathrm{~mol}^{-1}\right.\), \(\left.\Delta H_{\text {comb. }}\left(\mathrm{C}_{3} \mathrm{H}_{8}\right)=2220 \mathrm{~kJ} \mathrm{~mol}^{-1}\right)\) is [Karnataka NEET 2013]
Hint
(b) Step 1: Calculating the volume of methane and propane in the mixture
The balanced equation of combustion can be written as:
\(
\begin{array}{l}
\mathrm{CH}_4(\mathrm{~g})+2 \mathrm{O}_2(\mathrm{~g}) \rightarrow \mathrm{CO}_2(\mathrm{~g})+2 \mathrm{H}_2 \mathrm{O}(\mathrm{l}) \\
\mathrm{C}_3 \mathrm{H}_8(\mathrm{~g})+5 \mathrm{O}_2(\mathrm{~g}) \rightarrow 3 \mathrm{CO}_2(\mathrm{~g})+4 \mathrm{H}_2 \mathrm{O}(\mathrm{l})
\end{array}
\)Let us assume the volume of methane \(\left(\mathrm{CH}_4\right)\) in \(5 \mathrm{~L}\) mixture \(=x \mathrm{L}\)
Now, the volume of propane \(\left(\mathrm{C}_3 \mathrm{H}_8\right)\) in mixture \(=5-x L\)
From stoichiometry, 1 mole of methane consumes 2 moles of oxygen and 1 mole of propane consumes 5 moles of oxygen.
Hence, the total number of moles of oxygen consumed \(=2 x+5(5-x)=16\)
So, \(x=3\)
Hence, the volume of methane \(=3 \mathrm{~L}\)
The volume of propane \(=2 \mathrm{~L}\)
Step 2: Calculating amount of heat evolved by combustion of mixture
\(
\begin{array}{l}
\mathrm{n}_{\mathrm{CH}_4}=\frac{3 \mathrm{~L}}{22.4 \mathrm{~L}} \\
\mathrm{n}_{\mathrm{C}_3 \mathrm{H}_8}=\frac{2 \mathrm{~L}}{22.4 \mathrm{~L}}
\end{array}
\)Heat liberated \(=\mathrm{n}_{\mathrm{CH}_4} \times \Delta \mathrm{H}_{\mathrm{CH}_4}+\mathrm{n}_{\mathrm{C}_3 \mathrm{H}_8} \times \Delta \mathrm{H}_{\mathrm{C}_3 \mathrm{H}_8}\)
Heat liberated \(=\frac{3 \times 890+2 \times 2220}{22.4}=317 \mathrm{~kJ}\)
Hence, the correct option is (b). -
Question 11 of 99
11. Question
Three thermochemical equations are given below
(i) \(\mathrm{C}_{\text {(gaphite) }}+\mathrm{O}_{2(\mathrm{~g})} \rightarrow \mathrm{CO}_{2(\mathrm{~g})} ; \Delta_{r} H^{\circ}=x \mathrm{~kJ} \mathrm{~mol}^{-1}\)
(ii) \(\mathrm{C}_{\text {(gaphite) }}+1 / 2 \mathrm{O}_{2(\mathrm{~g})} \rightarrow \mathrm{CO}_{(\mathrm{g})} ; \Delta_{r} H^{\circ}=y \mathrm{~kJ} \mathrm{~mol}^{-1}\)
(iii) \(\mathrm{CO}_{(\mathrm{g})}+1 / 2 \mathrm{O}_{2(\mathrm{~g})} \rightarrow \mathrm{CO}_{2(g)} ; \Delta_{r} H^{\circ}=z \mathrm{~kJ} \mathrm{~mol}^{-1}\)Based on these equations which of the following options is correct [Karnataka NEET 2013]
Hint
(b) According to Hess’s law, equation (i) is equal to equations (ii) + (iii)
\(
\therefore x=y+z
\) -
Question 12 of 99
12. Question
In which of the following reactions, standard reaction entropy change \(\left(\Delta S^{\circ}\right)\) is positive and standard Gibb’s energy change \(\left(\Delta G^{\circ}\right)\) decreases sharply with increasing temperature? [NEET 2012]
Hint
(a) \(\mathrm{C}_{\text {(graphite) }}+\frac{1}{2} \mathrm{O}_{2(g)} \rightarrow \mathrm{CO}_{(\mathrm{g})}\)
\(
\Delta n_{g}=1-\frac{1}{2}=\frac{1}{2}
\)
As amount of gaseous substance is increasing in product, thus \(\Delta S\) is positive for this reaction.
And we know that \(\Delta G=\Delta H-T \Delta S\)
As \(\Delta S\) is positive, thus increase in temperature will make \(T \Delta S\) more negative and \(\Delta G\) will decrease -
Question 13 of 99
13. Question
The enthalpy of fusion of water is \(1.435 \mathrm{~kcal} / \mathrm{mol}\). The molar entropy change for the melting of ice at \(0^{\circ} \mathrm{C}\) is [NEET 2012]
Hint
(c) \(\Delta H_{f}=1.435 \mathrm{~kcal} / \mathrm{mol}\)
\(
\Delta S=\frac{\Delta H_{f}}{T_{f}}=\frac{1.435 \times 10^{3}}{273}=5.26 \mathrm{~cal} / \mathrm{mol} \mathrm{K}
\) -
Question 14 of 99
14. Question
Standard enthalpy of vaporisation \(\Delta_{\mathrm{vap}} H^{\circ}\) for water at \(100^{\circ} \mathrm{C}\) is \(40.66 \mathrm{~kJ} \mathrm{~mol}^{-1}\). The internal energy of vaporisation of water at \(100^{\circ} \mathrm{C}\) (in \(\mathrm{kJ} \mathrm{~mol}^{-1}\) ) is [NEET 2012] (Assume water vapour to behave like an ideal gas)
Hint
(a) \(
\Delta_{\mathrm{vap}} H^{\circ}=40.66 \mathrm{~kJ} \mathrm{~mol}^{-1}
\)
\(
T=100+273=373 \mathrm{~K}, \Delta E=?
\)
\(
\Delta H=\Delta E+\Delta n_g R T \Rightarrow \Delta E=\Delta H-\Delta n_g R T
\)
\(\Delta n_g=\) number of gaseous moles of products – number of gaseous moles of reactants
\(
\begin{array}{l}
\mathrm{H}_2 \mathrm{O}_{(l)} \rightleftharpoons \mathrm{H}_2 \mathrm{O}_{(g)} \\
\Delta n_g=1-0=1 \\
\begin{aligned}
\Delta E & =\Delta H-R T \\
\Delta E & =\left(40.66 \times 10^3\right)-(8.314 \times 373) \\
& =37559 \mathrm{~J} / \mathrm{mol} \text { or } 37.56 \mathrm{~kJ} / \mathrm{mol}
\end{aligned}
\end{array}
\) -
Question 15 of 99
15. Question
If the enthalpy change for the transition of liquid water to steam is \(30 \mathrm{~kJ} \mathrm{~mol}^{-1}\) at \(27^{\circ} \mathrm{C}\), the entropy change for the process would be [NEET 2011]
Hint
(d) We know that \(\Delta G=\Delta H-T \Delta S\)
\(
\begin{aligned}
&0=\Delta H-T \Delta S \quad[\because \Delta G=0] \\
&\Delta S=\frac{\Delta H}{T}=\frac{30 \times 10^{3}}{300}=100 \mathrm{~J} \mathrm{~mol}^{-1} \mathrm{~K}^{-1}
\end{aligned}
\) -
Question 16 of 99
16. Question
Enthalpy change for the reaction, \(4 \mathrm{H}_{(g)} \rightarrow 2 \mathrm{H}_{2(g)}\) is \(-869.6 \mathrm{~kJ}\)
The dissociation energy of \(\mathrm{H}-\mathrm{H}\) bond is [NEET 2011]Hint
\(
\begin{array}{l}
\text { (c) Given } \\
4 \mathrm{H}(\mathrm{g}) \longrightarrow 2 \mathrm{H}_2(\mathrm{~g}) ; \Delta \mathrm{H}=-869.6 \mathrm{~kJ} \\
\text { or } 2 \mathrm{H}_2(\mathrm{~g}) \longrightarrow 4 \mathrm{H}(\mathrm{g}) ; \Delta \mathrm{H}=869.6 \mathrm{~kJ} \\
\mathrm{H}_2(\mathrm{~g}) \longrightarrow 2 \mathrm{H}(\mathrm{g}) ; \Delta \mathrm{H}=\frac{869.6}{2}=434.8 \mathrm{~kJ}
\end{array}
\) -
Question 17 of 99
17. Question
Which of the following is correct option for free expansion of an ideal gas under adiabatic condition? [NEET 2011]
Hint
(c) For free expansion of an ideal gas under adiabatic condition \(q=0, \Delta T=0, w=0\)
Explanation:
For free expansion of ideal gas
\(
\begin{array}{l}
P_{\text {ext }}=0 \\
W_{p v}=0
\end{array}
\)
\(q=0\) (adiabatic process)
\(
\Delta E=q+w
\)
\(
\Delta E=0
\)
\(
\Delta E=n C_{v m} \Delta T=0
\)
\(
q=0, \Delta T=0, w=0
\) -
Question 18 of 99
18. Question
Consider the following processes:
\(\begin{array}{lc} & \Delta H(\mathbf{k} \mathbf{J} / \mathbf{m o l}) \\ \frac{1}{2} A \rightarrow B & +150 \\ 3 B \rightarrow 2 C+D & -125 \\ E+A \rightarrow 2 D & +350\end{array}\)
For \(B+D \rightarrow E+2 C, \Delta H\) will be [Mains 2011]Hint
\(
\begin{array}{ll}
\text { (b) Given } & \Delta H \\
\frac{1}{2} A \longrightarrow B & +150 \dots(i)\\
3 B \longrightarrow 2 C+D & -125 \dots(ii)\\
E+A \longrightarrow 2 D & +350 \dots(iii)
\end{array}
\)
To calculate \(\Delta H\) operate
\(
\begin{array}{l}
2 \times \text { eq. (i) }+ \text { eq. (ii) }- \text { eq. (iii) } \\
\Delta H=300-125-350=-175
\end{array}
\) -
Question 19 of 99
19. Question
For an endothermic reaction, energy of activation is \(E_{a}\) and enthalpy of reaction is \(\Delta H\) (both of these in \(\mathrm{kJ} / \mathrm{mol}\) ). Minimum value of \(E_{a}\) will be [NEET 2010]
Hint
(c) Activation energy is the minimum quantity of energy which the reacting species must possess in order to undergo a specified reaction.
\(\mathrm{E}_{\mathrm{a}}\)-Activation energy
Here, energy of products is higher than that of reactants.
This graph is for an endothermic reaction.
\(\triangle \mathrm{H}\) for an endothermic reaction is positive
See figure above, when the reaction reaches point \(P\), the reactants possess enough energy for the reaction to take place.
In further reaction, some energy is use up for conversion of reactants to products.
This used up energy must be less than \(\mathrm{E}_{\mathrm{a}}\), for an endothermic reaction Since,
We find that the least \(E_a\) will be more than \(\Delta H\) for an endothermic reaction since \(E_{\text {products }}>E_{\text {reactants }}\)
\(\therefore \mathrm{E}_{\mathrm{a}}> \Delta H\)
Hence, the activation energy must be more than \(\Delta H\)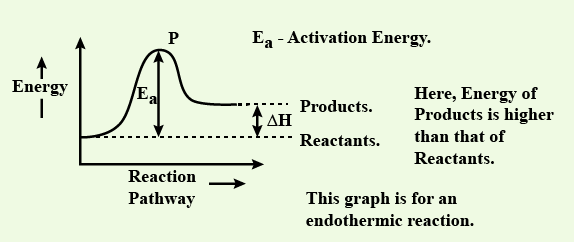
-
Question 20 of 99
20. Question
Standard entropies of \(X_{2}, Y_{2}\) and \(X Y_{3}\) are 60 , 40 and \(50 \mathrm{~J} \mathrm{~K}^{-1} \mathrm{~mol}^{-1}\) respectively. For the reaction \(1 / 2 \mathrm{X}_{2}+3 / 2 \mathrm{Y}_{2} \rightleftharpoons X Y_{3}, \Delta H=-30 \mathrm{~kJ}\), to be at equilibrium, the temperature should be [NEET 2011]
Hint
(a) Given reaction is:
\(
\frac{1}{2} X_{2}+\frac{3}{2} Y_{2} \rightleftharpoons X Y_{3}
\)
We know, \(\Delta S^{\circ}=\Sigma S_{\text {products }}^{\circ}-\Sigma S_{\text {reactants }}^{\circ}\) \(=50-(30+60)=-40 \mathrm{~JK}^{-1} \mathrm{~mol}^{-1}\)
At equilibrium \(\Delta G^{\circ}=0\)
\(
\begin{aligned}
& \Delta H^{\circ}=T \Delta S^{\circ} \\
\therefore & T=\frac{\Delta H^{\circ}}{\Delta S^{\circ}}=\frac{-30 \times 10^{3} \mathrm{~J} \mathrm{~mol}^{-1}}{-40 \mathrm{~J} \mathrm{~K}^{-1} \mathrm{~mol}^{-1}}=750 \mathrm{~K}
\end{aligned}
\) -
Question 21 of 99
21. Question
Match List I (Equations) with List II (Type of processes) and select the correct option. [NEET 2010]
\(
\begin{array}{|l|l|}
\hline \text { List I } & \text { List II } \\
\hline \text { Equations } & \text { Type of processes } \\
\hline \text { A. } K_P>Q & \text { (i) Non- Spontaneous } \\
\hline \text { B. } \Delta C^{\circ}<\mathrm{RT} \text { In } Q & \text { (ii) Equilibrium } \\
\hline \text { C. } K_P=Q & \text { (iii) Spontaneous and endothermic } \\
\hline \text { D. } T>\Delta H / \Delta S & \text { (iv) Spontaneous } \\
\hline
\end{array}
\)Hint
(c) When \(K_{p}>Q\), rate of forward reaction \(>\) rate of backward reaction.
\(\therefore\) Reaction is spontaneous.
When \(\Delta G^{\circ}<R T \ln Q, \Delta G^{\circ}\) is positive, reverse reaction is feasible, thus reaction is non spontaneous.When \(K_{p}=Q\), rate of forward reaction \(=\) rate of backward reaction.
\(\therefore\) Reaction is in equilibrium.
When \(T \Delta S>\Delta H, \Delta G\) will be negative only when \(\Delta H=+\) ve.
\(\therefore\) Reaction is spontaneous and endothermic. -
Question 22 of 99
22. Question
Three moles of an ideal gas expanded spontaneously into vacuum. The work done will be [Mains 2010]
Hint
(d) Hint: The work done is the product of pressure and the change in volume. The ideal gases expand spontaneously into vacuum if the external pressure will be zero.
Complete step by step answer:
The work done during the free expansion of an ideal gas is always zero when the process is irreversible. Given in the question that an ideal gas expands spontaneously into vacuum.
Work done is the product of pressure and the change in volume, the expression for work done will be:
Work done \(=-P \Delta V\)
Where, \(\mathrm{P}=\) external pressure
And \(\Delta V=\) the change in volume
Now, the ideal gas expands spontaneously in vacuum hence the external pressure will be zero
Work done \(=-(0) \times \Delta V=0\)
Hence the work done \(=0\) -
Question 23 of 99
23. Question
For vaporization of water at 1 atmospheric pressure, the values of \(\Delta H\) and \(\Delta S\) are \(40.63 \mathrm{~kJ} \mathrm{~mol}^{-1}\) and \(108.8 \mathrm{~J} \mathrm{~K}^{-1} \mathrm{~mol}^{-1}\), respectively. The temperature when Gibb’s energy change \((\Delta G)\) for this transformation will be zero, is [NEET 2009]
Hint
(c)According to Gibb’s equation,
\(
\Delta G=\Delta H-T \Delta S
\)
when \(\Delta G=0, \Delta H=T \Delta S\)
Given, \(\Delta H=40.63 \mathrm{~kJ} \mathrm{~mol}^{-1}=40.63 \times 10^{3} \mathrm{~J} \mathrm{~mol}^{-1}\)\(
\begin{aligned}
& \Delta S=108.8 \mathrm{~J} \mathrm{~K}^{-1} \mathrm{~mol}^{-1} \\
\therefore & T=\frac{\Delta H}{\Delta S}=\frac{40.63 \times 10^3}{108.8}=373.43 \mathrm{~K}
\end{aligned}
\) -
Question 24 of 99
24. Question
The following two reactions are known
\(
\begin{array}{l}
\mathrm{Fe}_2 \mathrm{O}_{3(s)}+3 \mathrm{CO}_{(g)} \rightarrow 2 \mathrm{Fe}_{(s)}+3 \mathrm{CO}_{2(g)} ; \Delta H=-26.8 \mathrm{~kJ}\\
\end{array}
\)
\(
\mathrm{FeO}_{(s)}+\mathrm{CO}_{(g)} \rightarrow \mathrm{Fe}_{(s)}+\mathrm{CO}_{2(g)} ; \Delta H=-16.5 \mathrm{~kJ}
\)
The value of \(\Delta H\) for the following reaction
\(\mathrm{Fe}_2 \mathrm{O}_{3(s)}+\mathrm{CO}_{(g)} \rightarrow 2 \mathrm{FeO}_{(s)}+\mathrm{CO}_{2(g)}\) is [Mains 2010]Hint
(d)
\(
\mathrm{Fe}_2 \mathrm{O}_{3(s)}+3 \mathrm{CO}_{(\mathrm{g})} \rightarrow 2 \mathrm{Fe}_{(s)}+3 \mathrm{CO}_{2(\mathrm{~g})}; \Delta H=-26.8 \mathrm{~kJ} \dots(i)
\)
\(
\mathrm{FeO}_{(s)}+\mathrm{CO}_{(g)} \rightarrow \mathrm{Fe}_{(s)}+\mathrm{CO}_{2(\mathrm{~g})}; \Delta H=-16.5 \mathrm{~kJ}; \ldots \text { (ii) }
\)
\(
\mathrm{Fe}_2 \mathrm{O}_{3(s)}+\mathrm{CO}_{(g)} \rightarrow 2 \mathrm{FeO}_{(s)}+\mathrm{CO}_{2(g)}; \Delta H=? \dots(iii)
\)
Eq. (iii) can be obtained as : \((i) – 2 \times (ii)\)
\(
=-26.8-2(-16.5)=-26.8+33.0=+6.2 \mathrm{~kJ}
\) -
Question 25 of 99
25. Question
The values of \(\Delta H\) and \(\Delta S\) for the reaction,
\(
\mathrm{C}_{\text {(graphite) }}+\mathrm{CO}_{2}(\mathrm{~g}) \rightarrow 2 \mathrm{CO}_{(g)}
\)
are \(170 \mathrm{~kJ}\) and \(170 \mathrm{~J} \mathrm{~K}^{-1}\), respectively. This reaction will be spontaneous at [NEET 2009]Hint
(b) For the reaction to be spontaneous, \(\Delta G=-\mathrm{ve}\).
Given, \(\Delta H=170 \mathrm{~kJ}=170 \times 10^{3} \mathrm{~J}\)
\(
\Delta S=170 \mathrm{~J} \mathrm{~K}^{-1} \mathrm{~mol}^{-1}
\)
Applying, \(\Delta G=\Delta H-T \Delta S\), the value of \(\Delta G=-\mathrm{ve}\)
only when \(T \Delta S>\Delta H\), which is possible only when \(T=1110 \mathrm{~K}\)
\(\therefore \Delta G=170 \times 10^{3}-(1110 \times 170)=-18700 \mathrm{~J}\)
Thus, reaction is spontaneous at \(T=1110 \mathrm{~K}\) -
Question 26 of 99
26. Question
From the following bond energies:
\(\begin{array}{ll}\mathrm{H}-\mathrm{H} \text { bond energy } & : 431.37 \mathrm{~kJ} \mathrm{~mol}^{-1} \\ \mathrm{C}=\mathrm{C} \text { bond energy } & : 606.10 \mathrm{~kJ} \mathrm{~mol}^{-1} \\ \mathrm{C} \text { – } \mathrm{C} \text { bond energy } & : 336.49 \mathrm{~kJ} \mathrm{~mol}^{-1} \\ \mathrm{C}-\mathrm{H} \text { bond energy } & : 410.50 \mathrm{~kJ} \mathrm{~mol}^{-1}\end{array}\)
Enthalpy for the reaction,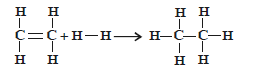
will be [NEET 2009]Hint
(b) For the given reaction, enthalpy of reaction can be calculated as
\(=\) B.E. \((\) reactant \()-B.E.(\) product \()\)
\(
=\left[\mathrm{B.} \mathrm{E.}_{(\mathrm{C}-\mathrm{C})}+\mathrm{B.} \mathrm{E.}_{(\mathrm{H}-\mathrm{H})}+4 \times \mathrm{B.} \mathrm{E.}_{(\mathrm{C}-\mathrm{H})}\right]-\left[\mathrm{B.} \mathrm{E.}_{(\mathrm{C}-\mathrm{C})}+6 \times \mathrm{B.} \mathrm{E.}_{(\mathrm{C}-\mathrm{H})}\right]
\)
\(
=[606.10+431.37+4 \times 410.50]-[336.49+6 \times 410.50]
\)
\(
=2679.47-2799.49=-120.02 \mathrm{~kJ} \mathrm{~mol}^{-1}
\) -
Question 27 of 99
27. Question
Bond dissociation enthalpy of \(\mathrm{H}_{2}, \mathrm{Cl}_{2}\) and \(\mathrm{HCl}\) are 434, 242 and \(431 \mathrm{~kJ} \mathrm{~mol}^{-1}\) respectively. Enthalpy of formation of \(\mathrm{HCl}\) is [NEET 2008]
Hint
(a)\(\mathrm{H}_{2}+\mathrm{Cl}_{2} \rightarrow 2 \mathrm{HCl}\)
\(\Delta H_{\text {reaction }}=\Sigma(\mathrm{B} . \mathrm{E})_{\text {reactant }}-\Sigma(\mathrm{B} . \mathrm{E})_{\text {product }}\)
\(=\left[(\mathrm{B} . \mathrm{E})_{\mathrm{H}-\mathrm{H}}+(\mathrm{B} \cdot \mathrm{E})_{\mathrm{Cl}-\mathrm{Cl}}\right]-\left[2 \mathrm{~B} \cdot \mathrm{E}_{(\mathrm{H}-\mathrm{Cl})}\right]\)
\(=434+242-(431) \times 2\)
\(\Delta H_{\text {reaction }}=-186 \mathrm{~kJ}\)
Heat of formation is the amount of heat absorbed or evolved when one mole of substance is directly obtained from its constituent element.
Hence, enthalpy of formation of \(\mathrm{HCl}=\frac{-186}{2} \mathrm{~kJ}\) \(=-93 \mathrm{~kJ} \mathrm{~mol}^{-1}\) -
Question 28 of 99
28. Question
For the gas phase reaction,
\(\mathrm{PCl}_{5(\mathrm{~g})} \rightleftharpoons \mathrm{PCl}_{3(\mathrm{~g})}+\mathrm{Cl}_{2(\mathrm{~g})}\)
which of the following conditions are correct? [NEET 2008]Hint
(d) Gas phase reaction
\(
\begin{aligned}
&\mathrm{PCl}_{5(g)} \rightleftharpoons \mathrm{PCl}_{3(g)}+\mathrm{Cl}_{2(g)} \\
&\Delta H=\Delta E+\Delta n_{g} R T
\end{aligned}
\)
\(\Delta n_{g}=\) Change in number of moles of product and reactant species.\(
\text { Here, } \Delta \mathrm{ng}=1+1-1=1>0
\)
Since \(\Delta n_{g}=+\mathrm{ve}\), hence \(\Delta H=+\mathrm{ve}\)
also one mole of \(\mathrm{PCl}_{5}\) is dissociated into two moles
of \(\mathrm{PCl}_{3}\) and \(\mathrm{Cl}_{2}\) in the same phase.
Therefore, \(\Delta S=S_{\text {product }}-S_{\text {reactant }}\)
\(\Delta S=+\) ve -
Question 29 of 99
29. Question
Which of the following are not state functions?
(I) \(q+w\)
(II) \(q\)
(III) \(w\)
(IV) \(H-T S\) [NEET 2008]Hint
(b) State functions or state variables are those which depend only on the state of the system and not on how the state was reached.
\(
\left.\begin{array}{l}
q+w=\Delta E \text { (internal energy) } \\
H-T S=G \text { (free energy) }
\end{array}\right\} \text { State functions }
\)
Path function depends on the path followed during a process as well as the end states. Work and heat are the path functions. -
Question 30 of 99
30. Question
Consider the following reactions:
(i) \(\mathrm{H}_{(a q)}^{+}+\mathrm{OH}_{(a q)}^{-}=\mathrm{H}_{2} \mathrm{O}_{(l)}; \Delta H=-X_1 \mathrm{~kJ} \mathrm{~mol}^{-1}\),
(ii) \(\mathrm{H}_{2(\mathrm{~g})}+1 / 2 \mathrm{O}_{2(\mathrm{~g})}=\mathrm{H}_{2} \mathrm{O}_{(l)}); \Delta H=-X_2 \mathrm{~kJ} \mathrm{~mol}^{-1}\)
(iii) \(\mathrm{CO}_{2(\mathrm{~g})}+\mathrm{H}_{2(\mathrm{~g})}=\mathrm{CO}_{(g)}+\mathrm{H}_{2} \mathrm{O}_{(l)}; \Delta H=-X_3 \mathrm{~kJ} \mathrm{~mol}^{-1}\),
(iv) \(\mathrm{C}_{2} \mathrm{H}_{2(\mathrm{~g})}+5 / 2 \mathrm{O}_{2(\mathrm{~g})}=2 \mathrm{CO}_{2(\mathrm{~g})}+\mathrm{H}_{2} \mathrm{O}_{(l)}; \Delta H=+X_{4} \mathrm{~kJ} \mathrm{~mol}^{-1}\),
Enthalpy of formation of \(\mathrm{H}_{2} \mathrm{O}_{(l)}\) is [NEET 2007]Hint
(d) The amount of heat absorbed or released when 1 mole of a substance is directly obtained from its constituent elements is called the heat of formation or enthalpy of formation.
Equation (i) represents neutralisation reaction, (iii) represents hydrogenation reaction and (iv) represents combustion reaction.
Note: This reaction shows the formation of \(\mathrm{H}_2 \mathrm{O}\), and the \(\mathrm{X}_2\) represents the enthalpy of formation of \(\mathrm{H}_2 \mathrm{O}\).
The enthalpy of formation is the heat evolved or absorbed when one mole of substance is formed from its constituent atoms. -
Question 31 of 99
31. Question
Given that bond energies of \(\mathrm{H}-\mathrm{H}\) and \(\mathrm{Cl}-\mathrm{Cl}\) are \(430 \mathrm{~kJ} \mathrm{~mol}^{-1}\) and \(240 \mathrm{~kJ} \mathrm{~mol}^{-1}\) respectively and \(\Delta H_{f}\) for \(\mathrm{HCl}\) is \(-90 \mathrm{~kJ} \mathrm{~mol}^{-1}\), bond enthalpy of \(\mathrm{HCl}\) is [NEET 2007]
Hint
(b) \(
\mathrm{HCl} \rightarrow \frac{1}{2} \mathrm{H}_2+\frac{1}{2} \mathrm{Cl}_2
\)
\(
\begin{aligned}
\Delta H & =\Sigma \text { B.E. }_{(\text {products) }}-\Sigma \text { B.E. }_{(\text {reactants })} \\
& =\frac{1}{2}\left[\text { B.E. }_{\left(\mathrm{H}_2\right)}+\text { B.E. }_{\left(\mathrm{Cl}_2\right)}\right]-\text { B.E. }_{(\mathrm{HCl})} \\
& =\frac{1}{2}(430+240)-(-90)=\frac{1}{2} \times 670+90 \\
& =335+90=425 \mathrm{~kJ} \mathrm{~mol}^{-1}
\end{aligned}
\) -
Question 32 of 99
32. Question
Identify the correct statement for change of Gibb’s energy for a system \(\left(\Delta G_{\text {system }}\right)\) at constant temperature and pressure.[NEET 2006]
Hint
(c) The criteria for spontaneity of a process in terms of \(\Delta G\) is as follows:
- If \(\Delta G\) is negative, the process is spontaneous.
- If \(\Delta G\) is positive, the process does not occur in the forward direction. It may occur in the backward direction.
- If \(\Delta G\) is zero, the system is in equilibrium
-
Question 33 of 99
33. Question
Assume each reaction is carried out in an open container. For which reaction will \(\Delta H=\Delta E\) ? [NEET 2006]
Hint
(b) \(\Delta H=\Delta E+\Delta n_{g} R T\)
For \(\mathrm{H}_{2(g)}+\mathrm{Br}_{2(g)} \rightarrow 2 \mathrm{HBr}_{(g)}\)
\(
\Delta n_{g}=2-(1+1)=0 \text {. i.e. } \Delta H=\Delta E
\) -
Question 34 of 99
34. Question
The enthalpy and entropy change for the reaction:
\(
\mathrm{Br}_{2(l)}+\mathrm{Cl}_{2(g)} \rightarrow 2 \mathrm{BrCl}_{(g)}
\)
are \(30 \mathrm{~kJ} \mathrm{~mol}^{-1}\) and \(105 \mathrm{~J} \mathrm{~K}^{-1} \mathrm{~mol}^{-1}\) respectively. The temperature at which the reaction will be in equilibrium is [NEET 2006]Hint
(b) \(\mathrm{Br}_{2(l)}+\mathrm{Cl}_{2(g)} \rightarrow 2 \mathrm{BrCl}_{(g)}\)
\(
\begin{aligned}
& \Delta H=30 \mathrm{~kJ} \mathrm{~mol}^{-1}, \Delta S=105 \mathrm{~J} \mathrm{~K}^{-1} \mathrm{~mol}^{-1} \\
& \Delta S=\frac{\Delta H}{T} \text { i.e. } 105=\frac{30}{T} \times 1000 \\
\therefore \quad T &=\frac{30 \times 1000}{105}=285.7 \mathrm{~K}
\end{aligned}
\) -
Question 35 of 99
35. Question
The enthalpy of hydrogenation of cyclohexene is \(-119.5 \mathrm{~kJ} \mathrm{~mol}^{-1}\). If resonance energy of benzene is \(-150.4 \mathrm{~kJ} \mathrm{~mol}^{-1}\), its enthalpy of hydrogenation would be [NEET 2006]
Hint
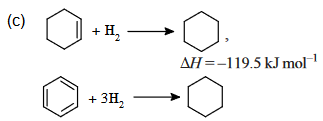
Enthalpy of hydrogenation of benzene
\(
\begin{aligned}
&=3 \times \Delta H-\text { resonance energy } \\
&=3 \times(-119.5)-(-150.4)=-358.5+150.4 \\
&=-208.1 \mathrm{~kJ} \mathrm{~mol}^{-1}
\end{aligned}
\) -
Question 36 of 99
36. Question
Which of the following pairs of a chemical reaction is certain to result in a spontaneous reaction? [NEET 2005]
Hint
(a) For spontaneous reaction \(\Delta H=-\mathrm{ve}, \Delta S\) \(=\) +ve
Spontaneity depends upon both critical minimum energy and maximum randomness / disorder
Note: If reaction is exothermic, therefore, \(\Delta H\) is – ve and on increasing disorder, \(\Delta S\) is \(+v e\) thus, at these condition, \(\Delta G\) is negative according to following equation.
\(
\begin{array}{l}
\Delta G=\Delta H-T \Delta S \\
\Delta G=-v e .
\end{array}
\)
Hence, for spontaneous reaction \(\Delta G\) must be \(-v e\). -
Question 37 of 99
37. Question
A reaction occurs spontaneously if [NEET 2005]
Hint
(c) \(\Delta G=\Delta H-T \Delta S\)
\(\Delta G=-\) ve for spontaneous reaction
When \(\Delta S=+\mathrm{ve}, \Delta H=+\mathrm{ve}\)
and \(T \Delta S>\Delta H \Rightarrow \Delta G=-\mathrm{ve}\) -
Question 38 of 99
38. Question
The absolute enthalpy of neutralisation of the reaction:
\(\mathrm{MgO}_{(s)}+2 \mathrm{HCl}_{(a q)} \rightarrow \mathrm{MgCl}_{2(a q)}+\mathrm{H}_{2} \mathrm{O}_{(l)}\) will be [NEET 2005]Hint
(c) MgO is the oxide of weak base and we know that heat of neutralisation of 1 eq. of strong acid with strong base is \(-57.33 \mathrm{~kJ} / \mathrm{mol}\).
\(\Rightarrow\) with weak base some heat is absorbed in dissociation of weak base.
\(\Rightarrow\) Heat of neutralisation of weak base with strong acid will be less than \(-57.33 \mathrm{~kJ} / \mathrm{mol}\)Note: The absolute value of enthalpy of neutralization of this reaction is greater than \(-57.33 \mathrm{~kJ} \mathrm{~mol}^{-1}\).
This is due to the very high enthalpy of hydration of \(\mathrm{Mg}^{2+}\) ion. The change in enthalpy of this reaction is nearly \(-146 k J\).
Thus enthalpy of neutralization per mole of \(H^{+}\)ion is nearly \(-73 k J\), which is greater than the enthalpy of neutralization strong acid against strong base i.e. \(-57.33 k J\). -
Question 39 of 99
39. Question
If the bond energies of \(\mathrm{H}-\mathrm{H}, \mathrm{Br}-\mathrm{Br}\), and \(\mathrm{H}-\mathrm{Br}\) are 433, 192 and \(364 \mathrm{~kJ} \mathrm{~mol}^{-1}\) respectively, the \(\Delta H^{\circ}\) for the reaction \(\mathrm{H}_{2(g)}+\mathrm{Br}_{2(g)} \rightarrow 2 \mathrm{HBr}_{(g)}\) is [NEET 2004]
Hint
(d)
\(
\begin{array}{rrr}
\mathrm{H}-\mathrm{H}+\mathrm{Br}-\mathrm{Br} & \rightarrow 2 \mathrm{H}-\mathrm{Br} \\
433+192 & 2 \times 364 \\
=625 & =728
\end{array}
\)
Energy absorbed Energy released
\(
\begin{array}{l}
\Delta \mathrm{H}^{\circ}=\sum \text { bond energy (reactants) }-\sum \text { bond energy (products) } \\
=433+192-2 \times 364=-103 \mathrm{~KJ}
\end{array}
\) -
Question 40 of 99
40. Question
Standard enthalpy and standard entropy changes for the oxidation of ammonia at \(298 \mathrm{~K}\) are \(-382.64 \mathrm{~kJ} \mathrm{~mol}^{-1}\) and \(-145.6 \mathrm{~kJ} \mathrm{~mol}^{-1}\), respectively. Standard Gibb’s energy change for the same reaction at \(298 \mathrm{~K}\) is [NEET 2004]
Hint
(b) \(\Delta G=\Delta H-T \Delta S=-382.64-298\left(\frac{-145.6}{1000}\right)\)
\(
=-382.64+43.38=-339.3 \mathrm{~kJ} \mathrm{~mol}^{-1}
\) -
Question 41 of 99
41. Question
Considering entropy \((S)\) as a thermodynamic parameter, the criterion for the spontaneity of any process is [NEET 2004]
Hint
(a) \(\Delta S=R \ln \frac{V_{2}}{V_{1}}\)
Here the volume of gas increases from \(V_{1}\) to \(V_{2}\) at constant temperature \(T\).Since \(V_{2}>V_{1}\), it is obvious that the spontaneous (irreversible) isothermal expansion of a gas is accompanied by an increase in the entropy of the system and its surroundings considered together.
\(
\Delta S_{\text {sys }}+\Delta S_{\text {surr }}>0
\) -
Question 42 of 99
42. Question
The work done during the expansion of a gas from a volume of \(4 \mathrm{dm}^3\) to \(6 \mathrm{dm}^3\) against a constant external pressure of \(3 \mathrm{~atm}\) is \((1 \mathrm{~L} \mathrm{~atm}=101.32 \mathrm{~J})\) [NEET 2004]
Hint
(b) The work done during the expansion of a gas can be calculated using the formula \(\mathrm{W}=-\mathrm{P} \Delta \mathrm{V}\), where \(W\) is the work done by the system (gas), \(P\) is the external pressure, and \(\Delta V\) is the change in volume. Since the external pressure is constant, we can use this formula directly to find the answer.
In this scenario, the gas expands from \(4 \mathrm{dm}^3\) to \(6 \mathrm{dm}^3\) against a constant external pressure of \(3 \mathrm{~atm}\). The change in volume is \(6 \mathrm{dm}^3-4 \mathrm{dm}^3=2 \mathrm{dm}^3\), which is equivalent to \(2 \mathrm{~L}\) (since \(1 \mathrm{dm}^3=1 \mathrm{~L}\) ). Using the conversion factor \(1 \mathrm{~L}\) \(\mathrm{atm}=101.32 \mathrm{~J}\), we can calculate the work done as:
\(
\begin{array}{l}
W=-(3 \mathrm{~atm})(2 \mathrm{~L})=-6 \mathrm{~L} \mathrm{~atm} \\
W=-6 \mathrm{~L} \mathrm{~atm} \times 101.32 \mathrm{~J} / \mathrm{L} \mathrm{~atm}=-608 \mathrm{~J}
\end{array}
\) -
Question 43 of 99
43. Question
For the reaction,
\(\mathrm{C}_{3} \mathrm{H}_{8(g)}+5 \mathrm{O}_{2(g)} \rightarrow 3 \mathrm{CO}_{2(g)}+4 \mathrm{H}_{2} \mathrm{O}_{(l)}\)
at constant temperature, \(\Delta H-\Delta E\) is [NEET 2003]Hint
\(
\begin{aligned}
&\text { (b) } \mathrm{C}_{3} \mathrm{H}_{8(g)}+5 \mathrm{O}_{2(g)} \rightarrow 3 \mathrm{CO}_{2(g)}+4 \mathrm{H}_{2} \mathrm{O}_{(l)} \\
&\Delta n_{g}=3-6=-3 \\
&\Delta H=\Delta E+P \Delta V \text { or } \Delta H-\Delta E=P \Delta V \\
&\Delta H-\Delta E=\Delta n_{g} R T=-3 R T
\end{aligned}
\) -
Question 44 of 99
44. Question
The densities of graphite and diamond at \(298 \mathrm{~K}\) are \(2.25\) and \(3.31 \mathrm{~g} \mathrm{~cm}^{-3}\), respectively. If the standard free energy difference \(\left(\Delta G^{\circ}\right)\) is equal to \(1895 \mathrm{~J} \mathrm{~mol}^{-1}\), the pressure at which graphite will be transformed into diamond at \(298 \mathrm{~K}\) is [NEET 2003]
Hint
(e) (None) \(\Delta G=-P \Delta V=\) Work done
\(
\begin{aligned}
&\Delta V=\left(\frac{12}{3.31}-\frac{12}{2.25}\right) \times 10^{-3} \mathrm{~L}=-1.71 \times 10^{-3} \mathrm{~L} \\
&\Delta G=\text { Work done }=-\left(-1.71 \times 10^{-3}\right) \times P \times 101.3 \mathrm{~J} \\
&\begin{aligned}
P &=\frac{1895}{1.71 \times 10^{-3} \times 101.3}=10.93 \times 10^{3} \mathrm{~atm} \\
&=11.07 \times 10^{8} \mathrm{~Pa}
\end{aligned}
\end{aligned}
\) -
Question 45 of 99
45. Question
What is the entropy change (in \(\mathrm{J} \mathrm{K}^{-1} \mathrm{~mol}^{-1}\) ) when one mole of ice is converted into water at \(0^{\circ} \mathrm{C}\) ? (The enthalpy change for the conversion of ice to liquid water is \(6.0 \mathrm{~kJ} \mathrm{~mol}^{-1}\) at \(0^{\circ} \mathrm{C}\).) [NEET 2003]
Hint
\(
\text { (d) } S=\frac{q_{r e v}}{T}=\frac{6000}{273}=21.978 \mathrm{~JK}^{-1} \mathrm{~mol}^{-1}
\) -
Question 46 of 99
46. Question
Formation of a solution from two components can be considered as
(i) Pure solvent \(\rightarrow\) separated solvent molecules, \(\Delta H_{1}\)
(ii) Pure solute \(\rightarrow\) separated solute molecules, \(\Delta \mathrm{H}_{2}\)
(iii) Separated solvent and solute molecules
Solution so formed will be ideal if [NEET 2003]Hint
(a) Heat of solution is defined as the amount of heat evolved or absorbed when one mole of the substance is dissolved in excess of the solvent. For hydrated salt and for salts which do not form hydrates, \(\Delta H\) is positive and for anhydrous salts, it is negative.
Note: Solution so formed will be ideal if \(\Delta \mathrm{H}_{\text {soln. }}=\Delta \mathrm{H}_1+\Delta \mathrm{H}_2+\Delta \mathrm{H}_3\)
Adding (i) to (iii)
pure solute + pure solvent \(\rightarrow\) solution;
\(
\Delta \mathrm{H}=\Delta \mathrm{H}_1+\Delta \mathrm{H}_2+\Delta \mathrm{H}_3
\) -
Question 47 of 99
47. Question
For which one of the following equations is \(\Delta H_{\text {react }}^{\circ}\) equal to \(\Delta H_{f}^{\circ}\) for the product? [NEET 2003]
Hint
For (c) \(\Delta H_{\text {reaction }}^{\circ}=\Delta H_f^{\circ}\left(\mathrm{XeF}_4\right)-\left[\Delta H_f^{\circ}(\mathrm{Xe})+2 \Delta H_f^{\circ}\left(\mathrm{F}_2\right)\right]\)
Enthalpies of formation of elementary substances \(\mathrm{Xe}\) and \(\mathrm{F}_2\) are taken as zero.
\(
\text { Thus, } \Delta H_{\text {reaction }}^{\circ}=\Delta H_f^{\circ}\left(\mathrm{XeF}_4\right)
\) -
Question 48 of 99
48. Question
The molar heat capacity of water at constant pressure, \(C\), is \(75 \mathrm{~J} \mathrm{~K}^{-1} \mathrm{~mol}^{-1}\). When \(1.0 \mathrm{~kJ}\) of heat is supplied to \(100 \mathrm{~g}\) of water which is free to expand, the increase in temperature of water is [NEET 2003]
Hint
(b) Molar heat capacity \(=75 \mathrm{~J} \mathrm{~K}^{-1} \mathrm{~mol}^{-1}\)
\(
\begin{aligned}
& 18 \mathrm{~g} \text { of water }=1 \text { mole }=75 \mathrm{~J} \mathrm{~K}^{-1} \mathrm{~mol}^{-1} \\
& 1 \mathrm{~g} \text { of water }=\frac{75}{18} \mathrm{~J} \mathrm{~K}^{-1} \\
& Q=m \times C \times \Delta t \text { or } 1000=100 \times \frac{75}{18} \times \Delta t \\
\Rightarrow & \Delta t=\frac{10 \times 18}{75}=2.4 \mathrm{~K}
\end{aligned}
\) -
Question 49 of 99
49. Question
Unit of entropy is [NEET 2002]
Hint
(a) Entropy change \((d S)\) is given by \(d S=\frac{d q}{T}\)
\(\therefore \quad\) Unit of entropy \(=\mathrm{J} / \mathrm{K} \mathrm{mol}\) (entropy per unit mole) \(=\mathrm{J} \mathrm{K}^{-1} \mathrm{~mol}^{-1}\)
-
Question 50 of 99
50. Question
In a closed insulated container a liquid is stirred with a paddle to increase the temperature which of the following is true? [NEET 2002]
Hint
(a) The mathematical form of first law of thermodynamics: \(q=\Delta E+W\)
Since the system is closed and insulated, \(q=0\)
Paddle work is done on system. \(\therefore W \neq 0\).
Temperature and hence internal energy of the system increases. \(\therefore \Delta E \neq 0\) -
Question 51 of 99
51. Question
Heat of combustion \(\Delta H\) for \(\mathrm{C}_{(s)}, \mathrm{H}_{2(\mathrm{~g})}\) and \(\mathrm{CH}_{4(\mathrm{~g})}\) are \(-94, -68\) and \(-213 \mathrm{~kcal} / \mathrm{mol}\), then \(\Delta H\) for \(\mathrm{C}_{(s)}+2 \mathrm{H}_{2(g)} \rightarrow \mathrm{CH}_{4(g)}\) is [NEET 2002]
Hint
(a) (i) \(\mathrm{C}_{(s)}+\mathrm{O}_{2(g)} \rightarrow \mathrm{CO}_{2(g)}; \Delta H_{\mathrm{i}}=-94 \mathrm{kcal} / \mathrm{mole}\)
(ii) \(2 \mathrm{H}_{2(\mathrm{~g})}+\mathrm{O}_{2(\mathrm{~g})} \rightarrow 2 \mathrm{H}_{2} \mathrm{O}_{(l)}; \Delta H_{\mathrm{ii}}=-68 \times 2 \mathrm{kcal} / \mathrm{mole}\)
(iii) \(\mathrm{CH}_{4(\mathrm{~g})}+2 \mathrm{O}_{2(\mathrm{~g})} \rightarrow \mathrm{CO}_{2(\mathrm{~g})}+2 \mathrm{H}_{2} \mathrm{O}_{(l)}; \Delta H_{\mathrm{iii}}=-213 \mathrm{kcal} / \mathrm{mole}\)
(iv) \(\mathrm{C}_{(s)}+2 \mathrm{H}_{2(\mathrm{~g})} \rightarrow \mathrm{CH}_{4(\mathrm{~g})}; \Delta H_{\mathrm{iv}}=\) ?
By applying Hess’s law we can compute \(\Delta H_{\mathrm{iv}}\).
\(
\begin{aligned}
\therefore \Delta H_{\mathrm{iv}}=\Delta H_{\mathrm{i}}+\Delta H_{\mathrm{ii}}-\Delta H_{\mathrm{iii}} \\
\quad=(-94-68 \times 2+213) \mathrm{kcal}=-17 \mathrm{kcal}
\end{aligned}
\) -
Question 52 of 99
52. Question
Which reaction is not feasible? [NEET 2002]
Hint
(b) The halogen are highly electronegative elements – their non-metallic character gradually decreases from fluorine to iodine. \(\mathrm{F}>\mathrm{Cl}>\mathrm{Br}>\mathrm{I}\). Fluorine can displace chlorine, bromine and iodine. Chlorine can displace bromine and iodine and bromine can displace iodine from their salts. Iodine cannot displace flourine, chlorine and bromine. Hence reaction (b) is not feasible.
-
Question 53 of 99
53. Question
2 mole of ideal gas at \(27^{\circ} \mathrm{C}\) temperature is expanded reversibly from 2 lit. to 20 lit. Find entropy change. \((R=2 \mathrm{cal} / \mathrm{mol} \mathrm{K})\)
Hint
(d) The change of entropy \(d S=\frac{d q}{T}\)
From the first law of thermodynamics,
\(
\begin{aligned}
d Q=d U+P d V=C_{V} d T+P d V \\
\Rightarrow \quad \frac{d Q}{T}=C_{V} \frac{d T}{T}+\frac{P}{T} d V \\
\Rightarrow \quad \frac{d Q}{T}=C_{V} \frac{d T}{T}+\frac{R d V}{V} \quad\left[\frac{P}{T}=\frac{R}{V}\right] \\
\therefore \quad d S=C_{V} \frac{d T}{T}+R \frac{d V}{V} \\
\Rightarrow \quad \Delta S=C_{V} \ln \frac{T_{2}}{T_{1}}+R \ln \frac{V_{2}}{V_{1}} \\
& \quad[\text { for one mole of ideal gas }] \\
\text { Here } T_{2}=T_{1}=27^{\circ} \mathrm{C}=300 \mathrm{~K} . \quad \therefore \ln \frac{T_{2}}{T_{1}}=0 \\
\therefore \quad \Delta S=R \ln \frac{V_{2}}{V_{1}}=2 \ln \frac{20}{2}=2 \ln 10=4.605 \\
\therefore \quad \Delta S=4.605 \mathrm{cal} / \mathrm{mol}-\mathrm{K} \\
\text { Entropy change for } 2 \mathrm{moles} \text { of gas } \\
\quad=2 \times 4.605 \mathrm{cal} / \mathrm{K}=9.2 \mathrm{cal} / \mathrm{K}
\end{aligned}
\) -
Question 54 of 99
54. Question
Change in enthalpy for reaction, \(2 \mathrm{H}_{2} \mathrm{O}_{2(l)} \rightarrow 2 \mathrm{H}_{2} \mathrm{O}_{(l)}+\mathrm{O}_{2(g)}\)
if heat of formation of \(\mathrm{H}_{2} \mathrm{O}_{2(l)}\) and \(\mathrm{H}_{2} \mathrm{O}_{(l)}\) are \(-188\) and \(-286 \mathrm{~kJ} / \mathrm{mol}\) respectively, is [NEET 2001]Hint
(a) \(\Delta H_{f}^{\circ}=\Sigma H_{f \text { (product) }}^{\circ}-\Sigma H_{f}^{\circ}\) (reactant)
For the given reaction
\(
\begin{aligned}
&2 \mathrm{H}_{2} \mathrm{O}_{2(l)} \rightarrow 2 \mathrm{H}_{2} \mathrm{O}_{(l)}+\mathrm{O}_{2(g)} \\
&\Delta H_{f}^{\circ}=2 \times \Delta H_{f}^{\circ}\left(\mathrm{H}_{2} \mathrm{O}\right)-2 \times \Sigma H_{f}^{\circ}\left(\mathrm{H}_{2} \mathrm{O}_{2}\right) \\
&\quad=2 \times-286 \mathrm{~kJ} \mathrm{~mol}^{-1}-2 \times(-188) \mathrm{kJ} \mathrm{mol}^{-1} \\
&\quad=-196 \mathrm{~kJ} \mathrm{~mol}^{-1}
\end{aligned}
\) -
Question 55 of 99
55. Question
When 1 mol of gas is heated at constant volume temperature is raised from 298 to \(308 \mathrm{~K}\). Heat supplied to the gas is \(500 \mathrm{~J}\). Then which statement is correct? [NEET 2001]
Hint
(b) \(\Delta H=\Delta E+P \Delta V\)
When \(\Delta V=0 ; w=0\).
\(\Delta H=\Delta E+0\) or \(\Delta H=\Delta E\)
As \(\Delta E=q+w, \Delta E=q\)
In the present problem, \(\Delta H=500 \mathrm{~J}\),
\(\Delta H=\Delta E=500 \mathrm{~J}, q=500 \mathrm{~J}, w=0\) -
Question 56 of 99
56. Question
Enthalpy of \(\mathrm{CH}_{4}+1 / 2 \mathrm{O}_{2} \rightarrow \mathrm{CH}_{3} \mathrm{OH}\) is negative. If enthalpy of combustion of \(\mathrm{CH}_{4}\) and \(\mathrm{CH}_{3} \mathrm{OH}\) are \(x\) and \(y\) respectively. Then which relation is correct? [NEET 2001]
Hint
(a) \(\mathrm{CH}_{4}+2 \mathrm{O}_{2} \rightarrow \mathrm{CO}_{2}+2 \mathrm{H}_{2} \mathrm{O}, \Delta H_{1}=-x \mathrm{~kJ} \ldots\) (i)
\(
\mathrm{CH}_{3} \mathrm{OH}+\frac{3}{2} \mathrm{O}_{2} \rightarrow \mathrm{CO}_{2}+2 \mathrm{H}_{2} \mathrm{O}, \Delta \mathrm{H}_{2}=-y \mathrm{~kJ} \dots(ii)
\)
Subtracting (ii) from (i), we get
\(
\begin{aligned}
&\mathrm{CH}_{4}+\frac{1}{2} \mathrm{O}_{2} \rightarrow \mathrm{CH}_{3} \mathrm{OH}, \Delta H_{3}=-\text { ve } \\
&\text { i.e., }-x-(-y)=-\text { ve } \\
&y-x=-\text { ve } \\
&\text { Hence, } x>y \text {. }
\end{aligned}
\) -
Question 57 of 99
57. Question
\(\mathrm{PbO}_{2} \rightarrow \mathrm{PbO} ; \Delta G_{298}<0\)
\(\mathrm{SnO}_{2} \rightarrow \mathrm{SnO} ; \Delta G_{298}>0\)
Most probable oxidation state of \(\mathrm{Pb}\) and \(\mathrm{Sn}\) will be [NEET 2001]Hint
(d) The sign and magnitude of Gibb’s free energy is a criterion of spontaneity for a process. When \(\Delta G>0\) or +ve, it means \(G_{\text {product }}>G_{\text {reactant }}\) as \(\Delta G=G_{\text {products }}-G_{\text {reactants }}\)
the reaction will not take place spontaneously, i.e. the reaction should be spontaneous in reverse direction\(
\begin{array}{l}
\quad \underset{+4}{\mathrm{SnO}_2} \rightarrow \underset{+2}{\mathrm{SnO~} ; \quad \Delta G>0} \\
\text { (more favourable) } \\
\end{array}
\)
\(\Delta G<0\) or \(-\mathrm{ve}\), the reaction or change occurs spontaneously.
\(\underset{+4}{\mathrm{PbO}_2} \rightarrow \underset{+2}{\mathrm{PbO}}\); \(\rightarrow \Delta G<0\)
\(\quad \quad \quad \quad \text { (more favourable) }\) -
Question 58 of 99
58. Question
Cell reaction is spontaneous when [NEET 2000]
Hint
(a) For a cell reaction to be spontaneous \(\Delta G^{\circ}\) should be negative.
As \(\Delta G^{\circ}=-n F E_{\text {cell }}^{\circ}\), so the value will be -ve only when \(E_{\text {cell }}^{\circ}\) is +ve
-
Question 59 of 99
59. Question
\(
\begin{aligned}
&2 \mathrm{Zn}+\mathrm{O}_{2} \rightarrow 2 \mathrm{ZnO} ; \Delta G^{\circ}=-616 \mathrm{~J} \\
&2 \mathrm{Zn}+\mathrm{S}_{2} \rightarrow 2 \mathrm{ZnS} ; \Delta G^{\circ}=-293 \mathrm{~J} \\
&\mathrm{~S}_{2}+2 \mathrm{O}_{2} \rightarrow 2 \mathrm{SO}_{2} ; \Delta G^{\circ}=-408 \mathrm{~J} \\
&\Delta G^{\circ} \text { for the following reaction } \\
&2 \mathrm{ZnS}+3 \mathrm{O}_{2} \rightarrow 2 \mathrm{ZnO}+2 \mathrm{SO}_{2} \text { is }
\end{aligned}
\) [NEET 2000]Hint
(a) For the reactions,
\(2 \mathrm{ZnS} \rightarrow 2 \mathrm{Zn}+\mathrm{S}_{2} ; \Delta G^{\circ}=+293 \mathrm{~J} \dots(1)\)
\(2 \mathrm{Zn}+\mathrm{O}_{2} \rightarrow 2 \mathrm{ZnO} ; \Delta G^{\circ}=-616 \mathrm{~J} \dots(2) \)
\(\mathrm{S}_{2}+2 \mathrm{O}_{2} \rightarrow 2 \mathrm{SO}_{2} ; \Delta G^{\circ}=-408 \mathrm{~J} \dots(3)\)
The \(\Delta G^{\circ}\) for the reaction,
\(2 \mathrm{ZnS}+3 \mathrm{O}_{2} \rightarrow 2 \mathrm{ZnO}+2 \mathrm{SO}_{2}\) can be obtained by adding eq. (1), (2) and (3).
So, \(\Delta G^{\circ}=293-616-408=-731 \mathrm{~J}\) -
Question 60 of 99
60. Question
At \(27^{\circ} \mathrm{C}\) latent heat of fusion of a compound is \(2930 \mathrm{~J} / \mathrm{mol}\). Entropy change is [NEET 2000]
Hint
\(
\text { (a) } \Delta S=\frac{Q}{T}=\frac{2930}{300}=9.77 \mathrm{~J} / \mathrm{mol} \mathrm{K}
\) -
Question 61 of 99
61. Question
For the reaction,
\(\mathrm{C}_{2} \mathrm{H}_{5} \mathrm{OH}_{(l)}+3 \mathrm{O}_{2(g)} \rightarrow 2 \mathrm{CO}_{2(g)}+3 \mathrm{H}_{2} \mathrm{O}_{(l)}\) which one is true [NEEET 2000]Hint
(a) \(\Delta H=\Delta E+P \Delta V\)
also \(P V=n R T\) (ideal gas equation)
\(
\begin{aligned}
\text { or } P \Delta V=\Delta n_{g} R T \\
\Delta n_{g} =\text { change in number of gaseous moles } \\
\therefore \quad \Delta H=\Delta E+\Delta n_{g} R T \Rightarrow \Delta n_{g}=2-3=-1 \\
\Rightarrow \Delta H=\Delta E-R T
\end{aligned}
\) -
Question 62 of 99
62. Question
In an endothermic reaction, the value of \(\Delta H\) is [AIPMT 1999]
Hint
(b) In endothermic reactions, energy of reactants is less than energy of products. Thus, \(E_{R}<E_{P}\).
\(
\Delta H=E_{P}-E_{R}=+\text { ve }
\) -
Question 63 of 99
63. Question
In the reaction: \(\mathrm{S}+3/2 \mathrm{~O}_{2} \rightarrow \mathrm{SO}_{3}+2 x\) kcal and \(\mathrm{SO}_{2}+1 / 2 \mathrm{O}_{2} \rightarrow \mathrm{SO}_{3}+y \mathrm{~kcal}\), the heat of formation of \(\mathrm{SO}_{2}\) is [AIPMT 1999]
Hint
(d) \(\mathrm{S}+\frac{3}{2} \mathrm{O}_{2} \rightarrow \mathrm{SO}_{3}+2 x \mathrm{~kcal} \dots(i)\)
\(
\mathrm{SO}_{2}+\frac{1}{2} \mathrm{O}_{2} \rightarrow \mathrm{SO}_{3}+y \mathrm{~kcal} \dots(ii)
\)
By substracting equation (ii) from (i) we get,
\(
\mathrm{S}+\mathrm{O}_{2} \rightarrow \mathrm{SO}_{2}+(2 x-y) \mathrm{~kcal}
\)
The heat of formation of \(\mathrm{SO}_{2}\) is \((2 x-y) \mathrm{~kcal} / \mathrm{mole}\) -
Question 64 of 99
64. Question
Identify the correct statement regarding entropy.[AIPMT 1998]
Hint
(c) The entropy of a substance increases with increase in temperature. However at absolute zero the entropy of a perfectly crystalline substance is taken as zero, which is also called as third law of thermodynamics.
-
Question 65 of 99
65. Question
One mole of an ideal gas at \(300 \mathrm{~K}\) is expanded isothermally from an initial volume of 1 litre to 10 litres. The \(\Delta E\) for this process is \(\left(R=2 \mathrm{cal} \mathrm{mol}^{-1} \mathrm{~K}^{-1}\right)\) [AIPMT 1998]
Hint
(b) Change in internal energy depends upon temperature. At constant temperature, the internal energy of the gas remains constant, so \(\Delta E=0\)
-
Question 66 of 99
66. Question
Given that \(\mathrm{C}+\mathrm{O}_{2} \rightarrow \mathrm{CO}_{2}, \Delta H^{\circ}=-x \mathrm{~kJ}\)
\(2 \mathrm{CO}+\mathrm{O}_{2} \rightarrow 2 \mathrm{CO}_{2}, \Delta H^{\circ}=-y \mathrm{~kJ}\)
The enthalpy of formation of carbon monoxide will be [AIPMT 1997]Hint
(a) \(\mathrm{C}_{(s)}+\mathrm{O}_{2(g)} \rightarrow \mathrm{CO}_{2(g)} ; \Delta H=-x \mathrm{~kJ} \dots(i)\) \(\mathrm{CO}_{(g)}+\frac{1}{2} \mathrm{O}_{2(g)} \rightarrow \mathrm{CO}_{2(g)} ; \Delta H=-\frac{y}{2} \mathrm{~kJ} \dots(ii)\)
By substracting equation (ii) from (i) we get,
\(
\begin{aligned}
&\mathrm{C}_{(s)}+\frac{1}{2} \mathrm{O}_{2(g)} \rightarrow \mathrm{CO}_{(g)} \\
&\Delta H=-x-\left(-\frac{y}{2}\right)=\frac{y-2 x}{2} {\mathrm{~kJ}}
\end{aligned}
\) -
Question 67 of 99
67. Question
Which of the following is the correct equation? [AIPMT 1996]
Hint
(b) This is the mathematical relation of first law of thermodynamics. Here \(\Delta U=\) change in internal energy; \(\Delta Q=\) heat absorbed by the system and \(W=\) work done by the system
-
Question 68 of 99
68. Question
If enthalpies of formation for \(\mathrm{C}_{2} \mathrm{H}_{4(\mathrm{~g})}, \mathrm{CO}_{2(\mathrm{~g})}\) and \(\mathrm{H}_{2} \mathrm{O}_{(l)}\) at \(25^{\circ} \mathrm{C}\) and 1 atm pressure are 52 , \(-394\) and \(-286 \mathrm{~kJ} / \mathrm{mol}\) respectively, then enthalpy of combustion of \(\mathrm{C}_{2} \mathrm{H}_{4(\mathrm{~g})}\) will be [AIPMT 1995]
Hint
(d)
\(
\begin{aligned}
&\mathrm{C}_{2} \mathrm{H}_{4}+3 \mathrm{O}_{2} \rightarrow 2 \mathrm{CO}_{2}+2 \mathrm{H}_{2} \mathrm{O} \\
&\Delta H=\Delta H_{\text {products }}-\Delta H_{\text {reactants }} \\
&=2 \times(-394)+2 \times(-286)-(52+0)=-1412 \mathrm{~kJ} / \mathrm{mol}
\end{aligned}
\) -
Question 69 of 99
69. Question
A chemical reaction is catalyzed by a catalyst \(X\). Hence \(X\) [AIPMT 1995]
Hint
(b) Since a catalyst affects equally both forward and backward reactions, therefore it does not affect equilibrium constant of reaction.
-
Question 70 of 99
70. Question
Standard state Gibb’s free energy change for isomerization reaction cis-2-pentene \(\rightleftharpoons\) trans-2-pentene is \(-3.67 \mathrm{~kJ} / \mathrm{mol}\) at \(400 \mathrm{~K}\). If more trans-2-pentene is added to the reaction vessel, then [AIPMT 1994]
Hint
(c) If more trans-2-pentene is added, then its concentration in right hand side will increase. But in order to maintain the constant \(K\), concentration of cis-2-pentene will also increase. Therefore more cis-2-pentene will be formed.
-
Question 71 of 99
71. Question
For a reaction to occur spontaneously
Hint
(c) For a reaction to be spontaneous, \(\Delta G\) (Gibb’s free energy change) must be negative.
\(
\Delta G=\Delta H-T \Delta S
\)
\(\Delta H=\) change in enthalpy, \(\Delta S=\) change in entropy -
Question 72 of 99
72. Question
During isothermal expansion of an ideal gas, its [AIPMT 1994,91]
Hint
(c) During isothermal expansion of an ideal gas, \(\Delta T=0\).
Now we know \(H=E+P V\)
\(
\begin{array}{ll}
\because & \Delta H=\Delta E+\Delta(P V)=\Delta E+\Delta(n R T) \\
\therefore & \Delta H=\Delta E+n R \Delta T=0+0=0
\end{array}
\) -
Question 73 of 99
73. Question
Following reaction occurring in an automobile \(2 \mathrm{C}_{8} \mathrm{H}_{18(g)}+25 \mathrm{O}_{2(g)} \rightarrow 16 \mathrm{CO}_{2(g)}+18 \mathrm{H}_{2} \mathrm{O}_{(g)}\)
The sign of \(\Delta H, \Delta S\) and \(\Delta G\) would be [AIPMT 1994]Hint
(d) (i) The given reaction is a combustion reaction, therefore \(\Delta H \) is less than 0 . Hence, \(\Delta H \) is negative.
(ii) Since there is increase in the number of moles, therefore \(\Delta S \) is positive
(iii) Since reaction is spontaneous, therefore \(\Delta G \)is negative. -
Question 74 of 99
74. Question
For the reaction \(\mathrm{N}_{2}+3 \mathrm{H}_{2} \rightleftharpoons 2 \mathrm{NH}_{3}, \Delta H=\) ? [AIPMT 1991]
Hint
\(
\text { (b) } \Delta n_{g}=2-4=-2, \Delta H=\Delta E-2 R T
\) -
Question 75 of 99
75. Question
If \(\Delta H\) is the change in enthalpy and \(\Delta E\), the change in internal energy accompanying a gaseous reaction, then [AIPMT 1990]
Hint
(d) If \(n_{p}<n_{r} ; \Delta n_{g}=n_{p}-n_{r}=-\mathrm{ve}\). Hence \(\Delta H<\Delta E\)
-
Question 76 of 99
76. Question
The correct option for free expansion of an ideal gas under adiabatic condition is [NEET 2020]
Hint
(d) Free expansion of ideal gas
\(
\begin{aligned}
&P_{\mathrm{ex}}=0 \\
&\therefore w=-P_{\mathrm{ex}} \Delta V=0 \\
&\because \text { Adiabatic process } \Rightarrow q=0 \\
&\Delta E=q+w \text { (first law of thermodynamics) } \\
&\therefore \Delta E=0 \\
&\Delta E=n C_{v} d T \Rightarrow \Delta E=0 \\
&\text { So, } q=0, \Delta T=0, w=0
\end{aligned}
\) -
Question 77 of 99
77. Question
An ideal gas expands isothermally from \(10^{-3} \mathrm{~m}^{3}\) to \(10^{-2} \mathrm{~m}^{3}\) at \(300 \mathrm{~K}\) against a constant pressure of \(10^{5} \mathrm{Nm}^{-2}\). The work done on the gas is [NEET Odisha 2019]
Hint
\(
\text { (c) } W=-P_{\text {and }}\left(V_{f}-V\right)=-10^{5}\left(10^{-2}-10^{-3}\right)=-900 \mathrm{~J}
\) -
Question 78 of 99
78. Question
Which one among the following is the correct option for right relationship between \(C_{p}\) and \(C_{V}\) for one mole of ideal gas? [NEET 2021]
Hint
(b)
For an ideal gas,
\(
C_{p}-C_{V}=n R
\)
(where, \(n=\) number of moles of gas,
\(C_{p^{\prime}} C_{V}=\) specific heat at constant
pressure and volume, \(R=\) universal gas constant)
As \(n=1\), so \(C_{p}-C_{V}=R\) -
Question 79 of 99
79. Question
For irreversible expansion of an ideal gas under isothermal condition the correct option is
[NEET 2021]Hint
(c)
The change in internal energy depends on the temperature. For isothermal process, \(\Delta T=0\).
So, \(\Delta U=0\).
With an expansion of an ideal gas, more space is available for the gaseous particles.
\(\therefore\) Entropy of gas increases so, entropy of system is not zero.
i.e. \(\Delta S \neq 0\) -
Question 80 of 99
80. Question
Which amongst the following options is correct graphical representation of Boyle’s Law? [NEET 2023]
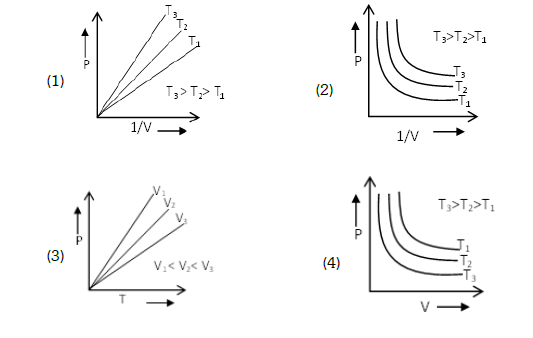
Hint
(d) According to Boyle’s law,
\(
\begin{array}{l}
P V=n R T \\
P=n R T\left(\frac{1}{V}\right)
\end{array}
\)P versus \(\left(\frac{1}{V}\right)\) gives straight line graph with slope \(nRT\).
-
Question 81 of 99
81. Question
Which amongst the following options is the correct relation between change in enthalpy and change in internal energy? [NEET 2023]
Hint
(a) Correct relation between change in enthalpy and change in internal energy is
\(
\Delta H=\Delta U+\Delta n_g R T
\) -
Question 82 of 99
82. Question
Which one is not correct mathematical equation for Dalton’s Law of partial pressure ? Here \(P=\) total pressure of gaseous mixture [NEET 2022]
Hint
(c) Dalton’s law of partial pressure :
Partial pressure of gas \(=\) mole fraction of gas in gaseous mixture \(\times\) Total pressure of gaseous mixture.
\(
\begin{array}{l}
\mathrm{p}_1=\mathrm{X}_1 \mathrm{p} \\
\mathrm{p}_2=\mathrm{X}_2 \mathrm{p} \\
\mathrm{p}_3=\mathrm{X}_3 \mathrm{p}
\end{array}
\)Total pressure,
\(
\mathrm{p}=\mathrm{p}_1+\mathrm{p}_2+\mathrm{p}_3
\)Therefore, statement-3 is incorrect.
-
Question 83 of 99
83. Question
A \(10.0 \mathrm{~L}\) flask contains \(64 \mathrm{~g}\) of oxygen at \(27^{\circ} \mathrm{C}\). (Assume \(\mathrm{O}_2\) gas is behaving ideally). The pressure inside the flask in bar is (Given \(\mathrm{R}=0.0831 \mathrm{~L} {\text {bar } \mathrm{K}^{-1} \mathrm{~mol}^{-1} \text { ) }}\) [NEET 2022]
Hint
(c)
\(
\begin{array}{ll}
\mathrm{V}=10 \mathrm{~L} & \mathrm{~W}_{\mathrm{O}_2}=64 \mathrm{~g} \\
\mathrm{~T}=27^{\circ} \mathrm{C} & \mathrm{n}_{\mathrm{O}_2}=2 \\
\mathrm{R}=0.083 . \mathrm{L} \text { bar K }^{-1} \mathrm{~mol}^{-1}
\end{array}
\)Ideal gas equation \(\mathrm{PV}=\mathrm{nRT}\)
\(
\begin{array}{l}
P=\frac{2 \times 0.0831 \times 300}{10} \\
P=4.9 \text { bar }
\end{array}
\) -
Question 84 of 99
84. Question
Choose the correct statement for the work done in the expansion and heat absorbed or released when 5 litres of an ideal gas at 10 atmospheric pressure isothermally expands into vacuum until volume is 15 litres: [NEET 2024 (Re-Examination)]
Hint
(c) Since it is isothermal, \(\Delta T =0\)
\(
\Delta U = nC _{ v } \Delta T =0
\)
Since expansion is taking place against vacuum
\(
\begin{aligned}
& P_{\text {ext }}=0 \\
& W=-P_{\text {ext }} \Delta V=0
\end{aligned}
\)
From first law of thermodynamics,
\(
\begin{aligned}
& \Delta U=q+W \\
& 0=q+0 \\
& q=0
\end{aligned}
\) -
Question 85 of 99
85. Question
For an endothermic reaction: [NEET 2024 (Re-Examination)]
(A) \(q_p\) is negative.
(B) \(\Delta_{ r } H\) is positive.
(C) \(\Delta_r H\) is negative.
(D) \(q_p\) is positive.
Choose the correct answer from the options given below:Hint
(a) For endothermic reactions, \(\Delta H_r=\) positive (Heat is absorbed)
and \(
q_p=+v e
\) -
Question 86 of 99
86. Question
For the following reaction at 300 K
\(
A _2(g)+3 B_2(g) \rightarrow 2 AB _3(g)
\)
the enthalpy change is +15 kJ , then the internal energy change is : [NEET 2024 (Re-Examination)]Hint
(a) To determine the internal energy change \((\Delta U )\) for the reaction at 300 K , we will use the relationship between enthalpy change \((\Delta H)\) and internal energy change:
\(
\Delta H=\Delta U+\Delta n R T
\)
where \(\Delta H\) is the enthalpy change, \(\Delta U\) is the internal energy change, \(\Delta n\) is the change in moles of gas, \(R\) is the universal gas constant, and \(T\) is the temperature.
Given:
\(
\begin{aligned}
& \Delta H=+15 kJ=15000 J \\
& T=300 K \\
& R=8.314 J mol ^{-1} K^{-1}
\end{aligned}
\)
\(\Delta n=\) moles of products – moles of reactants
From the balanced chemical equation:
\(
A _2(g)+3 B_2(g) \rightarrow 2 AB _3(g)
[latex]
Moles of reactants [latex]=1\left(\right.\) for \(\left.A _2\right)+3\left(\right.\) for \(\left.B _2\right)=4\) moles
Moles of products \(=2\left(\right.\) for \(\left.AB _3\right)\)
Therefore, \(\Delta n=2\) (products) -4 (reactants) \(=-2\)
Now, substituting these values into the equation:
\(
\begin{aligned}
& \Delta H=\Delta U+\Delta n R T \\
& 15000=\Delta U+(-2)(8.314)(300)
\end{aligned}
\)
Simplify the equation:
\(
15000=\Delta U-4988.4
\)
Therefore:
\(
\Delta U=15000+4988.4=19988.4 J
\)
Hence, the internal energy change is:
Option A: 19988.4 J -
Question 87 of 99
87. Question
In which of the following processes entropy increases? [NEET 2024]
A. A liquid evaporates to vapour.
B. Temperature of a crystalline solid lowered from 130 K to 0 K .
C. \(2 NaHCO _{3(s)} \rightarrow Na _2 CO _{3(s)}+ CO _{2(g)}+ H _2 O _{( g )}\)
D. \(Cl _{2(g)} \rightarrow 2 Cl _{(g)}\)
Choose the correct answer from the options given below:Hint
(c) A. A liquid evaporates to vapour.
During the evaporation of a liquid to form a vapour, the molecules of the substance move from a relatively ordered state (liquid) to a more disordered state (vapour). In a vapour, the molecules have more freedom of motion and are less confined than in a liquid. This transition from liquid to vapour increases the randomness or disorder of the system, hence, the entropy increases.
B. Temperature of a crystalline solid lowered from 130 K to 0 K .Reducing the temperature of a crystalline solid generally decreases the entropy of the system. As the temperature decreases, the molecular motion within the solid becomes more restricted, leading to a decrease in randomness. At absolute zero \((0 K)\), the entropy is at its lowest possible value (ideally zero for a perfect crystal), as the molecular motion is minimized to only quantum mechanical vibrations.
c. \(2 NaHCO _{3(s)} \rightarrow Na _2 CO _{3(s)}+ CO _{2(g)}+ H _2 O _{(g)}\)In this chemical reaction, solid sodium bicarbonate decomposes to form solid sodium carbonate, carbon dioxide gas, and water vapor. The formation of gases from a solid significantly increases the entropy of the system because gases have much higher randomness due to their free molecular motion compared to solids.
D. \(Cl _{2(g)} \rightarrow 2 Cl _{(g)}\)
The dissociation of chlorine gas \(\left( Cl _2\right)\) into atomic chlorine \(( Cl )\) represents a transition from a diatomic molecule to two separate atoms. This increases the number of particles in the gas phase, leading to increased randomness and disorder, hence, increasing the entropy.Conclusion: Based on our analysis, processes A, C, and D involve an increase in entropy, as they each lead to greater disorder or randomness in the system. Option B is incorrect as it wrongly includes lowering the temperature of a solid as increasing entropy. Therefore, the correct answer is:
Option C :
\(A, C\) and \(D\) -
Question 88 of 99
88. Question
Match List I with List II. [NEET 2024]
\(
\begin{array}{|l|l|l|l|}
\hline \text { List-I (Process) } & \text { List-II (Conditions) } \\
\hline \text { A. } & \text { Isothermal process } & \text { I. } & \text { No heat exchange } \\
\hline \text { B. } & \text { Isochoric process } & \text { II. } & \begin{array}{l}
\text { Carried out at constant } \\
\text { temperature }
\end{array} \\
\hline \text { C. } & \text { Isobaric process } & \text { III. } & \text { Carried out at constant volume } \\
\hline \text { D. } & \text { Adiabatic process } & \text { IV. } & \begin{array}{l}
\text { Carried out at constant } \\
\text { pressure }
\end{array} \\
\hline
\end{array}
\)
Choose the correct answer from the options given below:Hint
(d) The question involves matching different thermodynamic processes with their corresponding description or characteristics. Let’s analyze these processes:
Isothermal process (A): This process occurs at a constant temperature. Hence, \(A\) should match with “Carried out at constant temperature” (II).
Isochoric process (B): This process occurs at a constant volume. Therefore, \(B\) should be matched with “Carried out at constant volume” (III).
Isobaric process (C): This process happens at a constant pressure, making C correspond to “Carried out at constant pressure” (IV).
Adiabatic process (D): During this process, there is no heat exchange with the surroundings. Consequently, D should be paired with “No heat exchange” (I).
The correct matches, as elaborated above, are A-II, B-III, C-IV, D-I. Therefore, the correct answer here is Option D. -
Question 89 of 99
89. Question
The work done during reversible isothermal expansion of one mole of hydrogen gas at \(25^{\circ} C\) from pressure of 20 atmosphere to 10 atmosphere is (Given \(R =2.0 cal K ^{-1} mol^{-1}\) ) [NEET 2024]
Hint
(b) The work done in a reversible isothermal process can be calculated using the formula:
\(
W=-n R T \ln \left(\frac{V_f}{V_i}\right)
\)
Here:
\(W\) is the work done by the gas.
\(n\) is the number of moles of the gas.
\(R\) is the universal gas constant.
\(T\) is the temperature in Kelvin.
\(V_i\) and \(V_f\) are the initial and final volumes of the gas, respectively.
However, since the volumes are not directly provided but the pressures are given, we use the ideal gas law, \(P V=n R T\), to relate pressures and volumes at the same temperature and amount of gas:
For an ideal gas undergoing a change at constant temperature, we can also write the work done in terms of the initial and final pressures:
\(
W=-n R T \ln \left(\frac{P_i}{P_f}\right)
\)
where:
\(P_i\) and \(P_f\) are the initial and final pressures, respectively.
Given:
Substituting all values into the formula:
\(
\begin{aligned}
& W=-1 \cdot 2.0 \cdot 298.15 \ln \left(\frac{20}{10}\right) \\
& W=-2.0 \cdot 298.15 \cdot \ln (2)
\end{aligned}
\)
Now, solving this using the value of \(\ln (2) \approx 0.693\) :
\(
\begin{aligned}
& W \approx-2.0 \cdot 298.15 \cdot 0.693 \\
& W \approx-413.14 \text { calories }
\end{aligned}
\)
Thus, the work done during the process is about -413.14 calories, indicating that this amount of energy was done by the system (expansion work being done by the gas against external pressure), and hence is negative as it indicates work done by the system. Therefore, the correct option is:
\(
\begin{aligned}
& n=1 \text { (one mole of hydrogen) } \\
& T=25^{\circ} C =25+273.15=298.15 K \\
& R=2.0 cal K ^{-1} mol^{-1} \\
& P_i=20 atm \\
& P_f=10 atm
\end{aligned}
\) -
Question 90 of 99
90. Question
Consider the following reaction :
\(
2 H _2(g)+ O _2(g) \rightarrow 2 H _2 O ( g ) \Delta_{ r } H ^{\circ}=-483.64 kJ
\)
What is the enthalpy change for decomposition of one mole of water? (Choose the right option). [NEET Manipur 2024]Hint
(b)
\(
\begin{aligned}
&\text { Decomposition for } 1 \text { mole of water }\\
&\begin{aligned}
& H _2 O ( g ) \rightarrow H _2(g)+\frac{1}{2} O _2(g) ; \Delta H =+\frac{483.64}{2} \\
& \Delta H =+241.82 kJ
\end{aligned}
\end{aligned}
\) -
Question 91 of 99
91. Question
The equilibrium concentrations of the species in the reaction \(A + B \rightleftharpoons C + D\) are \(2,3,10\) and \(6 ~mol L { }^{-1}\), respectively at \(300 K . \Delta G ^0\) for the reaction is \(( R =2 cal / mol K )\) [NEET 2023]
Hint
(b)
\(
\begin{aligned}
&A + B \rightleftharpoons C + D\\
&\begin{aligned}
& {[A]=2 mol L ^{-1}} \\
& {[B]=3 mol L ^{-1}} \\
& {[C]=10 mol L ^{-1}} \\
& {[D]=6 mol L ^{-1}}
\end{aligned}
\end{aligned}
\)
\(
\begin{aligned}
\Delta G ^0 & =-2.303 RT \log K _{ eq } \\
& =-2.303 RT \log \frac{[ C ][ D ]}{[ A ][ B ]} \\
& =-2.303 \times 2 \times 300 \times \log \frac{10 \times 6}{2 \times 3} \\
& =-2.303 \times 2 \times 300 \times \log 10 \\
& =-1381.8 cal
\end{aligned}
\) -
Question 92 of 99
92. Question
One mole of an ideal gas at 300 K is expanded isothermally from 1 L to 10 L volume. \(\Delta U\) for this process is : [NEET 2022 phase 2]
(Use \(R =8.314 J k ^{-1} mol^{-1}\) )Hint
(a)
\(
\begin{aligned}
&\Delta U = nC _{ V } \Delta T\\
&\text { For isothermal condition; } \Delta T=0\\
&\therefore \Delta U =0
\end{aligned}
\) -
Question 93 of 99
93. Question
A vessel contains 3.2 g of dioxygen gas at STP ( 273.15 K and 1 atm pressure). The gas is now transferred to another vessel at constant temperature, where pressure becomes one third of the original pressure. The volume of new vessel in L is : (Given : molar volume at STP is 22.4 L ) [NEET 2022 Phase 2]
Hint
(b) At constant temperature and amount
\(
\begin{aligned}
& P_1 V_1=P_2 V_2 \\
& P_1 V_1=\frac{P_1}{3} V_2\left[\therefore P_2=\frac{P_1}{3}\right] \\
& V_2=3 V_1
\end{aligned}
\)
mole of \(O_2(g)=\frac{3.2}{32}=0.1\) mole
Volume of \(O_2(g)=(0.1 \times 22.4) L =2.24 L\)
At \(\operatorname{STP}\left( V _1\right)\)
\(
\begin{aligned}
& V_2=3 V_1=3 \times 2.24 \\
& =6.72 L
\end{aligned}
\) -
Question 94 of 99
94. Question
Which of the following p-V curve represents maximum work done? [NEET 2022 Phase 1]
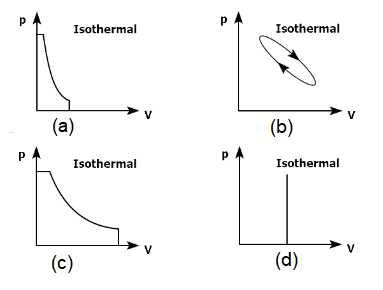
Hint
(c) Work done under any thermodynamic process can be determined by area under the ‘p-V’ graph. As it can be observed maximum area is covered in option ‘c’.
-
Question 95 of 99
95. Question
Hydrolysis of sucrose is given by the following reaction.
Sucrose \(+ H _2 O \rightleftharpoons\) Glucose + Fructose
If the equilibrium constant \(\left( K _{ c }\right)\) is \(2 \times 10^{13}\) at 800 K , the value of \(\Delta_r G^{\Theta}\) at the same temperature will be : [NEET 2020 Phase 1]Hint
(d)
\(
\Delta G=\Delta G^{\circ}+ RT \ln Q
\)
At equilibrium \(\Delta G=0, Q = K _{\text {eq }}\)
So, \(\Delta_r G^{\circ}=- RT\) in \(K _{\text {eq }}\)
\(
\Delta_r G^{\circ}=-8.314 J mol ^{-1} K^{-1} \times 300 K \times \ln \left(2 \times 10^{13}\right)
\) -
Question 96 of 99
96. Question
For the reaction, \(2 Cl ( g ) \rightarrow Cl _2(g)\), the correct option is : [NEET 2020 Phase 1]
Hint
(c) Given reaction,
\(
2 Cl ( g ) \rightarrow Cl _2(g)
\)
This is an endothermic reaction because it requires energy to break bonds.
So, the reverse reaction is exothermic
\(
\Delta_r H<0
\)
Also, two gaseous atoms combine together to form 1 gaseous molecule. So, the randomness,
\(
\Delta_r S<0
\) -
Question 97 of 99
97. Question
In which case change in entropy is negative? [NEET 2019]
Hint
(b)
\(
\begin{aligned}
&2 H ( g ) \rightarrow H _2(g)\\
&\text { Due to bond formation, entropy decreases. }
\end{aligned}
\) -
Question 98 of 99
98. Question
Under isothermal condition, a gas at 300 K expands from 0.1 L to 0.25 L against a constant external pressure of 2 bar . The work done by the gas is [Given that \(1 L bar =100 J\) ] [NEET 2019]
Hint
(c)
\(
\begin{aligned}
& W =- P _{\text {ext }}\left( V _2- V _1\right) \\
& P _{\text {ext }}=2 \text { bar } \\
& V _1=0.1 L \\
& V _2=0.25 L \\
& W =-2 \text { bar }[0.25-0.1] L \\
& W =-2 \times 0.15 \text { bar } L \\
& W =-0.30 \text { bar } L \\
& W =(-0.30) \times 100=-30 J
\end{aligned}
\) -
Question 99 of 99
99. Question
The bond dissociation energies of \(X_2, Y_2\) and \(X Y\) are in the ratio of \(1: 0.5: 1 . \Delta H\) for the formation of \(X Y\) is \(-200 ~kJ mol ^{-1}\). The bond dissociation energy of \(X_2\) will be [NEET 2018]
Hint
(a) Let bond dissociation energies of \(X_2, Y_2\) and \(X Y\) are
\(
x kJ mol ^{-1}, 0.5 \times kJ mol ^{-1} \text { and } xkJ mol ^{-1}
\)
\(
\frac{1}{2} X _2+\frac{1}{2} Y _2 \rightarrow XY ; \Delta H =-200 kJ mol ^{-1}
\)
\(
\Delta H=\Sigma( B . E )_{\text {Reactant }}-\Sigma( B . E )_{\text {Product }}
\)
\(
\begin{aligned}
&\Rightarrow-200=\left[\frac{1}{2} \times x+\frac{1}{2} \times 0.5 x\right]-[1 \times x]\\
&\text { On solving, } x=800 kJ mol ^{-1}
\end{aligned}
\)