NEET PYQs
Quiz Summary
0 of 81 Questions completed
Questions:
Information
You have already completed the quiz before. Hence you can not start it again.
Quiz is loading…
You must sign in or sign up to start the quiz.
You must first complete the following:
Results
Results
0 of 81 Questions answered correctly
Your time:
Time has elapsed
You have reached 0 of 0 point(s), (0)
Earned Point(s): 0 of 0, (0)
0 Essay(s) Pending (Possible Point(s): 0)
Categories
- Not categorized 0%
- 1
- 2
- 3
- 4
- 5
- 6
- 7
- 8
- 9
- 10
- 11
- 12
- 13
- 14
- 15
- 16
- 17
- 18
- 19
- 20
- 21
- 22
- 23
- 24
- 25
- 26
- 27
- 28
- 29
- 30
- 31
- 32
- 33
- 34
- 35
- 36
- 37
- 38
- 39
- 40
- 41
- 42
- 43
- 44
- 45
- 46
- 47
- 48
- 49
- 50
- 51
- 52
- 53
- 54
- 55
- 56
- 57
- 58
- 59
- 60
- 61
- 62
- 63
- 64
- 65
- 66
- 67
- 68
- 69
- 70
- 71
- 72
- 73
- 74
- 75
- 76
- 77
- 78
- 79
- 80
- 81
- Current
- Review
- Answered
- Correct
- Incorrect
-
Question 1 of 81
1. Question
Which one is the wrong statement? [NEET 2017]
CorrectIncorrectHint
(c) In case of hydrogen like atoms, energy depends on the principal quantum number only. Hence, \(2 s\)-orbital will have energy equal to \(2 p\)-orbital.
-
Question 2 of 81
2. Question
How many electrons can fit in the orbital for which \(n=3\) and \(l=1\) ? [NEET 2016]
CorrectIncorrectHint
(b) For \(n=3\) and \(l=1\), the subshell is \(3 p\) and a particular \(3 p\) orbital can accommodate only 6 electrons.
-
Question 3 of 81
3. Question
Which of the following pairs of \(d\)-orbitals will have electron density along the axes? [NEET-II 2016]
CorrectIncorrectHint
(c) \(d_{x^{2}-y^{2}}\) and \(d_{z 2}\) orbitals have electron density along the axes while \(d_{x y}, d_{y z}\) and \(d_{x z}\) orbitals have electron density in between the axes.
-
Question 4 of 81
4. Question
Two electrons occupying the same orbital are distinguished by (NEET-I 2016)
CorrectIncorrectHint
(b) For the two electrons occupying the same orbital values of \(n, l\) and \(m_{l}\) are same but \(m_{s}\) is different, i.e., \(+\frac{1}{2}\) and \(-\frac{1}{2}\).
-
Question 5 of 81
5. Question
Which is the correct order of increasing energy of the listed orbitals in the atom of titanium? (At. no. \(Z=22\) ) [NEET 2015]
CorrectIncorrectHint
(c) \(\operatorname{Ti}(22): 1 s^{2} 2 s^{2} 2 p^{6} 3 s^{2} 3 p^{6} 4 s^{2} 3 d^{2}\)
\(\therefore\) Order of increasing energy is \(3 s, 3 p, 4 s, 3 d\)
. -
Question 6 of 81
6. Question
The number of \(d\)-electrons in \(\mathrm{Fe}^{2+}(Z=26)\) is not equal to the number of electrons in which one of the following? [NEET 2015]
CorrectIncorrectHint
(d) Number of \(d\)-electrons in \(\mathrm{Fe}^{2+}=6\)
Number of \(p\)-electrons in \(\mathrm{Cl}=11\) -
Question 7 of 81
7. Question
The angular momentum of electron in ‘ \(d\) ‘ orbital is equal to [NEET 2015]
CorrectIncorrectHint
(c) Angular momentum \(=\sqrt{l(l+1)} h\); For \(d\) orbital, \(l=2\)
Angular momentum \(=\sqrt{2(2+1)} h=\sqrt{6} h\)
-
Question 8 of 81
8. Question
What is the maximum number of orbitals that can be identified with the following quantum numbers?
\(n=3, l=1, m_{l}=0\) [NEET 2014]CorrectIncorrectHint
(a) Only one orbital, \(3 p_{z}\) has following set of quantum numbers, \(n=3, l=1\) and \(m_{l}=0\).
-
Question 9 of 81
9. Question
Calculate the energy in joule corresponding to light of wavelength \(45 \mathrm{~nm}\). (Planck’s constant, \(h=6.63 \times 10^{-34} \mathrm{~J}\) s, speed of light, \(c=3 \times 10^{8} \mathrm{~m} \mathrm{~s}^{-1}\) ) [AIPMT 2014]
CorrectIncorrectHint
(d) \(E=\frac{h c}{\lambda}\) [Given, \(\lambda=45 \mathrm{~nm}=45 \times 10^{-9} \mathrm{~m}\) ]
On putting the given values in the equation, we get \(E=\frac{6.63 \times 10^{-34} \times 3 \times 10^{8}}{45 \times 10^{-9}}=4.42 \times 10^{-18} \mathrm{~J}\) -
Question 10 of 81
10. Question
\(\mathrm{Be}^{2+}\) is isoelectronic with which of the following ions? [NEET 2014]
CorrectIncorrectHint
(b)
\(
\begin{array}{cc}
\text { Species } & \text { No. of electrons } \\
\mathrm{Be}^{2+} & 2 \\
\mathrm{H}^{+} & 0 \\
\mathrm{Li}^{+} & 2 \\
\mathrm{Na}^{+} & 10 \\
\mathrm{Mg}^{2+} & 10
\end{array}
\) -
Question 11 of 81
11. Question
What is the maximum numbers of electrons that can be associated with the following set of quantum numbers?
\(n=3, l=1\) and \(m=-1\) [NEET 2013]CorrectIncorrectHint
(b) The orbital associated with \(n=3, l=1\) is \(3 p\). One orbital (with \(m=-1\) ) of \(3 p\)-subshell can accommodate maximum 2 electrons.
-
Question 12 of 81
12. Question
Based on equation \(E=-2.178 \times 10^{-18} \mathrm{~J}\left(\frac{Z^{2}}{n^{2}}\right)\), certain conclusions are written. Which of them is not correct? [NEET 2013]
CorrectIncorrectHint
(b) The electron is more tightly bound in the smallest allowed orbit.
Note: The lowest possible energy state is the one in which the electrons are most tightly bound, and it’s called the ground state. In the ground state there is more force of attraction which means elections in ground state are tightly bounded.
-
Question 13 of 81
13. Question
The value of Planck’s constant is \(6.63 \times 10^{-34} \mathrm{~J} \mathrm{~s}\). The speed of light is \(3 \times 10^{17} \mathrm{~nm} \mathrm{~s}^{-1}\). Which value is closest to the wavelength in nanometer of a quantum of light with frequency of \(6 \times 10^{15} \mathrm{~s}^{-1}\)? [NEET 2013]
CorrectIncorrectHint
(a) \(c=f \lambda\)
\(
\lambda=\frac{c}{f}=\frac{3 \times 10^{17}}{6 \times 10^{15}}=50 \mathrm{~nm}
\) -
Question 14 of 81
14. Question
The outer electronic configuration of \(\mathrm{Gd}\) (At. No. 64) is [Karnataka NEET 2013]
CorrectIncorrectHint
(c) Half-filled stabled configuration will be more favoured to Gd. Arrange the orbitals according to the increasing energy. The configuration of Gd is \([\mathrm{Xe}] 4 \mathrm{f}^7 5 \mathrm{~d}^1 6 \mathrm{~s}^2\).
-
Question 15 of 81
15. Question
According to law of photochemical equivalence the energy absorbed (in ergs/mole) is given as \(\left(h=6.62 \times 10^{-27} \operatorname{ergs}, c=3 \times 10^{10} \mathrm{~cm} \mathrm{~s}^{-1}\right.\), \(\left.N_{A}=6.02 \times 10^{-23} \mathrm{~mol}^{-1}\right)\) [Karnataka NEET 2013]
CorrectIncorrectHint
\(
\text { (a) We know that, } E=\frac{h c N_{A}}{\lambda}
\)\(
\begin{aligned}
&=\frac{6.62 \times 10^{-27} \times 3 \times 10^{10} \times 6.02 \times 10^{23}}{\lambda} \\
&=\frac{1.1955 \times 10^{8}}{\lambda}=\frac{1.196 \times 10^{8}}{\lambda} \mathrm{ergs} \mathrm{~mol}^{-1}
\end{aligned}
\) -
Question 16 of 81
16. Question
Maximum number of electrons in a subshell with \(l=3\) and \(n=4\) is [NEET 2012]
CorrectIncorrectHint
(a) \(l=3\) and \(n=4\) represent \(4 f\). So, total number of electrons in a subshell \(=2(2 l+1)=2(2 \times 3+1)=14\) electrons. Hence \(f\)-subshell can contain maximum 14 electrons.
-
Question 17 of 81
17. Question
The correct set of four quantum numbers for the valence electron of rubidium atom \((Z=37)\) is [NEET 2012]
CorrectIncorrectHint
(c) \(\mathrm{Rb}(37): 1 s^{2} 2 s^{2} 2 p^{6} 3 s^{2} 3 p^{6} 4 s^{2} 3 d^{10} 4 p^{6} 5 s^{1}\)
For \(5 s, n=5, l=0, m=0, s=+1 / 2\) or \(-1 / 2\)
-
Question 18 of 81
18. Question
The orbital angular momentum of a \(p\)-electron is given as [Mains 2012]
CorrectIncorrectHint
(a) Orbital angular momentum
\((m)=\sqrt{l(l+1)} \frac{h}{2 \pi}\)
For \(p\)-electrons; \(l=1\)
Thus, \(m=\sqrt{1(1+1)} \frac{h}{2 \pi}=\frac{\sqrt{2} h}{2 \pi}=\frac{h}{\sqrt{2} \pi}\) -
Question 19 of 81
19. Question
The total number of atomic orbitals in fourth energy level of an atom is [NEET 2011]
CorrectIncorrectHint
(b) Total number of atomic orbitals in any energy level is given by \(n^{2}\). It should be 16.
-
Question 20 of 81
20. Question
The energies \(E_{1}\) and \(E_{2}\) of two radiations are \(25 \mathrm{~eV}\) and \(50 \mathrm{~eV}\) respectively. The relation between their wavelengths i.e., \(\lambda_{1}\) and \(\lambda_{2}\) will be [NEET 2011]
CorrectIncorrectHint
(b) We know the relation between energy and lambda
\(
E_1=\frac{h c}{\lambda_1} \text { and } E_2=\frac{h c}{\lambda_2}
\)
\(
\begin{array}{l}
\frac{E_1}{E_2}=\frac{h c}{\lambda_1} \times \frac{\lambda_2}{h c}=\frac{\lambda_2}{\lambda_1} \\
\frac{25}{50}=\frac{\lambda_2}{\lambda_1} \text { or } \frac{1}{2}=\frac{\lambda_2}{\lambda_1} \Rightarrow \lambda_1=2 \lambda_2
\end{array}
\) -
Question 21 of 81
21. Question
If \(n=6\), the correct sequence for filling of electrons will be [NEET 2011]
CorrectIncorrectHint
(a) Principle quantum number \((n)\) tells us the number of shells in which the last electron enters.
Basically, the principal quantum number tells us about the valence shell.
According to Aufbau’s rule, electrons will fill lower energy atomic levels first and then get filled up in the higher energy levels.
Aufbau’s rule is represented below in the given diagram.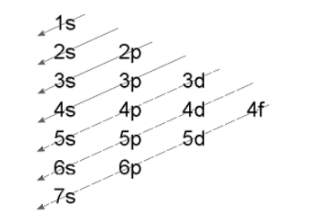
The electrons will start filling up in the order:
\(1s\) then \(2 s\) then \(2 p\) then \(3 s, 3 p, 4 s, 3 d, 4 p, 5 s, 4 d, 5 p, 6 s, 4 f, 5 d, 6 p\) and then \(7 s\) and \(7 p\).
\(1s\) orbital has the least amount of energy.
If the valence electron enters in the sixth orbital then, the electron enters in 6 s orbital followed by \(4 \mathrm{f}\) orbital then \(5 \mathrm{~d}\) orbital and then \(6 \mathrm{p}\) orbital.
Therefore, when \(n=6\), the electron fills in the order \(6 s, 4 f, 5 d\) and \(6 p\).
So, the option \(n s \rightarrow(n-2) f \rightarrow(n-1) d \rightarrow n p\) is correct. -
Question 22 of 81
22. Question
According to the Bohr theory, which of the following transitions in the hydrogen atom will give rise to the least energetic photon? [NEET 2011]
CorrectIncorrectHint
(c) We know that
\(\Delta E \propto\left[\frac{1}{n_{1}^{2}}-\frac{1}{n_{2}^{2}}\right]\), where \(n_{2}>n_{1}\)
\(\therefore n=6\) to \(n=5\) will give least energetic photon. -
Question 23 of 81
23. Question
A \(0.66 \mathrm{~kg}\) ball is moving with a speed of \(100 \mathrm{~m} / \mathrm{s}\). The associated wavelength will be \(\left(h=6.6 \times 10^{-34} \mathrm{~J} \mathrm{~s}\right)\)
CorrectIncorrectHint
(c) According to de-Broglie equation, \(\lambda=\frac{h}{m v}\) Given, \(h=6.6 \times 10^{-34} \mathrm{Js} ; m=0.66 \mathrm{~kg} ; v=100 \mathrm{~m} \mathrm{~s}^{-1}\)
\(
\therefore \quad \lambda=\frac{6.6 \times 10^{-34}}{0.66 \times 100}=1 \times 10^{-35} \mathrm{~m}
\) -
Question 24 of 81
24. Question
Maximum number of electrons in a subshell of an atom is determined by the following [NEET 2009]
CorrectIncorrectHint
(d) For a given shell, \(l\),
the number of subshells, \(m_{l}=(2 l+1)\)
Since each subshell can accommodate 2 electrons of opposite spin, so maximum number of electrons in a subshell \(=2(2 l+1)=4 l+2\). -
Question 25 of 81
25. Question
Which of the following is not permissible arrangement of electrons in an atom? [NEET 2009]
CorrectIncorrectHint
(b) In an atom, for any value of \(n\), the values of \(l=0\) to \((n-1)\).
For a given value of \(l\), the values of \(m_{l}=-l\) to 0 to \(+l\) and the value of \(s=+1 / 2\) or \(-1 / 2\).
In option (b), \(l=2\) and \(m_{l}=-3\)
This is not possible, as values of \(m_{l}\) which are possible for \(l=2\) are \(-2,-1,0,+1\) and \(+2\) only. -
Question 26 of 81
26. Question
The measurement of the electron position is associated with an uncertainty in momentum, which is equal to \(1 \times 10^{-18} \mathrm{~g} \mathrm{~cm} \mathrm{~s}^{-1}\). The uncertainty in electron velocity is (mass of an electron is \(\left.9 \times 10^{-28} \mathrm{~g}\right)\) [NEET 2008]
CorrectIncorrectHint
(c) Uncertainty in momentum
\(
(m \Delta v)=1 \times 10^{-18} \mathrm{~g} \mathrm{~cm} \mathrm{~s}^{-1}
\)
Uncertainty in velocity,
\(
(\Delta v)=\frac{1 \times 10^{-18}}{9 \times 10^{-28}}=1.1 \times 10^{9} \mathrm{~cm} \mathrm{~s}^{-1}
\) -
Question 27 of 81
27. Question
Consider the following sets of quantum numbers:
\(\begin{array}{lcccc} & n & l & m & s \\ \text { (i) } & 3 & 0 & 0 & +1 / 2 \\ \text { (ii) } & 2 & 2 & 1 & +1 / 2 \\ \text { (iii) } & 4 & 3 & -2 & -1 / 2 \\ \text { (iv) } & 1 & 0 & -1 & -1 / 2 \\ \text { (v) } & 3 & 2 & 3 & +1 / 2\end{array}\)
Which of the following sets of quantum number is not possible? [Prelims 2008]CorrectIncorrectHint
(b)
(i) represents an electron in \(3 s\) orbital.
(ii) is not possible as value of \(l\) varies from \(0,1, \ldots(n-1)\).
(iii) represents an electron in \(4 f\) orbital.
(iv) is not possible as value of \(m\) varies from \(-l \ldots+l\)
(v) is not possible as value of \(m\) varies from \(-l \ldots+l\), it can never be greater than \(l\). -
Question 28 of 81
28. Question
If uncertainty in position and momentum are equal, then uncertainty in velocity is [NEET 2008]
CorrectIncorrectHint
(c) From Heisenberg uncertainty principle \(\Delta p \cdot \Delta x \geq \frac{h}{4 \pi}\) or \(m \Delta v \times \Delta x \geq \frac{h}{4 \pi}\) or \((m \Delta v)^{2} \geq \frac{h}{4 \pi}\) \((\because \Delta x=\Delta p)\) or \(\Delta v \geq \frac{1}{2 m} \sqrt{\frac{h}{\pi}}\)
-
Question 29 of 81
29. Question
The orientation of an atomic orbital is governed by [NEET 2006]
CorrectIncorrectHint
(d) Magnetic quantum number
The orientation of an atomic orbital is governed by magnetic quantum number.
-
Question 30 of 81
30. Question
Given : The mass of electron is \(9.11 \times 10^{-31} \mathrm{~kg}\), Planck constant is \(6.626 \times 10^{-34} \mathrm{~J}\) s, the uncertainty involved in the measurement of velocity within a distance of 0.1 Å is [NEET 2006]
CorrectIncorrectHint
(b) \(\Delta x \cdot m \Delta v=h / 4 \pi\)
\(
\begin{array}{r}
0.1 \times 10^{-10} \times 9.11 \times 10^{-31} \times \Delta v=\frac{6.626 \times 10^{-34}}{4 \times 3.143} \\
\therefore \Delta v=\frac{6.626 \times 10^{-34}}{0.1 \times 10^{-10} \times 9.11 \times 10^{-31} \times 4 \times 3.143} \\
=5.79 \times 10^{6} \mathrm{~m} \mathrm{~s}^{-1}
\end{array}
\) -
Question 31 of 81
31. Question
The energy of second Bohr orbit of the hydrogen atom is \(-328 \mathrm{~kJ} \mathrm{~mol}^{-1}\); hence the energy of fourth Bohr orbit would be [NEET 2005]
CorrectIncorrectHint
(b) \(E_{n}=-K\left(\frac{Z}{n}\right)^{2}\)
\(Z=1\) for hydrogen \(; n=2\)
\(
\begin{gathered}
E_{2}=\frac{-K \times 1}{4} \Rightarrow E_{2}=-328 \mathrm{~kJ} \mathrm{~mol}^{-1} ; K=4 \times 328 \\
E_{4}=\frac{-K \times 1}{16} \Rightarrow E_{4}=-4 \times 328 \times \frac{1}{16}=-82 \mathrm{~kJ} \mathrm{~mol}^{-1}
\end{gathered}
\) -
Question 32 of 81
32. Question
The frequency of radiation emitted when the electron falls from \(n=4\) to \(n=1\) in a hydrogen atom will be (Given ionization energy of \(\mathrm{H}=2.18 \times 10^{-18} \mathrm{~J}\) atom \(^{-1}\) and \(h=6.625 \times 10^{-34} \mathrm{~J} \mathrm{~s}\) ) [NEET 2005]
CorrectIncorrectHint
(b)
Formula Used: Planck’s equation:
\(\Delta E=h \nu\) where \(\Delta E\) is the change in energy, \(\mathrm{h}\) is thee planck’s constant and \(\nu\) is the frequency.
Complete step by step answer: Ionization energy means it is the energy for the first orbit is given to be
\(2.18 \times 10^{-18}\) Jatom \(^{-1}\). Atomic number of hydrogen atom is 1 i.e. \(Z=1\), then energy for \(n^{\text {th }}\) orbit is given as:
\(
E_n=\frac{-2.18 \times 10^{-18}}{n^2} \dots(1)
\)
For \(n=1\)
\(
E=-2.18 \times 10^{-18}
\)
The change in energy when electrons fall from \(n=4\) to \(n=1\) is given as:
\(
\Delta E=E_4-E_1
\)
Then, using (1):
\(
\begin{array}{l}
\Delta E=\frac{-2.18 \times 10^{-18}}{4^2}-\left(\frac{-2.18 \times 10^{-18}}{1^2}\right) \\
\Rightarrow \Delta E=-2.18 \times 10^{-18}\left(\frac{1}{4^2}-\frac{1}{1^2}\right) \\
\Rightarrow \Delta E=-2.18 \times 10^{-18} \times\left(-\frac{15}{16}\right) \\
\Rightarrow \Delta E=2.0437 \times 10^{-18} \text { Jatom }^{-1}
\end{array}
\)
According to Planck’s equation the change in energy is directly proportional to frequency of electron and Planck’s constant ( \(h\) ) is used as the proportionality constant. So:
\(
\begin{array}{l}
\Delta E=h \nu \\
\Rightarrow \nu=\frac{\Delta E}{h}
\end{array}
\)
Substituting the known values to find the value of frequency, we get:
\(
\begin{array}{l}
\nu=\frac{2.0437 \times 10^{-18}}{6.625 \times 10^{-34}} \\
\Rightarrow \nu=3.084 \times 10^{15} \mathrm{~s}^{-1}
\end{array}
\) -
Question 33 of 81
33. Question
The value of Planck’s constant is \(6.63 \times 10^{-34} \mathrm{~J} \mathrm{~s}\). The velocity of light is \(3.0 \times 10^{8} \mathrm{~m} \mathrm{~s}^{-1}\). Which value is closest to the wavelength in nanometers of a quantum of light with frequency of \(8 \times 10^{15} \mathrm{~s}^{-1}\) ? [AIPMT 2003]
CorrectIncorrectHint
(c) Applying \((f)=c / \lambda\),
\(\lambda=\frac{c}{f}=\frac{3 \times 10^{8}}{8 \times 10^{15}}=37.5 \times 10^{-9} \mathrm{~m}=37.5 \mathrm{~nm} \approx 4 \times 10^{1} \mathrm{~nm}
\) -
Question 34 of 81
34. Question
In hydrogen atom, energy of first excited state is \(-3.4 \mathrm{~eV}\). Then find out \(K . E\). of same orbit of hydrogen atom [AIPMT 2002]
CorrectIncorrectHint
(a) Kinetic energy \(=\frac{1}{2} m v^{2}=\left(\frac{\pi e^{2}}{n h}\right)^{2} \times 2 m\)
\(
\left[\because v=\frac{2 \pi e^{2}}{n h}\right]
\)
Total energy \(E_{n}=-\frac{2 \pi^{2} m e^{4}}{n^{2} h^{2}}=-\left(\frac{\pi e^{2}}{n h}\right)^{2} \times 2 m=-K . E\).
\(\therefore\) Kinetic energy \(=-E_{n}\)
Energy of first excited state is \(-3.4 \mathrm{~eV}\)
\(\therefore\) Kinetic energy of same orbit \((n=2)\) will be \(+3.4 \mathrm{~eV}\). -
Question 35 of 81
35. Question
Main axis of a diatomic molecule is \(z\), molecular orbital \(p_{x}\) and \(p_{y}\) overlap to form which of the following orbitals. [AIPMT 2002]
CorrectIncorrectHint
(a) For \(\pi\) overlap, the lobes of the atomic orbitals are perpendicular to the line joining the nuclei.
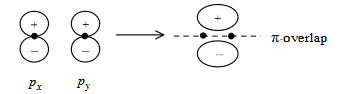
Hence, only sidewise overlapping takes place. -
Question 36 of 81
36. Question
The following quantum numbers are possible for how many orbitals : \(n=3, l=2, m=+2\) ? [NEET 2001]
CorrectIncorrectHint
Explanation for the correct option
(a ) 1
– \(\mathrm{n}=3, \mathrm{l}=2, \mathrm{~m}=+2\) denotes a \(3 \mathrm{~d}\) orbital containing the magnetic quantum number +2 .
– For \(\mathrm{n}=3, \mathrm{l}=2\), we know that \(\mathrm{m}=-2,-1,0,+1,+2\)
– No two orbitals have the same magnetic quantum number.
– As a result, the provided quantum number is only achievable for one orbital and two electrons.
– Hence this option is correct.Explanation for the incorrect options:
(d) 4
– There are no two orbitals with the same quantum number.
– Hence no 4 orbitals can have the same quantum numbers.
– This option is incorrect.
(c) 3
– There are no two orbitals with the same quantum number.
– Hence no 3 orbitals can have the same quantum numbers.
– This option is incorrect.
(b) 2
– There are no two orbitals with the same quantum number.
– Hence no 2 orbitals can have the same quantum numbers.
– This option is incorrect. -
Question 37 of 81
37. Question
For given energy, \(E=3.03 \times 10^{-19}\) Joules corresponding wavelength is \(\left(h=6.626 \times 10^{-34} \mathrm{~J} \mathrm{sec}, c=3 \times 10^{8} \mathrm{~m} / \mathrm{sec}\right)\) [AIPMT 2000]
CorrectIncorrectHint
(d)
\(
\begin{aligned}
E=\frac{h c}{\lambda} \Rightarrow \lambda &=\frac{6 \cdot 6 \times 10^{-34} \times 3 \times 10^{8}}{3.03 \times 10^{-19}} \\
&=656 \mathrm{~nm}
\end{aligned}
\) -
Question 38 of 81
38. Question
Isoelectronic species are [AIPMT 2000]
CorrectIncorrectHint
(a) Species having same no. of electrons are called isoelectronics.
The no. of electrons in \(\mathrm{CO}=\mathrm{CN}^{-}=\mathrm{NO}^{+}=\mathrm{C}_{2}^{2-}=14\).
So these are isoelectronics -
Question 39 of 81
39. Question
The uncertainty in momentum of an electron is \(1 \times 10^{-5} \mathrm{~kg} \mathrm{~m} / \mathrm{s}\). The uncertainty in its position will be \(\left(h=6.62 \times 10^{-34} \mathrm{~kg} \mathrm{~m}^{2} / \mathrm{s}\right)\) [AIPMT 1999]
CorrectIncorrectHint
(a) \(\Delta x \times \Delta p=\frac{n}{4 \pi}\)
(Heisenberg uncertainty principle)
\(
\Rightarrow \Delta x=\frac{6.62 \times 10^{-34}}{4 \times 3.14 \times 10^{-5}}=5.27 \times 10^{-30} \mathrm{~m}
\) -
Question 40 of 81
40. Question
Who modified Bohr’s theory by introducing elliptical orbits for electron path? [AIPMT 1999]
CorrectIncorrectHint
(d) Sommerfield modified Bohr’s theory considering that in addition to circular orbits electrons also move in elliptical orbits
-
Question 41 of 81
41. Question
The de Broglie wavelength of a particle with mass \(1 \mathrm{~g}\) and velocity \(100 \mathrm{~m} / \mathrm{s}\) is [AIPMT 1999]
CorrectIncorrectHint
\(
\begin{aligned}
&\text { (c) } \lambda=\frac{h}{m v}=\frac{6.63 \times 10^{-27} \mathrm{erg} \mathrm{sec}}{1 \mathrm{~g} \times 10^{4} \mathrm{~cm} / \mathrm{s}} \\
&=6.63 \times 10^{-31} \mathrm{~cm}=6.63 \times 10^{-33} \mathrm{~m}
\end{aligned}
\) -
Question 42 of 81
42. Question
The Bohr orbit radius for the hydrogen atom \((n=1)\) is approximately 0.530 Å. The radius for the first excited state \((n=2)\) orbit is (in Å) [AIPMT 1990]
CorrectIncorrectHint
(d) for \(n^{\text {th }}\) orbit of ‘ \(\mathrm{H}^{\prime}\) atom, \(r_{n}=n^{2} \times r_{1}\) \(\Rightarrow\) radius of \(2^{\text {nd }}\) Bohr’s orbit.
\(
r_{2}=4 \times r_{1}=4 \times 0.530=2.120 Å
\) -
Question 43 of 81
43. Question
The position of both, an electron and a helium atom is known within \(1.0 \mathrm{~nm}\). Further the nomentum of the electron is known within \(5.0 \times 10^{-26} \mathrm{~kg} \mathrm{~m} \mathrm{~s}^{-1}\). The minimum uncertainty n the measurement of the momentum of the helium atom is [AIPMT 1998]
CorrectIncorrectHint
(d) According to uncertainty principle the product of uncertainty in position and uncertainty in momentum is constant for a particle.
i.e., \(\Delta x \times \Delta p=\frac{h}{4 \pi}\)
As, \(\Delta x=1.0 \mathrm{~nm}\) for both electron and helium atom, so \(\Delta p\) is also same for both the particles.
Thus uncertainty in momentum of the helium atom is also \(5.0 \times 10^{-26} \mathrm{~kg} \mathrm{~m} \mathrm{~s}^{-1}\). -
Question 44 of 81
44. Question
The ion that is isoelectronic with \(\mathrm{CO}\) is [AIPMT1997]
CorrectIncorrectHint
(a) Since both \(\mathrm{CO}\) and \(\mathrm{CN}^{-}\)have 14 electrons, therefore these are isoelectronic (i.e. having same number of electrons).
-
Question 45 of 81
45. Question
What will be the longest wavelength line in Balmer series of spectrum? [AIPMT 1996]
CorrectIncorrectHint
(b) The longest wavelength means the lowest energy. We know that relation for wavelength
\(
\frac{1}{\lambda}=R_{H}\left(\frac{1}{n_{1}^{2}}-\frac{1}{n_{2}^{2}}\right)
\)here, \(n_1=2, n_2=3\)
\(R_{\mathrm{H}}\left(\right.\) Rydberg constant \(=109677 \mathrm{~cm}^{-1}\)
\(
\frac{1}{\lambda}=109677\left(\frac{1}{(2)^2}-\frac{1}{(3)^2}\right)=15233
\)
or, \(\lambda=\frac{1}{15233}=6.56 \times 10^{-5} \mathrm{~cm}=6.56 \times 10^{-7} \mathrm{~m}=656 \mathrm{~nm}\) -
Question 46 of 81
46. Question
In a Bohr’s model of an atom, when an electron jumps from \(n=1\) to \(n=3\), how much energy will be emitted or absorbed? [AIPMT 1996]
CorrectIncorrectHint
(d) Energy of an atom when \(n=1\)
\(
E_{1}=-\frac{1312}{(1)^{2}}=-1312 \mathrm{~kJ} \mathrm{~mol}^{-1}
\)
Similarly energy when \(n=3,\left(E_{3}\right)=-\frac{1312}{(3)^{2}}\) \(=-145.7 \mathrm{~kJ} \mathrm{~mol}^{-1}\)
The energy absorbed when an electron jumps from \(n=1\) to \(n=3\)
\(E_{3}-E_{1}=-145.7-(-1312)=1166.3 \mathrm{~kJ} \mathrm{~mol}^{-1}\)
\(
\begin{aligned}
&=\frac{1166.3}{6.023 \times 10^{23}}=193.6 \times 10^{-23} \mathrm{~kJ} \\
&=193.6 \times 10^{-20} \mathrm{~J}\left[1 \mathrm{Joule}=10^{7} \mathrm{ergs}\right] \\
&\Rightarrow 193.6 \times 10^{-13} \mathrm{ergs}=0.1936 \times 10^{-10} \mathrm{ergs}
\end{aligned}
\) -
Question 47 of 81
47. Question
Uncertainty in position of an electron (mass \(\left.=9.1 \times 10^{-28} \mathrm{~g}\right)\) moving with a velocity of \(3 \times 10^{4} \mathrm{~cm} / \mathrm{s}\) accurate upto \(0.001 \%\) will be (Use \(h /(4 \pi)\) in uncertainty expression where \(h=6.626 \times 10^{-27}\) erg second) [AIPMT 1995]
CorrectIncorrectHint
(c) Mass of an electron \((\mathrm{m})=9.1 \times 10^{-28} \mathrm{~g}\)
Velocity of electron \((v)=3 \times 10^{4} \mathrm{~cm} / \mathrm{s}\)
Accuracy \(=0.001 \%=\frac{0.001}{100}\) andPlanck’s constant \((h)=6.626 \times 10^{-27}\) erg-second
We know that actual velocity of the electron \((\Delta v)=3 \times 10^{4} \times \frac{0.001}{100}=0.3 \mathrm{~cm} / \mathrm{s}\) -
Question 48 of 81
48. Question
The radius of hydrogen atom in the ground state is 0.53 Å. The radius of \(\mathrm{Li}^{2+}\) ion (atomic number = 3) in a similar state is [AIPMT 1995]
CorrectIncorrectHint
(c) Due to ground state, state of hydrogen atom \((n)=1\)
Radius of hydrogen atom \((r)=0.53 Å\)
Atomic no. of \(\mathrm{Li}(Z)=3\)
Now, radius of Li \({ }^{2+}\) ion \(=r \times \frac{n^{2}}{7}=0.53 \times \frac{(1)^{2}}{3}=0.17 Å\) -
Question 49 of 81
49. Question
For which of the following sets of four quantum numbers, an electron will have the highest energy? [AIPMT 1994]
\(
\begin{array}{|l|l|l|l|l|}
\hline & {n} & {l} & {m} & {s}\\
\hline \text { (a) } & 3 & 2 & 1 & +1 / 2 \\
\hline \text { (b) } & 4 & 2 & -1 & +1 / 2 \\
\hline \text { (c) } & 4 & 1 & 0 & -1 / 2 \\
\hline \text { (d) } & 5 & 0 & 0 & -1 / 2 \\
\hline
\end{array}
\)CorrectIncorrectHint
(b) Energy of electron depends on the value of \((n+l)\). The subshell are \(3 d, 4 d, 4 p\) and \(5 s, 4 d\) has highest energy.
-
Question 50 of 81
50. Question
Which one of the following is not isoelectronic with \(\mathrm{O}^{2-}\) ? [AIPMT 1994]
CorrectIncorrectHint
(a) The number of electrons in \(\mathrm{O}^{2-}, \mathrm{N}^{3-}, \mathrm{~F^{-}}\) and \(\mathrm{Na}^{+}\)is 10 each. but number of electrons in \(\mathrm{Tl}^{+}\)is 80 .
-
Question 51 of 81
51. Question
Electronic configuration of calcium atom can be written as [AIPMT 1992]
CorrectIncorrectHint
(b) Atomic No. of \(\mathrm{Ca}=20\)
Electronic configuration of \(\mathrm{Ca}=[\mathrm{Ar}] 4 s^{2}\) -
Question 52 of 81
52. Question
The energy of an electron in the \(n^{\text {th }}\) Bohr orbit of hydrogen atom is [AIPMT 1992]
CorrectIncorrectHint
(c) Energy of an electron in \(n^{\text {th }}\) Bohr orbit of hydrogen atom \(=\frac{-13.6}{n^{2}} \mathrm{eV}\)
-
Question 53 of 81
53. Question
In a given atom no two electrons can have the same values for all the four quantum numbers. This is called [AIPMT 1991]
CorrectIncorrectHint
(d) This is a Pauli exclusion principle.
-
Question 54 of 81
54. Question
.For azimuthal quantum number \(l=3\), the maximum number of electrons will be [AIPMT 1991]
CorrectIncorrectHint
(d) \(l=3\) means \(f\)-subshell
Maximum no. of electrons in \(f\)-subshell \(=14\)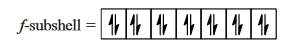
-
Question 55 of 81
55. Question
The order of filling of electrons in the orbitals of an atom will be [AIPMT 1991]
CorrectIncorrectHint
(b) As per Aufbau Principle.
The principle states : In the ground state of the atoms, the orbitals are filled in order of their increasing energies.
-
Question 56 of 81
56. Question
The electronic configuration of \(\mathrm{Cu}\) (atomic number 29) is [AIPMT 1991]
CorrectIncorrectHint
(b) Electronic configuration of \(\mathrm{Cu}\)
\(
=1 s^2 2 s^2 2 p^6 3 s^2 3 p^6 3 d^{10} 4 s^1
\) -
Question 57 of 81
57. Question
The total number of electrons that can b accommodated in all the orbitals having principal quantum number 2 and azimuthal quantum number 1 are [AIPMT 1990]
CorrectIncorrectHint
(c) \(n=2, l=1\)
It means \(2 p\)-orbitals
Total no. of electrons that can be accomodated in \(2 p\) orbitals \(=6\) -
Question 58 of 81
58. Question
An ion has 18 electrons in the outermost shell, it is [AIPMT 1990]
CorrectIncorrectHint
(a) Electronic configuration of \(\mathrm{Cu}^{+}=[\mathrm{Ar}] 3 d^{10}\)
-
Question 59 of 81
59. Question
Which of the following statements do not form a part of Bohr’s model of hydrogen atom? [AIPMT 1989]
CorrectIncorrectHint
(d) It is uncertainty principle and not Bohr’s postulate.
-
Question 60 of 81
60. Question
Number of unpaired electrons in \(\mathrm{N}^{2+}\) is/are [AIPMT 1989]
CorrectIncorrectHint
(c) \(\mathrm{N}^{2+}=1 s^{2} 2 s^{2} 2 p_{x}^{1}\)
No. of unpaired electrons \(=1\) -
Question 61 of 81
61. Question
The maximum number of electrons in a subshell is given by the expression [AIPMT 1989]
CorrectIncorrectHint
(b) No. of orbitals in a subshell \(=2 l+1\)
No. of electrons \(=2(2 l+1)=4 l+2\) -
Question 62 of 81
62. Question
The spectrum of He is expected to be similar to that [AIPMT 1988]
CorrectIncorrectHint
(b) Both \(\mathrm{He}\) and \(\mathrm{Li}^{+}\) contain 2 electrons each.
-
Question 63 of 81
63. Question
The number of spherical nodes in \(3 p\) orbitals are/is [AIPMT 1988]
CorrectIncorrectHint
(a) No. of radial nodes in \(3 p\)-orbital \(=n-l-1\)
\(
=3-1-1=1
\) -
Question 64 of 81
64. Question
If \(r\) is the radius of the first orbit, the radius of \(n^{\text {th }}\) orbit of H-atom is given by [AIPMT 1988]
CorrectIncorrectHint
(a) Radius of \(n^{\mathrm{th}}\) orbit of H-atom \(=r_{0} n^{2}\) where \(r_{0}=\) radius of the first orbit
-
Question 65 of 81
65. Question
Which of the following series of transitions in the spectrum of hydrogen atom falls in visible region? [ NEET2019]
CorrectIncorrectHint
-There are many waves that exist in this universe with different wavelengths and different frequencies. The waves with higher frequency have less wavelength and vice versa.
-Based on this, the spectrum is classified mainly as cosmic, gamma, X-ray, UV, visible light, infrared, microwave and radiowave region. The wavelength of visible light ranges from \(380 \mathrm{~nm}\) to \(780 \mathrm{~nm}\). Only the wavelengths of these ranges can be seen by the human eye.
-Hydrogen atom consists of 1 proton and 1 electron. The energy state of the orbitals of the atom was given by Bohr in his atomic model and the spectral emission is based on Schrodinger’s equation.
-Electrons jump from higher energy level to lower energy level releasing energy in form of spectral emissions. The spectral lines are grouped together to form the spectral series. Hydrogen shows 4 such series named Lyman, Balmer, Brackett and Paschen.
-The energy differences and the wavelengths of the emitted photons is related by Rydberg formula as
\(
\frac{1}{\lambda}=Z^2 R\left(\frac{1}{n_1{ }^2}-\frac{1}{n_2^2}\right)
\)
Where \(\mathrm{Z}\) is the atomic number, \(\mathrm{n}\) is the principal quantum number and the numbers 1 and 2 represent the lower and higher energy levels and \(\mathrm{R}\) is the Rydberg’s constant.
-Different series are obtained by changing the values of \(n\) as 1,2,3 and 4 for hydrogen. The value of \(\mathrm{R}\) for hydrogen is \(1.09677 \times 10^7 \mathrm{~m}^{-1}\).
-Putting the values of \(R\) and \(Z\), we can find the values of the wavelengths for different values of the energy levels and by doing so, it is observed that the range of wavelengths for visible light is obtained in the Balmer series only.
-The range for Lyman series starts from \(121 \mathrm{~nm}\) and decreases further, Balmer series starts from 656nm, Paschen series starts from \(1875 \mathrm{~nm}\) and Brackett series starts from \(4051 \mathrm{~nm}\).
Therefore we see that the Balmer series falls under the visible region in the hydrogen spectrum and the correct option is b. -
Question 66 of 81
66. Question
In hydrogen atom, the de-Broglie wavelength of an electron in the second Bohr orbit is [Given that Bohr radius, \(a_{0}=52.9 \mathrm{pm}\) ] [NEET Odisha 2019]
CorrectIncorrectHint
Bohr radius, \(a_0=52.9 p m n=2, r_n=n^2 a_0=(2)^2 a_0=4 \times 52.9 p m=211.6 p m\)
The angular momentum of an electron in a given stationary state can be expressed as in equation, \(m v r=n \cdot \frac{h}{2 \pi}=2 \times \frac{h}{2 \pi}=\frac{h}{\pi} \dots(i)\)
de-Broglie equation \(mvr\) \(\pi=h \lambda=\frac{h}{m v} ; {\lambda} m v=h \dots(ii)\)From equations, (i) and (ii), we get \(\lambda=\pi r\)
Putting the value of \(r, \lambda=211.6 \pi \mathrm{pm}\) -
Question 67 of 81
67. Question
Orbital having 3 angular nodes and 3 total nodes is [NEET Odisha 2019]
CorrectIncorrectHint
(d)
Orbital having angular node \((\ell)=3\)
Total node \(=\) Radial node + angular node
\(
\begin{array}{l}
=n-\ell-1+\ell \\
3=n-1 \\
n=4
\end{array}
\)Subshell ” \(n \ell\) ” \(=4 f\)
-
Question 68 of 81
68. Question
\(4 d, 5 p, 5 f\) and \(6 p\) orbitals are arranged in the order of decreasing energy. The correct option is: [ NEET 2019]
CorrectIncorrectHint
(a)
The \((n+l)\) values are given below,
\(
\begin{array}{l}
4 d=4+2=6 \\
5 p=5+1=6 \\
5 f=5+3=8 \\
6 p=6+1=7
\end{array}
\)The correct order of energy would be:
\(
5 f>6 p>5 p>4 d
\) -
Question 69 of 81
69. Question
A particular station of All India Radio, New Delhi, broadcasts on a frequency of 1,368 \(\mathrm{kHz}\) (kilohertz). The wavelength of the electromagnetic radiation emitted by the transmitter is [Speed of light, \(c=3.0 \times 10^{8} \mathrm{~ms}^{-1}\) ] [NEET 2021]
CorrectIncorrectHint
(a)
\(
\begin{array}{l}
\lambda=\frac{\mathrm{c}}{\mathrm{v}}\\
\lambda=\frac{3 \times 10^8}{1368 \times 10^3}=219.298 \mathrm{~m}=219.3 \mathrm{~m}
\end{array}
\) -
Question 70 of 81
70. Question
Incorrect set of quantum numbers from the following is: [NEET 2023 Manipur]
CorrectIncorrectHint
(b)
\(
\mathrm{n}=5, \ell=2, \mathrm{~m_l}=-2,-1,+1,+2, \mathrm{~m}_{\mathrm{s}}=+\frac{1}{2}
\) -
Question 71 of 81
71. Question
Question: Given below are two statements:
Statement I: The value of wave function, \(\psi\) depends upon the coordinates of the electron in the atom.
Statement II : The probability of finding an electron at a point within an atom is proportional to the orbital wave function.
In the light of the above statements, choose the correct answer from the options given below: [NEET 2023 Manipur]CorrectIncorrectHint
(a) Statement I is true and Statement II is false (From NCERT)
-
Question 72 of 81
72. Question
Select the correct Statements from the following:
A. Atoms of all elements are composed of two fundamental particles.
B. The mass of the electron is \(9.10939 \times 10^{-31} \mathrm{~kg}\).
C. All the isotopes of a given elements show same chemical properties.
D. Protons and electrons are collectively known as nucleons.
E. Dalton’s atomic theory, regarded the atom as an ultimate particle of matter.
Choose the correct answer from the options given below. [NEET 2023]CorrectIncorrectHint
(c) Statements B, C & E are correct.
(B) Mass of the electron is \(9.10939 \times 10^{-31} \mathrm{~kg}\)
(C) All the isotopes of given elements show same chemical properties.
(E) Dalton’s atomic theory, regarded the atom as an ultimate particle of matter. -
Question 73 of 81
73. Question
The relation between \(\mathrm{n}_{\mathrm{m}},\left(\mathrm{n}_{\mathrm{m}}=\right.\) the number of permissible values of magnetic quantum number \((\mathrm{m})\) ) for a given value of azimuthal quantum number \((l)\), is [NEET 2023]
CorrectIncorrectHint
(d) Number of permissible values of magnetic quantum number for a given value of azimuthal quantum \((l)\)
\(
\begin{aligned}
& \Rightarrow \mathrm{n}_{\mathrm{m}}=2 \ell+1 \\
& \Rightarrow \ell=\frac{\mathrm{n}_{\mathrm{m}}-1}{2}
\end{aligned}
\) -
Question 74 of 81
74. Question
Match List-I with List-II
\(
\begin{array}{|l|l|}
\hline \begin{array}{c}
\text { List-I } \\
\text { (quantum number) }
\end{array} & \begin{array}{c}
\text { List-II } \\
\text { (orbital) }
\end{array} \\
\hline (a) n=2, l=1 & (i) 2 \mathrm{~s} \\
\hline (b) \mathrm{n}=3, l=2 & (ii) 3 \mathrm{~s} \\
\hline (c) \mathrm{n}=3, l=0 & (iii) 2 \mathrm{p} \\
\hline (d) \mathrm{n}=2, l=0 & (iv) 3 \mathrm{~d} \\
\hline
\end{array}
\) [NEET 2022 Phase 2]CorrectIncorrectHint
(a)
\(
\begin{array}{|c|c|c|}
\hline n & l & \text { Subshell notation } \\
\hline 2 & 0 & 2 s \\
\hline 2 & 1 & 2 p \\
\hline 3 & 0 & 3 s \\
\hline 3 & 1 & 3 p \\
\hline 3 & 2 & 3 d \\
\hline
\end{array}
\) -
Question 75 of 81
75. Question
When electromagnetic radiation of wavelength \(300 \mathrm{~nm}\) falls on the surface of a metal, electrons are emitted with the kinetic energy of \(1.68 \times 10^5 \mathrm{~J} \mathrm{~mol}^{-1}\). What is the minimum energy needed to remove an electron from the metal?
\(
\left(\mathrm{h}=6.626 \times 10^{-34} \mathrm{Js}, \mathrm{c}=3 \times 10^8 \mathrm{~ms}^{-1}, \mathrm{~N}_{\mathrm{A}}=6.022 \times 10^{23} \mathrm{~mol}^{-1}\right)
\) [NEET 2022 Phase 2]CorrectIncorrectHint
(a) Energy of one photon \(=\frac{h c}{\lambda}(\lambda=300 \mathrm{~nm})\)
For one mole photons, \(E=\frac{h c}{\lambda} \times N_A\)
\(
\begin{aligned}
& E=\frac{6.626 \times 10^{-34} \times 3 \times 10^8 \times 6.023 \times 10^{23}}{300 \times 10^{-9}} \\
& E=3.99 \times 10^5 \mathrm{~J} \mathrm{~mol}^{-1}
\end{aligned}
\)Kinetic energy \(=1.68 \times 10^5 \mathrm{~J} \mathrm{~mol}^{-1}\)
\(
\begin{aligned}
& W_0=E-K . E . \\
& =3.99 \times 10^5-1.68 \times 10^5 \\
& =2.31 \times 10^5 \mathrm{~J} \mathrm{~mol}^{-1}
\end{aligned}
\) -
Question 76 of 81
76. Question
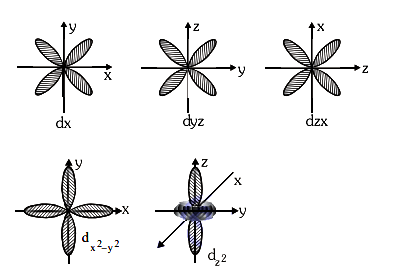
Identify the incorrect statement from the following. [NEET 2022 Phase 1]
CorrectIncorrectHint
(d) The shapes of \(d_{x y}, d_{y z}\) and \(d\) orbitals are similar to each other; and \(d_{x^2-y^2}\) and \(d_z^2\) are similar to each other.
-
Question 77 of 81
77. Question
If radius of second Bohr orbit of the \(\mathrm{He}^{+}\)ion is \(105.8 \mathrm{~pm}\), what is the radius of third Bohr orbit of \(\mathrm{Li}^{2+}\) ion? [NEET 2022 Phase 1]
CorrectIncorrectHint
(a)
\(
\begin{aligned}
&r_n \propto \frac{n^2}{Z}\\
&\begin{aligned}
& \frac{r_3\left(L i^{2+}\right)}{r_2\left(H e^{+}\right)}=\frac{\left(n_3\right)^2}{Z\left(L i^{2+}\right)} \times \frac{Z\left(H e^{+}\right)}{\left(n_2\right)^2} \\
& \frac{r_3\left(L i^{2+}\right)}{105.8}=\frac{(3)^2}{3} \times \frac{2}{(2)^2} \\
& =105.8 \times \frac{3}{2} \\
& r_3\left(L i^{2+}\right)=158.7 \mathrm{~pm}
\end{aligned}
\end{aligned}
\) -
Question 78 of 81
78. Question
The quantum numbers of four electrons are given below :
I. \(n=4 ; l=2 ; m_l=-2 ; s=-\frac{1}{2}\)
II. \(n=3 ; l=2 ; m_l=1 ; s=+\frac{1}{2}\)
III. \(n=4 ; l=1 ; m_l=0 ; s=+\frac{1}{2}\)
IV. \(n=3 ; l=1 ; m_l=-1 ; s=+\frac{1}{2}\)
The correct decrease order of energy of these electrons is [NEET 2024 (Re-Examination)]CorrectIncorrectHint
(b)
(I) \(n=4, l=2, m_l=-2, s=-\frac{1}{2}\); represents \(4 d(n+l=6)\)
(II) \(n=3, l=2, m_l=1, s=+\frac{1}{2}\); represents \(3 d(n+l=5)\)
(III) \(n=4, l=1, m_l=0, s=+\frac{1}{2}\); represents \(4 p(n+l=5)\)
(IV) \(n=3, l=1, m_l=-1, s=+\frac{1}{2}\); represents \(3 p(n+l=4)\)Order of energy depends on the \((n+l)\), greater is the \((n+l)\) value greater is the energy, if \((n+l)\) is same, then it depends on \(n\); if ‘ \(n\) ‘ is more, energy is more.
Step-1: According to \(( n + l )\)
Energy \(=\) (I) \(>\) (II) \(=\) (III) \(>\) (IV)
Step-2: If \(n \uparrow\), then energy increases
Energy \(=\) (I) \(>\) (III) \(>\) (II) \(>\) (IV) -
Question 79 of 81
79. Question
Given below are two statements:
Statement I: The Balmer spectral line for H atom with lowest energy is located at
\(
\begin{aligned}
& \frac{5}{36} R_H cm^{-1} \\
& \left( R _{ H }=\text { Rydberg constant }\right)
\end{aligned}
\)
Statement II: When the temperature of blackbody increases, the maxima of the curve (intensity and wavelength) shifts to shorter wavelength.
In the light of the above statements, choose the correct answer from the options given below: [NEET 2024 (Re-Examination)]CorrectIncorrectHint
(c)
\(
\begin{aligned}
&\text { For Balmer series }\\
&\begin{aligned}
n _1 & =2 \\
n _2 & =3 \\
\bar{v} & =\frac{1}{\lambda}=R_H\left[\frac{1}{n_1^2}-\frac{1}{n_2^2}\right] \\
\bar{v} & =R_H\left[\frac{1}{4}-\frac{1}{9}\right] \\
\bar{v} & =\frac{5 R_H}{36} cm^{-1}
\end{aligned}
\end{aligned}
\)
-
Question 80 of 81
80. Question
The energy of an electron in the ground state \(( n =1)\) for \(He ^{+}\)ion is \(-x J\), then that for an electron in \(( n =2)\) state for \(Be ^{3+}\) ion in \(J\) is [NEET 2024]
CorrectIncorrectHint
(a)
\(
E_n=-R_H\left(\frac{z^2}{n^2}\right) J
\)
For \(He ^{+}(n=1)\),
\(
\begin{aligned}
& E_n=-x=-R_H\left(\frac{2^2}{1^2}\right)=-4 R_H \\
& \therefore R_H=\frac{x}{4}
\end{aligned}
\)
For \(B e^{3+}(n=2)\),
\(
\begin{aligned}
& E_n=-R_H\left(\frac{Z^2}{n^2}\right) J \\
& =-\frac{x}{4} \times\left(\frac{4 \times 4}{2 \times 2}\right)=-x J
\end{aligned}
\) -
Question 81 of 81
81. Question
Match List I with List II.
\(
\begin{array}{|c|c|c|c|}
\hline & \text { List-I } & & \text { List-II } \\
& \text { (Quantum Number) } & & \text { (Information provided) } \\
\hline \text { (A) } & m_l & \text { (I) } & \text { Shape of orbital } \\
\hline \text { (B) } & m_s & \text { (II) } & \text { Size of orbital } \\
\hline \text { (C) } & { l } & \text { (III) } & \text { Orientation of orbital } \\
\hline \text { (D) } & n & \text { (IV) } & \text { Orientation of spin of electron } \\
\hline
\end{array}
\)CorrectIncorrectHint
(b) To match List I (Quantum Numbers) with List II (Information provided), we need to understand what each quantum number represents:
1. Principal Quantum Number \((n)\) : This quantum number determines the size and energy level of the orbital. An increase in \(n\) implies a higher energy level and a larger orbital size.
2. Azimuthal Quantum Number ( \(\ell\) ): (not directly listed but related to \(m_l\) because \(m _l\) depends on \(\ell\) ): This quantum number defines the shape of the orbital. Different values of \(\ell\) correspond to different shapes (s, p, d, f, etc.).
3. Magnetic Quantum Number \(\left(m_l\right)\) : This quantum number describes the orientation of the orbital in space. It can take values from \(-\ell\) to \(\ell\), where each value corresponds to a specific orientation of the orbital.
4. Spin Quantum Number \(\left( m _{ s }\right)\) : This quantum number specifies the orientation of the spin of the electron. The two possible values are \(+\frac{1}{2}\) and \(-\frac{1}{2}\), representing the two possible spin orientations (up or down).
Matching the given options:
A. \(m _l\) – should be matched with “Orientation of orbital” (III).
B. \(m _{ s }\) – should be matched with “Orientation of spin of electron” (IV).
C. I – This is likely intended to be \(\ell\), and would be matched with “Shape of orbital” (I).
D. \(n\) – relates to “Size of orbital” (II), as it affects the distance from the nucleus along with the energy level.Using the understanding above, we can identify the correct matches:
A-III (Orientation of orbital)
B-IV (Orientation of spin of electron)
C-I (Shape of orbital)
D-II (Size of orbital)
Therefore, the correct answer is:Option B: A-III, B-IV, C-I, D-II