NCERT Exemplar MCQs
Quiz Summary
0 of 42 Questions completed
Questions:
Information
You have already completed the quiz before. Hence you can not start it again.
Quiz is loading…
You must sign in or sign up to start the quiz.
You must first complete the following:
Results
Results
0 of 42 Questions answered correctly
Your time:
Time has elapsed
You have reached 0 of 0 point(s), (0)
Earned Point(s): 0 of 0, (0)
0 Essay(s) Pending (Possible Point(s): 0)
Categories
- Not categorized 0%
- 1
- 2
- 3
- 4
- 5
- 6
- 7
- 8
- 9
- 10
- 11
- 12
- 13
- 14
- 15
- 16
- 17
- 18
- 19
- 20
- 21
- 22
- 23
- 24
- 25
- 26
- 27
- 28
- 29
- 30
- 31
- 32
- 33
- 34
- 35
- 36
- 37
- 38
- 39
- 40
- 41
- 42
- Current
- Review
- Answered
- Correct
- Incorrect
-
Question 1 of 42
1. Question
Isostructural species are those which have the same shape and hybridisation. Among the given species identify the isostructural pairs.
CorrectIncorrectHint
\(
\text { (b) } \mathrm{NF}_3 \text { is pyramidal whereas } \mathrm{BF}_3 \text { is planar triangular. }
\)
\(
\mathrm{BF}_4^{-} \text {and } \mathrm{NH}_4^{+} \text { ions both are tetrahedral. }
\)
\(\mathrm{BCl}_3\) is triangular planar whereas \(\mathrm{BrCl}_3\) is pyramidal.
\(\mathrm{NH}_3\) is pyramidal whereas \(\mathrm{NO}_3^{-}\) is triangular planar. -
Question 2 of 42
2. Question
Polarity in a molecule and hence the dipole moment depends primarily on the electronegativity of the constituent atoms and the shape of a molecule. Which of the following has the highest dipole moment?
CorrectIncorrectHint
(c) \(\mathrm{H}_2 \mathrm{O}\) will have the highest dipole moment due to the maximum difference in electronegativity of \(\mathrm{H}\) and \(\mathrm{O}\) atoms.
Explanation: \(\mathrm{H}_2 \mathrm{O}\) has the highest dipole moment because it is highly electronegative and has two lone pair on it.
-
Question 3 of 42
3. Question
The types of hybrid orbitals of nitrogen in \(\mathrm{NO}_2^{+}, \mathrm{NO}_3^{-}\)and \(\mathrm{NH}_4^{+}\)respectively are expected to be
CorrectIncorrectHint
(b) The number of orbitals involved in hybridization can be determined by the application of formula:
\(
H=\frac{1}{2}[V+M-C+A]
\)
where \(\mathrm{H}=\) number of orbitals involved in hybridization
\(V=\) valence electrons of central atom
\(\mathrm{M}=\) number of monovalent atoms linked with central atom
\(\mathrm{C}=\) charge on the cation
\(A=\) charge on the anion
\(
\begin{aligned}
& \mathrm{NO}_2^{+}: H=\frac{1}{2}[5+0-1+0]=2 \text { or } s p \\
& \mathrm{NO}_3^{-}: H=\frac{1}{2}[5+0-0+1]=3 \text { or } s p^2 \\
& \mathrm{NH}_4^{+}: H=\frac{1}{2}[5+4-1+0]=4 \text { or } s p^3
\end{aligned}
\) -
Question 4 of 42
4. Question
Hydrogen bonds are formed in many compounds e.g., \(\mathrm{H}_2 \mathrm{O}, \mathrm{HF}, \mathrm{NH}_3\). The boiling point of such compounds depends to a large extent on the strength of hydrogen bond and the number of hydrogen bonds. The correct decreasing order of the boiling points of above compounds is:
CorrectIncorrectHint
HINT: Boiling point depends on the extent of hydrogen bonding.
Explanation:
(b) Strength of \(\mathrm{H}\)-bonding depends on the electronegativity of the atom which follows the order: \(\mathrm{F}>O>N\).
Strength of \(\mathrm{H}\)-bond is in the order:
\(
\mathrm{H} {\ldots} \ldots . \mathrm{F}>\mathrm{H} \ldots \ldots \mathrm{O}>\mathrm{H} {\ldots} \ldots \ldots \mathrm{N}
\)
But each \(\mathrm{H}_2 \mathrm{O}\) molecule is linked to 4 other \(\mathrm{H}_2 \mathrm{O}\) molecules through \(\mathrm{H}\)-bonds whereas each \(\mathrm{HF}\) molecule is linked only to two other \(\mathrm{HF}\) molecules.
Hence, the correct decreasing order of the boiling points is \(\mathrm{H}_2 \mathrm{O}>\mathrm{HF}>\mathrm{NH}_3\). -
Question 5 of 42
5. Question
In \(\mathrm{PO}_4^{3-}\) ion the formal charge on the oxygen atom of \(\mathrm{P}-\mathrm{O}\) bond is
CorrectIncorrectHint
(b) Formal charge of the atom in the molecule or ion = (Number of valence electrons in free atom) (Number of lone pair electrons \(+1 / 2\) Number of bonding electrons)
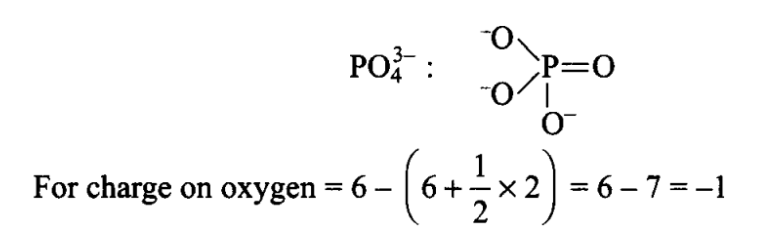
-
Question 6 of 42
6. Question
In \(\mathrm{NO}_3^{-}\)ion, the number of bond pairs and lone pairs of electrons on nitrogen atom are
CorrectIncorrectHint
(d) In \(\mathrm{N}\)-atom, number of valence electrons \(=5\)
Due to the presence of one negative charge, number of valence electrons \(=5+1=6\). One O -atom forms two bonds ( \(=\) bond) and two O -atom are shared with two electrons of \(\mathrm{N}\)-atom.
Thus, 3 O-atoms are shared with 8 electrons of \(\mathrm{N}\)-atom.
Number of bond pairs (or shared pairs) \(=4\)
Number of lone pairs \(=0\)
-
Question 7 of 42
7. Question
Which of the following species has tetrahedral geometry?
CorrectIncorrectHint
(a) \(\mathrm{BH}_4^{-}=\)tetrahedral, \(\mathrm{NH}_2^{-}=\mathrm{V}\)-shape, \(\mathrm{CO}_3^{2-}=\) triangular planar, \(\mathrm{H}_3 \mathrm{O}^{+}=\) pyramidal.
-
Question 8 of 42
8. Question
Number of \(\pi\) bonds and \(\sigma\) bonds in the following structure is-
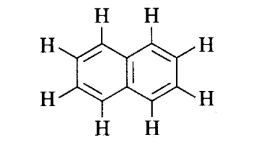 CorrectIncorrect
CorrectIncorrectHint
(c) HINT: \(\pi\) bond is double bond and \(\sigma\) bond is single bond. Explanation:
The given compound will have the correct structure as
\(
\text { There are } 5 \mathrm{C}=\mathrm{C} \pi \text { bonds }
\)
\(
8 \mathrm{C}-\mathrm{H}+11 \mathrm{C}-\mathrm{C} \sigma \text {-bonds, i.e., } 19 \sigma \text {-bonds are present in the above molecule. }
\) -
Question 9 of 42
9. Question
Which molecule/ion out of the following does not contain unpaired electrons?
CorrectIncorrectHint
(c)
\(
\begin{aligned}
& \mathrm{N}_2^{+}=\mathrm{KK} \sigma 2 s^2 \sigma^* 2 s^2 \pi 2 p_x^2=\pi 2 p_y^2 \sigma 2 p_z^1 \\
& \mathrm{O}_2=\mathrm{KK} \sigma 2 s^2 \sigma^* 2 s^2 \sigma 2 p_z^2 \pi 2 p_x^2=\pi 2 p_y^2 \pi^* 2 p_x^1=\pi^* 2 p_y^1 \\
& \mathrm{O}_2^{2-}=\mathrm{KK} \sigma 2 s^2 \sigma^* 2 s^2 \sigma 2 p_z^2 \pi 2 p_x^2=\pi 2 p_y^2 \pi^* 2 p_x^2=\pi^* 2 p_y^2 \\
& \mathrm{~B}_2=\mathrm{KK} \sigma 2 s^2 \sigma^* 2 s^2 \pi 2 p_x^1=\pi 2 p_y^1
\end{aligned}
\)
\(
\text { Thus, } \mathrm{O}_2^{2-} \text { has no unpaired electrons. }
\) -
Question 10 of 42
10. Question
In which of the following molecule/ion all the bonds are not equal?
CorrectIncorrectHint
(c) \(\mathrm{C}_2 \mathrm{H}_4\) has one double bond and four single bonds. Bond length of double bond \((\mathrm{C}=\mathrm{C})\) is smaller than single bond \((\mathrm{C}-\mathrm{H})\).
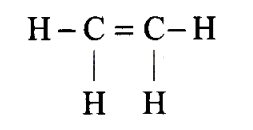
-
Question 11 of 42
11. Question
In which of the following substances will hydrogen bond be strongest?
CorrectIncorrectHint
(b) \(\mathrm{HCl} \mathrm{HI}\) and \(\mathrm{H}_2 \mathrm{~S}\) do not from \(\mathrm{H}\)-bonds. Only \(\mathrm{H}_2 \mathrm{O}\) forms hydrogen bonds. One \(\mathrm{H}_2 \mathrm{O}\) molecule forms four \(\mathrm{H}\)-bonding.
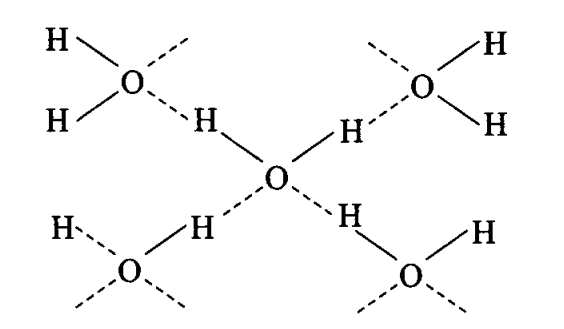
-
Question 12 of 42
12. Question
If the electronic configuration of an element is \(1 s^2 2 s^2 2 p^6 3 s^2 3 p^6 3 d^2 4 s^2\), the four electrons involved in chemical bond formation will be
CorrectIncorrectHint
(d) In transition elements ( \(n-1)\) d and \(ns\) orbitals take part in bond formation.
Explanation:
STEP 1:
The given electronic configuration shows that an element is a vanadium \((Z=22)\). It belongs to the d-block of the periodic table. In transition elements i.e., \(d\) block elements, electrons of \(n s\) and ( \(n-1) d\) subshell take part in bond formation.
STEP 2:
Hence, \(3 \mathrm{~d}\) and 4 s electrons participate in bond formation. Therefore, option 4 is the correct answer. -
Question 13 of 42
13. Question
Which of the following angle corresponds to \(s p^2\) hybridisation?
The electronic configurations of three elements, A, B and C are given below. Answer the questions 14 to 17 on the basis of these configurations.
\(\begin{array}{llllll}\text { A } & 1 s^2 & 2 s^2 & 2 p^6 & & \\ \text { B } & 1 s^2 & 2 s^2 & 2 p^6 & 3 s^2 & 3 p^3 \\ \text { C } & 1 s^2 & 2 s^2 & 2 p^6 & 3 s^2 & 3 p^5\end{array}\)CorrectIncorrectHint
(b) \(\mathrm{sp}^2\) hybridisation gives three \(\mathrm{sp}^2\) hybrid orbitals which are planar triangular forming an angle of \(120^{\circ}\) with each other. The electronic configurations of three elements \(\mathrm{A}, \mathrm{B}\) and \(\mathrm{C}\) are given below. Answer the questions from 14 to 17 on the basis of these configurations.
A \(1s^2 2 \mathrm{~s}^2 2 \mathrm{p}^6\)
B \(1s^2 2 s^2 2 p^6 3 s^2 3 p^3\)
C \(1s^2 2 s^2 2 p^6 3 s^2 3 p^5\) -
Question 14 of 42
14. Question
Stable form of A may be represented by the formula:
CorrectIncorrectHint
(a) The given electronic configuration shows that A represents noble gas because the octet is complete. \(\mathrm{A}\) is neon which has 10 atomic number.
-
Question 15 of 42
15. Question
Stable form of \(\mathbf{C}\) may be represented by the formula:
CorrectIncorrectHint
\(
\text { (b) The electronic configuration of } \mathrm{C} \text { represent chlorine. Its stable form is dichlorine }\left(\mathrm{Cl}_2\right) \text {, i.e., } \mathrm{C}_2 \text {. }
\) -
Question 16 of 42
16. Question
The molecular formula of the compound formed from \(\mathrm{B}\) and \(\mathrm{C}\) will be
CorrectIncorrectHint
\(
\text { (d) } B \text { represent } P \text { and } C \text { represents } \mathrm{C l} \text {. The stable compound is } P C l_3 \text { i.e., } \mathrm{BC}_3 \text {. }
\) -
Question 17 of 42
17. Question
The bond between \(\mathrm{B}\) and \(\mathrm{C}\) will be
CorrectIncorrectHint
\(
\text { (b) Both } \mathrm{B} \text { and } \mathrm{C} \text { are non-metals and therefore, bond formed between them will be covalent. }
\) -
Question 18 of 42
18. Question
Which of the following order of energies of molecular orbitals of \(\mathrm{N}_2\) is correct?
CorrectIncorrectHint
(a) Hint: Energy of \(\sigma 2 \mathrm{p}_{\mathrm{z}}\) molecular orbital is more than \(\pi^* 2 \mathrm{p}_{\mathrm{x}} \approx \pi^* 2 \mathrm{p}_{\mathrm{y}}\) molecular orbital.
Explanation: Molecules like \(\mathrm{B}_2, \mathrm{C}_2 \& \mathrm{~N}_2\) have 1 to 3 electrons in p orbital energy of \(\sigma 2 \mathrm{p}\) molecular orbital are greater than that of \(\pi 2 p_x\) and \(\pi 2 p_y\) molecular orbitals.
Step 1: The MOT diagram for \(\mathrm{N}_2\) is as follows: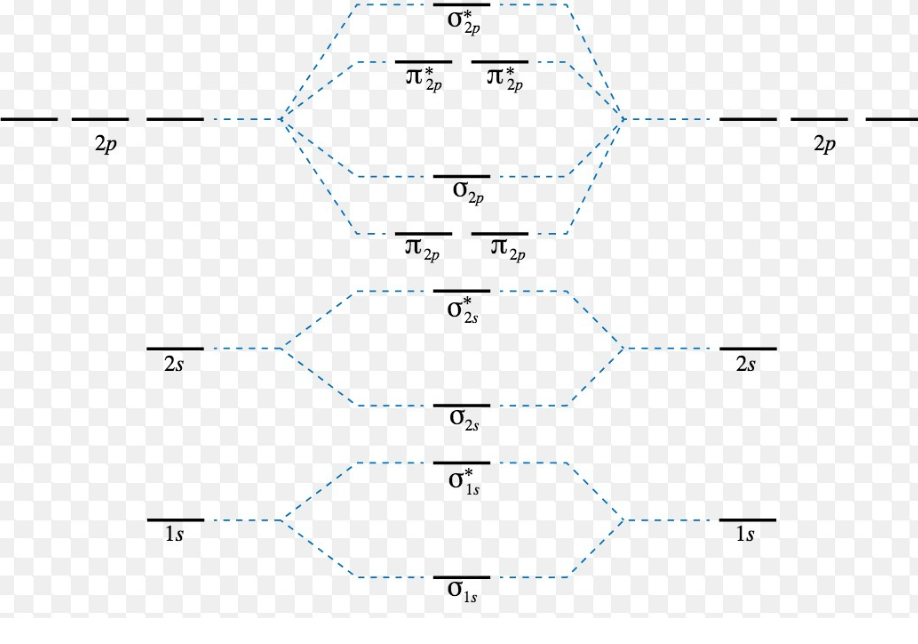
Step-2:
So According to the above molecular orbital diagram The order of energies of molecular orbitals of \(\mathrm{N}_2\) is as follows: \(\left(\pi 2 \mathrm{p}_{\mathrm{y}}\right)<\left(\sigma 2 \mathrm{p}_{\mathrm{z}}\right)<\left(\pi * 2 \mathrm{p}_{\mathrm{x}}\right) \approx\left(\pi * 2 \mathrm{p}_{\mathrm{y}}\right)\) -
Question 19 of 42
19. Question
Which of the following statement is not correct from the view point of molecular orbital theory?
CorrectIncorrectHint
(a) \(\mathrm{Be}_2(4+4=8)=\sigma 1 s^2, \sigma^* 1 s^2, \sigma 1 s^2, \sigma^* 2 s^2\)
Bond order \((\mathrm{BO})=\frac{1}{2}\) [Number of bonding electron \(\left(\mathrm{N}_{\mathrm{b}}\right)-\) Number of antibonding electrons \(\mathrm{N}_{\mathrm{a}}\) ]
\(
=\frac{4-4}{2}=0
\)
Here, bond order of \(\mathrm{He}_2\) is zero. Thus, it does not exist.
(b) \(\mathrm{He}_2(2+2=4)=\sigma 1 s^2, \sigma^* 1 s^2\)
\(
\mathrm{BO}=\frac{2-2}{2}=0
\)
Here, bond order of \(\mathrm{He}_2\) is zero. Thus, it does not exist.
\(
\begin{aligned}
& \mathrm{He}_2^{+}(2+2-1=3)=\sigma 1 s^2, \sigma^* 1 s^1 \\
& \mathrm{BO}=\frac{2-1}{2}=0.5
\end{aligned}
\)
Since, the bond order is not zero, this molecule is expected to exist.
(c)
\(
\begin{aligned}
\mathrm{N}_2(7+7 & =14)=\sigma 1 s^2, \sigma^* 1 s^2, \sigma 2 s^2, \sigma^* 2 s^2, \pi 2 p_x^2 \approx \pi 2 p_y^2, \sigma 2 p_z^2 \\
\mathrm{BO} & =\frac{10-4}{2}=3
\end{aligned}
\)
Thus, dinitrogen \(\left(\mathrm{N}_2\right)\) molecule contains triple bond and no other molecule of second period have more than double bond. Hence, bond strength of \(\mathrm{N}_2\) is maximum amongst the homonuclear diatomic molecules belonging to the second period.
(d) It is incorrect. The correct order of energies of molecular orbitals in \(\mathrm{N}_2\) molecule is
\(
\sigma 2 s<\sigma^* 2 s<\left(\pi 2 p_x \approx \pi 2 p_y\right)<\sigma 2 p_z<\pi^* 2 p_x \approx \pi^* 2 p_y<\sigma^* 2 p_z
\) -
Question 20 of 42
20. Question
Which of the following options represents the correct bond order :
CorrectIncorrectHint
(b)
\(
\begin{aligned}
& \mathrm{O}_2: \mathrm{KK} \sigma 2 s^2 \sigma^* 2 s^2 \sigma 2 p_z{ }^2 \pi 2 p_x{ }^2=\pi 2 p_y{ }^2 \pi^* 2 p_x{ }^1=\pi^* 2 p_y{ }^1 \\
& \mathrm{O}_2^{-}: \mathrm{KK} \sigma 2 s^2 \sigma^* 2 s^2 \sigma 2 p_z{ }^2 \pi 2 p_x{ }^2=\pi 2 p_y{ }^2 \pi^* 2 p_x{ }^2=\pi^* 2 p_y{ }^1 \\
& \mathrm{O}_2^{+}: \mathrm{KK} \sigma 2 s^2 \sigma^* 2 s^2 \sigma 2 p_z{ }^2 \pi 2 p_x{ }^2=\pi 2 p_y{ }^2 \pi^* 2 p_x{ }^1
\end{aligned}
\)
Bond order, \(\mathrm{O}_2 \quad \frac{8-4}{2}=2.0\)
Bond order, \(\mathrm{O}_2^{-} \quad \frac{8-5}{2}=1.5\)
Bond order, \(\mathrm{O}_2^{+} \quad \frac{8-3}{2}=2.5\)
\(
\therefore \quad \mathrm{O}_2^{+}>\mathrm{O}_2>\mathrm{O}_2^{-} \quad \text { or } \quad \mathrm{O}_2^{-}<\mathrm{O}_2<\mathrm{O}_2^{+}
\) -
Question 21 of 42
21. Question
The electronic configuration of the outer most shell of the most electronegative element is
CorrectIncorrectHint
(a) The electronic configuration represents
\(2 s^2 2 p^5=\) fluorine \(=\) most electronegative element
\(3 s^2 3 p^5=\) chlorine
\(4 s^2 4 p^5=\) bromine
\(5 s^2 5 p^5=\) iodine -
Question 22 of 42
22. Question
Amongst the following elements whose electronic configurations are given below, the one having the highest ionisation enthalpy is
CorrectIncorrectHint
(b)
(b) and (d) have exactly half-filled p-orbitals but (b) is smaller in size than Hence, (b) has highest ionization enthalpy.Alternate:
Hint: Elements having half-filled and fully-filled electronic configurations has high ionization enthalpy.
The electronic configuration of options (b) and (d) have exactly half-filled 3p orbitals (b) represents phosphorus and (c) represents arsenic but (b) is smaller in size than (d).
Hence, (b) has the highest ionization enthalpy increases left to right in the periodic table as the size decreases. -
Question 23 of 42
23. Question
Which of the following have an identical bond order?
CorrectIncorrectHint
(a, b)
\(
\begin{aligned}
& \mathrm{CN}^{-} \text {(number of electrons }=6+7+1=14 \text { ) } \\
& \mathrm{NO}^{+} \text {(number of electrons }=7+8-1=14 \text { ) } \\
& \mathrm{O}_2^{-} \text {(number of electrons }=8+8+1=17 \text { ) } \\
& \mathrm{O}_2^{2-} \text { (number of electrons }=8+8+2=18 \text { ) }
\end{aligned}
\)
Thus, \(\mathrm{CN}^{-}\)and \(\mathrm{NO}^{+}\)because of the presence of same number of electrons, have the same bond order. -
Question 24 of 42
24. Question
Which of the following attain the linear structure:
CorrectIncorrectHint
\((\mathrm{a}, \mathrm{d}) \mathrm{BeCl}_2(\mathrm{Cl}-\mathrm{Be}-\mathrm{Cl})\) and \(\mathrm{CS}_2(\mathrm{~S}=\mathrm{C}=\mathrm{S})\) both are linear, \(\mathrm{NCO}^{+}\)is non-linear. However, remember that \(\mathrm{NCO}(\mathrm{N}=\mathrm{C}=0)\) is linear because it is isoelectronic with \(\mathrm{CO}_2\). \(\mathrm{NO}_2\) is angular with bond angled \(132^{\circ}\) and each \(\mathrm{O}-\mathrm{N}\) bond length of \(1.20 \mathrm{~A}^{\circ}\) (intermediate between single and double bond).
-
Question 25 of 42
25. Question
\(\mathrm{CO}\) is isoelectronic with
CorrectIncorrectHint
\((a, b)\) Number of electrons in \(\mathrm{CO}=14\)
Number of electrons in \(\mathrm{NO}^{+}=14\)
Number of electrons in \(\mathrm{N}_2=14\)
Number of electrons in \(\mathrm{SnCl}_2=84\)
Number of electrons in \(\mathrm{NO}_2^{-}=24\) -
Question 26 of 42
26. Question
Which of the following species have the same shape?
CorrectIncorrectHint
\(\text { (c, d) } \mathrm{CO}_2 \rightarrow \text { Linear, } \mathrm{CCl}_4 \rightarrow \text { Tetrahedral, } \mathrm{O}_3 \rightarrow \text { Angular }\left(\mathrm{V} \text {-shaped), } \mathrm{NO}_2^{-} \rightarrow\right. \text { Angular (V-shaped) }\)
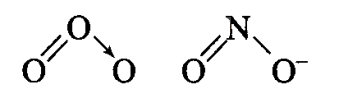
-
Question 27 of 42
27. Question
Which of the following statements are correct about \(\mathrm{CO}_3^{2-}\)?
CorrectIncorrectHint
(c,d)
Formal charge on \(\mathrm{O}\) atom (1): \(6-4-\frac{1}{2} \times 4=0\)
Formal charge on \(\mathrm{O}\) atom (2): \(6-6-\frac{1}{2} \times 2=-1\)
Formal charge on \(\mathrm{O}\) atom (3): \(6-6-\frac{1}{2} \times 2=-1\)
Average formal charge on each oxygen atom: \(\frac{-1+(-1)+0}{3}=-0.67\) All \(\mathrm{C}-\mathrm{O}\) bonds are equal due to resonance.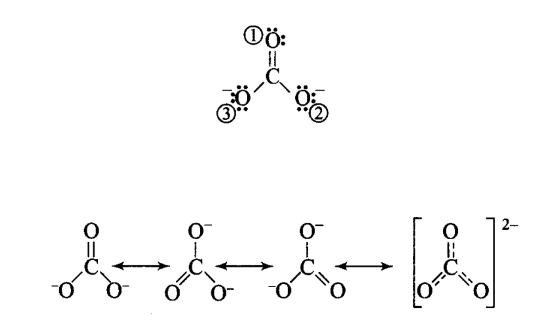
-
Question 28 of 42
28. Question
Diamagnetic species are those which contain no unpaired electrons. Which among the following are diamagnetic?
CorrectIncorrectHint
(a,d)
(a) Electronic configuration of \(\mathrm{N}_2=\sigma 1 s^2 \sigma^* 1 s^2 \sigma 2 s^2 \sigma^* 2 s^2 \pi 2 p_x^2 \approx \pi 2 p_y^2 \sigma 2 p_z^2\). It has no unpaired electron. This indicates it is a diamagnetic species.
(b) Electronic configuration of \(\mathrm{N}_2^{2-}\) ion \(=\sigma 1 s^2 \sigma^* 1 s^2 \sigma 2 s^2 \sigma^* 2 s^2\) \(\pi 2 p_x^2 \approx \pi 2 p_y^2 \sigma 2 p_z^2 \pi 2 p_x^1 \approx \pi * 2 p_y^1\).
It has two unpaired electrons, so it is paramagnetic in nature.
(c) Electronic configuration of \(\mathrm{O}_2=\dot{\sigma} 1 s^2 \sigma^* 1 s^2 \sigma 2 s^2 \sigma^* 2 s^2\)
\(
\sigma 2 p_z^2 \pi 2 p_x^2 \approx \pi 2 p_y^2 \pi^* 2 p_x^1 \approx \pi^* 2 p_y^1 \text {. }
\)
The presence of two unpaired electrons shows its paramagnetic nature.
(d) Electronic configuration of \(\mathrm{O}_2^{2-}\) ion \(=\sigma 1 s^2 \sigma^* 1 s^2 \sigma 2 s^2 \sigma^* 2 s^2\)
\(
\sigma 2 p_z^2 \pi 2 p_{\mathrm{x}}^2 \approx \sigma 2 p_y^2 \pi^* 2 p_x^2 \approx \pi^* 2 p_y^2 .
\)
It contains no unpaired electron, therefore, it is diamagnetic in nature. -
Question 29 of 42
29. Question
Species having same bond order are :
CorrectIncorrectHint
\(
\text { (c, d) The total number of electrons in } \mathrm{F}_2^{+} \text {and } \mathrm{O}_2^{-} \text {are same, i.e. } 17 \text { electrons. }
\)
\(
\mathrm{F}_2^{+}(17): \text { B.O. }=\frac{10-7}{2}=1.5
\)
\(
\mathrm{O}_2^{-}(17): \text { B.O. }=\frac{10-7}{2}=1.5
\) -
Question 30 of 42
30. Question
Which of the following statements are not correct?
CorrectIncorrectHint
(a) Ionic compounds are good conductors only in a molten state or aqueous solution since ions are not furnished in the solid state.
(b) In canonical structures there is a difference in the arrangement of electrons. -
Question 31 of 42
31. Question
Match the species in Column I with the geometry/shape in Column II.
\(
\begin{array}{|l|l|}
\hline \text { Column I } & \text { Column II } \\
\hline \text { (i) } \mathrm{H}_3 \mathrm{O}^{+} & \text {(a) Linear } \\
\hline \text { (ii) } \mathrm{HC} \equiv \mathrm{CH} & \text { (b) Angular } \\
\hline \text { (iii) } \mathrm{ClO}_2^{-} & \text {(c) Tetrahedral } \\
\hline \text { (iv) } \mathrm{NH}_4^{+} & \text {(d) Trigonal bipyramidal } \\
\hline & \text { (e) Pyramidal } \\
\hline
\end{array}
\)CorrectIncorrectHint
(a)
\(
\text { (i) } \rightarrow \text { (e); (ii) } \rightarrow \text { (a); (iii) } \rightarrow \text { (b); (iv) } \rightarrow \text { (c) }
\) -
Question 32 of 42
32. Question
Match the species in Column I with the bond order in Column II.
\(
\begin{array}{|l|l|l|l|}
\hline \text { Column I } & & \text { Column II } & \\
\hline \text { i } & N O & \text { A } & \mathbf{1 . 5} \\
\hline \text { ii } & C O & \text { B } & \mathbf{2 . 0} \\
\hline \text { iii } & O_2^{-} & \text {C } & \mathbf{2 . 5} \\
\hline \text { iv } & O_2 & \text { D } & \mathbf{3 . 0} \\
\hline
\end{array}
\)CorrectIncorrectHint
(b)
(i) \(\rightarrow\) c ; (ii) \(\rightarrow\) d; (iii) \(\rightarrow\) a ; (iv) \(\rightarrow\) b
\(
\begin{aligned}
& N O \rightarrow \text { B.O. }=\frac{1}{2}(10-5)=2.5 \\
& C O \rightarrow \text { B.O. }=\frac{1}{2}(10-4)=3 \\
& O_2^{-} \rightarrow \text { B.O. }=\frac{1}{2}(10-7)=1.5 \\
& O_2 \rightarrow \text { B.O. }=\frac{1}{2}(10-6)=2
\end{aligned}
\) -
Question 33 of 42
33. Question
Match the species in Column I with the type of hybrid orbitals in Column II.
\(
\begin{array}{|l|l|}
\hline \text { Column I } & \text { Column II } \\
\hline \text { (i) } \mathrm{SF}_4 & \text { (a) } \mathrm{sp}^3 \mathrm{~d}^2 \\
\hline \text { (ii) } \mathrm{IF}_5 & \text { (b) } \mathrm{d}^2 \mathrm{sp}^3 \\
\hline \text { (iii) } \mathrm{NO}_2{ }^{+} & \text {(c) } \mathrm{sp}^3 \mathrm{~d} \\
\hline \text { (iv) } \mathrm{NH}_4{ }^{+} & \text {(d) } \mathrm{sp}^3 \\
\hline & \text { (e) } \mathrm{sp} \\
\hline
\end{array}
\)CorrectIncorrectHint
(c) Definition of hybridisation.
Hybridization is the process of intermixing of the orbitals of slightly different energies to redistribute their energies, which results in the formation of new set of orbitals of equivalent energies and shape.
Hybridization helps in deciding the structure of the molecules.
Total number of hybrid orbitals \(=\) No. of sigma bonds made by central atom + No. of lone pairs of electrons on central atom.
Molecules Bond pairs(bp) lone pairs(lp) Total no.of hybrid orbitals Hybridisation
(i) \(\mathrm{SF}_4=\) number of \(\mathrm{bp}(4)+\) number of \(\mathrm{lp}(1)\) \(=\mathrm{sp}^3 \mathrm{~d}\) hybridisation(ii) \(I F_5=\) number of \(b p(5)+\) number of \(l p(1)\) \(=\mathrm{sp}^3 \mathrm{~d}^2\) bybridisation
(iii) \(\mathrm{NO}_2^{+}=\)number of \(b p(2)+\) number of \(\mathrm{lp}(0)\) \(=\mathrm{sp}\) hybridisation
(iv) \(\mathrm{NH}_4^{+}=\)number of \(b p(4)+\) number of \(\mathrm{lp}(0)\) \(=\mathrm{sp}^3\) hybridisation.
-
Question 34 of 42
34. Question
Match the items given in Column I with examples given in Column II.
\(
\begin{array}{|l|l|}
\hline \text { Column I } & \text { Column II } \\
\hline \text { (i) Hydrogen bond } & \text { (a) } \mathrm{C} \\
\hline \text { (ii) Resonance } & \text { (b) LiF } \\
\hline \text { (iii) Ionic solid } & \text { (c) } \mathrm{H}_2 \\
\hline \text { (iv) Covalent solid } & \text { (d) } \mathrm{HF} \\
\hline & \text { (e) } \mathrm{O}_3 \\
\hline
\end{array}
\)CorrectIncorrectHint
(a) Hydrogen bond – HF
Resonance \(-\mathrm{O}_3\)
Ionic solid – LiF
Covalent solid – C -
Question 35 of 42
35. Question
Match the shape of molecules in Column I with the type of hybridisation in Column II.
\(
\begin{array}{|l|l|}
\hline \text { Column I } & \text { Column II } \\
\hline \text { (i) Tetrahedral } & \text { (a) } \mathrm{sp}^2 \\
\hline \text { (ii) Trigonal } & \text { (b) } s p \\
\hline \text { (iii) Linear } & \text { (c) } s p^3 \\
\hline
\end{array}
\)CorrectIncorrectHint
(d)
(i) Tetrahedral shape-sp \({ }^3\) hybridisation
(ii) Trigonal shape- \(\mathrm{sp}^2\) hybridisation
(iii) Linear shape-sp hybridisation -
Question 36 of 42
36. Question
In the following questions, a statement of Assertion (A) followed by a statement of Reason (R) is given. Choose the correct option out of the choices given below each question.
Assertion (A): Sodium chloride formed by the action of chlorine gas on sodium metal is a stable compound.
Reason (R): This is because sodium and chloride ions acquire octet in sodium chloride formation.CorrectIncorrectHint
(a) Sodium chloride \(\left(\mathrm{Na}^{+} \mathrm{CL}^{-}\right)\)is stable ionic compound because both \(\mathrm{Na}^{+}\)and \(\mathrm{CL}^{-}\)ions have complete octet in outermost shell.
Explanation:
Assertion and reason both are correct and reason is the correct explanation of assertion.
\(
\underset{(2,8,1)}{\mathrm{Na}}+\underset{(2,8,7)}{\mathrm{Cl}} \rightarrow \underset{(2,8, 8)}{\mathrm{NaCl}}
\)
Here both \(\mathrm{Na}^{+}\)and \(\mathrm{Cl}^{-}\)have complete octet hence \(\mathrm{NaCl}\) is stable. -
Question 37 of 42
37. Question
In the following questions, a statement of Assertion (A) followed by a statement of Reason (R) is given. Choose the correct option out of the choices given below each question.
Assertion (A): Though the central atom of both \(\mathrm{NH}_3\) and \(\mathrm{H}_2 \mathrm{O}\) molecules are \(s p^3\) hybridised, yet \(\mathrm{H}-\mathrm{N}-\mathrm{H}\) bond angle is greater than that of \(\mathrm{H}-\mathrm{O}-\mathrm{H}\).
Reason (R): This is because nitrogen atom has one lone pair and oxygen atom has two lone pairs.CorrectIncorrectHint
(a)
\(\mathrm{H}_2 \mathrm{O}\) has two lone pairs while \(\mathrm{NH}_3\) has single lone pair, hence, \(\mathrm{H}_2 \mathrm{O}\) involves greater lone pair-bond pair repulsion.

-
Question 38 of 42
38. Question
In the following questions, a statement of Assertion (A) followed by a statement of Reason (R) is given. Choose the correct option out of the choices given below each question.
Assertion (A): Among the two \(\mathrm{O}-\mathrm{H}\) bonds in \(\mathrm{H}_2 \mathrm{O}\) molecule, the energy required to break the first \(\mathrm{O}-\mathrm{H}\) bond and the other \(\mathrm{O}-\mathrm{H}\) bond is the same.
Reason (R): This is because the electronic environment around oxygen is the same even after the breakage of one \(\mathrm{O}-\mathrm{H}\) bond.CorrectIncorrectHint
\(
\text { (d) Bond energy of two }(-\mathrm{O}-\mathrm{H}) \text { bonds in } \mathrm{H}_2 \mathrm{O} \text { will be different. }
\)
Correct assertion The bond enthalpies of the two \(\mathrm{O}-\mathrm{H}\) bonds in \(\mathrm{H}-\mathrm{O}-\mathrm{H}\) are not equal.
Correct reason This is because the electronic environment around \(\mathrm{O}\) is not the same after the breakage of one \(\mathrm{O}-\mathrm{H}\) bond. -
Question 39 of 42
39. Question
Comprehension given below is followed by some multiple-choice questions. Each question has one correct option. Choose the correct option.
Molecular orbitals are formed by the overlap of atomic orbitals. Two atomic orbitals combine to form two molecular orbitals called bonding molecular orbital (BMO) and anti bonding molecular orbital (ABMO). Energy of anti bonding orbital is raised above the parent atomic orbitals that have combined and the energy of the bonding orbital is lowered than the parent atomic orbitals. Energies of various molecular orbitals for elements hydrogen to nitrogen increase in the order :
\(
\sigma 1 s<\sigma^* 1 s<\sigma 2 s<\sigma^* 2 s<\left(\pi 2 p_x \approx \pi 2 p_y\right)<\sigma 2 p_z<\left(\pi^* 2 p_x \approx \pi^* 2 p_y\right)<\sigma^* 2 p_z
\)
for oxygen and fluorine order of energy of molecular orbitals is given below :
\(
\sigma \mathrm{ls}<\sigma^* 1 \mathrm{~s}<\sigma 2 \mathrm{~s}<\sigma^* 2 \mathrm{~s}<\sigma 2 p_{\mathrm{z}}<\left(\pi 2 p_x=\pi 2 p_y\right)<\left(\pi^* 2 p_x=\pi^* 2 p_y\right)<\sigma^* 2 p_{\mathrm{z}}
\)
Different atomic orbitals of one atom combine with those atomic orbitals of the second atom which have comparable energies and proper orientation. Further, if the overlapping is head-on, the molecular orbital is called ‘Sigma’, \((\sigma)\) and if the overlap is lateral, the molecular orbital is called ‘pi’, \((\pi)\). The molecular orbitals are filled with electrons according to the same rules as followed for the filling of atomic orbitals. However, the order for filling is not the same for all molecules or their ions. Bond order is one of the most important parameters to compare the strength of bonds.Which of the following statements is correct?
CorrectIncorrectHint
(a) In the formation of dioxygen from oxygen atoms, ten molecular orbitals will be formed.
\(
\mathrm{O}_2=\frac{\sigma 1 \mathrm{~s}^2}{1} \frac{\sigma^* 1 \mathrm{~s}^2}{2} \frac{\sigma 2 \mathrm{~s}^2}{3} \frac{\sigma^* 2 \mathrm{~s}^2}{4} \frac{\sigma_2 \mathrm{p}_{\mathrm{z}}^2}{5} \frac{\pi 2 \mathrm{p}_{\mathrm{x}}^2}{6} \frac{\pi 2 \mathrm{p}_{\mathrm{y}}^2}{7} \frac{\pi^* 2 \mathrm{p}_{\mathrm{x}}^1}{8} \frac{\pi^* 2 \mathrm{p}_{\mathrm{y}}^1}{9} \frac{\sigma^* 2 \mathrm{p}_{\mathrm{z}}^0}{10}
\) -
Question 40 of 42
40. Question
Comprehension given below is followed by some multiple choice questions. Each question has one correct option. Choose the correct option.
Molecular orbitals are formed by the overlap of atomic orbitals. Two atomic orbitals combine to form two molecular orbitals called bonding molecular orbital (BMO) and anti bonding molecular orbital (ABMO). Energy of anti bonding orbital is raised above the parent atomic orbitals that have combined and the energy of the bonding orbital is lowered than the parent atomic orbitals. Energies of various molecular orbitals for elements hydrogen to nitrogen increase in the order :
\(
\sigma 1 s<\sigma^* 1 s<\sigma 2 s<\sigma^* 2 s<\left(\pi 2 p_x \approx \pi 2 p_y\right)<\sigma 2 p_z<\left(\pi^* 2 p_x \approx \pi^* 2 p_y\right)<\sigma^* 2 p_z
\)
for oxygen and fluorine order of energy of molecular orbitals is given below :
\(
\sigma \mathrm{ls}<\sigma^* 1 \mathrm{~s}<\sigma 2 \mathrm{~s}<\sigma^* 2 \mathrm{~s}<\sigma 2 p_{\mathrm{z}}<\left(\pi 2 p_x=\pi 2 p_y\right)<\left(\pi^* 2 p_x=\pi^* 2 p_y\right)<\sigma^* 2 p_{\mathrm{z}}
\)
Different atomic orbitals of one atom combine with those atomic orbitals of the second atom which have comparable energies and proper orientation. Further, if the overlapping is head on, the molecular orbital is called ‘Sigma’, \((\sigma)\) and if the overlap is lateral, the molecular orbital is called ‘pi’, \((\pi)\). The molecular orbitals are filled with electrons according to the same rules as followed for filling of atomic orbitals. However, the order for filling is not the same for all molecules or their ions. Bond order is one of the most important parameters to compare the strength of bonds.Which of the following molecular orbitals has maximum number of nodal planes?
CorrectIncorrectHint
(b) The number of nodal planes are as follows
\(
\begin{aligned}
\sigma * 1 \mathrm{~s} & \rightarrow 1 . \\
\sigma * 2 \mathrm{p}_z & \rightarrow 3 \\
\pi 2 \mathrm{p}_{\mathrm{x}} & \rightarrow 1 \\
\pi * 2 \mathrm{p}_{\mathrm{y}} & \rightarrow 2
\end{aligned}
\)
Hence option (b) is the correct answer -
Question 41 of 42
41. Question
Comprehension given below is followed by some multiple choice questions. Each question has one correct option. Choose the correct option.
Molecular orbitals are formed by the overlap of atomic orbitals. Two atomic orbitals combine to form two molecular orbitals called bonding molecular orbital (BMO) and anti bonding molecular orbital (ABMO). Energy of anti bonding orbital is raised above the parent atomic orbitals that have combined and the energy of the bonding orbital is lowered than the parent atomic orbitals. Energies of various molecular orbitals for elements hydrogen to nitrogen increase in the order :
\(
\sigma 1 s<\sigma^* 1 s<\sigma 2 s<\sigma^* 2 s<\left(\pi 2 p_x \approx \pi 2 p_y\right)<\sigma 2 p_z<\left(\pi^* 2 p_x \approx \pi^* 2 p_y\right)<\sigma^* 2 p_z
\)
for oxygen and fluorine order of energy of molecular orbitals is given below :
\(
\sigma \mathrm{ls}<\sigma^* 1 \mathrm{~s}<\sigma 2 \mathrm{~s}<\sigma^* 2 \mathrm{~s}<\sigma 2 p_{\mathrm{z}}<\left(\pi 2 p_x=\pi 2 p_y\right)<\left(\pi^* 2 p_x=\pi^* 2 p_y\right)<\sigma^* 2 p_{\mathrm{z}}
\)
Different atomic orbitals of one atom combine with those atomic orbitals of the second atom which have comparable energies and proper orientation. Further, if the overlapping is head on, the molecular orbital is called ‘Sigma’, \((\sigma)\) and if the overlap is lateral, the molecular orbital is called ‘pi’, \((\pi)\). The molecular orbitals are filled with electrons according to the same rules as followed for filling of atomic orbitals. However, the order for filling is not the same for all molecules or their ions. Bond order is one of the most important parameters to compare the strength of bonds.Which of the following pair is expected to have the same bond order?
CorrectIncorrectHint
(b)
Bond order \(=\left(\mathrm{N}_{\mathrm{b}}-\mathrm{N}_{\mathrm{a}}\right) / 2\)
In \(\mathrm{O}_2^{+}\), number of electrons in bonding orbitals are 10 and in anti bonding are 5.
\(\sigma 1 \mathrm{~s}^2<\sigma * 1 \mathrm{~s}^2<\sigma 2 \mathrm{~s}^2<\sigma * 2 \mathrm{~s}^2<\sigma 2 \mathrm{p}_{\mathrm{z}}^2\left(\pi 2 \mathrm{p}_{\mathrm{x}}^2=\pi 2 \mathrm{p}_{\mathrm{x}}^2\right)<\left(\pi * 2 \mathrm{p}_{\mathrm{x}}^1=\pi * 2 \mathrm{p}_{\mathrm{x}}\right)<\sigma * 2 \mathrm{p}_{\mathrm{z}}\)
Bond order \(=(10-5) / 2=2.5\)
In \(\mathrm{N}_2{ }^{-}\), number of electrons in bonding orbitals are 10 and in anti- bonding are 5.
\(\sigma 1 \mathrm{~s}^2<\sigma * 1 \mathrm{~s}^2<\sigma 2 \mathrm{~s}^2<\sigma * 2 \mathrm{~s}^2<\left(\pi 2 \mathrm{p}_{\mathrm{x}}^2=\pi 2 \mathrm{p}_{\mathrm{x}}^2\right)<\sigma 2 \mathrm{p}_{\mathrm{z}}^2<\left(\pi * 2 \mathrm{p}_{\mathrm{x}}^1=\pi * 2 \mathrm{p}_{\mathrm{x}}\right)<\sigma *\)
\(2 \mathrm{p}_z\)
Bond order \(=(10-5) / 2=2.5\).
Bond order for
\(\mathrm{O}_2 \rightarrow 2\)
\(\mathrm{N}_2 \rightarrow 3\)
\(\mathrm{O}_2{ }^{-} \rightarrow 1.5\)
\(\mathrm{N}_2^{+} \rightarrow 2.5\) -
Question 42 of 42
42. Question
Comprehension given below is followed by some multiple choice questions. Each question has one correct option. Choose the correct option.
Molecular orbitals are formed by the overlap of atomic orbitals. Two atomic orbitals combine to form two molecular orbitals called bonding molecular orbital (BMO) and anti bonding molecular orbital (ABMO). Energy of anti bonding orbital is raised above the parent atomic orbitals that have combined and the energy of the bonding orbital is lowered than the parent atomic orbitals. Energies of various molecular orbitals for elements hydrogen to nitrogen increase in the order :
\(
\sigma 1 s<\sigma^* 1 s<\sigma 2 s<\sigma^* 2 s<\left(\pi 2 p_x \approx \pi 2 p_y\right)<\sigma 2 p_z<\left(\pi^* 2 p_x \approx \pi^* 2 p_y\right)<\sigma^* 2 p_z
\)
for oxygen and fluorine order of energy of molecular orbitals is given below :
\(
\sigma \mathrm{ls}<\sigma^* 1 \mathrm{~s}<\sigma 2 \mathrm{~s}<\sigma^* 2 \mathrm{~s}<\sigma 2 p_{\mathrm{z}}<\left(\pi 2 p_x=\pi 2 p_y\right)<\left(\pi^* 2 p_x=\pi^* 2 p_y\right)<\sigma^* 2 p_{\mathrm{z}}
\)
Different atomic orbitals of one atom combine with those atomic orbitals of the second atom which have comparable energies and proper orientation. Further, if the overlapping is head-on, the molecular orbital is called ‘Sigma’, \((\sigma)\) and if the overlap is lateral, the molecular orbital is called ‘pi’, \((\pi)\). The molecular orbitals are filled with electrons according to the same rules as followed for the filling of atomic orbitals. However, the order for filling is not the same for all molecules or their ions. Bond order is one of the most important parameters to compare the strength of bonds.In which of the following molecules, \(\sigma 2 p_z\) molecular orbital is filled after \(\pi 2 p_x\) and \(\pi 2 p_{\mathrm{y}}\) molecular orbitals?
CorrectIncorrectHint
(c) The first atomic orbitals on two atoms form two molecular orbitals designated as
\(\sigma 1 s\) and \(\sigma^* 1 s\)
Similarly, the \(2 \mathrm{~s}\) and \(2 \mathrm{p}\) atomic orbitals give rise to the eight molecular orbitals given below
Antibonding \(\operatorname{MOs} \sigma^* 2 s \sigma^* 2 p_z \pi^* 2 p_x \pi^* 2 p_y\)
Bonding \(\operatorname{Mos} \sigma 2 s \sigma 2 p_z \pi 2 p_x \pi 2 p_y\)
For molecules such as \(\mathrm{B}_2, \mathrm{C}_2, \mathrm{~N}_2\), etc. it is observed that the increasing order of energies of various molecular orbitals is –
Here, the important characteristic feature is that the energy of \(\sigma 2 p_z\) molecular orbital is higher than that of \(\pi 2 p_x\) and \(\pi 2 p_y\) molecular orbitals