NCERT Exemplar MCQs
Quiz Summary
0 of 36 Questions completed
Questions:
Information
You have already completed the quiz before. Hence you can not start it again.
Quiz is loading…
You must sign in or sign up to start the quiz.
You must first complete the following:
Results
Results
0 of 36 Questions answered correctly
Your time:
Time has elapsed
You have reached 0 of 0 point(s), (0)
Earned Point(s): 0 of 0, (0)
0 Essay(s) Pending (Possible Point(s): 0)
Categories
- Not categorized 0%
- 1
- 2
- 3
- 4
- 5
- 6
- 7
- 8
- 9
- 10
- 11
- 12
- 13
- 14
- 15
- 16
- 17
- 18
- 19
- 20
- 21
- 22
- 23
- 24
- 25
- 26
- 27
- 28
- 29
- 30
- 31
- 32
- 33
- 34
- 35
- 36
- Current
- Review
- Answered
- Correct
- Incorrect
-
Question 1 of 36
1. Question
Which of the following conclusions could not be derived from Rutherford’s \(\alpha\)-particle scattering experiment?
CorrectIncorrectHint
(c) The concept of circular paths of fixed energy was proposed by Bohr and not derived from Rutherford’s scattering experiment.
-
Question 2 of 36
2. Question
Which of the following options does not represent ground state electronic configuration of an atom?
CorrectIncorrectHint
\(
\text { (b) Correct configuration in ground state should be } 1 s^2 2 s^2 2 p^6 3 s^2 3 p^{10} 3 d^{10} 4 s^1
\)Hint: Fully filled subshell is more stable than partially filled subshell
Correct configuration should be \(1 s^2 2 s^2 2 p^6 3 s^2 3 p^6 3 d^{10} 4 s^1\) for the copper which has atomic number 29 \(
\left({ }_{29} \mathrm{Cu}\right)\). Due to the extra stability of fully filled orbital of \(\)d-\(\) subshell, the last electron enters into the d-orbital instead of the s-orbital. Hence, option second is the correct answer. -
Question 3 of 36
3. Question
The probability density plots of 1s and 2s orbitals are given in Fig. 2.1:
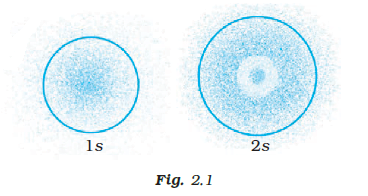
The density of dots in a region represents the probability density of finding electrons in the region. On the basis of above diagram which of the following statements is incorrect?
CorrectIncorrectHint
Hint: The probability of finding an electron decreases uniformly as the distance from the nucleus increases
The probability density of electrons in \(2 \mathrm{~s}\) orbital first increases then decreases and after that, it begins to increase again as distance increases from the nucleus. -
Question 4 of 36
4. Question
Which of the following statements is not correct about the characteristics of cathode rays?
CorrectIncorrectHint
(d) Cathode rays consist of negatively charged material particles called electrons. They were discovered by William Crookes. The characteristics of cathode rays do not depend upon the material of electrodes and the nature of the gas present in the cathode ray tube.
-
Question 5 of 36
5. Question
Which of the following statements about the electron is incorrect?
CorrectIncorrectHint
The mass of the electron is very small as compared to the mass of the neutron.
Mass of electron \(=9.1 \times 10^{-31} \mathrm{~kg}\)
Mass of neutron \(=1.67 \times 10^{-27} \mathrm{~kg}\) -
Question 6 of 36
6. Question
Which of the following properties of the atom could be explained correctly by the Thomson Model of the atom?
CorrectIncorrectHint
Hint: Atom is neutral
J J Thomson, in 1898, proposed the plum pudding, (raisin pudding or watermelon) model of an atom. An important feature of this model is that the mass of the atom is assumed to be uniformly distributed over the atom. This model was able to explain the overall neutrality of the atom. -
Question 7 of 36
7. Question
Two atoms are said to be isobars if
CorrectIncorrectHint
Hint: Elements have the same mass number and different atomic number Isobars have the same mass number (i.e., the sum of protons and neutrons) but a different atomic number (i.e., number of protons). e.g., \({ }_{18}^{40} \mathrm{Ar}\) and \({ }_{19}^{40} \mathrm{~K}\) are isobars.
\(\begin{array}{ll}{ }_{18}^{40} \mathrm{Ar} & { }_{19}^{40} \mathrm{~K}\end{array}\)
Atomic number \(=18\) Atomic number \(=19\)
Mass number \(=40 \quad\) Mass number \(=40\) -
Question 8 of 36
8. Question
The number of radial nodes for \(3 p\) orbital is _____.
CorrectIncorrectHint
Explanation:
Quantum numbers are characteristic quantities that are used to describe the various properties of an electron in an atom-like position, energy, or spin of the electrons. There are four quantum numbers namely, principal quantum number, azimuthal quantum number, magnetic quantum number, and spin quantum number.
Now for an orbital, the total number of nodes is \((n-1)\). Where \(\mathrm{n}\) is the principal quantum number. The principal quantum numbers of the shells are, for \(\mathrm{K}\) shell is 1 , for \(\mathrm{L}\) is 2 , for \(\mathrm{M}\) shell is 3 , for \(\mathrm{N}\) shell is 4 , etc. it cannot be fraction number.
Now the number of angular nodes is equal to the azimuthal quantum number denoted by ‘ \(\mathrm{l}\) ‘.
Therefore, the total number of radial nodes is, \((n-l-1)\)
Now, for \(3 p\) orbital, the value of \(\mathrm{n}\) is 3 , and the azimuthal quantum number is 1 . Therefore, the number of radial node is,
\(
\begin{aligned}
& (n-l-1) \\
= & (3-1-1) =1
\end{aligned}
\) -
Question 9 of 36
9. Question
Number of angular nodes for \(4 d\) orbital is _____.
CorrectIncorrectHint
Hint: Angular node is equal to I value
Number of angular nodes \(=1\)
For \(4^{\text {th }}\) orbital \((n=4)\) and \(l=2\) for \(d\)-orbital
Number of angular nodes \(=2\)Explanation: A radial node is a sphere that occurs when the radial wave function of the atomic orbital is zero or the sign of the wave function changes. On the other hand, angular nodes are either \(x, y\) or \(z\) planes where the electrons aren’t present.
The number of radial nodes can be solved on the basis of the following equation:
\(
{r}={n}-{l}-1
\)
The formula for the total number of nodes is, \({n}-1\)
Therefore angular nodes \(=\) total nodes-radial nodes.
For the \(4 \mathrm{~d}\) orbital, \({n}=4\) and \({l}=2\)
Therefore, \(r=4-2-1; r=1\).
Total number of nodes \(=4-1=3\)
Therefore the angular nodes \(=\) total nodes – radial nodes \(=3-1=2\)
Therefore the \(4 \mathrm{~d}\) orbital has 1 radial node and 2 angular nodes. -
Question 10 of 36
10. Question
Which of the following is responsible to rule out the existence of definite paths or trajectories of electrons?
CorrectIncorrectHint
(b) According to Heisenberg’s uncertainty principle, the position and velocity of an electron cannot be determined simultaneously with accuracy which rules out the existence of fixed paths.
Explanation: Hint: Position of electron and momentum of electron cannot calculate simultaneously
Werner Heisenberg, a German physicist in 1927 , stated uncertainty principle which states that it is impossible to determine simultaneously, the exact position and exact momentum of an electron.
Mathematically, \(\Delta \mathrm{x} \times \Delta \mathrm{p} \geq \frac{\mathrm{h}}{4 \pi}\)
The important implications of the Heisenberg uncertainty principle is that it rules out existence of definite paths or trajectories of electrons and other similar particles. -
Question 11 of 36
11. Question
The total number of orbitals associated with the third shell will be ________.
CorrectIncorrectHint
\(
\text { (c) No of orbitals in } 3^{\text {rd }} \text { shell }(n=3)=n^2=3^2=9 \text {. }
\)Explanation:
Hint: In atomic theory and quantum mechanics, an atomic orbital is a mathematical function describing the location and wave-like behaviour of an electron in an atom. This function can be used to calculate the probability of finding any electron of an atom in any specific region around the atom’s nucleus.
We have been asked about the number of orbitals associated with third shell, So, for that,
We know that there can be two electrons in one orbital maximum. The s sublevel has just one orbital, so can contain 2 electrons max. The p sublevel has 3 orbitals, so can contain 6 electrons max. The d sublevel has 5 orbitals, so can contain 10 electrons max.
Now, the orbit around the nucleus within which the electron rotates is called shells or Energy levels.” Each discrete distance orbit from the nucleus corresponds to a certain energy level. The electron that rotates in the lowest orbit has the lowest energy level and in the outermost orbit, electrons have higher energy levels.
We know that, the total number of orbitals in the nth shell: \(n^2\),
Where, \(n=\) shell/ principal quantum number,
So, we need to calculate the total number of orbitals in the third shell,
So, \(\mathrm{n}\) will be equal to 3 ,
Therefore, the orbitals would be: \(3^2=9\).
So, the orbitals are one 3-s orbital, three 3-p orbital and five 3-d orbitals. -
Question 12 of 36
12. Question
Orbital angular momentum depends on ____.
CorrectIncorrectHint
\(
\text { (a) Orbital angular momentum }=\sqrt{l(l+1)} \frac{h}{2 \pi} \text {. Hence, it depends only on ‘ } l \text { ‘. }
\)
Example: What is the Angular Quantum Number of d electrons?
Ans: The orbital angular momentum is \((\mathrm{L})=\sqrt{l(l+1)} \frac{h}{2 \pi}\)
Here, the orbital \(d\) is used. So, the value of \(l=2\)
Therefore, the orbital angular momentum can be represented as \(\sqrt{6} \frac{h}{2 \pi}\) -
Question 13 of 36
13. Question
Chlorine exists in two isotopic forms, \(\mathrm{Cl}-37\) and \(\mathrm{Cl}-35\) but its atomic mass is 35.5. This indicates the ratio of \(\mathrm{Cl}-37\) and \(\mathrm{Cl}-35\) is approximately
CorrectIncorrectHint
\(
\text { Hint: average atomic mass }=\frac{(\mathrm{Cl}-37 \text { isotope } \% \times \text { atomic mass })+(\mathrm{Cl}-35 \text { isotope } \% \times \text { atomic mass })}{100}
\)
Step 1:
The fractional mass of chlorine is 35.5. The isotopic percentage of \(\mathrm{Cl}-37\) is \(\mathrm{X} \%\) and the isotopic percentage of \(\mathrm{Cl}-35\) is \(100-\mathrm{x} \%\). average atomic mass \(=\frac{(\mathrm{Cl}-37 \text { isotope } \% \times \text { atomic mass })+(\mathrm{Cl}-35 \text { isotope } \% \times \text { atomic mass })}{100}\)
Step 2:
Calculate the ratio is as follows:
\(
\begin{aligned}
& 35.5=\frac{(\mathrm{X} \times 37)+((100-\mathrm{X}) \times 35)}{100} \\
& 3550=37 \mathrm{X}+3500-35 \mathrm{X} \\
& 3550-3500=2 \mathrm{X} \\
& 50=2 \mathrm{X} \\
& \mathrm{X}=25 \\
& 100-\mathrm{X}=75
\end{aligned}
\)
The ratio of \(\mathrm{Cl}-37\) and \(\mathrm{Cl}-35=\frac{25}{75}\) \(=1: 3\)Alternate:
\(
{ }_{17}^{37} \mathrm{Cl}:{ }_{17}^{35} \mathrm{Cl}
\)
\(
1: \quad \quad 3 \text { Ratio }
\)
\(
\begin{aligned}
\begin{array}{c}
\text { Average atomic mass }
\end{array} & =\frac{(37 \times 1)+(35 \times 3)}{1+3} \\
& =\frac{142}{4}=35.5
\end{aligned}
\) -
Question 14 of 36
14. Question
The pair of ions having same electronic configuration is ____.
CorrectIncorrectHint
\(
\begin{aligned}
& \text { (b) } \mathrm{Cr}^{3+}(Z=24)=[\mathrm{Ar}] 3 d^3 \\
& \mathrm{Fe}^{3+}(Z=26)=[\mathrm{Ar}] 3 d^5 \\
& \mathrm{Mn}^{2+}(Z=25)=[\mathrm{Ar}] 3 d^5 \\
& \mathrm{Co}^{3-}(Z=27)=[\mathrm{Ar}] 3 d^6
\end{aligned}
\)
\(
\mathrm{Sc}^{3+}(Z=21)=[\mathrm{Ar}] 3 d^0
\)
\(\mathrm{Fe}^{3+}\) and \(\mathrm{Mn}^{2+}\) will have the same number of electrons, i.e., 23 and, hence, have the same electronic configuration.Explanation:
Electronic configuration of \(\mathrm{Fe}^{3+}\)
– Electronic configuration of \(\mathrm{Fe}\) is \(1 s^2 2 s^2 2 p^6 3 s^2 3 p^6 4 s^2 3 d^6\).
– Three electrons must be released for \(\mathrm{Fe}^{3+}\).
– So, the electronic configuration of \(\mathrm{Fe}^{3+}\) is \(1 s^2 2 s^2 2 p^6 3 s^2 3 p^6 3 d^5\).
Electronic configuration of \(\mathrm{Mn}^{2+}\)
– Electronic configuration of \(\mathrm{Mn}\) is \(1 s^2 2 s^2 2 p^6 3 s^2 3 p^6 4 s^2 3 d^5\).
– Two electrons must be released for \(\mathrm{Mn}^{2+}\).
– So, the electronic configuration of \(\mathrm{Mn}^{2+}\) is \(1 s^2 2 s^2 2 p^6 3 s^2 3 p^6 3 d^5\).
So, pair of ions having the same electronic configuration is \(\mathrm{Fe}^{3+}, \mathrm{Mn}^{2+}\). The explanation for the incorrect options
– \(\mathrm{Cr}^{3+}, \mathrm{Fe}^{3+}\) :-The electronic configuration of \(\mathrm{Cr}^{3+}\) is \(1 s^2 2 s^2 2 p^6 3 s^2 3 p^6 3 d^3\).
– The electronic configuration of \(\mathrm{Fe}^{3+}\) is \(1 s^2 2 s^2 2 p^6 3 s^2 3 p^6 3 d^5\).
– \(\mathrm{Fe}^{3+}, \mathrm{Co}^{3+}\) :-The electronic configuration of \(\mathrm{Co}^{3+}\) is \(1 s^2 2 s^2 2 p^6 3 s^2 3 p^6 3 d^6\).
– \(\mathrm{Sc}^{3+}, \mathrm{Cr}^{3+}\) : The electronic configuration of \(\mathrm{Sc}^{3+}\) is \(1 s^2 2 s^2 2 p^6 3 s^2 3 p^6\).
– Hence. options (a), (c), and (d) are incorrect.
Hence, option(b) is correct. The pair of ions having the same electronic configuration is \(\mathrm{Fe}^{3+}, \mathrm{Mn}^{2+}\). -
Question 15 of 36
15. Question
For the electrons of the oxygen atom, which of the following statements is correct?
CorrectIncorrectHint
\(
\text { Hint: Two electrons present in any orbital have spin quantum numbers } m_s \text { but of opposite sign, that is, } \frac{-1}{2} \text { and } \frac{1}{2} \text {. }
\)(d) (a) Electrons in \(2 s\) and \(2 p\) orbitals have different screening effect. Hence, their \(Z_{\text {eff }}\) is different. \(Z_{\text {eff }}\) of \(2 s\) orbital \(>Z_{\text {eff }}\) of \(2 p\) orbital Therefore, it is not correct.
(b) Energy of \(2 s\) orbital \(<\) energy of \(2 p\) orbital. Hence, it is not correct.
(c) \(Z_{\text {eff }}\) of \(1 s\) orbital \(\neq Z_{\text {eff }}\) of 2 s orbital Hence, it is incorrect.
(d) For the two electrons of \(2 s\) orbital, the value of \(m_{\mathrm{s}}\) is \(+\frac{1}{2}\) and \(-\frac{1}{2}\). Hence, it is correct. -
Question 16 of 36
16. Question
If travelling at same speeds, which of the following matter waves have the shortest wavelength?
CorrectIncorrectHint
(b) \(\lambda=\frac{h}{m v}\). For same value of \(v\), larger the value of mass \(m\), shorter is the wavelength, \(\lambda\). Here, \(\alpha\)-particles have the largest mass.
Since, alpha particles have the largest mass, they have the shortest wavelength. -
Question 17 of 36
17. Question
Identify the pairs which are not of isotopes?
CorrectIncorrectHint
(a) \({ }_6^{12} \mathrm{X}\) and \({ }_6^{13} \mathrm{Y}\) have same atomic number but different mass number.
(b) \({ }_{17}^{35} \mathrm{X}\) and \({ }_{17}^{37} \mathrm{Y}\) have same atomic number but different mass number. Both these pairs are isotopes to each other.
(c) \({ }_6^{14} \mathrm{X}\) and \({ }_7^{14} \mathrm{Y}\) have different atomic number but same mass number.
(d) \({ }_4^8 \mathrm{X}\) and \({ }_5^8 \mathrm{Y}\) have different atomic number but same mass number.
(c, d) Isotopes have the same atomic number but different mass number. -
Question 18 of 36
18. Question
Out of the following pairs of electrons, identify the pairs of electrons present in degenerate orbitals :
CorrectIncorrectHint
(a, d) Degenerate orbitals mean the orbitals of the same sub-shell of the same main shell, i.e., their \(\mathrm{n}\) and l values are the same. Other two pairs have different values of \(n\) and \(l\) hence, cannot be having the same energy. i.e., their \(\mathrm{n}\) and l value.
(a) (i) \(3 \mathrm{~d}_{\mathrm{xy}}\) (ii) \(3 \mathrm{~d}_{\mathrm{yz}}\)
(b) (i) \(3 \mathrm{p}_{\mathrm{x}}\) (ii) \(3 \mathrm{~d}_{\mathrm{xy}}\)
(c) (i) \(4 \mathrm{~s}\) (ii) \(3 \mathrm{~d}_{\mathrm{xy}}\)
(d) (i) \(4 \mathrm{~d}_{\mathrm{x}^2-\mathrm{y}^2}\) (ii) \(3 \mathrm{~d}_{\mathrm{x}^2-\mathrm{y}^2}\)
Thus, \(3 \mathrm{~d}_{\mathrm{xy}}\) and \(3 \mathrm{~d}_{\mathrm{yz}} ; 3 \mathrm{~d}_{\mathrm{x}^2-\mathrm{y}^2}\) and \(3 \mathrm{~d}_{\mathrm{x}^2-\mathrm{y}^2}\) represent pair of degenerate orbitals. -
Question 19 of 36
19. Question
Which of the following sets of quantum numbers are correct?
\(
\begin{array}{rrrr}
& \boldsymbol{n} & \boldsymbol{l} & \boldsymbol{m}_{\boldsymbol{l}} \\
\text { (i) } & 1 & 1 & +2 \\
\text { (ii) } & 2 & 1 & +1 \\
\text { (iii) } & 3 & 2 & -2 \\
\text { (iv) } & 3 & 4 & -2
\end{array}
\)CorrectIncorrectHint
(b, c) If \(n=1, l \neq 1\). Hence, (i) is wrong.
If \(n=2, l=0,1\). For \(l=1, m=-1,0,+1\). Hence (ii) is correct.
If \(n=3,l=0,1,2\). For \(l=2, m=-2,-1,0,+1,+2\). Hence (iii) is correct.
If \(n=3, l \neq 4\). Hence, (iv) is wrong. -
Question 20 of 36
20. Question
In which of the following pairs, the ions are iso-electronic?
CorrectIncorrectHint
For,
\(
\begin{aligned}
& \mathrm{Na}^{+}=11-1=10 \mathrm{e}^{-} \\
& \mathrm{Mg}^{2+}=12-2=10 \mathrm{e}^{-}
\end{aligned}
\)
Thus, they have same number of electrons.
\(
\text { Fo, } \mathrm{Al}^{3+}=13-3=10 \mathrm{e}^{-}, \mathrm{O}^{-}=8+1=9 \mathrm{e}^{-}
\)
They do not have same number of electrons.
For, \(\mathrm{Na}^{+}=10 \mathrm{e}^{-}, \mathrm{O}^{2-}=8+2=10 \mathrm{e}^{-}\)
They have same number of electrons.
For, \(\mathrm{N}^{3-}=7+3=10 \mathrm{e}^{-}, \mathrm{Cl}^{-}=17+1=18 \mathrm{e}^{-}\)
They do not have same number of electrons.
Thus, \(\mathrm{Na}^{+}\)is isoelectronic with \(\mathrm{Mg}^{2+}\) and \(\mathrm{O}^{2-}\). -
Question 21 of 36
21. Question
Which of the following statements concerning the quantum numbers are correct?
CorrectIncorrectHint
(a, d)
(a) Azimuthal quantum number l is also known as orbital angular momentum or subsidiary quantum number. It determines the three-dimensional shape of the orbital.
(b) The principal quantum number determines the size of the orbit.
(c) Magnetic quantum number determines the orientation of the electron cloud in a subshell.
(d) An electron spins around its own axis, much in a similar way as the earth spins around its own axis while revolving around the sun. In other words, an electron has, besides charge and mass, intrinsic spin angular quantum number. -
Question 22 of 36
22. Question
Select the correct choice of arrangement of \(s, p\) and \(d\) sub-shells of a shell in the increasing order of effective nuclear charge \(\left(Z_{\text {eff }}\right)\) experienced by the electron present in them.
CorrectIncorrectHint
(a) s-orbitals shield the electrons from the nucleus more effectively than p-orbitals which in turn shield more effectively than d-orbitals. Hence, the arrangement of subshells in the increasing order of effective nuclear charge is:
\(d<p<s\) -
Question 23 of 36
23. Question
Find the distribution of electrons in oxygen atom (atomic number 8 ) using the orbital diagram given below.
 CorrectIncorrect
CorrectIncorrectHint
From the orbital diagram, it is seen that there are two unpaired electrons.
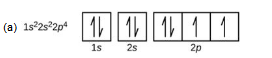
-
Question 24 of 36
24. Question
Nickel atom can lose two electrons to form \(\mathrm{Ni}^{2+}\) ion. The atomic number of nickel is 28. From which orbital will nickel lose two electrons.
CorrectIncorrectHint
\({ }_{28} \mathrm{Ni}=1 \mathrm{~s}^2, 2 \mathrm{~s}^2, 2 \mathrm{p}^6, 3 \mathrm{~s}^2, 3 \mathrm{p}^6, 3 \mathrm{~d}^8, 4 \mathrm{~s}^2\); Nickel will lose 2 electrons from \(4 \mathrm{~s}\) (outermost shell) to form \(\mathrm{Ni}^{2+}\) ion.
Hence, \({ }_{26} \mathrm{Ni}^{2+}=1 \mathrm{~s}^2, 2 \mathrm{~s}^2, 2 \mathrm{p}^6, 3 \mathrm{~s}^2, 3 \mathrm{p}^6, 3 \mathrm{~d}^8\) -
Question 25 of 36
25. Question
Which of the following orbitals are degenerate?
\(
3 d_{x y}, 4 d_{x y}, 3 d_{y z}, 3 d_{z^2}, 4 d_{y z}, 4 d_{z^2}:
\)CorrectIncorrectHint
Degenerate orbitals are the orbitals of the same subshell of the same main shell. Hence, these are
\(
\left(3 d_{x y}, 3 d_{z^2}, 3 d_{y z}\right) \text { and }\left(4 d_{x y}, 4 d_{x z}, 4 d_{z^2}\right) \text {. }
\) -
Question 26 of 36
26. Question
Calculate the total number of angular nodes and radial nodes present in \(3 p\) orbital.
CorrectIncorrectHint
For \(3 p\) orbital \(n=3, l=1\)
Number of angular nodes \(=l=1\)
Number of radial nodes \(=n-l-1=3-1-1=1\) -
Question 27 of 36
27. Question
An atom having atomic mass number 13 has 7 neutrons. What is the atomic number of the atom?
CorrectIncorrectHint
Atomic mass number \(=A=13, n=7\)
As \(A=n+p, p=A-n=13-7=6\)
Hence \(Z=p=6\) -
Question 28 of 36
28. Question
Match the following species with their corresponding ground state electronic configuration.
\(
\begin{array}{|l|l|}
\hline \text { Atom / ion } & \text { Electronic configuration } \\
\hline \text { (i) } \mathrm{Cu} & \text { (a) } 1 s^2 2 s^2 2 p^6 3 s^2 3 p^6 3 d^{10} \\
\hline \text { (ii) } \mathrm{Cu}^{2+} & \text { (b) } 1 s^2 2 s^2 2 p^6 3 s^2 3 p^6 3 d^{10} 4 s^2 \\
\hline \text { (iii) } Z n^{2+} & \text { (c) } 1 s^2 2 s^2 2 p^6 3 s^2 3 p^6 3 d^{10} 4 s^1 \\
\hline \text { (iv) } \mathrm{Cr}^{3+} & \text { (d) } 1 s^2 2 s^2 2 p^6 3 s^2 3 p^6 3 d^9 \\
\hline & \text { (e) } 1 s^2 2 s^2 2 p^6 3 s^2 3 p^6 3 d^3 \\
\hline
\end{array}
\)CorrectIncorrectHint
Hint: \(\mathrm{Cu}(\mathrm{Z}=29): 1 \mathrm{~s}^2 2 \mathrm{~s}^2 2 \mathrm{p}^6 3 \mathrm{~s}^2 3 \mathrm{p}^6 3 \mathrm{~d}^{10} 4 \mathrm{~s}^1\)
The electronic configuration of the given atom or ions are as follows:
A. \(\mathrm{Cu}(\mathrm{Z}=29): 1 \mathrm{~s}^2 2 \mathrm{~s}^2 2 \mathrm{p}^6 3 \mathrm{~s}^2 3 \mathrm{p}^6 3 \mathrm{~d}^{10} 4 \mathrm{~s}^1\)
B. \(\mathrm{Cu}^{2+}(\mathrm{Z}=29): 1 \mathrm{~s}^2 2 \mathrm{~s}^2 2 \mathrm{p}^6 3 \mathrm{~s}^2 3 \mathrm{p}^6 3 \mathrm{~d}^9\)
C. \(\mathrm{Zn}^{2+}(\mathrm{Z}=30): 1 \mathrm{~s}^2 2 \mathrm{~s}^2 2 \mathrm{p}^6 3 \mathrm{~s}^2 3 \mathrm{p}^6 3 \mathrm{~d}^{10}\)
D. \(\mathrm{Cr}^{3+}(\mathrm{Z}=24): 1 \mathrm{~s}^2 2 \mathrm{~s}^2 2 \mathrm{p}^6 3 \mathrm{~s}^2 3 \mathrm{p}^6 3 \mathrm{~d}^3\) -
Question 29 of 36
29. Question
Match the quantum numbers with the information provided by these.
\(
\begin{array}{|l|l|}
\hline \text { Quantum number } & \text { Information provided } \\
\hline \text { (i). Principal quantum number } & \text { a. Orientation of the orbital } \\
\hline \text { (ii). Azimuthal quantum number } & \text { b. Energy and size of orbital } \\
\hline \text { (iii). Magnetic quantum number } & \text { c. Spin of electron } \\
\hline \text { (iv). Spin quantum number } & \text { d. Shape of the orbital } \\
\hline
\end{array}
\)CorrectIncorrectHint
Hint: Orientation of the orbital is represented by m values.
Explanation:
(i). Principal quantum number is the most important quantum number as it determines the size and to large extent the energy of the orbital.
(ii). Azimuthal quantum number determines the angular momentum of the electron and defines the three-dimensional shape of the orbital.
(iii). Magnetic quantum number gives information about the spatial orientation of orbitals with respect to a standard set of coordinate axes.
(iv). Spin quantum number arises from the spectral evidence that an electron in its motion around the nucleus in an orbit also rotates or spin about its own axis. -
Question 30 of 36
30. Question
Match the following rules with their statements:
\(
\begin{array}{|l|l|}
\hline {\text { Rules }} & {\text { Statements }} \\
\hline \text { (i) Hund’s Rule } & \begin{array}{l}
\text { (a) No two electrons in an atom can have the same set of four } \\
\text { quantum numbers. }
\end{array} \\
\hline \text { (ii) Aufbau Principle } & \text { (b) Half – filled and completely filled orbitals have extra stability. } \\
\hline \begin{array}{l}
\text { (iii) Pauli Exclusion } \\
\text { Principle }
\end{array} & \begin{array}{l}
\text { (c) The pairing of electrons in the orbitals belonging to the same } \\
\text { subshell does not take place until each orbital is singly occupied. }
\end{array} \\
\hline \text { (iv) Heisenberg’s Uncertainty Principle } & \begin{array}{l}
\text { (d) It is impossible to determine the exact position and exact } \\
\text { momentum of a subatomic particle simultaneously. }
\end{array} \\
\hline & \begin{array}{l}
\text { (e) In the ground state of atoms, orbitals are filled in the order of } \\
\text { their increasing energies. }
\end{array} \\
\hline
\end{array}
\)CorrectIncorrectHint
Hint: Heisenberg’s Uncertainty Principle states that it is impossible to determine the exact position and exact momentum of a subatomic particle simultaneously.
Explanation:
(i) Hund’s rule states that pairing of electrons in the orbitals belonging to the same subshell ( \(p\), \(d\) or \(f\) ) does not take place until each orbital belonging to that subshell has one electron each i.e., it is singly occupied.
(ii) Aufbau principle states that in the ground state of the atoms, the orbitals are filled in order of their increasing energies.
(iii) According to the Pauli exclusion principle, no two electrons in an atom can have the same set of four quantum numbers.
(iv) Heisenberg’s uncertainty principle states that it is impossible to determine the exact position and exact momentum of a subatomic particle simultaneously. -
Question 31 of 36
31. Question
Match the following
\(
\begin{array}{|l|l|}
\hline \text { (i) } X \text {-rays } & \text { (a) } f=10^0-10^4 \mathrm{~Hz} \\
\hline \text { (ii) UV } & \text { (b) } f=10^{10} \mathrm{~Hz} \\
\hline \text { (iii) Long radio waves } & \text { (c) } f=10^{16} \mathrm{H} \\
\hline \text { (iv) Microwave } & \text { (d) } f=10^{18} \mathrm{H} \\
\hline
\end{array}
\)CorrectIncorrectHint
\(
\begin{array}{lll}
\text { Name } & \text { Frequency } & \text { Uses } \\
\text { (i) X-rays } & 2 \times 10^{16}-3 \times 10^{19} & \text { Medical pictures, medical } \\
& \mathrm{Hz} & \text { testing }
\end{array}
\)
\(
\text { (ii) Ultraviolet wave (UV) } 7.9 \times 10^{14}-2 \times 10^{16} \text { Germicidal lamp }
\)
\(
\begin{array}{lll}
\text { (iii) Long radio waves } & 10^0-10^4 \mathrm{~Hz} & \text { Signal transmission } \\
\text { (iv) Microwave } & 1 \times 10^9-5 \times 10^{11} & \text { Cooking radar }
\end{array}
\) -
Question 32 of 36
32. Question
Match the following
\(
\begin{array}{|l|l|}
\hline \text { (i) Photon } & \text { (a) Value is } 4 \text { for } N \text { shell } \\
\hline \text { (ii) Electron } & \text { (b) Probability density } \\
\hline \text { (iii) } \psi^2 & \text { (c) Always positive value } \\
\hline \text { (iv) Principal quantum number } n & \text { (d) Exhibits both momentum and wavelength } \\
\hline
\end{array}
\)CorrectIncorrectHint
Hint: \(\psi^2\) tells about probability density
(i) Photon has particle nature as well as wave nature. It exhibits both momentum and wavelength.
(ii) Electron also has particle nature as well as wave nature. Thus, it also exhibits both momentum and wavelength.
(iii) \(\psi^2\) represents the probability density of electrons and always has positive values.
(iv) Principle quantum number \(\mathrm{n}=4\) for \(\mathrm{N}\)-shell.
K L M N
\(\mathrm{n}=1234\)
It always has positive values. -
Question 33 of 36
33. Question
Match species given in Column I with the electronic configuration given in Column II.
\(
\begin{array}{|c|c|}
\hline \text { Column I } & \text { Column II } \\
\hline \text { (i) } \mathrm{Cr} & {a. [\mathrm{Ar}] 3 \mathrm{~d}^8 4 \mathrm{~s}^0} \\
\hline \text { (ii) } \mathrm{Fe}^{2+} & {b. [\mathrm{Ar}] 3 \mathrm{~d}^{10} 4 \mathrm{~s}^1} \\
\hline \text { (iii) } \mathrm{Ni}^{2+} & {c. [\mathrm{Ar}] 3 \mathrm{~d}^6 4 \mathrm{~s}^0} \\
\hline {\text { (iv) } \mathrm{Cu}} & {d. [\mathrm{Ar}] 3 \mathrm{~d}^5 4 \mathrm{~s}^1} \\
\hline & {e. [\mathrm{Ar}] 3 \mathrm{~d}^6 4 \mathrm{~s}^2} \\
\hline
\end{array}
\)CorrectIncorrectHint
Hint: Electronic configuration of \(\mathrm{Cr}\) is \(1 \mathrm{~s}^2 2 \mathrm{~s}^2 2 \mathrm{p}^6 3 \mathrm{~s}^2 3 \mathrm{p}^6 3 \mathrm{~d}^5 4 \mathrm{~s}^1\)
(i) \(\mathrm{Cr}(\mathrm{Z}=24)=1 \mathrm{~s}^2 2 \mathrm{~s}^2 2 \mathrm{p}^6 3 \mathrm{~s}^2 3 \mathrm{p}^6 3 \mathrm{~d}^5 4 \mathrm{~s}^1=[\mathrm{Ar}] 3 \mathrm{~d}^5 4 \mathrm{~s}^1\)
(ii) \(\mathrm{Fe}^{2+}(\mathrm{Z}=26)=1 \mathrm{~s}^2 2 \mathrm{~s}^2 2 \mathrm{p}^6 3 \mathrm{~s}^2 3 \mathrm{p}^6 3 \mathrm{~d}^6 4 \mathrm{~s}^0=[\mathrm{Ar}] 3 \mathrm{~d}^6 4 \mathrm{~s}^0\)
(iii) \(\mathrm{Ni}^{2+}(\mathrm{Z}=28)=1 \mathrm{~s}^2 2 \mathrm{~s}^2 2 \mathrm{p}^6 3 \mathrm{~s}^2 3 \mathrm{p}^6 3 \mathrm{~d}^8 4 \mathrm{~s}^0=[\mathrm{Ar}] 3 \mathrm{~d}^8 4 \mathrm{~s}^0\)
(iv) \(\mathrm{Cu}(\mathrm{Z}=29)=1 \mathrm{~s}^2 2 \mathrm{~s}^2 2 \mathrm{p}^6 3 \mathrm{~s}^2 3 \mathrm{p}^6 3 \mathrm{~d}^{10} 4 \mathrm{~s}^1=[\mathrm{Ar}] 3 \mathrm{~d}^{10} 4 \mathrm{~s}^1\) -
Question 34 of 36
34. Question
In the following questions a statement of Assertion (A) followed by a statement of Reason (R) is given. Choose the correct option out of the choices given below each question.
Assertion (A): All isotopes of a given element show the same type of chemical behaviour.
Reason (R): The chemical properties of an atom are controlled by the number of electrons in the atom.CorrectIncorrectHint
(a) Hint Chemical property depends on the number of electrons
Atoms with the same atomic number but with different atomic masses are called isotopes. In isotopes, a number of electrons are the same and these electrons are responsible for their chemical behaviour.
Hence, isotopes exhibit similar chemical properties -
Question 35 of 36
35. Question
In the following questions a statement of Assertion (A) followed by a statement of Reason (R) is given. Choose the correct option out of the choices given below each question.
Assertion (A) : Black body is an ideal body that emits and absorbs radiations of all frequencies.
Reason (R) : The frequency of radiation emitted by a body goes from a lower frequency to higher frequency with an increase in temperature.CorrectIncorrectHint
(b) Hint: With the temperature intensity of light increases.
A body that absorbs and emits all radiations falling on it is called a perfect black body. With rise in temperature, the frequency of radiation emitted by the body increases. -
Question 36 of 36
36. Question
In the following questions a statement of Assertion (A) followed by a statement of Reason (R) is given. Choose the correct option out of the choices given below each question.
Assertion (A): It is impossible to determine the exact position and exact momentum of an electron simultaneously.
Reason (R): The path of an electron in an atom is clearly defined.CorrectIncorrectHint
(iii) Hint: Heisenberg’s uncertainty principle.
Explanation:
According to Heisenberg’s uncertainty principle, the exact position and exact momentum of an electron cannot be determined simultaneously. The position of the electron and its path is not defined clearly in an atom.
Hence, the Assertion is true but the reason is false.