NCERT Exampler Questions
Quiz Summary
0 of 26 Questions completed
Questions:
Information
You have already completed the quiz before. Hence you can not start it again.
Quiz is loading…
You must sign in or sign up to start the quiz.
You must first complete the following:
Results
Results
0 of 26 Questions answered correctly
Your time:
Time has elapsed
You have reached 0 of 0 point(s), (0)
Earned Point(s): 0 of 0, (0)
0 Essay(s) Pending (Possible Point(s): 0)
Categories
- Not categorized 0%
- 1
- 2
- 3
- 4
- 5
- 6
- 7
- 8
- 9
- 10
- 11
- 12
- 13
- 14
- 15
- 16
- 17
- 18
- 19
- 20
- 21
- 22
- 23
- 24
- 25
- 26
- Current
- Review
- Answered
- Correct
- Incorrect
-
Question 1 of 26
1. Question
Thermodynamics is not concerned about ______.
CorrectIncorrectHint
Hint: Chemical kinetic tells about the rate of reaction
Thermodynamics is not concerned with the rate at which a reaction proceeds. Thermodynamics deals with the energy change, feasibility, and extent of a reaction, but not with the rate and mechanism of a process. The rate of reaction is dealt by kinetics. -
Question 2 of 26
2. Question
Which of the following statements is correct?
CorrectIncorrectHint
Hint: In a closed system, only an exchange of energy is taking place
(c) For a closed vessel made up of copper, no matter can be exchanged between the system and the surroundings but energy exchange can occur through its walls. Copper is a good conductor of heat. Thus, in a copper vessel, only heat can exchange but matter does not exchange. -
Question 3 of 26
3. Question
The state of a gas can be described by quoting the relationship between ______.
CorrectIncorrectHint
(d) State of a system can be described by state functions or state variables which are pressure, volume, temperature and amount of the gas ( \(P V=n R T)\).
-
Question 4 of 26
4. Question
The volume of gas is reduced to half from its original volume. The specific heat will be ______.
CorrectIncorrectHint
(c) The specific heat of a substance is the heat required to raise the temperature of \(1 \mathrm{gram}\) of a substance by one degree \(\left(1 \mathrm{~K}\right.\) or \(\left.1^{\circ} \mathrm{C}\right)\). It is an intensive property and is independent of the volume of the substance. The specific heat will remain constant.
-
Question 5 of 26
5. Question
During complete combustion of one mole of butane, \(2658 \mathrm{~kJ}\) of heat is released. The thermochemical reaction for above change is
CorrectIncorrectHint
HINT-Heat of combustion is heat released when 1 mole of any substance is burned in presence of oxygen.
(c) Exothermic reaction for combustion of one mole of butane is represented as:
\(
\mathrm{C}_4 \mathrm{H}_{10}(\mathrm{~g})+\frac{13}{2} \mathrm{O}_2(\mathrm{~g}) \longrightarrow 4 \mathrm{CO}_2(\mathrm{~g})+5 \mathrm{H}_2 \mathrm{O}(l) \Delta_{\mathrm{c}} H=-2658.0 \mathrm{~kJ} \mathrm{~mol}^{-1}
\)
Explanation:
Given that, the complete combustion of one mole of butane is represented by thermochemical reaction as
\(
\mathrm{C}_4 \mathrm{H}_{10}(\mathrm{~g})+\frac{13}{2} \mathrm{O}_2(\mathrm{~g}) \longrightarrow 4 \mathrm{CO}_2(\mathrm{~g})+5 \mathrm{H}_2 \mathrm{O}(l) \Delta_{\mathrm{c}} H=-2658.0 \mathrm{~kJ} \mathrm{~mol}^{-1}
\)
We have to take the combustion of one mole of \(\mathrm{C}_4 \mathrm{H}_{10}\) and \(\Delta_{\mathrm{c}} \mathrm{H}\) should be negative and have a value of \(2658 \mathrm{~kJ} \mathrm{~mol}^{-1}\) -
Question 6 of 26
6. Question
\(\Delta_f U^{\ominus}\) of formation of \(\mathrm{CH}_4(\mathrm{~g})\) at certain temperature is \(-393 \mathrm{~kJ} \mathrm{~mol}^{-1}\). The value of \(\Delta_f H^{\ominus}\) is
CorrectIncorrectHint
\(
\Delta_{\mathrm{f}} \mathrm{H}^{\ominus}=\Delta_{\mathrm{f}} \mathrm{U}^{\ominus}+\Delta \mathrm{n}_{\mathrm{g}} \mathrm{RT}
\)
The reaction is
The formula is as follows:
\(
\Delta_{\mathrm{f}} \mathrm{H}^{\ominus}=\Delta_{\mathrm{f}} \mathrm{U}^{\ominus}+\Delta \mathrm{n}_{\mathrm{g}} \mathrm{RT}
\)
\(
\text { For the given reaction, } \mathrm{C} \text { (graphite })+2 \mathrm{H}_2(\mathrm{~g}) \rightarrow \mathrm{CH}_4(\mathrm{~g})
\)
calculate the value of \(\Delta \mathrm{n}_{\mathrm{g}}\)
\(
\Delta \mathrm{n}_{\mathrm{g}}=\left(\mathrm{n}_{\mathrm{p}}-\mathrm{n}_{\mathrm{r}}\right)_{\mathrm{g}}=1-2=-1
\)
\(
\Delta_{\mathrm{f}} \mathrm{H}^{\ominus}=\Delta_{\mathrm{f}} \mathrm{U}^{\ominus}+\Delta \mathrm{n}_{\mathrm{g}} \mathrm{RT}
\)
\(
\Delta_{\mathrm{f}} \mathrm{H}^{\ominus}=\Delta_{\mathrm{f}} \mathrm{U}^{\ominus}-1 \mathrm{RT}
\)
Therefore, \(
\Delta_{\mathrm{f}} \mathrm{H}^{\ominus}<\Delta_{\mathrm{f}} \mathrm{U}^{\ominus}
\) -
Question 7 of 26
7. Question
In an adiabatic process, no transfer of heat takes place between system and surroundings. Choose the correct option for free expansion of an ideal gas under adiabatic condition from the following.
CorrectIncorrectHint
(c) For free expansion \(w=0\) For adiabatic process \(q=0\) From the first law of thermodynamics,
\(
\begin{aligned}
& \Delta U=q+W \\
& =0+0=0
\end{aligned}
\)
Since there is no change of internal energy, hence temperature will also remain constant, i.e., \(\Delta T=0\) -
Question 8 of 26
8. Question
The pressure-volume work for an ideal gas can be calculated by using the expression \(\mathrm{w}=-\int_{V_i}^{V_f} p_{e x} d V\). The work can also be calculated from the \(p \mathrm{V}-\) plot by using the area under the curve within the specified limits. When an ideal gas is compressed (a) reversibly or (b) irreversibly from volume \(\mathrm{V}_i\) to \(\mathrm{V}_f\). choose the correct option.
CorrectIncorrectHint
Hint: For compression, work done for the reversible process is less than the irreversible process
The correct option is W (reversible) < W (irreversible). This is because the area under the curve is always more in irreversible compression as can be seen from the given figure.
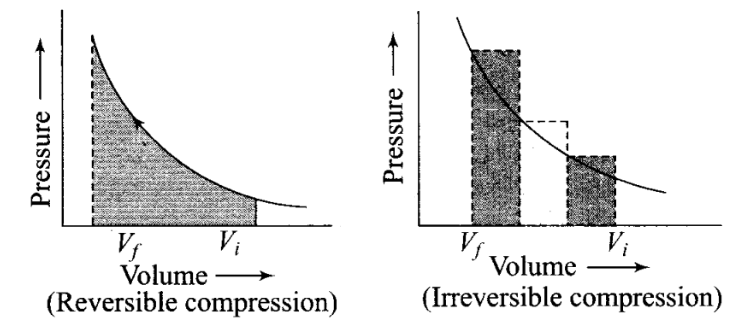
The area under the curve is greater in irreversible compression than that of reversible compression. -
Question 9 of 26
9. Question
The entropy change can be calculated by using the expression \(\Delta S=\frac{q_{\text {rev }}}{T}\). When water freezes in a glass beaker, choose the correct statement amongst the following:
CorrectIncorrectHint
(c) During the process of freezing energy is released, which is absorbed by the surroundings.
\(
\begin{aligned}
\therefore \quad & \Delta S_{\text {system }}=-\frac{q_{\text {rev }}}{T} ; \\
& \Delta S_{\text {surroundings }}=\frac{q_{\text {rev }}}{T}
\end{aligned}
\)Therefore, the entropy of the system decreases and that of the surroundings increases.
-
Question 10 of 26
10. Question
On the basis of thermochemical equations (a), (b) and (c), find out which of the algebric relationships given in options (i) to (iv) is correct.
\(
\text { (a) } \mathrm{C} \text { (graphite) }+\mathrm{O}_2 \text { (g) } \longrightarrow \mathrm{CO}_2(\mathrm{~g}) ; \Delta_r H=x \mathrm{~kJ} \mathrm{~mol}^{-1}
\)
\(
\text { (b) } \mathrm{C} \text { (graphite) }+\frac{1}{2} \mathrm{O}_2(\mathrm{~g}) \longrightarrow \mathrm{CO}(\mathrm{g}) ; \Delta_r H=y \mathrm{~kJ} \mathrm{~mol}^{-1}
\)
\(
\text { (c) } \mathrm{CO}(\mathrm{g})+\frac{1}{2} \mathrm{O}_2(\mathrm{~g}) \longrightarrow \mathrm{CO}_2(\mathrm{~g}) ; \Delta_r H=z \mathrm{~kJ} \mathrm{~mol}^{-1}
\)CorrectIncorrectHint
\(
\text { (c) } \mathrm{C} \text { (graphite) }+\mathrm{O}_2(\mathrm{~g}) \longrightarrow \mathrm{CO}_2(\mathrm{~g}) ; \quad \Delta_r H=x \mathrm{~kJ} \mathrm{~mol}^{-1} \dots(i)
\)
\(
\mathrm{C} \text { (graphite) }+\frac{1}{2} \mathrm{O}_2(\mathrm{~g}) \longrightarrow \mathrm{CO}(\mathrm{g}); \quad \Delta_r H=y \mathrm{~kJ} \mathrm{~mol}^{-1} \dots(ii)
\)
Subtracting equations (i) and (ii) we get
\(
\mathrm{CO}(\mathrm{g})+\frac{1}{2} \mathrm{O}_2(\mathrm{~g}) \longrightarrow \mathrm{CO}_2(\mathrm{~g}) ; \quad \Delta_r H=z \mathrm{~kJ} \mathrm{~mol}^{-1} \dots(iii)
\)
Equation (iii) is obtained by subtracting (ii) from (i) Therefore,
\(
\begin{aligned}
z & =x-y \\
\text { or } \quad x & =y+z
\end{aligned}
\) -
Question 11 of 26
11. Question
Consider the reactions given below. On the basis of these reactions find out which of the algebric relations given in options (i) to (iv) is correct?
\(
\text { (a) } \mathrm{C}(\mathrm{g})+4 \mathrm{H}(\mathrm{g}) \longrightarrow \mathrm{CH}_4(\mathrm{~g}) ; \Delta_r H=x \mathrm{~kJ} \mathrm{~mol}^{-1}
\)
\(
\text { (b) } \mathrm{C} \text { (graphite,s) }+2 \mathrm{H}_2(\mathrm{~g}) \longrightarrow \mathrm{CH}_4(\mathrm{~g}) ; \Delta_r H=y \mathrm{~kJ} \mathrm{~mol}^{-1}
\)CorrectIncorrectHint
c) \(x>y\) because the same bonds are formed in reactions (i) and (ii) but bonds between reactant molecules are broken only in reaction (ii). As energy is absorbed when bonds are broken, energy released in reaction (i) is greater than that in reaction (ii).
Alternate:
Hint: Enthalpy of formation of the compound can be positive or negative depending on the type of reaction.
Explanation:
In a chemical reaction, the reactant first converts into its atomic state, then combined, and formed a product.
In the first reaction, \(\mathrm{C}\) and \(\mathrm{H}\) are present in their atomic state hence they will combine and released \(\mathrm{X}\) amount of energy.
In the second reaction, \(\mathrm{H}_2\) molecule first converts into atomic hydrogen, then combined with a carbon atom and formed a product.
The overall \(\Delta \mathrm{H}_{\mathrm{r}}\) for both reactions are as follows:
i. \(\Delta H_r=x=-\) energy of the bonds being formed (four \(\mathrm{C}-\mathrm{H}\) bond formed)
ii. \(\Delta \mathrm{H}_{\mathrm{r}}=\mathrm{y}=\) energy of the bond being broken \((\mathrm{H}-\mathrm{H}\) bond break) – energy of the bonds being formed (four \(\mathrm{C}-\mathrm{H}\) bond formed)
In both the reaction’s energy of the bonds being formed is equal but in the second reaction, extra energy is used to break the hydrogen molecule bond. Thus, \(x>y\). -
Question 12 of 26
12. Question
The enthalpies of elements in their standard states are taken as zero. The enthalpy of formation of a compound
CorrectIncorrectHint
Hint: In the formation reaction energy always release.
Step 1:
The standard enthalpy change for the formation of one mole of a compound from its elements in their most stable states of aggregation (also known as reference states) is called the Standard Molar Enthalpy of Formation.Step 2:
The enthalpy of formation is always negative because when the compound is formed from its elements in their most stable states of aggregation then energy always releases. It is an exothermic reactionHence, enthalpy of formation is generally negative.
Alternate:
(c) Heat of formation of a compound may be positive or negative, e.g.,
\(
\mathrm{C}(\mathrm{s})+\mathrm{O}_2(\mathrm{~g}) \rightarrow \mathrm{CO}_2(\mathrm{~g}) ; \quad \Delta H^{\ominus}=-393.5 \mathrm{~kJ} \mathrm{~mol}^{-1}
\)
\(
\mathrm{N}_2(\mathrm{~g})+\frac{1}{2} \mathrm{O}_2(\mathrm{~g}) \rightarrow \mathrm{N}_2 \mathrm{O}(\mathrm{g}) ; \quad \Delta H^{\ominus}=+92 \mathrm{~kJ} \mathrm{~mol}^{-1}
\) -
Question 13 of 26
13. Question
Enthalpy of sublimation of a substance is equal to
CorrectIncorrectHint
(a) Enthalpy of sublimation of a substance is equal to enthalpy of fusion + enthalpy of vapourisation. Sublimation is the direct conversion of solid to vapour, i.e., solid \(\rightarrow\) vapour Writing in two steps, we have solid \(\rightarrow\) liquid \(\rightarrow\) vapour.
Solid \(\rightarrow\) liquid requires enthalpy of fusion.
Liquid \(\rightarrow\) vapour requires enthalpy of vapourisationAlternate:
Hint: Sublimation reaction in which solid substsnce directly convert into gas formed.
Step 1:
Sublimation process is a direct conversion of solid to vapour; solid \(\rightarrow\) vapour
Standard enthalpy of sublimation is the change in enthalpy when one mole of a solid substance sublimes at a constant temperature and under standard pressure (1bar).Step 2:
The sublimation process occurs in two steps, we have solid \(\rightarrow\) liquid \(\rightarrow\) vapour
solid \(\rightarrow\) liquid requires enthalpy of fusion and liquid \(\rightarrow\) vapour requires enthalpy of vaporisation. -
Question 14 of 26
14. Question
Which of the following is not correct?
CorrectIncorrectHint
Hint: For spontaneous reaction \(\Delta \mathrm{G}\) value is negative
\(\Delta \mathrm{G}\) gives criteria for spontaneity at constant pressure and temperature.
(i) If \(\Delta \mathrm{G}\) is negative ( \(<0\) ), the process is spontaneous.
(ii) If \(\Delta \mathrm{G}\) is positive ( \(>0\) ), the process is non-spontaneous
(iii) If \(\Delta \mathrm{G}\) is zero then the reaction is equilibrium. -
Question 15 of 26
15. Question
Thermodynamics mainly deals with
CorrectIncorrectHint
(a,d) Thermodynamics deals with the interrelation of various forms of energy and their transformation into each other. It also deals with thermal or mechanical equilibrium. However, if does not tell anything about the rate of reaction.
-
Question 16 of 26
16. Question
In an exothermic reaction, heat is evolved, and system loses heat to the surrounding. For such system
CorrectIncorrectHint
\(
\text { (a, b) For an exothermic reaction, } q_p=-v e, \Delta_r H=-v e
\)Exothermic reactions are those reactions which are accompanied by the evolution of heat.
e.g.,
\(
\begin{aligned}
& \mathrm{C}(\mathrm{s})+\mathrm{O}_2(\mathrm{~g}) \rightarrow \mathrm{CO}_2(\mathrm{~g})+393.5 \mathrm{~kJ} \\
& \mathrm{H}_2(\mathrm{~g})+\frac{1}{2} \mathrm{O}_2(\mathrm{~g}) \rightarrow \mathrm{H}_2 \mathrm{O}(\mathrm{l})+285.8 \mathrm{~kJ}
\end{aligned}
\)
\(\mathrm{q}_{\mathrm{p}}\) and \(\Delta_{\mathrm{r}} \mathrm{H}\) are negative for an exothermic reaction. -
Question 17 of 26
17. Question
The spontaneity means, having the potential to proceed without the assistance of an external agency. The processes which occur spontaneously are
CorrectIncorrectHint
(c, d) Gas expands or diffuses in available space spontaneously, e.g., leakage of cooking gas gives smell of ethyl mercaptan spontaneously. Moreover, burning of carbon to \(\mathrm{CO}_2\) is also spontaneous.
Options (a) and (b) can neither occur by themselves nor by initiation, (c) can occur by itself, (d) occur on initiation. Flowing of heat from warmer to colder body, expanding of gas and buring of carbon to give carbon dioxide, all are spontaneous process.
-
Question 18 of 26
18. Question
For an ideal gas, the work of reversible expansion under isothermal conditions can be calculated by using the expression \(\mathrm{w}=-n \mathrm{R} T \ln \frac{V_f}{V_i}\)
A sample containing \(1.0 \mathrm{~mol}\) of an ideal gas is expanded isothermally and reversibly to ten times of its original volume, in two separate experiments. The expansion is carried out at \(300 \mathrm{~K}\) and at \(600 \mathrm{~K}\) respectively. Choose the correct option.CorrectIncorrectHint
Given that, the work of reversible expansion under isothermal condition can be calculated by using the expression
\(
\begin{aligned}
& \mathrm{W}=-\mathrm{nRT} \ln \frac{\mathrm{V}_{\mathrm{f}}}{\mathrm{V}_{\mathrm{i}}} \\
& \mathrm{V}_{\mathrm{f}}=10 \mathrm{~V}_{\mathrm{i}} \\
& \mathrm{T}_2=600 \mathrm{~K} \\
& \mathrm{~T}_1=300 \mathrm{~K}
\end{aligned}
\)
Putting these values in above expression
\(
\begin{aligned}
& \mathrm{W}_{600 \mathrm{~K}}=1 \times \mathrm{R} \times 600 \mathrm{~K} \ln \frac{10}{1} \\
& \mathrm{~W}_{300 \mathrm{~K}}=1 \times \mathrm{R} \times 300 \mathrm{~K} \ln \frac{10}{1} \\
& \text { Ration }=\frac{\mathrm{W}_{600 \mathrm{~K}}}{\mathrm{~W}_{300 \mathrm{~K}}}=\frac{1 \times \mathrm{R} \times 600 \mathrm{~K} \ln \frac{10}{1}}{1 \times \mathrm{R} \times 300 \mathrm{~K} \ln \frac{10}{1}}=\frac{600}{300}=2
\end{aligned}
\)
For isothermal expansion of ideal gases, \(\Delta \mathrm{U}=0\). Since, temperature is constant this means there is no change is internal energy. -
Question 19 of 26
19. Question
Consider the following reaction between zinc and oxygen and choose the correct options out of the options given below :
\(
2 \mathrm{Zn}(s)+\mathrm{O}_2(g) \longrightarrow 2 \mathrm{ZnO}(s) ; \quad \Delta \mathrm{H}=-693.8 \mathrm{~kJ} \mathrm{~mol}^{-1}
\)CorrectIncorrectHint
\(
\Delta_f H=\sum_i a_i \Delta_f H-\sum_i b_i \Delta_f H
\)
The reaction depicted above is exothermic in nature, and, hence, the reactant enthalpy is more than the product enthalpy.Alternate:
For the reaction,
\(
2 \mathrm{Zn}(\mathrm{s})+\mathrm{O}_2(\mathrm{~g}) \rightarrow 2 \mathrm{ZnO}(\mathrm{s}) ; \Delta \mathrm{H}=-693.8 \mathrm{~kJ} \mathrm{~mol}^{-1}
\)
As we know that, \(\Delta \mathrm{H}=\mathrm{H}_{\mathrm{p}}-\mathrm{H}_{\mathrm{R}}\)
A negative value of \(\Delta \mathrm{H}\) shows that \(\mathrm{H}_{\mathrm{R}}>\mathrm{H}_{\mathrm{P}}\) or \(\mathrm{H}_{\mathrm{P}}<\mathrm{H}_{\mathrm{R}}\), i.e., enthalpy of two moles of \(\mathrm{ZnO}\) is less than the enthalpy of two moles of zinc and one mole of oxygen by \(693.8 \mathrm{~kJ}\). As \(\mathrm{H}_{\mathrm{R}}>\mathrm{H}_{\mathrm{P}}, 693.8 \mathrm{~kJ} \mathrm{~mol}^{-1}\) of energy is evolved in the reaction. -
Question 20 of 26
20. Question
In the following questions, more than one correlation is possible between options of both columns.
Match the following:\(
\begin{array}{|c|c|}
\hline \text { A } & \text { B } \\
\hline \text { (i) Adiabatic Process } & \text { (a) Heat } \\
\hline \text { (ii) Isolated system } & \text { (b) At constant volume } \\
\hline \text { (iii) Isothermal change } & \begin{array}{l}
\text { (c) First law of } \\
\text { thermodynamics }
\end{array} \\
\hline \text { (iv) Path function } & \begin{array}{l}
\text { (d) No exchange of energy } \\
\text { and matter }
\end{array} \\
\hline \text { (v) State function } & \text { (e) No transfer of heat } \\
\hline \text { (vi) } \Delta U=q & \text { (f) Constant temperature } \\
\hline \begin{array}{l}
\text { (vii)Law of conservation of } \\
\text { energy }
\end{array} & \text { (g) Internal energy } \\
\hline \text { (viii) Reversible process } & \text { (h) } P_{\text {ext }}=0 \\
\hline \text { (ix) Free expansion } & \text { (i) At constant pressure } \\
\hline \text { (x) } \Delta H=q & \begin{array}{l}
\text { (j) Infinitely slow process } \\
\text { which } \\
\text { proceeds through a series of } \\
\text { equilibrium states. }
\end{array} \\
\hline \text { (xi) Intensive property } & \text { (k) Entropy } \\
\hline \text { (xii) Extensive property } & \text { (l) Pressure } \\
\hline & \text { (m) Specific heat } \\
\hline
\end{array}
\)CorrectIncorrectHint
(i) \(\rightarrow\) (e), (ii) \(\rightarrow\) (d), (iii) \(\rightarrow\) (f), (iv) \(\rightarrow\) (a), (v) \(\rightarrow\) (g), (k), (l) \(\quad\) (vi) \(\rightarrow\) (b), \(\quad\) (vii) \(\rightarrow\) (c), \(\quad\) (viii) \(\rightarrow\) (j), \((\mathrm{ix}) \rightarrow(\mathrm{h}), \quad(\mathrm{x}) \rightarrow\) (i), \(\quad\) (xi) \(\rightarrow\) (a), (1), (m), \(\quad\) (xii) \(\rightarrow(\mathrm{g}),(\mathrm{k})\)
-
Question 21 of 26
21. Question
Match the following processes with entropy change:
\(
\begin{array}{|l|l|}
\hline \text { Reaction } & \text { Entropy change } \\
\hline \text { (i) A liquid vapourises } & \text { (a) } \Delta \mathrm{S}=0 \\
\hline \text { (ii) Reaction is non-spontaneous at all temperatures and } \Delta \mathrm{H} \text { is positive } & \text { (b) } \Delta \mathrm{S}=\text { positive } \\
\hline \text { (iii) Reversible expansion of an } & \text { (c) } \Delta \mathrm{S}=\text { negative } \\
\hline
\end{array}
\)CorrectIncorrectHint
\(
\begin{array}{|l|l|l|}
\hline \text { Reaction } & \text { Entropy change } \\
\hline \text { i } & \text { A liquid vaporises } & \text { As a liquid change to gaseous state, the movement of molecules will increase and hence the entropy will also increase. } \\
\hline \text { ii } & \text { Reaction is non-spontaneous at all temperature and } \Delta H \text { is positive. } & \Delta G \text { is positive as the process in non-spontaneous. Also, } \Delta H \text { is positive. } \Delta S \text { is negative } \\
\hline \text { iii } & \text { Reversible expansion of an ideal gas } & \text { This process at every stage is always in equal } \\
\hline
\end{array}
\) -
Question 22 of 26
22. Question
Match the following parameters with description for spontaneity :
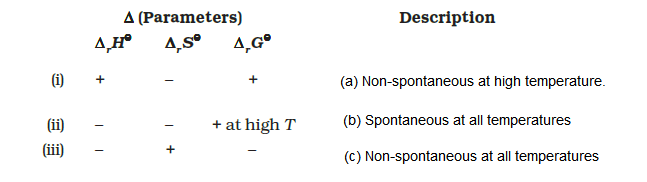 CorrectIncorrect
CorrectIncorrectHint
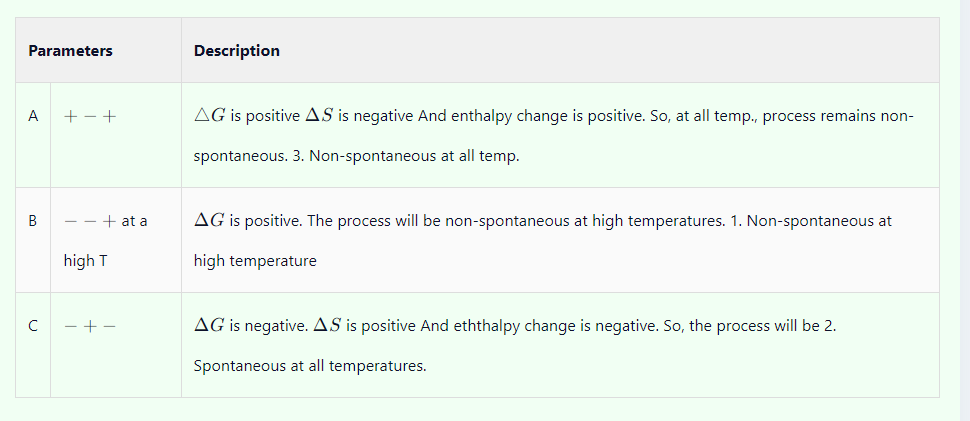
-
Question 23 of 26
23. Question
Match the following :
\(
\begin{array}{|l|l|}
\hline \text { (i) Entropy of vapourisation } & \text { (a) decreases } \\
\hline \text { (ii) } K \text { for spontaneous process } & \text { (b) is always positive } \\
\hline \text { (iii) Crystalline solid state } & \text { (c) lowest entropy } \\
\hline \text { (iv) } \Delta U \text { in adiabatic expansion of ideal gas } & \text { (d) } \Delta \mathrm{H}_{\text {vap }} / T_b \\
\hline
\end{array}
\)CorrectIncorrectHint
\(
\begin{array}{|l|l|l|l|}
\hline \text { i) } & \text { Entropy of vaporisation } & \text { b) } & \text { is always positive } \\
& & \text { d) } & \frac{\Delta H_{\text {vap }}}{T_b} \\
\hline \text { ii) } & \text { K for spontaneous process } & \text { b) } & \text { is alwasy positive } \\
\hline \text { iii) } & \text { Crystalline solid state } & \text { c) } & \text { lowest entropy } \\
\hline \text { iv) } & \Delta U \text { in adiabatic expansion of ideal gas } & \text { a) } & \text { decreases } \\
\hline
\end{array}
\) -
Question 24 of 26
24. Question
In the following questions, a statement of Assertion (A) followed by a statement of Reason (R) is given. Choose the correct option out of the choices given below each question.
Assertion (A): Combustion of all organic compounds is an exothermic reaction.
Reason R: The enthalpies of all elements in their standard state are zero.CorrectIncorrectHint
Hint: Combustion is an exothermic reaction
Combustion of organic compounds is always exothermic. Heat or enthalpy of the formation of natural elements in their standard the state is considered to be zero. Hence, both assertion and reason are true and the reason is not the correct explanation of assertion. -
Question 25 of 26
25. Question
In the following questions a statement of Assertion (A) followed by a statement of Reason (R) is given. Choose the correct option out of the choices given below each question.
Assertion (A) : Spontaneous process is an irreversible process and may be reversed by some external agency.
Reason (R) : Decrease in enthalpy is a contributory factor for spontaneity.CorrectIncorrectHint
Hint: For a spontaneous reaction, \(\Delta \mathrm{G}\) value is negative
Spontaneous processes are those that can proceed without any outside intervention. Spontaneous processes are irreversible.
For a spontaneous, \(\Delta \mathrm{H}\) value is negative and \(\Delta \mathrm{S}\) value is positive. Thus, a decrease in enthalpy is a contributing factor to
spontaneity. Hence, both assertion and reason are true and the reason is not the correct explanation of assertion. -
Question 26 of 26
26. Question
In the following questions, a statement of Assertion (A) followed by a statement of Reason (R) is given. Choose the correct option out of the choices given below each question.
Assertion (A): A liquid crystallises into a solid and is accompanied by decrease in entropy.
Reason (R): In crystals, molecules organise in an ordered manner.CorrectIncorrectHint
Hint: A liquid crystallises into a solid and is accompanied by a decrease in entropy.
When a liquid crystallises, entropy decreases because in crystalline form the molecules are more ordered as compared to the liquid. The movement of atoms is a solid form restricted hence, entropy decreases.Hence, both assertion and reason are true and the reason is the correct explanation of assertion.