Jee Math Test Series Complex Numbers Quiz-1
Multiple choice questions with one correct answer
Quiz Summary
0 of 50 Questions completed
Questions:
Information
You have already completed the quiz before. Hence you can not start it again.
Quiz is loading…
You must sign in or sign up to start the quiz.
You must first complete the following:
Results
Results
0 of 50 Questions answered correctly
Your time:
Time has elapsed
You have reached 0 of 0 point(s), (0)
Earned Point(s): 0 of 0, (0)
0 Essay(s) Pending (Possible Point(s): 0)
Categories
- Not categorized 0%
- 1
- 2
- 3
- 4
- 5
- 6
- 7
- 8
- 9
- 10
- 11
- 12
- 13
- 14
- 15
- 16
- 17
- 18
- 19
- 20
- 21
- 22
- 23
- 24
- 25
- 26
- 27
- 28
- 29
- 30
- 31
- 32
- 33
- 34
- 35
- 36
- 37
- 38
- 39
- 40
- 41
- 42
- 43
- 44
- 45
- 46
- 47
- 48
- 49
- 50
- Current
- Review
- Answered
- Correct
- Incorrect
-
Question 1 of 50
1. Question
1 point(s)If \((1+i)^{2 n}+(1-i)^{2 n}=-2^{n+1}\) (where, \(i=\sqrt{-1}\) ) for all those \(n\), which are
CorrectIncorrectHint
\(
\begin{aligned}
& \text { In }(1+i)^{2 n}+(1-i)^{2 n} \\
& =\left\{(1+i)^2\right\}^n+\left\{(1-i)^2\right\}^n \\
& =\left(1+i^2+2 i\right)^n+\left(1+i^2-2 i\right)^n \\
& =(1-1+2 i)^n+(1-1-2 i)^n
\end{aligned}
\)
\(
=2^n i^n+i^n(-2)^n=\mathrm{i}^n
\)
For all values of n the given equation does not satisfy. -
Question 2 of 50
2. Question
1 point(s)If \(i=\sqrt{-1}\) and \(n \in N\), then \(i^n+i^{n+1}+i^{n+2}+i^{n+3}\) is equal to
CorrectIncorrectHint
We have,
\(
i^n+i^{n+1}+i^{n+2}+i^{n+3}=i^n\left(1+i+i^2+i^3\right)=i^n(1+i-1-i)=0
\) -
Question 3 of 50
3. Question
1 point(s)If \(i=\sqrt{-1}\), then \(\left\{i^n+i^{-n}: n \in Z\right\}\) is equal to
CorrectIncorrectHint
We have the following cases:
CASEI
When \(n=4 m, m \in Z\)
In this case, we have
\(
\begin{aligned}
& i^n=i^{4 m}=\left(i^4\right)^m=1 \text { and } i^{-n}=\frac{1}{i^n}=\frac{1}{1}=1 \\
\therefore \quad & i^n+i^{-n}=2
\end{aligned}
\)
CASE II
When \(n=4 m+1, m \in Z\) In this case, we have
\(
\begin{aligned}
& i^n=i^{4 m+1}=\left(i^4\right)^m i^1=i \text { and, } i^{-n}=\frac{1}{i^n}=\frac{1}{i}=-i \\
\therefore \quad & i^n+i^{-n}=i-i=0
\end{aligned}
\)
CASE III
When \(n=4 m+2, m \in Z\)
In this case, we have
\(
\begin{aligned}
& i^n=i^{4 m+2}=\left(i^4\right)^m i^2=i^2=-1 \\
\therefore \quad & i^n+i^n=(-1)+(-1)=-2
\end{aligned}
\)
CASE IV
When \(n=4 m+3, m \in Z\)
In this case, we have
\(
\begin{aligned}
& \quad i^n=i^{4 m+3}=\left(i^4\right)^m i^3=i^3=-i \text { and, } i^{-n}=\frac{1}{i^n}=\frac{1}{i^3}=i \\
& \therefore \quad i^n+i^{-n}=(-i)+(i)=0 \\
& \text { Hence, } S=(-2,0,2)
\end{aligned}
\) -
Question 4 of 50
4. Question
1 point(s)The value of \(\sum_{n=1}^{13}\left(i^n+i^{n+1}\right)\), where \(i=\sqrt{-1}\) equals
CorrectIncorrectHint
We have,
\(
\begin{aligned}
& \sum_{n=1}^{13}\left(i^n+i^{n+1}\right) \\
& =\sum_{n=1}^{13} i^n+\sum_{n=1}^{13} i^{n+1} \\
& =i\left(\frac{1-i^{13}}{1-i}\right)+i^2\left(\frac{1-i^{13}}{1-i}\right)=i \frac{(1-i)}{1-i}-\left(\frac{1-i}{1-i}\right)=i-1
\end{aligned}
\) -
Question 5 of 50
5. Question
1 point(s)If \(n\) is an odd integer, then \((1+i)^{6 n}+(1-i)\) is equal to
CorrectIncorrectHint
We have,
\(
\begin{aligned}
& (1+i)^{6 n}+(1-i)^{6 n}=\left\{(1+i)^2\right\}^{3 n}+\left\{(1-i)^2\right\}^{3 n} \\
& =(2 i)^{3 n}+(-2 i)^{3 n}=2^{3 n}\left\{i^{3 n}+(-i)^{3 n}\right\} \\
& =2^{3 n}\left\{i^{3 n}-i^{3 n}\right\}=0
\end{aligned}
\) -
Question 6 of 50
6. Question
1 point(s)Which of the following is correct?
CorrectIncorrectHint
The notion of linear ordering, greater than or less than, does not apply to complex numbers.
Thus the statement \(Z_1<Z_2\) and \(Z_1>Z_2\) have no meaning unless \(Z_1\) and \(Z_2\) are both real.
-
Question 7 of 50
7. Question
1 point(s)Let \(z_1, z_2\) be two complex numbers such that \(z_1+z_2\) and \(z_1 z_2\) both are real, then
CorrectIncorrectHint
Given, \(z_1+z_2\) and \(z_1 z_2\) are real.
Let, \(z_1=a+i b, z_2=c+i d\)
Since, \(z_1+z_2\) is real,
\(
\begin{array}{lll}
\Rightarrow & (a+c)+i(b+d) \text { is real } & \\
\Rightarrow b+d & =0 & \left[\mathrm{z}_1+\mathrm{z}_2 \text { is real }\right] \\
\Rightarrow \quad b & =-d & \ldots \ldots(1)
\end{array}
\)
Also, \(z_1 z_2\) is real,
\(
\begin{aligned}
& =(a+i b) \cdot(c+i d) \text { is real } \\
& =a c+i a d+i b c+i^2 b d \text { is real } \\
& =a c+i(a d+b c)-b d \text { is real } \\
& =(a c-b d)+i(a d+b c) \text { is real } \\
& \Rightarrow \quad a d+b c=0 \\
& \Rightarrow a(-b)+b c=0 \\
& \Rightarrow \quad a=c \dots(2)
\end{aligned}
\)
Therefore,
\(
\begin{aligned}
z_1 & =a+i b & & \\
& =c-i d & & {[\text { From }(1) \text { and }(2)] } \\
& =\overline{z_2} & & {\left[\because z_2=c+i d\right] }
\end{aligned}
\) -
Question 8 of 50
8. Question
1 point(s)If the complex numbers \(z_1, z_2, z_3\) are in AP, then they lie on a
CorrectIncorrectHint
By definition of the arithmetic progression we know that if \(a, b, c\) are in A.P then, \(2 b=a+c\).
So, if \(z_1, z_2, z_3\) are in A.P then,
\(
\begin{gathered}
2 z_2=z_1+z_3 \\
z_2=\frac{z_1+z_3}{2}
\end{gathered}
\)
It means that \(z_2\) is the mid point of \(z_1, z_3\).
Therefore, \(z_1, z_2, z_3\) lie on a straight line. -
Question 9 of 50
9. Question
1 point(s)The smallest positive integer \(n\) for which \(\left(\frac{1+i}{1-i}\right)^n=1\) is
CorrectIncorrectHint
Given,
\(
\left(\frac{1+i}{1-i}\right)^n=1
\)
Multiply and divide numerator by \((1+i)^n\) we get
\(
\begin{aligned}
& \left(\frac{(1+i)(1+i)}{(1+i)(1-i)}\right)^n=1 \\
& \Rightarrow\left(\frac{1+i^2+2 i}{1-i^2}\right)^n=1\left(\because i^2=-1\right) \\
& \Rightarrow\left(\frac{2 i}{2}\right)^n=1 \\
& \Rightarrow i^n=1
\end{aligned}
\)
Hence, the smallest value of \(n\) for this is 4 . -
Question 10 of 50
10. Question
1 point(s)The locus of the point \(z\) satisfying arg \(\left(\frac{z-1}{z+1}\right)=k\), where \(k\) is non-zero real, is
CorrectIncorrectHint
We have, \(\arg \left(\frac{z-1}{z+1}\right)=k,(k \neq 0)\)
Put \(z=x+i y\), we get
\(
\begin{aligned}
& \arg \left(\frac{x-1+i y}{x+1+i y}\right)=k \\
& \Rightarrow \arg \left[\frac{(x-1+i y)(x+1-i y)}{(x+1+i y)(x+1-i y)}\right]=k \\
& \Rightarrow \arg \left[\frac{\left(x^2-1\right)+i y(2)+y^2}{(x+1)^2+y^2}\right]=k \\
& \Rightarrow \arg \left[\frac{x^2+y^2-1+2 i y}{(x+1)^2+y^2}\right]=k \\
& \Rightarrow \arg \left[\frac{x^2+y^2-1+2 i y}{(x+1)^2+y^2}\right]=k \\
& \Rightarrow \frac{2 y}{x^2+y^2-1}=k \\
& {\left[\because \arg (z)=\tan ^{-1} \frac{y}{x}\right]} \\
& \Rightarrow\left(x^2+y^2\right) k-k-2 y=0 \\
& \Rightarrow k x^2+k y^2-2 y-k=0 \\
& \Rightarrow x^2+y^2-\frac{2 y}{k}-1=0
\end{aligned}
\)
Which represent a circle of centre \(\left(0, \frac{1}{k}\right)\) i.e. on \(y\)-axis. -
Question 11 of 50
11. Question
1 point(s)If \(\sqrt{x+i y}= \pm(a+i b)\), then \(\sqrt{-x-i y}\) is equal to
CorrectIncorrectHint
\(
\begin{aligned}
& \sqrt{x+i y}=a+i b \\
& \Rightarrow x=a^2-b^2 \\
& y=2 a b \\
& \sqrt{-x-i y} \\
& =\sqrt{b^2-a^2-2 a b i} \\
& =\sqrt{(b-i a)^2}= \pm(b-i a)
\end{aligned}
\) -
Question 12 of 50
12. Question
1 point(s)The locus of the points \(z\) satisfying the condition \(\arg \left(\frac{z-1}{z+1}\right)=\frac{\pi}{3}\) is a
CorrectIncorrectHint
Given, \(\arg \left(\frac{z-1}{z+1}\right)=\frac{\pi}{3}\)
\(
\begin{aligned}
& \text { Let } z=x+i y \therefore \frac{z-1}{z+1}=\frac{x+i y-1}{x+i y+1} \\
& =\frac{x^2+y^2-1+2 i y}{(x+1)^2+y^2} \\
& \therefore \arg \left(\frac{z-1}{z+1}\right)=\tan ^{-1} \frac{2 y}{x^2+y^2-1}=\frac{\pi}{3} \\
& \Rightarrow \frac{2 y}{x^2+y^2-1}=\tan \frac{\pi}{3}=\sqrt{3} \\
& \Rightarrow x^2+y^2-1=\frac{2}{\sqrt{3}} y \\
& \Rightarrow x^2+y^2-\frac{2}{\sqrt{3}} y-1=0
\end{aligned}
\)
Which is the equation of circle. -
Question 13 of 50
13. Question
1 point(s)If \((\sqrt{3}+i)^{10}=a+i b\), then \(a\) and \(b\) are respectively
CorrectIncorrectHint
We have,
\(
\begin{aligned}
& (\sqrt{3}+i)^{10}=a+i b \\
& \Rightarrow i^{10}(1-i \sqrt{3})^{10}=a+i b \\
& \Rightarrow-(-2 \omega)^{10}=a+i b \quad\left[\because \omega=-\frac{1}{2}+i \frac{\sqrt{3}}{2}\right] \\
& \Rightarrow-2^{10} \omega^{10}=a+i b \\
& \Rightarrow-2^{10} \omega=a+i b \\
& \Rightarrow-2^{10}\left(\frac{-1}{2}+i \frac{\sqrt{3}}{2}\right)=a+i b \\
& \Rightarrow 2^9-2^9 \sqrt{3} i=a+i b \Rightarrow a=2^9 \text { and } \\
& b=-2^9 \sqrt{3}
\end{aligned}
\) -
Question 14 of 50
14. Question
1 point(s)If \(\operatorname{Re}\left(\frac{z-8 i}{z+6}\right)=0\), then \(z\) lies on the curve
CorrectIncorrectHint
If \(z\) is a complex number then
\(
2+\bar{z}=2 \operatorname{Re}(z)
\)
Eqn of a circle in complex form \(\quad|z|^2+\alpha \bar{z}+\bar{\alpha} z+k=0\)
center \(=-\alpha\), radius \(\sqrt{|\alpha|^2-k}\)
\(
2 \operatorname{Re}\left(\frac{z-8 i}{z+6}\right)=0
\)
\(
\frac{z-8 i}{z+6}+\overline {\left(\frac{z-8 i}{z+6}\right)}
\)
\(
\frac{z-8 i}{z+6}+\frac{\bar{z}+8 i}{\bar{z}+6}=0
\)
\(
\frac{2 z \bar{z}-8 i \bar{z}-48 i+8 i z+6 \bar{z}+48 i}{(z+6)(\bar{z}+6)}=0
\)
\(
\begin{aligned}
& z \bar{z}=|z|^2 \\
& 2|z|^2+z(6+8 i)+\bar{z}(6-8 i)=0 \\
& |z|^2+(3+4 i) z+(3-4 i) \bar{z}=0 \\
& 3-4 i=\alpha \\
& |z|^2+\bar{\alpha} z+\alpha \bar{z}=0
\end{aligned}
\)
\(
\begin{aligned}
\text { center }= & -\alpha, \quad-3+4 i \\
& \text { point form } (-3,4)
\end{aligned}
\)
\(
\gamma=\sqrt{|\alpha|^2}=\sqrt{3^2+4^2}=5
\)
\(
(x+3)^2+(y-4)^2=25 \Rightarrow x^2+y^2+6 x-8 y+25=25
\)
\(
x^2+y^2+6 x-8 y=0
\) -
Question 15 of 50
15. Question
1 point(s)If \(z=\left(\frac{\sqrt{3}}{2}+\frac{i}{2}\right)^5+\left(\frac{\sqrt{3}}{2}-\frac{i}{2}\right)^5\), then
CorrectIncorrectHint
\(
\begin{aligned}
& z=\left(\frac{\sqrt{3}}{2}+\frac{i}{2}\right)^5+\left(\frac{\sqrt{3}}{2}-\frac{i}{2}\right)^5 \\
& z=\left(e^{\frac{i \pi}{6}}\right)^5+\left(e^{\frac{-i \pi}{6}}\right)^5 \\
&=e^{i \frac{5 \pi}{6}}+e^{-\frac{5 \pi}{6}} \\
&=\cos \frac{5 \pi}{6}+i \sin \frac{5 \pi}{6}+\cos \frac{5 \pi}{6}-i \sin \frac{5 \pi}{6} \\
& z=2 \cos \frac{5 \pi}{6} \\
& \operatorname{Im}(z)=0~ \& \operatorname{Re}(z)<0
\end{aligned}
\) -
Question 16 of 50
16. Question
1 point(s)If \(z=x+i y\) and \(z=\frac{1-i z}{z-i}\), then \(|w|=1\) implies that in the complex plane
CorrectIncorrectHint
We have,
\(
|\mathrm{w}|=1 \Longrightarrow\left|\frac{1-\mathrm{iz}}{\mathrm{z}-\mathrm{i}}\right|=1
\)
\(
\begin{aligned}
& |1-i z|=|z-i|(1) \\
& |1-i(x+i y)|=|x+i y-i|, \text { where } z=x+i y
\end{aligned}
\)
\(
|1+y-i x|=|x+i(y-1)|
\)
\(
\begin{aligned}
& \sqrt{(1+y)^2+(-x)^2}=\sqrt{x^2+(y-1)^1} \\
& (1+y)^2+x^2=x^2+(y-1)^2 \\
& y=0
\end{aligned}
\)
\(
\Longrightarrow \mathrm{z}={x}+{i \times 0}={x} \text {, which is purely real. }
\) -
Question 17 of 50
17. Question
1 point(s)Let \(3-i\) and \(2+i\) be affixes of two points \(A\) and \(B\) in the argand plane and \(P\) represents the complex number \(z=x+i y\). Then, the locus of \(P\) if \(|z-3+i|=|z-2-i|\) is
CorrectIncorrectHint
We have a complex number \(z=x+i y\)
\(
\begin{aligned}
& \text { and }|z-3+i|=|z-2-i| \\
& \Rightarrow|x+i y-3+i|=|x+i y-2-i| \\
& \Rightarrow(x-3)^2+(y+1)^2=(x-2)^2+(y-1)^2 \\
& \Rightarrow x^3-6 x+9+y^2+2 y+1 \\
& =x^2-4 x+4+y^2-2 y+1 \\
& \Rightarrow-2 x+4 y+5=0 \ldots(i)
\end{aligned}
\)
So, it represent a line
Point \(A(3,-1)\) and \(B(2,1)\)
So, mid-point of \(A B=\left(\frac{5}{2}, 0\right)\)
\(
m_1=\text { slope of } A B=\frac{1-(-1)}{2-3}=-2
\)
Point \(\left(\frac{5}{2}, 0\right)\) satisfies the equation \(-2 x+4 y+5=0\)
and slope of line \(=m_2=\frac{1}{2}\)
Now, \(m_1 m_2=-2 \times \frac{1}{2}=-1\)
So, line \(-2 x+4 y+5\) is perpendicular to \(A B\). Hence, locus of point \(p\) is the perpendicular bisector of \(A B\) -
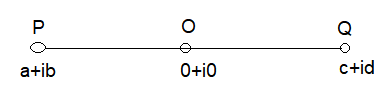
Question 18 of 50
18. Question
1 point(s)\(P O Q\) is a straight line through the origin \(O . P\) and \(Q\) represent the complex numbers \(a+i b\) and \(c+i d\) respectively and \(O P=O Q\). Then, which one of the following is true?
CorrectIncorrectHint
\(
a+i b=c+i d
\)
\(
\frac{a+c}{2}=0
\)
\(
\frac{b+d}{2}=0
\)
\(
a+c=b+d
\)
\(
\begin{aligned}
& \arg (c+i d)=0 \\
& \arg (a+i b)=\pi
\end{aligned}
\)
\(
\arg (a+i b)-\arg (c+i d)=\pi
\)
\(
|a+i b|=|c+i d|
\) -
Question 19 of 50
19. Question
1 point(s)If \(z_1=a+i b\) and \(z_2=c+i d\) are complex numbers such that \(\left|z_1\right|=\left|z_2\right|=1\) and \(\operatorname{Re}\left(z_1 \bar{z}_2\right)=0\), then the pair of complex numbers \(w_1=a+i c\) and \(w_2=b+i d\) satisfy
CorrectIncorrectHint
Since, \(z_1=a+i b\) and \(z_2=c+i d\)
\(
\Rightarrow\left|z_1\right|^2=a^2+b^2=1 \text { and }\left|z_2\right|^2=c^2+d^2=1 \dots(i)
\)
Also, \(\operatorname{Re}\left(z_1 \overline{z_2}\right)=0 \Rightarrow a c+b d=0\)
\(
\Rightarrow \quad \frac{a}{b}=-\frac{d}{c}=\lambda \dots(ii)
\)
From Eqs. (i) and (ii), \(b^2 \lambda^2+b^2=c^2+\lambda^2 c^2\)
\(
\Rightarrow b^2=c^2 \text { and } a^2=d^2
\)
Also, given \(w_1=a+i c\) and \(w_2=b+i d\)
Now,
\(
\begin{aligned}
& \left|w_1\right|=\sqrt{a^2+c^2}=\sqrt{a^2+b^2}=1 \\
& \left|w_2\right|=\sqrt{b^2+d^2}=\sqrt{a^2+b^2}=1
\end{aligned}
\)
and
\(
\begin{aligned}
& \operatorname{Re}\left(w_1 \overline{w_2}\right)=a b+c d=(b \lambda) b+c(-\lambda c) \\
& =\lambda\left(b^2-c^2\right)=0 \quad \text { [from Eq. (i)] }
\end{aligned}
\) -
Question 20 of 50
20. Question
1 point(s)Let \(z_1\) and \(z_2\) be two complex numbers such that \(z_1 \neq z_2\) and \(\left|z_1\right|=\left|z_2\right|\). If \(z_1\) has positive real part and \(z_2\) has negative imaginary part, then \(\frac{z_1+z_2}{z_1-z_2}\)
CorrectIncorrectHint
Given, \(\left|z_1\right|=\left|z_2\right|\),
Now, \(\quad \frac{z_1+z_2}{z_1-z_2} \times \frac{\overline{z_1}-\overline{z_2}}{\overline{z_1}-\overline{z_2}}\)
\(
=\frac{z_1 \overline{z_1}-z_1 \overline{z_2}+z_2 \overline{z_1}-z_2 \overline{z_2}}{\left|z_1-z_2\right|^2}
\)
\(
=\frac{\left|z_1\right|^2+\left(z_2 \overline{z_1}-z_1 \overline{z_2}\right)-\left|z_2\right|^2}{\left|z_1-z_2\right|^2}
\)
\(
=\frac{z_2 \overline{z_1}-z_1 \overline{z_2}}{\left|z_1-z_2\right|^2} \quad\left(\because\left|z_1\right|^2=\left|z_2\right|^2\right)
\)
As, we know \(z-\bar{z}=2 i \operatorname{Im}(z)\)
\(
\begin{aligned}
& \therefore \quad z_2 \overline{z_1}-z_1 \overline{z_2}=2 i \operatorname{Im}\left(z_2 \overline{z_1}\right) \\
& \therefore \quad \frac{z_1+z_2}{z_1-z_2}=\frac{2 i \operatorname{Im}\left(z_2 \overline{z_1}\right)}{\left|z_1-z_2\right|^2} \\
&
\end{aligned}
\)
Which is purely imaginary -
Question 21 of 50
21. Question
1 point(s)The value of \(\sum_{k=1}^6\left(\sin \frac{2 \pi k}{7}-i \cos \frac{2 \pi k}{7}\right)\) is
CorrectIncorrectHint
\(
\sin \frac{2 \pi k}{7}-i \cos \frac{2 \pi k}{7}
\)
\(
=-\mathrm{i}\left(\cos \frac{2 \pi \mathrm{k}}{7}+\mathrm{i} \sin \frac{2 \pi \mathrm{k}}{7}\right)
\)
\(
=-\mathrm{ie}^{2 \pi \mathrm{k} / 7 \mathrm{i}}
\)
\(
\begin{aligned}
& \Rightarrow \mathrm{S}=\sum_{\mathrm{k}=1}^6-\mathrm{i}\left(\mathrm{e}^{2 \pi \mathrm{k} / \mathrm{i}}\right) \\
& =-\mathrm{i}\left(\sum_{\mathrm{k}=1}^6 \mathrm{e}^{\mathrm{i} 2 \pi \mathrm{k} / 7}\right)
\end{aligned}
\)
\(
=-\mathrm{i} \text { [sum of roots of the equation }\left.x^6+x^5+x^4+x^3+x^2+1=0\right]
\)
\(
\begin{aligned}
& =-\mathrm{i}(-1) \\
& =\mathrm{i}
\end{aligned}
\) -
Question 22 of 50
22. Question
1 point(s)The equation \(\bar{b} z+b \bar{z}=c\), where \(b\) is a non-zero complex constant and \(c\) is a real number, represents
CorrectIncorrectHint
Key concept:- If \(z=a+i b\).
\(
\bar{z}=a-i b . \quad \text { \{conjugate }\}
\)
\(
\begin{aligned}
& \bar{b} z+b \bar{z}=c . \\
& b=u+i v, \bar{b}=u-i v . \\
& z=x+i y, \bar{z}=x-i y . \\
&(u-i v)(x+i y)+(u+i v)(x-i y)=0 .
\end{aligned}
\)
\(
\begin{aligned}
& 2 u x+2 v y=c \\
& u x+v y=\frac{c}{2} .
\end{aligned}
\)
This is in the form of
\(
a x+b y=c .
\)
is a straight line -
Question 23 of 50
23. Question
1 point(s)If \(\left|a_i\right|<1, \lambda_i \geq 0\) for \(i=1,2, \ldots. n\) and \(\lambda_1+\lambda_2+\ldots.\lambda_n=1\), then the value of \(\left|\lambda_1 a_1+\lambda_2 a_2+\ldots. +\lambda_n a_n\right|\) is
CorrectIncorrectHint
\(
\left|a_i\right|<1 \text { and } \lambda_i \geqslant 0 \text {, and } \lambda_1+\lambda_2+\cdots+\lambda_n=1
\)
\(
\left|\lambda_1 a_1+\lambda_2 a_2+\cdots+\lambda_n a_n\right| \leqslant\left|\lambda_1 a_1\right|+\left|\lambda_2 a_2\right|+\cdots+\left|\lambda_n a_n\right|
\)
\(
\begin{aligned}
& \leqslant\left|\lambda_1\right|\left|a_1\right|+\left|\lambda_2\right|\left|a_2\right|+\cdots+\left|\lambda_n\right|\left|a_n\right| \\
& \leqslant\left|\lambda_1\right|(1)+\left|\lambda_2\right|(1)+\cdots+\left|\lambda_n\right|(1) \\
& =\left|\lambda_1\right|+\left|\lambda_2\right|+\cdots+\left|\lambda_n\right| \\
& =\left|\lambda_1+\lambda_2+\cdots+\lambda_n\right|, \lambda_i ‘s \text { are +ve } \\
& =|1|=1
\end{aligned}
\)
\(
\text { so, }\left|\lambda a_1+\lambda_2 a_2+\cdots+\lambda_n a_n\right| \leqslant 1
\) -
Question 24 of 50
24. Question
1 point(s)For any two complex numbers \(z_1, z_2\) and any two real numbers \(a\) and \(b, \quad\left|a z_1-b z_2\right|^2+\left|b z_1+a z_2\right|^2=\)
CorrectIncorrectHint
\(
\begin{aligned}
& \left|a z_1-b z_2\right|^2+\left|b z_1+a z_2\right|^2=\left(a z_1-b z_2\right)\left(a \overline{z_1}-b \overline{z_2}\right)+\left(a z_1+b z_2\right)\left(a \overline{z_1}+b \overline{z_2}\right) \\
& =a^2\left|z_1\right|^2-a b z_1 \overline{z_2}-a b z_2 \overline{z_1}+b^2\left|z_2\right|^2+a^2\left|z_1\right|^2+a b z_1 \overline{z_2}+a b z_2 \overline{z_2}+b^2\left|z_2\right|^2 \\
& \Rightarrow\left|a z_1-b z_2\right|^2+\left|b z_1+a z_2\right|^2=\left(a^2+b^2\right)\left(\left|z_1\right|^2+\left|z_2\right|^2\right)
\end{aligned}
\) -
Question 25 of 50
25. Question
1 point(s)If \(i=\sqrt{-1}\), the number of values of \(i^n+i^{-n}\) for different \(n \in z\) is
CorrectIncorrectHint
If \(\mathrm{n}=0\) we get the answer as 2 .
If \(\mathrm{n}\) is even and \(\mathrm{n}\) is simply a multiple of 2 but not a multiple of 4 , we get the answer as -2 . if \(\mathrm{n}\) is odd,
Let \(\mathrm{n}=3\) we get
\(
\begin{aligned}
& -\mathrm{i}-\frac{1}{\mathrm{i}} \\
& =\frac{1-1}{\mathrm{i}} \\
& =0 \\
& \mathrm{n}=5 \text { we get } \\
& \mathrm{i}+\frac{1}{\mathrm{i}} \\
& =0
\end{aligned}
\)
Hence in total there are only 3 values,
\(
-2,0,2
\) -
Question 26 of 50
26. Question
1 point(s)If \(a>0\) and \(b<0\), then \(\sqrt{a} \sqrt{b}\) is equal to (where, \(i=\sqrt{-1}\) )
CorrectIncorrectHint
In this question we have been given two conditions \(a>0\) and \(b<0\).
Hence we need to find \(\sqrt{a} \sqrt{b}\).
Now \(b<0\), which means that the values are negative, hence we can write \(\sqrt{b}\) as \(\sqrt{|b| \cdot i}\).
i.e. \(\sqrt{(-b)}=\sqrt{|b|(-1)}=i \sqrt{|b|}\), this is formed because \(\mathrm{b}\) is negative.We know that \(\mathrm{a}>0\). Hence, \(\sqrt{a}\) can be written as such. Thus, we can write \(\sqrt{a} \sqrt{b}\) as,
\(
\sqrt{a} \sqrt{b}=\sqrt{a} \sqrt{|b|} \cdot i
\)We got, \(\sqrt{b}=\sqrt{|b|} \cdot i\)
Thus simplifying the above expression we get,
\(
\sqrt{a} \sqrt{b}=\sqrt{a|b|} \cdot i
\)Thus we got the value of \(\sqrt{a} \sqrt{b}\) as \(\sqrt{a|b|} \cdot i\).
-
Question 27 of 50
27. Question
1 point(s)Consider the following statements.
\(S_1:-6=2 i \times 3 i=\sqrt{(-4)} \times \sqrt{(-9)}\) (where, \(i=\sqrt{-1}\) )
\(
\begin{aligned}
& S_2: \sqrt{(-4)} \times \sqrt{(-9)}=\sqrt{(-4) \times(-9)} \\
& S_3: \sqrt{(-4) \times(-9)}=\sqrt{36} \\
& S_4: \sqrt{36}=6
\end{aligned}
\)
Of these statements, the incorrect one isCorrectIncorrectHint
\(
\begin{aligned}
& \mathrm{S}_1=\sqrt{-4} \times \sqrt{-9} \\
& =\sqrt{-1} \times \sqrt{4} \times \sqrt{-1} \times \sqrt{9} \\
& =\sqrt{-1} \times 2 \times \mathrm{i} \times 3 \\
& =2 \mathrm{i} \times 3 \mathrm{i} \\
& =6 \mathrm{i}^2=-6 \text { is true } \\
& \mathrm{S}_2=\sqrt{(-4) \times(-9)} \\
& =\sqrt{36}=\sqrt{4 \times 9} \neq \sqrt{4} \times \sqrt{9} \neq \sqrt{-4} \times \sqrt{-9} \text { since } \\
& \sqrt{-4} \times \sqrt{-9}=2 \mathrm{i} \times 3 \mathrm{i}=6 \mathrm{i}^2=-6
\end{aligned}
\)
Hence \(\mathrm{S}_2\) is not true.
\(S_3=\sqrt{(-4) \times(-9)}=\sqrt{36}\) is true.
\(S_4=\sqrt{36}=\sqrt{6 \times 6}=6\) is true -
Question 28 of 50
28. Question
1 point(s)The value of \(\sum_{n=0}^{50} i^{(2 n+1) !}(\) where, \(i=\sqrt{-1})\) is
CorrectIncorrectHint
\(
\begin{aligned}
& \sum_{n=0}^{50} i^{(2 n+1) !}=i^{1 !}+i^{3 !}+i^{5 !}+\ldots . i^{101 !} \\
& \quad=i+i^6+\left(i^{4 m}+i^{4 m}+\ldots .49 \text { times }\right) \\
& \quad=i-1+49 \\
& \quad=48+i
\end{aligned}
\) -
Question 29 of 50
29. Question
1 point(s)The value of \(\sum_{r=-3}^{1003} i^r(\) where \(i=\sqrt{-1})\) is
CorrectIncorrectHint
\(
\begin{gathered}
\sum_{r=-3}^{1003} i^r=i^{-3}+i^{-2}+i^{-1}+i^0+i^{1}+i^2+i^3+i^4+\cdots +i^{1003} \\
\end{gathered}
\)
\(
=\frac{1}{i^3}+\frac{1}{i^2}+\frac{1}{i}+1+i-1-i+1+\ldots . .+i^{1003}
\)
\(
\begin{aligned}
& \Rightarrow-\frac{1}{i}+(-1)+\frac{1}{i}+1+i-1-i+1+\ldots i^{1001}+i^{1002}+i^{1003} \\
&
\end{aligned}
\)
\(
\Rightarrow \quad\left(i^4\right)^{250} \cdot i+\left(i^4\right)^{250} \cdot i^2+\left(i^4\right)^{250} \cdot i^3
\)
\(
\Rightarrow \quad i+i^2+i^3 \Rightarrow i-1-i=-1
\) -
Question 30 of 50
30. Question
1 point(s)The digit in the unit’s place of \((153)^{98}\) is
CorrectIncorrectHint
153 has 3 in the unit’s place.
\(153^2\) has 9 in the unit’s place.
\(153^3\) has 7 in the unit’s place.
\(153^4\) has 1 in the unit’s place.
\(154^5\) has 3 in the unit’s place.
From next, the digit in the unit’s place gets repeated. So the unit’s place can be either 3 or 9 or 7 or 1 .
\(
(153)^{98}=(153)^{(4 \times 24)+2}=(153)^{(4 \times 24)} \times(153)^2 .
\)
The digit in the unit’s place of \((153)^{(4 \times 24)}\) is 1 and that in \(\left(153^2\right)\) is 9 .
Therefore the digit in the unit’s place of \((153)^{(4 \times 24)} \times(153)^2\) is 9 .
Therefore, the digit in the unit’s place of \((153)^{98}\) is 9 . -
Question 31 of 50
31. Question
1 point(s)The digit in the unit’s place of \((141414)^{12121}\) is
CorrectIncorrectHint
We are required to find the digit in unit’s place of \((141414)^{12121}\)
Since the unit digit of 141414 is 4 , we will divide the index with 4 for the remainder.
Upon dividing 12121 with 4, we get quotient as 303 and the remainder is 1.
Now, we can write the index using the division algorithm as: dividend = quotient \(\times\) divisor + remainder (i.e., \(a=\) bq + r) as: \(12121=303(4)+1\)
Therefore, \((141414)^{12121}=(141414)^{303(4)+1}\)
It can also be written as: \((141414)^{303(4)+1}=\left(141414^4\right)^{303}(141414)^1\)
Now, we only need the unit place of 141414 , therefore, we can write the above equation as: \(\left(4^4\right)^{303}(4)^1\)
Now, the unit digits in various powers of 4 can be written as: \(4^1=4,4^2=6,4^3=4,4^4=6\) and so on. Hence, we can write the equation \(\left(4^4\right)^{303}(4)^1\) as \((6)^{303}(4)\).
Now, we know that for every power of 6 , the unit digit is always 6 . Hence, we can write \((6)^{303}(4)\) as \(6 \times 4=24\) and the digit at unit place is 4 . -
Question 32 of 50
32. Question
1 point(s)Find the value of \(1+i^2+i^4+i^6+\ldots+i^{2 n}\), where \(i=\sqrt{-1}\) and \(n \in N\). Assume n is odd.
CorrectIncorrectHint
\(\because 1+i^2+i^4+i^6+\ldots+i^{2 n}=1-1+1-1+\ldots+(-1)^n\)
Case I: If \(n\) is odd, then
\(
1+i^2+i^4+i^6+\ldots+i^{2 n}=1-1+1-1+\ldots+1-1=0
\)Case II: If \(n\) is even, then
\(
1+i^2+i^4+i^6+\ldots+i^{2 n}=1-1+1-1+\ldots+1=1
\) -
Question 33 of 50
33. Question
1 point(s)If \(a=\frac{1+i}{\sqrt{2}}\), where \(i=\sqrt{-1}\), then find the value of \(a^{1929}\).
CorrectIncorrectHint
\(
\begin{aligned}
a^2=\left(\frac{1+i}{\sqrt{2}}\right)^2 & =\left(\frac{1+i^2+2 i}{2}\right) \\
& =\left(\frac{1-1+2 i}{2}\right)=i \\
a^{1929} & =a \cdot a^{1928}=a \cdot\left(a^2\right)^{964}=a(i)^{964} \\
& =a(i)^{4 \times 241}=a \cdot\left(i^4\right)^{241}=a
\end{aligned}
\) -
Question 34 of 50
34. Question
1 point(s)Find the value of \(\sum_{n=1}^{13}\left(i^n+i^{n+1}\right)\) (where, \(i=\sqrt{-1}\) )
CorrectIncorrectHint
\(
\sum_{n=1}^{13}\left(i^n+i^{n+1}\right)=\sum_{n=1}^{13} i^n+\sum_{n=1}^{13} i^{n+1}=(i+0)+\left(i^2+0\right)
\)
\(=i-1 \left[\begin{array}{l}
\because \sum_{n=2}^{13} i^n=0 \text { and } \sum_{n=2}^{13} i^{n+1}=0 \\
\text { (three sets of four consecutive powers of } i \text { ) }
\end{array}\right]
\) -
Question 35 of 50
35. Question
1 point(s)Find the value of \(\sum_{n=0}^{100} i^{n !}\) (where, \(i=\sqrt{-1}\) ).
CorrectIncorrectHint
\(n !\) is divisible by \(4, \forall n \geq 4\).
\(
\begin{aligned}
\therefore \sum_{n=4}^{100} i^{n !} & =\sum_{n=1}^{97} i^{(n+3) !} \\
& =i^0+i^0+i^0+\ldots 97 \text { times }=97 \dots(i)
\end{aligned}
\)
\(
\begin{aligned}
\therefore \sum_{n=0}^{100} i^{n !} & =\sum_{n=0}^3 i^{n !}+\sum_{n=4}^{100} i^{n !} \\
& =i^{0 !}+i^{1 !}+i^{2 !}+i^{3 !}+97
\end{aligned}
\)
[from Eq. (i)]
\(
\begin{aligned}
& =i^1+i^1+i^2+i^6+97=i+i-1-1+97 \\
& =95+2 i
\end{aligned}
\) -
Question 36 of 50
36. Question
1 point(s)Common roots of the equations \(z^3+2 z^2+2 z+1=0\) and \(z^{1985}+z^{100}+1=0\) are
CorrectIncorrectHint
The first equation may be written as
\(
(z+1)(z+z+1)=0:
\)
its roots are \(-1, \omega\) and \(\omega^2\).
The root \(z=-1\) does not satisfy the second equation but \(z=\omega\) and \(z=\omega^2\) satisfy it as \(\omega^{3 n}=1\) Hence and \(\omega\) and \(\omega^2\) are the common roots.Explanation;
\(
\begin{aligned}
& z^3+2 z^2+2 z+1=0 \\
& z^{1985}+z^{100}+1=0
\end{aligned}
\)
We know that \(1+\omega+\omega^2=0, \omega^3=1\) check to be root,
\(
\begin{aligned}
& \text { (1) } \omega^3+2 \omega^2+2 \omega+1 \\
& =1+\omega+\omega^2+1+\omega+\omega^2=0+0=0 \\
& \text { (2) } \omega^{1985}+\omega^{100}+1 \\
& \omega^2+\omega+1=0
\end{aligned}
\)
\(\omega\) is a common root check \(\omega^2\) to be a root
\(
\begin{aligned}
& \text { (1) } \omega^6+2 \omega^4+2 \omega^2+1 \\
& =\left(\omega^3\right)^2+2 \omega\left(\omega^3\right)+2 \omega^2+1 \\
& =1+\omega+\omega^2+1+\omega+\omega^2=0 \\
& \text { (2) }=\omega^{3970}+\omega^{200}+1 \\
& =\omega+\omega^2+1=0
\end{aligned}
\)
\(\omega^2\) is a common root -
Question 37 of 50
37. Question
1 point(s)If \(z_1\) and \(z_2\) are two complex numbers such that \(\left|\frac{z_1-z_2}{1-\bar{z}_1 z_2}\right|=1\), then which one of the following is not true?
CorrectIncorrectHint
\(
\text { Given: }\left|\frac{z_1-z_2}{1-\bar{z}_1 z_2}\right|=1 \Rightarrow \frac{\left|z_1-z_2\right|}{1-\bar{z}_1 z_2 \mid}=1 \Rightarrow\left|z_1-z_2\right|=\left|1-\bar{z}_1 z_2\right|
\)
\(
\text { Squaring both sides }\left|z_1-z_2\right|^2=\left|1-\bar{z}_1 z_2\right|^2
\)
\(
\left(z_1-z_2\right)\left(\bar{z}_1-\bar{z}_2\right)=\left(1-\overline{z_1} z_2\right)\left(1-z_1 \bar{z}_2\right)\left[\because(z)^2=z \cdot \bar{z}\right]
\)
\(
\begin{aligned}
& \Rightarrow\left|z_1\right|^2+\left|z_2\right|^2=1+\left|z_1\right|^2\left|z_2\right|^2 \\
& \Rightarrow 1-\left|z_1\right|^2-\left|z_2\right|^2+\left|z_1\right|^2\left|z_2\right|^2=0 \\
& \Rightarrow\left(1-\left|z_1\right|^2\right)\left(1-\left|z_2\right|^2\right)=0 \Rightarrow\left|z_1\right|=1,\left|z_2\right|=1
\end{aligned}
\) -
Question 38 of 50
38. Question
1 point(s)The points representing cube roots of unity
CorrectIncorrectHint
The cube roots of unity are given by \(A(1,0), B(-1 / 2,\sqrt{3} / 2, C(-1 / 2,-\sqrt{3} / 2)\)
The value for each of \(A B, B C\) and \(C A\) is \(\sqrt{3}\) Hence these points represent an equilateral triangle
-
Question 39 of 50
39. Question
1 point(s)If \(z_1\) and \(z_2\) are two complex numbers such that \(\left|\frac{z_1-z_2}{z_1+z_2}\right|=1\), then
CorrectIncorrectHint
\(
\left|\frac{z_1-z_2}{z_1+z_2}\right|
\)
Replace \(z_1=i k z_2\) in the above equation and solve it you will get 1. -
Question 40 of 50
40. Question
1 point(s)If \(z_1, z_2\) are two complex numbers such that \(\left|\frac{z_1-z_2}{z_1+z_2}\right|=1\) and \(i z_1=k z_2\) where \(k \in R\), then the angle between \(z_1-z_2\) and \(z_1+z_2\) is
CorrectIncorrectHint
\(
\text { Given: }\left|\frac{z_1-z_2}{z_1+z_2}\right|=1
\)
\(
\Rightarrow \frac{\left|z_1-z_2\right|}{\left|z_1+z_2\right|}=1 \Rightarrow\left|z_1-z_2\right|=\left|z_1+z_2\right|
\)
\(
\Rightarrow\left|\frac{z_1}{z_2}-1\right|=\left|\frac{z_1}{z_2}+1\right|
\)
\(
\text { squaring: }\left|\frac{z_1}{z_2}-1\right|^2=\left|\frac{z_1}{z_2}+1\right|^2
\)
\(
\Rightarrow\left|\frac{z_1}{z_2}\right|^2+1-2 \operatorname{Re}\left(\frac{z_1}{z_2}\right)=\left|\frac{z_1}{z_2}\right|^2+1+2 \operatorname{Re}\left(\frac{z_1}{z_2}\right)
\)
\(
\Rightarrow 4 \operatorname{Re}\left(\frac{z_1}{z_2}\right)=0 \Rightarrow \frac{z_1}{z_2} \text { is purely imagnary } \Rightarrow \frac{i z_1}{z_2}=k, k \in \mathbb{R}
\)
\(
\text { let } \theta \text { be the angle between } z_1-z_2 \text { and } z_1+z_2
\)
\(
\theta=\operatorname{Arg}\left(\frac{z_1-z_2}{z_1+z_2}\right)=\operatorname{Arg}\left(\frac{\frac{z_1}{z_2}-1}{\frac{z_1}{z_2}+1}\right)
\)
\(
=\operatorname{Arg}\left(\frac{-i k-1}{-i k+1}\right)=\operatorname{Arg}\left(\frac{1+i k}{-1+i k}\right)
\)
\(
=\operatorname{Arg}\left(\frac{(1+i k)(1+i k)}{(-1+i k)(1+i k)}\right)=\operatorname{Arg}\left(\frac{1-k^2+2 i k}{-k^2-1}\right)=\operatorname{Arg}\left(\frac{k^2-1-2 i k}{k^2+1}\right)
\)
\(
\text { so, } \theta=\tan ^{-1}\left(\frac{-2 k}{k^2-1}\right)=\tan ^{-1}\left(\frac{2 k}{1-k^2}\right)
\) -
Question 41 of 50
41. Question
1 point(s)If \(n\) is a positive integer greater than unity and \(z\) is a complex number satisfying the equation \(z^n=(z+1)^n\), then
CorrectIncorrectHint
\(
\begin{aligned}
& z^n=(z+1)^n \\
& \frac{z^n}{(z+1)^n}=1 \\
& \left(\frac{z}{z+1}\right)^n=1 \\
& \left(\frac{z}{z+1}\right)=(1)^{1 / n}{\text {th }} \text { root of unity }
\end{aligned}
\)\(
\begin{gathered}
\frac{|z|}{|z+1|}=1 \Rightarrow \quad|z|^2=|z+1|^2 \\
(z \bar{z}=(z+1)(\overline{z+1}) \\
(x+i y)(x-i y)=((x+1)+i y) \cdot((x+1)-i y)
\end{gathered}
\)
\(
x=-\frac{1}{2}
\)
Let
\(
\begin{aligned}
z & =x+i y \\
\bar{z} & =x-i y
\end{aligned}
\)
\(
\operatorname{Re}(z)<0
\)as \(x=-\frac{1}{2}\)
-
Question 42 of 50
42. Question
1 point(s)If \(n\) is a positive integer greater than unity and \(z\) is a complex number satisfying the equation \(z^n=(z+1)^n\), then
CorrectIncorrectHint
\(
\begin{aligned}
\frac{z}{1+z}=1^{1 / n} \Rightarrow\left|\frac{z}{1+z}\right|=1 & \Rightarrow|z|^2=|1+z|^2 \\
& \Rightarrow|z|^2=1+|z|^2+2 \operatorname{Re}(z)
\end{aligned}
\)
let \(z=x+i y \Rightarrow 2 x+1=0 \Rightarrow x=-\frac{1}{2}\) \(y\) can take any real value. So, none of the options is correct. -
Question 43 of 50
43. Question
1 point(s)If at least one value of the complex number \(z=x+i y\) satisfy the condition \(|z+\sqrt{2}|=\sqrt{a^2-3 a+2}\) and the inequality \(|z+i \sqrt{2}|<a\), then
CorrectIncorrectHint
Let \(\mathrm{z}=\mathrm{x}+\) iy be a complex number satisfying the given condition. then \(\mathrm{a}^2-3 \mathrm{a}+2=|\mathrm{z}+\sqrt{2}|=|\mathrm{z}+\mathrm{i} \sqrt{2}-\mathrm{i} \sqrt{2}+\sqrt{2}|\) \(\Rightarrow \mathrm{a}^2-3 \mathrm{a}+2 \leq|\mathrm{z}+\mathrm{i} \sqrt{2}|+\sqrt{2}|1-\mathrm{i}|<\mathrm{a}^2+2 \Rightarrow \mathrm{a}>0\)
Since \(\mathrm{a}^2-3 \mathrm{a}+2=|\mathrm{z}+\sqrt{2}|\) represents a circle with center at \(\mathrm{A}(-\sqrt{2}, 0)\) and radius \(\sqrt{a^2-3 a+2}\)
and \(|z+i \sqrt{2}|<a^2\) represents the interior of the circle with center at \(B(0, \sqrt{2})\) and radius \(a\).
Thus there will be a complex number satisfying the given condition and the given inequality if the distance \(A B\) is less than the sum or difference of the two radii of 2 circles. i.e.
\(
\Rightarrow \sqrt{(-\sqrt{2}-0)^2+(0+\sqrt{2})^2}<\sqrt{a^2-3 a+2} \pm a \Rightarrow 2 \pm a<\sqrt{a^2-3 a+2}
\)
Square both sides to get, \(-\mathrm{a}<-2\) or \(7 \mathrm{a}<-2 \Rightarrow \mathrm{a}>2, \quad \mathrm{a}<\frac{-2}{7}\)
But \(\mathrm{a}>0\) thus \(\mathrm{a}>2\) -
Question 44 of 50
44. Question
1 point(s)Given \(z\) is a complex number with modulus 1 . Then, the equation \(\left(\frac{1+i a}{1-i a}\right)^4=z\) has
CorrectIncorrectHint
\(
\begin{aligned}
& \left(\frac{1+i a}{1-i a}\right)^4=z \\
& \left(\frac{-i(i-a)}{-i(i+a)}\right)^4=z \\
& \left(\frac{a-i}{a+i}\right)^4=z \\
& \left|\frac{a-i}{a+i}\right|^4=|z|=1 \\
& |a-i|^4=|a+i|^4 \\
& |a-i|=|a+i|
\end{aligned}
\)
\(\therefore\) a lies on the perpendicular bisector of \(\mathrm{i}\) and \(-\mathrm{i}\). a lies on real axis. Hence the roots all are real and distinct. -
Question 45 of 50
45. Question
1 point(s)The centre of a regular polygon of \(n\) sides is located at the point \(z=0\), and one of its vertex \(z_1\) is known. If \(z_2\) be the vertex adjacent to \(z_1\), then \(z_2\) is equal to
CorrectIncorrectHint
Concept:
If the centre of a regular polygon of \(n\) sides is located at the point \(z=0\) and one of its vertex \(z_1\) is known, then the adjecent vertex \(z_2\) can be obtained by rotating \(z 1\) by an angle \(\frac{2 \pi}{\mathrm{n}}\) either in clockwise or anticlockwise direction.
\(
\mathrm{e}^{ \pm} \frac{2 \pi \mathrm{i}}{\mathrm{n}}=\cos \frac{2 \pi}{\mathrm{n}} \pm \mathrm{i} \sin \frac{2 \pi}{\mathrm{n}}
\)
Calculations:
Given, the centre of a regular polygon of \(n\) sides is located at the point \(z=0\) and one of its vertex \(z_1\) is known.
\(\Rightarrow z_2\) be the vertex adjacent to \(z_1\).
\(\Rightarrow z_2\) can be obtained by rotating \(z_1\) by an angle \(\frac{2 \pi}{n}\) either in clockwise or anticlockwise direction.
\(
\begin{aligned}
& \Rightarrow \mathrm{z}_2=\mathrm{z}_1 \mathrm{e}^{ \pm} \frac{2 \pi \mathrm{i}}{\mathrm{n}} \\
& \Rightarrow\left|\mathrm{z}_2\right|=\left|\mathrm{z}_1\right| \mathrm{e}^{ \pm} \frac{2 \pi \mathrm{i}}{\mathrm{n}} \\
& \Rightarrow \mathrm{z}_2=z_1\left(\cos \frac{2 \pi}{n} \pm i \sin \frac{2 \pi}{n}\right)
\end{aligned}
\)
Hence, the centre of a regular polygon of \(n\) sides is located at the point \(z=0\) and one of its vertex \(z_1\) is known. If \(z_2\) be the vertex adjacent to \(z_1\), then \(z_2\) is equal to
\(
z_1\left(\cos \frac{2 \pi}{n} \pm i \sin \frac{2 \pi}{n}\right)
\) -
Question 46 of 50
46. Question
1 point(s)For any complex number \(z\), the minimum value of \(|z|+|z-1|\) is
CorrectIncorrectHint
Let \(z=x+i y\) with \(x, y\) real.
\(
|z|+|z-1|=\sqrt{x^2+y^2}+\sqrt{(x-1)^2+y^2}
\)
Clearly if this has minimum value, then \(y=0\), since any other value of \(y\) would make it larger.
So we must find the minimum value of
\(
\begin{aligned}
& \sqrt{x^2}+\sqrt{(x-1)^2} \\
& |x|+|x-1|
\end{aligned}
\)
Consider the function:
\(
f(x)=|x|+|x-1|
\)
We consider six cases:
Case 1: \(x=0\) Then \(f(x)=f(0)=0+1=1\)
Case 2: \(x=1\) Then \(f(x)=f(1)=1+0=1\)
Case 3: \(x>0\) and \(x-1>0\) which implies \(x>1\)
Then \(f(x)=x+x=1=2 x-1\)
Case 4: \(x>0\) and \(x-1<0\) which implies 00 which is impossible
Then \(f(x)=x+x=1=2 x-1\)
Case 5: \(x<0\) and \(x-1>0\) which is impossible
Case 6: \(x<0\) and \(x-1<0\) which implies \(x<0\)
Then \(f(x)=-x-(x-1)=x-x+1=-2 x+1\)
So \(f(x)=|x|+|x-1|\) is the piecewise function:
\(
f(x)=\left\{\begin{array}{cl}
-2 x+1 & \text { if } x<0 \\
1 & \text { if } 0 \leqslant x<1 \\
2 x-1 & \text { if } x \geqslant 1
\end{array}\right.
\)
Thus the minimum value is of \(|z|+|z-1|\) is 1 . -
Question 47 of 50
47. Question
1 point(s)The inequality \(|z-4|<|z-2|\) represents
CorrectIncorrectHint
Let, \(\mathrm{z}=\mathrm{x}+\mathrm{iy}\)
Putting in the given inequality,
\(
\begin{aligned}
& |(x-4)-i y|<|(x-2)+i y| \\
& \Rightarrow \sqrt{(x-4)^2+y^2}<\sqrt{(x-2)^2+y^2}
\end{aligned}
\)
squaring both sides,
\(
\begin{aligned}
& \Rightarrow(\mathrm{x}-4)^2+\mathrm{y}^2<(\mathrm{x}-2)^2+\mathrm{y}^2 \\
& \Rightarrow-8 \mathrm{x}+16<-4 \mathrm{x}+4 \\
& \Rightarrow 4 \mathrm{x}>12 \Rightarrow \mathrm{x}>3 \Rightarrow \operatorname{Re}(\mathrm{z})>3
\end{aligned}
\) -
Question 48 of 50
48. Question
1 point(s)Number of non-zero integral solutions of the equation \((1-i)^n=2^n\) is
CorrectIncorrectHint
Lets first find the value of \(|1-i|\)
Complex number is of the form \(x+i y\)
Where \(x=1\)
\(
\begin{aligned}
y & =-1 \\
|1-i| & =\sqrt{x^2+y^2}=\sqrt{2}
\end{aligned}
\)
\(
\text { Hence, }|1-i|=\sqrt{2}
\)
\(
\text { Given }|1-i|^n=2^n
\)
\(
\begin{aligned}
& \text { Putting }|1-i|=\sqrt{2} \\
& \qquad \begin{aligned}
(\sqrt{2})^n=2^n \\
(\sqrt{2})^n=(\sqrt{2} \times \sqrt{2})^n \\
(\sqrt{2})^n=(\sqrt{2})^{2 \times n} \\
(\sqrt{2})^n=\sqrt{2}^{2 n}
\end{aligned}
\end{aligned}
\)
Comparing Powers
\(
\begin{aligned}
& n=2 n \\
& n-2 n=0 \\
& -n=0 \\
& n=0
\end{aligned}
\)
Hence, There is no non-zero integral solution -
Question 49 of 50
49. Question
1 point(s)If \(\operatorname{Im}\left(\frac{2 z+1}{i z+1}\right)=-2\), then locus of \(z\) is
CorrectIncorrectHint
\(
\begin{aligned}
& z=x+i y \\
& \frac{2(x+i y)+1}{i(x+i y)+1}=-2 \\
= & \frac{2(x+i y)+1}{i x-y+1}
\end{aligned}
\)
\(
=\frac{(2 x+1)+2 i y}{(1-y)+i x} \times \frac{(1-y)-i x}{(1-y)-i x}
\)
\(
=\frac{[(2 x+1)+2 i y][(1-y)-i x]}{(1-y)^2+x^2}
\)
\(
-2 x^2-x+2 y-2 y^2=\left[x^2+(1-y)^2\right](-2)
\)
\(
\begin{aligned}
-x-2 y & =-2 \\
x+2 y & =2 \rightarrow \text { a straight line }
\end{aligned}
\) -
Question 50 of 50
50. Question
1 point(s)Let \(\mathrm{p}, \mathrm{q} \in \mathbb{R}\) and \((1-\sqrt{3} \mathrm{i})^{200}=2^{199}(\mathrm{p}+\mathrm{iq})\), \(\mathrm{i}=\sqrt{-1}\) Then \(\mathrm{p}+\mathrm{q}+\mathrm{q}^2\) and \(\mathrm{p}-\mathrm{q}+\mathrm{q}^2\) are roots of the equation.
CorrectIncorrectHint
\(
\begin{aligned}
& (1-\sqrt{3} i)^{200}=2^{199}(p+i q) \\
& 2^{200}\left(\cos \frac{\pi}{3}-i \sin \frac{\pi}{3}\right)^{200}=2^{199}(p+i q)
\end{aligned}
\)
\(
\begin{aligned}
& 2\left(-\frac{1}{2}-\mathrm{i} \frac{\sqrt{3}}{2}\right)=\mathrm{p}+\mathrm{iq} \\
& \mathrm{p}=-1, \mathrm{q}=-\sqrt{3} \\
& \alpha=\mathrm{p}+\mathrm{q}+\mathrm{q}^2=2-\sqrt{3} \\
& \beta=\mathrm{p}-\mathrm{q}+\mathrm{q}^2=2+\sqrt{3} \\
& \alpha+\beta=4 \\
& \alpha \cdot \beta=1 \\
& \text { equation } \mathrm{x}^2-4 \mathrm{x}+1=0
\end{aligned}
\)