Exercise Problems
Quiz Summary
0 of 51 Questions completed
Questions:
Information
You have already completed the quiz before. Hence you can not start it again.
Quiz is loading…
You must sign in or sign up to start the quiz.
You must first complete the following:
Results
Results
0 of 51 Questions answered correctly
Your time:
Time has elapsed
You have reached 0 of 0 point(s), (0)
Earned Point(s): 0 of 0, (0)
0 Essay(s) Pending (Possible Point(s): 0)
Categories
- Not categorized 0%
- 1
- 2
- 3
- 4
- 5
- 6
- 7
- 8
- 9
- 10
- 11
- 12
- 13
- 14
- 15
- 16
- 17
- 18
- 19
- 20
- 21
- 22
- 23
- 24
- 25
- 26
- 27
- 28
- 29
- 30
- 31
- 32
- 33
- 34
- 35
- 36
- 37
- 38
- 39
- 40
- 41
- 42
- 43
- 44
- 45
- 46
- 47
- 48
- 49
- 50
- 51
- Current
- Review
- Answered
- Correct
- Incorrect
-
Question 1 of 51
1. Question
A vector has components along the \(X\)-axis equal to 25 units and along the Y-axis equal to 60 units. What is the magnitude and direction of the vector?
CorrectIncorrectHint
The given vector is the resultant of two perpendicular vectors, one along the \(X\)-axis of magnitude 25 unit and the other along the \(Y\)-axis of magnitude 60 units. The resultant has a magnitude \(A\) given by
\(
\begin{aligned}
A &=\sqrt{(25)^2+(60)^2+2 \times 25 \times 60 \cos 90^{\circ}} \\
&=\sqrt{(25)^2+(60)^2}=65 .
\end{aligned}
\)
The angle \(\alpha\) between this vector and the \(X\)-axis is given by
\(
\tan \alpha=\frac{60}{25}
\) -
Question 2 of 51
2. Question
Find the resultant of the three vectors shown in the figure below.
 CorrectIncorrect
CorrectIncorrectHint
The \(x\)-component of the \(5.0 \mathrm{~m}\) vector \(=5.0 \mathrm{~m} \cos 37^{\circ}\) \(=4 \cdot 0 \mathrm{~m}\),
the \(x\)-component of the \(3.0 \mathrm{~m}\) vector \(=3.0 \mathrm{~m}\) and the \(x\)-component of the \(2.0 \mathrm{~m}\) vector \(=2.0 \mathrm{~m} \cos 90^{\circ}\) \(=0\).
Hence, the \(x\)-component of the resultant
\(
=4.0 \mathrm{~m}+3.0 \mathrm{~m}+0=7.0 \mathrm{~m} \text {. }
\)
The \(y\)-component of the \(5.0 \mathrm{~m}\) vector \(=5.0 \mathrm{~m} \sin 37^{\circ}\)
\(
=3.0 \mathrm{~m} \text {, }
\)
the \(y\)-component of the \(3.0 \mathrm{~m}\) vector \(=0\)
and the \(y\)-component of the \(2.0 \mathrm{~m}\) vector \(=2.0 \mathrm{~m}\).
Hence, the \(y\)-component of the resultant
\(
=3.0 \mathrm{~m}+0+2.0 \mathrm{~m}=5.0 \mathrm{~m} \text {. }
\)
The magnitude of the resultant vector
\(
\begin{aligned}
&=\sqrt{(7 \cdot 0 \mathrm{~m})^2+(5 \cdot 0 \mathrm{~m})^2} \\
&=8.6 \mathrm{~m} .
\end{aligned}
\)
If the angle made by the resultant with the \(X\)-axis is \(\theta\), then
\(
\tan \theta=\frac{y \text {-component }}{x \text {-component }}=\frac{5 \cdot 0}{7 \cdot 0} \text { or, } \theta=35 \cdot 5^{\circ} .
\) -
Question 3 of 51
3. Question
The sum of the three vectors shown in the figure below is zero. Find the magnitudes of the vectors \(\overrightarrow{O B}\) and \(\overrightarrow{O C}\).
 CorrectIncorrect
CorrectIncorrectHint
The \(x\)-component of \(\overrightarrow{O A}=(O A) \cos 90^{\circ}=0\).
The \(x\)-component of \(\overrightarrow{O B}=(O B) \cos 0^{\circ}=O B\).
The \(x\)-component of \(\overrightarrow{O C}=(O C) \cos 135^{\circ}=-\frac{1}{\sqrt{2}} O C\).
Hence, the \(x\)-component of the resultant
\(
=O B-\frac{1}{\sqrt{ } 2} O C \text {. } \dots(i)
\)
It is given that the resultant is zero and hence its \(x\)-component is also zero. From (i),
\(
O B=\frac{1}{\sqrt{2}} O C \text {. } \dots(ii)
\)
The \(y\)-component of \(\overrightarrow{O A}=O A \cos 180^{\circ}=-O A\).
The \(y\)-component of \(\overrightarrow{O B}=O B \cos 90^{\circ}=0\).
The \(y\)-component of \(\overrightarrow{O C}=O C \cos 45^{\circ}=\frac{1}{\sqrt{2}} O C\).
Hence, the \(y\)-component of the resultant
\(
=\frac{1}{\sqrt{2}} O C-O A \dots(iii)
\)
As the resultant is zero, so is its \(y\)-component. From (iii),
\(
\frac{1}{\sqrt{2}} O C=O A, \text { or, } O C=\sqrt{ } 2 O A=5 \sqrt{ } 2 \mathrm{~m} .
\)
From (ii), \(O B=\frac{1}{\sqrt{2}} O C=5 \mathrm{~m}\). -
Question 4 of 51
4. Question
The magnitudes of vectors \(\overrightarrow{O A}, \overrightarrow{O B}\), and \(\overrightarrow{O C}\) in the figure below are equal. Find the direction of \(\overrightarrow{O A}+\overrightarrow{O B}-\overrightarrow{O C}\).
 CorrectIncorrect
CorrectIncorrectHint
Let \(O A=O B=O C=F\).
\(x\)-component of \(\overrightarrow{O A}=F \cos 30^{\circ}=F \frac{\sqrt{3}}{2}\).
\(x\)-component of \(\overrightarrow{O B}=F \cos 60^{\circ}=\frac{F}{2}\)
\(x\)-component of \(\overrightarrow{O C}=F \cos 135^{\circ}=-\frac{F}{\sqrt{2}}\).
\(x\)-component of \(\overrightarrow{O A}+\overrightarrow{O B}-\overrightarrow{O C}\)
\(
=\left(\frac{F \sqrt{ } 3}{2}\right)+\left(\frac{F}{2}\right)-\left(-\frac{F}{\sqrt{ } 2}\right)
\)
\(
=\frac{F}{2}(\sqrt{ } 3+1+\sqrt{ } 2) \text {. }
\)
\(y\)-component of \(\overrightarrow{O A}=F \cos 60^{\circ}=\frac{F}{2}\).
\(y\)-component of \(\overrightarrow{O B}=F \cos 150^{\circ}=-\frac{F \sqrt{3}}{2}\).
\(y\)-component of \(\overrightarrow{O C}=F \cos 45^{\circ}=\frac{F}{\sqrt{2}}\).
\(y\)-component of \(\overrightarrow{O A}+\overrightarrow{O B}-\overrightarrow{O C}\)
\(
\begin{aligned}
&=\left(\frac{F}{2}\right)+\left(-\frac{F \sqrt{ } 3}{2}\right)-\left(\frac{F}{\sqrt{ } 2}\right) \\
&=\frac{F}{2}(1-\sqrt{ } 3-\sqrt{ } 2) .
\end{aligned}
\)
Angle of \(\overrightarrow{O A}+\overrightarrow{O B}-\overrightarrow{O C}\) with the \(X\)-axis
\(
=\tan ^{-1} \frac{\frac{F}{2}(1-\sqrt{ } 3-\sqrt{ } 2)}{\frac{F}{2}(1+\sqrt{ } 3+\sqrt{ } 2)}=\tan ^{-1} \frac{(1-\sqrt{3}-\sqrt{ } 2)}{(1+\sqrt{ } 3+\sqrt{ } 2)} .
\) -
Question 5 of 51
5. Question
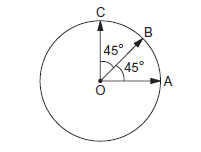
Find the resultant of the three vectors \(\overrightarrow{O A}, \overrightarrow{O B}\) and \(\overrightarrow{O C}\) shown in the figure below. Radius of the circle is \(R\).
 CorrectIncorrect
CorrectIncorrectHint
\(O A=O C\).
\(\overrightarrow{O A}+\overrightarrow{O C}\) is along \(\overrightarrow{O B}\) (bisector) and its magnitude is \(2 R \cos 45^{\circ}=R \sqrt{ } 2\)
\((\overrightarrow{O A}+\overrightarrow{O C})+\overrightarrow{O B}\) is along \(\overrightarrow{O B}\) and its magnitude is \(R \sqrt{ } 2+R=R(1+\sqrt{ } 2)\) -
Question 6 of 51
6. Question
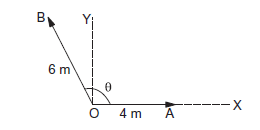
The resultant of vectors \(\overrightarrow{O A}\) and \(\overrightarrow{O B}\) is perpendicular to \(\overrightarrow{O A}\) (figure below). Find the angle \(A O B\).
 CorrectIncorrect
CorrectIncorrectHint
Take the dotted lines as \(X, Y\) axes. \(x\)-component of \(\overrightarrow{O A}=4 \mathrm{~m}, x\)-component of \(\overrightarrow{O B}=6 \mathrm{~m} \cos \theta\)
\(x\)-component of the resultant \(=(4+6 \cos \theta) \mathrm{m}\).
But it is given that the resultant is along \(Y\)-axis. Thus, the \(x\)-component of the resultant \(=0\)
\(4+6 \cos \theta=0\) or, \(\cos \theta=-2 / 3\). -
Question 7 of 51
7. Question
\(
\text { Write the unit vector in the direction of } \vec{A}=5 \vec{i}+\vec{j}-2 \vec{k} \text {. }
\)CorrectIncorrectHint
\(\quad|\vec{A}|=\sqrt{5^2+1^2+(-2)^2}=\sqrt{30}\)
The required unit vector is \(\frac{\vec{A}}{|\vec{A}|}\)
\(
=\frac{5}{\sqrt{30}} \vec{i}+\frac{1}{\sqrt{30}} \vec{j}-\frac{2}{\sqrt{30}} \vec{k}
\) -
Question 8 of 51
8. Question
\(
\text { If }|\vec{a}+\vec{b}|=|\vec{a}-\vec{b}| \text { show that } \vec{a} \perp \vec{b}
\). Is this statement true?CorrectIncorrectHint
\(
\begin{aligned}
&\text { We have }|\vec{a}+\vec{b}|^2=(\vec{a}+\vec{b}) \cdot(\vec{a}+\vec{b})\\
&=\vec{a} \cdot \vec{a}+\vec{a} \cdot \vec{b}+\vec{b} \cdot \vec{a}+\vec{b} \cdot \vec{b}\\
&=a^2+b^2+2 \vec{a} \cdot \vec{b} \text {. }\\
&|\vec{a}-\vec{b}|^2=(\vec{a}-\vec{b}) \cdot(\vec{a}-\vec{b})\\
&=a^2+b^2-2 \vec{a} \cdot \vec{b} \text {. }\\
&\text { If } \quad|\vec{a}+\vec{b}|=|\vec{a}-\vec{b}| \text {, }\\
&a^2+b^2+2 \vec{a} \cdot \vec{b}=a^2+b^2-2 \vec{a} \cdot \vec{b}\\
&\text { or, } \quad \vec{a} \cdot \vec{b}=0\\
&\text { or, } \quad \vec{a} \perp \vec{b}
\end{aligned}
\) -
Question 9 of 51
9. Question
If \(\vec{a}=2 \vec{i}+3 \vec{j}+4 \vec{k}\) and \(\vec{b}=4 \vec{i}+3 \vec{j}+2 \vec{k}\), find the angle between \(\vec{a}\) and \(\vec{b}\).
CorrectIncorrectHint
We have \(\vec{a} \cdot \vec{b}=a b \cos \theta\) or, \(\quad \cos \theta=\frac{\vec{a} \cdot \vec{b}}{a b}\) where \(\theta\) is the angle between \(\vec{a}\) and \(\vec{b}\).
Now
\(
\begin{aligned}
\vec{a} \cdot \vec{b} &=a_x b_x+a_y b_y+a_z b_z \\
&=2 \times 4+3 \times 3+4 \times 2=25 .
\end{aligned}
\)
Also
\(
\begin{aligned}
a &=\sqrt{a_x^2+a_y^2+a_z^2} \\
&=\sqrt{4+9+16}=\sqrt{29}
\end{aligned}
\)
and
\(
b=\sqrt{b_x^2+b_y^2+b_z^2}=\sqrt{16+9+4}=\sqrt{29} .
\)
Thus,
\(
\cos \theta=\frac{25}{29}
\)
or,
\(
\theta=\cos ^{-1}\left(\frac{25}{29}\right) .
\) -
Question 10 of 51
10. Question
If \(\vec{A}=2 \vec{i}-3 \vec{j}+7 \vec{k}, \quad \vec{B}=\vec{i}+2 \vec{k}\) and \(\vec{C}=\vec{j}-\vec{k} \quad\) find \(\vec{A} \cdot(\vec{B} \times \vec{C})\)
CorrectIncorrectHint
\(
\begin{aligned}
\vec{B} \times \vec{C} &=(\vec{i}+2 \vec{k}) \times(\vec{j}-\vec{k}) \\
&=\vec{i} \times(\vec{j}-\vec{k})+2 \vec{k} \times(\vec{j}-\vec{k}) \\
&=\vec{i} \times \vec{j}-\vec{i} \times \vec{k}+2 \vec{k} \times \vec{j}-2 \vec{k} \times \vec{k} \\
&=\vec{k}+\vec{j}-2 \vec{i}-0=-2 \vec{i}+\vec{j}+\vec{k} \\
\vec{A} \cdot(\vec{B} \times \vec{C}) &=(2 \vec{i}-3 \vec{j}+7 \vec{k}) \cdot(-2 \vec{i}+\vec{j}+\vec{k}) \\
&=(2)(-2)+(-3)(1)+(7)(1) \\
&=0
\end{aligned}
\) -
Question 11 of 51
11. Question
The volume of a sphere is given by
\(
V=\frac{4}{3} \pi R^3
\)
where \(R\) is the radius of the sphere.
(a) Find the rate of change of volume with respect to \(R\).
(b) Find the change in volume of the sphere as the radius is increased from \(20.0 \mathrm{~cm}\) to \(20.1 \mathrm{~cm}\). Assume that the rate does not appreciably change between \(R=20.0 \mathrm{~cm}\) to \(R=20.1 \mathrm{~cm}\).CorrectIncorrectHint
(a) \(\quad V=\frac{4}{3} \pi R^3\)
or, \(\quad \frac{d V}{d R}=\frac{4}{3} \pi \frac{d}{d R}(R)^3=\frac{4}{3} \pi \cdot 3 R^2=4 \pi R^2\).
(b) At \(R=20 \mathrm{~cm}\), the rate of change of volume with the radius is
\(
\begin{aligned}
\frac{d V}{d R} &=4 \pi R^2=4 \pi\left(400 \mathrm{~cm}^2\right) \\
&=1600 \pi \mathrm{cm}^2 .
\end{aligned}
\)
The change in volume as the radius changes from \(20.0 \mathrm{~cm}\) to \(20.1 \mathrm{~cm}\) is
\(
\begin{aligned}
\Delta V &=\frac{d V}{d R} \Delta R \\
&=\left(1600 \pi \mathrm{cm}^2\right)(0.1 \mathrm{~cm}) \\
&=160 \pi \mathrm{cm}^3 .
\end{aligned}
\) -
Question 12 of 51
12. Question
Find the derivative of the following functions with respect to \(x\). (a) \(y=x^2 \sin x\), (b) \(y=\frac{\sin x}{x}\) and (c) \(y=\sin \left(x^2\right)\).
CorrectIncorrectHint
(a)
\(
\begin{aligned}
y &=x^2 \sin x \\
\frac{d y}{d x} &=x^2 \frac{d}{d x}(\sin x)+(\sin x) \frac{d}{d x}\left(x^2\right) \\
&=x^2 \cos x+(\sin x)(2 x) \\
&=x(2 \sin x+x \cos x)
\end{aligned}
\)(b)
\(
\begin{aligned}
y &=\frac{\sin x}{x} \\
\frac{d y}{d x} &=\frac{x \frac{d}{d x}(\sin x)-\sin x\left(\frac{d x}{d x}\right)}{x^2} \\
&=\frac{x \cos x-\sin x}{x^2} .
\end{aligned}
\)(c)
\(
\begin{aligned}
\frac{d y}{d x} &=\frac{d}{d x^2}\left(\sin x^2\right) \cdot \frac{d\left(x^2\right)}{d x} \\
&=\cos x^2(2 x) \\
&=2 x \cos x^2
\end{aligned}
\) -
Question 13 of 51
13. Question
Find the maximum or minimum values of the function
\(
y=x+\frac{1}{x} \text { for } x>0
\)CorrectIncorrectHint
\(
\begin{aligned}
y &=x+\frac{1}{x} \\
\frac{d y}{d x} &=\frac{d}{d x}(x)+\frac{d}{d x}\left(x^{-1}\right) \\
&=1+\left(-x^{-2}\right) \\
&=1-\frac{1}{x^2} .
\end{aligned}
\)
For \(y\) to be maximum or minimum,
\(\frac{d y}{d x}=0\)
or, \(\quad 1-\frac{1}{x^2}=0\)
Thus, \(\quad x=1\) or \(-1\).
For \(x>0\) the only possible maximum or minimum is at \(x=1\). At \(x=1, y=x+\frac{1}{x}=2\).Near \(x=0, y=x+\frac{1}{x}\) is very large because of the term \(\frac{1}{x}\). For very large \(x\), again \(y\) is very large because of the term \(x\). Thus \(x=1\) must correspond to a minimum. Thus, \(y\) has only a minimum for \(x>0\). This minimum occurs at \(x=1\) and the minimum value of \(y\) is \(y=2\).
-
Question 14 of 51
14. Question
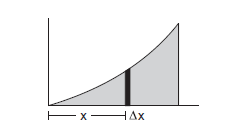
Figure below shows the curve \(y=x^2\). Find the area of the shaded part between \(x=0\) and \(x=6\).
 CorrectIncorrect
CorrectIncorrectHint
The area can be divided into strips by drawing ordinates between \(x=0\) and \(x=6\) at a regular interval of \(d x\). Consider the strip between the ordinates at \(x\) and \(x+d x\). The height of this strip is \(y=x^2\). The area of this strip is \(d A=y d x=x^2 d x\).
The total area of the shaded part is obtained by summing up these strip-areas with \(x\) varying from 0 to 6. Thus
\(
A=\int_0^6 x^2 d x
\)
\(
=\left[\frac{x^3}{3}\right]_0^6=\frac{216-0}{3}=72 .
\) -
Question 15 of 51
15. Question
\(
\text { Evaluate } \int_0^t A \sin \omega t d t \text { where } A \text { and } \omega \text { are constants. }
\)CorrectIncorrectHint
\(
\begin{aligned}
& \int_0^t A \sin \omega t d t \\
=& A\left[\frac{-\cos \omega t}{\omega}\right]_0^t=\frac{A}{\omega}(1-\cos \omega t) .
\end{aligned}
\) -
Question 16 of 51
16. Question
The velocity \(v\) and displacement \(x\) of a particle executing simple harmonic motion are related as
\(
v \frac{d v}{d x}=-\omega^2 x .
\)
At \(x=0, v=v_0\). Find the velocity \(v\) when the displacement becomes \(x\).CorrectIncorrectHint
We have
\(
\begin{aligned}
v \frac{d v}{d x} &=-\omega^2 x \\
\text { or, } & v d v &=-\omega^2 x d x \\
\text { or, } & \int_{v_0}^v v d v &=\int_0^x-\omega^2 x d x \dots(i)
\end{aligned}
\)
When summation is made on \(-\omega^2 x d x\) the quantity to be varied is \(x\). When summation is made on \(v d v\) the quantity to be varied is \(v\). As \(x\) varies from 0 to \(x\) the velocity varies from \(v_0\) to \(v\). Therefore, on the left the limits of integration are from \(v_0\) to \(v\) and on the right, they are from 0 to \(x\). Simplifying (i),
\(
\begin{aligned}
{\left[\frac{1}{2} v^2\right]_{v_0}^v } =-\omega^2\left[\frac{x^2}{2}\right]_0^x \\
\text { or, } \frac{1}{2}\left(v^2-v_0^2\right) =-\omega^2 \frac{x^2}{2} \\
\text { or, } v^2 =v_0^2-\omega^2 x^2 \\
\text { or, } v =\sqrt{v_0^2-\omega^2 x^2} .
\end{aligned}
\) -
Question 17 of 51
17. Question
The charge flown through a circuit in the time interval between \(t\) and \(t+d t\) is given by \(d q=e^{-t / \tau} d t\), where \(\tau\) is a constant. Find the total charge flown through the circuit between \(t=0\) to \(t=\tau\).
CorrectIncorrectHint
The total charge flown is the sum of all the \(d q\) ‘s for \(t\) varying from \(t=0\) to \(t=\tau\). Thus, the total charge flown is
\(
\begin{aligned}
Q &=\int_0^\tau e^{-t / \tau} d t \\
&=\left[\frac{e^{-t / \tau}}{-1 / \tau}\right]_0^\tau=\tau\left(1-\frac{1}{e}\right)
\end{aligned}
\) -
Question 18 of 51
18. Question
\(
\text { Evaluate }(21 \cdot 6002+234+2732 \cdot 10) \times 13
\)CorrectIncorrectHint
\(
\begin{gathered}
\quad \quad 21.6002 \\
234 \\
2732 \cdot 10 \\
\hline
\end{gathered}
\)\(
\begin{array}{r}
22 \\
\quad 234 \\
2732 \\
\hline 2988
\end{array}
\)The three numbers are arranged with their decimal points aligned (shown on the left part above). The column just left to the decimals has 4 as the doubtful digit. Thus, all the numbers are rounded to this column. The rounded numbers are shown on the right part above. The required expression is \(2988 \times 13=38844\). As 13 has only two significant digits the product should be rounded off after two significant digits. Thus the result is 39000.
-
Question 19 of 51
19. Question
A vector \(\vec{A}\) makes an angle of \(20^{\circ}\) and \(\vec{B}\) makes an angle of \(110^{\circ}\) with the \(X\)-axis. The magnitudes of these vectors are \(3 \mathrm{~m}\) and \(4 \mathrm{~m}\) respectively. Find the resultant.
CorrectIncorrectHint
Angle between \(\vec{A}\) and \(\vec{B}=110^{\circ}-20^{\circ}=90^{\circ},|\vec{A}|=3 \mathrm{~m}\) and \(|\vec{B}|=4 \mathrm{~m}\)
Magnitude of the resultant vector is given by
\(
\begin{aligned}
& R=\sqrt{A^2+B^2+2 A B \cos \theta} \\
& =\sqrt{3^2+4^2+2 \times 3 \times 4 \times \cos 90^{\circ}} \\
& =5 \mathrm{~m}
\end{aligned}
\)
Let \(\beta\) be the angle between \(\vec{R}\) and \(\vec{A}\).
\(
\begin{aligned}
& \beta=\tan ^{-1}\left(\frac{A \sin 90^{\circ}}{A+B \cos 90^{\circ}}\right) \\
& =\tan ^{-1}\left(\frac{4 \sin 90^{\circ}}{3+4 \cos 90^{\circ}}\right) \\
& =\tan ^{-1} \frac{4}{3} \\
& =\tan ^{-1}(1.333) \\
& =53^{\circ}
\end{aligned}
\)
Now, angle made by the resultant vector with the \(X\)-axis \(=53^{\circ}+20^{\circ}=73^{\circ}\)
\(\therefore\) The resultant \(\vec{R}\) is \(5 \mathrm{~m}\) and it makes an angle of \(73^{\circ}\) with the \(\mathrm{x}\)-axis. -
Question 20 of 51
20. Question
Let \(\vec{A}\) and \(\vec{B}\) be the two vectors of magnitude 10 unit each. If they are inclined to the \(X\)-axis at angles \(30^{\circ}\) and \(60^{\circ}\) respectively, find the resultant.
CorrectIncorrectHint
Angle between \(\vec{A}\) and \(\vec{B}\) is \(\theta=60^{\circ}-30^{\circ}=30^{\circ}\)
\(|\vec{A}|\) and \(|\vec{B}|=10\) unit
\(R=\sqrt{10^2+10^2+2 \cdot 10 \cdot 10 \cdot \cos 30^{\circ}}=19.3\)
\(\beta\) be the angle between \(\vec{R}\) and \(\vec{A}\)
\(
\beta=\tan ^{-1}\left(\frac{10 \sin 30^{\circ}}{10+10 \cos 30^{\circ}}\right)=\tan ^{-1}\left(\frac{1}{2+\sqrt{3}}\right)=\tan ^{-1}(0.26795)=15^{\circ}
\)
Resultant makes \(15^{\circ}+30^{\circ}=45^{\circ}\) angle with \(x\)-axis. -
Question 21 of 51
21. Question
Add vectors \(\vec{A}, \vec{B}\) and \(\vec{C}\) each having magnitude of 100 unit and inclined to the \(X\)-axis at angles \(45^{\circ}, 135^{\circ}\) and \(315^{\circ}\) respectively.
CorrectIncorrectHint
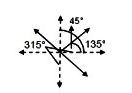
\(x\) component of \(\vec{A}=100 \cos 45^{\circ}=100 / \sqrt{2}\) unit
\(x\) component of \(\vec{B}=100 \cos 135^{\circ}=100 / \sqrt{2}\)
\(x\) component of \(\dot{C}=100 \cos 315^{\circ}=100 / \sqrt{2}\)
Resultant \(x\) component \(=100 / \sqrt{2}-100 / \sqrt{2}+100 / \sqrt{2}=100 / \sqrt{2}\)
\(y\) component of \(\vec{A}=100 \sin 45^{\circ}=100 / \sqrt{2}\) unit
\(y\) component of \(\vec{B}=100 \sin 135^{\circ}=100 / \sqrt{2}\)
\(y\) component of \(\vec{C}=100 \sin 315^{\circ}=-100 / \sqrt{2}\)
Resultant \(y\) component \(=100 / \sqrt{2}+100 / \sqrt{2}-100 / \sqrt{2}=100 / \sqrt{2}\)
Resultant \(=100\)
Tan \(\alpha=\frac{y \text { component }}{x \text { component }}=1\)
\(\Rightarrow \alpha=\tan ^{-1}(1)=45^{\circ}\)
The resultant is 100 unit at \(45^{\circ}\) with \(x\)-axis. -
Question 22 of 51
22. Question
Let \(\vec{a}=4 \vec{i}+3 \vec{j}\) and \(\vec{b}=3 \vec{i}+4 \vec{j}\). (a) Find the magnitudes of (a) \(\vec{a}\), (b) \(\vec{b}\), (c) \(\vec{a}+\vec{b}\) and (d) \(\vec{a}-\vec{b}\)
CorrectIncorrectHint
Given: \(\vec{a}=4 \vec{i}+3 \vec{j}\) and \(\vec{b}=3 \vec{i}+4 \vec{j}\)
(a) Magnitude of \(\vec{a}\) is given by \(|\vec{a}|=\sqrt{4^2+3^2}=\sqrt{16+9}=5\)
Magnitude of \(\vec{b}\) is given by \(|\vec{b}|=\sqrt{3^2+4^2}=\sqrt{9+16}=5\)
(c) \(\vec{a}+\vec{b}=(4 \hat{i}+3 \hat{j})+(3 \hat{i}+4 \hat{j})=(7 \hat{i}+7 \hat{j})\)
\(\therefore\) Magnitude of vector \(\vec{a}+\vec{b}\) is given by \(|\vec{a}+\vec{b}|=\sqrt{49+49}=\sqrt{98}=7 \sqrt{2}\)
(d) \(\vec{a}-\vec{b}=(4 \vec{i}+3 \vec{j})-(3 \vec{i}+4 \vec{j})=\vec{i}-\vec{j}\)
\(\therefore\) Magnitude of vector \(\vec{a}-\vec{b}\) is given by \(|\vec{a}-\vec{b}|=\sqrt{(1)^2+(-1)^2}=\sqrt{2}\) -
Question 23 of 51
23. Question
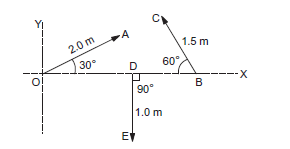
Find (a) the magnitude, (b) \(x\) and \(y\) components and (c) the angle with the \(X\)-axis of the resultant of \(\overrightarrow{O A}, \overrightarrow{B C}\) and \(\overrightarrow{D E}\).
 CorrectIncorrect
CorrectIncorrectHint
First, let us find the components of the vectors along the \(x\) and \(y\)-axes. Then we will find the resultant \(x\) and \(y\)-components.
x-component of \(\overrightarrow{O A}=2 \cos 30^{\circ}=\sqrt{3}\)
x-component of \(\overrightarrow{B C}=1.5 \cos 120^{\circ}\)
\(
=-\frac{(1.5)}{2}=-7.5
\)
\(
\begin{aligned}
& \text { x-component of } \overrightarrow{D E}=1 \cos 270^{\circ} \\
& \quad=1 \times 0=0 \mathrm{~m}
\end{aligned}
\)
\(\mathrm{y}\)-component of \(\overrightarrow{O A}=2 \sin 30^{\circ}=1\)
\(\mathrm{y}\)-component of \(\overrightarrow{B C}=1.5 \sin 120^{\circ}\)
\(
=\frac{(\sqrt{3} \times 1.5)}{2}=1.3
\)
\(
\begin{aligned}
& \text { y-component of } \overrightarrow{D E}=1 \sin 270^{\circ}=-1 \\
& \text { x-component of resultant } R_x=\sqrt{3}-0.75+0=0.98 \mathrm{~m} \\
& y \text {-component of resultant } R_y=1+1.3-1=1.3 \mathrm{~m} \\
& \therefore \text { Resultant, } R=\sqrt{\left(R_x\right)^2+\left(R_y\right)^2} \\
& =\sqrt{(0.98)^2+(1.3)^2} \\
& =\sqrt{0.96+1.69} \\
& =\sqrt{2.65} \\
& =1.6 \mathrm{~m}
\end{aligned}
\)
If it makes an angle \(\alpha\) with the positive \(x\)-axis, then
\(
\begin{aligned}
& \tan \alpha=\frac{y \text {-component }}{\mathrm{x} \text { – component }} \\
& =\frac{1.3}{0.98}=1.332 \\
& \therefore \alpha=\tan ^{-1}(1.32)
\end{aligned}
\) -
Question 24 of 51
24. Question
Two vectors have magnitudes 3 unit and 4 unit respectively. What should be the angle between them if the magnitude of the resultant is (a) 1 unit, (b) 5 unit, and (c) 7 unit.
CorrectIncorrectHint
Let the two vectors be \(\vec{a}\) and \(\vec{b}\)
Now,
\(
|\vec{a}|=3 \text { and }|\vec{b}|=4
\)
(a) If the resultant vector is 1 unit, then
\(
\begin{aligned}
& \sqrt{\vec{a}^2+\vec{b}^2+2 \cdot \vec{a} \cdot \vec{b} \cos \theta}=1 \\
& \Rightarrow \sqrt{3^2+4^2+2 \cdot 3 \cdot 4 \cos \theta}=1
\end{aligned}
\)
Squaring both sides, we get:
\(
\begin{aligned}
& 25+24 \cos \theta=1 \\
& \Rightarrow \theta=180^{\circ}
\end{aligned}
\)
Hence, the angle between them is \(180^{\circ}\).
(b) If the resultant vector is 5 units, then
\(
\begin{aligned}
& \sqrt{\vec{a}^2+\vec{b}^2+2 \cdot \vec{a} \cdot \vec{b} \cos \theta}=5 \\
& \Rightarrow \sqrt{3^2+4^2+2 \cdot 3 \cdot 4 \cos \theta}=5
\end{aligned}
\)
Squaring both sides, we get:
\(
\begin{aligned}
& 25+24 \cos \theta=25 \\
\Rightarrow & \cos \theta=90^{\circ}
\end{aligned}
\)
Hence, the angle between them is \(90^{\circ}\).
(c) If the resultant vector is 7 units, then
\(
\begin{aligned}
& \sqrt{\vec{a}^2+\vec{b}^2+2 \cdot \vec{a} \cdot \vec{b} \cos \theta}=1 \\
& \Rightarrow \sqrt{3^2+4^2+2 \cdot 3 \cdot 4 \cos \theta}=7
\end{aligned}
\)
Squaring both sides, we get:
\(
\begin{aligned}
& 25+24 \cos \theta=49, \\
& \Rightarrow \theta=\cos ^{-1} 1=0^{\circ}
\end{aligned}
\)
Hence, the angle between them is \(0^{\circ}\). -
Question 25 of 51
25. Question
A spy report about a suspected car reads as follows. “The car moved \(2.00 \mathrm{~km}\) towards east, made a perpendicular left turn, ran for \(500 \mathrm{~m}\), made a perpendicular right turn, ran for \(4.00 \mathrm{~km}\) and stopped”. Find the displacement of the car.
CorrectIncorrectHint

The displacement of the car is represented by \(\overrightarrow{A D}\).
\(
\begin{aligned}
& \overrightarrow{A D}=2 \hat{i}+0.5 \hat{j}+4 \hat{i} \\
& =6 \hat{i}+0.5 \hat{j}
\end{aligned}
\)
Magnitude of \(\overrightarrow{A D}\) is given by
\(
\begin{aligned}
& A D=\sqrt{A E^2+D E^2} \\
& =\sqrt{6^2+(0.5)^2} \\
& =\sqrt{36+0.25}=6.02 \mathrm{~km}
\end{aligned}
\)
Now,
\(
\begin{aligned}
& \tan \theta=\frac{D E}{A E}=\frac{1}{12} \\
& \Rightarrow \theta=\tan ^{-1}\left(\frac{1}{12}\right)
\end{aligned}
\)
Hence, the displacement of the car is \(6.02 \mathrm{~km}\) along the direction \(\tan ^{-1}\left(\frac{1}{12}\right)\) with positive the \(x\)-axis. -
Question 26 of 51
26. Question
A carrom board ( \(4 \mathrm{ft} \times 4 \mathrm{ft}\) square) has the queen at the centre. The queen, hit by the striker moves to the front edge, rebounds and goes in the hole behind the striking line. Find the magnitude of displacement of the queen (a) from the centre to the front edge, (b) from the front edge to the hole and (c) from the centre to the hole.
CorrectIncorrectHint
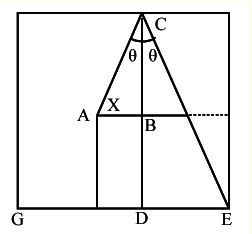
\(
\begin{aligned}
& \text { In } \triangle A B C, \tan \theta=\frac{x}{2} \\
& \text { and in } \triangle D C E, \tan \theta=\frac{2-x}{4} \\
& \tan \theta=\frac{x}{2}=\frac{(2-x)}{4} \\
& \Rightarrow 6 x=4 \\
& \Rightarrow x=\frac{2}{3} \mathrm{ft} \\
& \text { (a) } \ln \triangle A B C, A C=\sqrt{A^2+B C^2} \\
& =\sqrt{\left(\frac{2^2}{3}\right)}+2^2 \\
& =\sqrt{\frac{4}{9}+4}=\sqrt{\frac{40}{9}} \\
& =\frac{2}{3} \sqrt{10} \mathrm{ft}
\end{aligned}
\)
(b) In \(\triangle C D E, D E=2-\frac{2}{3}=\frac{6-2}{3}=\frac{4}{3} \mathrm{ft}\) since \(C D=4 \mathrm{ft}\), therefore \(C E\)
\(
\begin{aligned}
& =\sqrt{C D^2+D E^2} \\
& =\sqrt{4^2+\left(\frac{4^2}{3}\right)}=\frac{4}{3} \sqrt{10} \mathrm{ft}
\end{aligned}
\)
\(
\begin{aligned}
& \text { (c) In } \triangle A G E, A E=\sqrt{\mathrm{AG}^2+G E^2} \\
& =\sqrt{2^2+2^2} \\
& =\sqrt{8}=2 \sqrt{2} \mathrm{ft}
\end{aligned}
\) -
Question 27 of 51
27. Question
A mosquito net over a \(7 \mathrm{ft} \times 4 \mathrm{ft}\) bed is \(3 \mathrm{ft}\) high. The net has a hole at one corner of the bed through which a mosquito enters the net. It flies and sits at the diagonally opposite upper corner of the net. (a) Find the magnitude of the displacement of the mosquito. (b) Taking the hole as the origin, the length of the bed as the \(X\)-axis, its width as the \(Y\)-axis, and vertically up as the \(Z\)-axis, write the components of the displacement vector.
CorrectIncorrectHint

Displacement vector
\(
\vec{r}=7 \hat{i}+4 \hat{i}+3 \hat{k}
\)
(a) Magnitude of displacement
\(
\begin{aligned}
& =\sqrt{7^2+4^2+3^2} \\
& =\sqrt{74} \mathrm{ft}
\end{aligned}
\)
(b) Components of the displacement vector are \(7 \mathrm{ft}, 4 \mathrm{ft}\) and \(3 \mathrm{ft}\). -
Question 28 of 51
28. Question
Suppose \(\vec{a}\) is a vector of magnitude \(4.5\) unit due north. What is the vector (a) \(3 \vec{a}\), (b) \(-4 \vec{a}\)?
CorrectIncorrectHint
Given: \(\vec{a}\) is a vector of magnitude \(4.5\) units due north.
Case (a):
\(
3|\vec{a}|=3 \times 4.5=13.5
\)
\(\therefore 3 \vec{a}\) is a vector of magnitude \(13.5\) units due north.
Case (b):
\(|-4 \vec{a}|=4 \times 4.5=18\) units
\(\therefore-4 \vec{a}\) is a vector of magnitude 18 units due south. -
Question 29 of 51
29. Question
Two vectors have magnitudes \(2 \mathrm{~m}\) and \(3 \mathrm{~m}\). The angle between them is \(60^{\circ}\). Find (a) the scalar product of the two vectors, (b) the magnitude of their vector product.
CorrectIncorrectHint
Let the two vectors be \(|\vec{a}|=2 m\) and \(|\vec{b}|=3 m\).
Angle between the vectors, \(\theta=60^{\circ}\)
(a) The scalar product of two vectors is given by \(\vec{a} \cdot \vec{b}=|\vec{a}| \cdot|\vec{b}| \cos \theta^{\circ}\)
\(
\begin{aligned}
& \therefore \vec{a} \cdot \vec{b}=|\vec{a}| \cdot|\vec{b}| \cos 60^{\circ} \\
& =2 \times 3 \times \frac{1}{2}=3 m^2
\end{aligned}
\)
(b) The vector product of two vectors is given by \(|\vec{a} \times \vec{b}|=|\vec{a}||\vec{a}| \sin \theta^{\circ}\).
\(
\begin{aligned}
& \therefore|\vec{a} \times \vec{b}|=|\vec{a}||\vec{a}| \sin 60^{\circ} \\
& =2 \times 3 \times \frac{\sqrt{3}}{2} \\
& =3 \sqrt{3} m^2
\end{aligned}
\) -
Question 30 of 51
30. Question
Let \(A_1 A_2 A_3 A_4 A_5 A_6 A_1\) be a regular hexagon. Write the \(x\)-components of the vectors represented by the six sides taken in order. Find \(\cos 0+\cos \pi / 3+\cos 2 \pi / 3+\cos 3 \pi / 3+\cos 4 \pi / 3+\cos 5 \pi / 3\).
 CorrectIncorrect
CorrectIncorrectHint
According to the polygon law of vector addition, the resultant of these six vectors is zero.
Here, \(a=b=c=d=e=f\) (magnitudes), as it is a regular hexagon. A regular polygon has all sides equal to each other.
So, \(R_x=A \cos 0+A \cos \frac{\pi}{3}+A \cos \frac{2 \pi}{3}+A \cos \frac{3 \pi}{3}+A \cos \frac{4 \pi}{3}+A \cos \frac{5 \pi}{3}=0\)
[As the resultant is zero, the \(x\)-component of resultant \(R_X\) is zero]
\(
\Rightarrow \cos 0+\cos \frac{\pi}{3}+\cos \frac{2 \pi}{3}+\cos \frac{3 \pi}{3}+\cos \frac{4 \pi}{3}+\cos \frac{5 \pi}{5}=0
\) -
Question 31 of 51
31. Question
Let \(\vec{a}=2 \vec{i}+3 \vec{j}+4 \vec{k}\) and \(\vec{b}=3 \vec{i}+4 \vec{j}+5 \vec{k}\). Find the angle between them.
CorrectIncorrectHint
We have:
\(
\begin{aligned}
& \vec{a}=2 \vec{i}+3 \vec{j}+4 \vec{k} \\
& \vec{b}=3 \vec{i}+4 \vec{j}+5 \vec{k}
\end{aligned}
\)
Using scalar product, we can find the angle between vectors \(\vec{a}\) and \(\vec{b}\). i.e.,
\(\vec{a} \cdot \vec{b}=|\vec{a}||\vec{b}| \cos \theta\)
So, \(\theta=\cos ^{-1}\left(\frac{\vec{a} \cdot \vec{b}}{|\vec{a}||\vec{b}|}\right)\)
\(
=\cos ^{-1}\left(\frac{2 \times 3+3 \times 4+4 \times 5}{\sqrt{\left(2^2+3^2+4^2\right)} \sqrt{\left(3^2+4^2+5^2\right)}}\right)
\)
\(
=\cos ^{-1}\left(\frac{38}{\sqrt{29} \sqrt{50}}=\cos ^{-1} \frac{38}{\sqrt{1450}}\right)
\)
\(\therefore\) The required angle is \(\cos ^{-1} \frac{38}{\sqrt{1450}}\). -
Question 32 of 51
32. Question
\(
\text { Find } \vec{A} \cdot(\vec{A} \times \vec{B}) \text {. }
\)CorrectIncorrectHint
Vector product is given by \(\vec{A} \times \vec{B}=|\vec{A}||\vec{B}| \sin \hat{n}\)
\(
|\vec{A}||\vec{B}| \sin \hat{n}
\)
is a vector which is perpendicular to the plane containing
\(\vec{A}\) and \(\vec{B}\). This implies that it is also perpendicular to \(\vec{A}\). We know that the dot product of two perpendicular vectors is zero.
\(
\therefore \vec{A} \cdot(\vec{A} \times \vec{B})=0
\) -
Question 33 of 51
33. Question
\(
\text { If } \vec{A}=2 \vec{i}+3 \vec{j}+4 \vec{k} \text { and } \vec{B}=4 \vec{i}+3 \vec{j}+2 \vec{k} \text {, find } \vec{A} \times \vec{B}
\)CorrectIncorrectHint
Given:
\(
\begin{aligned}
& \vec{A}=2 \hat{i}+3 \hat{j}+4 \hat{k} \text { and } \\
& \vec{B}=4 \hat{i}+3 \hat{j}+2 \hat{k}
\end{aligned}
\)
The vector product of \(\vec{A} \times \vec{B}\) can be obtained as follows:
\(
\begin{aligned}
& \vec{A} \times \vec{B}=\left|\begin{array}{ccc}
\hat{i} & \hat{j} & \hat{k} \\
2 & 3 & 4 \\
4 & 3 & 2
\end{array}\right| \\
& =\hat{i}(6-12)-\hat{j}(4-16)+\hat{k}(6-12) \\
& =-6 \hat{i}+12 \hat{j}-6 \hat{k}
\end{aligned}
\) -
Question 34 of 51
34. Question
If \(\vec{A}, \vec{B}, \vec{C}\) are mutually perpendicular, show that \(\vec{C} \times(\vec{A} \times \vec{B})=0\). Is the converse true?
CorrectIncorrectHint
Given that \(\vec{A}, \vec{B}\) and \(\vec{C}\) are mutually perpendicular
\(\overrightarrow{\mathrm{A}} \times \overrightarrow{\mathrm{B}}\) is a vector which direction is perpendicular to the plane containing \(\overrightarrow{\mathrm{A}}\) and \(\overrightarrow{\mathrm{B}}\)
Also \(\vec{C}\) is perpendicular to \(\vec{A}\) and \(\vec{B}\)
Angle between \(\overrightarrow{\mathrm{C}}\) and \(\overrightarrow{\mathrm{A}} \times \overrightarrow{\mathrm{B}}\) is \(0^{\circ}\) or \(180^{\circ}\) (figure below)
So, \(\overrightarrow{\mathrm{C}} \times(\overrightarrow{\mathrm{a}} \times \overrightarrow{\mathrm{B}})=0\)
The converse is not true.
For example, if two of the vector are parallel, (figure below), then also \(\overrightarrow{\mathrm{C}} \times(\overrightarrow{\mathrm{A}} \times \overrightarrow{\mathrm{B}})=0\)
So, they need not be mutually perpendicular.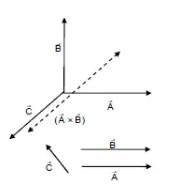
-
Question 35 of 51
35. Question
A particle moves on a given straight line with a constant speed \(v\). At a certain time it is at a point \(P\) on its straight line path. \(O\) is a fixed point. Then \(\overrightarrow{O P} \times \vec{v}\) is independent of the position \(P\). Is this true?
CorrectIncorrectHint
It is independent of the position \(\mathrm{P}\) because
The particle moves on the straight line PP’ at speed v. From the figure,
\(
\overrightarrow{\mathrm{OP}} \times v=(O P) v \sin \theta \hat{n}=v(O P) \sin \theta \hat{n}=v(O Q) \hat{n}
\)
It can be seen from the figure, \(O Q=O P \sin \theta=O P^{\prime} \sin \theta\)
So, whatever may be the position of the particle, the magnitude and direction of \(\overrightarrow{\mathrm{OP}} \times \vec{v}\) remain constant.
\(\overrightarrow{\mathrm{OP}} \times \overrightarrow{\mathrm{V}}\) is independent of the position \(\mathrm{P}\).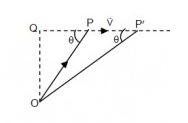
-
Question 36 of 51
36. Question
The force on a charged particle due to electric and magnetic fields is given by \(\vec{F}=q \vec{E}+q \vec{v} \times \vec{B}\). Suppose \(\vec{E}\) is along the \(X\)-axis and \(\vec{B}\) along the \(Y\)-axis. In what direction and with what minimum speed \(v\) should a positively charged particle be sent so that the net force on it is zero?
CorrectIncorrectHint
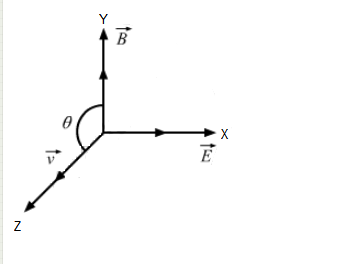
According to the problem, the net electric and magnetic forces on the particle should be zero.
i.e.,
\(
\begin{aligned}
& \vec{F}=q \vec{E}+q(\vec{\nu} \times \vec{B})=0 \\
& \Rightarrow E=-(\vec{\nu} \times \vec{B})
\end{aligned}
\)
So, the direction of \(\vec{\nu} \times \vec{B}\) should be opposite to the direction of \(\vec{E}\) Hence, \(\vec{\nu}\) should be along the positive \(z\)-direction.
Again, \(E=v B \sin \theta\)
\(
\Rightarrow \nu=\frac{E}{B} \sin \theta
\)
For \(v\) to be minimum, \(\theta=90\) and, thus, \(\nu_{\min }=\frac{E}{B}\)
So, the particle must be projected at a minimum speed of \(\frac{E}{B}\) along the \(z\)-axis. -
Question 37 of 51
37. Question
A curve is represented by \(y=\sin x\). If \(x\) is changed from \(\frac{\pi}{3}\) to \(\frac{\pi}{3}+\frac{\pi}{100}\), find approximately the change in \(y\).
CorrectIncorrectHint
\(
y=\sin x \quad \ldots(i)
\)
Now, consider a small increment \(\Delta \mathrm{x}\) in \(\mathrm{x}\). Then \(\mathrm{y}+\Delta \mathrm{y}=\sin (\mathrm{x}+\Delta \mathrm{x}) \quad\)…(ii) Here, \(\Delta y\) is the small change in \(y\).
Subtracting (ii) from (i), we get:
\(
\begin{aligned}
& \Delta y=\sin (x+\Delta x)-\sin x \\
& =\sin \left(\frac{\pi}{3}+\frac{\pi}{100}\right)-\sin \frac{\pi}{3} \\
& =0.0157
\end{aligned}
\) -
Question 38 of 51
38. Question
The electric current in a charging \(R-C\) circuit is given by \(i=i_0 e^{-t / R C}\) where \(i_0, R\) and \(C\) are constant parameters of the circuit and \(t\) is time. Find the rate of change of current at (a) \(t=0\), (b) \(t=R C\), (c) \(t=10 \mathrm{RC}\).
CorrectIncorrectHint
\(
\begin{aligned}
& \text { Given: } \mathrm{i}=\mathrm{i}_0 \mathrm{e}^{-\mathrm{t} / \mathrm{RC}} \\
& \text { Rate of change of current } \\
& =\frac{d i}{d t} \\
& =i_0\left(\frac{-1}{R C}\right) e^{-t / R C} \\
& =\frac{-i_0}{R C} \times e^{-t / R C}
\end{aligned}
\)
On applying the conditions given in the questions, we get:
(a) At \(t=0, \frac{d i}{d t}=\frac{-i_0}{R C} \times e^0=\frac{-i_0}{R C}\)
(b) At \(t=R C, \frac{d i}{d t}=\frac{-i_0}{R C} \times e^{-1}=\frac{-i_0}{R C e}\)
(c) At \(t=10 R C, \frac{d t}{d i}=\frac{-i_0}{R C} \times e^{10}=\frac{-i_0}{R C e^{10}}\) -
Question 39 of 51
39. Question
The electric current in a discharging \(\mathrm{R}-\mathrm{C}\) circuit is given by \(i=i_0 e^{-t / R C}\) where \(i_0, R\) and \(C\) are constant parameters and \(t\) is time. Let \(i_0=2.00 \mathrm{~A}, \quad R=6.00 \times 10^5 \Omega\) and \(C=0.500 \mu \mathrm{F}\). (a) Find the current at \(t=0.3 \mathrm{~s}\). (b) Find the rate of change of current at \(t=0.3 \mathrm{~s}\). (c) Find approximately the current at \(t=0.31 \mathrm{~s}\).
CorrectIncorrectHint
Electric current in a discharging R-C circuit is given by the below equation:
\(
\begin{aligned}
& i=i_0 \cdot e^{-t / R C} \quad \ldots \text {..(i) } \\
& \text { Here, } \mathrm{i}_0=2.00 \mathrm{~A} \\
& R=6 \times 10^5 \Omega \\
& C=0.0500 \times 10^{-6} \mathrm{~F} \\
& =5 \times 10^{-7} \mathrm{~F} \\
&
\end{aligned}
\)
On substituting the values of \(R, C\) and \(i_0\) in equation (i), we get:
\(
i=2.0 e^{-t / 0.3} \dots(ii)
\)
According to the question, we have:
(a) current at \(\mathrm{t}=0.3 \mathrm{~s}\)
\(
i=2 \times e^{-1}=\frac{2}{e} A
\)
(b) rate of change of current at \(t=0.3 \mathrm{~s}\)
\(
\frac{d i}{d t}=\frac{-i_0}{R C} \cdot e^{-t / R C}
\)
When \(t=0.3 \mathrm{~s}\), we have:
\(
\frac{d i}{d t}=\frac{2}{0.30} \cdot e^{\left(\frac{-0.3}{0.3}\right)}=\frac{-20}{3 e} A / s
\)
(c) approximate current at \(\mathrm{t}=0.31 \mathrm{~s}\)
\(
\begin{aligned}
& i=2 e^{\left(\frac{-0.3}{0.3}\right)} \\
& =\frac{5.8}{3 e} A(\text { approx. })
\end{aligned}
\) -
Question 40 of 51
40. Question
Find the area bounded under the curve \(y=3 x^2+6 x+7\) and the \(X\)-axis with the ordinates at \(x=5\) and \(x=10\).
CorrectIncorrectHint
The area bounded under the curve is
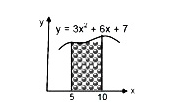
\(
y=3 x^2+6 x+7
\)
Area bounded by the curve, \(x\) axis with coordinates with \(x=5\) and \(x=10\) is given by.
\(
\text { Area } \left.\left.\left.=\int_0^y d y=\int_5^{10}\left(3 x^2+6 x+7\right) d x=3 \frac{x^3}{3}\right]_5^{10}+5 \frac{x^2}{3}\right]_5^{10}+7 x\right]_5^{10}=1135 \text { sq.units. }
\) -
Question 41 of 51
41. Question
Find the area enclosed by the curve \(y=\sin x\) and the \(X\)-axis between \(x=0\) and \(x=\pi\).
CorrectIncorrectHint
The given equation of the curve is \(y=\sin x\).
The required area can be found by integrating \(y\) w.r.t \(x\) within the proper limits.
\(
\begin{aligned}
& \text { Area }=\int_{x_1}^{x_2} \text { y } d x=\int_0^\pi \sin \mathrm{x} d x \\
& =[-\cos x]_0^\pi \\
& =-\cos \pi-(-\cos 0) \\
& =1+1=2 \text { sq. unit }
\end{aligned}
\)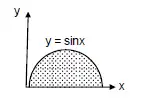
-
Question 42 of 51
42. Question
Find the area bounded by the curve \(y=e^{-x}\), the \(X\)-axis and the \(Y\)-axis.
CorrectIncorrectHint
The given function is \(y=e^{-x}\).
When \(x=0, y=e^{-0}=1\)
When \(x\) increases, the value of \(y\) decrease. Also, only when \(x=\infty, y=0\)
So, the required area can be determined by integrating the function from 0 to \(\infty\).
\(
\begin{aligned}
& \text { Area }=\int_0^{\infty} e^{-x} d x \\
& =-\left[e^{-x}\right]_0^{\infty} \\
& =-[0-1]=1 \text { sq . unit }
\end{aligned}
\) -
Question 43 of 51
43. Question
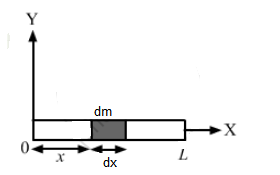
A rod of length \(L\) is placed along the \(X\)-axis between \(x=0\) and \(x=L\). The linear density (mass/length) \(\rho\) of the rod varies with the distance \(x\) from the origin as \(\rho=a+b x\). Find the mass of the rod in terms of \(a, b\) and \(L\).
CorrectIncorrectHint
Let us consider a small element of length \(\mathrm{dx}\) at a distance \(\mathrm{x}\) from the origin as shown in the figure given below:
\(
\begin{aligned}
d m & =\text { mass of the element } \\
& =\rho d x \\
& =(a+d x) d x
\end{aligned}
\)
Therefore, Mass of the rod \(=\int d m\)
\(
\begin{aligned}
& =\int_0^L(a+b x) d x \\
& =\left[a x+\frac{b x^2}{2}\right]_0^L \\
& =a L+\frac{b L^2}{2}
\end{aligned}
\)
-
Question 44 of 51
44. Question
The momentum \(p\) of a particle changes with time \(t\) according to the relation \(\frac{d p}{d t}=(10 \mathrm{~N})+(2 \mathrm{~N} / \mathrm{s}) t\). If the momentum is zero at \(t=0\), what will the momentum be at \(t=10 \mathrm{~s}\)?
CorrectIncorrectHint
According to the question, we have:
\(
\frac{d p}{d t}=(10 N)+(2 N / s) t
\)
Momentum is zero at time, \(\mathrm{t}=0\)
Now, \(d p=\left[(10 \mathrm{~N})+\left(2 \mathrm{Ns}^{-1}\right) \mathrm{t}\right] \mathrm{dt}\)
On integrating the above equation, we get:
\(
\begin{aligned}
& p=\int_0^{10} d p=\int_0^{10} 10 d t+\int_0^{10}(2 t d t) \\
& \Rightarrow p=\left[10 t+2 \frac{t^2}{2}\right]_0^{10} \\
& \Rightarrow p=10 \times 10+100-0 \\
& p=100+100=200 \mathrm{~kg} \mathrm{~m} / \mathrm{s}
\end{aligned}
\) -
Question 45 of 51
45. Question
The changes in a function \(y\) and the independent variable \(x\) are related as \(\frac{d y}{d x}=x^2\). Find \(y\) as a function of \(x\).
CorrectIncorrectHint
Changes in a function of \(y\) and the independent variable \(x\) are related as follows:
\(
\begin{aligned}
& \frac{d y}{d x}=x^2 \\
& \Rightarrow d y=x^2 d x
\end{aligned}
\)
Integrating of both sides, we get:
\(
\begin{aligned}
& \int d y=\int x^2 d x \\
& \Rightarrow y=\frac{x^3}{3}+c
\end{aligned}
\)
where \(c\) is a constant
Therefore, \(y\) as a function of \(x\) is represented by
\(
y=\frac{x^3}{3}+c
\) -
Question 46 of 51
46. Question
Write the number of significant digits in (a) 1001, (b) \(100 \cdot 1\), (c) \(100 \cdot 10\), (d) \(0 \cdot 001001\).
CorrectIncorrectHint
(a) 1001
Number of significant digits \(=4\)
(b) \(100.1\)
Number of significant digits \(=4\)
(c) \(100.10\)
Number of significant digits \(=5\)
(d) \(0.001001\)
Number of significant digits \(=4\) -
Question 47 of 51
47. Question
A metre scale is graduated at every millimetre. How many significant digits will be there in a length measurement with this scale ?
CorrectIncorrectHint
The metre scale is graduated at every millimetre.
i.e., \(1 \mathrm{~m}=1000 \mathrm{~mm}\)
The minimum number of significant digits may be one (e.g., for measurements like \(4 \mathrm{~mm}\) and \(6 \mathrm{~mm}\) ) and the maximum number of significant digits may be 4 (e.g., for measurements like \(1000 \mathrm{~mm}\) ).
Hence, the number of significant digits may be \(1,2,3\) or 4 . -
Question 48 of 51
48. Question
Round the following numbers to 2 significant digits.
(a) 3472, (b) 84.16, (c) 2.55 and (d) 28.5.CorrectIncorrectHint
(a) In 3472, 7 comes after the digit 4. Its value is greater than 5. So, the next two digits are neglectec and 4 is increased by one.
Therefore, the value becomes 3500.
(b) 84
(c) 2.6
(d) 28 -
Question 49 of 51
49. Question
The length and the radius of a cylinder measured with a slide callipers are found to be \(4.54 \mathrm{~cm}\) and \(1.75 \mathrm{~cm}\) respectively. Calculate the volume of the cylinder.
CorrectIncorrectHint
Length of the cylinder, \(l=4.54 \mathrm{~cm}\)
Radius of the cylinder, \(r=1.75 \mathrm{~cm}\)
Volume of the cylinder, \(V=\pi r^2 l=(\pi) \times(4.54) \times(1.75)^2\)
The minimum number of significant digits in a particular term is three. Therefore, the result should have three significant digits, while the other digits should be rounded off.
\(
\begin{aligned}
& \text { Volume, } V=\pi r^2 l \\
& =(3.14) \times(1.75) \times(1.75) \times(4.54) \\
& =43.6577 \mathrm{~cm}^3
\end{aligned}
\)
Since the volume is to be rounded off to 3 significant digits, we have:
\(
V=43.7 \mathrm{~cm}^3
\) -
Question 50 of 51
50. Question
The thickness of a glass plate is measured to be 2.17 mm, 2.17 mm and 2.18 mm at three different places. Find the average thickness of the plate from this data.
CorrectIncorrectHint
\(
\begin{aligned}
& \text { Average thickness }=\frac{2.17+2.17+2.18}{3} \\
& =2.1733 \mathrm{~mm}
\end{aligned}
\)
Rounding off to three significant digits, the average thickness becomes \(2.17 \mathrm{~mm}\). -
Question 51 of 51
51. Question
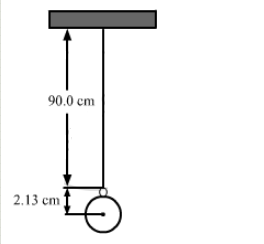
The length of the string of a simple pendulum is measured with a metre scale to be 90.0 cm. The radius of the bob plus the length of the hook is calculated to be 2.13 cm using measurements with a slide callipers. What is the effective length of the pendulum? (The
effective length is defined as the distance between the point of suspension and the centre of the bob.)CorrectIncorrectHint
Consider the figure shown below:
Actual effective length \(=(90.0+2.13) \mathrm{cm}\)
However, in the measurement \(90.0 \mathrm{~cm}\), the number of significant digits is only two.
So, the effective length should contain only two significant digits.
i.e., effective length \(=90.0+2.13=92.1 \mathrm{~cm}\).