Quiz Level-1
Quiz Summary
0 of 10 Questions completed
Questions:
Information
You have already completed the quiz before. Hence you can not start it again.
Quiz is loading…
You must sign in or sign up to start the quiz.
You must first complete the following:
Results
Results
0 of 10 Questions answered correctly
Your time:
Time has elapsed
You have reached 0 of 0 point(s), (0)
Earned Point(s): 0 of 0, (0)
0 Essay(s) Pending (Possible Point(s): 0)
Categories
- Not categorized 0%
- 1
- 2
- 3
- 4
- 5
- 6
- 7
- 8
- 9
- 10
- Current
- Review
- Answered
- Correct
- Incorrect
-
Question 1 of 10
1. Question
The number 13 is prime, and when its digits are reversed, 31 is also prime. Besides 13 and 31, how many other 2-digit primes also satisfy this condition?
CorrectIncorrectHint
The 7 other primes are 11, 17, 71, 37, 73, 79, and 97.
-
Question 2 of 10
2. Question
Writing 48 as a product of its prime factors gives:
CorrectIncorrectHint
\(
\text { Writing } 48 \text { as a product of its prime factors gives } 2 \times 2 \times 2 \times 2 \times 3 =2^4 \times 3
\) -
Question 3 of 10
3. Question
Common prime factors of 256 and 156 are:
CorrectIncorrectHint
Prime factorisation of \(256=2 \times 2 \times 2 \times 2 \times 2 \times 2 \times 2 \times 2\)
Prime factorisation of \(156=2 \times 2 \times 3 \times 13\)
The common prime factor of 256 and 156 is 2. -
Question 4 of 10
4. Question
List the common factors of 152 and 76.
CorrectIncorrectHint
Prime factorisation of \(152=2 \times 2 \times 2 \times 19\)
Prime factorisation of \(76=2 \times 2 \times 19\)
The common prime factors of 152 and 76 are 2 and 19 . -
Question 5 of 10
5. Question
Find the prime factorisation of 118724.
CorrectIncorrectHint
The prime factorisation of 118724 is 2 × 2 × 67 × 443.
-
Question 6 of 10
6. Question
Prime factorisation of 284 × Prime factorisation of 456 = Prime factorisation of (284 × 456). Is this true?
CorrectIncorrectHint
Prime factorisation of 284 = 2 × 2 × 71
Prime factorisation of 456 = 2 × 2 × 2 × 3 × 19
Prime factorisation of (283 × 456) = prime factorisation of 129504
= 2 × 2 × 2 × 2 × 2 × 3 × 19 × 71
Clearly,
Prime factorisation of 284 × Prime factorisation of 456 = Prime factorisation of (284 × 456).
-
Question 7 of 10
7. Question
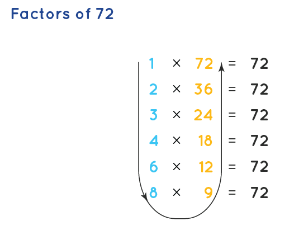
List all the positive factors of 72.
CorrectIncorrectHint
The positive factors of 72 can be listed as 1, 2, 3, 4, 6, 8, 9, 12, 18, 24, 36, and 72.
-
Question 8 of 10
8. Question
What is the Prime Factorization of 72?
CorrectIncorrectHint
\(
\text { Prime Factorization of } 72: 2 \times 2 \times 2 \times 3 \times 3 \text { or } 2^3 \times 3^2
\) -
Question 9 of 10
9. Question
What is the common factors of 72 and 71?
CorrectIncorrectHint
The factors of 72 are 1, 2, 3, 4, 6, 8, 9, 12, 18, 24, 36 and 72.
The factors of 71 are 1 and 71.
Thus, the common factor of 72 and 71 is 1
-
Question 10 of 10
10. Question
How many prime factors of 72 are there?
CorrectIncorrectHint
The prime factors shown above (2, 2, 2, 3, and 3) are completely unique to 72. When we multiply all of them together the result will be 72 and this is what we call the product of prime factors of 72. The prime factor products of 72 are listed below:
2 x 2 x 2 x 3 x 3 = 72