Exponents of (a+b)

Let us have a look at the following identities done earlier. Let us start with an exponent of 0 and build upwards.

\(
\begin{aligned}
& (a+b)^0=1  \quad a+b \neq 0\\
& (a+b)^1=a+b \\
& (a+b)^2=a^2+2 a b+b^2 \\
& (a+b)^3=a^3+3 a^2 b+3 a b^2+b^3 \\
& (a+b)^4=(a+b)^3(a+b)=a^4+4 a^3 b+6 a^2 b^2+4 a b^3+b^4
\end{aligned}
\)

In these expansions, we observe that there is a pattern.

• The total number of terms in the expansion is one more than the index. For example, in the expansion of \((a+b)^2\), the number of terms is 3 whereas the index of \((a+b)^2\) is 2.
• Powers of the first quantity ‘ \(a\) ‘ go on decreasing by 1 whereas the powers of the second quantity ‘ \(b\) ‘ increase by 1, in successive terms.
• In each term of the expansion, the sum of the indices of \(a\) and \(b\) is the same and is equal to the index of \(a+b\).

The Pattern

Let’s take the example of cube of \((a+b)\)
\(
a^3+3 a^2 b+3 a b^2+b^3
\)
Now, notice the exponents of a. They start at 3 and go down: \(3,2,1,0\) :
\(
a^3+3 a^2 b+3 a b^2+b^3
\)
Likewise, the exponents of b go upwards: \(0,1,2,3\) :
\(
a^3+3 a^2 b+3 a b^2+b^3
\)
If we number the terms 0 to \(n\), we get this:
\(
\begin{array}{cccc}
k=0 & k=1 & k=2 & k=3 \\
a^3 & a^2 & a & 1 \\
1 & b & b^2 & b^3
\end{array}
\)
Which can be brought together into this:
\(
a^{n-k_b k}
\)

Coefficients

Just look at the coefficients in the expression below; we will find a pattern like this as the exponent increases.

\(
\begin{array}{lc}
(a+b)^0= & 1 \\
(a+b)^1= & a+b \\
(a+b)^2= & a^2+2 a b+b^2 \\
(a+b)^3= & a^3+3 a^2 b+3 a b^2+b^3 \\
(a+b)^4= & a^4+4 a^3 b+4 a^2 b^2+4 a b^3+b^4 \\
\end{array}
\)

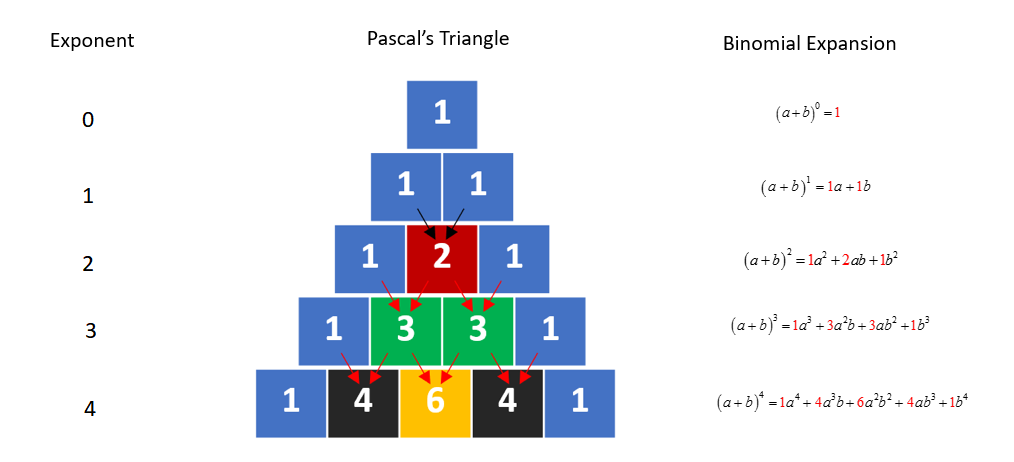
They actually form Pascal’s Triangle

Each number is just the two numbers above it added together (except for the edges, which are all “1”). It can be seen that the addition of 1’s in the row for index 1 gives rise to 2 in the row for index 2. The addition of 1, 2 and 2, 1 in the row for index 2, gives rise to 3 and 3 in the row for index 3 and so on. Also, 1 is present at the beginning and at the end of each row.

Pascal’s Triangle

This array of numbers shown above is known as Pascal’s triangle, after the name of French mathematician Blaise Pascal. It is also known as Meru Prastara by Pingla. Expansions for the higher powers of a binomial are also possible by using Pascal’s triangle.

Example 1: Let us expand \((2 x+3 y)^5\) by using Pascal’s triangle. 

Solution:

Using Pascal’s triangle we can find the row for index 5 is:      1      5      10      10      5      1

Using this row and our observations from above Pascal’s triangle, we get

\(
\begin{aligned}
(2 x+3 y)^5 & =(2 x)^5+5(2 x)^4(3 y)+10(2 x)^3(3 y)^2+10(2 x)^2(3 y)^3+5(2 x)(3 y)^4+(3 y)^5 \\
& =32 x^5+240 x^4 y+720 x^3 y^2+1080 x^2 y^3+810 x y^4+243 y^5
\end{aligned}
\)

Pascal’s triangle using the concept of combinations

We make use of the concept of combinations studied earlier to rewrite the numbers in Pascal’s triangle. We know that \({ }^n \mathrm{C}_k=\frac{n !}{k !(n-k) !}, 0 \leq k \leq n\) and \(n\) is a non-negative integer. Also, \({ }^n \mathrm{C}_0=1={ }^n \mathrm{C}_n\) The Pascal’s triangle can now be rewritten as shown below.

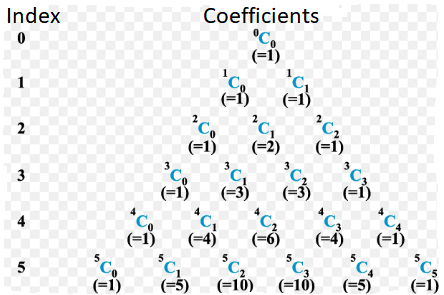
Observing this pattern, we can now write the row of the Pascal’s triangle for any index without writing the earlier rows. For example, for the index 7 the row would be

\(
{ }^7 \mathrm{C}_0   { }^7 \mathrm{C}_1   { }^7 \mathrm{C}_2   { }^7 \mathrm{C}_3   { }^7 \mathrm{C}_4   { }^7 \mathrm{C}_5   { }^7 \mathrm{C}_6   { }^7 \mathrm{C}_7 .
\)

Example 2: Let’s expand \((a+b)^7\)

Solution:

\(
(a+b)^7={ }^7 \mathrm{C}_0 a^7+7 \mathrm{C}_1 a^6 b+{ }^7 \mathrm{C}_2 a^5 b^2+{ }^7 \mathrm{C}_3 a^4 b^3+7 \mathrm{C}_4 a^3 b^4+{ }^7 \mathrm{C}_5 a^2 b^5+{ }^7 \mathrm{C}_6 a b^6+{ }^7 \mathrm{C}_7 b^7
\)

Proof of Bionominal theorem for any positive integer 

\(
(a+b)^n={ }^n \mathrm{C}_0 a^n+{ }^n \mathrm{C}_1 a^{n-1} b+{ }^n \mathrm{C}_2 a^{n-2} b^2+\ldots+{ }^n \mathrm{C}_{n-1} a \cdot b^{n-1}+{ }^n \mathrm{C}_n b^n
\)

The proof can be obtained by applying the principle of mathematical induction. Let the given statement be

\(
\mathrm{P}(n):(a+b)^n={ }^n \mathrm{C}_0 a^n+{ }^n \mathrm{C}_1 a^{n-1} b+{ }^n \mathrm{C}_2 a^{n-2} b^2+\ldots+{ }^n \mathrm{C}_{n-1} a \cdot b^{n-1}+{ }^n \mathrm{C}_n b^n
\)
For \(n=1\), we have
\(
\mathrm{P}(1):(a+b)^1={ }^1 \mathrm{C}_0 a^1+{ }^1 \mathrm{C}_1 b^1=a+b
\)
Thus, \(\mathrm{P}(1)\) is true.
Suppose \(\mathrm{P}(k)\) is true for some positive integer \(k\), i.e.
\(
(a+b)^k={ }^k \mathrm{C}_0 a^k+{ }^k \mathrm{C}_1 a^{k-1} b+{ }^k \mathrm{C}_2 a^{k-2} b^2+\ldots+{ }^k \mathrm{C}_k b^k \dots(1)
\)
We shall prove that \(\mathrm{P}(k+1)\) is also true, i.e.,
\(
(a+b)^{k+1}={ }^{k+1} \mathrm{C}_0 a^{k+1}+{ }^{k+1} \mathrm{C}_1 a^k b+{ }^{k+1} \mathrm{C}_2 a^{k-1} b^2+\ldots+{ }^{k+1} \mathrm{C}_{k+1} b^{k+1}
\)
Now, \((a+b)^{k+1}=(a+b)(a+b)^k\)
\(
=(a+b)\left({ }^k \mathrm{C}_0 a^k+{ }^k \mathrm{C}_1 a^{k-1} b+{ }^k \mathrm{C}_2 a^{k-2} b^2+\ldots+{ }^k \mathrm{C}_{k-1} a b^{k-1}+{ }^k \mathrm{C}_k b^k\right)
\)
[from (1)]
\(
\begin{aligned}
= & { }^k \mathrm{C}_0 a^{k+1}+{ }^k \mathrm{C}_1 a^k b+{ }^k \mathrm{C}_2 d^{k-1} b^2+\ldots+{ }^k \mathrm{C}_{k-1} a^2 b^{k-1}+{ }^k \mathrm{C}_k a b^k+{ }^k \mathrm{C}_0 a^k b \\
& +{ }^k \mathrm{C}_1 a^{k-1} b^2+{ }^k \mathrm{C}_2 a^{k-2} b^3+\ldots+{ }^k \mathrm{C}_{k-1} a b^k+{ }^k \mathrm{C}_k b^{k+1}
\end{aligned}
\)
[by actual multiplication]
\(
\begin{aligned}
& ={ }^k \mathrm{C}_0 a^{k+1}+\left({ }^k \mathrm{C}_1+{ }^k \mathrm{C}_0\right) a^k b+\left({ }^k \mathrm{C}_2+{ }^k \mathrm{C}_1\right) a^{k-1} b^2+\ldots \\
& +\left({ }^k \mathrm{C}_k+{ }^k \mathrm{C}_{k-1}\right) a b^k+{ }^k \mathrm{C}_k b^{k+1} \\
\end{aligned}
\)
[by grouping like terms]
\(
={ }^{k+1} \mathrm{C}_0 a^{k+1}+{ }^{k+1} \mathrm{C}_1 a^k b+{ }^{k+1} \mathrm{C}_2 a^{k-1} \mathrm{~b}^2+\ldots+{ }^{k+1} \mathrm{C}_k a b^k+{ }^{k+1} \mathrm{C}_{k+1} b^{k+1}
\)
\(
\text { (by using }{ }^{k+1} \mathrm{C}_0=1,{ }^k \mathrm{C}_r+{ }^k \mathrm{C}_{r-1}={ }^{k+1} \mathrm{C}_r \quad \text { and }{ }^k \mathrm{C}_k=1={ }^{k+1} \mathrm{C}_{k+1} \text { ) }
\)
Thus, it has been proved that \(\mathrm{P}(k+1)\) is true whenever \(\mathrm{P}(k)\) is true. Therefore, by the principle of mathematical induction, \(\mathrm{P}(n)\) is true for every positive integer \(n\).

Example 3: Expand using binomial theorem \((x+2)^6\)

Solution:

\(
\begin{aligned}
(x+2)^6 & ={ }^6 \mathrm{C}_0 x^6+{ }^6 \mathrm{C}_1 x^5 \cdot 2+{ }^6 \mathrm{C}_2 x^4 2^2+{ }^6 \mathrm{C}_3 x^3 \cdot 2^3+{ }^6 \mathrm{C}_4 x^2 \cdot 2^4+{ }^6 \mathrm{C}_5 x \cdot 2^5+{ }^6 \mathrm{C}_6 \cdot 2^6 . \\
& =x^6+12 x^5+60 x^4+160 x^3+240 x^2+192 x+64
\end{aligned}
\)
Thus \((x+2)^6=x^6+12 x^5+60 x^4+160 x^3+240 x^2+192 x+64\).

Properties of Binomial Theorem

Some of the properties of the binomial theorem which helps to solve a lot of problems are as follows: For the sake of convenience, the coefficients \({ }^n C_0,{ }^n C_1, \ldots .{ }^n C_n\) are usually represented as \(C_0, C_1, \ldots, C_n\).
1. \(C_0+C_1+C_2+\ldots+C_n=2^n\)
2. \(C_0-C_1+C_2-\ldots+(-1)^n C_n=0\)
3. \(C_0+C_2+C_4+\ldots=C_1+C_3+C_5+\ldots=2^{n-1}\)
4. \({ }^n C_k={ }^n C_{n-k}\)
5. \(k\left({ }^n C_k\right)=n^{n-1} C_{k-1}\)
6. \(\frac{{ }^n C_k}{k+1}=\frac{{ }^{n+1} C_{k+1}}{n+1}\)
7. \({ }^n C_k+{ }^n C_{k-1}={ }^{n+1} C_k\)
Where \(n \in N, k \in W\) and \(k \leq n\)

Some special cases

Case-1: In the expansion of \((a+b)^n\) taking \(a=x \text { and } b=-y\).

\(
\begin{aligned}
(x-y)^n & =[x+(-y)]^n \\
& ={ }^n \mathrm{C}_0 x^n+{ }^n \mathrm{C}_1 x^{n-1}(-y)+{ }^n \mathrm{C}_2 x^{n-2}(-y)^2+{ }^n \mathrm{C}_3 x^{n-3}(-y)^3+\ldots+{ }^n \mathrm{C}_n(-y)^n \\
& ={ }^n \mathrm{C}_0 x^n-{ }^n \mathrm{C}_1 x^{n-1} y+{ }^n \mathrm{C}_2 x^{n-2} y^2-{ }^n \mathrm{C}_3 x^{n-3} y^3+\ldots+(-1)^n{ }^n \mathrm{C}_n y^n
\end{aligned}
\)
Thus \((x-y)^n={ }^n \mathrm{C}_0 x^n-{ }^n \mathrm{C}_1 x^{n-1} y+{ }^n \mathrm{C}_2 x^{n-2} y^2+\ldots+(-1)^n{ }^n \mathrm{C}_n y^n\)

Example 4: Evaluate \((x-2 y)^5\)

Solution:

\(
\begin{aligned}
(x-2 y)^5= & { }^5 \mathrm{C}_0 x^5-{ }^5 \mathrm{C}_1 x^4(2 y)+{ }^5 \mathrm{C}_2 x^3(2 y)^2-{ }^5 \mathrm{C}_3 x^2(2 y)^3+ \\
& { }^5 \mathrm{C}_4 x(2 y)^4-{ }^5 \mathrm{C}_5(2 y)^5 \\
= & x^5-10 x^4 \mathrm{y}+40 x^3 y^2-80 x^2 y^3+80 x y^4-32 y^5 .
\end{aligned}
\)

Case-2: In the expansion of \((a+b)^n\) taking \(a=1 \text { and } b=x\).

\(
\begin{aligned}
(1+x)^n & ={ }^n \mathrm{C}_0(1)^n+{ }^n \mathrm{C}_1(1)^{n-1} x+{ }^n \mathrm{C}_2(1)^{n-2} x^2+\ldots+{ }^n \mathrm{C}_n x^n \\
& ={ }^n \mathrm{C}_0+{ }^n \mathrm{C}_1 x+{ }^n \mathrm{C}_2 x^2+{ }^n \mathrm{C}_3 x^3+\ldots+{ }^n \mathrm{C}_n x^n
\end{aligned}
\)
Thus \(\quad(1+x)^n={ }^n \mathrm{C}_0+{ }^n \mathrm{C}_1 x+{ }^n \mathrm{C}_2 x^2+{ }^n \mathrm{C}_3 x^3+\ldots+{ }^n \mathrm{C}_n x^n\)
In particular, for \(x=1\), we have
\(
2^n={ }^n \mathrm{C}_0+{ }^n \mathrm{C}_1+{ }^n \mathrm{C}_2+\ldots+{ }^n \mathrm{C}_n
\)

Case-3: In the expansion of \((a+b)^n\) taking \(a=1 \text { and } b=-x\).

\(
(1-x)^n={ }^n \mathrm{C}_0-{ }^n \mathrm{C}_1 x+{ }^n \mathrm{C}_2 x^2-\ldots+(-1)^n{ }^n \mathrm{C}_n x^n
\)
In particular, for \(x=1\), we get
\(
0={ }^n \mathrm{C}_0-{ }^n \mathrm{C}_1+{ }^n \mathrm{C}_2-\ldots+(-1)^n{ }^n \mathrm{C}_n
\)

Example 5: \(\text { Expand }\left(x^2+\frac{3}{x}\right)^4, x \neq 0\)

Solution:

By using binomial theorem, we have
\(
\begin{aligned}
\left(x^2+\frac{3}{x}\right)^4 & ={ }^4 \mathrm{C}_0\left(x^2\right)^4+{ }^4 \mathrm{C}_1\left(x^2\right)^3\left(\frac{3}{x}\right)+{ }^4 \mathrm{C}_2\left(x^2\right)^2\left(\frac{3}{x}\right)^2+{ }^4 \mathrm{C}_3\left(x^2\right)\left(\frac{3}{x}\right)^3+{ }^4 \mathrm{C}_4\left(\frac{3}{x}\right)^4 \\
& =x^8+4 \cdot x^6 \cdot \frac{3}{x}+6 \cdot x^4 \cdot \frac{9}{x^2}+4 \cdot x^2 \cdot \frac{27}{x^3}+\frac{81}{x^4} \\
& =x^8+12 x^5+54 x^2+\frac{108}{x}+\frac{81}{x^4} .
\end{aligned}
\)

Example 6: \(\text { Compute }(98)^5\)

Solution:

We express 98 as the sum or difference of two numbers whose powers are easier to calculate, and then use Binomial Theorem.
Write \(98=100-2\)
Therefore, \((98)^5=(100-2)^5\)
\(
\begin{aligned}
= & { }^5 \mathrm{C}_0(100)^5-{ }^5 \mathrm{C}_1(100)^4 \cdot 2+{ }^5 \mathrm{C}_2(100)^3 2^2 \\
& -{ }^5 \mathrm{C}_3(100)^2(2)^3+{ }^5 \mathrm{C}_4(100)(2)^4-{ }^5 \mathrm{C}_5(2)^5 \\
= & 10000000000-5 \times 100000000 \times 2+10 \times 1000000 \times 4-10 \times 10000 \\
& \times 8+5 \times 100 \times 16-32 \\
= & 10040008000-1000800032=9039207968 .
\end{aligned}
\)

Example 7: \(\text { Which is larger }(1.01)^{1000000} \text { or } 10,000 ?\)

Solution:

Splitting 1.01 and using binomial theorem to write the first few terms we have

\(
\begin{aligned}
(1.01)^{1000000} & =(1+0.01)^{1000000} \\
& ={ }^{1000000} \mathrm{C}_0+{ }^{10000000} \mathrm{C}_1(0.01)+\text { other positive terms } \\
& =1+1000000 \times 0.01+\text { other positive terms } \\
& =1+10000+\text { other positive terms } \\
& >10000
\end{aligned}
\)
Hence \(\quad(1.01)^{1000000}>10000\)

Example 8: Using binomial theorem, prove that \(6^n-5 n\) always leaves remainder 1 when divided by 25 .

Solution:

For two numbers \(a\) and \(b\) if we can find numbers \(q\) and \(r\) such that \(a=b q+r\), then we say that \(b\) divides \(a\) with \(q\) as quotient and \(r\) as remainder. Thus, in order to show that \(6^n-5 n\) leaves remainder 1 when divided by 25 , we prove that \(6^n-5 n=25 k+1\), where \(k\) is some natural number.
We have
\(
(1+a)^n={ }^n \mathrm{C}_0+{ }^n \mathrm{C}_1 a+{ }^n \mathrm{C}_2 a^2+\ldots+{ }^n \mathrm{C}_n a^n
\)
For \(a=5\), we get
\(
(1+5)^n={ }^n \mathrm{C}_0+{ }^n \mathrm{C}_1 5+{ }^n \mathrm{C}_2 5^2+\ldots+{ }^n \mathrm{C}_n 5^n
\)
i.e. \(\quad(6)^n=1+5 n+5^{2 n} \cdot \mathrm{C}_2+5^3 \cdot{ }^n \mathrm{C}_3+\ldots+5^n\)
i.e. \(\quad 6^n-5 n=1+5^2\left({ }^n \mathrm{C}_2+{ }^n \mathrm{C}_3 5+\ldots+5^{n-2}\right)\)
or \(\quad 6^n-5 n=1+25\left({ }^n \mathrm{C}_2+5 \cdot{ }^n \mathrm{C}_3+\ldots+5^{n-2}\right)\)
or \(\quad 6^n-5 n=25 k+1 \quad\) where \(k={ }^n \mathrm{C}_2+5 \cdot{ }^n \mathrm{C}_3+\ldots+5^{n-2}\).
This shows that when divided by \(25,6^n-5 n\) leaves remainder 1.

Example 9: What will be the remainder when \(7^{103}\) is divided by 25

Solution:

\(
\begin{aligned}
& 7^{103}=7(50-1)^{51}=7\left(50^{51-}{ }^{51} C_1 50^{50}+{ }^{51} C_2 50^{49}-\ldots-1\right) \\
& =7\left(50^{51-}{ }^{51} C_1 50^{50}+\ldots+{ }^{51} C_{50} 50\right)-7-18+18 \\
& =7\left(50^{51-}{ }^{51} C_1 50^{50}+\ldots+{ }^{51} C_{50} 50\right)-25+18 \\
& \Rightarrow \text { remainder is } 18 .
\end{aligned}
\)

Example 10: \(\text { What will be the last two digits of } 11^{25} \text { ? }\)

Solution:

\(
\begin{aligned}
& 11^{25}=(1+10)^{25}=\left(\begin{array}{c}
25 \\
0
\end{array}\right)+10 \cdot\left(\begin{array}{c}
25 \\
1
\end{array}\right)+\sum_{k=2}^{25}\left(\begin{array}{c}
25 \\
k
\end{array}\right) \cdot 10^k \\
& =1+25 \cdot 10+\sum_{k=2}^{25}\left(\begin{array}{c}
25 \\
k
\end{array}\right) \cdot 10^k \\
& =1+250+10^2 \cdot\left(\sum_{k=2}^{25}\left(\begin{array}{c}
25 \\
k
\end{array}\right) \cdot 10^{k-2}\right)
\end{aligned}
\)
Hence, the last two digits are 5 and 1

Example 11: If \(n\) is a positive integer, prove that \(3^{3 n}-26 n-1\) is divisible by 676

Solution:

\(
\begin{aligned}
& \left(3^{3 n}-26^n-1\right)=(27)^n-26 n-1 \\
& =(1+26)^n-26 n-1 \\
& =1+26 n+(26)^2\left[{ }^n C_2+26^n C_3+—+(26)^{n-2}\right]-26 n-1 \\
& \text { Let }\left[{ }^n C_2+26^n C_3+—+(26)^{n-2}\right]=k \\
& =676 \mathrm{k} \\
& \therefore\left(3^{3 n}-26 n-1\right) \text { is divisible by } 676
\end{aligned}
\)

Example 12: Evaluate the following expression \((1+2 \sqrt{x})^5+(1-2 \sqrt{x})^5\)

Solution:

\((1+2 \sqrt{x})^5+(1-2 \sqrt{x})^5\)
\(
\begin{aligned}
& =\left[{ }^5 C_0+{ }^5 C_1(2 \sqrt{x})+{ }^5 C_2(2 \sqrt{x})^2+–+{ }^5 C_5(2 \sqrt{x})^5\right] \\
& +\left[{ }^5 C_0-{ }^5 C_1(2 \sqrt{x})+{ }^5 C_2(2 \sqrt{x})^2+–{ }^5 C_5(2 \sqrt{x})^5\right] \\
& =2\left[{ }^5 C_0+{ }^5 C_2(2 \sqrt{x})^2+{ }^5 C_4(2 \sqrt{x})^4\right] \\
& =2\left[1+10 \times 4 \times x+5 \times 16 x^2\right] \\
& =160 x^2+80 x+2
\end{aligned}
\)