NCERT Exemplar MCQs
Summary
- A number of the form \(a+i b\), where \(a\) and \(b\) are real numbers, is called a complex number, \(a\) is called the real part and \(b\) is called the imaginary part of the complex number.
- Let \(z_1=a+i b\) and \(z_2=c+i d\). Then
(i) \(z_1+z_2=(a+c)+i(b+d)\)
(ii) \(z_1 z_2=(a c-b d)+i(a d+b c)\) - For any non-zero complex number \(z=a+i b(a \neq 0, b \neq 0)\), there exists the complex number \(\frac{a}{a^2+b^2}+i \frac{-b}{a^2+b^2}\), denoted by \(\frac{1}{z}\) or \(z^{-1}\), called the multiplicative inverse of \(z\) such that \((a+i b) (\frac{a}{a^2+b^2}+i \frac{-b}{a^2+b^2})=1+i 0\) \(=1\)
- For any integer \(k, i^{4 k}=1, i^{4 k+1}=i, i^{4 k+2}=-1, i^{4 k+3}=-i\)
- The conjugate of the complex number \(z=a+i b\), denoted by \(\bar{z}\), is given by \(\bar{z}=a-i b\).
Solution of a quadratic equation
The equations \(a x^2+b x+c=0\), where \(a, b\) and \(c\) are numbers (real or complex, \(a \neq 0\) ) is called the general quadratic equation in variable \(x\). The values of the variable satisfying the given equation are called roots of the equation.
The quadratic equation \(a x^2+b x+c=0\) with real coefficients has two roots given by \(\frac{-b+\sqrt{ D }}{2 a}\) and \(\frac{-b-\sqrt{ D }}{2 a}\), where \(D =b^2-4 a c\), called the discriminant of the equation.
- When \(D=0\), roots of the quadratic equation are real and equal. When \(D>0\), roots are real and unequal. Further, if \(a, b, c \in Q\) and \(D\) is a perfect square, then the roots of the equation are rational and unequal, and if \(a, b, c \in Q\) and \(D\) is not a perfect square, then the roots are irrational and occur in pair. When \(D <0\), roots of the quadratic equation are non real (or complex).
- Let \(\alpha, \beta\) be the roots of the quadratic equation \(a x^2+b x+c=0\), then sum of the roots
\((\alpha+\beta)=\frac{-b}{a}\) and the product of the roots \((\alpha, \beta)=\frac{c}{a}\).
- Let \(S\) and \(P\) be the sum of roots and product of roots, respectively, of a quadratic equation. Then the quadratic equation is given by \(x^2-S x+P=0\).
Quiz Summary
0 of 42 Questions completed
Questions:
Information
You have already completed the quiz before. Hence you can not start it again.
Quiz is loading…
You must sign in or sign up to start the quiz.
You must first complete the following:
Results
Results
0 of 42 Questions answered correctly
Your time:
Time has elapsed
You have reached 0 of 0 point(s), (0)
Earned Point(s): 0 of 0, (0)
0 Essay(s) Pending (Possible Point(s): 0)
Categories
- Not categorized 0%
- 1
- 2
- 3
- 4
- 5
- 6
- 7
- 8
- 9
- 10
- 11
- 12
- 13
- 14
- 15
- 16
- 17
- 18
- 19
- 20
- 21
- 22
- 23
- 24
- 25
- 26
- 27
- 28
- 29
- 30
- 31
- 32
- 33
- 34
- 35
- 36
- 37
- 38
- 39
- 40
- 41
- 42
- Current
- Review
- Answered
- Correct
- Incorrect
-
Question 1 of 42
1. Question
For any two complex numbers \(z_1, z_2\) and any real numbers \(a, b\), \(\left|a z_1-b z_2\right|^2+\left|b z_1+a z_2\right|^2=\ldots \ldots\)
CorrectIncorrectHint
(a)
\(
\begin{aligned}
\mid a z_1 &-\left.b z_2\right|^2+\left|b z_1+a z_2\right|^2 \\
&=\left|a z_1\right|^2+\left|b z_2\right|^2-2 \operatorname{Re}\left(a z_1 \times b \bar{z}_2\right)+\left|b z_1\right|^2+\left|a z_2\right|^2+2 \operatorname{Re}\left(b z_1 \times a \bar{z}_2\right) \\
\quad &=\left(a^2+b^2\right)\left|z_1\right|^2+\left(a^2+b^2\right)\left|z_2\right|^2=\left(a^2+b^2\right)\left(\left|z_1\right|^2+\left|z_2\right|^2\right)
\end{aligned}
\) -
Question 2 of 42
2. Question
\(
\text { The value of } \sqrt{-25} \times \sqrt{-9} \text { is }
\)CorrectIncorrectHint
(b)
\(
\sqrt{-25} \times \sqrt{-9}=i \sqrt{25} \times i \sqrt{9}=i^2(5 \times 3)=-15
\) -
Question 3 of 42
3. Question
\(
\text { The number } \frac{(1-i)^3}{1-i^3} \text { is equal to }
\)CorrectIncorrectHint
(d)
\(
\frac{(1-i)^3}{1-i^3}=\frac{(1-i)^3}{(1-i)\left(1+i+i^2\right)}=\frac{(1-i)^2}{i}=\frac{1+i^2-2 i}{i}=\frac{-2 i}{i}=-2
\) -
Question 4 of 42
4. Question
\(
\text { The sum of the series } i+i^2+i^3+\ldots \text { up to } 1000 \text { terms is }
\)CorrectIncorrectHint
(c)
\(i+i^2+i^3+\ldots\) upto 1000 terms
\(=\left(i+i^2+i^3+i^4\right)+\left(i^5+i^6+i^7+i^8\right)+\ldots 250\) brackets
\(=0+0+0 \ldots+0 \quad\left[\because i^n+i^{n+1}+i^{n+2}+i^{n+3}=0\right.\), where \(\left.n \in N\right]\) -
Question 5 of 42
5. Question
\(
\text { Multiplicative inverse of } 1+i \text { is }
\)CorrectIncorrectHint
(a)
\(
\text { Multiplicative inverse of } 1+i=\frac{1}{1+i}=\frac{1-i}{1-i^2}=\frac{1}{2}(1-i)
\) -
Question 6 of 42
6. Question
If \(z_1\) and \(z_2\) are complex numbers such that \(z_1+z_2\) is a real number, then \(z_2=\ldots\)
CorrectIncorrectHint
(a)
Let \(z_1=x_1+i y_1\) and \(z_2=x_2+i y_2\) \(z_1+z_2=\left(x_1+x_2\right)+i\left(y_1+y_2\right)\), which is real \(\Rightarrow y_1+y_2=0 \Rightarrow y_1=-y_2\)
\(\because z_2=x_1-i y_1 \quad\) [Assuming \(x_1=x_2\) ] \(\Rightarrow \quad z_2=\bar{z}_1\) -
Question 7 of 42
7. Question
\(
\arg (z)+\arg \bar{z}(\bar{z} \neq 0) \text { is } \dots
\)CorrectIncorrectHint
(b)
\(
\arg (z)+\arg (\bar{z})=\theta+(-\theta)=0
\) -
Question 8 of 42
8. Question
\(
\text { If }|z+4| \leq 3 \text {, then the greatest and least values of }|z+1| \text { are } \ldots . . \text { and } \ldots . .
\)CorrectIncorrectHint
(d) Given that, \(|z+4| \leq 3\)
For the greatest value of \(|z+1|\),
\(
|z+1|=|z+4-3|
\)
So, greatest value of \(|z+1|\) is 6 .
We know that the least value of the modulus of a complex number is zero. So, the least value of \(|z+1|\) is zero. -
Question 9 of 42
9. Question
If \(\left|\frac{z-2}{z+2}\right|=\frac{\pi}{6}\) then the locus of \(z\) is …..
CorrectIncorrectHint
(a) We have \(\left|\frac{z-2}{z+2}\right|=\frac{\pi}{6}\)
\(
\Rightarrow \quad \frac{|x+i y-2|}{|x+i y+2|}=\frac{\pi}{6} \Rightarrow \frac{|x-2+i y|}{|x+2+i y|}=\frac{\pi}{6}
\)
\(
\begin{aligned}
&\Rightarrow \quad 6 \mid x-2+i y]=\pi|x+2+i y| \Rightarrow 36|x-2+i y|^2=\pi^2|x+2+i y|^2 \\
&\Rightarrow \quad 36\left[x^2-4 x+4+y^2\right]=\pi^2\left[x^2+4 x+4+y^2\right]
\end{aligned}
\)
\(\Rightarrow \quad\left(36-\pi^2\right) x^2+\left(36-\pi^2\right) y^2-\left(144+4 \pi^2\right) x+144-4 \pi^2=0\), which is a circle. -
Question 10 of 42
10. Question
\(
\text { If }|z|=4 \text { and } \arg (z)=\frac{5 \pi}{6} \text {, then } z= \dots
\)CorrectIncorrectHint
(a) Let \(z=|z|(\cos \theta+i \sin \theta)\)
Where \(\theta=\arg (z)\)
Given that \(|z|=4\) and \(\arg (z)=\frac{5 \pi}{6}\)
\(
\begin{aligned}
\Rightarrow \quad z &=4\left[\cos \frac{5 \pi}{6}+i \sin \frac{5 \pi}{6}\right] \\
&=4\left[-\frac{\sqrt{3}}{2}+i \frac{1}{2}\right]=-2 \sqrt{3}+2 i
\end{aligned}
\) -
Question 11 of 42
11. Question
State True or False for the following
The order relation is defined on the set of complex numbers.
CorrectIncorrectHint
(b) False
We can compare two complex numbers when they are purely real. Otherwise, the comparison of complex numbers is not possible or has no meaning. -
Question 12 of 42
12. Question
State True or False for the following:
Multiplication of a non-zero complex number by \(-i\) rotates the point about origin through a right angle in the anti-clockwise direction.CorrectIncorrectHint
Ans: False
Let \(z=x+y i\)
\(z . i=(x+y i) i=x i-y\)
Which rotates at angle of \(180^{\circ}\) -
Question 13 of 42
13. Question
State True or False for the following :
\(
\text { For any complex number } z \text { the minimum value of }|z|+|z-1| \text { is } 1 \text {. }
\)CorrectIncorrectHint
(a) True
\(
|z|+|z-1|
\)
We know that \(\left|z_1\right|+\left|z_2\right| \geq\left|z_1-z_1\right|\)
\(
\Rightarrow \quad|z|+|z-1| \geq|z-(z-1)| \quad \Rightarrow|z|+|z-1| \geq 1
\)
So, minimum value of \(|z|+|z-1|\) is 1 . -
Question 14 of 42
14. Question
State True or False for the following :
locus represented by \(|z-1|=|z-i|\) is a line perpendicular to the join of \((1,0)\) and \((0,1)\)
CorrectIncorrectHint
(a) True
We have, \(|z-1|=|z-\mathrm{i}|\)
Putting \(z=x+i y\), we get
\(
\begin{aligned}
&\Rightarrow \quad|x-1+i y|=|x-i(1-y)| \\
&\Rightarrow \quad(x-1)^2+y^2=x^2+(1-y)^2 \Rightarrow x^2-2 x+1+y^2=x^2+1+y^2-2 y \\
&\Rightarrow \quad-2 x+1=1-2 y \quad \Rightarrow-2 x+2 y=0 \quad \Rightarrow x-y=0
\end{aligned}
\)
Now, equation of a line through the points \((1,0)\) and \((0,1)\) is:
\(
y-0=\frac{1-0}{0-1}(x-1)
\)
or \(\quad x+y=1\)
This line is perpendicular to the line \(x-y=0\). -
Question 15 of 42
15. Question
State True or False for the following :
\(
\text { If } z \text { is a complex number such that } z \neq 0 \text { and } \operatorname{Re}(z)=0 \text {, then } \operatorname{Im}\left(z^2\right)=0 \text {. }
\)CorrectIncorrectHint
(b) True
\(
\begin{aligned}
&z=x+i y \\
&\operatorname{Re} (z)=0 \quad x=0 \\
&z=i y \\
&z^2=i^2 y^2 \\
&=-y^2 \\
&\operatorname{Im}\left(z^2\right)=0
\end{aligned}
\) -
Question 16 of 42
16. Question
State True or False for the following :
\(
\text { The inequality }|z-4|<|z-2| \text { represents the region given by } x>3 \text {. }
\)CorrectIncorrectHint
(a) True
Given that: \(|z-4|<|z-2|\)
Let \(z=x+y i\)
\(
\begin{aligned}
&\Rightarrow|x+y i-4|<|x+y i-2| \\
&\Rightarrow|(x-4)+y i|<|(x-2)+y i| \\
&\Rightarrow \sqrt{(x-4)^2+y^2}<\sqrt{(x-2)^2+y^2} \\
&\Rightarrow(x-4)^2+y^2<(x-2)^2+y^2 \\
&\Rightarrow(x-4)^2<(x-2)^2 \\
&\Rightarrow x^2+16-8 x<x^2+4-4 x \\
&\Rightarrow-8 x+4 x<-16+4 \\
&\Rightarrow-4 x<-12 \\
&\Rightarrow x>3
\end{aligned}
\) -
Question 17 of 42
17. Question
State True or False for the following :
Let \(z_1\) and \(z_2\) be two complex numbers such that \(\left|z_1+z_2\right|=\left|z_1\right|+\left|z_2\right|\), then \(\arg \left(z_1-z_2\right)=0\)
CorrectIncorrectHint
(a) False
Explanation:
Given \(\left|z_1+z_2\right|=\left|z_1\right|+\left|z_2\right|\)
\(
\begin{aligned}
&\Rightarrow\left|z_1+z_2\right|^2=\left|z_1\right|^2+\left|z_2\right|^2+2\left|z_1\right|\left|z_2\right| \\
&\Rightarrow\left|z_1\right|^2+\left|z_2\right|^2+2 \operatorname{Re}\left(z_1 \bar{z}_2\right)=\left|z_1\right|^2+\left|z_2\right|^2+2\left|z_1\right|\left|z_2\right| \\
&\Rightarrow 2 \operatorname{Re}\left(z_1 \bar{z}_2\right)=2\left|z_1\right|\left|z_2\right| \\
&\Rightarrow \cos \left(\theta_1-\theta_2\right)=1 \\
&\Rightarrow \theta_1-\theta_2=0 \\
&\therefore \arg \left(z_1\right)-\arg \left(z_2\right)=0
\end{aligned}
\) -
Question 18 of 42
18. Question
State True or False for the following :
2 is not a complex number.
CorrectIncorrectHint
(a) False
\(
\text { Since, every real number is a complex number. So, } 2 \text { is a complex number. Hence, it is false. }
\) -
Question 19 of 42
19. Question
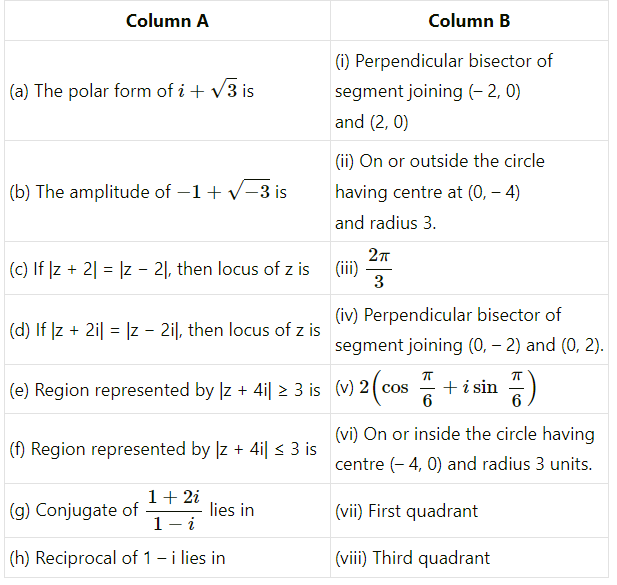 CorrectIncorrect
CorrectIncorrectHint
(a) Given that, \(z=i+\sqrt{3}\).
So, \(|z|=|i+\sqrt{3}|=\sqrt{1^2+(\sqrt{3})^2}=2\)
Also, \(z\) lies in first quadrant.
\(
\Rightarrow \arg (z)=\tan ^{-1} \frac{1}{\sqrt{3}}=\frac{\pi}{6}
\)
So, the polar from of \(z\) is \(2\left(\cos \frac{\pi}{6}+i \sin \frac{\pi}{6}\right)\).
(b) We have, \(z=-1+\sqrt{-3}=-1+i \sqrt{3}\)
Here \(z\) lies in second quadrant.
\(
\Rightarrow \quad \arg (z)=\operatorname{amp}(z)=\pi-\tan ^{-1}\left|\frac{\sqrt{3}}{-1}\right|=\pi-\tan ^{-1} \sqrt{3}=\pi-\frac{\pi}{3}=\frac{2 \pi}{3}
\)
(c) Given that, \(|z+2|=|z-2|\)
\(\Rightarrow \quad|x+2+i y|=|x-2+i y|\)
\(\Rightarrow \quad(x+2)^2+y^2=(x-2)^2+y^2 \quad \Rightarrow x^2+4 x+4=x^2-4 x+4\)
\(\Rightarrow \quad 8 x=0\)
\(\therefore x=0\)
It is a straight line which is a perpendicular bisector of segment joining the points \((-2,0)\) and \((2,0)\).
1) We have \(|z+2 i|=|z-2 i|\)
Putting \(z=x+i y\), we get
\(
\begin{aligned}
&\Rightarrow|x+i(y+2)|^2=|x+i(y-2)|^2 \quad \Rightarrow x^2+(y+2)^2=x^2+(y-2)^2 \\
&\Rightarrow 4 y=0 \quad \Rightarrow y=0
\end{aligned}
\)
It is a straight line, which is a perpendicular bisector of segment joining \((0,-2)\) and \((0,2)\)
(e) Given that, \(|z+4 i| \geq 3\)
\(
\begin{array}{ll}
\Rightarrow \quad|x+i y+4 i| \geq 3 & \Rightarrow|x+i(y+4)| \geq 3 \\
\Rightarrow \quad \sqrt{x^2+(y+4)^2} \geq 3 & \Rightarrow x^2+y^2+8 y+16 \geq 9 \\
\Rightarrow \quad x^2+y^2+8 y+7 \geq 0 &
\end{array}
\)
This represents the region on or outside the circle having centre \((0,-4)\) and radius 3 .
(f) Given that, \(|z+4| \leq 3\)
\(\Rightarrow|x+i y+4| \leq 3 \quad \Rightarrow|x+4+i y| \leq 3\)
\(\Rightarrow \quad \sqrt{(x+4)^2+y^2} \leq 3 \quad \Rightarrow(x+4)^2+y^2 \leq 9\)
\(\Rightarrow \quad x^2+8 x+16+y^2 \leq 9 \quad \Rightarrow x^2+8 x+y^2+7 \leq 0\)
This represents the region on inside circle having centre \((-4,0)\) and radius 3.
(g) \(z=\frac{1+2 i}{1-i}=\frac{(1+2 i)(1+i)}{(1+i)(1+i)}=\frac{1+2 i+i+2 i^2}{1-i^2}\)
\(
=\frac{1-2+3 i}{1+1}=\frac{-1}{2}+\frac{3 i}{2}
\)
Hence, \(\bar{z}\) lies in the third quadrant.
(h) Given that, \(z=1-i\)
\(
\Rightarrow \quad \frac{1}{z}=\frac{1}{1-i}=\frac{1+i}{(1-i)(1+i)}=\frac{1+i}{1-i^2}=\frac{1}{2}(1+i)
\)
Thus, reciprocal of \(z\) lies in first quadrant. -
Question 20 of 42
20. Question
\(
\text { What is the conjugate of } \frac{2-i}{(1-2 i)^2} ?
\)CorrectIncorrectHint
(a) We have \(z=\frac{2-i}{(1-2 i)^2}\)
\(
\begin{array}{ll}
\Rightarrow \quad z & =\frac{2-i}{1+4 i^2-4 i}=\frac{2-i}{1-4-4 i}=\frac{2-i}{-3-4 i} \\
& =\frac{(2-i)}{-(3+4 i)}=-\left[\frac{(2-i)(3-4 i)}{(3+4 i)(3-4 i)}\right] \\
& =-\left(\frac{6-8 i-3 i+4 i^2}{9+16}\right)=-\frac{(-11 i+2)}{25} \\
& =\frac{-1}{25}(2-11 i)=\frac{1}{25}(-2+11 i) \\
\therefore \quad \bar{z} & =\frac{1}{25}(-2-11 i)=\frac{-2}{25}-\frac{11}{25} i
\end{array}
\) -
Question 21 of 42
21. Question
\(
\text { If }\left|z_1\right|=\left|z_2\right| \text {, is it necessary that } z_1=z_2 \text { ? }
\)CorrectIncorrectHint
Ans: not necessary
If \(\left|Z_1\right|=\left|Z_2\right|\) then \(z_1\) and \(z_2\) are at the same distance from origin.
But if \(\arg \left(z_1\right) \neq \arg \left(z_2\right)\), then \(z_1\) and \(z_2\) are different.
So, if \(\left|z_1\right|=\left|z_2\right|\), then it is not necessary that \(z_1=z_2\).
Consider \(Z_1=3+4 i\) and \(Z_2=4+3 i\) -
Question 22 of 42
22. Question
\(
\text { If } \frac{\left(a^2+1\right)^2}{2 a-i}=x+i y \text {, what is the value of } x^2+y^2 \text { ? }
\)CorrectIncorrectHint
(a)
\(
\begin{aligned}
&\left(a^2+1\right)^2 / 2 a-i=x+i y \\
&\Rightarrow \quad\left|\frac{\left.(a^2+1\right)^2}{2 a-i}\right|=|x+i y| \\
&\Rightarrow \quad \frac{\left|\left(a^2+1\right)^2\right|}{|2 a-i|}=|x+i y| \Rightarrow \frac{\left(a^2+1\right)^2}{\sqrt{(2 a)^2+(-1)^2}}=\sqrt{x^2+y^2} \\
&\Rightarrow \quad x^2+y^2=\frac{\left(a^2+1\right)^4}{4 a^2+1}
\end{aligned}
\) -
Question 23 of 42
23. Question
\(
\text { Find } z \text { if }|z|=4 \text { and } \arg (z)=\frac{5 \pi}{6} \text {. }
\)CorrectIncorrectHint
(b) Let \(z=|z|(\cos \theta+i \sin \theta)\), where \(\theta=\arg (z)\).
Given that, \(|z|=4\) and \(\arg (z)=\frac{5 \pi}{6}\).
\(
\begin{array}{rlr}
\Rightarrow \quad z & =4\left[\cos \frac{5 \pi}{6}+i \sin \frac{5 \pi}{6}\right] \quad \text { ( } z \text { lies in II quadrant) } \\
& =4\left[-\frac{\sqrt{3}}{2}+i \frac{1}{2}\right]=-2 \sqrt{3}+2 i &
\end{array}
\) -
Question 24 of 42
24. Question
\(
\text { Find }\left|(1+i) \frac{(2+i)}{(3+i)}\right|
\)CorrectIncorrectHint
(b)
\(
\begin{aligned}
&\left|(1+i) \frac{(2+i)}{(3+i)} \times \frac{3-i}{3-i}\right|=\left|(1+i) \cdot \frac{6-2 i+3 i-i^2}{9-i^2}\right| \\
&=\left|\frac{(1+i)(7+i)}{9+1}\right| \\
&=\left|\frac{7+i+7 i+i^2}{9+1}\right| \\
&=\left|\frac{7+i+7 i+i^2}{10}\right| \\
&=\left|\frac{7+8 i-1}{10}\right| \\
&=\left|\frac{6+8 i}{10}\right| \\
&=\left|\frac{3}{5}+\frac{4}{5} i\right| \\
&=\sqrt{\left(\frac{3}{5}\right)^2+\left(\frac{4}{5}\right)^2}=1
\end{aligned}
\) -
Question 25 of 42
25. Question
\(
\text { Find principal argument of }(1+i \sqrt{3})^2
\)CorrectIncorrectHint
(c)
\(
\begin{gathered}
(1+i \sqrt{3})^2=1+i^2 \cdot 3+2 \sqrt{3} i \\
=1-3+2 \sqrt{3} i=-2+2 \sqrt{3} i \\
\tan \alpha=\left|\frac{2 \sqrt{3}}{-2}\right| \\
\tan \alpha=|-\sqrt{3}|=\sqrt{3} \\
\tan \alpha=\tan \frac{\pi}{3} \\
\alpha=\frac{\pi}{3}
\end{gathered}
\)
Now \(\operatorname{Re}(z)<0\) and image(z)>0 \(\arg (z)=\pi-\alpha=\pi-\frac{\pi}{3}=\frac{2 \pi}{3}\) Hence, the principal \(\arg =\frac{2 \pi}{3}\) -
Question 26 of 42
26. Question
\(
\text { Where does } z \text { lie, if }\left|\frac{z-5 i}{z+5 i}\right|=1 \text {. }
\)CorrectIncorrectHint
(b) We have \(|z-5 i / z+5 i|\)
\(
\begin{array}{ll}
\Rightarrow & |z-5 i|=|z+5 i| \quad \Rightarrow|x+i y-5 i|=|x+i y+5 i| \\
\Rightarrow & |x+i(y-5)|^2=|x+i(y+5)|^2 \quad \Rightarrow x^2+(y-5)^2=x^2+(y+5)^2 \\
\Rightarrow & 20 y=0 \quad \Rightarrow y=0
\end{array}
\)
So, \(z\) lies on the \(x\)-axis (real axis). -
Question 27 of 42
27. Question
\(\sin x+i \cos 2 x\) and \(\cos x-i \sin 2 x\) are conjugate to each other for:
CorrectIncorrectHint
(d)
let \(z=\sin x+i \cos 2 x \quad \bar{z}=\sin x-i \cos 2 x\)
But we are given that \(\bar{z}=\cos x-i \sin 2 x\)
\(\sin x-\mathrm{i} \cos 2 x=\cos x-i \sin 2 x\) Comparing the real and imaginary part, we get
\(\sin \mathrm{x}=\cos \mathrm{x} \quad\) and \(\quad \cos 2 \mathrm{x}=\sin 2 \mathrm{x}\)
\(\tan x=1\) and \(\tan 2 x=1\)
Now, \(\tan 2 x=1\)
\(\Rightarrow \frac{2 \tan x}{1-\tan ^2 x}=1\), which is not satisfied by \(\tan x=1\)
Hence, no value of \(x\) is possible. -
Question 28 of 42
28. Question
The real value of \(\alpha\) for which the expression \(\frac{1-i \sin \alpha}{1+2 i \sin \alpha}\) is purely real is:
CorrectIncorrectHint
(c)
\(z=\frac{1-i \sin \alpha}{1+2 i \sin \alpha}\)
\(
\begin{aligned}
&=\frac{(1-i \sin \alpha)(1-2 i \sin \alpha)}{(1+2 i \sin \alpha)(1-2 i \sin \alpha)}=\frac{1-i \sin \alpha-2 i \sin \alpha+2 i^2 \sin ^2 \alpha}{1-4 i^2 \sin ^2 \alpha} \\
&=\frac{1-3 i \sin \alpha-2 \sin ^2 \alpha}{1+4 \sin ^2 \alpha}=\frac{1-2 \sin ^2 \alpha}{1+4 \sin ^2 \alpha}-\frac{3 i \sin \alpha}{1+4 \sin ^2 \alpha}
\end{aligned}
\)
It is given that \(z\) is a purely real.
\(
\begin{aligned}
&\Rightarrow \quad \frac{-3 \sin \alpha}{1+4 \sin ^2 \alpha}=0 \quad \Rightarrow-3 \sin \alpha=0 \quad \Rightarrow \sin \alpha=0 \\
&\Rightarrow \quad \alpha=n \pi, n \in I
\end{aligned}
\) -
Question 29 of 42
29. Question
If \(z=x+i y\) lies in the third quadrant, then \(\frac{\bar{z}}{z}\) also lies in the third quadrant if
CorrectIncorrectHint
(b) Given that: \(z=x+i y\)
If z lies in the third quadrant.
So \(x<0\) and \(y<0\).
\(
\begin{aligned}
&\bar{z}=\mathrm{x}-\mathrm{i} y \\
&\frac{\bar{z}}{z}=\frac{x-i y}{x+i y} \\
&=\frac{x-i y}{x+i y} \times \frac{x-i y}{x-i y} \\
&=\frac{x^2+i^2 y^2-2 x y i}{x^2-i^2 y^2} \\
&=\frac{x^2-y^2-2 x y i}{x^2+y^2} \\
&=\frac{x^2-y^2}{x^2+y^2}-\frac{2 x y}{x^2+y^2} i
\end{aligned}
\)
When z lies in third quadrant then \(\frac{\bar{z}}{z}\) will also be lie in third quadrant \(\therefore \frac{x^2-y^2}{x^2+y^2}<0\) and \(\frac{-2 x y}{x^2+y^2}<0\)
\(\Rightarrow \mathrm{x}^2-\mathrm{y}^2<0\) and \(2 \mathrm{xy}>0\)
\(\Rightarrow \mathrm{x}^2<\mathrm{y}^2\) and \(\mathrm{xy}>0\)
So \(x<y<0\). -
Question 30 of 42
30. Question
The value of \((z+3)(\bar{z}+3)\) is equivalent to
CorrectIncorrectHint
(a) Let \(z=x+i y\) So, \((z+3)(\bar{z}+3)=(x+i y+3)(x-i y+3)\)
\(
\begin{aligned}
&=[(x+3)+i y][(x+3)-i y] \\
&=(x+3)^2-y^2 i^2=(x+3)^2+y^2 \\
&=|x+3+i y|^2=|z+3|^2
\end{aligned}
\) -
Question 31 of 42
31. Question
If \(\left(\frac{1+i}{1-i}\right)^x=1\), then
CorrectIncorrectHint
\(
\begin{aligned}
& \text { (b) : Given }\left(\frac{1+i}{1-i}\right)^x=1 \\
& \Rightarrow\left(\frac{2 i}{2}\right)^x=1 \\
& \Rightarrow i^x=1 \\
& \Rightarrow i^x=(i)^{4 n} \\
& \Rightarrow x=4 n, n \in I^{+}
\end{aligned}
\) -
Question 32 of 42
32. Question
A real value of \(x\) satisfies the equation \(\left(\frac{3-4 i x}{3+4 i x}\right)=\alpha-i \beta(\alpha, \beta \in \mathbf{R})\) if \(\alpha^2+\beta^2=\)
CorrectIncorrectHint
(a)
\(
\begin{aligned}
&\text { Given that } \frac{3-4 i x}{3+4 i x}=\alpha-i \beta \\
&=\frac{3-4 i x}{3+4 i x} \times \frac{3-4 i x}{3-4 i x}=\alpha-i \beta \\
&\frac{9-12 i x-12 i x+16 i^2 x^2}{9-16 i^2 x^2}=\alpha-i \beta \\
&=\frac{9-16 x^2}{9+16 x^2}-i\frac{24 x}{9+16 x^2}=\alpha-i \beta \ldots \ldots \text { (i) } \\
&\frac{9-16 x^2}{9+16 x^2}+i\frac{24 x}{9+16 x^2}=\alpha+i \beta \ldots \ldots \ldots \text { (ii) }
\end{aligned}
\)
Multiplying eqn (i )and (ii )we get
\(
\begin{aligned}
&\left(\frac{9-16 x^2}{9+16 x^2}\right)^2+\left(\frac{24 x}{9+16 x^2}\right)^2=\alpha^2+\beta^2 \\
&\frac{81+256 x^4-288 x^2+576 x^2}{\left(9+16 x^2\right)^2}=\alpha^2+\beta^2 \\
&\frac{81+256 x^4+288 x^2}{\left(9+16 x^2\right)^2}=\alpha^2+\beta^2 \\
&\frac{\left(9+16 x^2\right)^2}{\left(9+16 x^2\right)^2}=\alpha^2+\beta^2=1
\end{aligned}
\) -
Question 33 of 42
33. Question
Which of the following is correct for any two complex numbers \(z_1\) and \(z_2\)?
CorrectIncorrectHint
(a) Let, \(z_1=r_1 e^{i \alpha}\) and \(z_2=r_2 e^{i \beta}\)
\(\left|z_1\right|=r_1\) and \(\left|z_2\right|=r_2\)
Option A:
\(z_1 z_2=r_1 \Gamma_2 e^{i(\alpha+\beta)}\)
\(\left|z_1 z_2\right|=r_1 r_2=\left|z_1\right|\left|z_2\right|\)
Option A correct
Option B:
\(\arg \left(z_1 z_2\right)=\alpha+\beta\)
\(=\arg \left(z_1\right)+\arg \left(z_2\right)\)
Option B not correct
Let, \(z_1=a+i b\) and \(z_2=c+i d\)
Option C
\(z_1+z_2=(a+c)+i(b+d)\)
\(\left|\mathrm{z}_1+\mathrm{z}_2\right|=\sqrt{(\mathrm{a}+\mathrm{c})^2+(\mathrm{b}+\mathrm{d})^2}\)
\(\left|\mathrm{z}_1\right|=\sqrt{\mathrm{a}^2+\mathrm{b}^2}\) and \(\left|\mathrm{z}_2\right|=\sqrt{\mathrm{c}^2+\mathrm{d}^2}\)
We cannot say anything about option c and option d -
Question 34 of 42
34. Question
The point represented by the complex number \(2-i\) is rotated about origin through an angle \(\frac{\pi}{2}\) in the clockwise direction, the new position of point is:
CorrectIncorrectHint
(b) Given that: \(z=2-i\)
If z rotated through an angle of \(\frac{\pi}{2}\) about the origin in the clockwise direction. Then the new position \(=z \cdot e^{-\left(\frac{\pi}{2}\right)}\)
\(
\begin{aligned}
&=(2-i) e^{-\left(\frac{\pi}{2}\right)} \\
&=(2-i)\left[\cos \left(\frac{-\pi}{2}\right)+i \sin \left(\frac{-\pi}{2}\right)\right] \\
&=(2-\mathrm{i})(0-\mathrm{i}) \\
&=-1-2 \mathrm{i}
\end{aligned}
\) -
Question 35 of 42
35. Question
Let \(x, y \in \mathbf{R}\), then \(x+i y\) is a non real complex number if:
CorrectIncorrectHint
(d) \(x+y i\) is a non-real complex number if \(y \neq 0\) If \(x, y \in R\)
-
Question 36 of 42
36. Question
If \(a+i b=c+i d\), then
CorrectIncorrectHint
(d) Given that: \(a+i b=c+i d\)
\(
\begin{aligned}
&\Rightarrow|\mathrm{a}+\mathrm{ib}|=|c+i \mathrm{~d}| \\
&\Rightarrow \sqrt{a^2+b^2}=\sqrt{c^2+d^2}
\end{aligned}
\)
Squaring both sides, we get \(a^2+b^2=c^2+d^2\) -
Question 37 of 42
37. Question
The complex number \(z\) which satisfies the condition \(\left|\frac{i+z}{i-z}\right|=1\) lies on
CorrectIncorrectHint
(b) Let \(z=x+i y\)
\(
\begin{aligned}
&\left|\frac{i+z}{i-z}\right|=1 \\
&\Rightarrow|i+z|=|i-z| \\
&\Rightarrow|x+y i+i|=|i-x-i y| \\
&\Rightarrow|x+i(y+1)|=|-x+i(1-y)| \\
&\Rightarrow \sqrt{x^2+(y+1)^2}=\sqrt{x^2+(1-y)^2} \\
&\Rightarrow x^2+(y+1)^2=x^2+(1-y)^2 \\
&\Rightarrow y+1=1-y \\
&\Rightarrow 2 y=0 \\
&\Rightarrow y=0
\end{aligned}
\)It lies on the x-axis.
-
Question 38 of 42
38. Question
\(\left|z_1+z_2\right|=\left|z_1\right|+\left|z_2\right|\) is possible if
CorrectIncorrectHint
(c) Let \(z_1=r_1\left(\cos \theta_1+i \sin \theta_1\right)\) and \(z_2=r_2\left(\cos \theta_2+i \sin \theta_2\right)\)
Since \(\left|z_1+z_2\right|=\left|z_1\right|+\left|z_2\right|\)
\(
\begin{aligned}
&\left|\mathrm{z}_1+\mathrm{z}_2\right|=\mathrm{r}_1 \cos \theta_1+\mathrm{i} \mathrm{r}_1 \sin \theta_1+\mathrm{r}_2 \cos \theta_2+\mathrm{i} r_2 \sin \theta_2 \\
&\left|\mathrm{z}_1+\mathrm{z}_2\right|=\sqrt{r_1^2 \cos ^2 \theta_{+} r_2^2 \cos ^2 \theta_2+2 r_1 r_2 \cos \theta_1 \cos \theta_2+r_1^2 \sin ^2 \theta_1+r_2^2 \sin ^2 \theta_2+2 r_1 r_2 \sin \theta_1 \sin \theta_2} \\
&=\sqrt{r_1^2+r_2^2+2 r_1 r_2 \cos \left(\theta_1-\theta_2\right)} \\
&\text { But }\left|\mathrm{z}_1+\mathrm{z}_2\right|=\left|\mathrm{z}_1\right|+\left|\mathrm{z}_2\right| \\
&\text { So } \sqrt{r_1^2+r_2^2+2 r_1 r_2 \cos \left(\theta_1-\theta_2\right)}=r_1+r_2 \\
&\text { Squaring both sides, we get } \\
&r_1^2+r_2^2+2 r_1 r_2 \cos \left(\theta_1-\theta_2\right)=r_1^2+r_2^2+2 r_1 r_2 \\
&\Rightarrow 2 r_1 r_2-2 r_1 r_2 \cos \left(\theta_1-\theta_2\right)=0 \\
&\Rightarrow 1-\cos \left(\theta_1-\theta_2\right)=0 \\
&\Rightarrow \cos \left(\theta_1-\theta_2\right)=1 \\
&\Rightarrow \theta_1-\theta_2=0 \\
&\Rightarrow \theta_1=\theta_2 \\
&\text { So, arg }\left(z_1\right)=\arg \left(\mathrm{z}_2\right)
\end{aligned}
\) -
Question 39 of 42
39. Question
If \(z\) is a complex number, then
CorrectIncorrectHint
(b) Let \(z=x+y i\)
\(
\begin{aligned}
&|z|=|x+y i| \text { and }|z|^2=|x+y i|^2 \\
&\Rightarrow|z|^2=x^2+y^2 \\
&\text { Now } z^2=x^2+y^2 i^2+2 x y i \\
&z^2=x^2-y^2+2 x y i \\
&|z^2|=\sqrt{\left(x^2-y^2\right)^2+(2x y)^2} \\
&=\sqrt{x^4+y^4-2 x^2 y^2+4 x^2 y^2} \\
&=\sqrt{x^4+y^4+2 x^2 y^2} \\
&=\sqrt{\left(x^2+y^2\right)^2} \\
&\text { So }|z|^2=x^2 + y^2=|z^2| \\
&\text { So }|z|^2=\left|z^2\right|
\end{aligned}
\) -
Question 40 of 42
40. Question
The real value of \(\theta\) for which the expression \(\frac{1+i \cos \theta}{1-2 i \cos \theta}\) is a real number is:
CorrectIncorrectHint
(c) We have, \(\frac{1+i \cos \theta}{1-2 i \cos \theta}=\frac{1+i \cos \theta}{1-2 i \cos \theta} \times \frac{1+2 i \cos \theta}{1+2 i \cos \theta}\) \(=\frac{1+3 i \cos \theta+2 i^2 \cos ^2 \theta}{1^2-(2 i \cos \theta)^2}=\frac{1-2 \cos ^2 \theta}{1+4 \cos ^2 \theta}+i\left(\frac{3 \cos \theta}{1+4 \cos ^2 \theta}\right)\), Since \(\frac{1+i \cos \theta}{1-2 i \cos \theta}\) is a real number.
\(
\begin{aligned}
&\therefore \frac{3 \cos \theta}{1+4 \cos ^2 \theta}=0 \\
&\Rightarrow \cos \theta=0 \\
&\Rightarrow \cos \theta=\cos \frac{\pi}{2} \\
&\therefore \text { General solution is } \theta=2 n \pi \pm \frac{\pi}{2}
\end{aligned}
\) -
Question 41 of 42
41. Question
The value of \(\arg (x)\) when \(x<0\) is:
CorrectIncorrectHint
(c) Let \(z=x+0\) i and \(x<0\)
\(
\Rightarrow|z|=\sqrt{(-1)^2+0^2}=1
\)
Since the point \((-x, 0)\) lies on the negative side of the real axis, \(\therefore\) Principal argument \((z)=\pi\) -
Question 42 of 42
42. Question
If \(f(z)=\frac{7-z}{1-z^2}\), where \(z=1+2 i\), then \(|f(z)|\) is
CorrectIncorrectHint
(a)
\(
\begin{aligned}
&f(z)=\frac{7-z}{1-z^2} \\
&=\frac{7-(1+2 i)}{1-(1+2 i)^2} \\
&=\frac{7-1-2 i}{1-\left(1^2+2^2 i^2+4 i\right)} \\
&=\frac{6-2 i}{1-1+4-4 i} \\
&=\frac{6-2 i}{4-4 i} \\
&=\frac{6-2 i}{4-4 i} \times \frac{4+4 i}{4+4 i} \\
&=\frac{24+24 i-8 i-8 i^2}{4^2-4^2 i^2} \\
&=\frac{24+16 i+8}{16+16} \\
&=\frac{32+16 i}{32} \\
&=1+\frac{1}{2} i
\end{aligned}
\)
Since \(
\begin{aligned}
&z=1+2 i \\
&\therefore|z|=\sqrt{(1)^2+(2)^2} \\
&=\sqrt{1+4} \\
&=\sqrt{5} \\
&\therefore|f(z)|=\sqrt{(1)^2+\left(\frac{1}{2}\right)^2} \\
&=\sqrt{1+\frac{1}{4}} \\
&=\frac{\sqrt{5}}{2} \\
&=\frac{|z|}{2}
\end{aligned}
\)