The absolute value of a complex number, \(z=a+b i\), is defined as the distance between the origin \((0,0)\) and the point \((a, b)\) in the complex plane. In other words, it is the length of the hypotenuse of the right triangle formed (by the Pythagorean Theorem).
\(|z|=|a+b i|=\sqrt{a^2+b^2}\)
This is also called the modulus of a complex number.

 

 

Example 1:  Find the absolute value of \(3+2 i\).

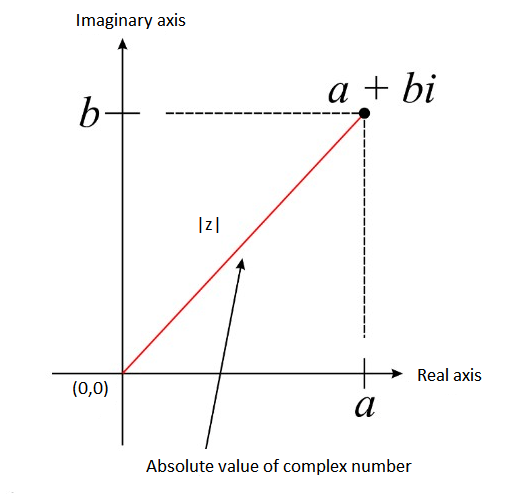
Solution: \(|3+2 i|=\sqrt{3^2+2^2}\)
\(=\sqrt{9+4}\)
\(=\sqrt{13}\)
The absolute value of \(3+2 i=\sqrt{13}\).

Example 2: Find \(|1-3 i|\).

Solution: \(|1-3 i|=\sqrt{1^2+3^2}\)
\(=\sqrt{1+9}\)
\(=\sqrt{10}\)
Therefore, \(|1-3 i|=\sqrt{10}\).

 

Example 3: If \(z\) is a complex number of magnitude \(\sqrt{45}\) and its real part is 3 . Find the imaginary part and \(z\).

Solution: Let \(z=a+ib\), \(\sqrt{a^2+b^2}=\sqrt{45}\)
\(
\begin{aligned}
&\sqrt{3^2+b^2}=\sqrt{45} \\
&\sqrt{9+b^2}=\sqrt{45} \\
&9+b^2=45 \\
&b^2=45-9 \\
&b^2=36 \\
&b=\sqrt{36} \\
&b=\pm 6
\end{aligned}
\)
The complex number \(z=3\pm 6 i\)

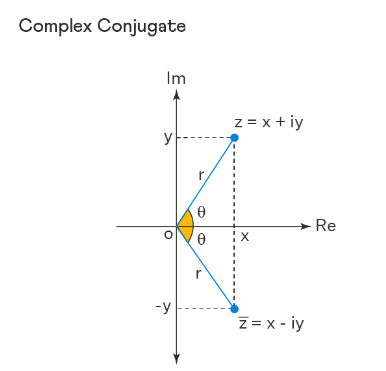
Complex Conjugate Number

The complex conjugate of a complex number, z, is its mirror image with respect to the horizontal axis (or \(x\)-axis). The complex conjugate of complex number \(z\) is denoted by \(\bar{z}\). An easy way to determine the conjugate of a complex number is to replace ‘ \(i\) ‘ with ‘- \(i\) ‘ in the original complex number. The complex conjugate of \(x+iy\) is \(x- iy\) and the complex conjugate of \(x- iy\) is \(x+iy\). As in the image given below, if the complex number z lies in the first quadrant, its image about the horizontal axis, that is, the complex conjugate \(\bar{z}\) lies in the fourth quadrant.

Example 4: Find the complex conjugate of \(5-3 \mathbf{i}\)

Solution: \(5+3 \mathbf{i}\) (Replace \(\mathbf{i}\) with \(\mathbf{-i}\))

Example 5: Prove that \(z \bar{z}=|z|^2\), where \(z=a+i b\) is a complex number and \(\bar{z}\) its conjugate.

Proof: Let \(z=a+i b\) be a complex number. Then, the modulus of \(z\), denoted by \(|z|\), is defined to be the non-negative real number \(\sqrt{a^2+b^2}\), i.e., \(|z|=\sqrt{a^2+b^2}\) and the conjugate of \(z\), denoted as \(\bar{z}\), is the complex number \(a-i b\), i.e., \(\bar{z}=a-i b\).
Observe that the multiplicative inverse of the non-zero complex number \(z\) is given by
\(
z^{-1}=\frac{1}{a+i b}=\frac{a}{a^2+b^2}+i \frac{-b}{a^2+b^2}=\frac{a-i b}{a^2+b^2}=\frac{\bar{z}}{|z|^2}
\)
or \(\quad z \bar{z}=|z|^2\)

Important Note:

For any two complex numbers \(z_1\) and \(z_2\), we have
(i) \(\left|z_1 z_2\right|=\left|z_1\right|\left|z_2\right|\)
(ii) \(\left|\frac{z_1}{z_2}\right|=\frac{\left|z_1\right|}{\left|z_2\right|}\) provided \(\left|z_2\right| \neq 0\)
(iii) \(\overline{z_1 z_2}=\overline{z_1}\) \(\overline{z_2}\)
(iv) \(\overline{z_1 \pm z_2}=\overline{z_1} \pm \overline{z_2}(\mathrm{v}) \overline{\left(\frac{z_1}{z_2}\right)}=\frac{\bar{z}_1}{\bar{z}_2}\) provided \(z_2 \neq 0\).

Example 6: Find the multiplicative inverse of \(2-3 i\).

Solution:  Let \(z=2-3 i\)
Then \(\quad \bar{z}=2+3 i\) and \(\quad|z|^2=2^2+(-3)^2=13\)
Therefore, the multiplicative inverse of \(2-3 i\) is given by
\(z^{-1}=\frac{\bar{z}}{|z|^2}=\frac{2+3 i}{13}=\frac{2}{13}+\frac{3}{13} i\)
The above working can be reproduced in the following manner also,
\(
\begin{aligned}
z^{-1} &=\frac{1}{2-3 i}=\frac{2+3 i}{(2-3 i)(2+3 i)} \\
&=\frac{2+3 i}{2^2-(3 i)^2}=\frac{2+3 i}{13}=\frac{2}{13}+\frac{3}{13} i
\end{aligned}
\)

Example 7: Express the following in the form \(a+i b\)
(i) \(\frac{5+\sqrt{2} i}{1-\sqrt{2} i}\)

(ii) \(i^{-35}\)

Solution: (i) We have, \(\frac{5+\sqrt{2} i}{1-\sqrt{2} i}=\frac{5+\sqrt{2} i}{1-\sqrt{2} i} \times \frac{1+\sqrt{2} i}{1+\sqrt{2} i}=\frac{5+5 \sqrt{2} i+\sqrt{2} i-2}{1-(\sqrt{2} i)^2}\)

\(
=\frac{3+6 \sqrt{2} i}{1+2}=\frac{3(1+2 \sqrt{2} i)}{3}=1+2 \sqrt{2} i .
\)

(ii) \(i^{-35}=\frac{1}{i^{35}}=\frac{1}{\left(i^2\right)^{17} i}=\frac{1}{-i} \times \frac{i}{i}=\frac{i}{-i^2}=i\)